Phase Transitions and Hysteresis for a Simple Model Liquid Crystal by Replica-Exchange Monte Carlo Simulations
Abstract
:1. Introduction
2. Method
2.1. Hess–Su Model
2.2. Simulation Conditions
2.3. Replica-Exchange Method
2.4. Heat Capacity
2.5. WHAM
2.6. Bond Order Parameters
3. Results
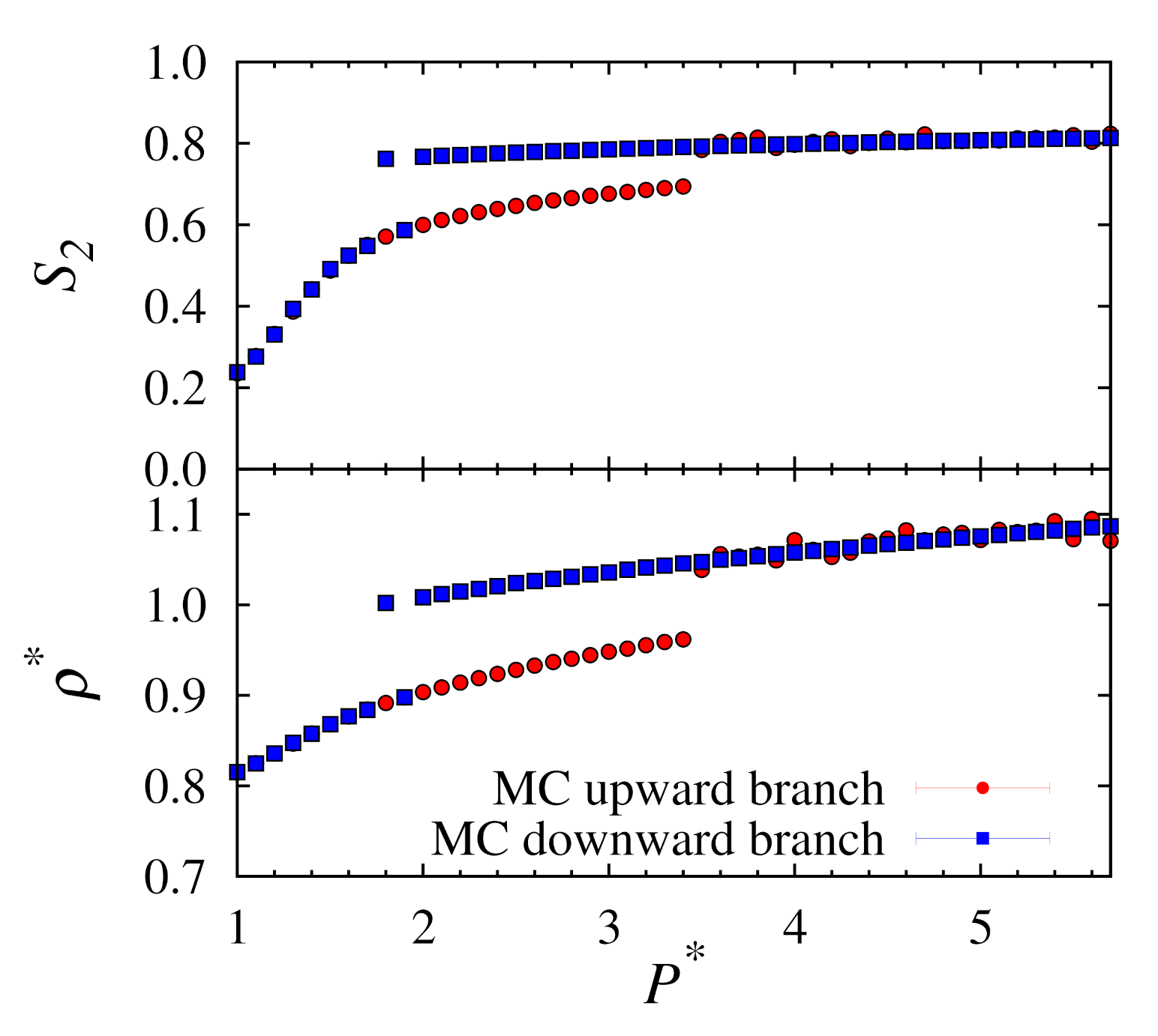
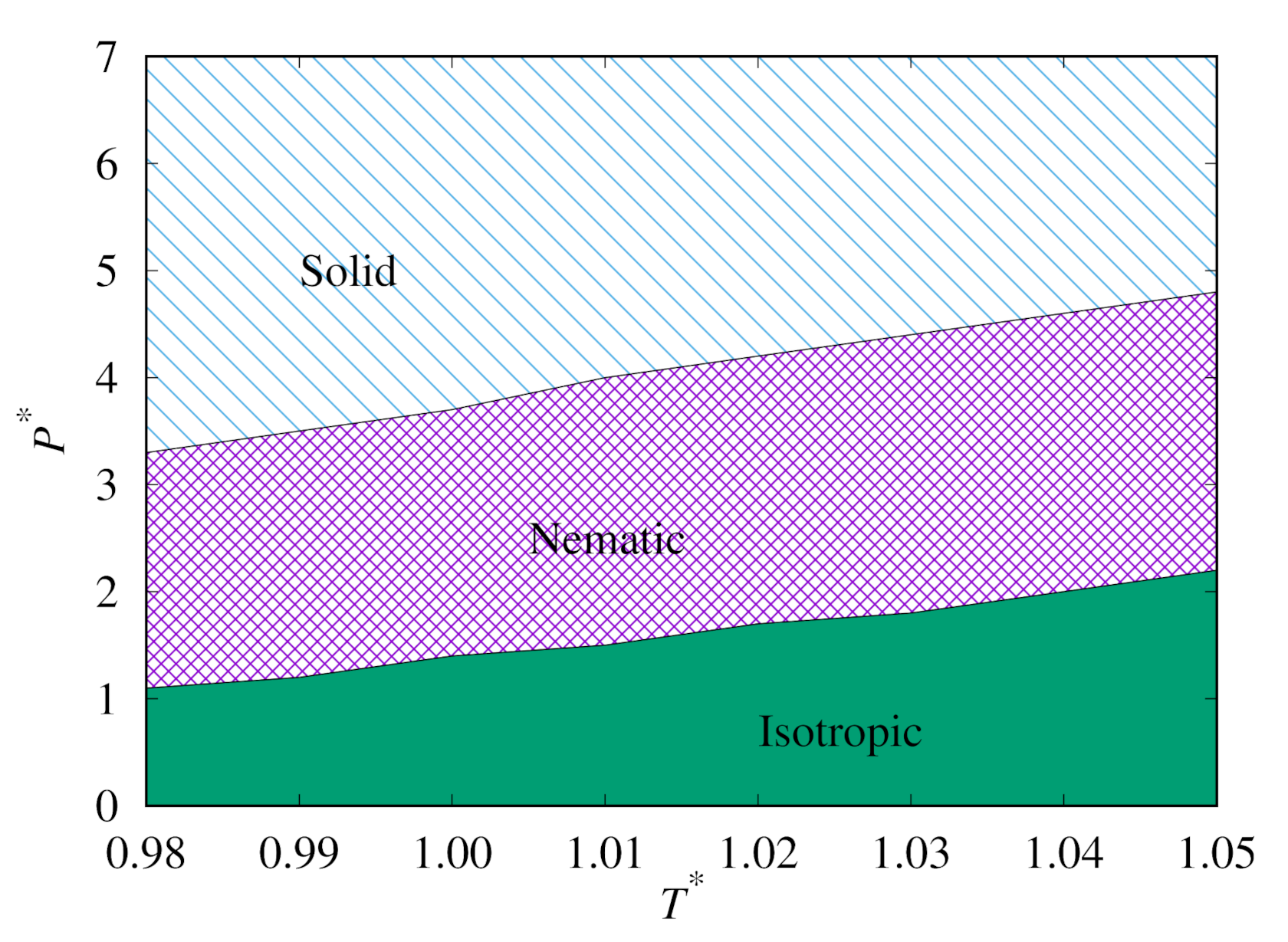
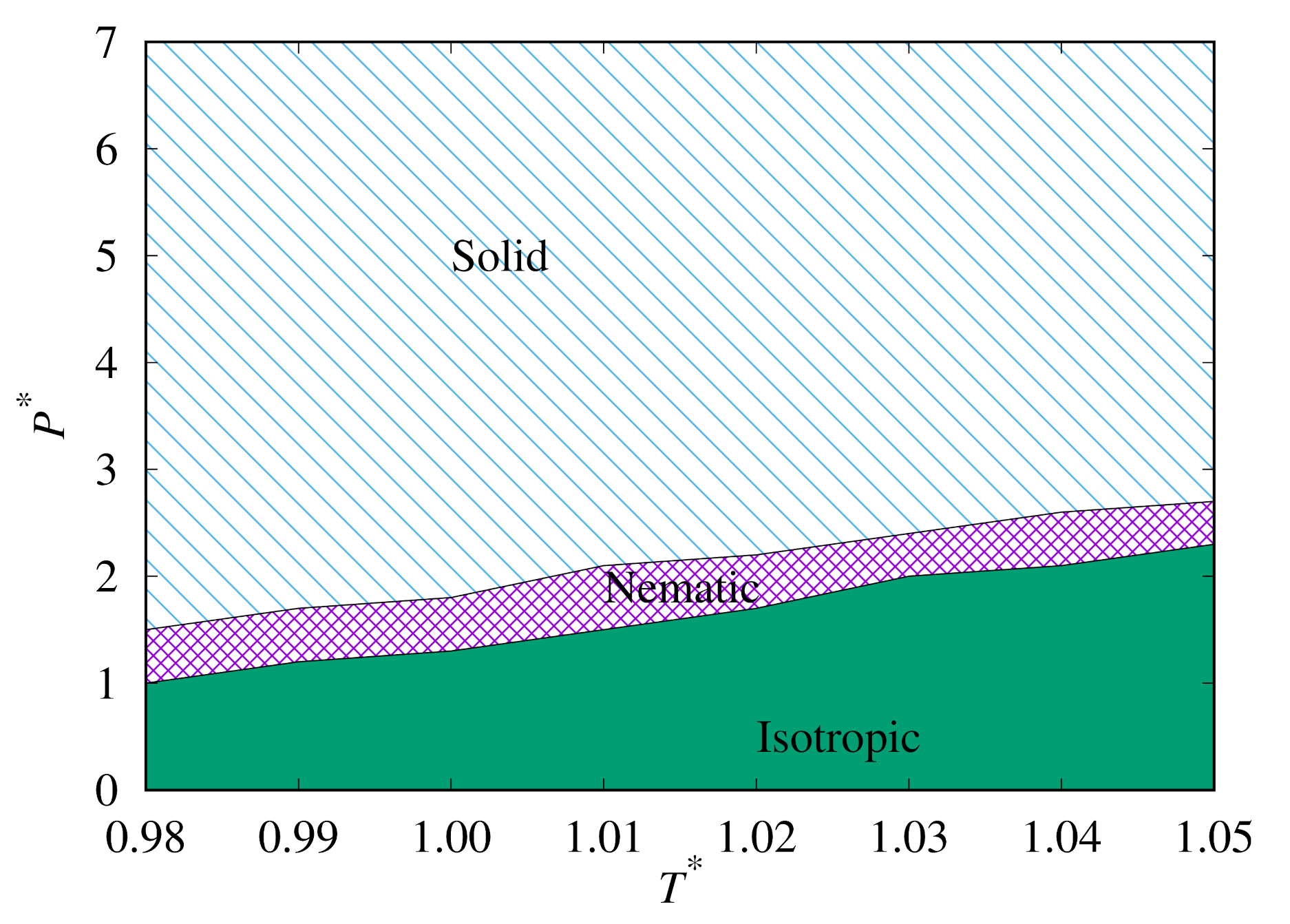
3.1. Conventional MC
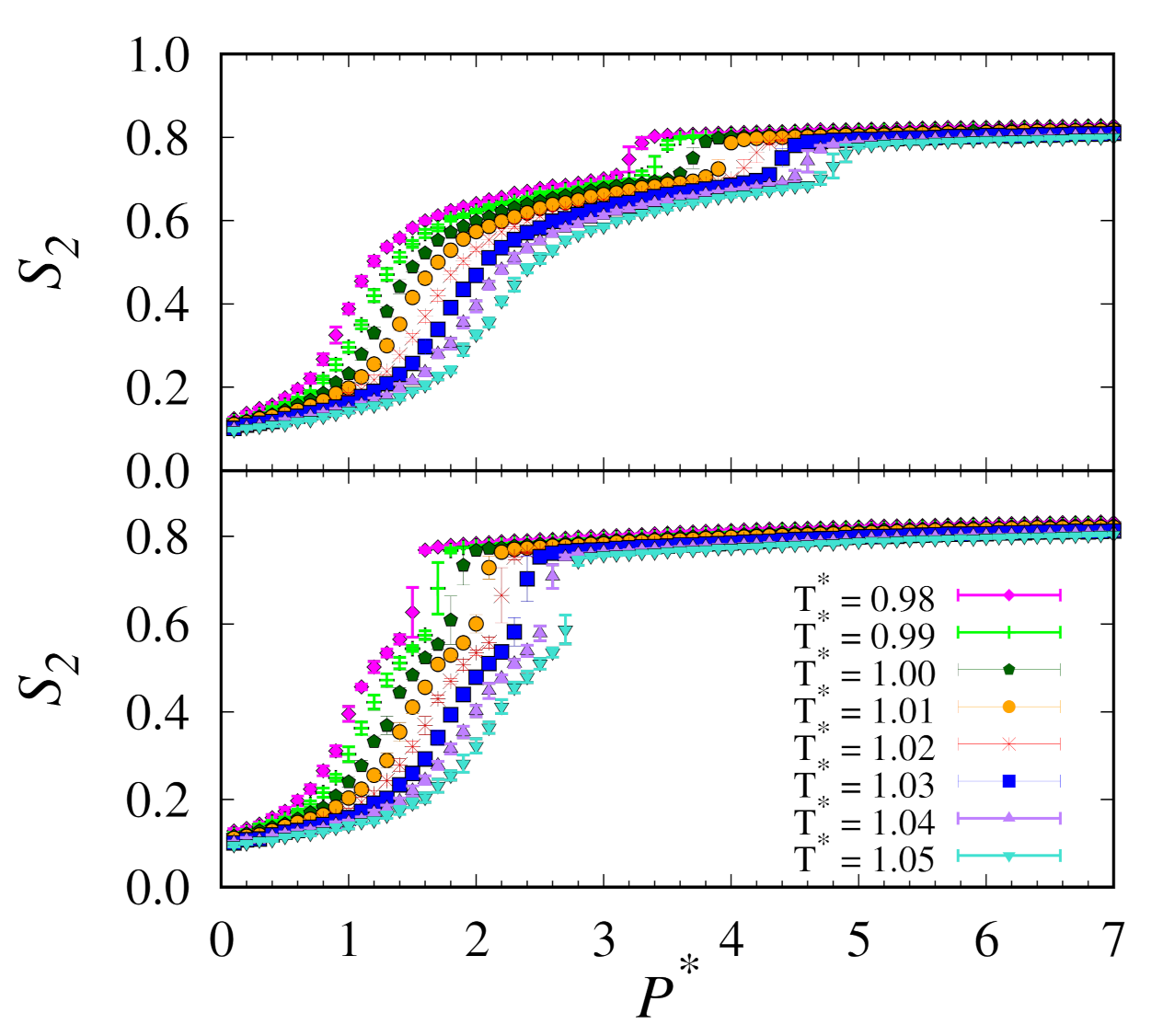
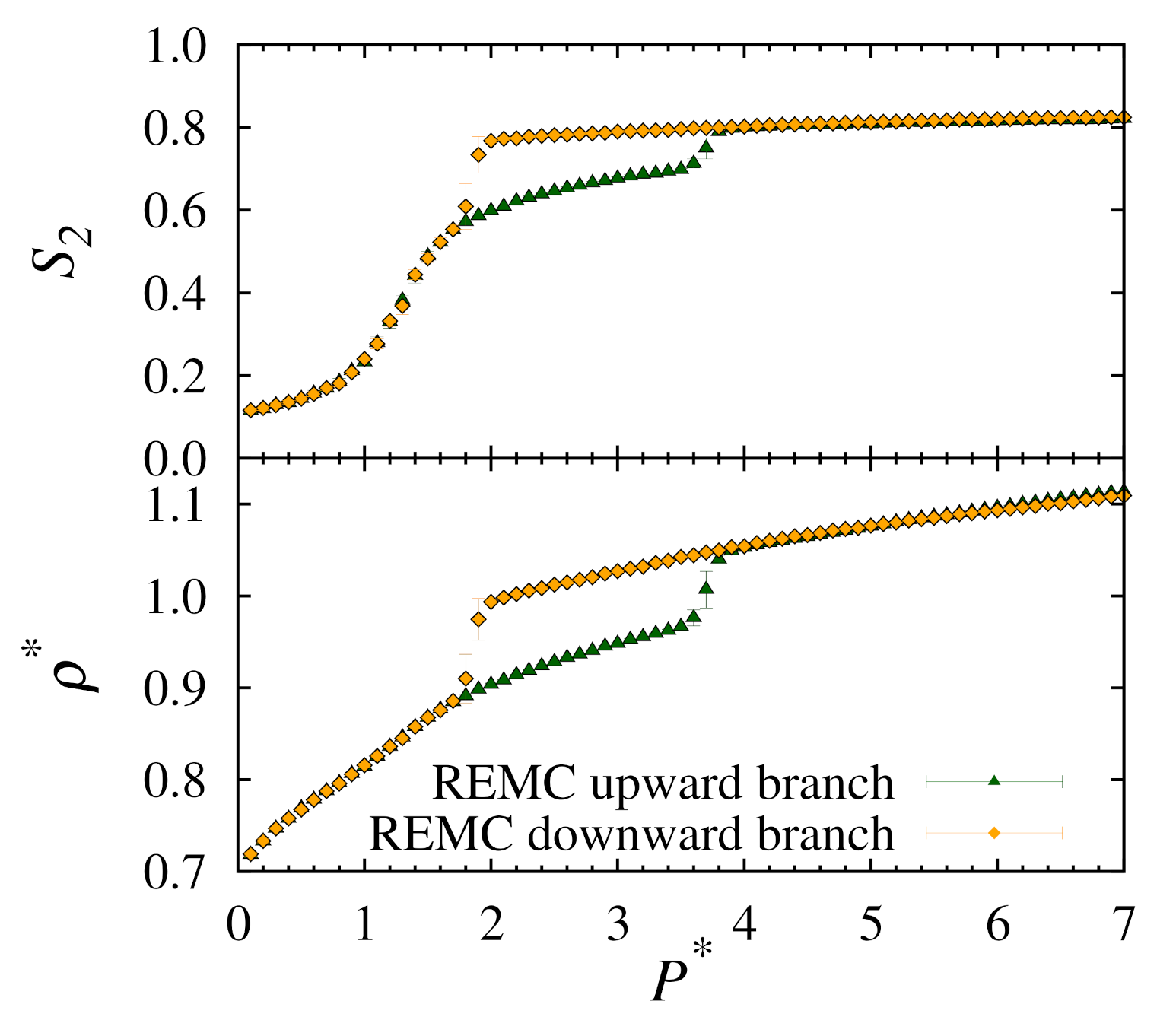
3.2. Replica-Exchange MC
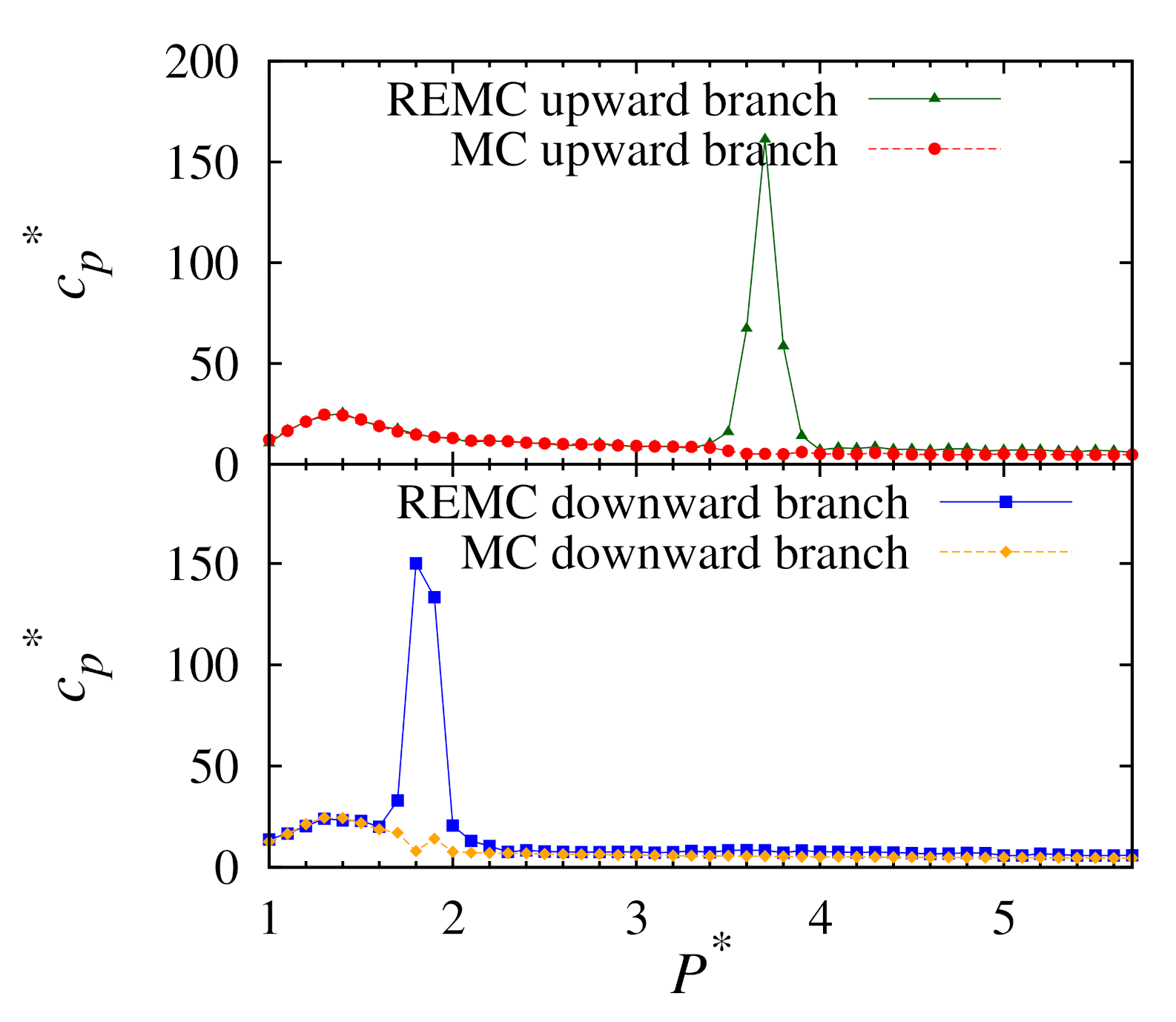
3.3. Heat Capacity
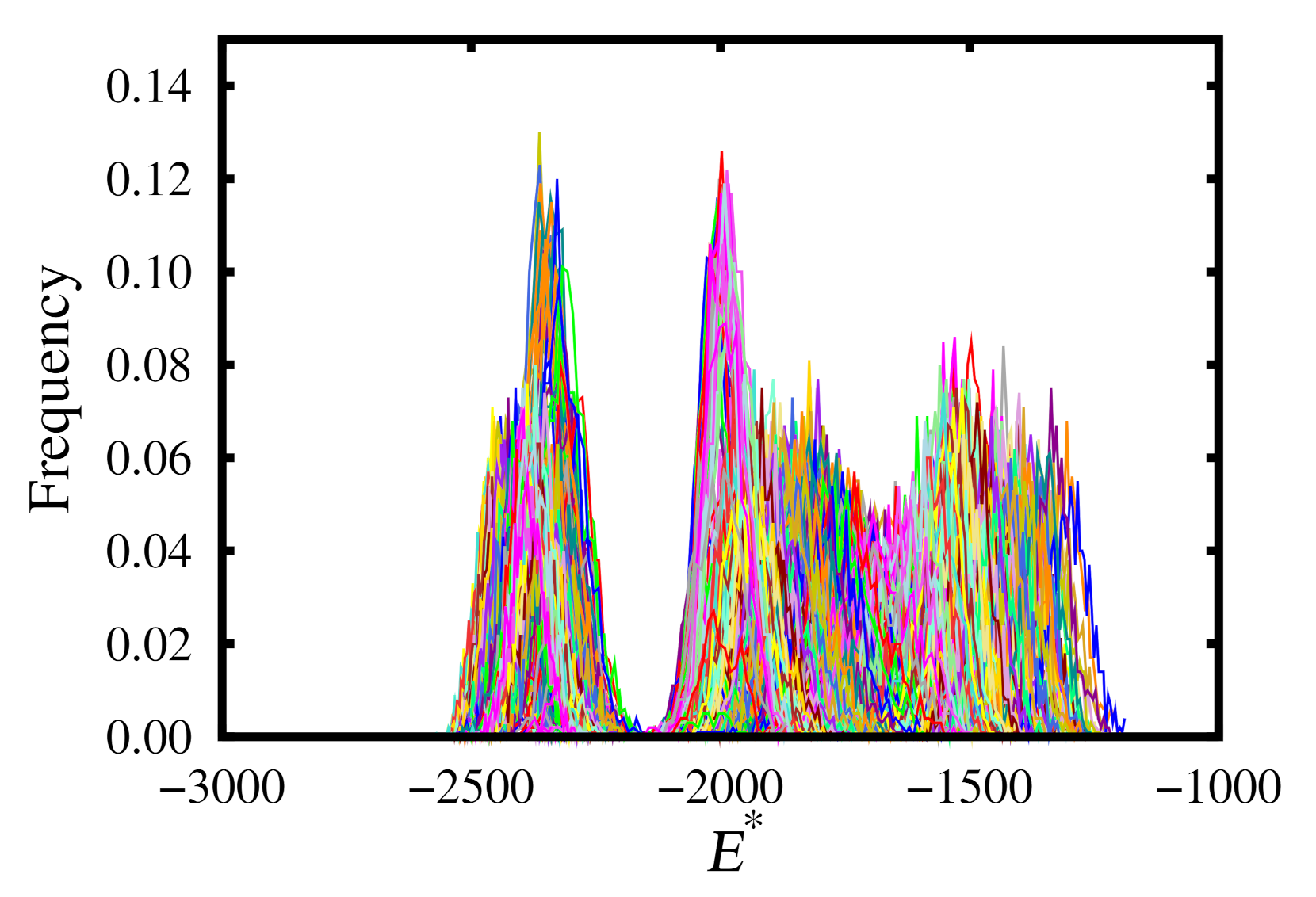
3.4. Overlapping Energy Distributions
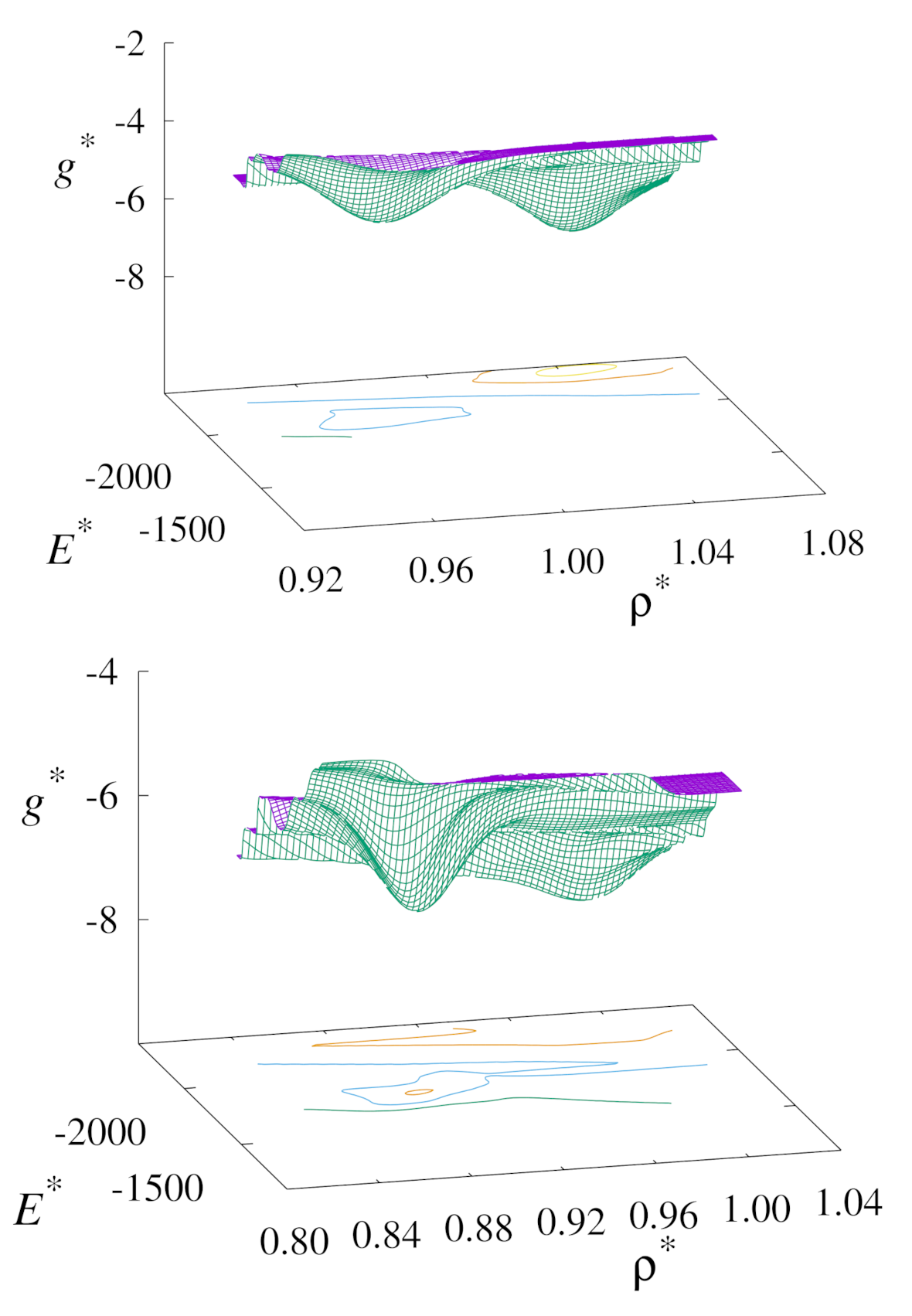
3.5. WHAM Landscape Pathways
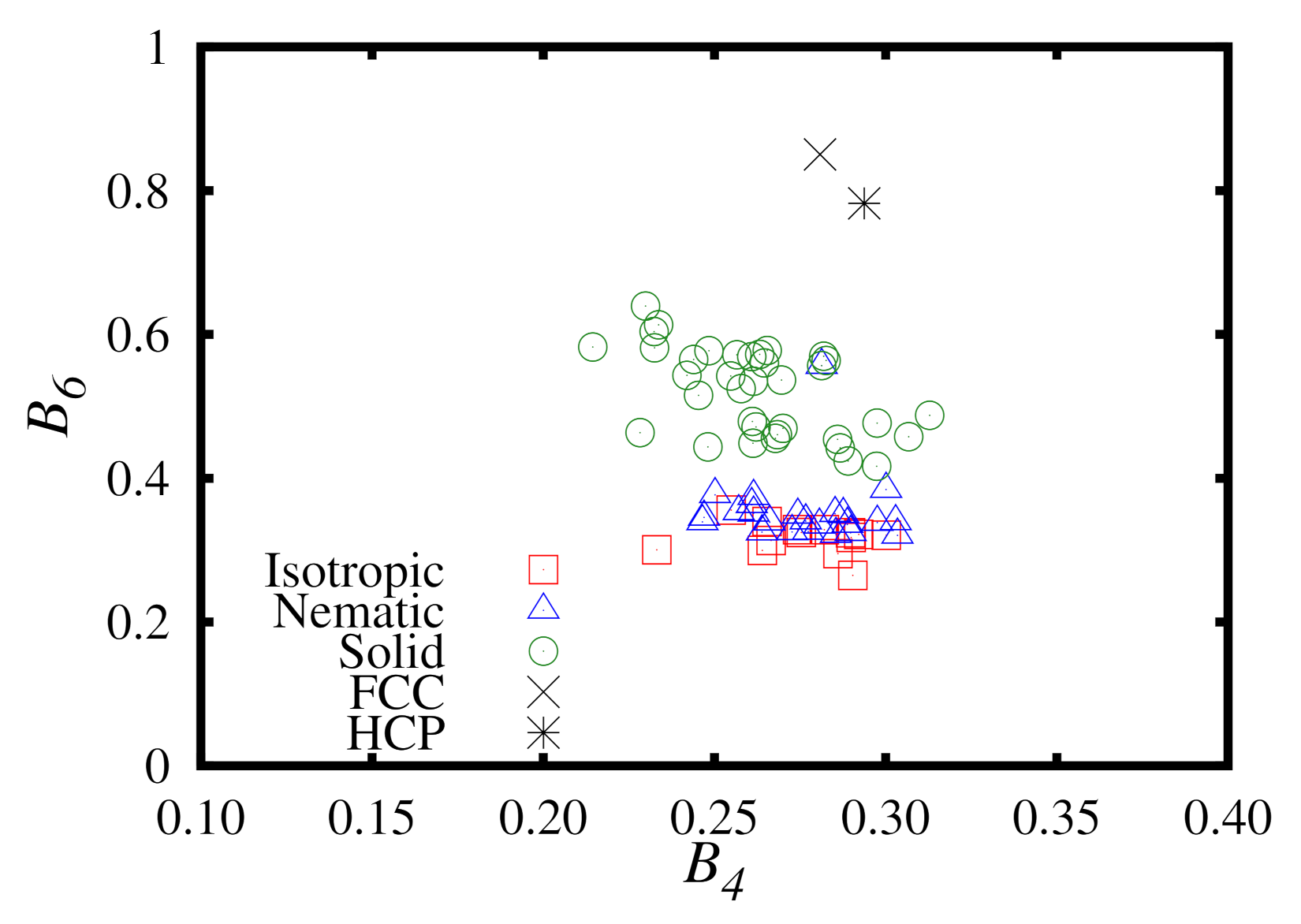
3.6. Bond-Order Parameters
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| MC | Monte Carlo |
| REMC | Replica-Exchange Monte Carlo |
| WHAM | Weighted Histogram Analysis Method |
References
- Chodera, J.D.; Swope, W.C.; Pitera, J.W.; Seok, C.; Dill, K.A. Use of the weighted histogram analysis method for the analysis of simulated and parallel tempering simulations. J. Chem. Theory Comput. 2007, 3, 26–41. [Google Scholar] [CrossRef] [Green Version]
- Andrienko, D. Introduction to liquid crystals. J. Mol. Liq. 2018, 267, 520–541. [Google Scholar] [CrossRef]
- Singh, S. Phase transitions in liquid crystals. Phys. Rep. 2000, 324, 107–269. [Google Scholar] [CrossRef]
- Luckhurst, G.; Stephens, R.; Phippen, R. Computer simulation studies of anisotropic systems. XIX. Mesophases formed by the Gay-Berne model mesogen. Liq. Cryst. 1990, 8, 451–464. [Google Scholar] [CrossRef]
- Frenkel, D.; Lekkerkerker, H.; Stroobants, A. Thermodynamic stability of a smectic phase in a system of hard rods. Nature 1988, 332, 822–823. [Google Scholar] [CrossRef] [Green Version]
- Frenkel, D.; Mulder, B. The hard ellipsoid-of-revolution fluid: I. Monte Carlo simulations. Mol. Phys. 1985, 55, 1171–1192. [Google Scholar] [CrossRef]
- Hess, S.; Su, B. Pressure and isotropic-nematic transition temperature of model liquid crystals. Z. Naturforsch. A 1999, 54, 559–569. [Google Scholar] [CrossRef] [Green Version]
- Greschek, M.; Schoen, M. Finite-size scaling analysis of isotropic-nematic phase transitions in an anisometric Lennard-Jones fluid. Phys. Rev. E 2011, 83, 011704. [Google Scholar] [CrossRef]
- Giura, S.; Schoen, M. Density-functional theory and Monte Carlo simulations of the phase behavior of a simple model liquid crystal. Phys. Rev. E 2014, 90, 022507. [Google Scholar] [CrossRef]
- Greschek, M.; Schoen, M. Orientational prewetting of planar solid substrates by a model liquid crystal. J. Chem. Phys. 2011, 135, 204702. [Google Scholar] [CrossRef] [PubMed]
- Greschek, M.; Melle, M.; Schoen, M. Isotropic–nematic phase transitions in confined mesogenic fluids. The role of substrate anchoring. Soft Matter 2010, 6, 1898–1909. [Google Scholar] [CrossRef]
- Melle, M.; Schlotthauer, S.; Mazza, M.G.; Klapp, S.H.; Schoen, M. Defect topologies in a nematic liquid crystal near a patchy colloid. J. Chem. Phys. 2012, 136, 194703. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Stieger, T.; Schoen, M.; Mazza, M.G. Effects of flow on topological defects in a nematic liquid crystal near a colloid. J. Chem. Phys. 2014, 140, 054905. [Google Scholar] [CrossRef]
- Berardi, R.; Emerson, A.P.J.; Zannoni, C. Monte Carlo investigations of a Gay—Berne liquid crystal. J. Chem. Soc. Faraday Trans. 1993, 89, 4069–4078. [Google Scholar] [CrossRef]
- De Miguel, E.; Rull, L.F.; Chalam, M.K.; Gubbins, K.E. Liquid crystal phase diagram of the Gay-Berne fluid. Mol. Phys. 1991, 74, 405–424. [Google Scholar] [CrossRef]
- Konishi, Y.; Tokoro, H.; Nishino, M.; Miyashita, S. Monte Carlo simulation of pressure-induced phase transitions in spin-crossover materials. Phys. Rev. Lett. 2008, 100, 067206. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cuetos, A.; Martınez-Haya, B.; Rull, L.; Lago, S. Monte Carlo study of liquid crystal phases of hard and soft spherocylinders. J. Chem. Phys. 2002, 117, 2934–2946. [Google Scholar] [CrossRef]
- Steuer, H.; Hess, S.; Schoen, M. Pressure, alignment and phase behavior of a simple model liquid crystal. A Monte Carlo simulation study. Physica A 2003, 328, 322–334. [Google Scholar] [CrossRef]
- Steuer, H.; Hess, S.; Schoen, M. Phase behavior of liquid crystals confined by smooth walls. Phys. Rev. E 2004, 69, 031708. [Google Scholar] [CrossRef]
- Okabe, T.; Kawata, M.; Okamoto, Y.; Mikami, M. Replica-exchange Monte Carlo method for the isobaric–isothermal ensemble. Chem. Phys. Lett. 2001, 335, 435–439. [Google Scholar] [CrossRef]
- Basurto, E.; Gurin, P.; Varga, S.; Odriozola, G. Ordering, clustering, and wetting of hard rods in extreme confinement. Phys. Rev. Res. 2020, 2, 013356. [Google Scholar] [CrossRef] [Green Version]
- Šarmanová, M.; Vítek, A.; Ćosić, R.; Kalus, R. Photoabsorption markers of pressure-induced phase changes in small mercury clusters. A case study on Hg 8. RSC Adv. 2019, 9, 37258–37266. [Google Scholar] [CrossRef] [Green Version]
- Ganguly, A.; Thiel, W.; Crane, B.R. Glutamine amide flip elicits long distance allosteric responses in the LOV protein Vivid. J. Am. Chem. Soc. 2017, 139, 2972–2980. [Google Scholar] [CrossRef] [Green Version]
- Nomura, K.; Kaneko, T.; Bai, J.; Francisco, J.S.; Yasuoka, K.; Zeng, X.C. Evidence of low-density and high-density liquid phases and isochore end point for water confined to carbon nanotube. Proc. Natl. Acad. Sci. USA 2017, 114, 4066–4071. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Berardi, R.; Zannoni, C.; Lintuvuori, J.S.; Wilson, M.R. A soft-core Gay–Berne model for the simulation of liquid crystals by Hamiltonian replica exchange. J. Chem. Phys. 2009, 131, 174107. [Google Scholar] [CrossRef] [PubMed]
- Kumar, S.; Rosenberg, J.M.; Bouzida, D.; Swendsen, R.H.; Kollman, P.A. The weighted histogram analysis method for free-energy calculations on biomolecules. I. The method. J. Comput. Chem. 1992, 13, 1011–1021. [Google Scholar] [CrossRef]
- Ferrenberg, A.M.; Swendsen, R.H. New Monte Carlo technique for studying phase transitions. Phys. Rev. Lett. 1988, 61, 2635. [Google Scholar] [CrossRef] [Green Version]
- Ferrenberg, A.M.; Swendsen, R.H. Optimized monte carlo data analysis. Comput. Phys. 1989, 3, 101–104. [Google Scholar] [CrossRef] [Green Version]
- Okumura, H.; Okamoto, Y. Molecular dynamics simulations in the multibaric–multithermal ensemble. Chem. Phys. Lett. 2004, 391, 248–253. [Google Scholar] [CrossRef]
- Zhang, H.; Xi, W.; Hansmann, U.H.; Wei, Y. Fibril–barrel transitions in cylindrin amyloids. J. Chem. Theory Comput. 2017, 13, 3936–3944. [Google Scholar] [CrossRef]
- Panagiotopoulos, A.Z.; Wong, V.; Floriano, M.A. Phase equilibria of lattice polymers from histogram reweighting Monte Carlo simulations. Macromolecules 1998, 31, 912–918. [Google Scholar] [CrossRef] [Green Version]
- Weidler, D.; Gross, J. Transferable anisotropic united-atom force field based on the Mie potential for phase equilibria: Aldehydes, ketones, and small cyclic alkanes. Ind. Eng. Chem. Res. 2016, 55, 12123–12132. [Google Scholar] [CrossRef]
- Allen, M.P.; Tildesley, D.J. Computer Simulation of Liquids; Oxford University Press: Oxford, UK, 2017. [Google Scholar]
- Kronome, G.; Kristóf, T.; Liszi, J.; Szalai, I. Heat capacities of two-centre Lennard–Jones fluids from NpT ensemble Monte Carlo simulations. Fluid Phase Equilib. 1999, 155, 157–166. [Google Scholar] [CrossRef]
- Okumura, H.; Okamoto, Y. Multibaric–multithermal ensemble molecular dynamics simulations. J. Comput. Chem. 2006, 27, 379–395. [Google Scholar] [CrossRef] [PubMed]
- Kaneko, T.; Bai, J.; Yasuoka, K.; Mitsutake, A.; Zeng, X.C. Liquid-solid and solid-solid phase transition of monolayer water: High-density rhombic monolayer ice. J. Chem. Phys. 2014, 140, 184507. [Google Scholar] [CrossRef] [PubMed]
- Halperin, B.; Nelson, D.R. Theory of two-dimensional melting. Phys. Rev. Lett. 1978, 41, 121. [Google Scholar] [CrossRef] [Green Version]
- Kralj, S.; Cordoyiannis, G.; Jesenek, D.; Zidanšek, A.; Lahajnar, G.; Novak, N.; Amenitsch, H.; Kutnjak, Z. Dimensional crossover and scaling behavior of a smectic liquid crystal confined to controlled-pore glass matrices. Soft Matter 2012, 8, 2460–2470. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kowaguchi, A.; Brumby, P.E.; Yasuoka, K. Phase Transitions and Hysteresis for a Simple Model Liquid Crystal by Replica-Exchange Monte Carlo Simulations. Molecules 2021, 26, 1421. https://doi.org/10.3390/molecules26051421
Kowaguchi A, Brumby PE, Yasuoka K. Phase Transitions and Hysteresis for a Simple Model Liquid Crystal by Replica-Exchange Monte Carlo Simulations. Molecules. 2021; 26(5):1421. https://doi.org/10.3390/molecules26051421
Chicago/Turabian StyleKowaguchi, Akie, Paul E. Brumby, and Kenji Yasuoka. 2021. "Phase Transitions and Hysteresis for a Simple Model Liquid Crystal by Replica-Exchange Monte Carlo Simulations" Molecules 26, no. 5: 1421. https://doi.org/10.3390/molecules26051421
APA StyleKowaguchi, A., Brumby, P. E., & Yasuoka, K. (2021). Phase Transitions and Hysteresis for a Simple Model Liquid Crystal by Replica-Exchange Monte Carlo Simulations. Molecules, 26(5), 1421. https://doi.org/10.3390/molecules26051421





