Computational Study of Elastic, Structural, Electronic, and Optical Properties of GaMF3 (M = Be and Ge) Fluoroperovskites, Based on Density Functional Theory
Abstract
:1. Introduction
2. Computational Methodology
3. Results and Discussion
3.1. Structural Properties
3.2. Electronic Properties (Density of States and Energy Band Structures)
3.3. Elastic Properties
3.4. Optical Properties
3.4.1. The Dielectric Function
3.4.2. The Refractive Index
3.4.3. Absorption Coefficient
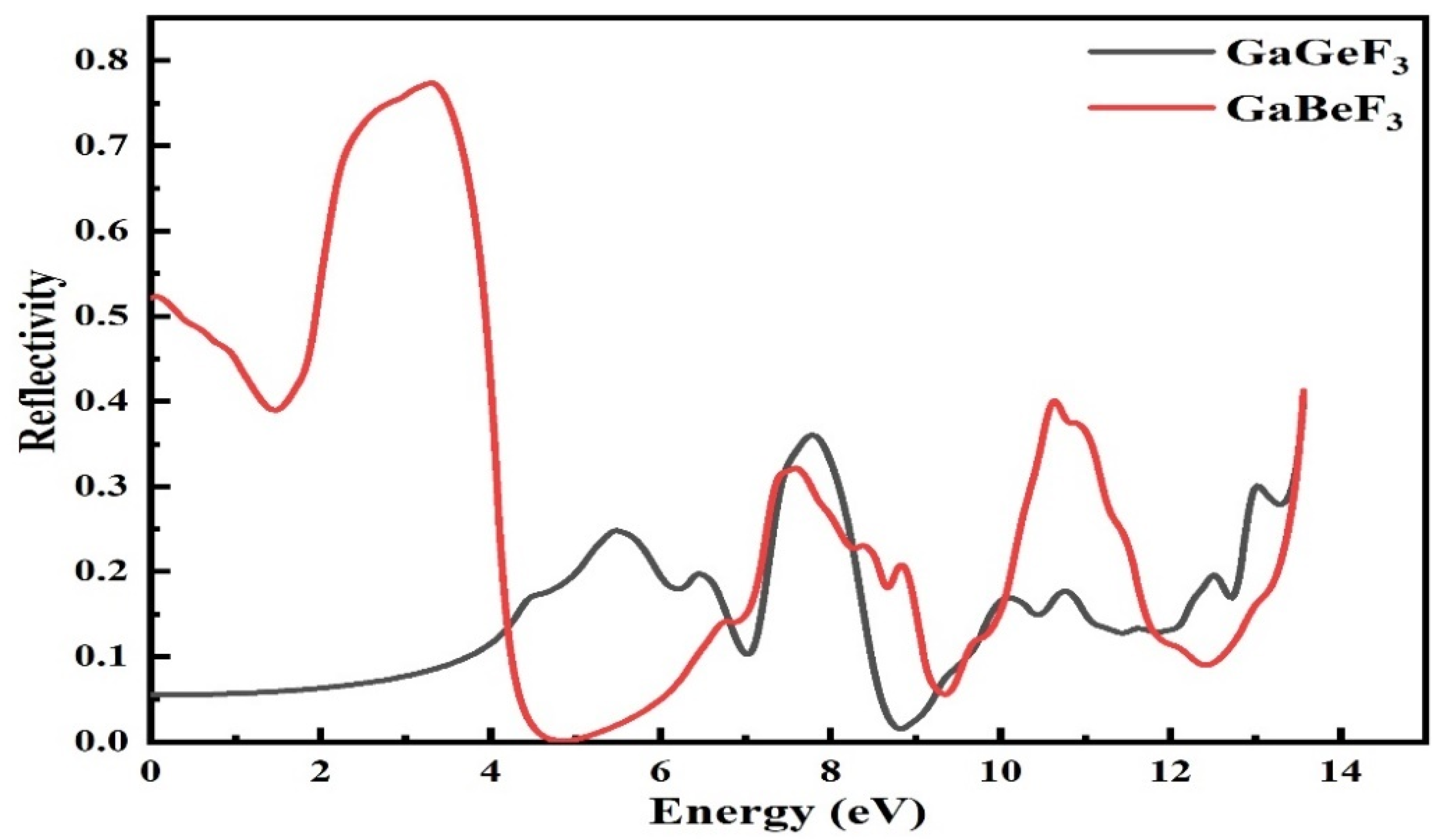
3.4.4. Reflectivity R(ω) Values
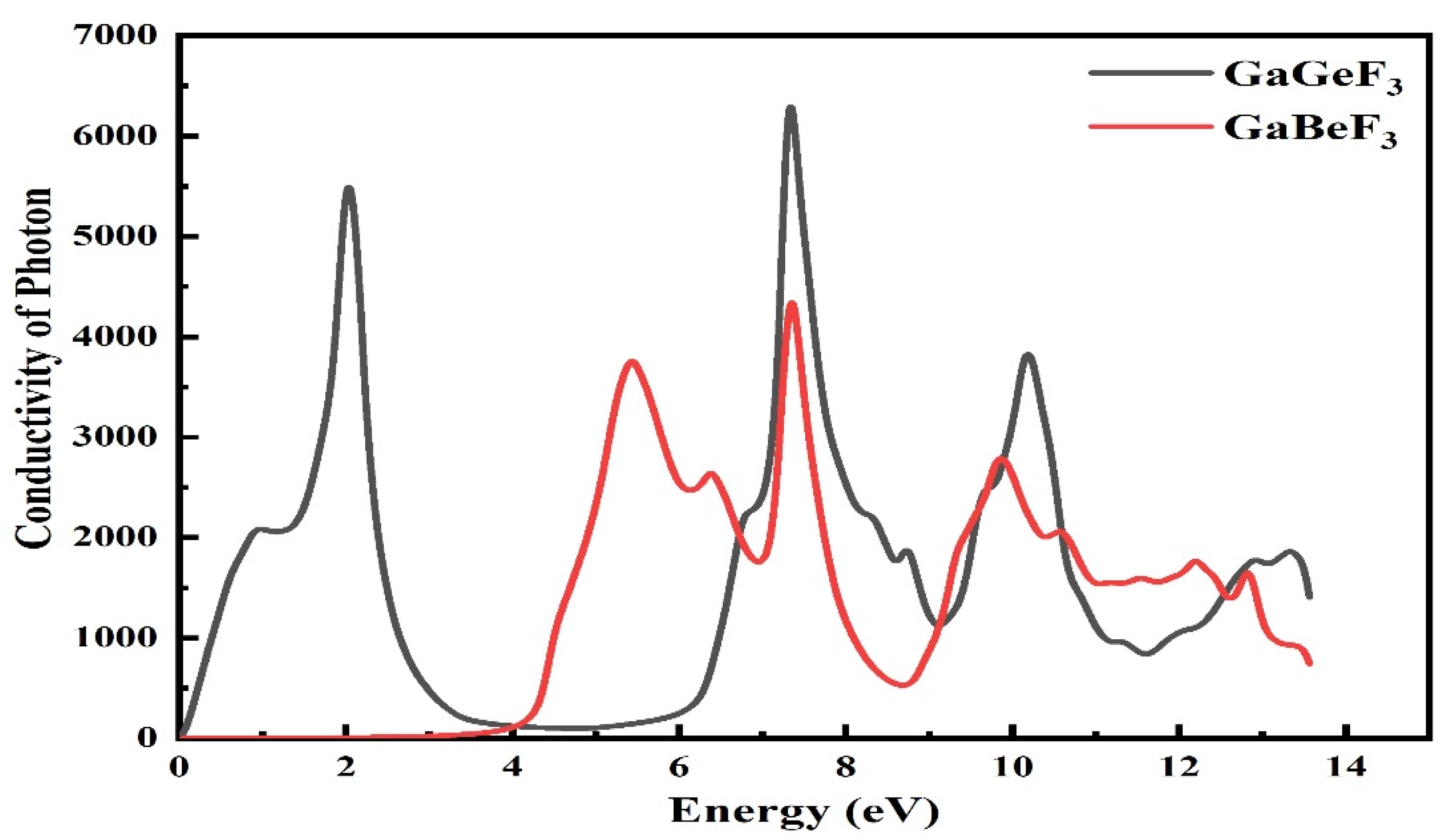
3.4.5. Optical Conductivity
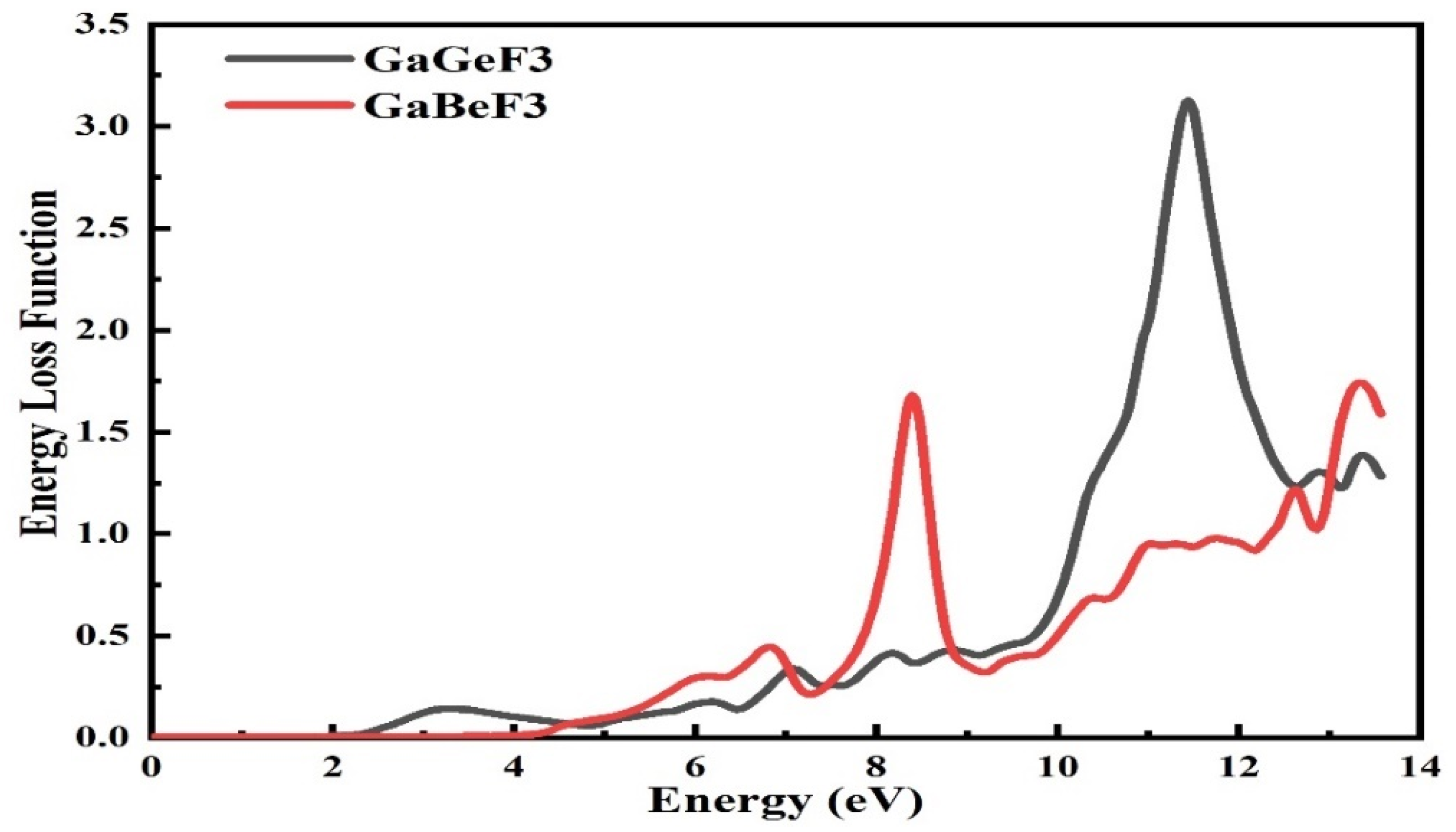
3.5. The Energy Loss Function (ELF)
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Nishimatsu, T.; Terakubo, N.; Mizuseki, H.; Kawazoe, Y.; Pawlak, D.A.; Shimamura, K.; Fukuda, T. Band structures of perovskite-like fluorides for vacuum-ultraviolet-transparent lens materials. Jpn. J. Appl. Phys. 2002, 41, L365. [Google Scholar]
- Dotzler, C.; Williams, G.; Edgar, A. RbCdF3: Mn2+: A potential ultraviolet dosimeter material. Appl. Phys. Lett. 2007, 91, 181909. [Google Scholar]
- Vaitheeswaran, G.; Kanchana, V.; Kumar, R.S.; Cornelius, A.L.; Nicol, M.F.; Svane, A.; Delin, A.; Johansson, B. High-pressure structural, elastic, and electronic properties of the scintillator host material KMgF3. Phys. Rev. B 2007, 76, 14107. [Google Scholar]
- Naeem, S.; Murtaza, G.; Khenata, R.; Khalid, M. First principle study of CsSrM3 (M = F, Cl). Phys. B Condens. Matter 2013, 414, 91–96. [Google Scholar]
- Mubarak, A. Ab initio study of the structural, electronic and optical properties of the fluoropervskite SrXF3 (X= Li, Na, K and Rb) compounds. Comput. Mater. Sci. 2014, 81, 478–482. [Google Scholar]
- Mubarak, A.; Al-Omari, S. First-principles calculations of two cubic fluoropervskite compounds: RbFeF3 and RbNiF3. J. Magn. Magn Mater. 2015, 382, 211–218. [Google Scholar]
- Khan, R.; Althubeiti, K.; Algethami, M.; Rahman, N.; Sohail, M.; Mao, Q.; Zaman, Q.; Ullah, A.; Ilyas, N.; Afzal, A.M.; et al. Observation of Quantum Criticality in Antiferromagnetic based (Ce1−xYx)2Ir3Ge5 Kondo-Lattice System. J. Magn. Magn. Mater. 2022, 556, 169361. [Google Scholar]
- Dimov, N.; Nishimura, A.; Chihara, K.; Kitajou, A.; Gocheva, I.D.; Okada, S. Transition metal NaMF3 compounds as model systems for studying the feasibility of ternary Li-MF and Na-MF single phases as cathodes for lithium–ion and sodium–ion batteries. Electrochim. Acta 2013, 110, 214–220. [Google Scholar]
- Donaldson, J.; Williams, G.; Raymond, S. Characterization of a Fluoroperovskite Based Fibre Coupled Optical Dosimeter for Radiotherapy. Annual Condensed Matter and Materials Meeting; Australian Institute of Physics: Canberra, Australia, 2014. [Google Scholar]
- Shimamura, K.; Fujita, T.; Sato, H.; Bensalah, A.; Sarukura, N.; Fukuda, T. Growth and characterization of KMgF3 single crystals by the Czochralski technique under CF4 atmosphere. Jpn. J. Appl. Phys. 2000, 39, 6807. [Google Scholar]
- Bensalah, A.; Shimamura, K.; Nakano, K.; Fujita, T.; Fukuda, T. Growth and characterization of LiSrGaF6 single crystal. J. Cryst. Growth 2001, 231, 143–147. [Google Scholar]
- Husain, M.; Ahmad, M.S.; Rahman, N.; Sajjad, M.; Rauf, A.; Habib, A.; Mahmood, H.; Nisar, M.; Hussain, A.; Imran, M.; et al. First principle study of the structural, electronic, and Mechanical properties of cubic fluoroperovskites:(ZnXF3, X = Y, Bi). Fluoride 2020, 53, 657–667. [Google Scholar]
- Ahmad, M.S.; Habib, A.; Rauf, A.; Haq, M.U.; Saddique, J.; Nisar, M.; Shah, S.; Maouche, C.; Zulfiqar, S.; Rehman, M.U.; et al. Theoretical investigation of the structural, electronic, and mechanical properties of the magnesium-based fluoroperovskite compounds XMgF3 (X= Ga, Al, In). Theoretical Invest. 2020, 1, 542–553. [Google Scholar]
- Rahman, N.; Husain, M.; Yang, J.; Sajjad, M.; Murtaza, G.; Haq, M.U.; Habib, A.; Zulfiqar, R.A.; Karim, A.; Nisar, M.; et al. First principle study of structural, electronic, optical and mechanical properties of cubic fluoro-perovskites:(CdXF3, X = Y, Bi). Eur. Physic. J. Plus 2021, 136, 1–11. [Google Scholar]
- Harmel, M.; Khachai, H.; Haddou, A.; Khenata, R.; Murtaza, G.; Abbar, B.; Binomran, S.; Khalfa, M. Ab initio study of the mechanical, thermal and optoelectronic properties of the cubic CsBaF3. Acta Phys. Pol. 2015, 128, 34–42. [Google Scholar]
- Daniel, D.J.; Madhusoodanan, U.; Nithya, R.; Ramasamy, P. Irradiation effect on luminescence properties of fluoroperovskite single crystal (LiBaF3: Eu2+). Radiat. Phys. Chem. 2014, 96, 135–139. [Google Scholar]
- Maqbool, M.; Ahmad, I.; Richardson, H.; Kordesch, M. Direct ultraviolet excitation of an amorphous AlN: Praseodymium phosphor by codoped Gd3+ cathodoluminescence. Appl. Phys. Lett. 2007, 91, 193511. [Google Scholar]
- Murtaza, G.; Ahmad, I. Shift of indirect to direct bandgap and optical response of LaAlO3 under pressure. J. Appl. Phys. 2012, 111, 123116. [Google Scholar]
- Murtaza, G.; Ahmad, I.; Amin, B.; Afaq, A.; Maqbool, M.; Maqssod, J.; Khan, I.; Zahid, M. Investigation of structural and optoelectronic properties of BaThO3. Opt. Mater. 2011, 33, 553–557. [Google Scholar]
- Saddique, J.; Husain, M.; Rahman, N.; Khan, R.; Zulfiqar; Iqbal, A.; Sohail, M.; Khattak, S.A.; Khan, S.N.; Khan, A.A.; et al. Modeling structural, elastic, electronic and optical properties of ternary cubic barium based fluoroperovskites MBaF3 (M = Ga and In) compounds based on DFT. Mater. Sci. Semicond. Process. 2022, 139, 106345. [Google Scholar]
- Madsen, G.K.; Blaha, P.; Schwarz, K.; Sjostedt, E.; Nordstrom, L. Efficient linearization of the augmented plane-wave method. Phys. Rev. B 2001, 64, 195134. [Google Scholar]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865. [Google Scholar]
- Tran, F.; Blaha, P. Accurate band gaps of semiconductors and insulators with a semilocal exchange-correlation potential. Phys. Rev. Lett. 2009, 102, 226401. [Google Scholar]
- Murnaghan, F. The compressibility of media under extreme pressures. Proc. Natl. Acad. Sci. USA 1944, 30, 244. [Google Scholar]
- Jamal, M.; Bilal, M.; Ahmad, I.; Jalali-Asadabadi, S. IRelast package. J. Alloys Compd. 2018, 735, 569–579. [Google Scholar]
- Bechhoefer, J. Kramers–kronig, bode, and the meaning of zero. Am. J. Phys. 2011, 79, 1053–1059. [Google Scholar]
- Kim, C.C.; Garland, J.; Raccah, P. Modeling the optical dielectric function of the alloy system AlxGa1−xAs. Phys. Rev. B 1993, 47, 1876. [Google Scholar]
- Dufek, P.; Blaha, P.; Schwarz, K. Applications of Engel and Vosko’s generalized gradient approximation in solids. Phys. Rev. B 1994, 50, 7279. [Google Scholar]
- Charifi, Z.; Baaziz, H.; Hassan, F.E.H.; Bouarissa, N. High pressure study of structural and electronic properties of calcium chalcogenides. J. Phys. Condens. Matter 2005, 17, 4083. [Google Scholar]
- Ali, M.A.; Alam, N.; Meena; Ali, S.; Dar, S.A.; Khan, A.; Murtaza, G.; Laref, A. A theoretical study of the structural, thermoelectric, and spin-orbit coupling influenced optoelectronic properties of CsTmCl3 halide perovskite. Int. J. Quant. Chem. 2020, 120, 26141. [Google Scholar]
- Mohamed, A.; El Houssine, A.; Nejmaa, F.; Ibrahim, B. Ab-initio study of electronic, optical and thermoelectric properties of TiO2 phases using mBJ approximation. In Proceedings of the 2020 IEEE 6th International Conference on Optimization and Applications, Beni Mellal, Morocco, 20–21 April 2020; pp. 1–5. [Google Scholar]
- Mehl, M.J. Pressure dependence of the elastic moduli in aluminum-rich Al-Li compounds. Phys. Rev. B 1993, 47, 2493. [Google Scholar]
- Wang, J.; Yip, S.; Phillpot, S.; Wolf, D. Crystal instabilities at finite strain. Phys. Rev. Lett. 1993, 71, 4182. [Google Scholar]
- Hill, R. The elastic behaviour of a crystalline aggregate. Proc. Phys. Soc. 1952, 65, 349. [Google Scholar]
- Voigt, W. Lehrbuch der Kristallphysik (Textbook of Crystal Physics); BG Teubner: Germany, Berlin, 1928. [Google Scholar]
- Russ, A.A.A. The origin of rheology. Mater. Phys. 1929, 49. [Google Scholar]
- Pettifor, D. Theoretical predictions of structure and related properties of intermetallics. Mater. Sci. Technol. 1992, 8, 345–349. [Google Scholar]
- Pugh, S. XCII. Relations between the elastic moduli and the plastic properties of polycrystalline pure metals. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1954, 45, 823–843. [Google Scholar]
- Frantsevich, I.; Voronov, F.F.; Bakuta, S.A. Elastic Constants and Elastic Moduli of Metals and Insulators hand book. In Reference Book; Naukova Dumka: Kiev, Ukraine, 1982. [Google Scholar]
- Penn, D.R. Electron mean-free-path calculations using a model dielectric function. Phys. Rev. B 1987, 35, 482. [Google Scholar]
- Naseri, M.; Jalilian, J.; Reshak, A.H. Electronic and optical properties of pentagonal-B2C monolayer: A first-principlescalculation. Int. J. Mod. Phys. B 2017, 31, 1750044. [Google Scholar]
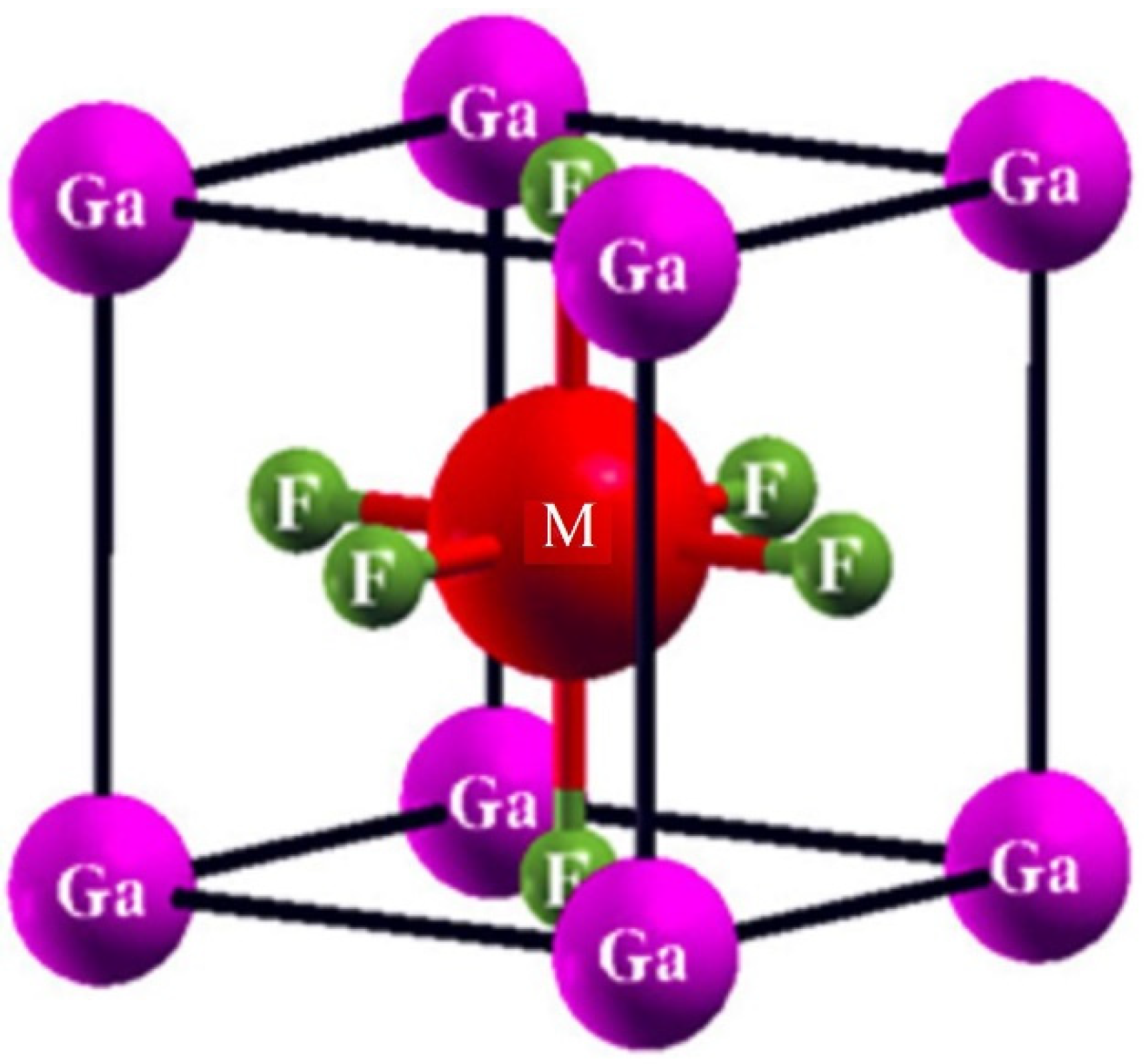
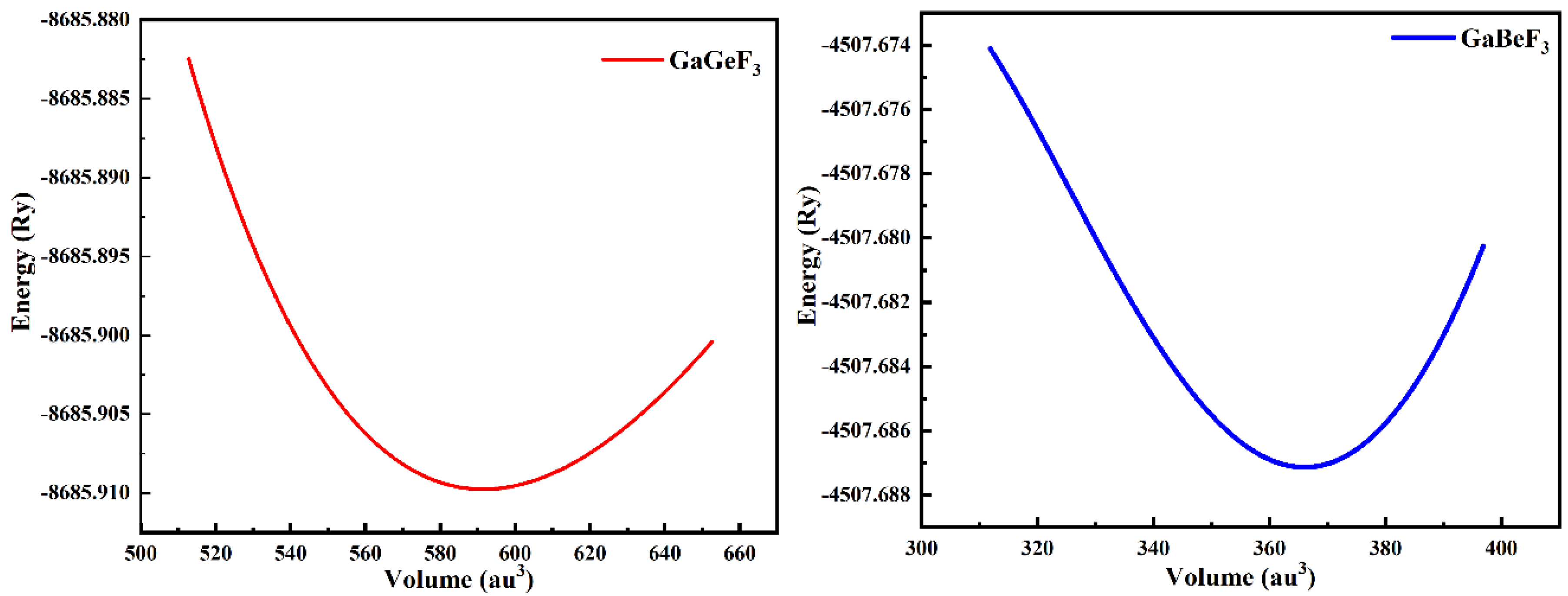

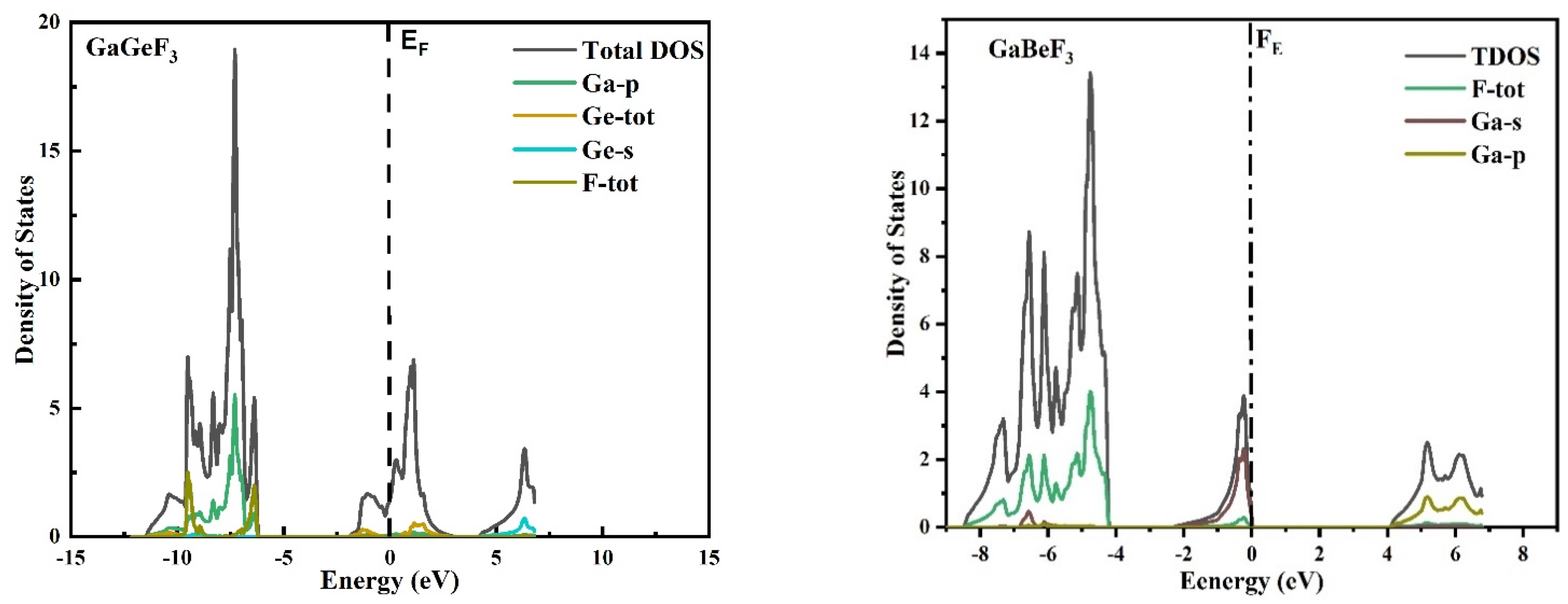

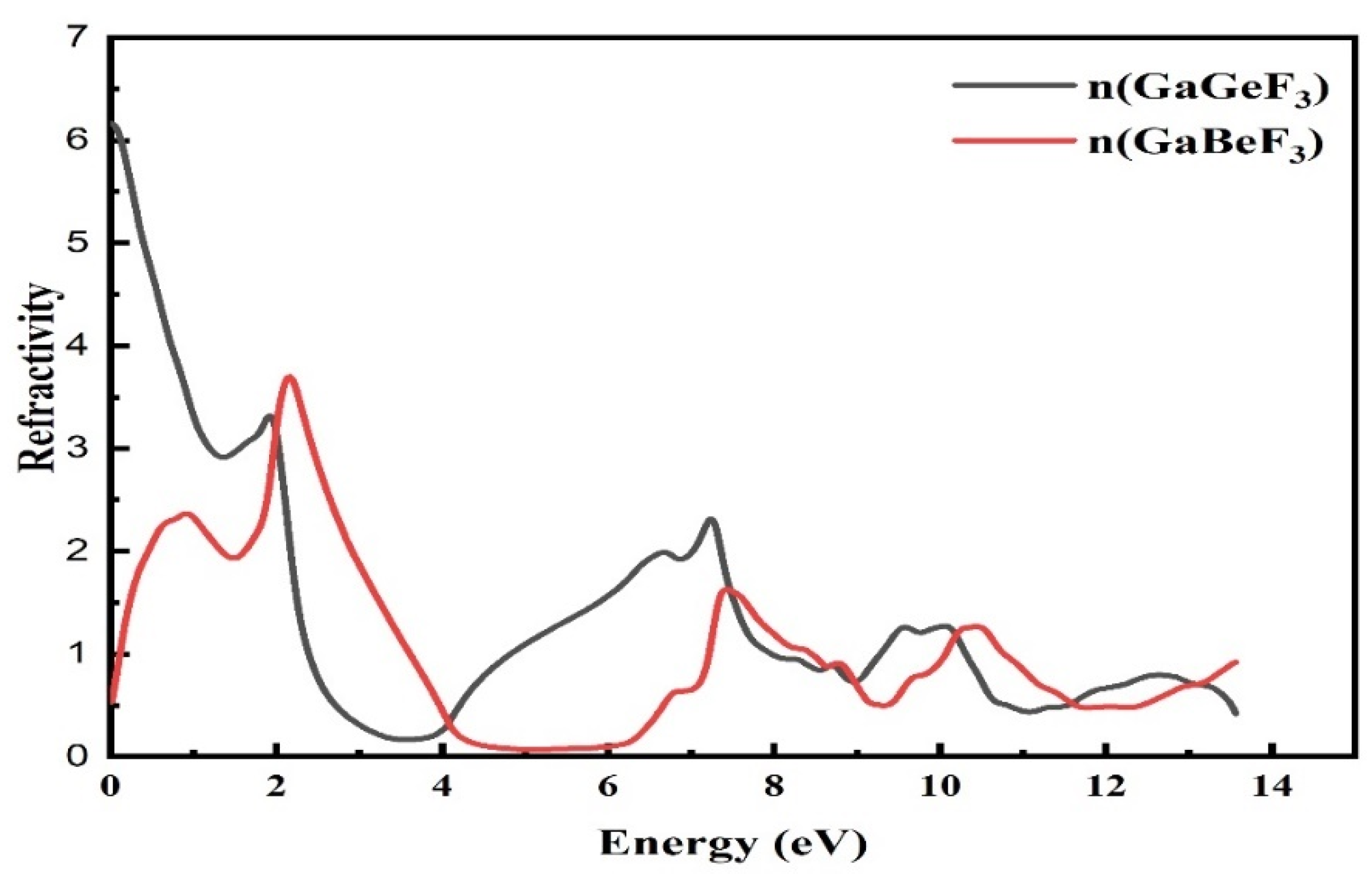

| Structural Specification | GaBeF3 | GaGeF3 |
|---|---|---|
| a0 (Å) | 5.113 | 5.1035 |
| V0 (a.u3) | 891.00 | 897.01 |
| B0 (Gpa) | 33.20 | 33.79 |
| B0/(Gpa) | 4.7814 | 4.9494 |
| E0 (Ry) | 20,766.82 | 28,645.22 |
| Elastic Parameters | GaGeF3 | GaBeF3 |
|---|---|---|
| C11(GPa) | 98.525 | 93.4057 |
| C12(GPa) | 62.046 | 102.992 |
| C44(GPa) | −7.316 | 68.648 |
| B (GPa) | 172.03 | 172.03 |
| A | −0.40 | −14.322 |
| E (in GPa) | 8.745 | 109.485 |
| ʋ | 0.492 | 0.394 |
| B/G | −25.127 | 13.29 |
| G (GPa) | −6.846 | 12.94 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, H.; Sohail, M.; Rahman, N.; Khan, R.; Hussain, M.; Ullah, A.; Khan, A.; Alataway, A.; Dewidar, A.Z.; Elansary, H.O.; et al. Computational Study of Elastic, Structural, Electronic, and Optical Properties of GaMF3 (M = Be and Ge) Fluoroperovskites, Based on Density Functional Theory. Molecules 2022, 27, 5264. https://doi.org/10.3390/molecules27165264
Khan H, Sohail M, Rahman N, Khan R, Hussain M, Ullah A, Khan A, Alataway A, Dewidar AZ, Elansary HO, et al. Computational Study of Elastic, Structural, Electronic, and Optical Properties of GaMF3 (M = Be and Ge) Fluoroperovskites, Based on Density Functional Theory. Molecules. 2022; 27(16):5264. https://doi.org/10.3390/molecules27165264
Chicago/Turabian StyleKhan, Hukam, Mohammad Sohail, Nasir Rahman, Rajwali Khan, Mudasser Hussain, Asad Ullah, Aurangzeb Khan, Abed Alataway, Ahmed Z. Dewidar, Hosam O. Elansary, and et al. 2022. "Computational Study of Elastic, Structural, Electronic, and Optical Properties of GaMF3 (M = Be and Ge) Fluoroperovskites, Based on Density Functional Theory" Molecules 27, no. 16: 5264. https://doi.org/10.3390/molecules27165264
APA StyleKhan, H., Sohail, M., Rahman, N., Khan, R., Hussain, M., Ullah, A., Khan, A., Alataway, A., Dewidar, A. Z., Elansary, H. O., & Yessoufou, K. (2022). Computational Study of Elastic, Structural, Electronic, and Optical Properties of GaMF3 (M = Be and Ge) Fluoroperovskites, Based on Density Functional Theory. Molecules, 27(16), 5264. https://doi.org/10.3390/molecules27165264









