Fine Structure and the Huge Zero-Field Splitting in Ni2+ Complexes
Abstract
1. Introduction
2. Theoretical Background
2.1. General Considerations
2.2. The Hamiltonian
3. Coordination Geometries
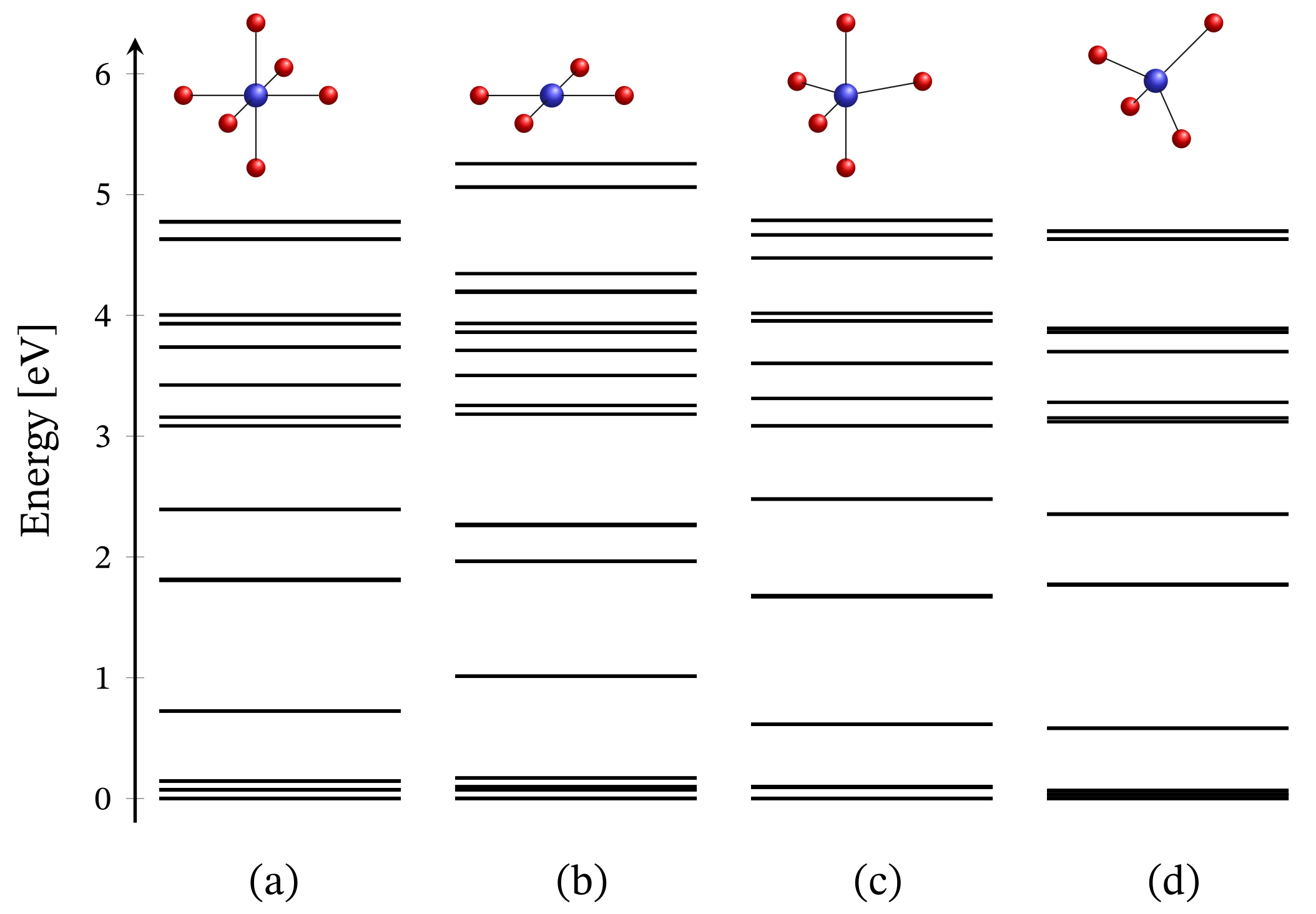
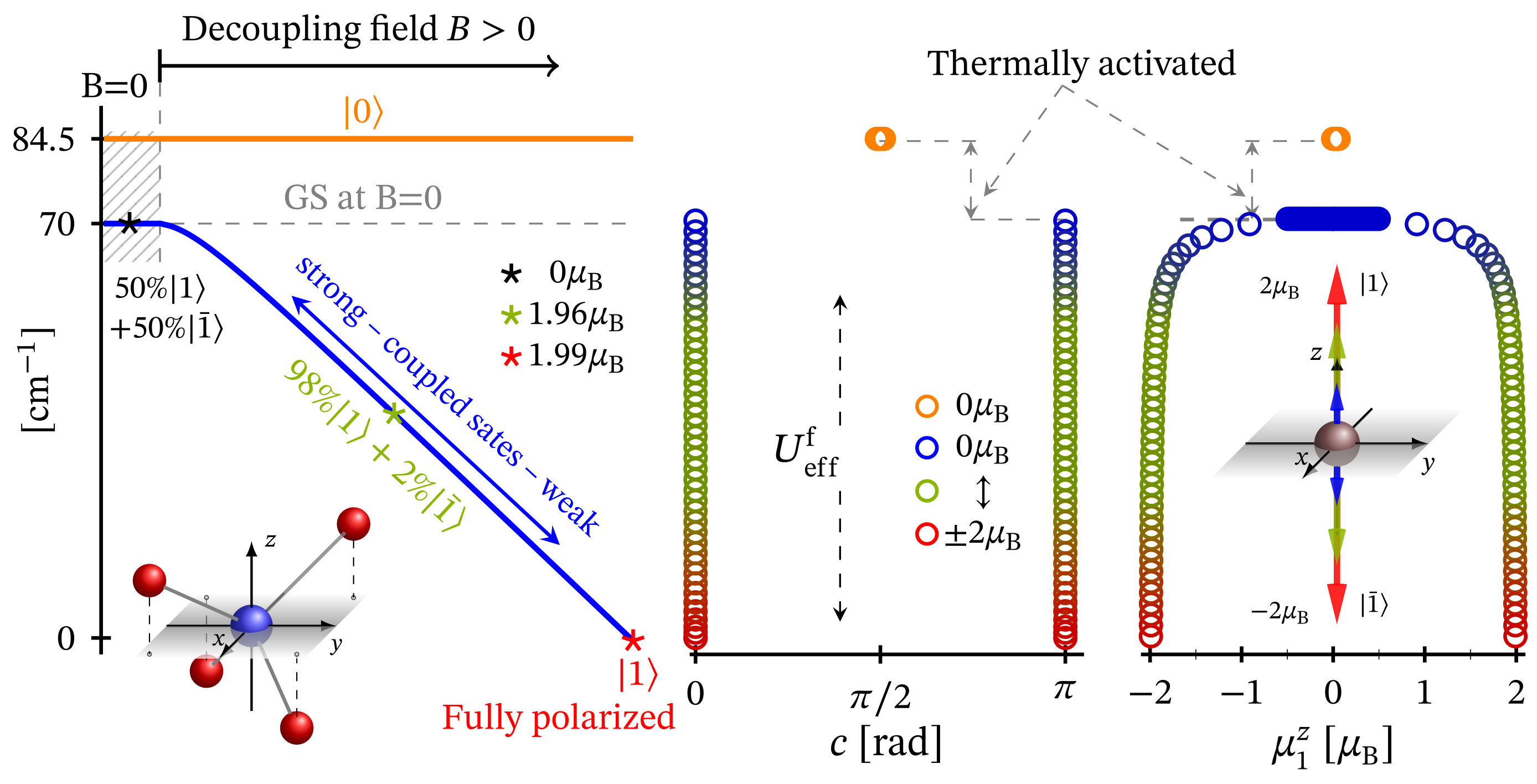
3.1. Octahedral
3.2. Square Planar
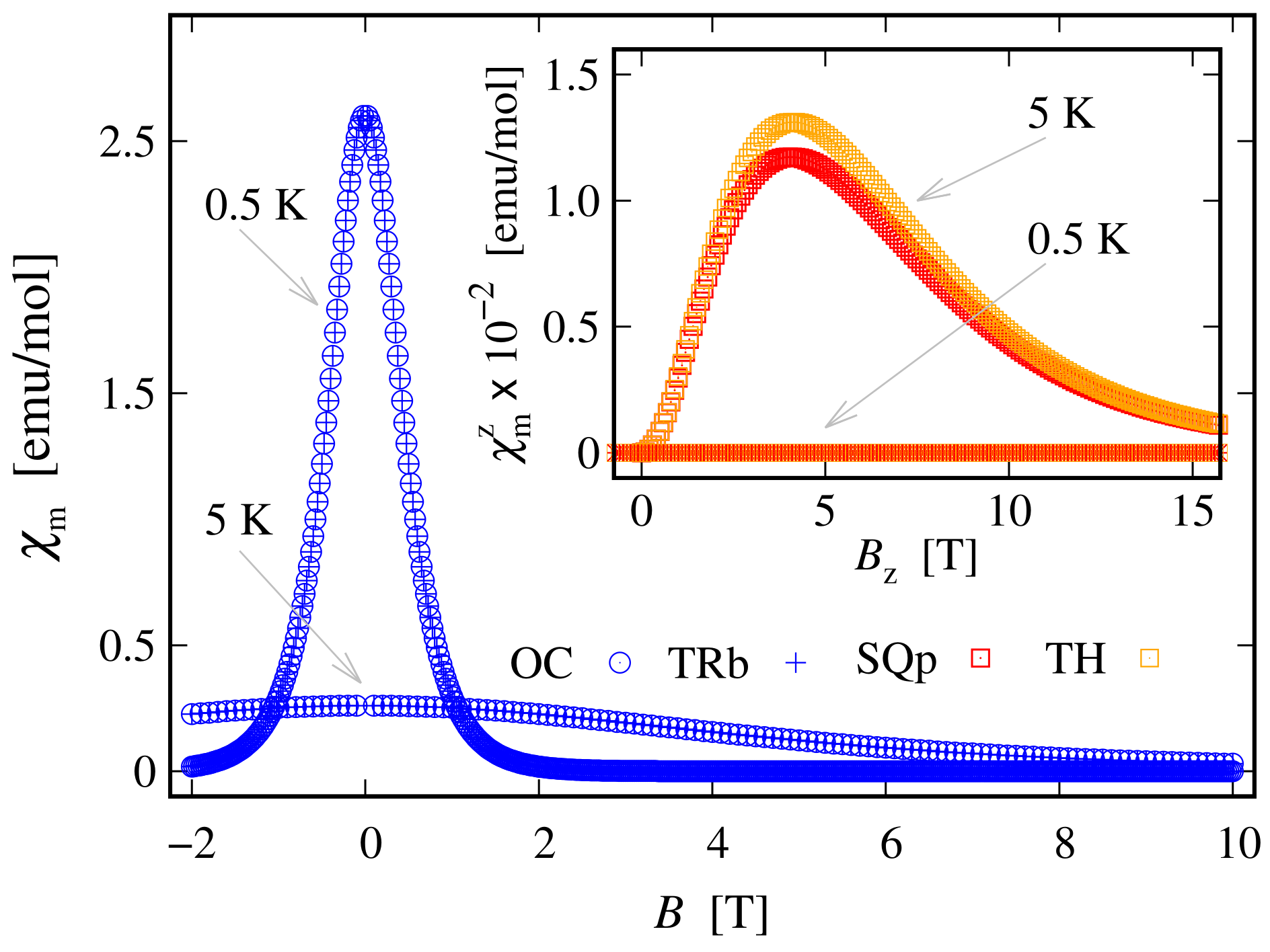
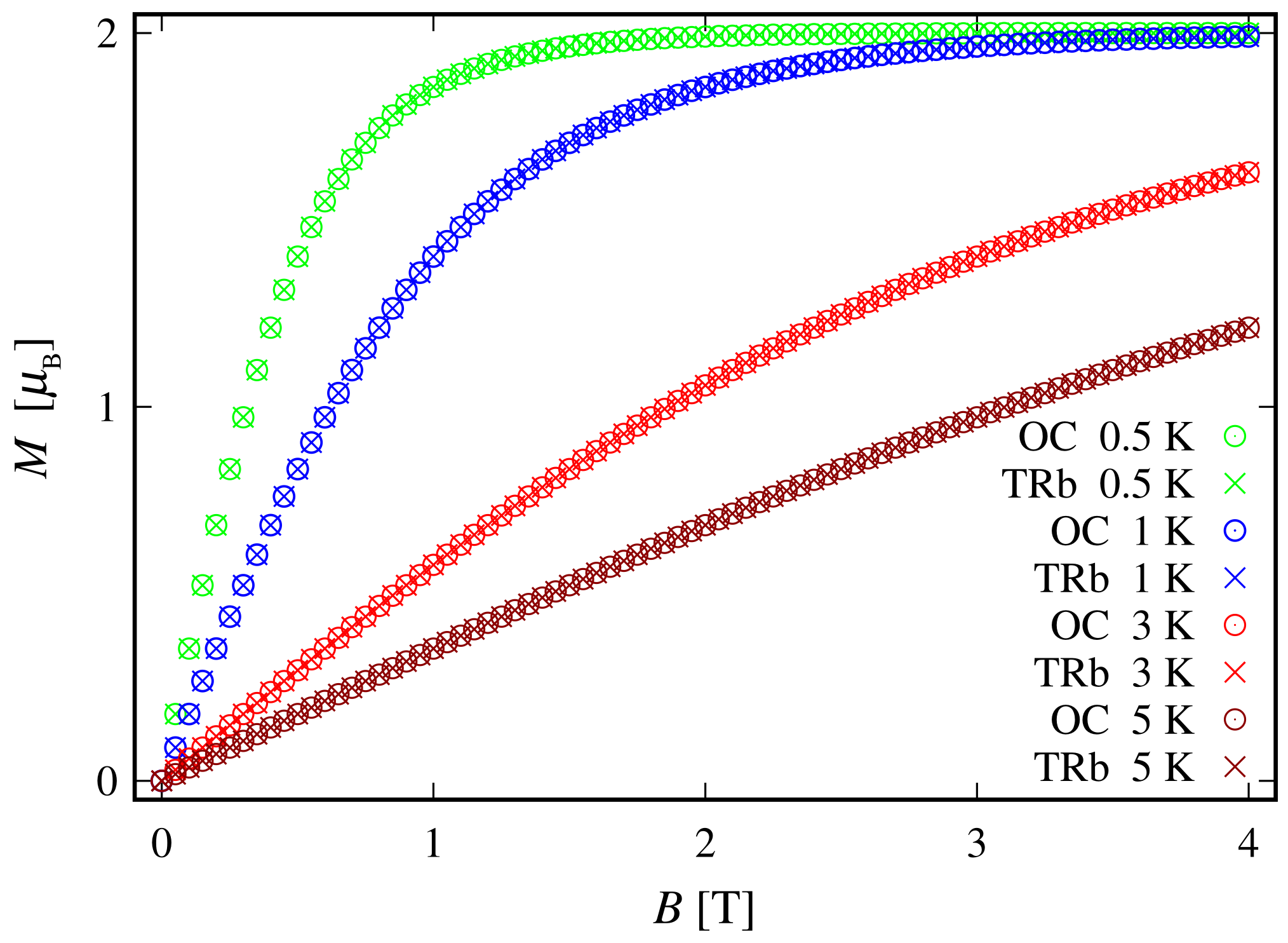
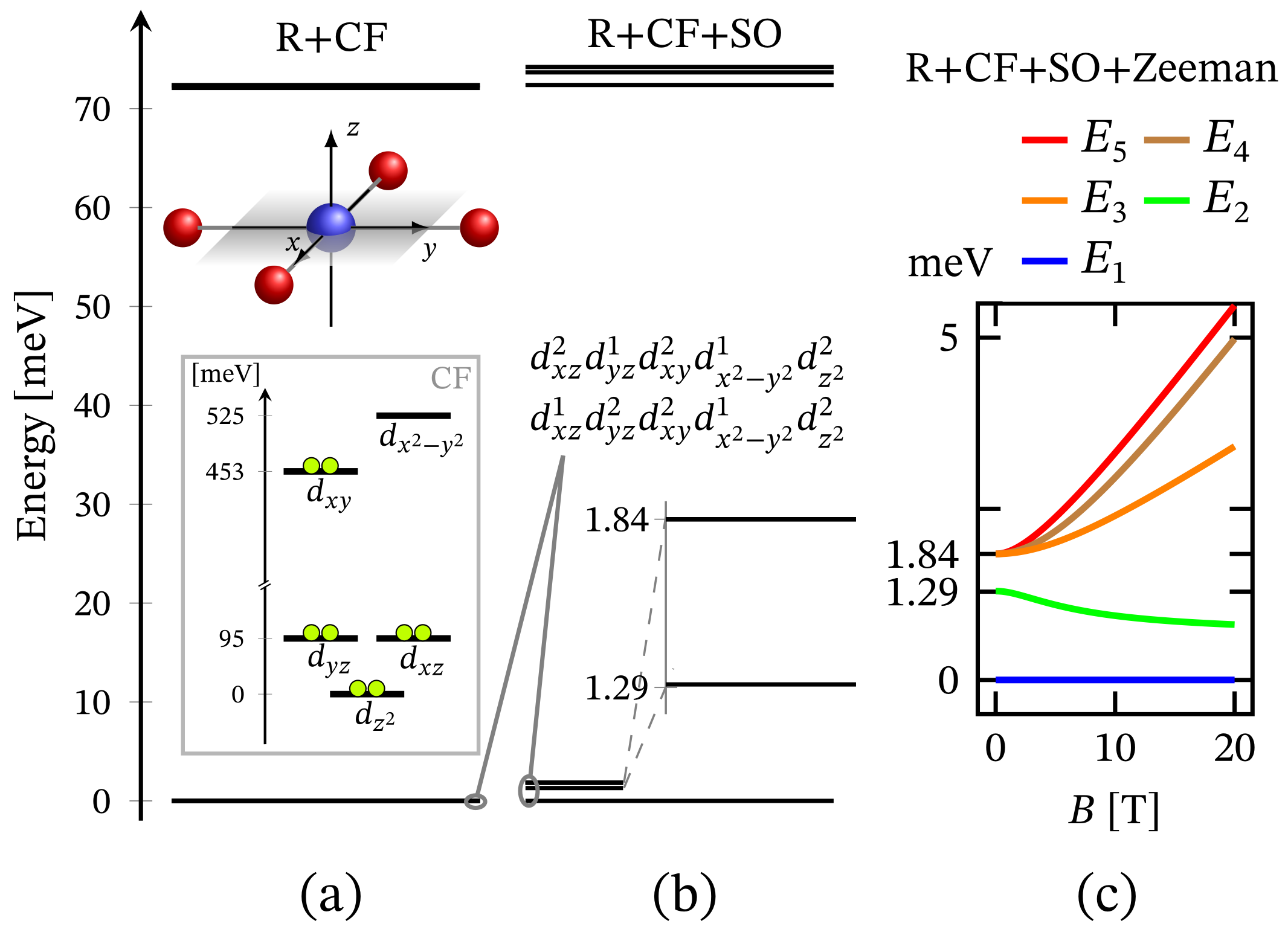
3.3. Trigonal Bipyramidal
3.4. Tetrahedral
4. Summary
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Boča, R. Theoretical Foundations of Molecular Magnetism, 1st ed.; Elsevier: New York, NY, USA, 1999. [Google Scholar]
- Kahn, O. Molecular Magnetism, 4th ed.; Wiley-VCH: New York, NY, USA, 2001. [Google Scholar]
- Linert, W.; Verdaguer, M. (Eds.) Molecular Magnets Recent Highlights; Springer: Vienna, Austria, 2003. [Google Scholar] [CrossRef]
- Coulon, C.; Miyasaka, H.; Clérac, R. Single-Chain Magnets: Theoretical Approach and Experimental Systems. In Single-Molecule Magnets and Related Phenomena; Winpenny, R., Ed.; Springer: Berlin/Heidelberg, Germany, 2006; Volume 122, pp. 163–206. [Google Scholar] [CrossRef]
- Winpenny, R. Molecular Cluster Magnets; Volume 3, World Scientific Series in Nanoscience and Nanotechnology; World Scientific: Singapore, 2011. [Google Scholar] [CrossRef]
- Bartolomé, J.; Luis, F.; Fernández, J.F. (Eds.) Molecular Magnets: Physics and Applications; NanoScience and Technology; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar] [CrossRef]
- Hołyńska, M. Single-Molecule Magnets: Molecular Architectures and Building Blocks for Spintronics; Wiley: Weinheim, Germany, 2019. [Google Scholar]
- Sessoli, R. Magnetic molecules back in the race. Nature 2017, 548, 400–401. [Google Scholar] [CrossRef] [PubMed]
- Sieklucka, B.; Pinkowicz, D. (Eds.) Molecular Magnetic Materials: Concepts and Applications; Wiley: Weinheim, Germany, 2017. [Google Scholar]
- Gatteschi, D.; Sessoli, R.; Villain, J. Molecular Nanomagnets; Oxford University Press: New York, NY, USA, 2006. [Google Scholar] [CrossRef]
- Coronado, E. Molecular magnetism: From chemical design to spin control in molecules, materials and devices. Nat. Rev. Mater. 2020, 5, 87–104. [Google Scholar] [CrossRef]
- Hu, J.; Wu, R. Giant Magnetic Anisotropy of Transition-Metal Dimers on Defected Graphene. Nano Lett. 2014, 14, 1853–1858. [Google Scholar] [CrossRef] [PubMed]
- Ou, X.; Wang, H.; Fan, F.; Li, Z.; Wu, H. Giant Magnetic Anisotropy of Co, Ru, and Os Adatoms on MgO (001) Surface. Phys. Rev. Lett. 2015, 115, 257201. [Google Scholar] [CrossRef] [PubMed]
- Karbowiak, M.; Rudowicz, C. Ground state of Ho atoms on Pt(111) metal surfaces: Implications for magnetism. Phys. Rev. B 2016, 93, 184415. [Google Scholar] [CrossRef]
- Zhang, K.C.; Li, Y.F.; Liu, Y.; Zhu, Y.; Shi, L.B. Giant magnetic anisotropy of rare-earth adatoms and dimers adsorbed by graphene oxide. Phys. Chem. Chem. Phys. 2017, 19, 13245–13251. [Google Scholar] [CrossRef]
- Rudowicz, C.; Tadyszak, K.; Ślusarski, T.; Verissimo-Alves, M.; Kozanecki, M. Modeling Spin Hamiltonian Parameters for Fe2+ (S = 2) Adatoms on Cu2N/Cu(100) Surface Using Semiempirical and Density Functional Theory Approaches. Appl. Magn. Reson. 2019, 50, 769–783. [Google Scholar] [CrossRef]
- Zuo, P.; Wang, H.; Wang, Z.; Wu, R. Large magnetic anisotropy of single transition metal adatoms on WS2. J. Magn. Magn. Mater. 2020, 506, 166796. [Google Scholar] [CrossRef]
- Thompson, L. Polynuclear coordination complexes—From dinuclear to nonanuclear and beyond. Coord. Chem. Rev. 2002, 233–234, 193–206. [Google Scholar] [CrossRef]
- Murrie, M. Cobalt(II) single-molecule magnets. Chem. Soc. Rev. 2010, 39, 1986. [Google Scholar] [CrossRef]
- Maurice, R.; de Graaf, C.; Guihéry, N. Magnetic anisotropy in binuclear complexes in the weak-exchange limit: From the multispin to the giant-spin Hamiltonian. Phys. Rev. B 2010, 81, 214427. [Google Scholar] [CrossRef]
- Liu, C.M.; Zhang, D.Q.; Zhu, D.B. Hexanuclear [Ni2 Ln2] clusters exhibiting enhanced magnetocaloric effect and slow magnetic relaxation. RSC Adv. 2014, 4, 53870–53876. [Google Scholar] [CrossRef]
- Tang, J.; Zhang, P. Polynuclear Lanthanide Single Molecule Magnets. In Lanthanides and Actinides in Molecular Magnetism; Layfield, R.A., Murugesu, M., Eds.; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2015; pp. 61–88. [Google Scholar] [CrossRef]
- Peng, Y.; Powell, A.K. What do 3d-4f butterflies tell us? Coord. Chem. Rev. 2021, 426, 213490. [Google Scholar] [CrossRef]
- Oldengott, J.C.; Schnack, J.; Glaser, T. Optimization of Single-Molecule Magnets by Suppression of Quantum Tunneling of the Magnetization. Eur. J. Inorg. Chem. 2020, 2020, 3222–3235. [Google Scholar] [CrossRef]
- Arian Zad, H.; Kenna, R.; Ananikian, N. Magnetic and thermodynamic properties of the octanuclear nickel phosphonate-based cage. Phys. A Stat. Mech. Appl. 2020, 538, 122841. [Google Scholar] [CrossRef]
- Freedman, D.E.; Harman, W.H.; Harris, T.D.; Long, G.J.; Chang, C.J.; Long, J.R. Slow Magnetic Relaxation in a High-Spin Iron(II) Complex. J. Am. Chem. Soc. 2010, 132, 1224–1225. [Google Scholar] [CrossRef] [PubMed]
- Krzystek, J.; Telser, J. Measuring giant anisotropy in paramagnetic transition metal complexes with relevance to single-ion magnetism. Dalton Trans. 2016, 45, 16751–16763. [Google Scholar] [CrossRef]
- Singh, S.K.; Rajaraman, G. Deciphering the origin of giant magnetic anisotropy and fast quantum tunnelling in Rhenium(IV) single-molecule magnets. Nat. Commun. 2016, 7, 10669. [Google Scholar] [CrossRef]
- Roy Chowdhury, S.; Mishra, S. Large Magnetic Anisotropy in Linear CoII Complexes - Ab Initio Investigation of the Roles of Ligand Field, Structural Distortion, and Conformational Dynamics. Eur. J. Inorg. Chem. 2017, 2017, 659–668. [Google Scholar] [CrossRef]
- Kumar, P.; SantaLucia, D.J.; Kaniewska-Laskowska, K.; Lindeman, S.V.; Ozarowski, A.; Krzystek, J.; Ozerov, M.; Telser, J.; Berry, J.F.; Fiedler, A.T. Probing the Magnetic Anisotropy of Co(II) Complexes Featuring Redox-Active Ligands. Inorg. Chem. 2020, 59, 16178–16193. [Google Scholar] [CrossRef]
- Hakey, B.M.; Leary, D.C.; Xiong, J.; Harris, C.F.; Darmon, J.M.; Petersen, J.L.; Berry, J.F.; Guo, Y.; Milsmann, C. High Magnetic Anisotropy of a Square-Planar Iron-Carbene Complex. Inorg. Chem. 2021, 60, 18575–18588. [Google Scholar] [CrossRef] [PubMed]
- Mašlejová, A.; Boča, R.; Dlháň, L.; Herchel, R. Magnetic anisotropy in nickel complexes as determined by combined magnetic susceptibility/magnetization/theoretical studies. J. Magn. Magn. Mater. 2004, 272–276, 380–381. [Google Scholar] [CrossRef]
- Charron, G.; Bellot, F.; Cisnetti, F.; Pelosi, G.; Rebilly, J.N.; Rivière, E.; Barra, A.L.; Mallah, T.; Policar, C. Glycoligands Tuning the Magnetic Anisotropy of NiII Complexes. Chem. Eur. J. 2007, 13, 2774–2782. [Google Scholar] [CrossRef]
- Kubica, A.; Kowalewski, J.; Kruk, D.; Odelius, M. Zero-field splitting in nickel(II) complexes: A comparison of DFT and multi-configurational wavefunction calculations. J. Chem. Phys. 2013, 138, 064304. [Google Scholar] [CrossRef] [PubMed]
- Ruamps, R.; Maurice, R.; Batchelor, L.; Boggio-Pasqua, M.; Guillot, R.; Barra, A.L.; Liu, J.; Bendeif, E.E.; Pillet, S.; Hill, S.; et al. Giant Ising-Type Magnetic Anisotropy in Trigonal Bipyramidal Ni(II) Complexes: Experiment and Theory. J. Am. Chem. Soc. 2013, 135, 3017–3026. [Google Scholar] [CrossRef]
- Gómez-Coca, S.; Cremades, E.; Aliaga-Alcalde, N.; Ruiz, E. Huge Magnetic Anisotropy in a Trigonal-Pyramidal Nickel(II) Complex. Inorg. Chem. 2014, 53, 676–678. [Google Scholar] [CrossRef]
- Gruden-Pavlović, M.; Perić, M.; Zlatar, M.; García-Fernández, P. Theoretical study of the magnetic anisotropy and magnetic tunnelling in mononuclear Ni(II) complexes with potential molecular magnet behavior. Chem. Sci. 2014, 5, 1453. [Google Scholar] [CrossRef]
- Singh, S.K.; Gupta, T.; Badkur, P.; Rajaraman, G. Magnetic Anisotropy of Mononuclear NiII Complexes: On the Importance of Structural Diversity and the Structural Distortions. Chem. Eur. J. 2014, 20, 10305–10313. [Google Scholar] [CrossRef]
- Rudowicz, C.; Açıkgöz, M.; Gnutek, P. Superposition model analysis of nickel(II) ions in trigonal bipyramidal complexes exhibiting huge zero field splitting (aka ‘giant magnetic anisotropy’). J. Magnet. Magnet. Mater. 2017, 434, 56–61. [Google Scholar] [CrossRef]
- Rudowicz, C.; Gnutek, P.; Açıkgöz, M.; Verissimo-Alves, M.; Ślusarski, T. Semiempirical and DFT/ab initio modeling of ZFS for nickel(II) complexes exhibiting very large ZFS. In Proceedings of the Third Joint Conference of Asia-Pacific EPR/ESR Society and Inter. EPR (ESR) Society (IES) 2018, Brisbane, Australia, 23–27 September 2018. Invited Talk. [Google Scholar]
- Craig, G.A.; Sarkar, A.; Woodall, C.H.; Hay, M.A.; Marriott, K.E.R.; Kamenev, K.V.; Moggach, S.A.; Brechin, E.K.; Parsons, S.; Rajaraman, G.; et al. Probing the origin of the giant magnetic anisotropy in trigonal bipyramidal Ni(II) under high pressure. Chem. Sci. 2018, 9, 1551–1559. [Google Scholar] [CrossRef]
- Chen, S.Y.; Cui, H.H.; Zhang, Y.Q.; Wang, Z.; Ouyang, Z.W.; Chen, L.; Chen, X.T.; Yan, H.; Xue, Z.L. Magnetic anisotropy and relaxation behavior of six-coordinate tris(pivalato)-Co(II) and -Ni(II) complexes. Dalton Trans. 2018, 47, 10162–10171. [Google Scholar] [CrossRef] [PubMed]
- Schulte, K.A.; Vignesh, K.R.; Dunbar, K.R. Effects of coordination sphere on unusually large zero field splitting and slow magnetic relaxation in trigonally symmetric molecules. Chem. Sci. 2018, 9, 9018–9026. [Google Scholar] [CrossRef] [PubMed]
- Suaud, N.; Rogez, G.; Rebilly, J.N.; Bouammali, M.A.; Guihéry, N.; Barra, A.L.; Mallah, T. Playing with Magnetic Anisotropy in Hexacoordinated Mononuclear Ni(II) Complexes, An Interplay Between Symmetry and Geometry. Appl. Magn. Reson. 2020, 51, 1215–1231. [Google Scholar] [CrossRef]
- Rogez, G.; Rebilly, J.N.; Barra, A.L.; Sorace, L.; Blondin, G.; Kirchner, N.; Duran, M.; van Slageren, J.; Parsons, S.; Ricard, L.; et al. Very Large Ising-Type Magnetic Anisotropy in a Mononuclear NiII Complex. Angew. Chem. Int. Ed. 2005, 44, 1876–1879. [Google Scholar] [CrossRef]
- Rebilly, J.N.; Charron, G.; Rivière, E.; Guillot, R.; Barra, A.L.; Serrano, M.; van Slageren, J.; Mallah, T. Large Magnetic Anisotropy in Pentacoordinate NiII Complexes. Chem. Eur. J. 2008, 14, 1169–1177. [Google Scholar] [CrossRef]
- Ruamps, R.; Batchelor, L.J.; Maurice, R.; Gogoi, N.; Jiménez-Lozano, P.; Guihéry, N.; de Graaf, C.; Barra, A.L.; Sutter, J.P.; Mallah, T. Origin of the Magnetic Anisotropy in Heptacoordinate NiII and CoII Complexes. Chem. Eur. J. 2013, 19, 950–956. [Google Scholar] [CrossRef]
- Darmanović, D.; Shcherbakov, I.N.; Duboc, C.; Spasojević, V.; Hanžel, D.; Anđelković, K.; Radanović, D.; Turel, I.; Milenković, M.; Gruden, M.; et al. Combined Experimental and Theoretical Investigation of the Origin of Magnetic Anisotropy in Pentagonal Bipyramidal Isothiocyanato Co(II), Ni(II), and Fe(III) Complexes with Quaternary-Ammonium-Functionalized 2,6-Diacetylpyridine Bisacylhydrazone. J. Phys. Chem. C 2019, 123, 31142–31155. [Google Scholar] [CrossRef]
- Ray, M.; Yap, G.P.A.; Rheingold, A.L.; Borovik, A.S. Synthesis and characterization of a trigonal monopyramidal nickel(II) complex. J. Chem. Soc. Chem. Commun. 1995, 17, 1777. [Google Scholar] [CrossRef]
- Marriott, K.E.R.; Bhaskaran, L.; Wilson, C.; Medarde, M.; Ochsenbein, S.T.; Hill, S.; Murrie, M. Pushing the limits of magnetic anisotropy in trigonal bipyramidal Ni(II). Chem. Sci. 2015, 6, 6823–6828. [Google Scholar] [CrossRef]
- Rudowicz, C.; Karbowiak, M. Disentangling intricate web of interrelated notions at the interface between the physical (crystal field) Hamiltonians and the effective (spin) Hamiltonians. Coord. Chem. Rev. 2015, 287, 28–63. [Google Scholar] [CrossRef]
- Abragam, A.; Bleaney, B. Electron Paramagnetic Resonance of Transition Ions; Oxford Classic Texts in the Physical Sciences; Oxford University Press: Oxford, UK, 2012. [Google Scholar]
- Misra, S.K. (Ed.) Multifrequency Electron Paramagnetic Resonance: Theory and Applications; Wiley-VCH: Weinheim, Germany, 2011. [Google Scholar]
- Buschow, K.H.J.; de Boer, F.R. Physics of Magnetism and Magnetic Materials; Kluwer: New York, NY, USA, 2003. [Google Scholar]
- White, R. Quantum Theory of Magnetism: Magnetic Properties of Materials; Springer Series in Solid-State Sciences; Springer: Berlin/Heidelberg, Germany, 2007; Volume 32. [Google Scholar]
- Rudowicz, C.; Misra, S.K. Spin-Hamiltonian formalisms in electron magnetic resonance (EMR) and related spectroscopies. Appl. Spectrosc. Rev. 2001, 36, 11–63. [Google Scholar] [CrossRef]
- Georgiev, M.; Chamati, H. Magnetostructural Dependencies in 3d2 Systems: The Trigonal Bipyramidal V3+ Complex. Phys. Status Solidi B 2022, 259, 2100645. [Google Scholar] [CrossRef]
- Georgiev, M.; Chamati, H. An Exchange Mechanism for the Magnetic Behavior of Er3+ Complexes. Molecules 2021, 26, 4922. [Google Scholar] [CrossRef]
- Furrer, A.; Krämer, K.W.; Strässle, T.; Biner, D.; Hauser, J.; Güdel, H.U. Magnetic and neutron spectroscopic properties of the tetrameric nickel compound [Mo12O28(μ2-OH)9(μ2-OH)3{Ni(H2O)3}4] · 13H2O. Phys. Rev. B 2010, 81, 214437. [Google Scholar] [CrossRef]
- Nehrkorn, J.; Höck, M.; Brüger, M.; Mutka, H.; Schnack, J.; Waldmann, O. Inelastic neutron scattering study and Hubbard model description of the antiferromagnetic tetrahedral molecule Ni4Mo12. Eur. Phys. J. B 2010, 73, 515. [Google Scholar] [CrossRef][Green Version]
- Georgiev, M.; Chamati, H. Magnetization steps in the molecular magnet Ni4Mo12 revealed by complex exchange bridges. Phys. Rev. B 2020, 101, 094427. [Google Scholar] [CrossRef]
- Georgiev, M.; Chamati, H. Single-Ion Magnets with Giant Magnetic Anisotropy and Zero-Field Splitting. ACS Omega 2022, 7, 42664. [Google Scholar] [CrossRef]
- Läuchli, A.; Mila, F.; Penc, K. Quadrupolar Phases of the S = 1 Bilinear-Biquadratic Heisenberg Model on the Triangular Lattice. Phys. Rev. Lett. 2006, 97, 087205. [Google Scholar] [CrossRef]
- Smerald, A.; Shannon, N. Theory of spin excitations in a quantum spin-nematic state. Phys. Rev. B 2013, 88, 184430. [Google Scholar] [CrossRef]
- Penc, K.; Läuchli, A.M. Spin Nematic Phases in Quantum Spin Systems. In Introduction to Frustrated Magnetism; Lacroix, C., Mendels, P., Mila, F., Eds.; Springer: Berlin/Heidelberg, Germany, 2011; Volume 164, Chapter 13; p. 331. [Google Scholar]


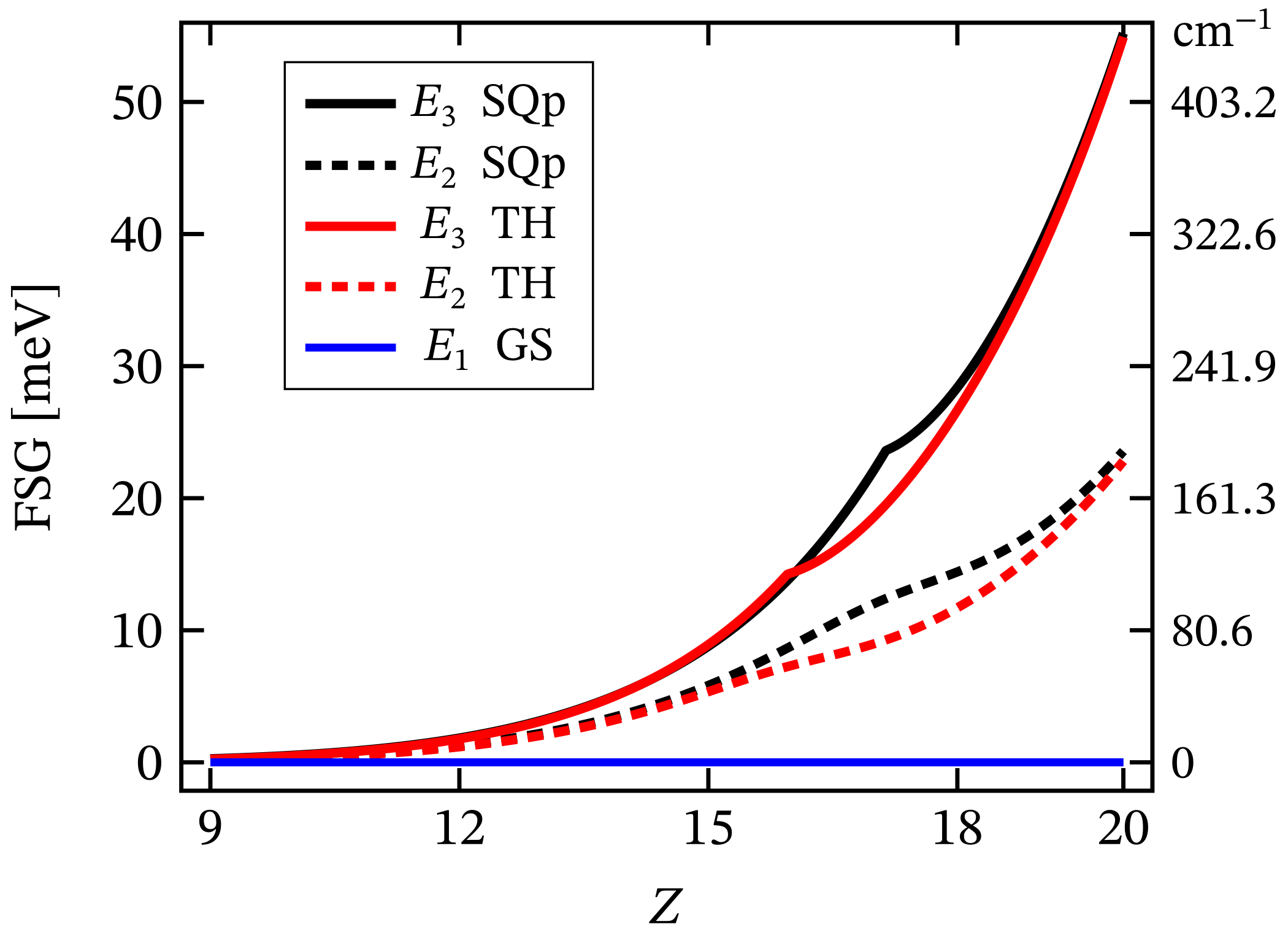

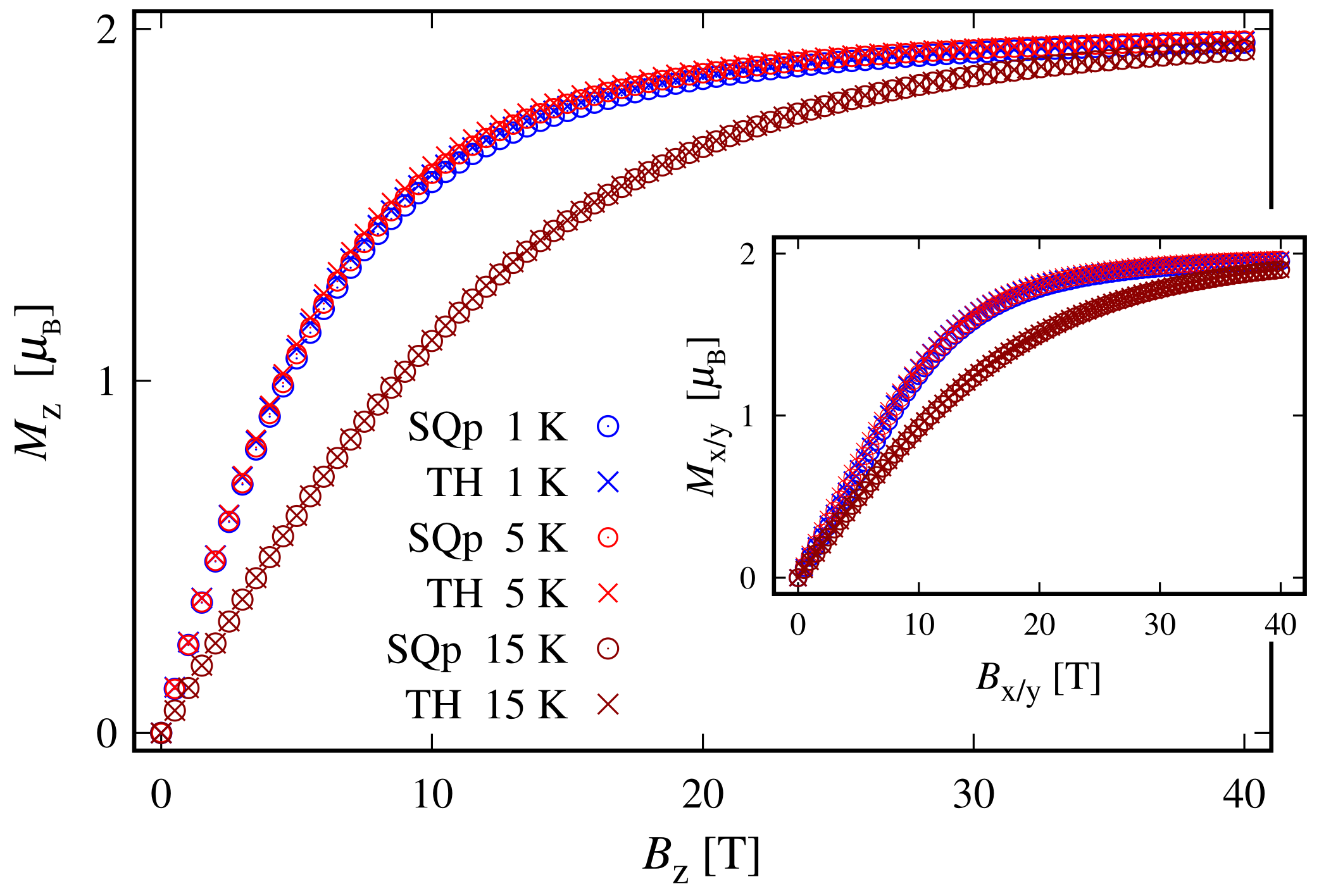
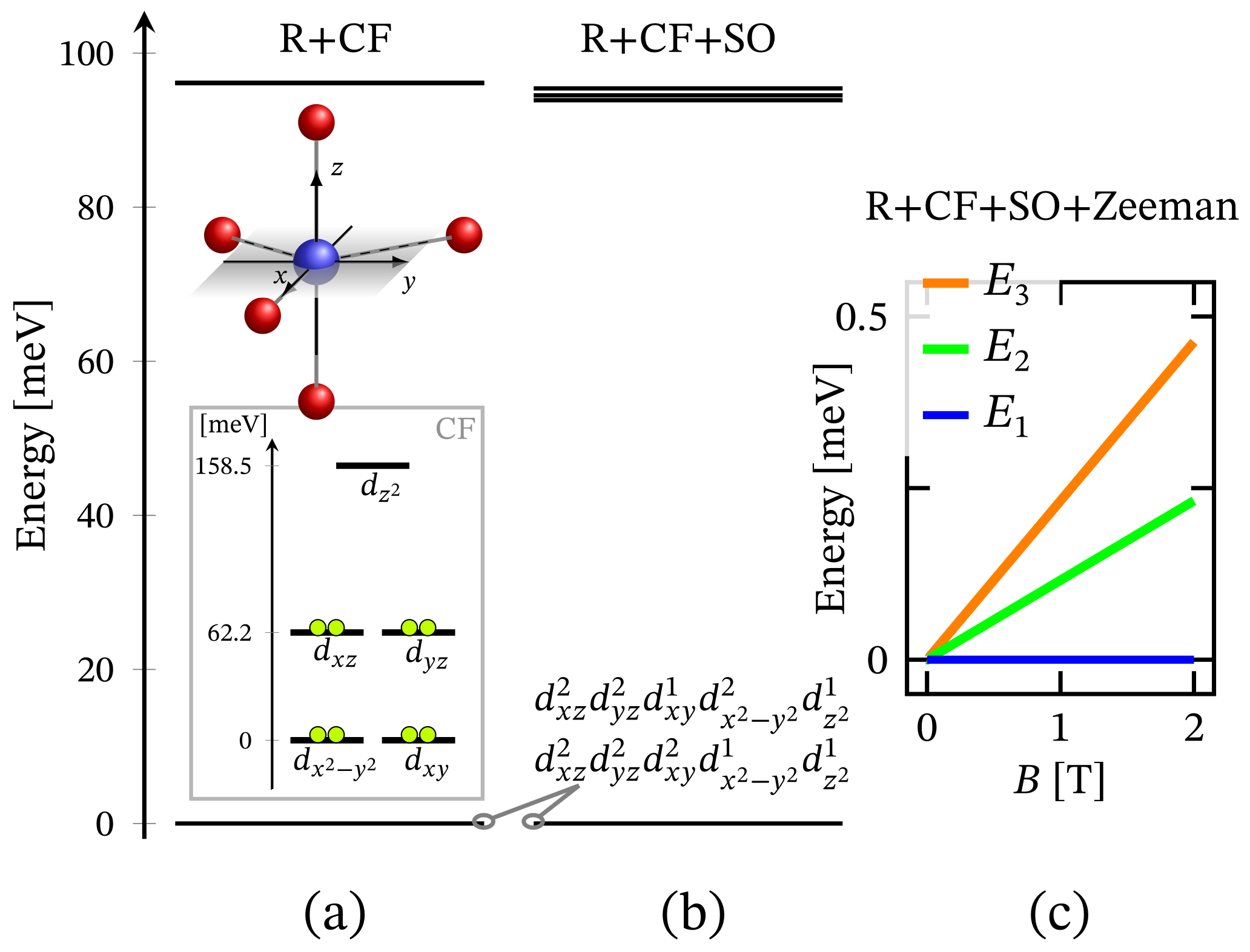
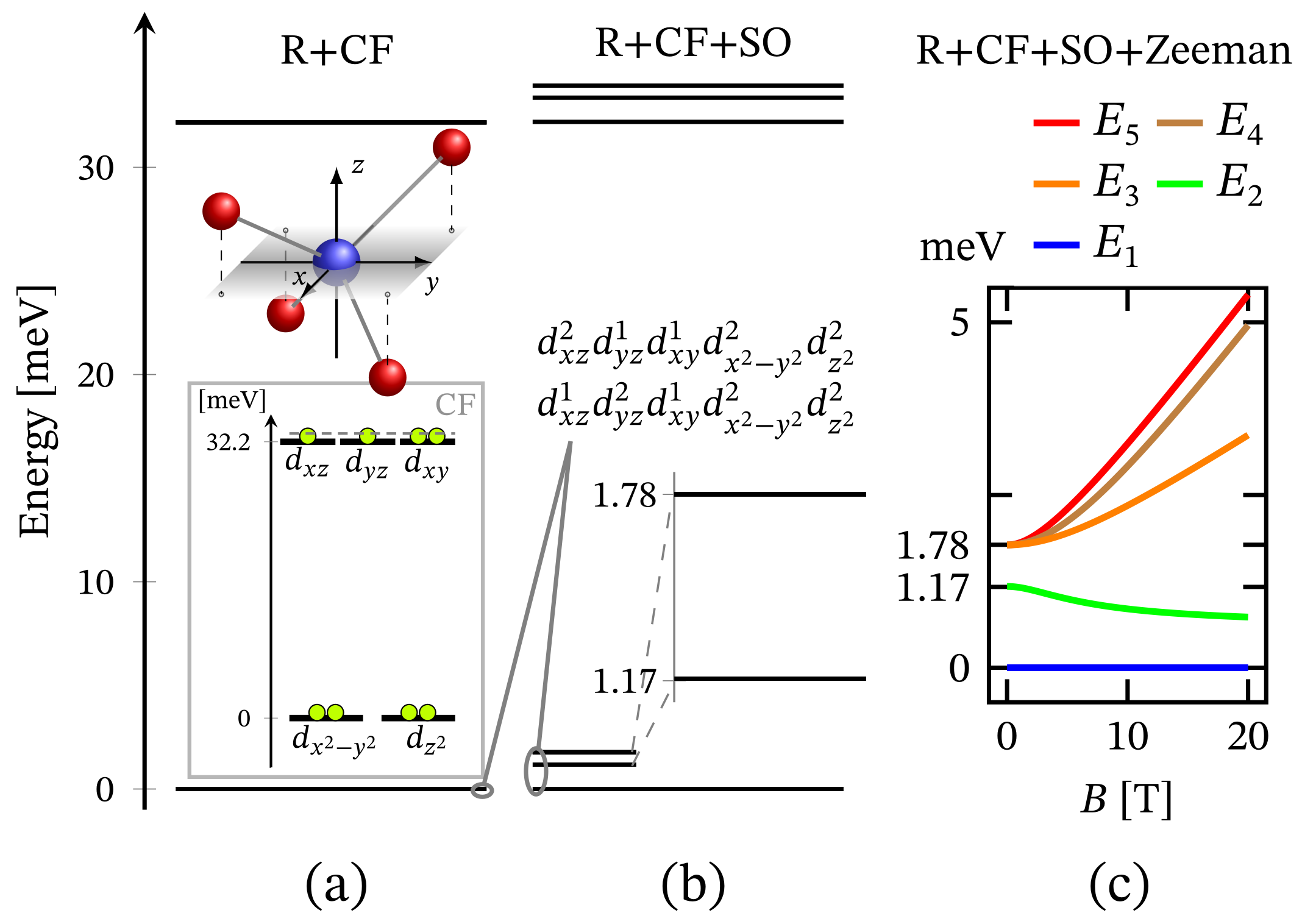
| Overall ZFS [cm] | [] | [] | [cm] | [cm] | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Complexes | V | Ni | V | Ni | V | Ni | V | Ni | V | Ni |
| Octahedral | 123 | – | 0 | 0 | – | 81 | – | |||
| Square pyramidal | 136 | 0 | 0 | 0 | 105 | 70 | ||||
| Square planar | 15 | 0 | 0 | 0 | 72 | |||||
| Trigonal bipyramidal | 278 | – | 0 | 0 | – | 169 | – | |||
| Trigonal pyramidal | 288 | – | 0 | 0 | – | 209 | – | |||
| Trigonal planar | 154 | 0 | 0 | 0 | 0 | 0 | 0 | 88 | ||
| Tetrahedral | 0 | 0 | 0 | 0 | 0 | 0 | 70 | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Georgiev, M.; Chamati, H. Fine Structure and the Huge Zero-Field Splitting in Ni2+ Complexes. Molecules 2022, 27, 8887. https://doi.org/10.3390/molecules27248887
Georgiev M, Chamati H. Fine Structure and the Huge Zero-Field Splitting in Ni2+ Complexes. Molecules. 2022; 27(24):8887. https://doi.org/10.3390/molecules27248887
Chicago/Turabian StyleGeorgiev, Miroslav, and Hassan Chamati. 2022. "Fine Structure and the Huge Zero-Field Splitting in Ni2+ Complexes" Molecules 27, no. 24: 8887. https://doi.org/10.3390/molecules27248887
APA StyleGeorgiev, M., & Chamati, H. (2022). Fine Structure and the Huge Zero-Field Splitting in Ni2+ Complexes. Molecules, 27(24), 8887. https://doi.org/10.3390/molecules27248887






