Acid–Base Properties of Oxides Derived from Oxide Melt Solution Calorimetry
Abstract
:1. Introduction
2. Acid–Base Concepts and Definitions
3. Acid–Base Strength from the Viewpoint of Chemical Bonding
4. Acid–Base Strength from the Viewpoint of Thermodynamics
5. High-Temperature Oxide Melt Solution Calorimetry, Acid–Base Reactions and Properties of Molten Oxide Solvents
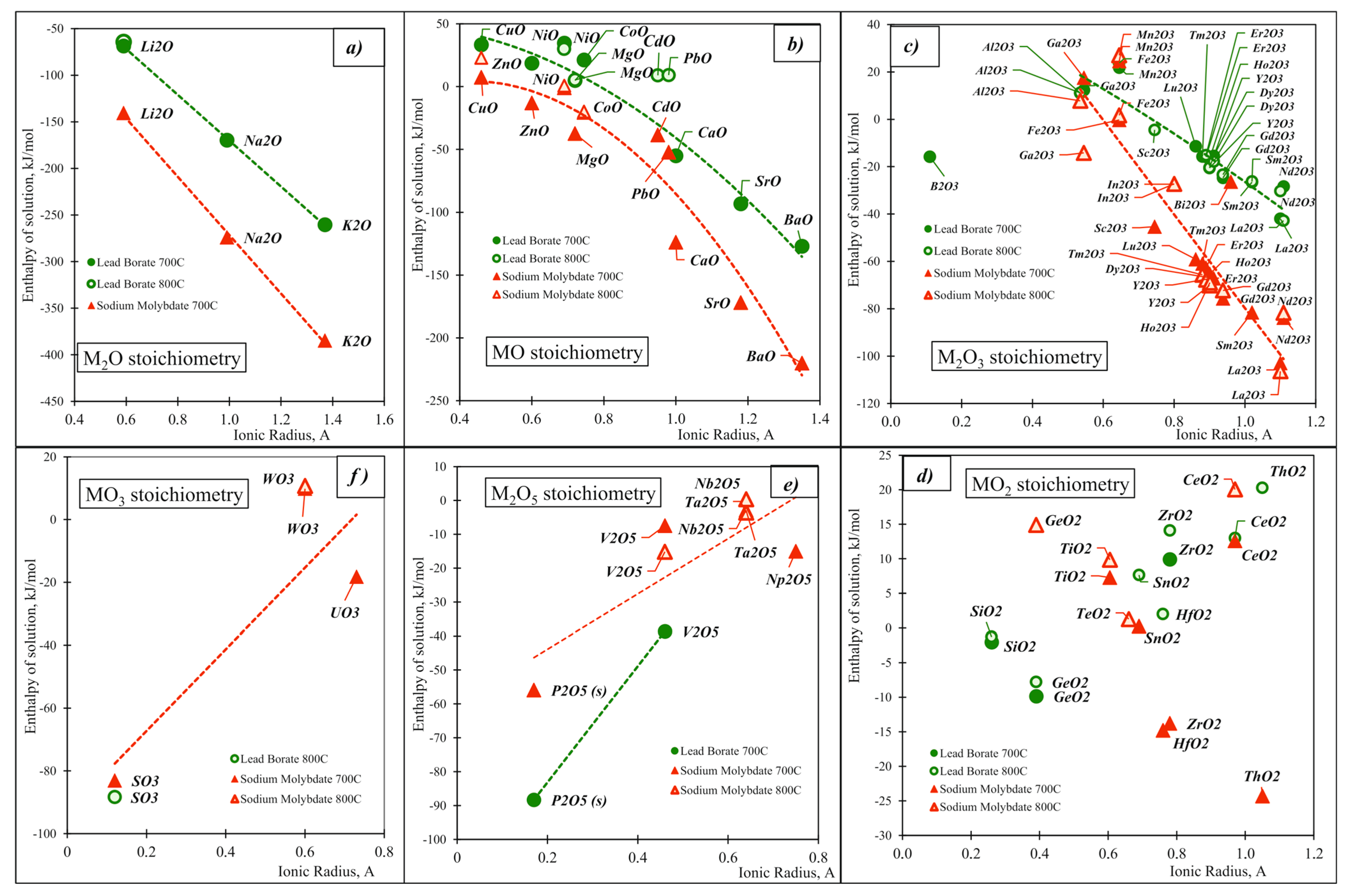
6. Enthalpies of Solution of Crystalline Binary Oxides in Molten Oxide Solvents as a Probe of Their Acid–Base Character
7. Application in Complex Aluminosilicate Melts
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Pauling, L. General Chemistry, 3rd ed.; Dover Publications: New York, NY, USA, 1988; p. 990. [Google Scholar]
- Huheey, J.E. Inorganic Chemistry, Principles of Structure and Reactivity, 3rd ed.; Harper & Row: New York, NY, USA, 1983; p. 696. [Google Scholar]
- Korzhinsky, D.S. Acid-base interactions of components in silicate melts and direction of coctic lines. Russ. Doklady AN SSSR 1959, 128, 383–386. [Google Scholar]
- Navrotsky, A. Thermochemistry of Borosilicate Melts and Glasses—From Pyrex to Pegmatites. Rev. Miner. 1996, 33, 165–180. [Google Scholar]
- Shultz, M.M.; Vedishcheva, N.M.; Shakhmatkin, B.A. Acid-base concepts in oxide glasses. Thermochim. Acta 1987, 110, 443–447. [Google Scholar] [CrossRef]
- Shultz, M.M. Acid-base concept as applied to oxide melts and glasses and the Mendeleev theory of the vitreous state. Fizika I Khimiya Stekla 1984, 10, 129. (In Russian) [Google Scholar]
- Stolyarova, V.L. High temperature mass spectrometric study of oxide systems and materials. Rapid Commun. Mass Spectrom. 1993, 7, 1022–1032. [Google Scholar] [CrossRef]
- Stolyarova, V.L.; Semenov, G.A. Mass Spectrometric Study of Vaporization Processes of Oxide Systems; Beynon, J.H., Ed.; Wiley & Sons, Ltd.: Chichester, UK, 1994; Chapter “Vaporization processes and Acid-Base Interactions in Oxide Melts”; pp. 320–333. [Google Scholar]
- Navrotsky, A. Progress and New Directions in High Temperature Calorimetry. Phys. Chem. Miner. 1977, 2, 89–104. [Google Scholar] [CrossRef]
- Navrotsky, A. Progress and New Directions in High Temperature Calorimetry. Revisited. Phys. Chem. Miner. 1997, 24, 222–241. [Google Scholar] [CrossRef]
- Navrotsky, A. Progress and New Directions in Calorimetry: A 2014 Perspective. J. Am. Ceram. Soc. 2014, 97, 3349–3359. [Google Scholar] [CrossRef]
- Brönsted, J.N. Einige Bemerkungen über den Begriff der Säuren und Basen [Some observations about the concept of acids and bases]. Recl. Des Trav. Chim. Des Pays-Bas 1923, 42, 718–728. [Google Scholar] [CrossRef]
- Lowry, T.M. The uniqueness of hydrogen. J. Soc. Chem. Ind. 1923, 42, 43–47. [Google Scholar] [CrossRef]
- Flood, H.; Förland, T. The Acidic and Basic Properties of O xides. Acta Chem. Scand. 1947, 1, 592–604. [Google Scholar] [CrossRef] [PubMed]
- Lewis, G.N. Valence and the Structure of Atoms and Molecules; Monograph Series; American Chemical Society: New York, NY, USA, 1923; p. 142. [Google Scholar]
- Usanovich, M.I. Research in the Field of the Theory of Solutions and the Theory of Acids and Bases; Nauka: Alma-Ata, Kazakhstan, 1970; p. 363. (In Russian) [Google Scholar]
- Urusov, V.S. Energy Crystal Chemistry; Nauka: Moscow, Russia, 1975; p. 335. (In Russian) [Google Scholar]
- Jensen, W.B. The Lewis acid-base definitions: A status report. Chem. Rev. 1978, 78, 1–22. [Google Scholar]
- Klopman, G. Chemical reactivity and the concept of charge- and frontier-controlled reactions. J. Am. Chem. Soc. 1968, 90, 223–234. [Google Scholar] [CrossRef]
- Laurence, C.; Gal, J.-F. Lewis Basicity and Affinity Scales: Data and Measurement; John Wiley & Sons: Chichester, UK, 2010; p. 476. [Google Scholar]
- Ramberg, H. Some crystal energetic relationships in oxysalts. J. Chem. Phys. 1952, 20, 1532–1537. [Google Scholar] [CrossRef]
- Kireev, V.A. On the acid-base properties of oxides. Russ. J. Phys. Chem. 1964, 38, 1881–1894. [Google Scholar]
- Gutmann, V.; Steininger, A.; Wychera, E. Donorstärken in 1.2-Dichloräthan. Mon. Für Chem. 1966, 97, 460–467. [Google Scholar] [CrossRef]
- Kleppa, O.J. Evolution and application of high-temperature reaction calorimetry at the University of Chicago from 1951 to 2000. J. Alloys Compd. 2001, 321, 153–163. [Google Scholar] [CrossRef]
- Navrotsky, A.; Kleppa, O.J. Enthalpy of the Anatase-Rutile Transformation. J. Am. Ceram. Soc. 1967, 50, 626. [Google Scholar]
- Navrotsky, A.; Kleppa, O.J. Enthalpies of Formation of Some Tungstates MWO4. Inorg. Chem. 1969, 8, 756–758. [Google Scholar] [CrossRef]
- Østvold, T.; Kleppa, O. Thermochemistry of Liquid Borates. II. Partial Enthalpies of Solution of Boris oxide in its liquid mixtures with lithium, sodium and potassium oxides. Inorg. Chem. 1970, 9, 1395–1400. [Google Scholar] [CrossRef]
- Chase, M.W. NIST-JANAF Thermochemical Tables 2 Volume-Set (Journal of Physical and Chemical Reference Data Monographs), 4th ed.; American Institute of Physics: College Park, MD, USA, 1998; p. 1951. [Google Scholar]
- Wu, L.; Koryttseva, A.; Groß, C.B.M.; Navrotsky, A. Thermochemical investigation of lithium borate glasses and crystals. J. Am. Ceram. Soc. 2019, 102, 4538–4545. [Google Scholar] [CrossRef]
- Robie, R.A.; Hemingway, B.S.; Fisher, J.R. Thermodynamic Properties of Minerals and Related Substances at 298.15 K and 1 Bar (105 Pascals) Pressure and at High Temperatures; U.S. Geological Survey Bulletin 1452: Washington, DC, USA, 1978; p. 464.
- Robie, R.A.; Hemingway, B.S. Thermodynamic Properties of Minerals and Related Substances at 298.15 K and 1 Bar (105 Pascals) Pressure and at Higher Temperatures; U.S. Geological Survey Bulletin 2131: Washington, DC, USA, 1995; p. 470.
- Hayun, S.; Lilova, K.; Salhov, S.; Navrotsky, A. Enthalpies of formation of high entropy and multicomponent alloys using oxide melt solution calorimetry. Intermetallics 2020, 125, 106897. [Google Scholar] [CrossRef]
- Zhang, L.; Dzik, E.A.; Sigmon, G.E.; Szymanowski, J.E.S.; Navrotsky, A.; Burns, P.S. Experimental thermochemistry of neptunium oxides: Np2O5 and NpO2. J. Nucl. Mater. 2018, 501, 398–403. [Google Scholar] [CrossRef]
- Mazeina, L.; Ushakov, S.; Navrotsky, A.; Boatner, L.A. Formation enthalpy of ThSiO4 and enthalpy of the thorite → huttonite phase transition. Geochim. Et Cosmochim. Acta 2005, 69, 4675–4683. [Google Scholar] [CrossRef]
- Lee, T.A.; Navrotsky, A. Enthalpy of formation of cubic yttria-stabilized hafnia. J. Mat. Res. 2004, 19, 1855–1861. [Google Scholar] [CrossRef]
- Guo, X.; Szenknect, S.; Mesbah, A.; Labs, S.; Clavier, N.; Poinssot, C.; Ushakov, S.V.; Curtius, H.; Bosbach, D.; Ewing, R.C.; et al. Thermodynamics of formation of coffinite, USiO4. Proc. Natl. Acad. Sci. USA 2015, 112, 6551–6555. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lepple, M.; Ushakov, S.V.; Lilova, K.; Macauley, C.A.; Fernandez, A.N.; Levi, C.G.; Navrotsky, A. Thermochemistry and phase stability of the polymorphs of yttrium tantalate, YTaO4. J. Eur. Ceram. Soc. 2021, 41, 1629–1638. [Google Scholar] [CrossRef]
- Putnam, R.L.; Navrotsky, A.; Woodfield, B.F.; Boerio-Goates, J.; Shapiro, J.L. Thermodynamics of formation for zirconolite (CaZrTi2O7) from T = 298.15K to T = 1500K. J. Chem. Thermodyn. 1999, 31, 229–243. [Google Scholar] [CrossRef]
- Barin, I.; Knacke, O.; Kubaschewski, O. Thermochemical Properties of Inorganic Substances; Springer: Berlin, Germany, 1977; p. 861. [Google Scholar]
- Abramchuk, M.; Lilova, K.; Subramani, T.; Yoo, R.; Navrotsky, A. Development of high-temperature oxide melt solution calorimetry for p-block element containing materials. J. Mater. Res. 2020, 35, 2239–2246. [Google Scholar] [CrossRef]
- Yokokawa, T.; Kleppa, O. A calorimetric Study of the lead (II) oxide-vanadium (V) oxide system at 680°. Inorg. Chem. 1964, 3, 954–957. [Google Scholar] [CrossRef]
- Holm, J.; Kleppa, O. Thermochemistry of the liquid system lead (II) oxide—Boron oxide at 800°. Inorg. Chem. 1967, 6, 645–648. [Google Scholar] [CrossRef]
- Østvold, T.; Kleppa, O. Thermochemistry of the liquid system lead oxide—Silica at 900°. Inorg. Chem. 1969, 8, 78–82. [Google Scholar] [CrossRef]
- Muller, F.; Kleppa, O. A calorimetric study of the liquid system lead (II) oxide-germanium oxide at 900 °C. Z. Anorg. Allg. Chem. 1973, 397, 171–178. [Google Scholar] [CrossRef]
- Navrotsky, A.; Kleppa, O.J. A calorimetric study of molten Na2MoO4-MoO3 mixtures at 970 K. Inorg. Chem. 1967, 6, 2119–2121. [Google Scholar] [CrossRef]
- Koryttseva, A.; Navrotsky, A. High-temperature calorimetric study of oxide component dissolution in a CaO–MgO–Al2O3–SiO2 slag at 1450 °C. J. Am. Ceram. Soc. 2017, 100, 1172–1177. [Google Scholar] [CrossRef]
- Costa, G.; Harder, B.J.; Bansal, N.P.; Stokes, J.L.; Lilova, K.; Subramani, T.; Ushakov, S.V.; Meisner, K.J.; Navrotsky, A. Energetics of reactions between ceramic coating materials and their binary oxide components with silicate melts. J. Am. Ceram. Soc. 2022, 105, 7795–7805. [Google Scholar] [CrossRef]
- Takayama-Muromachi, E.; Navrotsky, A. Energetics of compounds (A2+B4+O3) with the Perovskite Structure. J. Solid State Chem. 1988, 72, 244–256. [Google Scholar] [CrossRef]
- Brousse, C.; Newton, R.C.; Kleppa, O.J. Enthalpy of formation of forsterite, enstatite, akermanite, monticellite and merwinite at 1073 K determined by alkali borate solution calorimetry. Geochim. Cosmochim. Acta 1984, 48, 1081–1088. [Google Scholar] [CrossRef]
- Geiger, C.A.; Kleppa, O.J.; Mysen, B.O.; Lattimer, J.M.; Grossman, L. Enthalpies of formation of CaAl4O7 and CaAl12O19 (hibonite) by high temperature, alkali borate solution calorimetry. Geochim. Cosmochim. Acta 1988, 52, 1729–1736. [Google Scholar] [CrossRef]
- Mulliken, R.S. A new affinity scale, together with data on valence states and valence ionization potentials and electron affinities. J. Chem. Phys. 1934, 2, 782–793. [Google Scholar] [CrossRef]
- Viting, L.M. Vysokotemperaturnye Rastvory-Rasplavy (High Temperature Solutions-Melts); Moscow University Publ.: Moscow, Russia, 1991; p. 220. (In Russian) [Google Scholar]
- Sanderson, R.T. Chemical Periodicity; ACS Publications: New York, NY, USA, 1960; p. 330. [Google Scholar]
- Shannon, R.D. Revised Effective Ionic Radii and Systematic Studies of Interatomic Distances in Halides and Chalcogenides. Acta Cryst. 1976, A32, 751–767. [Google Scholar] [CrossRef]
- Morishita, M.; Navrotsky, A.; Wilding, M.C. Direct Measurement of Relative Partial Molar Enthalpy of SiO2 in SiO2–M2O (M = Li, Na, K, Cs) Binary and SiO2–CaO–Al2O3 Ternary Melts. J. Am. Ceram. Soc. 2004, 87, 1550–1555. [Google Scholar] [CrossRef]
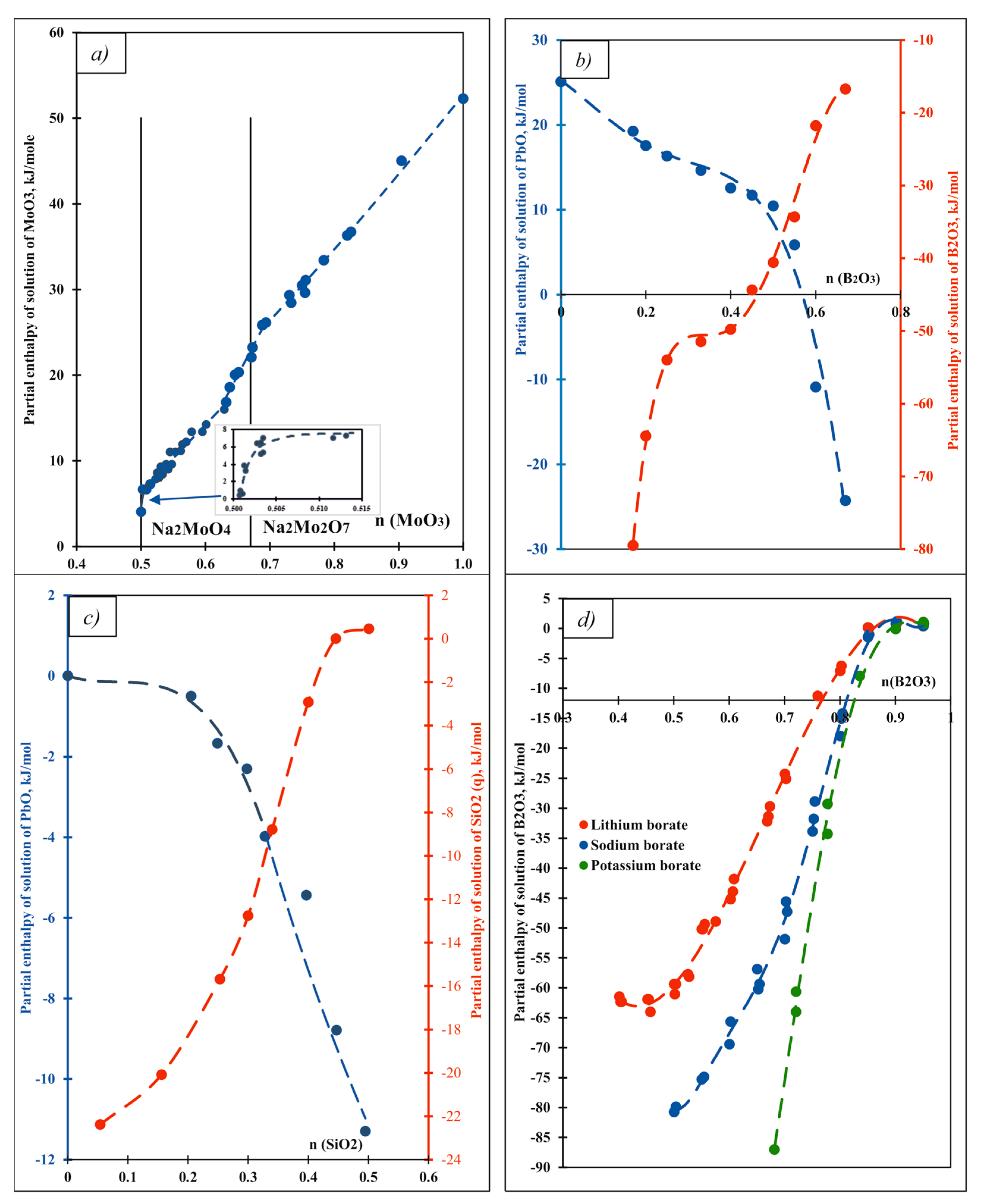

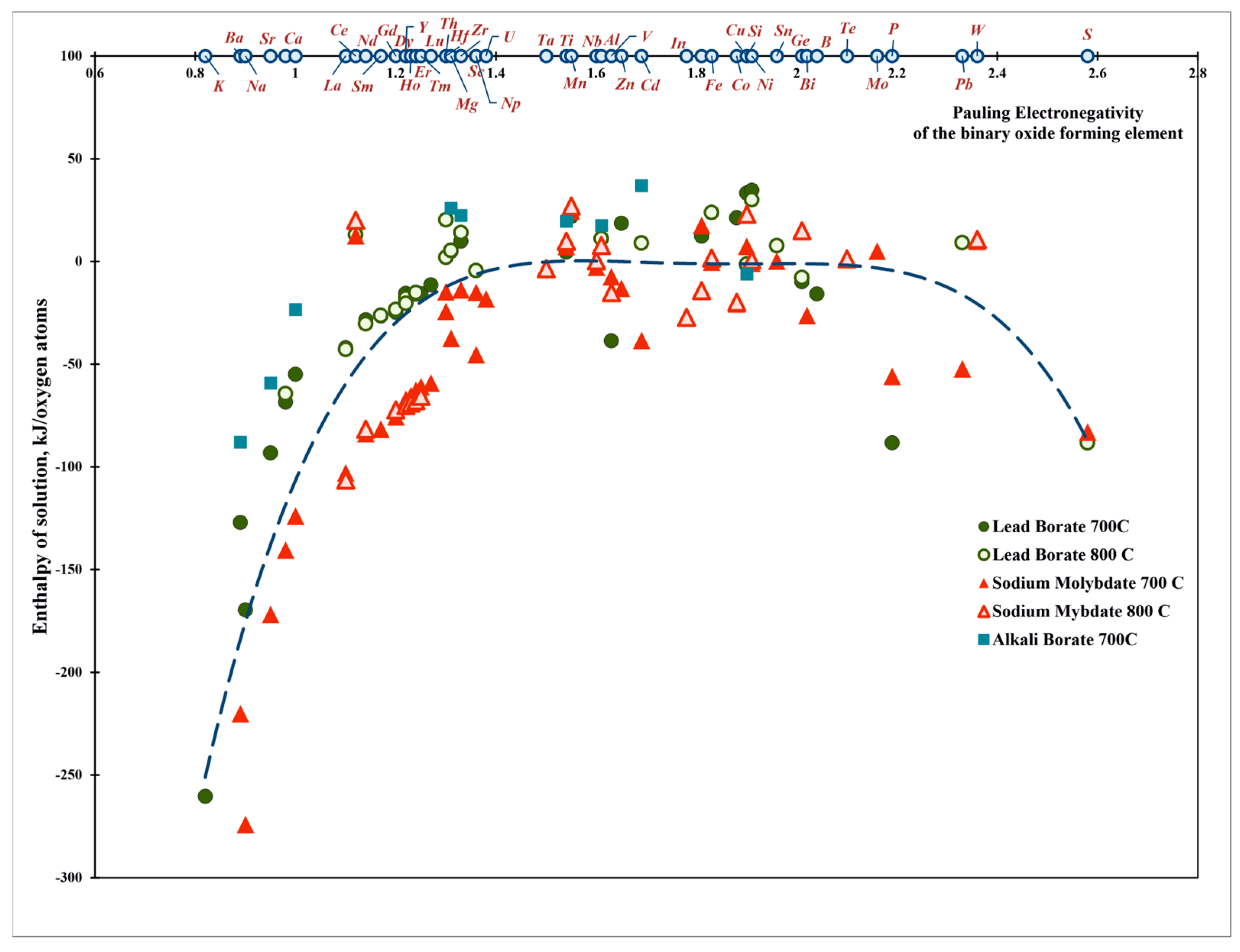
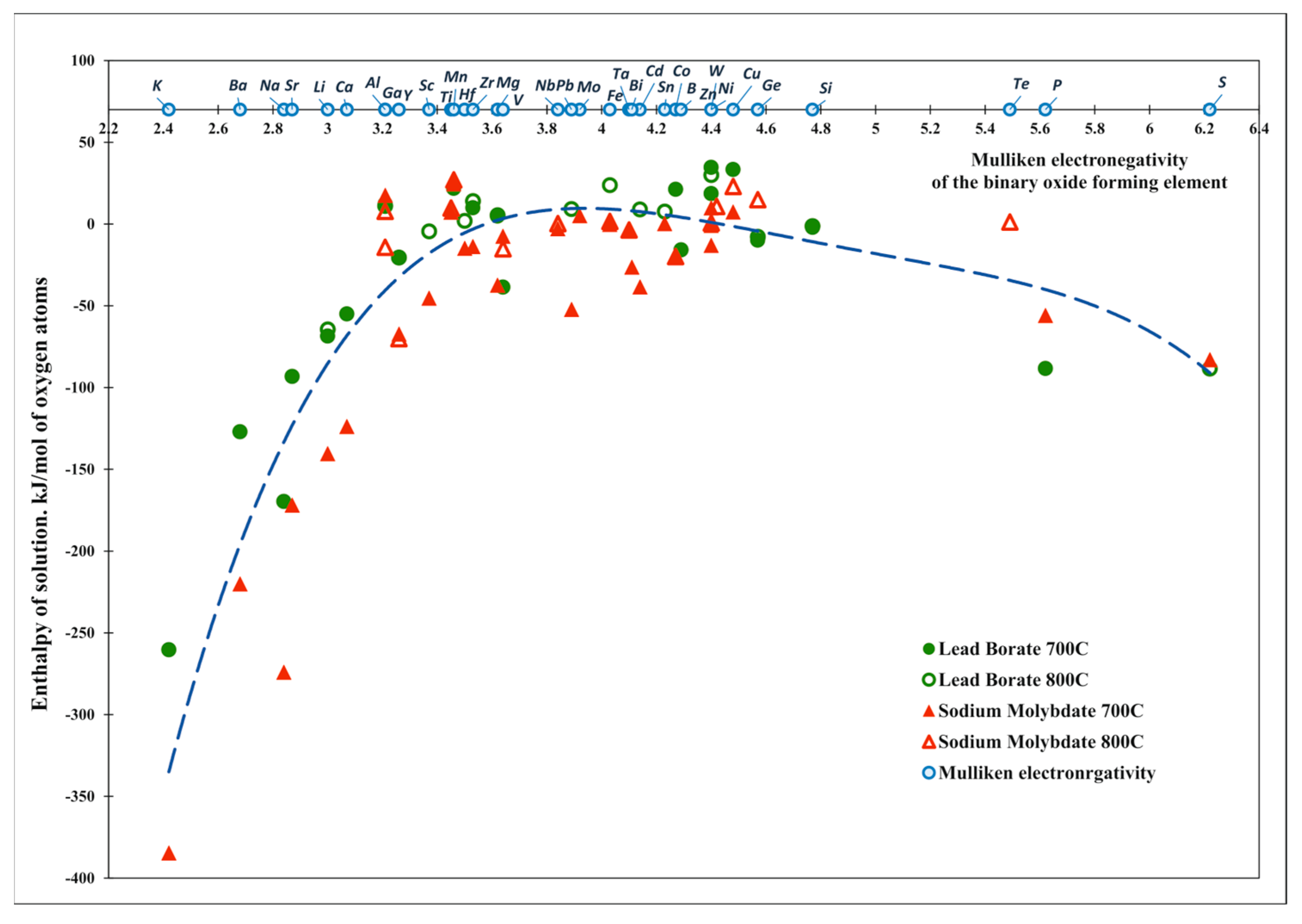
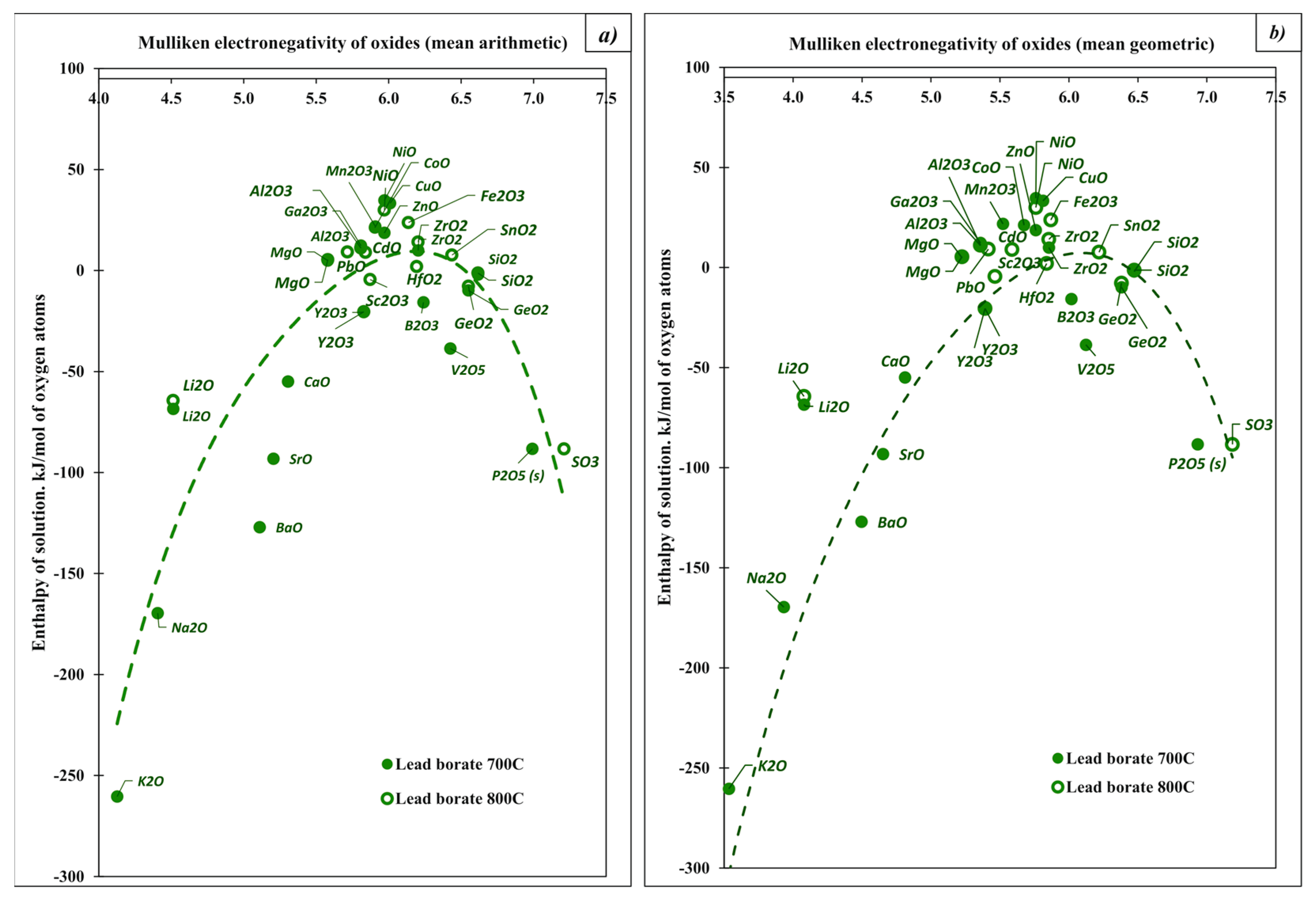


| Oxide | Pauling Electro-Negativity α | Heat Content H0(T)-H0(25 °C), kJ/mol | Enthalpy of Drop Solution (kJ/mol) and Enthalpy of Solution (kJ/mol of Oxygen Atoms) | ||||
|---|---|---|---|---|---|---|---|
| Lead Borate (2PbO·B2O3) | Sodium Molybdate (3Na2O·4MoO3) | ||||||
| 700 °C | 800 °C | ||||||
| K2O (s) | 0.82 | 66.70 [28] | 78.17 [28] | −193.68 ± 1.10 [11] | −260.38 | −318.0 ± 3.1 [11] | −384.7 |
| BaO (s) | 0.89 | 35.55 [28] | 41.20 [28] | −91.5 ± 1.9 [11] | −127.05 | −184.61 ± 3.21 [11] | −220.16 |
| Na2O (s) | 0.9 | 56.52 [28] | 68.76 [28] | −113.10 ± 0.83 [11] | −169.62 | −217.56 ± 4.25 [11] | −274.08 |
| SrO (s) | 0.95 | 34.67 [28] | 40.21 [28] | −58.5 ± 2.0 [11] | −93.17 | −137.2 ± 3.8 [11] | −171.87 |
| Li2O (s) | 0.98 | 50.20 [28] | 58.52 [28] | −18.28 ± 2.17 [11] | −68.48 | −90.3 ± 2.5 [11] | −140.5 |
| −5.85 ± 0.65 [29] | −64.37 | ||||||
| CaO (s) | 1.00 | 33.55 [28] | 38.87 [28] | −21.4 ± 1.9 [11] | −54.95 | −90.3 ± 1.8 [11] | −123.85 |
| La2O3 (s, A-type) | 1.10 | 83.73 [30] | 97.43 [30] | −42.3 ± 4.4 [11] | −42.01 | −225.1 ± 3.16 [11] | −102.94 |
| −30.91 ± 0.61 [11] | −42.78 | −221.81 ± 2.25 [11] | −106.41 | ||||
| CeO2 (s, cub) | 1.12 | 49.19 [31] | 57.33 [31] | 74.37 ± 0.75 [11] | 12.59 | ||
| 83.34 ± 1.86 [11] | 13.00 | 97.40 ± 0.98 [32] | 20.04 | ||||
| Nd2O3 (s, A-type, hex) | 1.14 | 88.09 [30] | 102.78 [30] | 3.04 ± 3.70 [11] | −28.35 | −163.36 ± 3.44 [11] | −83.82 |
| 11.82 ± 0.99 [11] | −30.32 | −142.20 ± 0.83 [11] | −81.66 | ||||
| Sm2O3 (s, B-type, mon.) | 1.17 | 91.15 [30] | 106.17 [30] | 11.5 ± 4.1 [11] | −26.55 | −153.62 ± 2.86 [11] | −81.59 |
| 27.3 ± 0.6 [11] | −26.29 | ||||||
| Gd2O3 (s, C-type, cubic) | 1.20 | 82.58 [30] | 95.86 [30] | 8.4 ± 3.4 [11] | −24.73 | −144.34 ± 1.35 [11] | −75.64 |
| 25.7 ± 0.2 [11] | −23.39 | −121.0 ± 3.2 [11] | −72.29 | ||||
| Dy2O3 (s, C-type, cub) | 1.22 | 86.70 [30] | 100.39 [30] | 40.2 ± 1.2 [11] | −15.5 | −114.88 ± 2.22 [11] | −67.19 |
| 46.9 ± 0.4 [11] | −17.83 | ||||||
| Y2O3 (s, C-type, cub) | 1.22 | 81.19 [30] | 94.11 [30] | 19.6 ± 1.1 [11] | −20.53 | −120.74 ± 0.94 [11] | −67.31 |
| 32.8 ± 0.8 [11] | −20.44 | −116.3 ± 1.2 [11] | −70.14 | ||||
| Ho2O3 (s, C-type, cub) | 1.23 | 84.13 [30] | 97.35 [30] | 35.1 ± 5.6 [11] | −16.34 | −111.72 ± 3.68 [11] | −65.28 |
| −109.51 ± 1.84 [11] | −68.95 | ||||||
| Er2O3 (s, C-type, cub) | 1.24 | 82.91 [30] | 96.13 [30] | 35.3 ± 1.7 [11] | −15.87 | −105.26 ± 2.48 [11] | −62.72 |
| 50.6 ± 0.4 [11] | −15.18 | −107.2 ± 1.8 [11] | −67.78 | ||||
| Tm2O3 (s) | 1.25 | 85.71 [30] | 99.06 [30] | 38.6 ± 2.8 [11] | −15.70 | −97.12 ± 2.38 [11] | −60.94 |
| −97.97 ± 1.10 [11] | −65.68 | ||||||
| Lu2O3 (s, C-type, cub) | 1.27 | 80.40 [30] | 93.61 [30] | 46.2 ± 1.2 [11] | −11.4 | −96.90 ± 1.90 [11] | −59.10 |
| ThO2 (s) | 1.3 | 49.50 [33] | 57.5 ± 1.7 [34] | 0.89 ± 0.48 [31] | −24.31 | ||
| 98.1 ± 1.7 [34] | 20.3 ± 2.4 | ||||||
| HfO2 (s, monocl) | 1.3 | 49.56 [30] | 57.78 [30] | 20.0 ± 2.2 [35] | −14.78 | ||
| 61.75 ± 1.38 [11] | 1.99 | ||||||
| MgO (s) | 1.31 | 31.60 [28] | 36.71 [28] | 36.48 ± 0.50 [11] | 4.88 | −5.79 ± 0.15 [11] | −37.39 |
| 42.09 ± 0.41 [11] | 5.38 | ||||||
| ZrO2 (s) | 1.33 | 47.07 [28] | 54.68 [28] | 66.93 ± 0.92 [11] | 9.93 | 19.5 ± 0.9 [11] | −13.79 |
| 82.9 ± 0.7 [11] | 14.11 | ||||||
| Sc2O3 (s) | 1.36 | 78.25 [30] | 91.21 [30] | −57.72 ± 0.98 [11] | −45.32 | ||
| 77.83 ± 1.89 [11] | −4.46 | ||||||
| Np2O5 (s) | 1.36 | 109.26 [33] | 34.22 ± 5.34 [33] | −15.00 | |||
| γ-UO3 (s) | 1.38 | 64.22 [33] | 9.49 ± 1.53 [36] | −18.24 | |||
| 26.67 ± 4.02 [36] | |||||||
| Ta2O5 (s) | 1.5 | 110.71 [28] | 129.29 [28] | 95.8 ± 3.6 [37] | −2.98 | ||
| 111.41 ± 1.61 [32] | −3.58 | ||||||
| TiO2 (s, rutile) | 1.54 | 46.26 [28] | 53.70 [28] | 55.4 ± 1.2 [38] | 4.57 | 60.81 ± 0.11 [11] | 7.28 |
| 73.37 ± 0.36 [32] | 9.84 | ||||||
| Mn2O3 (s, cub) | 1.55 | 81.10 [31] | 94.75 [31] | 146.60 ± 1.6 [11] | 21.83 | 154.70 ± 1.00 [11] | 24.53 |
| 175.79 ± 1.38 [32] | 27.01 | ||||||
| Nb2O5 (s) | 1.6 | 108.00 [28] | 125.40 [28] | 93.97 ± 1.60 [11] | −2.81 | ||
| 127.05 ± 0.86 [32] | 0.33 | ||||||
| α-Al2O3 (s, cor.) | 1.61 | 74.26 [28] | 86.68 [28] | 107.38 ± 0.15 [11] | 11.04 | ||
| 120.12 ± 0.17 [11] | 11.15 | 110.08 ± 1.17 [32] | 7.80 | ||||
| V2O5 (s) | 1.63 | 177.19(l) [28] | 196.25(l) [28] | −15.92 ± 0.45 [11] | −38.62 | 140.0 ± 2.1 [11] | −7.44 |
| 120.46 ± 0.57 [32] | −15.16 | ||||||
| ZnO (s, hex.) | 1.65 | 32.40 [31] | 37.66 | 51.03 ± 0.36 [11] | 18.63 | 19.4 ± 0.7 [11] | −13.00 |
| CdO (s) | 1.69 | 33.64 [39] | 39.00 [39] | −4.82 ± 0.28 [11] | −38.46 | ||
| 47.98 ± 0.81 [11] | 8.98 | ||||||
| In2O3 (s) | 1.78 | 81.11 [39] | 93.98 [39] | −1.12 ± 0.25 [11] | −27.41 | ||
| 12.23 ± 1.03 [11] | −27.25 | ||||||
| Ga2O3 (s) | 1.81 | 77.45 [30] | 90.11 [30] | 114.38 ± 1.17 [11] | 12.31 | 130.16 ± 1.66 [11] | 17.57 |
| 132.46 ± 1.88 [11] | 14.17 | ||||||
| Fe2O3 (s) | 1.83 | 96.43 (II-modif) [28] | 110.94 (III-modif) [28] | 95.63 ± 0.50 [11] | −0.27 | ||
| 182.29 ± 1.34 [11] | 23.78 | 115.92 ± 1.57 [32] | 1.66 | ||||
| CoO (s) | 1.88 | 36.25 [28] | 41.80 [28] | 57.48 ± 0.93 [11] | 21.23 | 15.66 ± 0.59 [11] | −20.59 |
| 21.92 ± 0.36 [32] | −19.88 | ||||||
| CuO (s) | 1.90 | 34.55 [28] | 40.07 [28] | 67.9 ± 0.6 [11] | 33.35 | 41.9 ± 0.6 [11] | 7.35 |
| 63.05 ± 0.40 [32] | 22.98 | ||||||
| SiO2 (s, quartz) | 1.90 | 43.46 [28] | 50.37 [28] | 39.4 ± 0.4 [11] | −2.03 | ||
| 47.79 ± 0.32 [11] | −1.29 | ||||||
| NiO (s) | 1.91 | 36.60 (cubic) [31] | 42.10 (cubic) [31] | 71.3 ± 0.8 [11] | 34.7 | 35.73 ± 0.95 [11] | −0.87 |
| 72.08 ± 0.57 [11] | 29.98 | 42.77 ± 0.35 [32] | 0.67 | ||||
| SnO2 (s) | 1.96 | 49.52 (tetragon) [31] | 57.78 (tetragon) [31] | 50.05 ± 0.21 [11] | 0.27 | ||
| 73.07 ± 0.86 [11] | 7.65 | ||||||
| GeO2 (s, quartz) | 2.01 | 44.76 [30] | 52.29 [30] | 25.06 ± 0.29 [11] | −9.85 | ||
| 36.7 ± 0.3 [11] | −7.80 | 82.11 ± 0.86 [11] | 14.91 | ||||
| Bi2O3 (s) | 2.02 | 84.23 (s) [31] | 96.89 (s) [31] | 5.21 ± 0.53 [11] | −26.34 | ||
| B2O3 | 2.04 | 94.06 (l) [28] | 107.03(l) [28] | 46.75 ± 1.52 [29] | −15.77 | ||
| TeO2 | 2.1 | 48.84 (s) [39] | 88.37 (l) [39] | ||||
| 90.89 ± 1.67 [40] | 1.26 | ||||||
| MoO3 (s) | 2.16 | 60.96 (s) [28] | 71.56 (s) [28] | 76.21± 1.47 [11] | 5.08 | ||
| P2O5 (s) | 2.19 | 115.00 [28] | −326.48 ± 1.21 [11] | −88.30 | −164.60 ± 0.85 [11] | −55.92 | |
| PbO (red, tetragon) | 2.33 | 36.86 [28] | 42.88 [28] | −15.39 ± 1.14 [11] | −52.25 | ||
| 52.07 ± 0.43 [11] | 9.19 | ||||||
| WO3 (s) | 2.36 | 62.15 (orthorhomb) [28] | 73.61 (tetragon) [28] | 91.68 ± 1.34 [11] | 9.84 | ||
| 105.78 ± 0.87 [32] | 10.73 | ||||||
| SO3(g) | 2.58 | 45.43 [28] | 53.11 [28] | −203.7 ± 4.091 [11] | −83.04 | ||
| −211.95 ± 3.55 [11] | −88.35 | ||||||
| Oxide | Electro-Negativity (Pauling) [1] | Enthalpy of Solution in Lithium–Sodium Borate (LiBO2–NaBO2 Eutectic) (800 °C) | Enthalpy of Solution in CMAS with 35 mol.% Silica (1450 °C) [46] | Enthalpy of Solution in CMAS with 42 mol.% Silica (1450°C) [47] |
|---|---|---|---|---|
| BaO | 0.89 | −87.94 [48] | ||
| SrO | 0.95 | −59.15 [48] | ||
| CaO | 1 | −23.39 [48] | −4.30 | −185.48 |
| Gd2O3 | 1.2 | −20.12 | ||
| Y2O3 | 1.22 | −3.03 | ||
| MgO | 1.31 | 25.94 [49] | 56.70 | −2.07 |
| ZrO2 | 1.33 | 22.47 [48] | −6.07 | |
| TiO2 | 1.54 | 19.595 [48] | ||
| Al2O3 | 1.61 | 17.53 [50] | 13.87 | |
| CdO | 1.69 | 36.99 [48] | ||
| SiO2 | 1.90 | −6.065 [49] | −10.20 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Navrotsky, A.; Koryttseva, A. Acid–Base Properties of Oxides Derived from Oxide Melt Solution Calorimetry. Molecules 2023, 28, 4623. https://doi.org/10.3390/molecules28124623
Navrotsky A, Koryttseva A. Acid–Base Properties of Oxides Derived from Oxide Melt Solution Calorimetry. Molecules. 2023; 28(12):4623. https://doi.org/10.3390/molecules28124623
Chicago/Turabian StyleNavrotsky, Alexandra, and Anastasia Koryttseva. 2023. "Acid–Base Properties of Oxides Derived from Oxide Melt Solution Calorimetry" Molecules 28, no. 12: 4623. https://doi.org/10.3390/molecules28124623
APA StyleNavrotsky, A., & Koryttseva, A. (2023). Acid–Base Properties of Oxides Derived from Oxide Melt Solution Calorimetry. Molecules, 28(12), 4623. https://doi.org/10.3390/molecules28124623






