Optimizing Thermoelectric Performance of Tellurium via Doping with Antimony and Selenium
Abstract
:1. Introduction
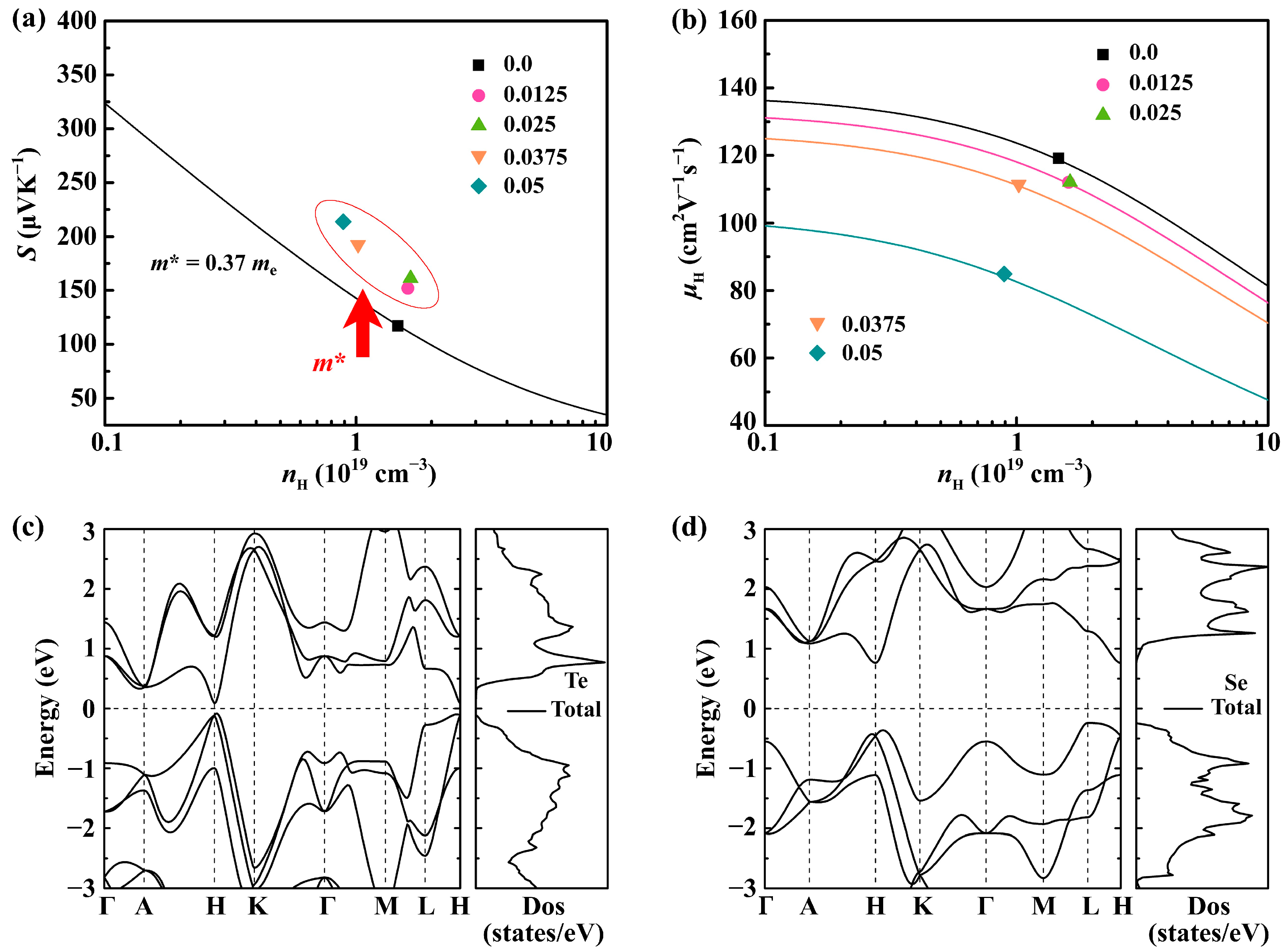
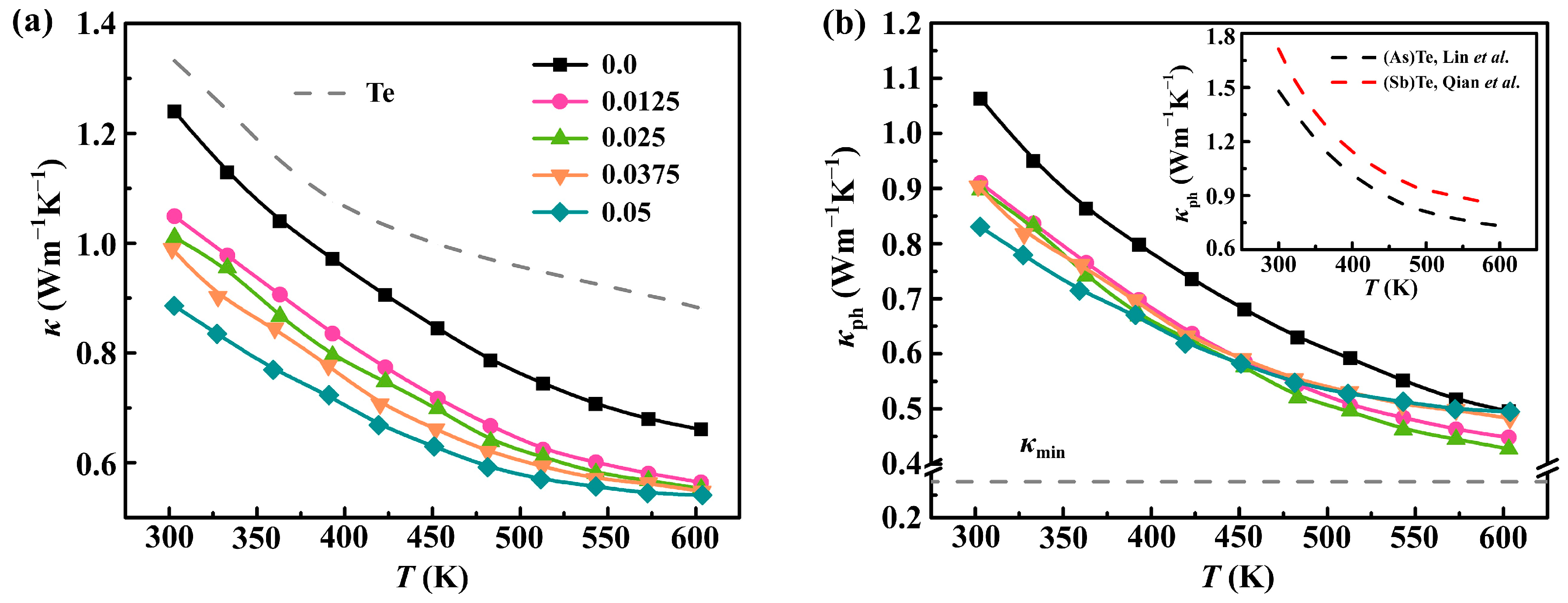
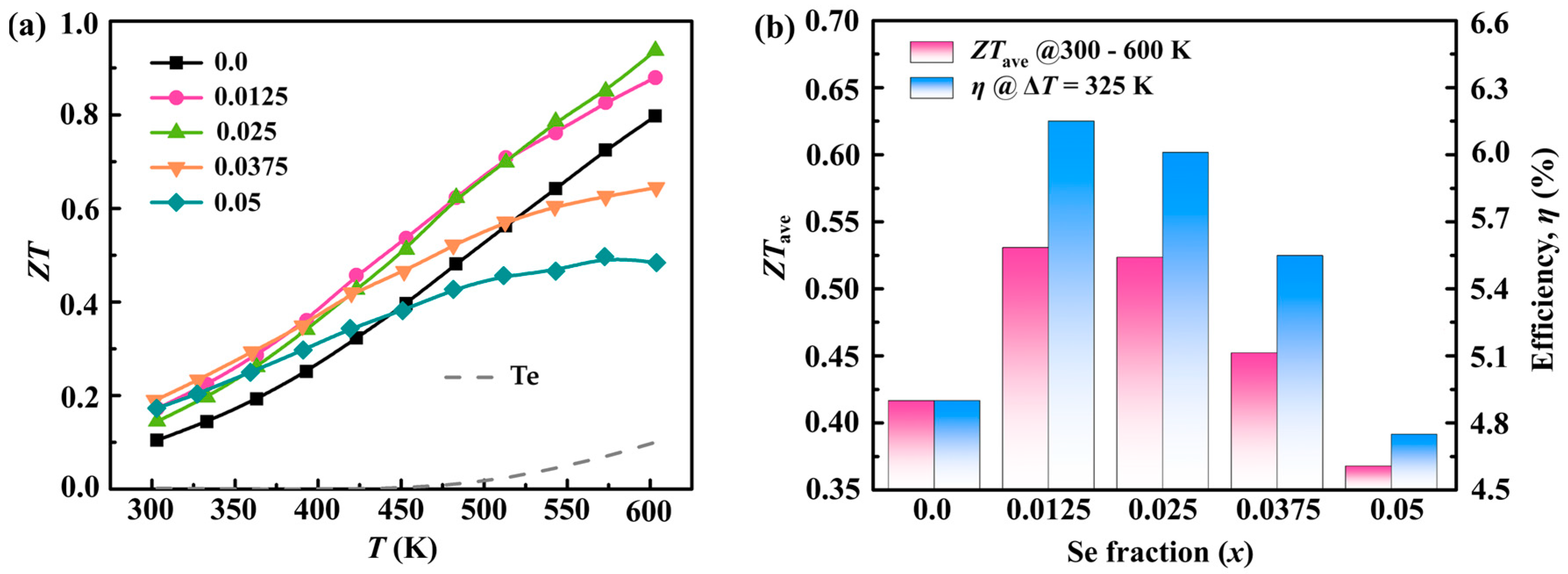
2. Results
3. Experimental Procedure
3.1. Sample Preparation
3.2. Physical Measurements
3.3. Computational Method
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- He, R.; Zhu, H.; Sun, J.; Mao, J.; Reith, H.; Chen, S.; Schierning, G.; Nielsch, K.; Ren, Z. Improved thermoelectric performance of n-type half-heusler MCo1−xNixSb (M = Hf, Zr). Mater. Today Phys. 2017, 1, 24–30. [Google Scholar] [CrossRef]
- Sun, J.; Shuai, J.; Ren, Z.; Singh, D. Computational modelling of the thermoelectric properties of p-type zintl compound CaMg2Bi2. Mater. Today Phys. 2017, 2, 40–45. [Google Scholar] [CrossRef]
- He, J.; Terry, M.T. Advances in thermoelectric materials research: Looking back and moving forward. Science 2017, 357, eaak9997. [Google Scholar] [CrossRef]
- Olabi, A.G.; Elsaid, K.; Sayed, E.T.; Mahmoud, M.S.; Wilberforce, T.; Hassiba, R.J.; Abdelkareem, M.A. Application of nanofluids for enhanced waste heat recovery: A review. Nano Energy 2021, 84, 105871. [Google Scholar] [CrossRef]
- Luo, D.; Sun, Z.; Wang, R. Performance investigation of a thermoelectric generator system applied in automobile exhaust waste heat recovery. Energy 2022, 238, 121816. [Google Scholar] [CrossRef]
- Sootsman, J.R.; Chung, D.Y.; Kanatzidis, M.G. New and old concepts in thermoelectric materials. Angew. Chem. Int. Ed. 2009, 48, 8616–8639. [Google Scholar] [CrossRef]
- Kanatzidis, M.G. Nanostructured thermoelectrics: The new paradigm?†. Chem. Mater. 2010, 22, 648–659. [Google Scholar] [CrossRef]
- Yang, M.; Li, X.; Duan, S.; Zhang, X.; Sun, H.; Chen, X.; Su, T.; Gu, L.; Liu, X. Superior Thermoelectric performance of black phosphorus in elemental tellurium. Adv. Energy Mater. 2022, 12, 2203014. [Google Scholar] [CrossRef]
- Liu, Z.; Wang, Y.; Yang, T.; Ma, Z.; Zhang, H.; Li, H.; Xia, A. Alloying engineering for thermoelectric performance enhancement in p-type skutterudites with synergistic carrier concentration optimization and thermal conductivity reduction. J. Adv. Ceram. 2023, 12, 539–552. [Google Scholar] [CrossRef]
- Fan, J.; Schnelle, W.; Antonyshyn, I.; Veremchuk, I.; Carrillo-Cabrera, W.; Shi, X.; Grin, Y.; Chen, L.-D. Structural evolvement and thermoelectric properties of Cu3−xSnxSe3 compounds with diamond-like crystal structure. Dalton Trans. 2014, 43, 16788–16794. [Google Scholar] [CrossRef]
- Liu, Z.; Gao, W.; Guo, F.; Cai, W.; Zhang, Q.; Sui, J. Challenges for thermoelectric power generation: From a material perspective. Mater. Lab. 2022, 1, 220003. [Google Scholar] [CrossRef]
- Pei, Y.; Zheng, L.; Li, W.; Lin, S.; Chen, Z.; Wang, Y.; Xu, X.; Yu, H.; Chen, Y.; Ge, B. Interstitial point defect scattering contributing to high thermoelectric performance in SnTe. Adv. Electron.Mater. 2016, 2, 1600019. [Google Scholar] [CrossRef]
- Qin, B.; Wang, D.; Zhao, L.-D. Slowing down the heat in thermoelectrics. InfoMat 2021, 3, 755–789. [Google Scholar] [CrossRef]
- Zhang, Q.; Liao, B.; Lan, Y.; Lukas, K.; Liu, W.; Esfarjani, K.; Opeil, C.; Broido, D.; Chen, G.; Ren, Z. High thermoelectric performance by resonant dopant indium in nanostructured SnTe. Proc. Natl. Acad. Sci. USA 2013, 110, 13261–13266. [Google Scholar] [CrossRef]
- Tan, G.; Zhao, L.; Shi, F.; Doak, J.W.; Lo, S.-H.; Sun, H.; Wolverton, C.; Dravid, V.P.; Uher, C.; Kanatzidis, M.G. High thermoelectric performance of p-type SnTe via a synergistic band engineering and nanostructuring approach. J. Am. Chem. Soc. 2014, 13, 7006–7017. [Google Scholar] [CrossRef] [PubMed]
- Nolas, G.S.; Cohn, J.L.; Slack, G.A.; Schujman, S.B. Semiconducting Ge clathrates: Promising candidates for thermoelectric applications. Appl. Phys. Lett. 1998, 73, 178–180. [Google Scholar] [CrossRef]
- Liu, W.; Yin, K.; Su, X.; Li, H.; Yan, Y.; Tang, X.; Uher, C. Enhanced hole concentration through Ga doping and excess of Mg and thermoelectric properties of p-type Mg2(1+z)(Si0.3Sn0.7)1−yGay. Intermetallics 2013, 32, 352–361. [Google Scholar] [CrossRef]
- Fulkerson, W.; Moore, J.P.; Williams, R.K.; Graves, R.S.; McElroy, D.L. Thermal conductivity, electrical resistivity, and Seebeck coefficient of silicon from 100 to 1300 K. Phys. Rev. 1968, 167, 765–782. [Google Scholar] [CrossRef]
- Dismukes, J.P.; Ekstrom, L.; Steigmeier, E.F.; Kudman, I.; Beers, D.S. Thermal and electrical properties of heavily doped Ge-Si alloys up to 1300 K. J. Appl. Phys. 1964, 35, 2899–2907. [Google Scholar] [CrossRef]
- Yim, W.M.; Amith, A. Bi-Sb alloys for magneto-thermoelectric and thermomagnetic cooling. Solid State Electron. 1972, 15, 1141–1165. [Google Scholar] [CrossRef]
- Zhu, B.; Liu, X.; Wang, Q.; Qiu, Y.; Shu, Z.; Guo, Z.; Tong, Y.; Cui, J.; Gu, M.; He, J. Realizing record high performance in n-type Bi2Te3-based thermoelectric materials. Energy Environ. Sci. 2020, 13, 2106–2114. [Google Scholar] [CrossRef]
- Meroz, O.; Ben-Ayoun, D.; Beeri, O.; Gelbstein, Y. Development of Bi2Te2.4Se0.6 alloy for thermoelectric power generation applications. J. Alloys Compd. 2016, 679, 196–201. [Google Scholar] [CrossRef]
- Ananya, B.; Shenoy, U.S.; Anand, S.; Waghmare, U.V.; Kanishka, B. Mg alloying in SnTe facilitates valence band convergence and optimizes thermoelectric properties. Chem. Mater. 2015, 27, 581–587. [Google Scholar]
- Kim, C.E.; Soon, A.; Stampfl, C. Unraveling the origins of conduction band valley degeneracies in Mg2Si1−xSnx thermoelectrics. Phys. Chem. Chem. Phys. 2016, 18, 939–946. [Google Scholar] [CrossRef] [PubMed]
- Lai, H.; Peng, Y.; Wang, M.; Shi, R.; Chen, J.; Liu, C.; Wang, Y.; Miao, L.; Wei, H. Thermoelectric enhancement of p-type Si80Ge20 alloy via co-compositing of dual oxides: Respective regulation for power factor and thermal conductivity by β-Ga2O3 and SiO2 aerogel powders. J. Adv. Ceram. 2023, 012, 228–241. [Google Scholar] [CrossRef]
- Fu, C.; Zhu, T.; Pei, Y.; Xie, H.; Wang, H.; Snyder, G.J.; Liu, Y.; Liu, Y.; Zhao, X. High band degeneracy contributes to high thermoelectric performance in p-type half-heusler compounds. Adv. Energy Mater. 2014, 4, 1400600. [Google Scholar] [CrossRef]
- Rani, B.; Wani, A.F.; Sharopov, U.B.; Patra, L.; Singh, J.; Ali, A.M.; Abd El-Rehim, A.F.; Khandy, S.A.; Dhiman, S.; Kaur, K. Effective mass-related thermoelectric properties of PdXSn (X = Zr, Hf) half heuslers. Molecules 2022, 27, 6567. [Google Scholar] [CrossRef]
- Liu, Y.; Zhi, J.; Li, W.; Yang, Q.; Zhang, L. Oxide materials for thermoelectric conversion. Molecules 2023, 28, 5894. [Google Scholar] [CrossRef]
- Peng, H.; Kioussis, N.; Snyder, G.J. Elemental tellurium as a chiral p-type thermoelectric material. Phys. Rev. B 2014, 89, 195206. [Google Scholar] [CrossRef]
- Lin, S.; Li, W.; Chen, Z.; Shen, J.; Ge, B.; Pei, Y. Tellurium as a high-performance elemental thermoelectric. Nat. Commun. 2016, 7, 10287. [Google Scholar] [CrossRef]
- Yang, M.; Su, T.; Zhu, H.; Li, S.; Hu, M.; Hu, Q.; Ma, H.; Jia, X. Thermoelectric performance of Te doped with As and alloyed with Se. J. Mater. Sci. 2018, 53, 11524–11533. [Google Scholar] [CrossRef]
- Lin, S.; Li, W.; Zhang, X.; Li, J.; Chen, Z.; Pei, Y. Sb induces both doping and precipitation for improving the thermoelectric performance of elemental Te. Inorg. Chem. Front. 2017, 4, 1066–1072. [Google Scholar] [CrossRef]
- Yang, M.; Su, T.; Zhou, D.; Zhu, H.; Li, S.; Hu, M.; Hu, Q.; Ma, H.; Jia, X. High-pressure synthesis and thermoelectric performance of tellurium doped with bismuth. J. Mater. Sci. 2017, 52, 10526–10532. [Google Scholar] [CrossRef]
- Yang, M.; Zhu, H.; Yi, W.; Li, S.; Hu, M.; Hu, Q.; Du, B.; Liu, X.; Su, T. Electrical transport and thermoelectric properties of Te-Se solid solutions. Phys. Lett. A 2019, 383, 2615–2620. [Google Scholar] [CrossRef]
- Yang, M.; Su, T.; Li, S.; Li, S.; Hu, M.; Liu, X. Facile synthesis and high thermoelectric performance of tellurium with antimony doping. J. Alloys Compd. 2021, 887, 161342. [Google Scholar] [CrossRef]
- Wu, Y.; Zhang, Q.; Liu, F.; Fang, T.; Zhu, T.; Zhao, X. Scattering mechanisms and compositional optimization of high performance elemental Te as a thermoelectric material. Adv. Electron Mater. 2020, 6, 202000038. [Google Scholar] [CrossRef]
- Saparamadu, U.; Li, C.; He, R.; Zhu, H.; Ren, Z.; Mao, J.; Song, S.; Sun, J.; Chen, S.; Zhang, Q.; et al. Improved thermoelectric performance of Tellurium by alloying with a small concentration of selenium to decrease lattice thermal conductivity. ACS Appl. Mater. Interfaces 2019, 11, 511–516. [Google Scholar] [CrossRef]
- Zhu, J.; Zhang, X.; Guo, M.; Li, J.; Hu, J.; Cai, S.; Cai, W.; Zhang, Y.; Sui, J. Restructured single parabolic band model for quick analysis in thermoelectricity. npj Comput. Mater. 2021, 7, 116. [Google Scholar] [CrossRef]
- Kim, H.S.; Gibbs, Z.M.; Tang, Y.; Wang, H.; Snyder, G.J. Characterization of lorenz number with Seebeck coefficient measurement. APL Mater. 2015, 3, 041506. [Google Scholar] [CrossRef]
- Cahill, D.G.; Pohl, R.O. Heat flow and lattice vibrations in glasses. Solid State Commun. 1989, 70, 927–930. [Google Scholar] [CrossRef]
- Qian, X.; Xiao, Y.; Zheng, L.; Qin, B.; Zhou, Y.; Pei, Y.; Yuan, B.; Gong, S.; Zhao, L.-D. Effective dopants in p-type elementary Te thermoelectrics. RSC Adv. 2017, 7, 17682–17688. [Google Scholar] [CrossRef]
- Nandihalli, N.; Wijethunge, D.; Kim, K.; Kim, J.; Gayner, C. High efficient nanostructured PbSe0.5Te0.5 exhibiting broad figure-of-merit plateau. J. Alloys Compd. 2019, 785, 862–870. [Google Scholar] [CrossRef]
- Kresse, G.G.; Furthmüller, J.J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1997, 78, 3865. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, M.; Yang, M.; Li, Y.; Chen, Y.; Song, Y.; Jia, J.; Su, T. Optimizing Thermoelectric Performance of Tellurium via Doping with Antimony and Selenium. Molecules 2023, 28, 7287. https://doi.org/10.3390/molecules28217287
Yang M, Yang M, Li Y, Chen Y, Song Y, Jia J, Su T. Optimizing Thermoelectric Performance of Tellurium via Doping with Antimony and Selenium. Molecules. 2023; 28(21):7287. https://doi.org/10.3390/molecules28217287
Chicago/Turabian StyleYang, Manman, Mengxiang Yang, Yimin Li, Yuqi Chen, Yuling Song, Jin Jia, and Taichao Su. 2023. "Optimizing Thermoelectric Performance of Tellurium via Doping with Antimony and Selenium" Molecules 28, no. 21: 7287. https://doi.org/10.3390/molecules28217287
APA StyleYang, M., Yang, M., Li, Y., Chen, Y., Song, Y., Jia, J., & Su, T. (2023). Optimizing Thermoelectric Performance of Tellurium via Doping with Antimony and Selenium. Molecules, 28(21), 7287. https://doi.org/10.3390/molecules28217287








