Promising M2CO2/MoX2 (M = Hf, Zr; X = S, Se, Te) Heterostructures for Multifunctional Solar Energy Applications
Abstract
:1. Introduction
2. Experimental Section
2.1. Computational Details
2.2. Data Analysis
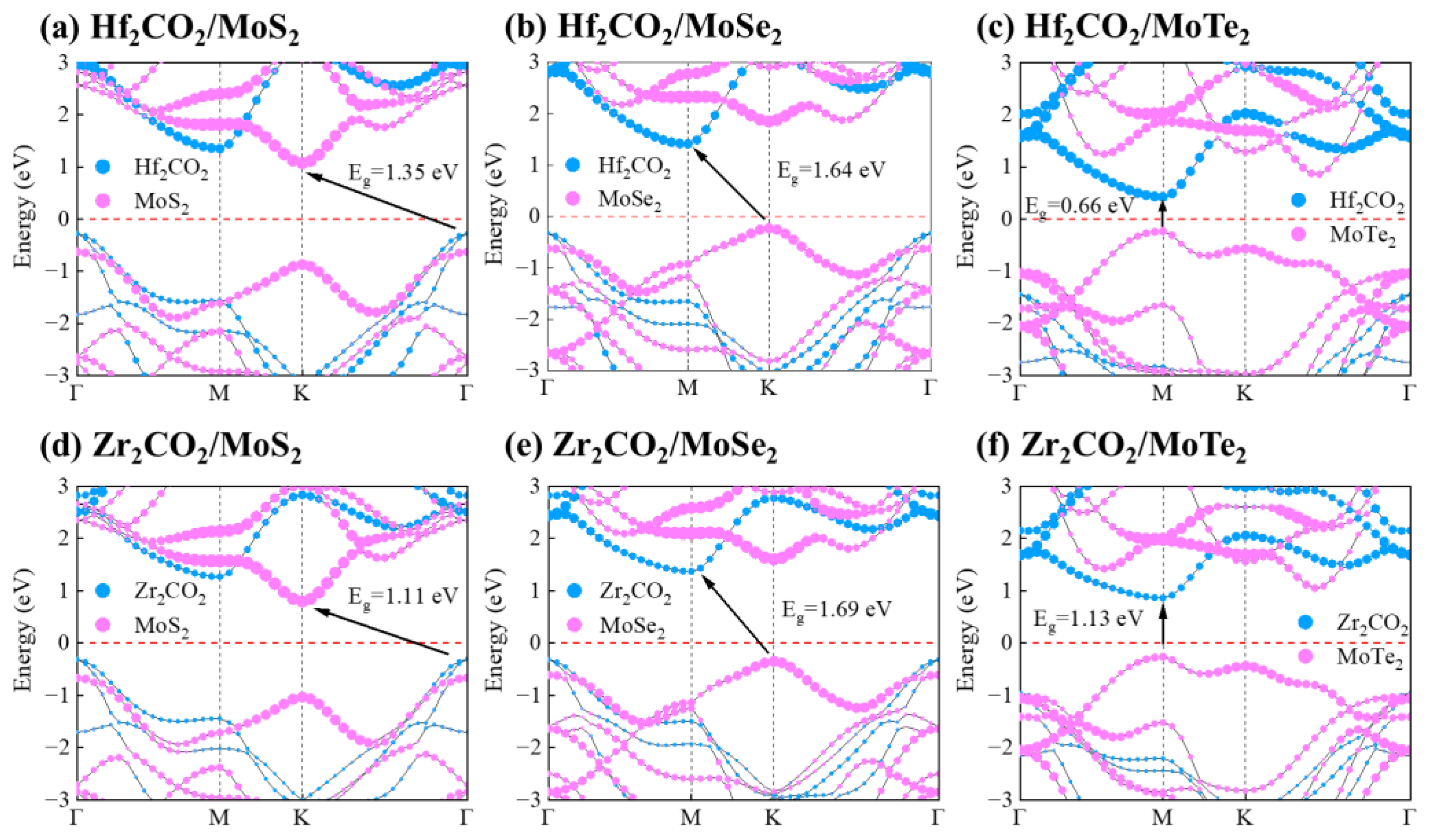
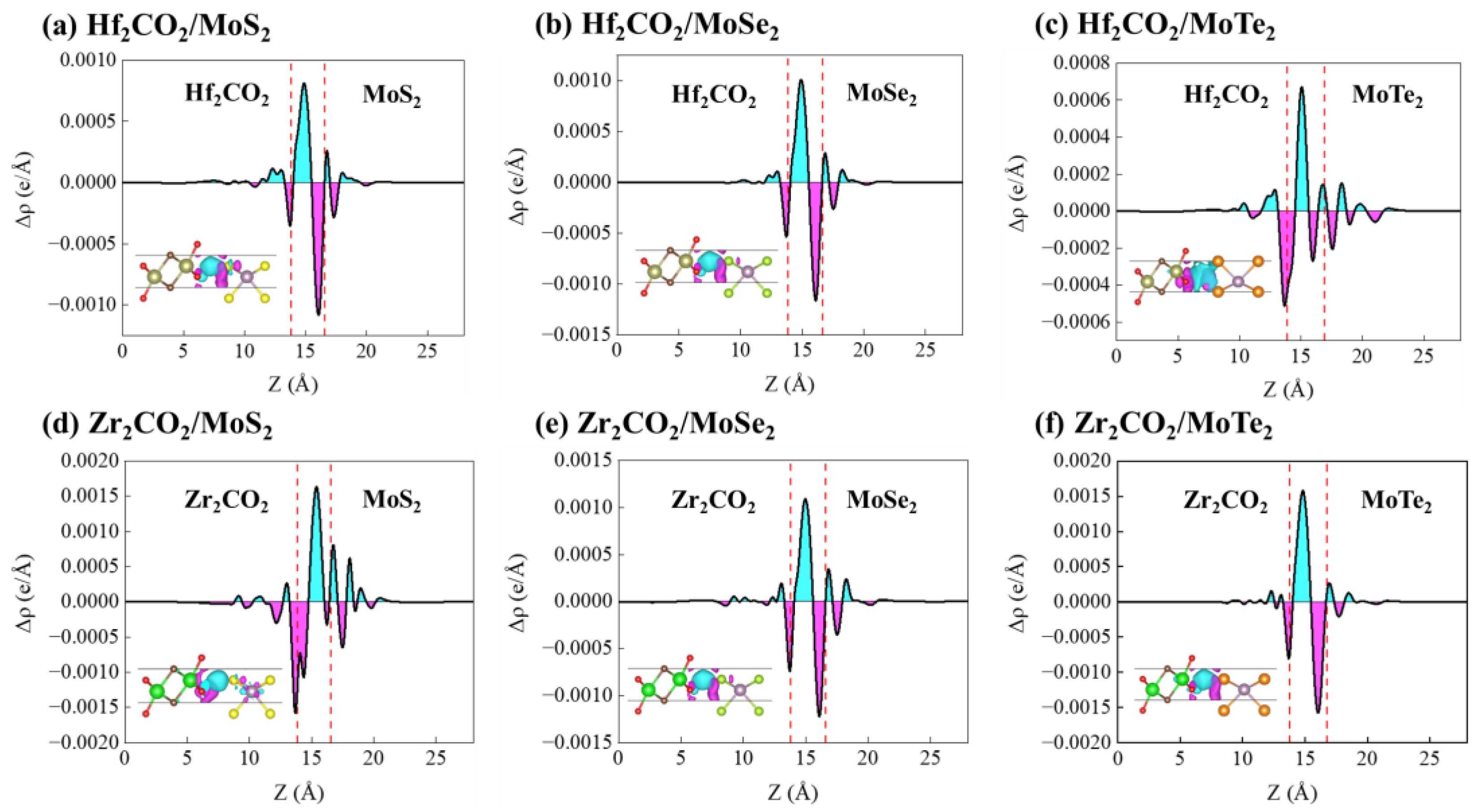
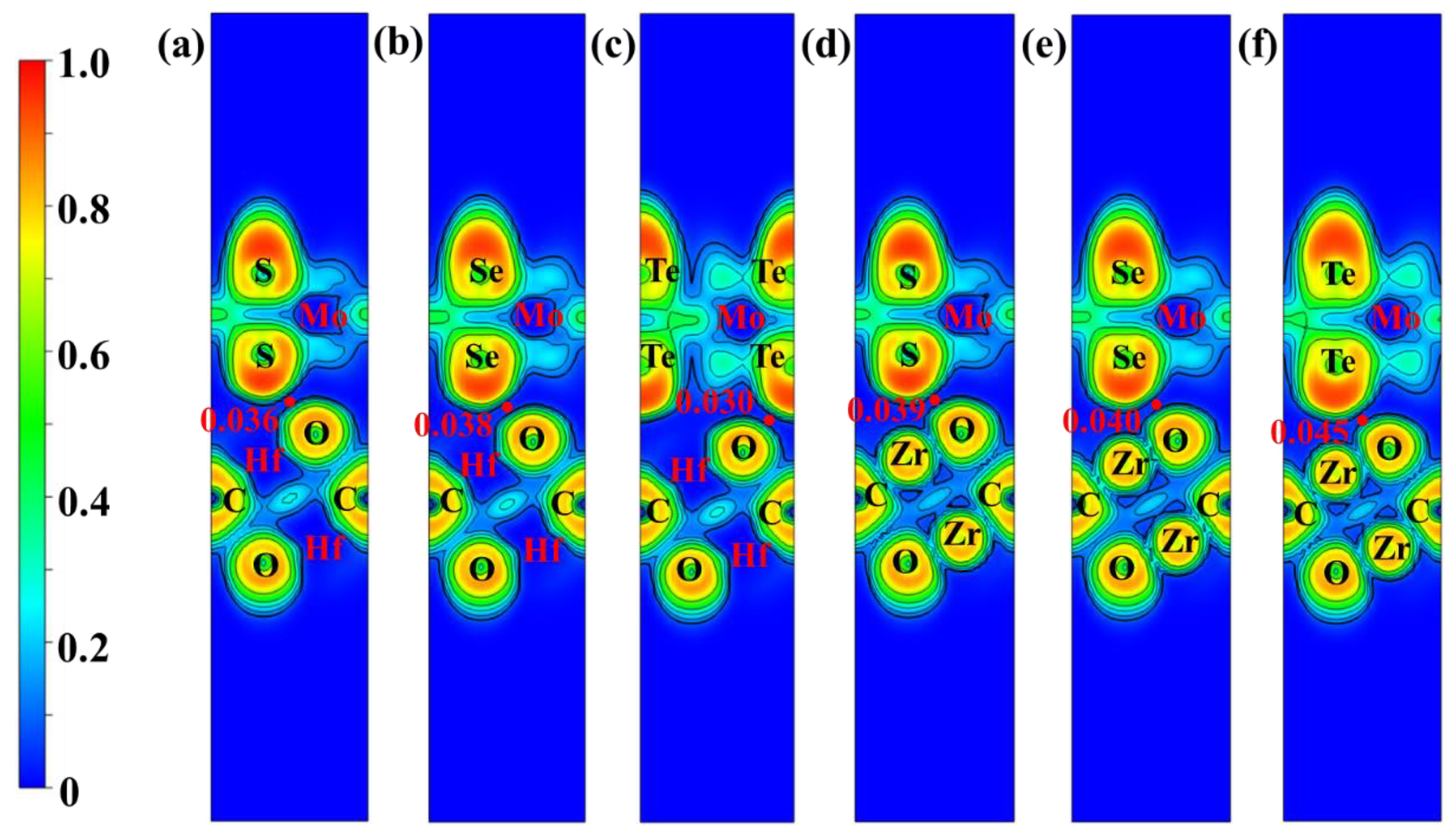
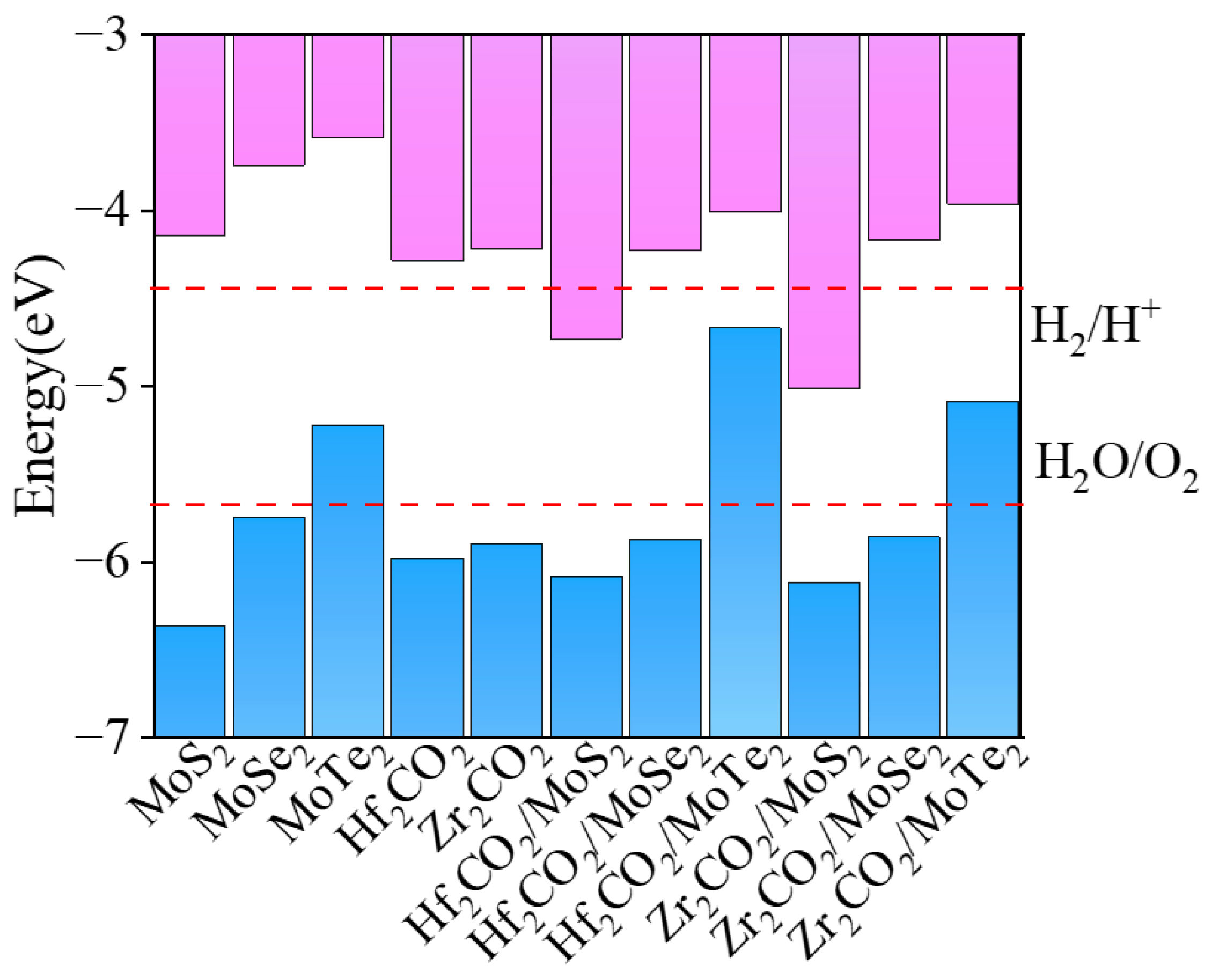
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Khan, K.; Tareen, A.K.; Aslam, M.; Sagar, R.U.R.; Zhang, B.; Huang, W.; Mahmood, A.; Mahmood, N.; Khan, K.; Zhang, H.; et al. Recent progress, challenges, and prospects in Two-Dimensional Photo-Catalyst materials and environmental remediation. Nano-Micro Lett. 2020, 12, 167. [Google Scholar] [CrossRef]
- Luo, B.; Liu, G.; Wang, L. Recent advances in 2D materials for photocatalysis. Nanoscale 2016, 8, 6904–6920. [Google Scholar] [CrossRef] [PubMed]
- Lewis, N.S.; Nocera, D.G. Powering the planet: Chemical challenges in solar energy utilization. Proc. Natl. Acad. Sci. USA 2006, 103, 15729–15735. [Google Scholar] [CrossRef]
- Ginley, D.; Green, M.A.; Collins, R. Solar Energy Conversion Toward 1 Terawatt. MRS Bull. 2008, 33, 355–364. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Katsnelson, M.I.; Grigorieva, I.V.; Dubonos, S.V.; Firsov, A.A. Two-dimensional gas of massless Dirac fermions in graphene. Nature 2005, 438, 197–200. [Google Scholar] [CrossRef]
- Geim, A.K.; Novoselov, K.S. The rise of graphene. Nat. Mater. 2007, 6, 183–191. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Fal Ko, V.I.; Colombo, L.; Gellert, P.R.; Schwab, M.G.; Kim, K. A roadmap for graphene. Nature 2012, 490, 192–200. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric field effect in atomically thin carbon films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [PubMed]
- Shein, I.R.; Ivanovskii, A.L. Graphene-like nanocarbides and nanonitrides of d metals (MXenes): Synthesis, properties and simulation. Micro Nano Lett. 2013, 8, 59–62. [Google Scholar] [CrossRef]
- Anasori, B.; Lukatskaya, M.R.; Gogotsi, Y. 2D metal carbides and nitrides (MXenes) for energy storage. Nat. Rev. Mater. 2017, 2, 16098. [Google Scholar] [CrossRef]
- Fu, H.; Ramalingam, V.; Kim, H.; Lin, C.; Fang, X.; Alshareef, H.N.; He, J. MXene-Contacted Silicon Solar Cells with 11.5% Efficiency. Adv. Energy Mater. 2019, 9, 1900180. [Google Scholar] [CrossRef]
- Li, X.; Wang, J.; Fang, Y.; Zhang, H.; Fu, X.; Wang, X. Roles of Metal-Free materials in photoelectrodes for water splitting. Acc. Mater. Res. 2021, 2, 933–943. [Google Scholar] [CrossRef]
- Coleman, J.N.; Lotya, M.; O Neill, A.; Bergin, S.D.; King, P.J.; Khan, U.; Young, K.; Gaucher, A.; De, S.; Smith, R.J.; et al. Two-Dimensional nanosheets produced by liquid exfoliation of layered materials. Science 2011, 331, 568–571. [Google Scholar] [CrossRef] [PubMed]
- Chhowalla, M.; Shin, H.S.; Eda, G.; Li, L.; Loh, K.P.; Zhang, H. The chemistry of two-dimensional layered transition metal dichalcogenide nanosheets. Nat. Chem. 2013, 5, 263–275. [Google Scholar] [CrossRef]
- Sun, H.; Yan, Z.; Liu, F.; Xu, W.; Cheng, F.; Chen, J. Self-Supported Transition-Metal-Based electrocatalysts for hydrogen and oxygen evolution. Adv. Mater. 2020, 32, 1806326. [Google Scholar] [CrossRef]
- Yang, Y.; Gao, J.; Zhang, Z.; Xiao, S.; Xie, H.; Sun, Z.; Wang, J.; Zhou, C.; Wang, Y.; Guo, X.; et al. Black phosphorus based photocathodes in wideband bifacial Dye-Sensitized solar cells. Adv. Mater. 2016, 28, 8937–8944. [Google Scholar] [CrossRef] [PubMed]
- Zhao, J.; Liu, H.; Yu, Z.; Quhe, R.; Zhou, S.; Wang, Y.; Liu, C.C.; Zhong, H.; Han, N.; Lu, J.; et al. Rise of silicene: A competitive 2D material. Prog. Mater. Sci. 2016, 83, 24–151. [Google Scholar] [CrossRef]
- Naguib, M.; Kurtoglu, M.; Presser, V.; Lu, J.; Niu, J.; Heon, M.; Hultman, L.; Gogotsi, Y.; Barsoum, M.W. Two-Dimensional nanocrystals produced by exfoliation of Ti3AlC2. Adv. Mater. 2011, 23, 4248–4253. [Google Scholar] [CrossRef]
- Naguib, M.; Mashtalir, O.; Carle, J.; Presser, V.; Lu, J.; Hultman, L.; Gogotsi, Y.; Barsoum, M.W. Two-Dimensional transition metal carbides. ACS Nano 2012, 6, 1322–1331. [Google Scholar] [CrossRef] [PubMed]
- Gan, J.; Li, F.; Tang, Q. Vacancies-Engineered M2CO2 MXene as an efficient hydrogen evolution reaction electrocatalyst. J. Phys. Chem. Lett. 2021, 12, 4805–4813. [Google Scholar] [CrossRef]
- Khazaei, M.; Arai, M.; Sasaki, T.; Chung, C.; Venkataramanan, N.S.; Estili, M.; Sakka, Y.; Kawazoe, Y. Novel electronic and magnetic properties of Two-Dimensional transition metal carbides and nitrides. Adv. Funct. Mater. 2013, 23, 2185–2192. [Google Scholar] [CrossRef]
- Khazaei, M.; Ranjbar, A.; Arai, M.; Sasaki, T.; Yunoki, S. Electronic properties and applications of MXenes: A theoretical review. J. Mater. Chem. C 2017, 5, 2488–2503. [Google Scholar] [CrossRef]
- Guo, Z.; Zhou, J.; Zhu, L.; Sun, Z. MXene: A promising photocatalyst for water splitting. J. Mater. Chem. A 2016, 4, 11446–11452. [Google Scholar] [CrossRef]
- Zhang, Y.; Xiong, R.; Sa, B.; Zhou, J.; Sun, Z. MXenes: Promising donor and acceptor materials for high-efficiency heterostructure solar cells. Sustain. Energy Fuels 2021, 5, 135–143. [Google Scholar] [CrossRef]
- Ghidiu, M.; Lukatskaya, M.R.; Zhao, M.; Gogotsi, Y.; Barsoum, M.W. Conductive two-dimensional titanium carbide ‘clay’ with high volumetric capacitance. Nature 2014, 516, 78–81. [Google Scholar] [CrossRef] [PubMed]
- Seh, Z.W.; Fredrickson, K.D.; Anasori, B.; Kibsgaard, J.; Strickler, A.L.; Lukatskaya, M.R.; Gogotsi, Y.; Jaramillo, T.F.; Vojvodic, A. Two-Dimensional molybdenum carbide (MXene) as an efficient electrocatalyst for hydrogen evolution. ACS Energy Lett. 2016, 1, 589–594. [Google Scholar] [CrossRef]
- Withers, F.; Del Pozo-Zamudio, O.; Schwarz, S.; Dufferwiel, S.; Walker, P.M.; Godde, T.; Rooney, A.P.; Gholinia, A.; Woods, C.R.; Blake, P.; et al. WSe2 Light-Emitting tunneling transistors with enhanced brightness at room temperature. Nano Lett. 2015, 15, 8223–8228. [Google Scholar] [CrossRef] [PubMed]
- Schwarz, S.; Kozikov, A.; Withers, F.; Maguire, J.K.; Foster, A.P.; Dufferwiel, S.; Hague, L.; Makhonin, M.N.; Wilson, L.R.; Geim, A.K.; et al. Electrically pumped single-defect light emitters in WSe2. 2D Mater. 2016, 3, 25038. [Google Scholar] [CrossRef]
- Zheng, W.; Jiang, Y.; Hu, X.; Li, H.; Zeng, Z.; Wang, X.; Pan, A. Light emission properties of 2D transition metal dichalcogenides: Fundamentals and applications. Adv. Opt. Mater. 2018, 6, 1800420. [Google Scholar] [CrossRef]
- Wang, H.; Yuan, H.; Sae Hong, S.; Li, Y.; Cui, Y. Physical and chemical tuning of two-dimensional transition metal dichalcogenides. Chem. Soc. Rev. 2015, 44, 2664–2680. [Google Scholar] [CrossRef]
- Vante, N.A.; Jaegermann, W.; Tributsch, H.; Hoenle, W.; Yvon, K. Electrocatalysis of oxygen reduction by chalcogenides containing mixed transition metal clusters. J. Am. Chem. Soc. 1987, 109, 3251–3257. [Google Scholar] [CrossRef]
- Bag, S.; Arachchige, I.U.; Kanatzidis, M.G. Aerogels from metal chalcogenides and their emerging unique properties. J. Mater. Chem. 2008, 18, 3628–3632. [Google Scholar] [CrossRef]
- Pumera, M.; Sofer, Z.; Ambrosi, A. Layered transition metal dichalcogenides for electrochemical energy generation and storage. J. Mater. Chem. A 2014, 2, 8981–8987. [Google Scholar] [CrossRef]
- Gelly, R.J.; Renaud, D.; Liao, X.; Pingault, B.; Bogdanovic, S.; Scuri, G.; Watanabe, K.; Taniguchi, T.; Urbaszek, B.; Park, H.; et al. Probing dark exciton navigation through a local strain landscape in a WSe2 monolayer. Nat. Commun. 2022, 13, 232. [Google Scholar] [CrossRef]
- Jariwala, D.; Marks, T.J.; Hersam, M.C. Mixed-dimensional van der Waals heterostructures. Nat. Mater. 2017, 16, 170–181. [Google Scholar] [CrossRef] [PubMed]
- Rivera, P.; Seyler, K.L.; Yu, H.; Schaibley, J.R.; Yan, J.; Mandrus, D.G.; Yao, W.; Xu, X. Valley-polarized exciton dynamics in a 2D semiconductor heterostructure. Science 2016, 351, 688–691. [Google Scholar] [CrossRef] [PubMed]
- Xiong, R.; Hu, R.; Zhang, Y.; Yang, X.; Lin, P.; Wen, C.; Sa, B.; Sun, Z. Computational discovery of PtS2/GaSe van der Waals heterostructure for solar energy applications. Phys. Chem. Chem. Phys. 2021, 23, 20163–20173. [Google Scholar] [CrossRef] [PubMed]
- Xiong, R.; Shu, Y.; Yang, X.; Zhang, Y.; Wen, C.; Anpo, M.; Wu, B.; Sa, B. Direct Z-scheme WTe2/InSe van der Waals heterostructure for overall water splitting. Catal. Sci. Technol. 2022, 12, 3272–3280. [Google Scholar] [CrossRef]
- Li, N.; Zhang, Y.; Jia, M.; Lv, X.; Li, X.; Li, R.; Ding, X.; Zheng, Y.; Tao, X. 1T/2H MoSe2-on-MXene heterostructure as bifunctional electrocatalyst for efficient overall water splitting. Electrochim. Acta 2019, 326, 134976. [Google Scholar] [CrossRef]
- Xiao, W.; Yan, D.; Zhang, Y.; Yang, X.; Zhang, T. Heterostructured MoSe2/Oxygen-Terminated Ti3C2 MXene architectures for efficient electrocatalytic hydrogen evolution. Energy Fuels 2021, 35, 4609–4615. [Google Scholar] [CrossRef]
- Furthmüller, J.; Kresse, G. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef]
- Wang, G.; Peng, L.; Li, K.; Zhu, L.; Zhou, J.; Miao, N.; Sun, Z. ALKEMIE: An intelligent computational platform for accelerating materials discovery and design. Comput. Mater. Sci. 2021, 186, 110064. [Google Scholar] [CrossRef]
- Wang, G.; Li, K.; Peng, L.; Zhang, Y.; Zhou, J.; Sun, Z. High-Throughput Automatic Integrated Material Calculations and Data Management Intelligent Platform and the Application in Novel Alloys. Acta Metall. Sin. 2022, 58, 75–88. [Google Scholar]
- Joubert, D.; Kresse, G. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758–1775. [Google Scholar] [CrossRef]
- Burke, K.; Ernzerhof, M.; Perdew, J.P. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef]
- Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef]
- Grimme, S.; Ehrlich, S.; Goerigk, L. Effect of the damping function in dispersion corrected density functional theory. J. Comput. Chem. 2011, 32, 1456–1465. [Google Scholar] [CrossRef] [PubMed]
- Heyd, J.; Scuseria, G.E. Efficient hybrid density functional calculations in solids: Assessment of the Heyd–Scuseria–Ernzerhof screened Coulomb hybrid functional. J. Chem. Phys. 2004, 121, 1187–1192. [Google Scholar] [CrossRef]
- Oba, F.; Tanaka, I.; Togo, A. First-principles calculations of the ferroelastic transition between rutile-type and CaCl2-type SiO2 at high pressures. Phys. Rev. B 2008, 78, 134106. [Google Scholar] [CrossRef]
- Nosé, S. A unified formulation of the constant temperature molecular dynamics methods. J. Chem. Phys. 1984, 81, 511–519. [Google Scholar] [CrossRef]
- Hoover, W.G. Canonical dynamics: Equilibrium phase-space distributions. Phys. Rev. A 1985, 31, 1695–1697. [Google Scholar] [CrossRef]
- Washburn, J.; Chen, Y. Structural transition in Large-Lattice-Mismatch heteroepitaxy. Phys. Rev. Lett. 1996, 77, 4046–4049. [Google Scholar] [CrossRef]
- Liao, J.; Sa, B.; Zhou, J.; Ahuja, R.; Sun, Z. Design of High-Efficiency Visible-Light photocatalysts for water splitting: MoS2/AlN(GaN) heterostructures. J. Phys. Chem. C 2014, 118, 17594–17599. [Google Scholar] [CrossRef]
- Shi, G.; Kioupakis, E. Electronic and optical properties of nanoporous silicon for Solar-Cell applications. ACS Photon. 2015, 2, 208–215. [Google Scholar] [CrossRef]
- Shockley, W.; Bardeen, J. Deformation potentials and mobilities in Non-Polar crystals. Phys. Rev. 1950, 80, 72–80. [Google Scholar] [CrossRef]
- Gandi, A.N.; Alshareef, H.N.; Schwingenschlögl, U. Thermoelectric performance of the MXenes M2CO2 (M = Ti, Zr, or Hf). Chem. Mater. 2016, 28, 1647–1652. [Google Scholar] [CrossRef]
- Munawar, M.; Idrees, M.; Ahmad, I.; Din, H.U.; Amin, B. Intriguing electronic, optical and photocatalytic performance of BSe, M2CO2 monolayers and BSe–M2CO2 (M = Ti, Zr, Hf) van der Waals heterostructures. RSC Adv. 2022, 12, 42–52. [Google Scholar] [CrossRef]
- Fu, C.; Li, X.; Luo, Q.; Yang, J. Two-dimensional multilayer M2CO2 (M = Sc, Zr, Hf) as photocatalysts for hydrogen production from water splitting: A first principles study. J. Mater. Chem. A 2017, 5, 24972–24980. [Google Scholar] [CrossRef]
- Xu, X.; Ge, X.; Liu, X.; Li, L.; Fu, K.; Dong, Y.; Meng, F.; Si, R.; Zhang, M. Two-dimensional M2CO2/MoS2 (M = Ti, Zr and Hf) van der Waals heterostructures for overall water splitting: A density functional theory study. Ceram. Int. 2020, 46, 13377–13384. [Google Scholar] [CrossRef]
- Zhuang, H.L.; Hennig, R.G. Computational search for Single-Layer Transition-Metal dichalcogenide photocatalysts. J. Phys. Chem. C 2013, 117, 20440–20445. [Google Scholar] [CrossRef]
- Xu, X.; Wu, X.; Tian, Z.; Zhang, M.; Li, L.; Zhang, J. Modulating the electronic structures and potential applications of Zr2CO2/MSe2 (M = Mo, W) heterostructures by different stacking modes: A density functional theory calculation. Appl. Surf. Sci. 2022, 599, 154014. [Google Scholar] [CrossRef]
- Li, X.; Cui, X.; Xing, C.; Cui, H.; Zhang, R. Strain-tunable electronic and optical properties of Zr2CO2 MXene and MoSe2 van der Waals heterojunction: A first principles calculation. Appl. Surf. Sci. 2021, 548, 149249. [Google Scholar] [CrossRef]
- Wang, B.; Wang, X.; Wang, P.; Kuang, A.; Zhou, T.; Yuan, H.; Chen, H. Bilayer MoTe2/XS2 (X = Hf, Sn, Zr) heterostructures with efficient carrier separation and light absorption for photocatalytic water splitting into hydrogen. Appl. Surf. Sci. 2021, 544, 148842. [Google Scholar] [CrossRef]
- Chen, J.; He, X.; Sa, B.; Zhou, J.; Xu, C.; Wen, C.; Sun, Z. III–VI van der Waals heterostructures for sustainable energy related applications. Nanoscale 2019, 11, 6431–6444. [Google Scholar] [CrossRef]
- Gulans, A.; Krasheninnikov, A.V.; Nieminen, R.M.; Björkman, T. Van der Waals Bonding in Layered Compounds from Advanced Density-Functional First-Principles Calculations. Phys. Rev. Lett. 2012, 108, 235502. [Google Scholar] [CrossRef]
- Mannix, A.J.; Zhou, X.; Kiraly, B.; Wood, J.D.; Alducin, D.; Myers, B.D.; Liu, X.; Fisher, B.L.; Santiago, U.; Guest, J.R.; et al. Synthesis of borophenes: Anisotropic, two-dimensional boron polymorphs. Science 2015, 350, 1513–1516. [Google Scholar] [CrossRef]
- Penev, E.S.; Kutana, A.; Yakobson, B.I. Can Two-Dimensional boron superconduct? Nano Lett. 2016, 16, 2522–2526. [Google Scholar] [CrossRef]
- Guo, G.; Wang, D.; Wei, X.; Zhang, Q.; Liu, H.; Lau, W.; Liu, L. First-Principles study of phosphorene and graphene heterostructure as anode materials for rechargeable Li batteries. J. Phys. Chem. Lett. 2015, 6, 5002–5008. [Google Scholar] [CrossRef]
- Liu, J. Origin of high photocatalytic efficiency in monolayer g-C3N4/CdS heterostructure: A hybrid DFT study. J. Phys. Chem. C 2015, 119, 28417–28423. [Google Scholar] [CrossRef]
- Xie, M.; Zhang, S.; Cai, B.; Huang, Y.; Zou, Y.; Guo, B.; Gu, Y.; Zeng, H. A promising two-dimensional solar cell donor: Black arsenic–phosphorus monolayer with 1.54 eV direct bandgap and mobility exceeding 14,000 cm2V−1s−1. Nano Energy 2016, 28, 433–439. [Google Scholar] [CrossRef]
- Xiong, R.; Yang, H.; Peng, Q.; Sa, B.; Wen, C.; Wu, B.; Sun, Z. First-principle investigation of TcSe2 monolayer as an efficient visible light photocatalyst for water splitting hydrogen production. Res. Chem. Intermed. 2017, 43, 5271–5282. [Google Scholar] [CrossRef]
- Scharber, M.C.; Mühlbacher, D.; Koppe, M.; Denk, P.; Waldauf, C.; Heeger, A.J.; Brabec, C.J. Design rules for donors in Bulk-Heterojunction solar cells—Towards 10 % Energy-Conversion efficiency. Adv. Mater. 2006, 18, 789–794. [Google Scholar] [CrossRef]
- Loferski, J.J. Theoretical considerations governing the choice of the optimum semiconductor for photovoltaic solar energy conversion. J. Appl. Phys. 1956, 27, 777–784. [Google Scholar] [CrossRef]
- Sa, B.; Hu, R.; Zheng, Z.; Xiong, R.; Zhang, Y.; Wen, C.; Zhou, J.; Sun, Z. High-Throughput computational screening and machine learning modeling of janus 2D III–VI van der waals heterostructures for solar energy applications. Chem. Mater. 2022, 34, 6687–6701. [Google Scholar] [CrossRef]



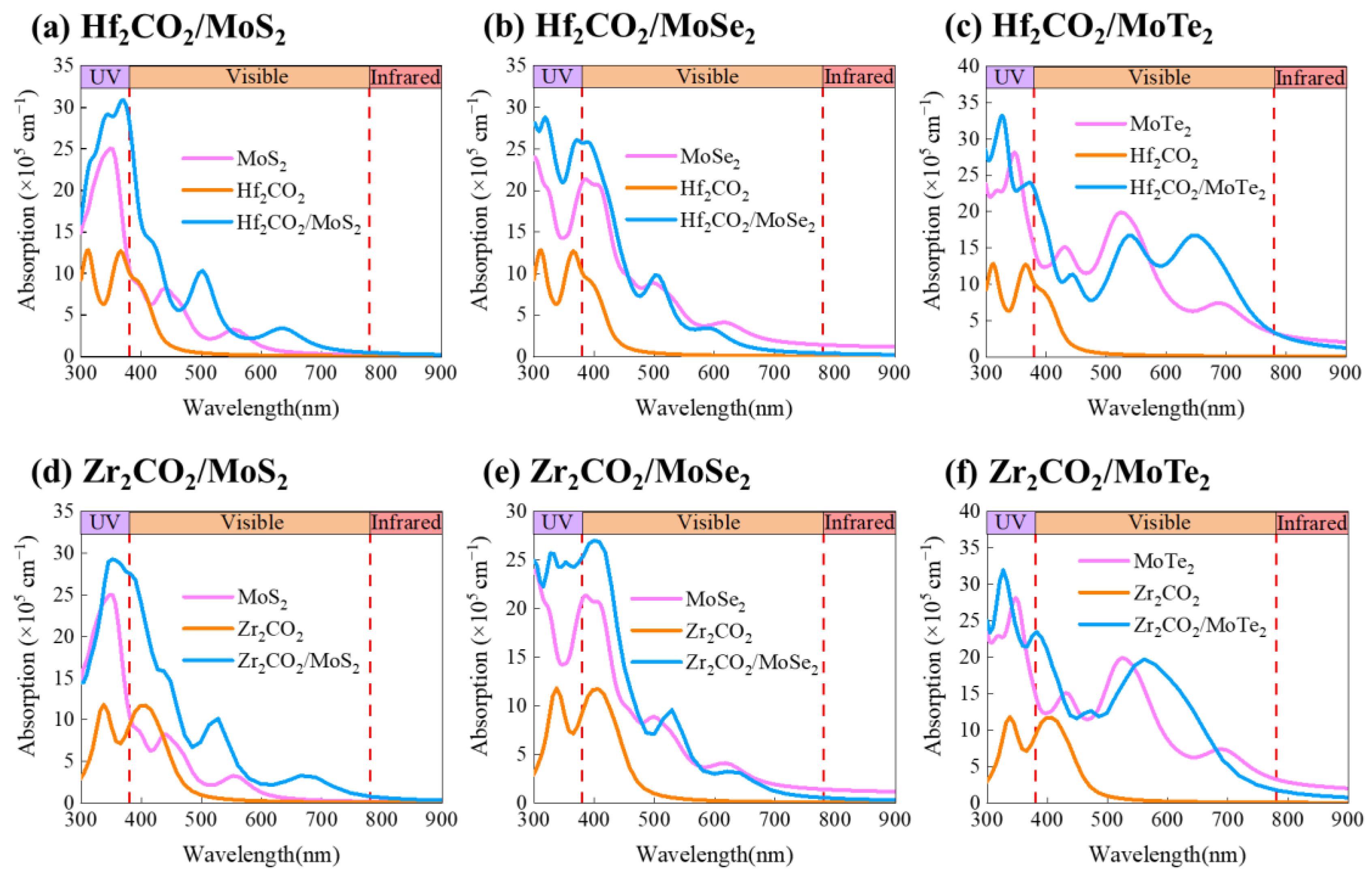
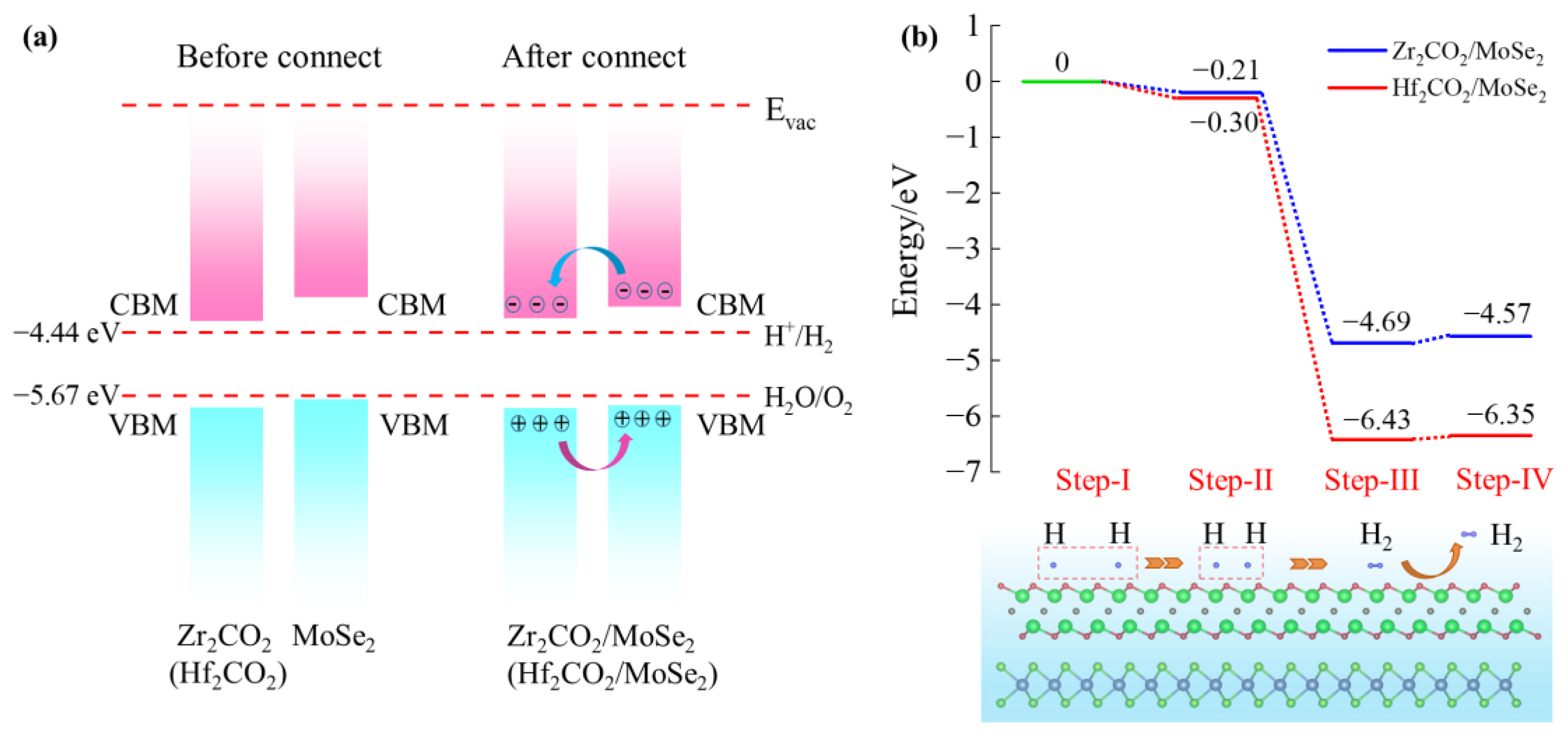
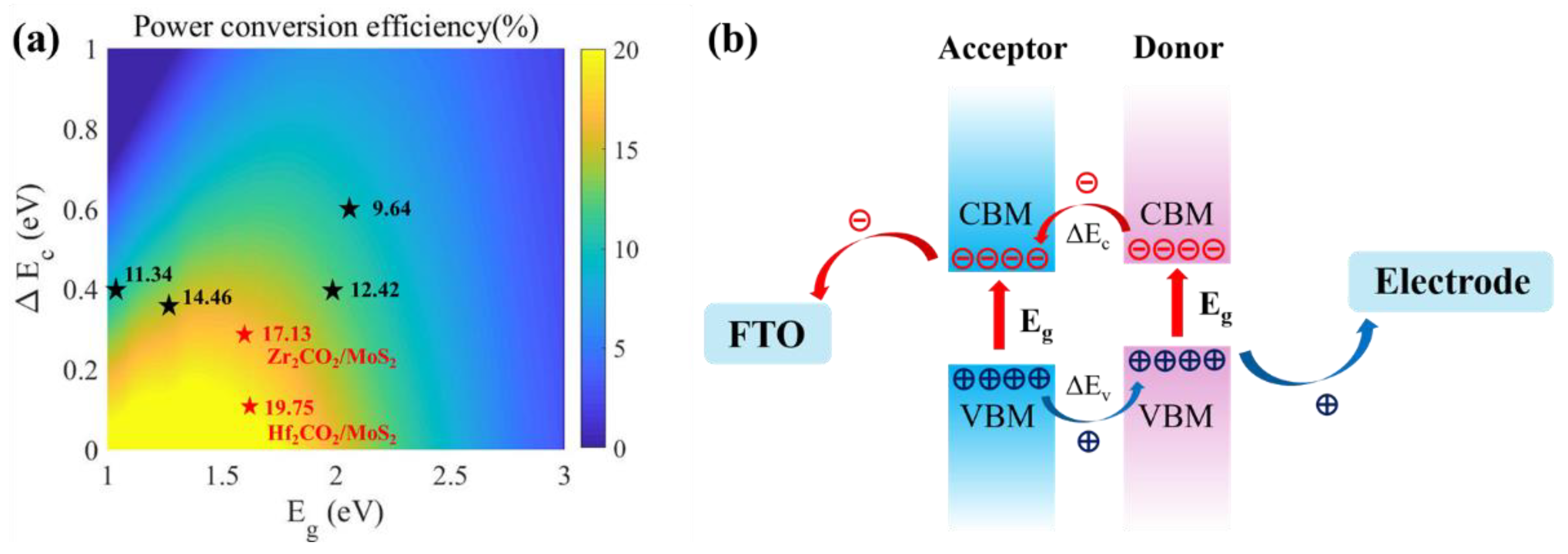
| System | Direction | Carrier Type | E1 | C2D | m* | μ |
|---|---|---|---|---|---|---|
| Hf2CO2/MoS2 | x | e | −10.48 | 428.34 | 1.16 | 41.00 |
| h | 2.06 | 428.34 | −0.55 | 4707.47 | ||
| y | e | −9.40 | 421.71 | 2.00 | 16.71 | |
| h | 1.33 | 421.71 | −0.61 | 9140.34 | ||
| Hf2CO2/MoSe2 | x | e | 8.44 | 419.80 | 0.51 | 323.12 |
| h | 2.80 | 419.80 | −0.66 | 1743.19 | ||
| y | e | 7.63 | 417.61 | 2.09 | 23.01 | |
| h | 2.72 | 417.61 | −0.58 | 2393.98 | ||
| Hf2CO2/MoTe2 | x | e | 7.94 | 409.12 | 0.56 | 295.46 |
| h | −3.75 | 409.12 | −1.30 | 243.67 | ||
| y | e | 5.87 | 404.39 | 2.22 | 33.41 | |
| h | −1.78 | 404.39 | −0.49 | 7592.80 | ||
| Zr2CO2/MoS2 | x | e | −11.47 | 394.34 | 0.80 | 65.10 |
| h | 5.21 | 394.34 | −0.72 | 396.73 | ||
| y | e | −11.49 | 335.30 | 1.37 | 19.08 | |
| h | 2.88 | 335.30 | −0.61 | 1555.99 | ||
| Zr2CO2/MoSe2 | x | e | 6.82 | 368.68 | 0.76 | 192.50 |
| h | 1.32 | 368.68 | −0.87 | 3928.96 | ||
| y | e | 4.43 | 392.74 | 2.68 | 39.24 | |
| h | 2.19 | 392.74 | −0.60 | 3227.15 | ||
| Zr2CO2/MoTe2 | x | e | 10.55 | 323.53 | 8.85 | 0.52 |
| h | −3.29 | 323.53 | −1.64 | 155.33 | ||
| y | e | 6.10 | 369.17 | 2.95 | 16.00 | |
| h | −3.81 | 369.17 | −0.55 | 1192.02 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wen, J.; Cai, Q.; Xiong, R.; Cui, Z.; Zhang, Y.; He, Z.; Liu, J.; Lin, M.; Wen, C.; Wu, B.; et al. Promising M2CO2/MoX2 (M = Hf, Zr; X = S, Se, Te) Heterostructures for Multifunctional Solar Energy Applications. Molecules 2023, 28, 3525. https://doi.org/10.3390/molecules28083525
Wen J, Cai Q, Xiong R, Cui Z, Zhang Y, He Z, Liu J, Lin M, Wen C, Wu B, et al. Promising M2CO2/MoX2 (M = Hf, Zr; X = S, Se, Te) Heterostructures for Multifunctional Solar Energy Applications. Molecules. 2023; 28(8):3525. https://doi.org/10.3390/molecules28083525
Chicago/Turabian StyleWen, Jiansen, Qi Cai, Rui Xiong, Zhou Cui, Yinggan Zhang, Zhihan He, Junchao Liu, Maohua Lin, Cuilian Wen, Bo Wu, and et al. 2023. "Promising M2CO2/MoX2 (M = Hf, Zr; X = S, Se, Te) Heterostructures for Multifunctional Solar Energy Applications" Molecules 28, no. 8: 3525. https://doi.org/10.3390/molecules28083525
APA StyleWen, J., Cai, Q., Xiong, R., Cui, Z., Zhang, Y., He, Z., Liu, J., Lin, M., Wen, C., Wu, B., & Sa, B. (2023). Promising M2CO2/MoX2 (M = Hf, Zr; X = S, Se, Te) Heterostructures for Multifunctional Solar Energy Applications. Molecules, 28(8), 3525. https://doi.org/10.3390/molecules28083525








