DFT Surface Infers Ten-Vertex Cationic Carboranes from the Corresponding Neutral closo Ten-Vertex Family: The Computed Background Confirming Their Experimental Availability
Abstract
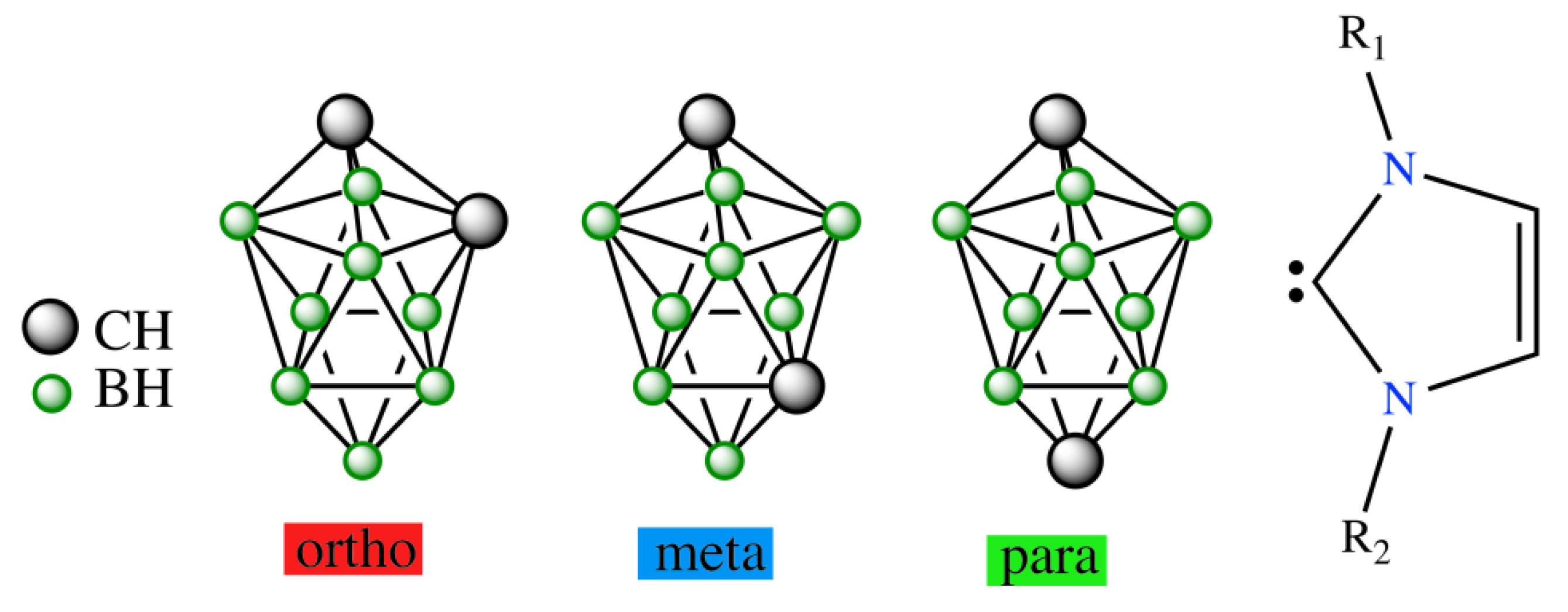
:1. Introduction
2. Results and Discussion
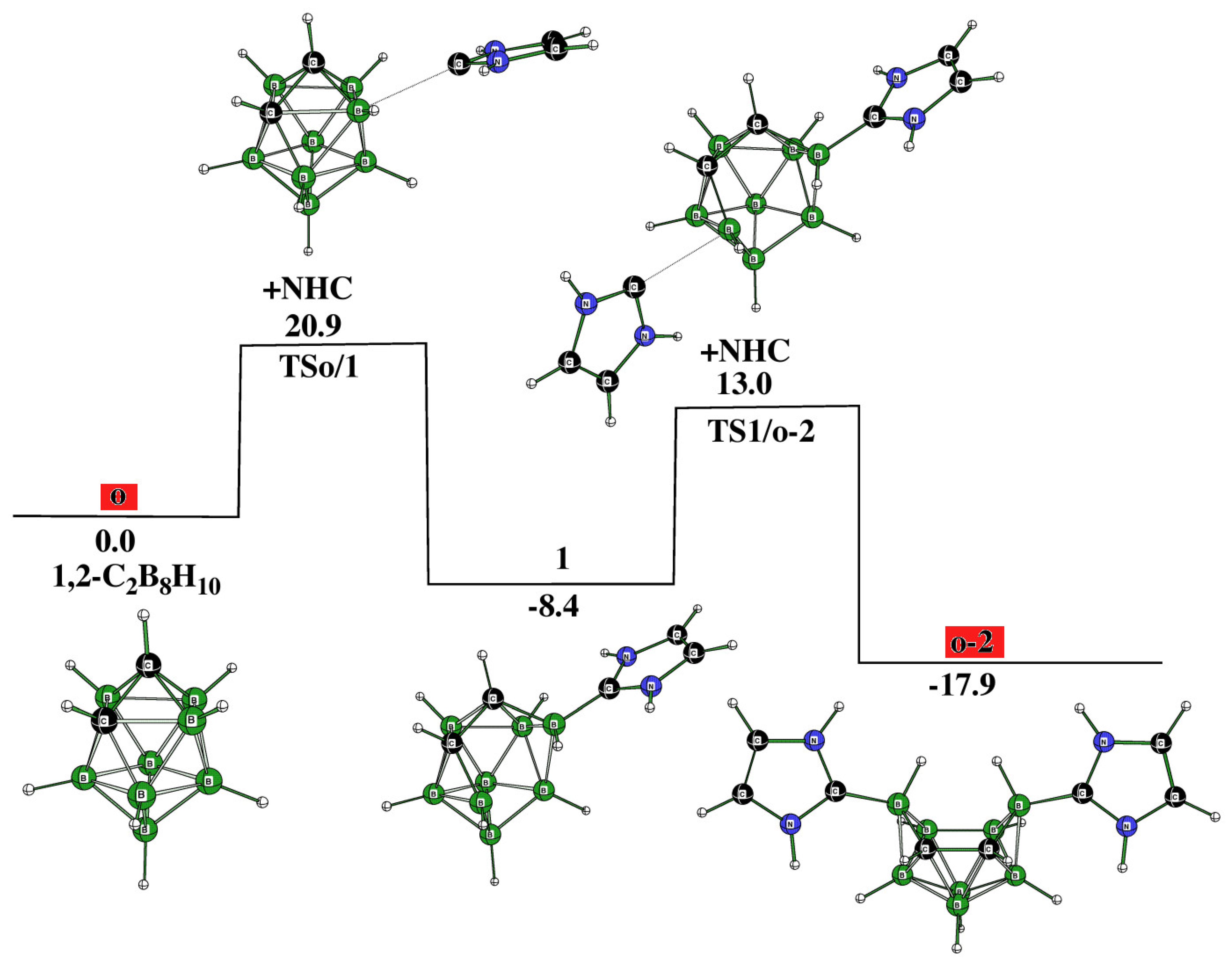
2.1. The Reactions of closo-1,2-C2B8H10 (o) with NHC
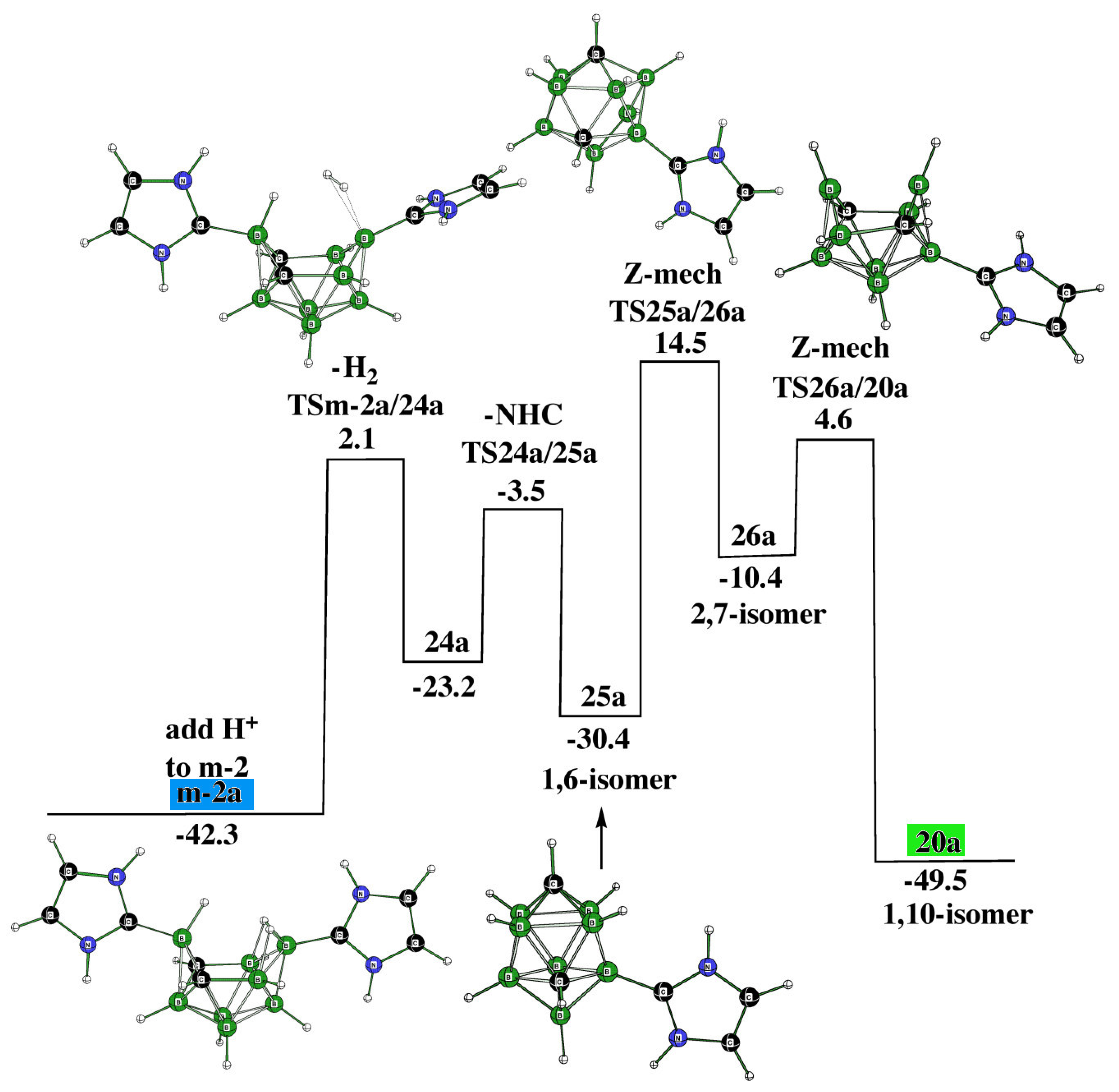
2.2. The Reactions of closo-1,6-C2B8H10 (m) with NHC
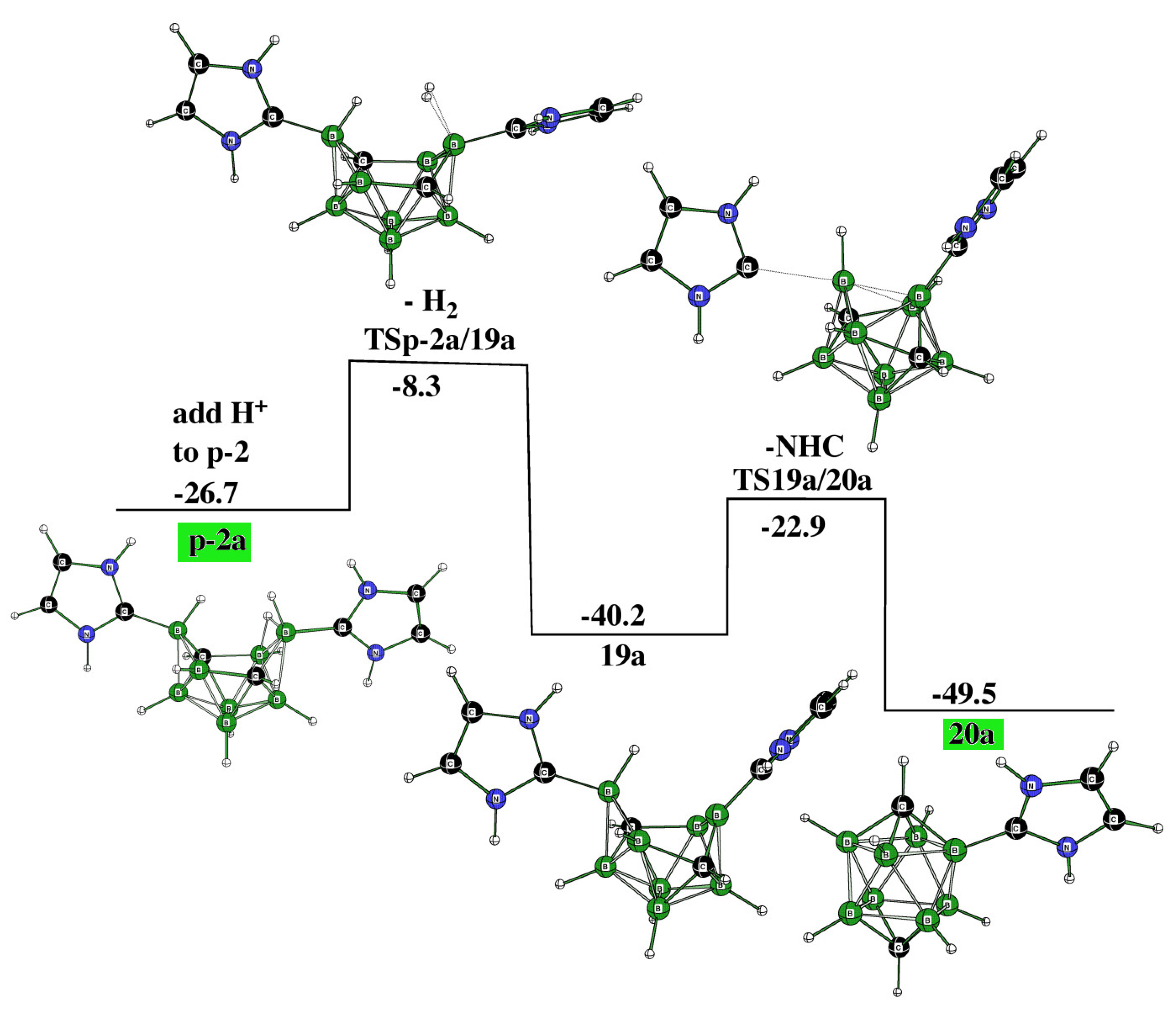
2.3. The Reactions of closo-1,10-C2B8H10 (p) with NHC
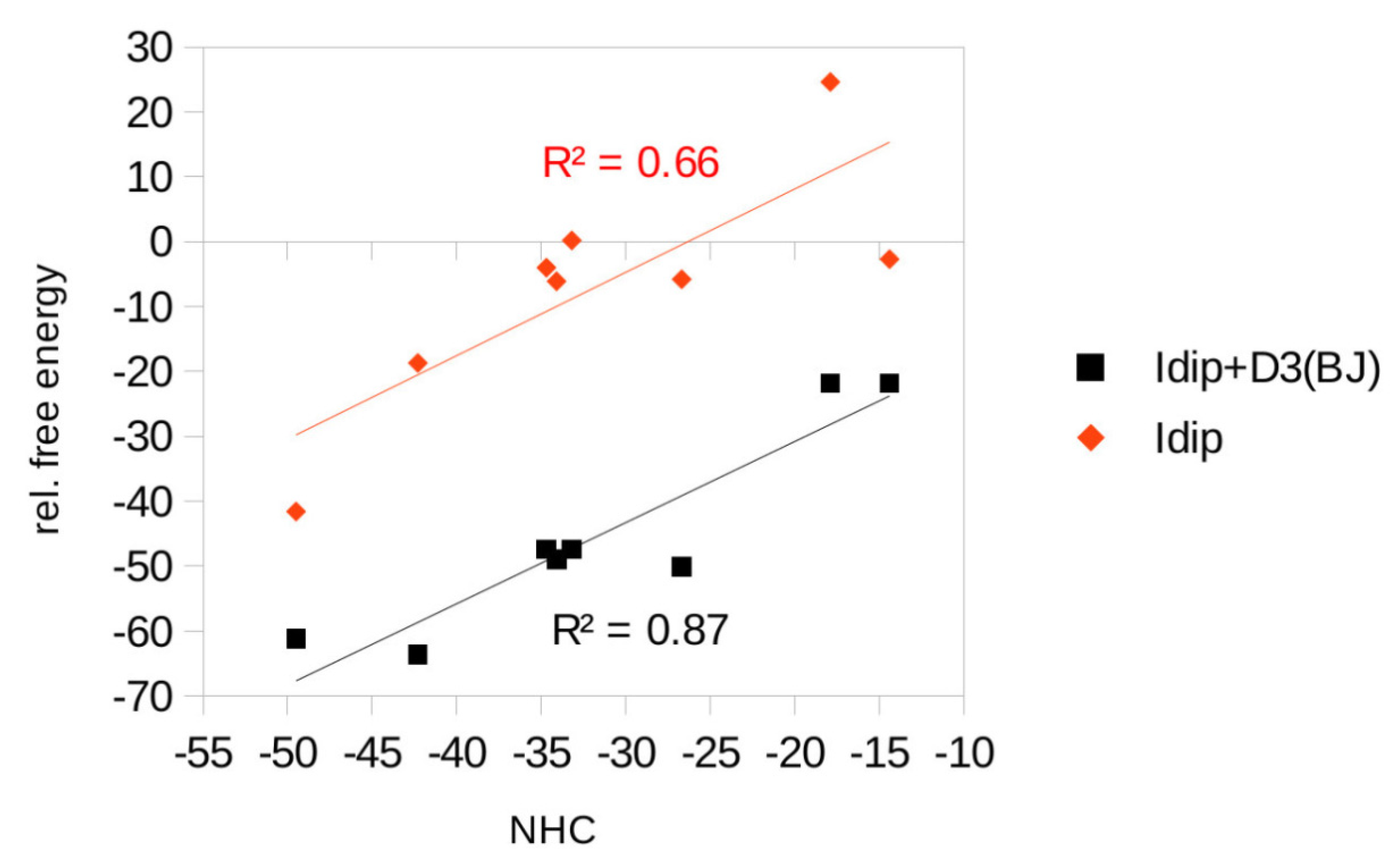
2.4. Further Possible Ten-Vertex Carbocations
3. Methods
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Hnyk, D.; Wann, D.A. Molecular Structures of Free Boron Clusters. In Boron: The Fifth Element; Challenges and Advances in Computational Chemistry and Physics; Hnyk, D., McKee, M., Eds.; Springer: Dordrecht, The Netherlands, 2016; Volume 20, pp. 17–48. [Google Scholar]
- Grimes, R.N. Carboranes, 3rd ed.; Academic Press: Cambridge, MA, USA, 2016. [Google Scholar]
- Melichar, P.; Hnyk, D.; Fanfrlík, J. A Systematic Examination of Classical and Multi-center Bonding in Heteroborane Clusters. Phys. Chem. Chem. Phys. 2018, 20, 4666–4675. [Google Scholar] [CrossRef] [PubMed]
- Keller, W.; Hofmann, M.; Sárosi, M.B.; Fanfrlík, J.; Hnyk, D. Reactivity of Perhalogenated Octahedral Phospha- and Arsaboranes toward THF: A Joint Experimental/Computational Study. Inorg. Chem. 2022, 61, 16565–16572. [Google Scholar] [CrossRef] [PubMed]
- McKee, M.L. Deconvoluting the Reaction Path from B10H14 Plus BH4− to B12H122−. Can Theory Make a Contribution? In Boron: The Fifth Element; Challenges and Advances in Computational Chemistry and Physics; Hnyk, D., McKee, M., Eds.; Springer: Dordrecht, The Netherlands, 2016; Volume 20, pp. 121–138. [Google Scholar]
- McKay, D.; Macgregor, S.A.; Welch, A.J. Isomerisation of nido-[C2B10H12]2− Dianions: Unprecedented Rearrangements and New Structural Motifs in Carborane Cluster Chemistry. Chem. Sci. 2015, 6, 3117–3128. [Google Scholar] [CrossRef] [PubMed]
- Shameena, O.; Pathak, B.; Jemmis, E.D. Theoretical Study of the Reaction of B20H16 with MeCN: Closo/Closo to Closo/Nido Conversion. Inorg. Chem. 2008, 47, 4375–4382. [Google Scholar] [CrossRef] [PubMed]
- Štíbr, B.; Holub, J.; Bakardjiev, M.; Lane, P.D.; McKee, M.L.; Wann, D.A.; Hnyk, D. Unusual Cage Rearrangements in 10-Vertex nido-5,6-Dicarbaborane Derivatives: An Interplay between Theory and Experiment. Inorg. Chem. 2017, 56, 852–860. [Google Scholar] [CrossRef] [PubMed]
- Schleyer, P.V.R.; Najafian, K. Stability and Three-Dimensional Aromaticity of closo-Monocarbaborane Anions, CBn-1Hn-, and closo-Dicarboranes, C2Bn−2Hn. Inorg. Chem. 1998, 37, 3454–3457. [Google Scholar] [CrossRef] [PubMed]
- Hnyk, D.; Holub, J. Handles for the Dicarbadodecaborane Basket Based on [arachno-5,10-C2B8H13]–: Oxygen. Dalton Trans. 2006, 22, 2620–2622. [Google Scholar] [CrossRef] [PubMed]
- Janoušek, Z.; Dostál, R.; Macháček, J.; Hnyk, D.; Štíbr, B. The First Member of the Eleven-Vertex Azadicarbaborane Series, 1,6,9-NC2B8H13 and its N-alkyl Derivatives. Dalton Trans. 2006, 39, 4664–4671. [Google Scholar] [CrossRef] [PubMed]
- Holub, J.; Fanfrlík, J.; McKee, M.L.; Hnyk, D. Reactions of Experimentally Known Closo-C2B8H10 with Bases. A Computational Study. Crystals 2020, 10, 896. [Google Scholar] [CrossRef]
- Holub, J.; Bakardjiev, M.; McKee, M.L. Ten-Vertex Closo-Carboranes React with “Wet” Fluoride: A Direct Closo-to-Arachno Transformation as a Result of a Hydride Transfer. Inorg. Chim. Acta 2022, 538, 12100. [Google Scholar] [CrossRef]
- Vrána, J.; Holub, J.; Samsonov, M.A.; Růžičková, Z.; Cvačka, J.; McKee, M.L.; Fanfrlík, J.; Hnyk, D.; Růžička, A. Access to Cationic Polyhedral Carboranes via Dynamic Cage Surgery with N-heterocyclic Carbenes. Nat. Commun. 2021, 12, 4971. [Google Scholar] [CrossRef] [PubMed]
- Tok, O.L.; Bakardjiev, M.; Štíbr, B.; Hnyk, D.; Holub, J.; Padělková, Z.; Růžička, A. Click Dehydrogenation of Carbon-Substituted nido-5,6-C2B8H12 Carboranes: A General Route to closo-1,2-C2B8H10 Derivatives. Inorg. Chem. 2016, 55, 8839–8843. [Google Scholar] [CrossRef] [PubMed]
- Bakardjiev, M.; Holub, J.; Růžičková, Z.; Růžička, A.; Fanfrlík, J.; Štíbr, B.; McKee, M.L.; Hnyk, D. Transformation of Various Multicenter Bondings within Bicapped-Square Antiprismatic Motifs: Z-Rearrangement. Dalton Trans. 2021, 50, 12019–12334. [Google Scholar] [CrossRef] [PubMed]
- Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A Consistent and Accurate Ab initio Parametrization of Density Functional Dispersion Correction (DFT-D) for the 94 Elements H–Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef] [PubMed]
- Gimarc, B.M.; Ott, J.J. Isomerization of Carboranes C2B6H8, C2B8H10 and C2B9H11 by the Diamond-Square-Diamond Rearrangement. J. Am. Chem. Soc. 1987, 109, 1388–1392. [Google Scholar] [CrossRef]
- Hnyk, D.; Všetečka, V.; Drož, L.; Exner, O. Charge Distribution within 1,2-Dicarba-closo-dodecaborane: Dipole Moments of its Phenyl Derivatives. Collect. Czech. Chem. Commun. 2001, 66, 1375–1379. [Google Scholar] [CrossRef]
- Maué, D.; Streber, P.H.; Bernhard, D.; Rösel, S.; Schreiner, P.R.; Gerhards, M. Dispersion-Bound Isolated Dimers in the Gas Phase: Observation of the Shortest Intermolecular C-H…H-C Distance via Stimulated Raman Spectroscopy. Angew. Chem. Int. Ed. 2021, 60, 11305–11309. [Google Scholar] [CrossRef] [PubMed]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16, Revision C.01; Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]






| Reactant to Product | B3LYP a | B3LYP b |
|---|---|---|
| o to o-1 | −14.4 | −21.8 (−2.7) |
| o to o-2 | −17.9 | −21.8 (24.6) c |
| m to m-2 | −34.7 | −47.4 (−4.0) c |
| p to p-2 | −34.1 | −49.0 (−6.1) |
| o-2 to o-2a | −33.2 | −47.4 (0.2) |
| m-2 to m-2a | −42.3 | −63.6 (−18.7) |
| p-2 to p-2a | −26.7 | −50.1 (−5.8) |
| p-2a to 20a | −49.5 | −61.2 (−41.6) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
McKee, M.L.; Vrána, J.; Holub, J.; Fanfrlík, J.; Hnyk, D. DFT Surface Infers Ten-Vertex Cationic Carboranes from the Corresponding Neutral closo Ten-Vertex Family: The Computed Background Confirming Their Experimental Availability. Molecules 2023, 28, 3645. https://doi.org/10.3390/molecules28083645
McKee ML, Vrána J, Holub J, Fanfrlík J, Hnyk D. DFT Surface Infers Ten-Vertex Cationic Carboranes from the Corresponding Neutral closo Ten-Vertex Family: The Computed Background Confirming Their Experimental Availability. Molecules. 2023; 28(8):3645. https://doi.org/10.3390/molecules28083645
Chicago/Turabian StyleMcKee, Michael L., Jan Vrána, Josef Holub, Jindřich Fanfrlík, and Drahomír Hnyk. 2023. "DFT Surface Infers Ten-Vertex Cationic Carboranes from the Corresponding Neutral closo Ten-Vertex Family: The Computed Background Confirming Their Experimental Availability" Molecules 28, no. 8: 3645. https://doi.org/10.3390/molecules28083645
APA StyleMcKee, M. L., Vrána, J., Holub, J., Fanfrlík, J., & Hnyk, D. (2023). DFT Surface Infers Ten-Vertex Cationic Carboranes from the Corresponding Neutral closo Ten-Vertex Family: The Computed Background Confirming Their Experimental Availability. Molecules, 28(8), 3645. https://doi.org/10.3390/molecules28083645








