Thermodynamic Assessment of the Pyrazinamide Dissolution Process in Some Organic Solvents
Abstract
1. Introduction
2. Results
Experimental Solubility ()
3. Thermodynamic Functions
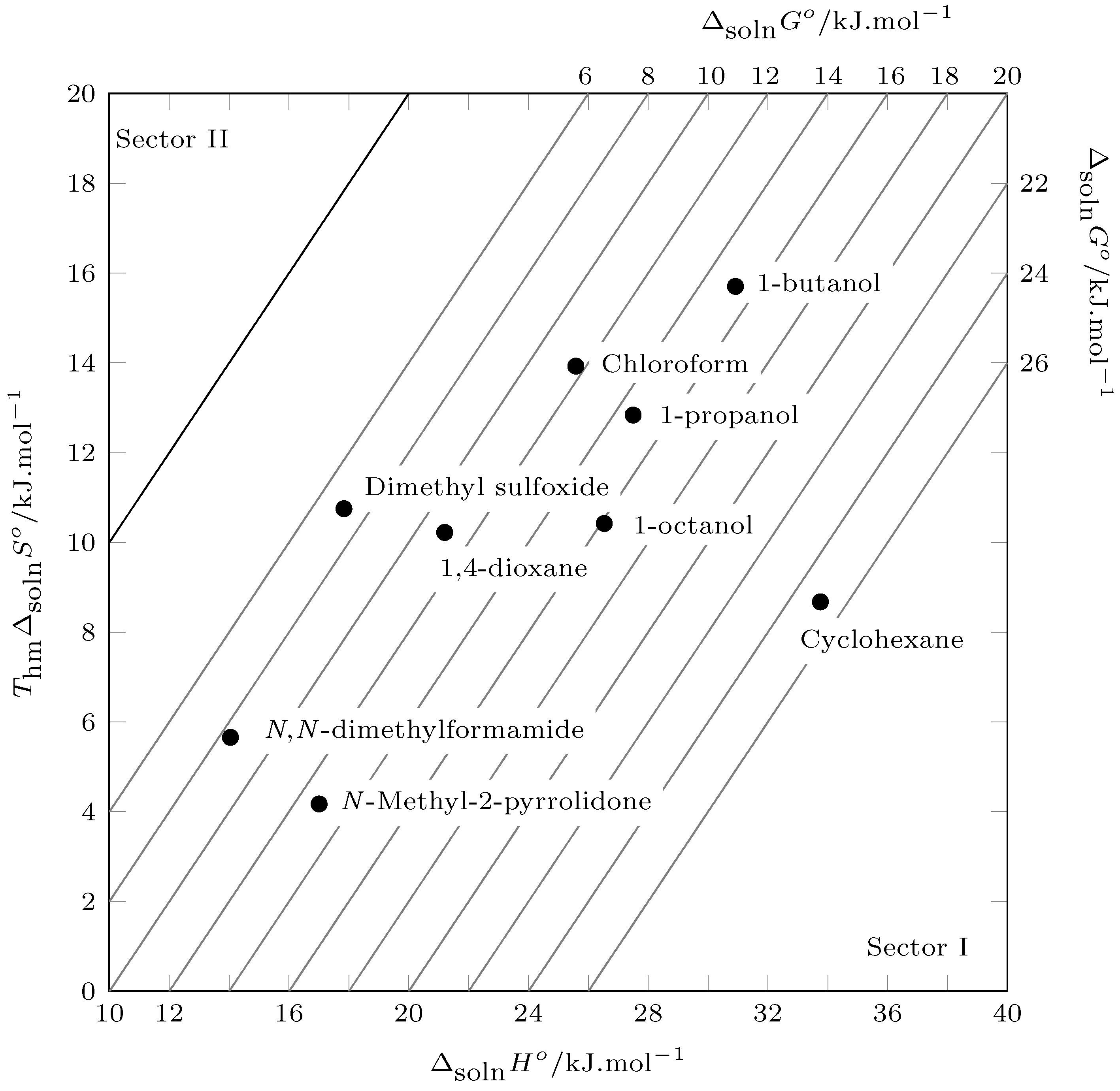
3.1. Thermodynamic Functions of Solution
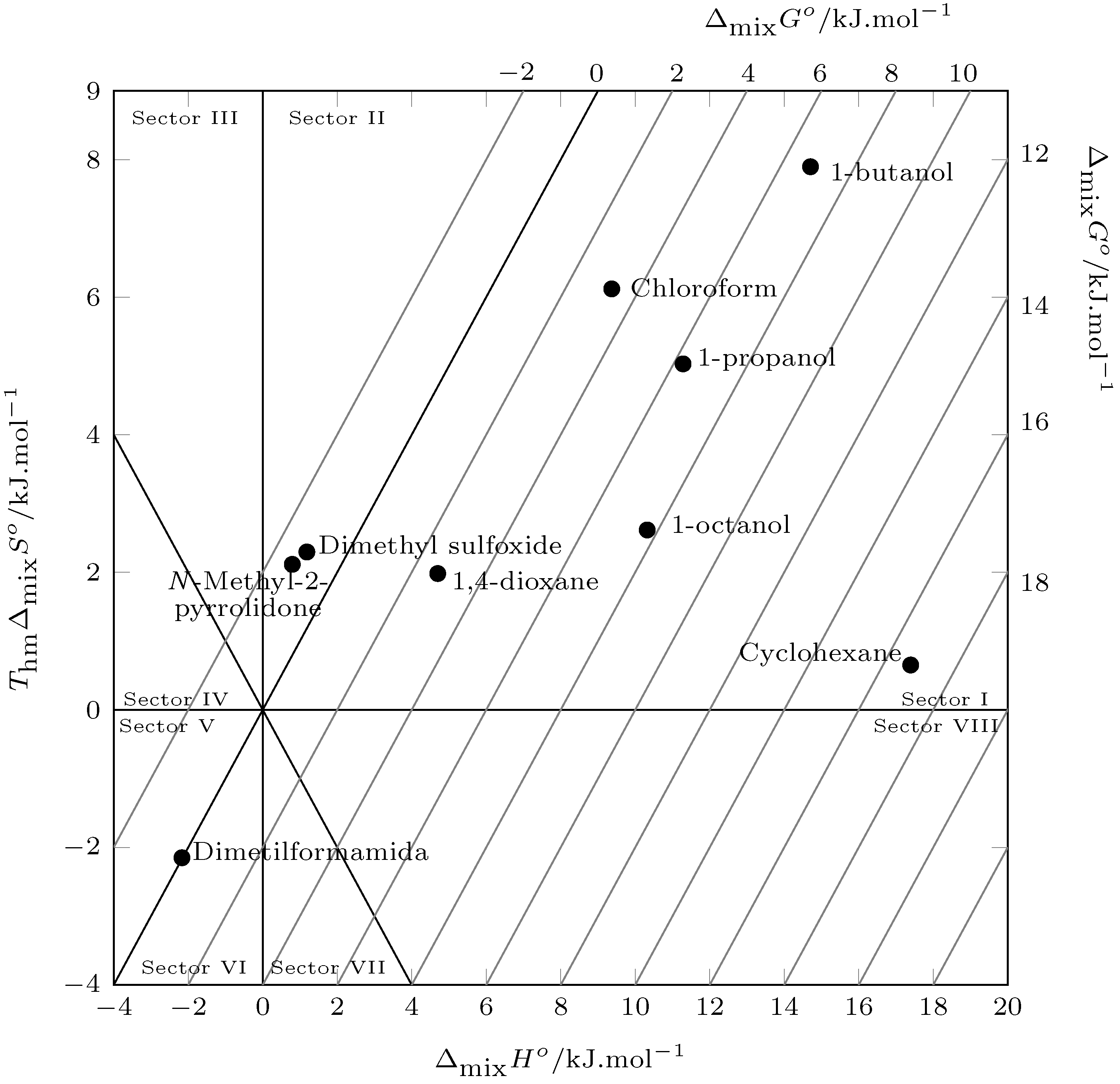
- Sector I:
- Sector II:
- Sector III:
- Sector IV:
- Sector V:
- Sector VI:
- Sector VII:
- Sector VIII:
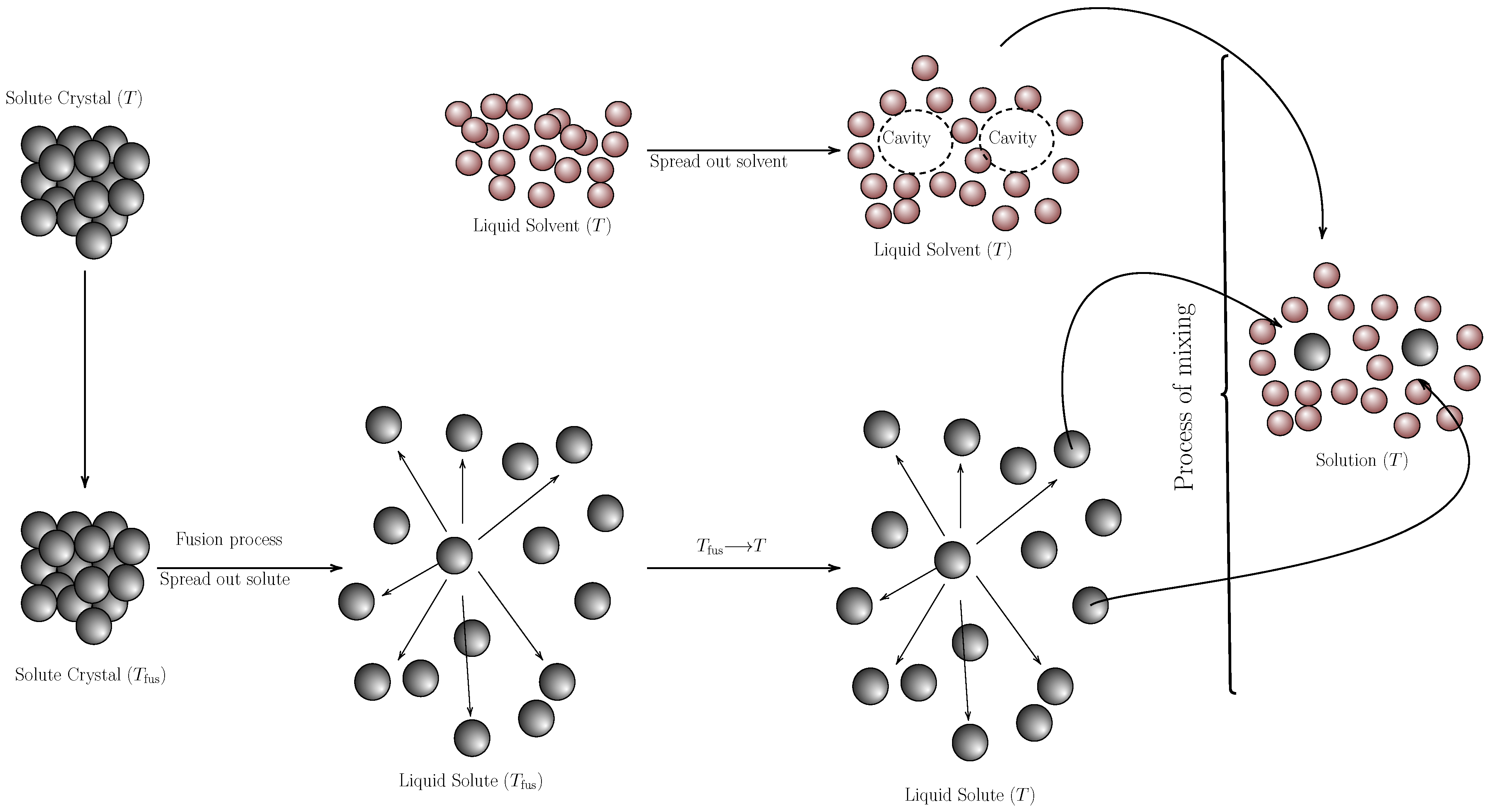
3.2. Thermodynamic Functions of Mixing
- The hypothetical melting of the solute to sub-cooled liquid and the restructuring of the solvent molecules to form the cavity that will house the solute molecule.
- Interaction between the solute and solvent molecules (mixing process) to form the solution.
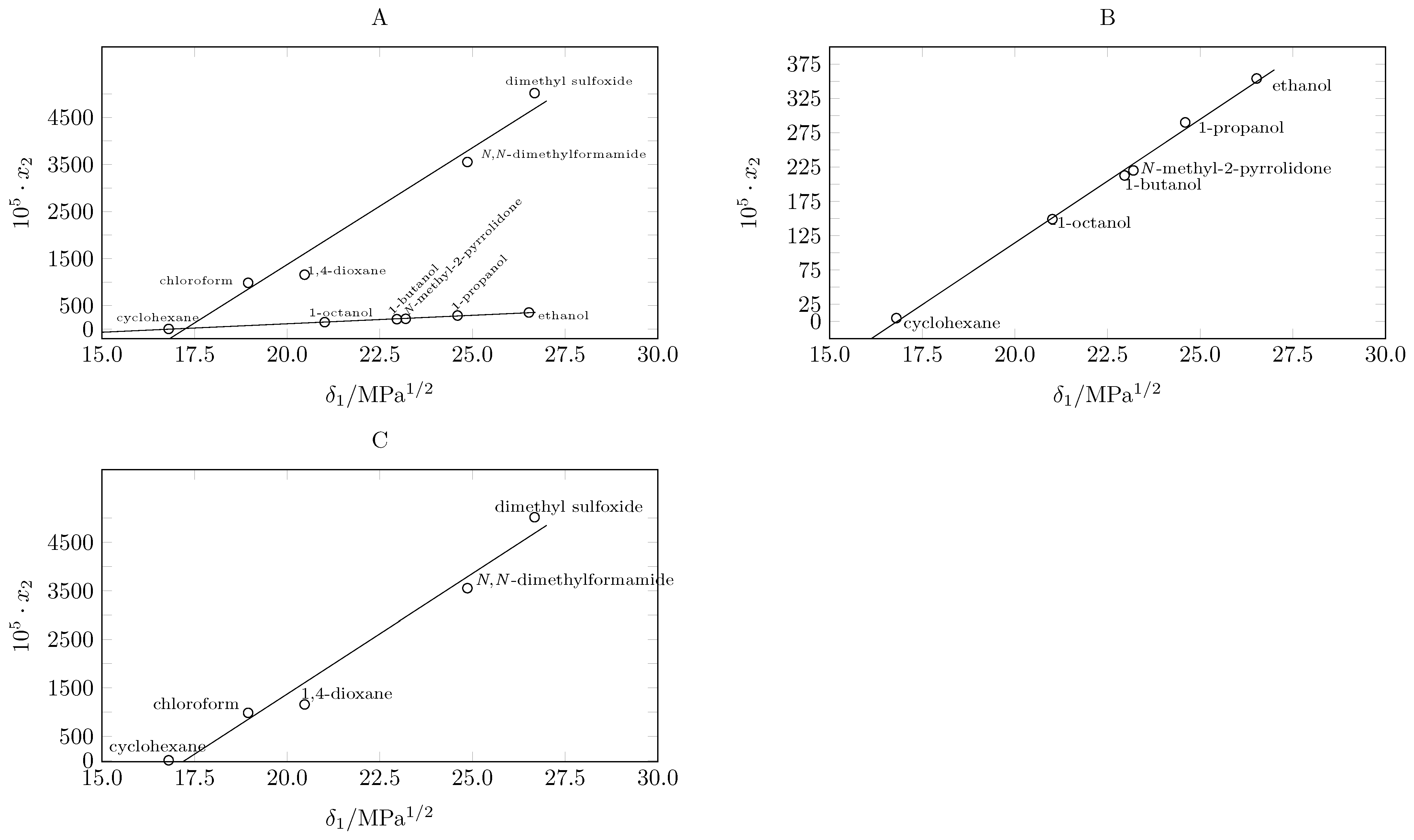
3.3. Solubility Parameters of Pyrazinamide
4. Materials and Methods
4.1. Reagents
4.2. Solubility Determination
- Saturation of the solvent: In an amber colored bottle, 5.0 mL of solvent is added; then, pyrazinamide is added with vigorous stirring until a saturated solution is obtained (this process is verified by measuring the concentration of the drug until a constant concentration is obtained).
- Thermodynamic equilibrium: To ensure solvent saturation, the samples remain for 36 h at constant temperature (at each of the study temperatures) in a recirculation bath (Medingen K-22/T100, Medingen, Germany). To ensure thermodynamic equilibrium, in all cases, a sufficient amount of pyrazinamide is added to generate an equilibrium between the saturated solution and a quantity of undissolved solid drug (usually remaining at the bottom of the flask).
- Filtration: To ensure that no undissolved solids are taken up at the time of quantification, the samples are filtered through 0.45 μm membranes (Millipore Corp. Swinnex-13, Burlington, MA, USA).
- Quantification: The method used is UV/Vis spectrometry; thus, the wavelength of maximum absorbance of pyrazinamide (267 nm ()) is determined and a calibration curve is designed in the range of compliance with the Lambert–Beer law (UV/Vis EMC-11- UV spectrophotometer, Duisburg, Germany). For solutions with very low concentrations, the standard addition method described by Caviedes-Rubio et al. [66] is used.
- Evaluation of the solid phase: To evaluate possible polymorphic changes or decomposition of pyrazinamide, the solid phases in equilibrium with the saturated solutions are analyzed by DSC. The solution is first saturated at a temperature higher than the study temperature so that the drug portion precipitates. This precipitate is collected, dried, and subjected to DSC analysis to determine whether polymorphic changes have occurred with respect to the original sample.
4.3. Calorimetric Study
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Suárez, I.; Fünger, S.M.; Kröger, S.; Rademacher, J.; Fätkenheuer, G.; Rybniker, J. The diagnosis and treatment of tuberculosis. Dtsch Arztebl Int. 2019, 116, 729–735. [Google Scholar] [CrossRef]
- World Health Organization (WHO). Treatment of Tuberculosis: Guidelines; World Health Organization: Geneva, Switzerland, 2010. [Google Scholar]
- Sosa, E.J.; Burguener, G.; Lanzarotti, E.; Defelipe, L.; Radusky, L.; Pardo, A.M.; Marti, M.; Turjanski, A.G.; Fernández Do Porto, D. Target-Pathogen: A structural bioinformatic approach to prioritize drug targets in pathogens. Nucleic Acids Res. 2017, 46, D413–D418. [Google Scholar] [CrossRef]
- Jones, N.T.; Abadie, R.; Keller, C.L.; Jones, K.; Ledet, L.F., III; Fox, J.E.; Klapper, V.G.; Potharaju, P.; Siddaiah, H.; Kaye, A.M.; et al. Treatment and toxicity considerations in tuberculosis: A narrative review. Cureus 2024, 16, e62698. [Google Scholar] [CrossRef] [PubMed]
- Delgado, D.R. LaTeX Code of the Chemical Structure of Some Drugs; Universidad Cooperativa de Colombia: Arauca, Colombia, 2024. [Google Scholar] [CrossRef]
- NORMAN Network. Emerging Substances. 2023. Available online: https://www.norman-network.com/nds/susdat/susdatSearchShow.php (accessed on 28 August 2014).
- Rubio, D.I.C.; Camacho Feria, D.M.; Delgado, D.R. Tratamientos para la remoción de antibacteriales y agentes antimicrobiales presentes en aguas residuales. Rev. Logos Cienc. Tecnol. 2017, 9, 43–62. [Google Scholar] [CrossRef]
- Harrower, J.; McNaughtan, M.; Hunter, C.; Hough, R.; Zhang, Z.; Helwig, K. Chemical fate and partitioning behavior of antibiotics in the aquatic environment—A review. Environ. Toxicol. Chem. 2021, 40, 3275–3298. [Google Scholar] [CrossRef] [PubMed]
- Yalkowsky, S.H. Solubility and Solubilization in Aqueous Media; American Chemical Society: Washington, DC, USA, 1999. [Google Scholar]
- Sinko, P.J. Martin’s Physical Pharmacy and Pharmaceutical Sciences, 8th ed.; Lippincott Williams & Wilkins: Philadelphia, PA, USA, 2011. [Google Scholar]
- Cristancho, D.M.; Delgado, D.R.; Martínez, F. Meloxicam solubility in ethanol+ water mixtures according to the extended Hildebrand solubility approach. J. Solut. Chem. 2013, 42, 1706–1716. [Google Scholar] [CrossRef]
- Jouyban, A.; Fakhree, M.A.A.; Shayanfar, A. Review of pharmaceutical applications of N-methyl-2-pyrrolidone. J. Pharm. Pharm. Sci. 2010, 13, 524–535. [Google Scholar] [CrossRef]
- Liu, X.; Abraham, M.H.; Acree, W.E. Abraham model descriptors for melatonin; prediction of Solution, biological and thermodynamic properties. J. Solut. Chem. 2022, 51, 992–999. [Google Scholar] [CrossRef]
- Llompart, P.; Minoletti, C.; Baybekov, S.; Horvath, D.; Marcou, G.; Varnek, A. Will we ever be able to accurately predict solubility? Sci. Data Vol. 2024, 11, 303. [Google Scholar] [CrossRef]
- Gupta Soni, A.; Joshi, R.; Soni, D.; Deep Kaur, C.; Saraf, S.; Kumar Singh, P. Predicting Drug Properties: Computational Strategies for Solubility and Permeability Rates. In Software and Programming Tools in Pharmaceutical Research; Bentham Science Publishers: Sharjah, United Arab Emirates, 2024; pp. 135–152. [Google Scholar]
- Macedo, B.; Taveira-Gomes, T. Automating drug discovery. In Artificial Intelligence for Drug Product Lifecycle Applications; Pais, A., Vitorino, C., Nunes, S., Cova, T., Eds.; Academic Press: Cambridge, MA, USA, 2025; pp. 83–97. [Google Scholar] [CrossRef]
- Yuan, H.; Yang, J.; Wang, M.; Li, H.; Li, Y.; Li, T.; Ren, B. Solubility measurement and data correlation of 2-ethoxy-1-naphthoic acid in twelve pure solvents at temperatures from 278.15 to 323.15 K. J. Chem. Thermodyn. 2025, 200, 107365. [Google Scholar] [CrossRef]
- Sorkun, M.C.; Koelman, J.M.V.A.; Er, S. Pushing the limits of solubility prediction via quality-oriented data selection. iScience 2021, 24, 101961. [Google Scholar] [CrossRef] [PubMed]
- Redlich, O.; Kwong, J.N.S. On the thermodynamics of solutions. V. an equation of state. Fugacities of gaseous solutions. Chem. Rev. 1949, 44, 233–244. [Google Scholar] [CrossRef] [PubMed]
- Yaws, C.L.; Narasimhan, P.K.; Lou, H.H.; Pike, R.W. Solubility of Chemicals in Water. In Water Encyclopedia; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2005; pp. 555–559. [Google Scholar] [CrossRef]
- Hokkala, E.; Strachan, C.J.; Agopov, M.; Järvinen, E.; Semjonov, K.; Heinämäki, J.; Yliruusi, J.; Svanbäck, S. Thermodynamic solubility measurement without chemical analysis. Int. J. Pharm. 2024, 653, 123890. [Google Scholar] [CrossRef] [PubMed]
- Sharapova, A.V.; Ol’khovich, M.V.; Blokhina, S.V. Thermodynamic consideration of dissolution and distribution behavior of carvedilol in pharmaceutical significant media. J. Chem. Thermodyn. 2024, 190, 107207. [Google Scholar] [CrossRef]
- Lee, J.L.; Chong, G.H.; Kanno, A.; Ota, M.; Guo, H.; Smith, R.L. Local composition-regular solution theory for analysis of pharmaceutical solubility in mixed-solvents. J. Mol. Liq. 2024, 397, 124012. [Google Scholar] [CrossRef]
- Jouyban, A.; Khezri, S.; Jafari, P.; Zarghampour, A.; Acree, W.E., Jr. A new set of solute descriptors to calculate solubility of drugs in mono-solvents. Ann. Pharm. Françaises 2023, 81, 1109–1117. [Google Scholar] [CrossRef]
- Marcus, Y. The Properties of Solvents, 1st ed.; John Wiley & Sons: Hoboken, NJ, USA, 1998. [Google Scholar]
- Marcus, Y. Solvent Mixtures: Properties and Selective Solvation; CRC Press: Boca Raton, FL, USA, 1998. [Google Scholar]
- Marcus, Y. On the preferential solvation of drugs and PAHs in binary solvent mixtures. J. Mol. Liq. 2008, 140, 61–67. [Google Scholar] [CrossRef]
- Tayyebi, A.; Alshami, A.; Rabiei, Z.; Yu, X.; Ismail, N.; Talukder, M.; Power, J. Prediction of organic compound aqueous solubility using machine learning: A comparison study of descriptor-based and fingerprints-based models. J Cheminf 2023, 25, 1–16. [Google Scholar] [CrossRef]
- Cuellar-Carmona, Y.L.; Cerquera, N.E.; Cardenas-Torres, R.E.; Ortiz, C.P.O.; Martínez, F.; Delgado, D.R.D. Correlation of the solubility of isoniazid in some aqueous cosolvent mixtures using different mathematical models. Braz. J. Chem. Eng. 2024, 1–14. [Google Scholar] [CrossRef]
- Hildebrand, J.H. Solubility. XII. Regular solutions. J. Am. Chem. Soc. 1929, 51, 66–80. [Google Scholar] [CrossRef]
- Rivas-Ozuna, D.A.; Ortiz, C.P.; Delgado, D.R.; Martínez, F. Solubility and preferential solvation of pyrazinamide in some aqueous-cosolvent mixtures at 298.15 K. Int. J. Thermophys. 2024, 45, 39. [Google Scholar] [CrossRef]
- Barton, A.F.M. Handbook of Solubility Parameters and Other Cohesion Parameters, 2nd ed.; CRC Press: Boca Raton, FL, USA, 1991. [Google Scholar]
- Maharana, A.; Sarkar, D. Solubility measurements and thermodynamic modeling of pyrazinamide in five different solvent-antisolvent mixtures. Fluid Phase Equilibria 2019, 497, 33–54. [Google Scholar] [CrossRef]
- Hermanto, M.W.; Yeoh, A.; Soh, B.; Chow, P.S.; Tan, R.B. Robust crystallization process development for the metastable δ-form of pyrazinamide. Org. Process Res. Dev. 2015, 19, 1987–1996. [Google Scholar] [CrossRef]
- Xi, S.; Wang, S.; Lu, G.; Wang, J. Solubility measurement, correlation and cosolvent phenomena for pyrazinamide in two mixed solvents. J. Beijing Univ. Chem. Technol. (Nat. Sci.) 2019, 46, 12–21. [Google Scholar]
- Blokhina, S.V.; Ol’khovich, M.V.; Sharapova, A.V.; Volkova, T.V.; Perlovich, G.L. Solution thermodynamics of pyrazinamide, isoniazid, and p-aminobenzoic acid in buffers and octanol. J. Chem. Thermodyn. 2015, 91, 396–403. [Google Scholar] [CrossRef]
- Zhang, K.; Shen, H.; Xu, S.; Zhang, H.; Zhu, M.; Shi, P.; Fu, X.; Yang, Y.; Gong, J. Thermodynamic study of solubility for pyrazinamide in ten solvents from T = (283.15 to 323.15) K. J. Chem. Thermodyn. 2017, 112, 204–212. [Google Scholar] [CrossRef]
- Bernstein, J. Polymorphism in drug design and delivery. Prog. Clin. Biol. Res. 1989, 289, 203–215. [Google Scholar]
- Singhal, D.; Curatolo, W. Drug polymorphism and dosage form design: A practical perspective. Adv. Drug Deliv. Rev. 2004, 56, 335–347. [Google Scholar] [CrossRef]
- Grunenberg, A.; Keil, B.; Henck, J.O. Polymorphism in binary mixtures, as exemplified by nimodipine. Int. J. Pharm. 1995, 118, 11–21. [Google Scholar] [CrossRef]
- Cherukuvada, S.; Thakuria, R.; Nangia, A. Pyrazinamide polymorphs: Relative stability and vibrational spectroscopy. Cryst. Growth Des. 2010, 10, 3931–3941. [Google Scholar] [CrossRef]
- Negoro, A.; Miki, T.; Ueda, S.; Sanada, T.; Okada, R. Solubility phenomena of pyridine- and pyrazine-monocarboxamides. II: Heats of fusion of picolinamide, nicotinamide, isonicotinamide, and pyrazincarboxamide. Yakugaku Zasshi 1960, 80, 670–673. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Castro, R.A.E.; Maria, T.M.R.; Évora, A.O.L.; Feiteira, J.C.; Silva, M.R.; Beja, A.M.; Canotilho, J.; Eusébio, M.E.S. A new insight into pyrazinamide polymorphic forms and their thermodynamic relationships. Cryst. Growth Des. 2010, 10, 274–282. [Google Scholar] [CrossRef]
- Baaklini, G.; Dupray, V.; Coquerel, G. Inhibition of the spontaneous polymorphic transition of pyrazinamide γ form at room temperature by co-spray drying with 1,3-dimethylurea. Int. J. Pharm. 2015, 479, 163–170. [Google Scholar] [CrossRef] [PubMed]
- Bustamante, C.; Bustamante, P. Nonlinear enthalpy–entropy compensation for the solubility of phenacetin in dioxane–water solvent mixtures. J. Pharm. Sci. 1996, 85, 1109–1111. [Google Scholar] [CrossRef]
- Peña, M.A.; Bustamante, P.; Escalera, B.; Reíllo, A.; Bosque-Sendra, J.M. Solubility and phase separation of benzocaine and salicylic acid in 1,4-dioxane–water mixtures at several temperatures. J. Pharm. Biomed. Anal. 2004, 36, 571–578. [Google Scholar] [CrossRef]
- Krug, R.R.; Hunter, W.G.; Grieger, R.A. Enthalpy-entropy compensation. 1. Some fundamental statistical problems associated with the analysis of van’t Hoff and Arrhenius data. J. Phys. Chem. 1976, 80, 2335–2341. [Google Scholar] [CrossRef]
- Krug, R.R.; Hunter, W.G.; Grieger, R.A. Enthalpy-entropy compensation. 2. Separation of the chemical from the statistical effect. J. Phys. Chem. 1976, 80, 2341–2351. [Google Scholar] [CrossRef]
- Delgado, D.R.; Rodríguez, G.A.; Martínez, F. Thermodynamic study of the solubility of sulfapyridine in some ethanol+water mixtures. J. Mol. Liq. 2013, 177, 156–161. [Google Scholar] [CrossRef]
- Yalkowsky, S.H.; Wu, M. Estimation of the ideal solubility (crystal-liquid fugacity ratio) of organic compounds. J. Pharm. Sci. 2010, 99, 1100–1106. [Google Scholar] [CrossRef]
- Hildebrand, J.H.; Prausnitz, J.M.; Scott, R.L. Regular and Related Solutions: The Solubility of Gases, Liquids, and Solids; Van Nostrand Reinhold: New York, NY, USA, 1970. [Google Scholar]
- Neau, S.H.; Flynn, G.L. Solid and liquid heat capacities of n-alkyl para-aminobenzoates near the melting point. Pharm. Res. 1990, 7, 157–1162. [Google Scholar] [CrossRef]
- Neau, S.H.; Bhandarkar, S.V.; Hellmuth, E.W. Differential molar heat capacities to test ideal solubility estimations. Pharm. Res. 1997, 14, 601–605. [Google Scholar] [CrossRef] [PubMed]
- Opperhuizen, A.; Gobas, F.A.P.C.; Van der Steen, J.M.D.; Hutzinger, O. Aqueous solubility of polychlorinated biphenyls related to molecular structure. Environ. Sci. Technol. 1988, 22, 638–646. [Google Scholar] [CrossRef]
- Ortiz, C.P.; Cardenas-Torres, R.E.; Herrera, M.; Delgado, D.R. Numerical analysis of sulfamerazine solubility in acetonitrile + 1-Propanol cosolvent mixtures at different temperatures. Sustainability 2023, 15, 6596. [Google Scholar] [CrossRef]
- van Krevelen, D.; te Nijenhuis, K. Properties of Polymers: Their Correlation with Chemical Structure; Their Numerical Estimation and Prediction from Additive Group Contributions; Elsevier Science: Amsterdam, The Netherlands, 2009. [Google Scholar]
- van Krevelen, D.; Hoftyzer, P. Properties of Polymers, Their Estimation and Correlation with Chemical Structure; Elsevier Science: Amsterdam, The Netherlands, 1976. [Google Scholar]
- Ravindra, R.; Krovvidi, K.R.; Khan, A. Solubility parameter of chitin and chitosan. Carbohydr. Polym. 1998, 36, 121–127. [Google Scholar] [CrossRef]
- Bustamante, P.; Martin, A.; Gonzalez-Guisandez, M. Partial solubility parameters and solvatochromie parameters for predicting the solubility of single and multiple drugs in individual solvents. J. Pharm. Sci. 1993, 82, 635–640. [Google Scholar] [CrossRef]
- Peña-Fernandez, M.A.; Spano, G.; Torres-Pabón, N.S.; Martínez, F. Solubility data and solubility parameters of barnidipine in different pure solvents. Ars Pharm. 2023, 64, 329–341. [Google Scholar] [CrossRef]
- Bustamante, P.; Ochoa, R.; Reillo, A.; Escalera, J.B. Chameleonic effect of sulfanilamide and sulfamethazine in solvent mixtures. Solubility curves with two maxima. Chem. Pharm. Bull. 1994, 42, 1129–1133. [Google Scholar] [CrossRef]
- Higuchi, T.; Connors, K. Advances in Analytical Chemistry and Instrumentation; Interscience Publishers, Inc.: New York, NY, USA, 1965. [Google Scholar]
- Dittert, L.W.; Higuchi, T.; Reese, D.R. Phase solubility technique in studying the formation of complex salts of triamterene. J. Pharm. Sci. 1964, 53, 1325–1328. [Google Scholar] [CrossRef]
- Mader, W.J.; Higuchi, T. Phase solubility analysis. CRC Crit. Rev. Anal. Chem. 1970, 1, 193–215. [Google Scholar] [CrossRef]
- Ortiz, C.P.; Cardenas-Torres, R.E.; Herrera, M.; Delgado, D.R. Thermodynamic analysis of the solubility of propylparaben in acetonitrile + water cosolvent mixtures. Sustainability 2023, 15, 4795. [Google Scholar] [CrossRef]
- Caviedes-Rubio, D.I.; Ortiz, C.P.; Martinez, F.; Delgado, D.R. Thermodynamic assessment of triclocarban dissolution process in N-Methyl-2-pyrrolidone + water cosolvent mixtures. Molecules 2023, 28, 7216. [Google Scholar] [CrossRef] [PubMed]



| Solvent | Temperature/K b | ||||
| 278.15 | 283.15 | 288.15 | 293.15 | 298.15 | |
| Cyclohexane c | 1.73 ± 0.05 | 2.48 ± 0.05 | 3.302 ± 0.026 | 4.21 ± 0.08 | |
| 1,4-dioxane d | 8.42 ± 0.20 | 9.25 ± 0.09 | 11.58 ± 0.23 | ||
| Chloroform d | 3.83 ± 0.26 | 5.24 ± 0.08 | 6.8 ± 0.1 | 8.47 ± 0.07 | 9.85 ± 0.27 |
| DMF d | 21.8 ± 0.6 | 25.13 ± 0.29 | 28.4 ± 0.4 | 31.5 ± 0.7 | 35.5 ± 0.5 |
| DMSO d | 46.6 ± 0.4 | 50.2 ± 1.2 | |||
| NMP d | 1.19 ± 0.07 | 1.14 ± 0.03 | 1.16 ± 0.10 | 1.82 ± 0.16 | 2.13 ± 0.12 |
| 1-propanol d | 1.167 ± 0.033 | 1.532 ± 0.03 | 1.83 ± 0.04 | 2.29 ± 0.05 | 2.9 ± 0.05 |
| 1-butanol d | 0.923 ± 0.03 | 1.089 ± 0.01 | 1.41 ± 0.04 | 1.75 ± 0.04 | 2.2 ± 0.022 |
| 1-octanol d | 0.717 ± 0.017 | 0.849 ± 0.015 | 1.052 ± 0.012 | 1.235 ± 0.019 | 1.49 ± 0.031 |
| Solvent | Temperature/K b | ||||
| 303.15 | 308.15 | 313.15 | 318.15 | ||
| Cyclohexane c | 5.15 ± 0.13 | 6.34 ± 0.09 | 7.42 ± 0.21 | 8.59 ± 0.14 | |
| 1,4-dioxane d | 12.99 ± 0.18 | 14.8 ± 0.4 | 16.5 ± 0.4 | 19.3 ± 0.4 | |
| Chloroform d | 11.56 ± 0.15 | 12.65 ± 0.27 | 14.64 ± 0.32 | 16.00 ± 0.18 | |
| DMF d | 37.6 ± 0.7 | 41.9 ± 1.4 | 44.0 ± 1.0 | 46.8 ± 0.9 | |
| DMSO d | 58.8 ± 1.0 | 66.4 ± 2.0 | 72.4 ± 0.5 | 81.6 ± 0.4 | |
| NMP d | 2.34 ± 0.09 | 2.59 ± 0.09 | 2.83 ± 0.14 | 2.99 ± 0.09 | |
| 1-propanol d | 3.403 ± 0.025 | 3.99 ± 0.08 | 4.61 ± 0.09 | 5.11 ± 0.17 | |
| 1-butanol d | 2.73 ± 0.10 | 3.26 ± 0.06 | 4.09 ± 0.10 | 4.69 ± 0.13 | |
| 1-octanol d | 1.818 ± 0.026 | 2.21 ± 0.033 | 2.56 ± 0.08 | 2.93 ± 0.03 | |
| Sample | Polymorphic Transition | Fusion | Ref. | ||
|---|---|---|---|---|---|
| /K | /kJ·mol−1 | /K | /kJ·mol−1 | ||
| Original sample | 422.00.5 | 1.700.5 | 463.90.5 | 25.30.5 | [31] |
| 422.0 | 1.63 | 463.0 | 27.41 | [42] | |
| 420.05 | 1.63 | 461.45 | 28.10 | [43] | |
| 420.65 | 1.40 | 461.55 | 24.71 | [44] | |
| 421.11 | 1.52 | 462.11 | 25.47 | [33] | |
| Cyclohexane | − | − | 463.20.5 | This work | |
| 1,4-dioxane | − | − | 463.50.5 | This work | |
| Chloroform | − | − | 461.90.5 | This work | |
| DMF | − | − | 464.20.5 | This work | |
| DMSO | − | − | 463.70.5 | This work | |
| NMP | − | − | 464.30.5 | This work | |
| 1-propanol | − | − | 462.80.5 | This work | |
| 1-butanol | − | − | 463.10.5 | This work | |
| 1-octanol | − | − | 463.40.5 | This work | |
| Form | 428.25 | 1.3 | 461.1 | 26.1 | [41] |
| Form | 372.55 | 0.78 | 461.2 | 25.5 | [41] |
| Form | − | − | 461.1 | 26.5 | [41] |
| Form | 404.355 | 1.66 | 461.95 | 26.9 | [41] |
| Solvent | /kJ·mol−1 | /kJ·mol−1 | /kJ·mol−1 |
|---|---|---|---|
| Cyclohexane b | 25.08 ± 0.06 | 33.8 ± 1.5 | 8.7 ± 1.5 |
| 1,4-dioxane c | 10.980 ± 0.027 | 21.2 ± 0.8 | 10.2 ± 0.8 |
| Chloroform d | 11.65 ± 0.07 | 25.6 ± 1.6 | 13.9 ± 1.6 |
| DMF d | 8.387 ± 0.024 | 14.0 ± 0.6 | 5.7 ± 0.6 |
| DMSO e | 7.083 ± 0.021 | 17.8 ± 0.8 | 10.8 ± 0.8 |
| NMP d | 15.35 ± 0.03 | 17.0 ± 0.6 | 1.7 ± 0.6 |
| 1-propanol d | 14.66 ± 0.04 | 27.5 ± 0.8 | 12.8 ± 0.8 |
| 1-butanol d | 15.212 ± 0.02 | 30.9 ± 0.5 | 15.7 ± 0.5 |
| 1-octanol d | 16.11 ± 0.016 | 26.5 ± 0.4 | 10.4 ± 0.4 |
| Solvent | /kJ·mol−1 | /kJ·mol−1 | /kJ·mol−1 |
|---|---|---|---|
| cyclohexane b | 16.74 ± 0.08 | 17.4 ± 1.6 | 0.7 ± 1.6 |
| 1,4-dioxane c | 2.72 ± 0.06 | 4.7 ± 1 | 2.0 ± 1.0 |
| Chloroform d | 3.25 ± 0.08 | 9.4 ± 1.7 | 6.1 ± 1.7 |
| DMF d | −0.02 ± 0.06 | −2.2 ± 0.8 | −2.2 ± 0.8 |
| DMSO e | −1.11 ± 0.05 | 1.2 ± 0.9 | 2.3 ± 0.9 |
| NMP d | −1.32 ± 0.06 | 0.8 ± 0.8 | 2.1 ± 0.8 |
| 1-propanol d | 6.25 ± 0.06 | 11.3 ± 1 | 5.0 ± 1.0 |
| 1-butanol d | 6.81 ± 0.05 | 14.7 ± 0.7 | 7.9 ± 0.7 |
| 1-octanol d | 7.71 ± 0.05 | 10.3 ± 0.6 | 2.6 ± 0.6 |
| Group | n | Hoftyzer-van Krevelen Parameters | ||
|---|---|---|---|---|
| −CH= | 3 | 0 a | 0a | |
| >C= | 1 | 70 a | 0 a | 0 a |
| Ring | 1 | 190 a | 0 b | 0 b |
| −N= | 2 | = 10,000 | ||
| −CO− | 1 | 290 a | 2000 a | |
| −NH2 | 1 | 280 a | 8400 a | |
| ∑ | 1470 | 1,969,000 | 15,400 a | |
| (MPa1/2) | (1,969,000)1/2 | (15,400/71.9)1/2 | ||
| MPa1/2 | ||||
| Solvent | Hansen Solubility Parameters a | ||||
|---|---|---|---|---|---|
| /MPa0.5 | 0.5 | 0.5 | 0.5 | ||
| Cyclohexane | 16.8 | 0.0 | 0.2 | 16.8 | −9.943 |
| Chloroform | 17.8 | 3.1 | 5.7 | 18.9 | −4.620 |
| 1,4-dioxane | 19.0 | 1.8 | 7.4 | 20.5 | −4.458 |
| Acetonitrile | 15.3 | 18.0 | 6.1 | 24.4 | −5.831 b |
| Water | 15.6 | 16.0 | 42.3 | 47.8 | −5.644 c |
| DMF | 17.4 | 13.7 | 11.3 | 24.9 | −3.337 |
| DMSO | 18.4 | 16.4 | 10.2 | 26.7 | −2.992 |
| NMP | 18.0 | 12.3 | 7.2 | 23.0 | −6.153 |
| Methanol | 15.1 | 12.3 | 22.3 | 29.6 | −5.36 b |
| Ethanol | 15.8 | 8.8 | 19.4 | 26.5 | −5.960 c |
| 1-propanol | 16.0 | 6.8 | 17.4 | 24.6 | −5.843 |
| 2-propanol | 15.8 | 7.2 | 16.0 | 23.6 | −6.101 b |
| 1-butanol | 16.0 | 5.7 | 15.8 | 23.2 | −6.119 |
| 2-butanol | 15.8 | 5.7 | 14.5 | 22.2 | −6.277 b |
| 1-octanol | 17.0 | 3.3 | 11.9 | 21.0 | −6.509 |
| Chemical Name | CAS a | Purity in Mass Fraction | Analytic Technique b |
|---|---|---|---|
| pyrazinamide c | 98-96-4 | >0.990 | HPLC |
| ethanol c | 64-17-5 | 0.998 | GC |
| cyclohexane d | 110-82-7 | 0.998 | GC |
| 1,4-dioxane d | 123-91-1 | 0.998 | GC |
| chloroform d | 67-66-3 | 0.998 | GC |
| N,N-dimethylformamide (DMF) d | 68-12-2 | 0.998 | GC |
| dimethyl sulfoxide (DMSO) d | 67-68-5 | 0.998 | GC |
| N-Methyl-2-pyrrolidone (NMP) d | 872-50-4 | 0.998 | GC |
| 1-propanol e | 71-23-8 | 0.998 | GC |
| 1-butanol e | 71-36-3 | 0.998 | GC |
| 1-octanol e | 111-87-5 | 0.998 | GC |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tovar-Amézquita, J.; Rincón-Guio, C.; Torres-Suarez, F.E.; Florez, M.M.; Ortiz, C.P.; Martinez, F.; Delgado, D.R. Thermodynamic Assessment of the Pyrazinamide Dissolution Process in Some Organic Solvents. Molecules 2024, 29, 5089. https://doi.org/10.3390/molecules29215089
Tovar-Amézquita J, Rincón-Guio C, Torres-Suarez FE, Florez MM, Ortiz CP, Martinez F, Delgado DR. Thermodynamic Assessment of the Pyrazinamide Dissolution Process in Some Organic Solvents. Molecules. 2024; 29(21):5089. https://doi.org/10.3390/molecules29215089
Chicago/Turabian StyleTovar-Amézquita, Jesus, Cristian Rincón-Guio, Francy Elaine Torres-Suarez, Magda Melissa Florez, Claudia Patricia Ortiz, Fleming Martinez, and Daniel Ricardo Delgado. 2024. "Thermodynamic Assessment of the Pyrazinamide Dissolution Process in Some Organic Solvents" Molecules 29, no. 21: 5089. https://doi.org/10.3390/molecules29215089
APA StyleTovar-Amézquita, J., Rincón-Guio, C., Torres-Suarez, F. E., Florez, M. M., Ortiz, C. P., Martinez, F., & Delgado, D. R. (2024). Thermodynamic Assessment of the Pyrazinamide Dissolution Process in Some Organic Solvents. Molecules, 29(21), 5089. https://doi.org/10.3390/molecules29215089









