Experimental and Theoretical Electron Paramagnetic Resonance (EPR) Study on the Temperature-Dependent Structural Changes of Methylsulfanylmethane
Abstract
:1. Introduction
1.1. Theoretical Considerations
1.2. Computational Details
2. Results and Discussion
2.1. Room Temperature Spectra
2.2. High Temperature Spectra
2.3. Low Temperature Spectra
3. Experimental Section
4. Conclusions
- At higher temperatures near to melting point (109 °C) the line widths became narrower and lines became sharper but the spectra did not change appreciably.
- When the temperature was decreased between −50 °C and −160 °C, the central intense line split into 1:3:3:1 pattern with constant value of 1.3 mT. The average g value was measured to be 2.0115 which showed that the CH3 group keeps rotating, and 33S lines became smaller and lay under the intense lines. Calculations showed that the unpaired electron population shifted toward the CH3 group.
- When the temperature was decreased below 180 °C, the spectra converted to anisotropic two different 1:1 patterns with average g value of 2.0058, and average hyperfine values of 1.6 mT and 0.43 mT which showed that the CH3 group stopped rotation. Estimations on the structure with the help of molecular orbital calculations showed that one of the oxygen atoms of the radical gets closer to CH3 group and the other one goes away. The closer oxygen atom polarizes the unpaired electron distribution on the CH3 group and as a result the distribution on one of the hydrogen atoms becomes too small and on the other two oxygen atoms it becomes unequal.
Supporting information
Acknowledgments
References
- Tsuruta, Y; Ito, Y; Harada, K; Narita, Y; Ohbayashi, T; Azekura, H; Fukagawa, M; Narita, M; Maeda, K. Measurements of blood DMSO and DMSO2 in a healthy person and a hemodialysis patient. Clin. Exp. Nephrol. 2001, 5, 158–162. [Google Scholar]
- Williams, KIH; Burstein, SH; Layne, DS. Dimethyl sulfone: Isolation from cows’ milk. Proc. Soc. Exp. Biol. Med. 1996, 122, 865–866. [Google Scholar]
- Engelke, UF; Tangerman, A; Willemsen, MA; Moskau, D; Loss, S; Mudd, SH; Wevers, RA. Dimethyl sulfone in human cerebrospinal fluid and blood plasma confirmed by one-dimensional (1)H and two-dimensional (1)H-(13)C NMR. NMR Biomed 2005, 18, 331–336. [Google Scholar]
- Rose, SE; Chalk, JB; Galloway, GJ; Doddrell, DM. Detection of dimethyl sulfone in the human brain by in vivo proton magnetic resonance spectroscopy. Magn. Reson. Imaging 2000, 18, 95–98. [Google Scholar]
- Hucker, HB; Ahmed, PM; Miller, EA; Brobyn, R. Metabolism of dimethyl sulphoxide to dimethyl sulphone in the rat and man. Nature 1966, 619–620. [Google Scholar]
- Murav’ev, IuV; Venikova, MS; Pleskovskaia, GN; Riazantseva, TA; Sigidin, IaA. Effect of dimethyl sulfoxide and dimethyl sulfone on a destructive process in the joints of mice with spontaneous arthritis. Patol. Fiziol. Eksp. Ter. 1991, 2, 37–39. [Google Scholar]
- Jacob, SW; Herschler, R. Pharmacology of DMSO. Cryobiology 1986, 23, 14–27. [Google Scholar]
- Jacob, SW; Wood, DC. Dimethyl sulfoxide (DMSO) toxicology, pharmacology, and clinical experience. Am. J. Surg. 1967, 114, 414–426. [Google Scholar]
- Milne, PJ; Zica, RG; Saltzman, ES. biogenic sulfur in the environment. Am. Chem. Soc. Symp. Ser. 1989, 393, 518–528. [Google Scholar]
- Ramírez-Anguita, JM; González-Lafont, A; Lluch, JM. A theoretical study of the DMS.OH scavenging reaction by OH. Its relevance in DMSO formation. Comput. Theor. Chem. 2011, 965, 249–258. [Google Scholar]
- Kojima, T; Mitaka, T; Mizuguchi, T; Mochizuki, Y. Effects of oxygen radical scavengers on connexins 32 and 26 expression in primary cultures of adult rat hepatocytes. Carcinogenesis 1996, 17, 537–544. [Google Scholar]
- Herscu-Kluska, R; Masarwa, A; Saphier, M; Cohen, H; Meyerstein, D. Mechanism of the reaction of radicals with peroxides and dimethyl sulfoxide in aqueous solution. Chem. Eur. J. 2008, 14, 5880–5889. [Google Scholar]
- Becker, D; Swarts, S; Champagne, M; Sevilla, MD. An ESR investigation of the reactions of glutathione, cysteine and penicillamine thiyl radicals: Competitive formation of RSO., R., RSSR-., and RSS(.). Int. J. Radiat. Biol. Relat. Stud. Phys. Chem. Med. 1988, 53, 767–786. [Google Scholar]
- Andersen, RS. Electron spin resonance study of X-irradiated single crystals of dimethyl sulfone. J. Chem. Phys 1977, 66, 5610–5613. [Google Scholar]
- Kasai, PH. Dissociative electron capture of sulfones and sulfonates: Matrix isolation ESR study. J. Am. Chem. Soc. 1991, 113, 3317–3321. [Google Scholar]
- Nishikida, K; Williams, F. Angular dependence of proton hyperfine splittings in the electron spin resonance spectrum of the methylsulfinyl radical. J. Am. Chem. Soc. 1974, 96, 4781–4784. [Google Scholar]
- Ciofini, I; Adamo, C; Barone, V. Complete structural and magnetic characterization of biological radicals in solution by an integrated quantum mechanical approach: Glycyl radical as a case study. J. Chem. Phys. 2004, 121, 6710–6718. [Google Scholar]
- Atherton, NM. Electron Spin Resonance: Theory and Applications; John-Wiley and Sons: New York, NY, USA, 1973. [Google Scholar]
- Weil, JA; Bolton, JR. Electron Paramagnetic Resonance, Elementary Theory and Practical Applications, 2nd ed.; John Wiley and Sons, Inc.: Hoboken, NJ, USA, 2007. [Google Scholar]
- Rieger, PH. Electron Spin Resonance, Analysis and Interpretation; The Royal Society of Chemistry Publishing: Cambridge, UK, 2007. [Google Scholar]
- Harriman, JE. The Theorethical Foundations of Electron Spin Resonance (Physical Chemistry); Academic Press Inc.: London, UK, 1978. [Google Scholar]
- Munzarova, ML. Calculation of NMR and EPR Parameter, Part D: DFT Calculation of EPR Hyperfine Coupling Tensors; Kaupp, M, Bühl, M, Malkin, VG, Eds.; Wiley-Vch: Weinheim, Germany, 2004. [Google Scholar]
- Neese, F. Prediction of electron paramagnetic resonance g-values by coupled perturbed Hartree-Fock and Kohn-Sham theory. J. Chem. Phys 2001, 115, 11080–11096. [Google Scholar]
- Estebes, MC; Rocha, AB; Vugman, NV; Bielschowsky, CE. DFT calculation of EPR parameters of antisite defect in gallium arsenide. Chem. Phys. Lett. 2008, 453, 188–191. [Google Scholar]
- Angstl, R. Contribution of the relativistic mass correction to the g-tensor of molecules. Chem. Phys. 1989, 132, 435–442. [Google Scholar]
- Stone, AJ. Gauge invariance of the g-tensor. Proc. R. Soc. Lond., Ser. A 1963, 271, 424–434. [Google Scholar]
- Langs, DA; Silverton, JV; Bright, WM. Chemical analysis by x-ray crystallography—structure of dimethyl sulphone. J. Chem. Soc. D 1970, 24, 1653–1654. [Google Scholar]
- McLachlan, RD; Carter, VB. Vibrational spectra of crystalline dimethyl sulfone. Spectrochim. Acta A. 1970, 26, 1121–1127. [Google Scholar]
- Oberhammer, H; Zeil, W. Molecular structure of dimethyl sulfone as determined by gas electron diffraction. J. Mol. Struct. 1970, 6, 399–404. [Google Scholar]
- Godbout, N; Salahub, DR; Andzelm, J; Wimmer, E. Optimization of gaussian type basis sets for local spin density functional calculations. Part I. Boron through neon, optimization technique and validation. Can. J. Chem. 1992, 70, 560–571. [Google Scholar]
- Hermosilla, L; Calle, P; Sieiro, C. Assignments of hyperfine splittings by DFT methods of radicals containing 33S (I = 3/2), 31P(I = 1/2), and 29Si (I = 1/2) Nuclei. Phosphorus Sulfur Silicon 2005, 180, 1421–1422. [Google Scholar]
- Gaussian 03, Revision E.01; Frisch, MJ; Trucks, GW; Schlegel, HB; Scuseria, GE; Robb, MA; Cheeseman, JR; Montgomery, JA, Jr; Vreven, T; Kudin, KN; Burant, JC; et al. (Eds.) Gaussian, Inc.: Pittsburgh, PA, USA, 2003; Available online: http://www.bear.bham.ac.uk/bluebear/applications/g03_E01.shtml (accessed on 21 July 2011).
- Atkins, PW; Horsfield, A; Symons, MCR. Oxides and oxyions of the non-metals. Part VII. SO2− and ClO2. J. Chem. Soc 1964, 5220–5225. [Google Scholar]
- Huie, RE; Clifton, CL; Altstein, NA. A pulse radiolysis and flash photolysis study of the radicals SO2−, SO3−, SO4− and SO5−. Radiat. Phys. Chem. 1989, 33, 361–370. [Google Scholar]
- Swarts, SG; Becker, D; DeBolt, S; Sevilla, MD. Electron spin resonance investigation of the structure and formation of sulfinyl radicals: reaction of peroxyl radicals with thiols. J. Phys. Chem 1989, 93, 155–161. [Google Scholar]
- Chipman, DM. Quantum Mechanical Electronic Structure Calculations with Chemical Accuracy; Kluwer Academic Press: Dordrecht, The Netherlands, 1995. [Google Scholar]
- Dmitriev, YuA; Zhidnikov, RA. EPR study of methyl radicals. anisotropy and tumbling motion in low-temperature matrices. J. Low Temp. Phys 2001, 122, 163–170. [Google Scholar]
- Givan, A; Grothe, H; Loewenschuss, A. Spectral evidence of solid state interactions in mixed dimethyl sulfone-water ices. J. Mol. Struct. 2003, 648, 159–169. [Google Scholar]
- McLachlan, RD; Carter, VB. Vibrational spectra of crystalline dimethyl sulfone. Spectrachim. Acta A 1970, 26, 1121–1127. [Google Scholar]

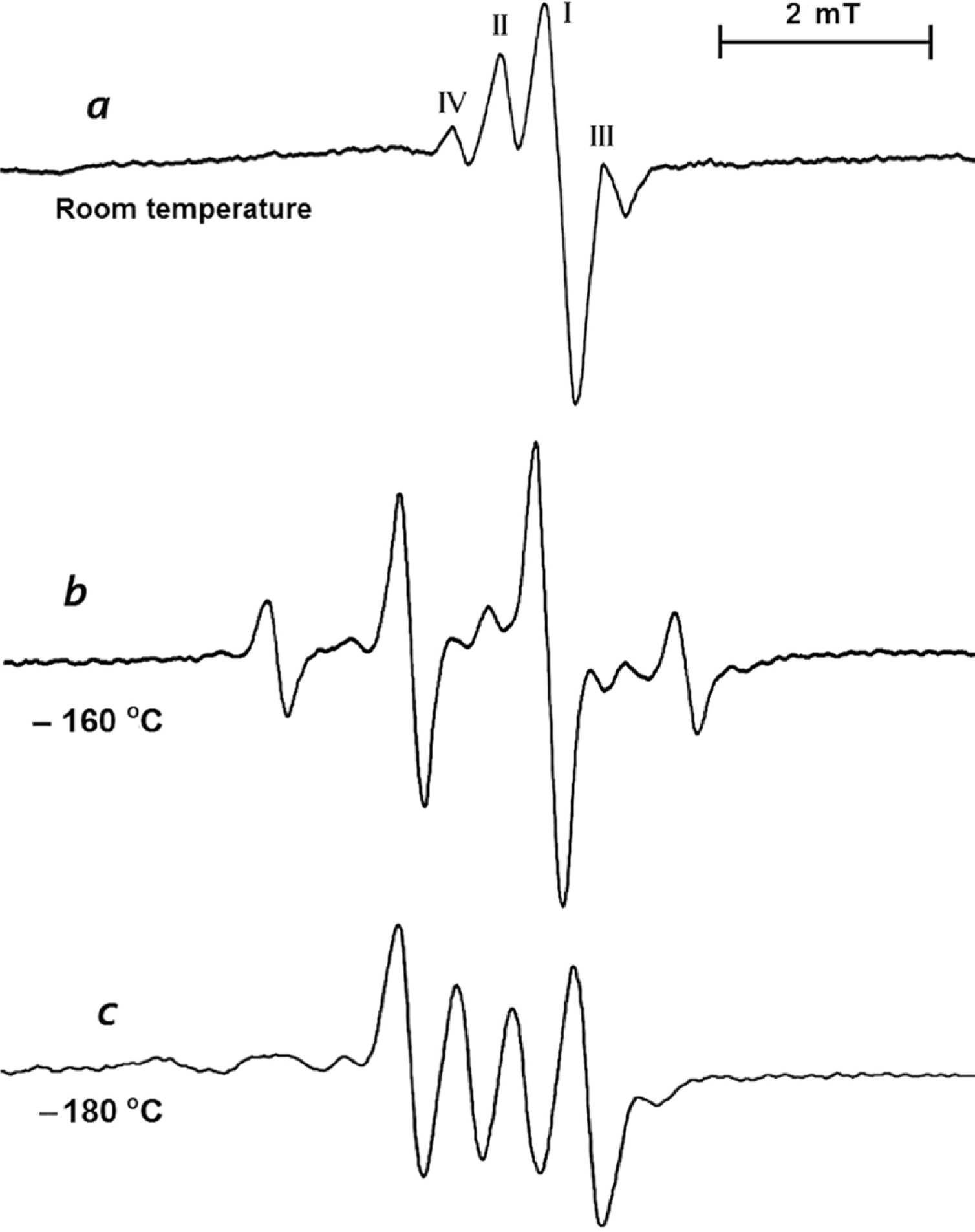



| Room temperature spectra | ||||
| Measured g values of line I, II–III doublet and 33S lines | Comment | |||
| g// = 2.0036 | g⊥ = 2.0075 | < g >=2.0062 | II–III doublet splitting is 1.2 mT (constant) | |
| Measured g values of line IV | ||||
| g// = 2.0143 | g⊥ = 2.0078 | < g >=2.0010 | ||
| Hyperfine coupling constants of 33S lines (mT). | ||||
| A// = 9.1 | A⊥ = 6.2 | < A >= 7.2 | g values are the same as those of line I. | |
| Spectra at −160 °C | ||||
| gx = 2.0076 | gy = 2.0109 | gz = 2.0160 | < g >=2.0115 | Hyperfine coupling constant of three equivalent methyl protons are isotropic with the value of 1.3 mT. 33S hyperfine lines could not be detected. |
| Spectra below −180 °C | ||||
| A// = 2.6 | A⊥ = 1.1 | < A >=1.6 | For one of the CH3 hydrogen atoms | |
| A// = 0.3 | A⊥ = 0.5 | < A >=0.43 | For other hydrogen atom | |
| g// = 2.0037 | g⊥ = 2.0069 | < g >= 2.0058 | HFCC of third hydrogen atom is too small to measure. 33S hyperfine lines could not be detected. | |
| R1 | R2 | R3 | |||
| Atom | Aiso | Atom | Aiso | Atom | Aiso |
| S1 | 6.37 | S1 | 4.33 | S1 | 0.51 |
| H7 | −0.33 | H3 | 2.17 | H3 | 0.47 |
| H8 | −0.33 | H4 | −0.14 | H4 | −0.31 |
| H9 | 0.82 | H5 | 0.03 | H5 | 0.28 |
| H7 | 2.17 | H7 | 0.47 | ||
| H8 | 0.03 | H8 | 0.28 | ||
| H9 | −0.17 | H9 | −0.31 | ||
| < A(CH3) > | 0.49 | 0.79 | 0.35 | ||
| < g > | 2.0063 | 2.0089 | 2.0047 | ||
| R4 and R4* (in parenthesis) | R5 | R6 | |||
| Atom | Aiso | Atom | Aiso | Atom | Aiso |
| S1 | 0.87 (0.80) | S1 | 1.45 | S1 | 1.08 |
| H7 | 1.60 (1.50) | H7 | 0.74 | H7 | 0.84 |
| H8 | 1.59 (2.01) | H8 | 0.74 | H8 | 0.84 |
| H9 | −0.05 (0.08) | H9 | 0.43 | H9 | 0.50 |
| < A(CH3) > | 1.08 (1.20) | 0.64 | 0.73 | ||
| < g > | 2.0120 (2.009) | 2.0178 | 2.0276 | ||
© 2011 by the authors; licensee MDPI, Basel, Switzerland. This article is an open-access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Tapramaz, R.; Türkkan, E.; Dereli, Ö. Experimental and Theoretical Electron Paramagnetic Resonance (EPR) Study on the Temperature-Dependent Structural Changes of Methylsulfanylmethane. Int. J. Mol. Sci. 2011, 12, 4909-4922. https://doi.org/10.3390/ijms12084909
Tapramaz R, Türkkan E, Dereli Ö. Experimental and Theoretical Electron Paramagnetic Resonance (EPR) Study on the Temperature-Dependent Structural Changes of Methylsulfanylmethane. International Journal of Molecular Sciences. 2011; 12(8):4909-4922. https://doi.org/10.3390/ijms12084909
Chicago/Turabian StyleTapramaz, Recep, Ercan Türkkan, and Ömer Dereli. 2011. "Experimental and Theoretical Electron Paramagnetic Resonance (EPR) Study on the Temperature-Dependent Structural Changes of Methylsulfanylmethane" International Journal of Molecular Sciences 12, no. 8: 4909-4922. https://doi.org/10.3390/ijms12084909
APA StyleTapramaz, R., Türkkan, E., & Dereli, Ö. (2011). Experimental and Theoretical Electron Paramagnetic Resonance (EPR) Study on the Temperature-Dependent Structural Changes of Methylsulfanylmethane. International Journal of Molecular Sciences, 12(8), 4909-4922. https://doi.org/10.3390/ijms12084909





