The Effect of Cadmium on GFR Is Clarified by Normalization of Excretion Rates to Creatinine Clearance
Abstract
1. Introduction
2. Results
2.1. Tabular Analyses
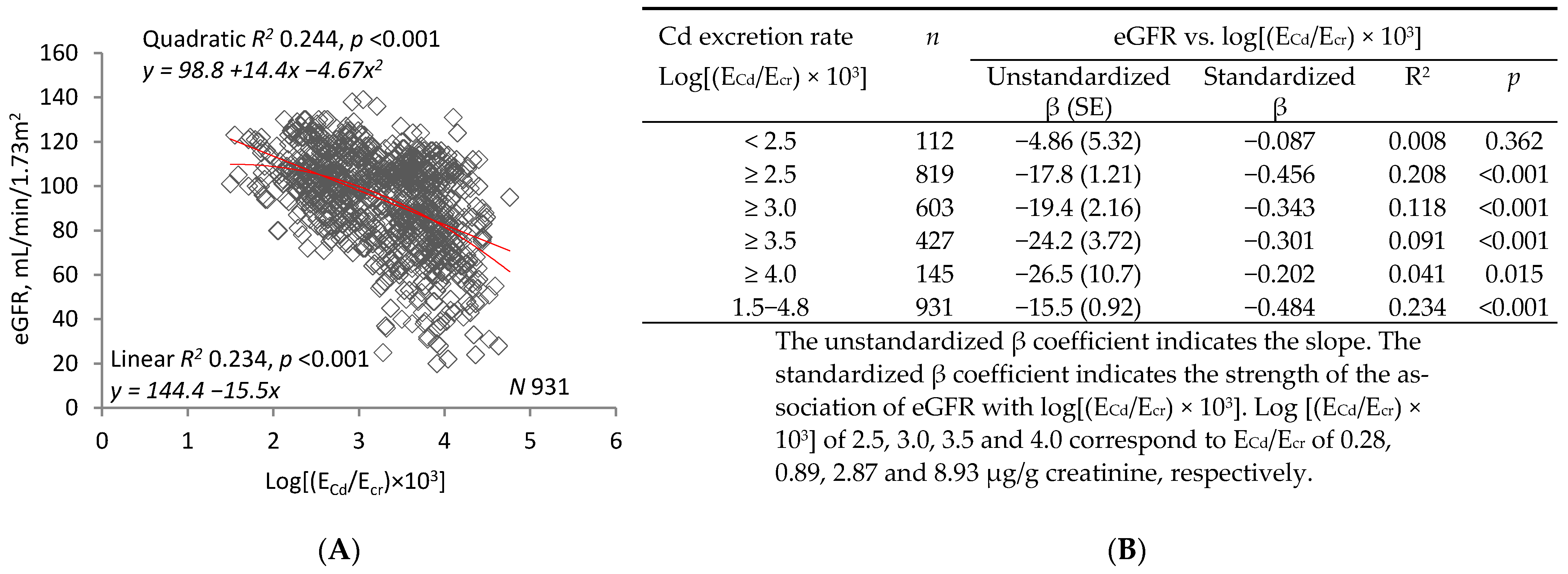
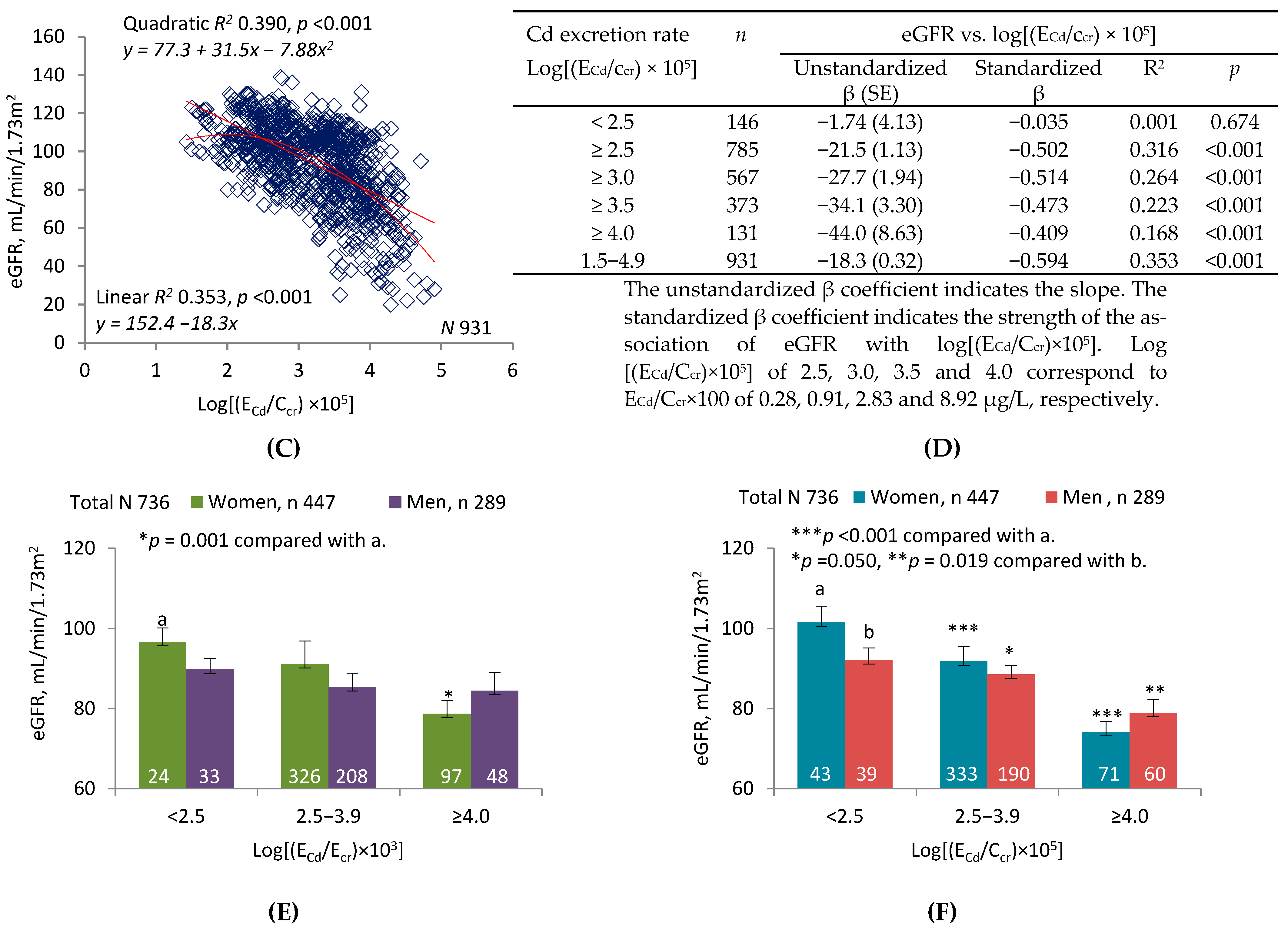
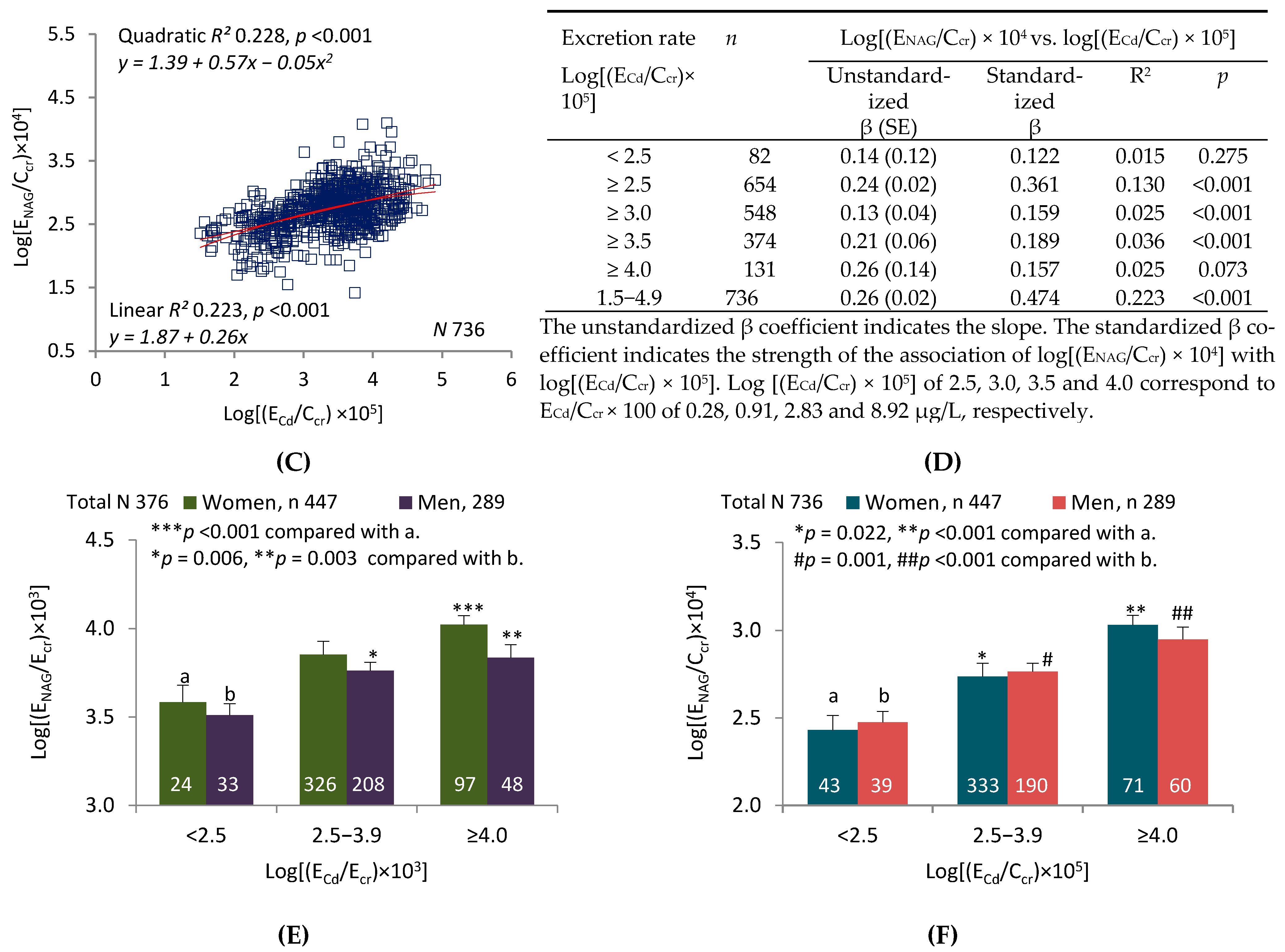
2.2. Graphic Analyses
3. Discussions
3.1. Interpretation of Tabular Analyses
3.2. Interpretation of Graphic Analyses
3.3. Creatinine Excretion, Creatinine Clearance, and GFR
3.4. Tubular Release of Cd and NAG Necessitates Normalization of Excretion Rates to Ccr
4. Materials and Methods
4.1. Study Population
4.2. Specimen Collection and Analysis
4.3. Normalization of Excretion Rates to Ecr and Ccr
4.4. Estimated Glomerular Filtration Rates (eGFR)
4.5. Statistical Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| GFR | Glomerular filtration rate, mL/min |
| eGFR | Estimated glomerular filtration rate, mL/min/1.73m2 (of body surface area) |
| CKD-EPI | Chronic kidney disease epidemiology collaboration |
| Cd | Cadmium |
| NAG | N-acetyl-β-D-glucosaminidase |
| Cr | Creatinine |
| Ccr | Creatinine clearance (a surrogate for GFR), mL/min |
| Vu | Rate of urine flow, volume/time |
| [x]u | Urine concentration of substance x (cr, Cd, or NAG), mass/volume |
| Ex | Excretion rate of substance x (cr, Cd, or NAG), mass/time |
| Ex/Ecr | Amount of x excreted per gram of creatinine excreted, mass/mass |
| [x]u/[cr]u | Ratio of urine concentration of x to urine concentration of cr, mass/mass |
| Ex/Ccr | Amount of x excreted per volume of filtrate, mass/volume |
| MT | Metallothionein |
| CdMT | Cd-metallothionein complex |
| POR | Probability odds ratio |
References
- Satarug, S. Dietary cadmium intake and its effects on kidneys. Toxics 2018, 6, 15. [Google Scholar] [CrossRef]
- Satarug, S.; Phelps, K.R. Cadmium Exposure and Toxicity. In Metal Toxicology Handbook; Bagchi, D., Bagchi, M., Eds.; CRC Press: Boca Raton, FL, USA, 2021; pp. 219–274. [Google Scholar]
- Satarug, S.; Gobe, G.C.; Vesey, D.A.; Phelps, K.R. Cadmium and lead exposure, nephrotoxicity, and mortality. Toxics 2020, 8, 86. [Google Scholar] [CrossRef]
- Price, R.G. Measurement of N-acetyl-beta-glucosaminidase and its isoenzymes in urine: Methods and clinical applications. Eur. J. Clin. Chem. Clin. Biochem. 1992, 30, 693–705. [Google Scholar] [PubMed]
- Kawada, T.; Koyama, H.; Suzuki, S. Cadmium, NAG activity, and β2-microglobulin in the urine of cadmium pigment workers. Br. J. Ind. Med. 1989, 46, 52–55. [Google Scholar] [PubMed]
- Kawada, T.; Shinmyo, R.R.; Suzuki, S. Urinary cadmium and N-acetyl-β-D-glucosaminidase excretion of inhabitants living in a cadmium-polluted area. Int. Arch. Occup. Environ. Health 1992, 63, 541–546. [Google Scholar] [CrossRef] [PubMed]
- Swaddiwudhipong, W.; Limpatanachote, P.; Nishijo, M.; Honda, R.; Mahasakpan, P.; Krintratun, S. Cadmium-exposed population in Mae Sot District, Tak Province: 3. Associations between urinary cadmium and renal dysfunction, hypertension, diabetes, and urinary stones. J. Med. Assoc. Thai. 2010, 93, 231–238. [Google Scholar] [PubMed]
- Zhang, Y.R.; Wang, P.; Liang, X.X.; Tan, C.S.; Tan, J.B.; Wang, J.; Huang, Q.; Huang, R.; Li, Z.X.; Chen, W.C.; et al. Associations between urinary excretion of cadmium and renal biomarkers in non-smoking females: A cross-sectional study in rural areas of South China. Int. J. Environ. Res. Public Health 2015, 12, 11988–12001. [Google Scholar] [CrossRef] [PubMed]
- Kim, Y.D.; Yim, D.H.; Eom, S.Y.; Moon, S.I.; Park, C.H.; Kim, G.B.; Yu, S.D.; Choi, B.S.; Park, J.D.; Kim, H. Temporal changes in urinary levels of cadmium, N-acetyl-beta-d-glucosaminidase and beta2-microglobulin in individuals in a cadmium-contaminated area. Environ. Toxicol. Pharmacol. 2015, 39, 35–41. [Google Scholar] [CrossRef]
- Eom, S.Y.; Seo, M.N.; Lee, Y.S.; Park, K.S.; Hong, Y.S.; Sohn, S.J.; Kim, Y.D.; Choi, B.S.; Lim, J.A.; Kwon, H.J.; et al. Low-level environmental cadmium exposure induces kidney tubule damage in the general population of Korean adults. Arch. Environ. Contam. Toxicol. 2017, 73, 401–409. [Google Scholar] [CrossRef] [PubMed]
- Jin, Y.; Lu, Y.; Li, Y.; Zhao, H.; Wang, X.; Shen, Y.; Kuang, X. Correlation between environmental low-dose cadmium exposure and early kidney damage: A comparative study in an industrial zone vs. a living quarter in Shanghai, China. Environ. Toxicol. Pharmacol. 2020, 79, 103381. [Google Scholar] [CrossRef]
- Bernard, A.; Thielemans, N.; Roels, H.; Lauwerys, R. Association between NAG-B and cadmium in urine with no evidence of a threshold. Occup. Environ. Med. 1995, 52, 177–180. [Google Scholar] [CrossRef] [PubMed]
- Noonan, C.W.; Sarasua, S.M.; Campagna, D.; Kathman, S.J.; Lybarger, J.A.; Mueller, P.W. Effects of exposure to low levels of environmental cadmium on renal biomarkers. Environ. Health Perspect. 2002, 110, 151–155. [Google Scholar] [CrossRef]
- Thomas, D.K.; Hodgson, S.; Nieuwenhuijsen, M.; Jarup, L. Early kidney damage in a population exposed to cadmium and other heavy metals. Environ. Health Perspect. 2009, 117, 181–184. [Google Scholar] [CrossRef] [PubMed]
- Satarug, S.; Vesey, D.A.; Ruangyuttikarn, W.; Nishijo, M.; Gobe, G.C.; Phelps, K.R. The source and pathophysiologic significance of excreted cadmium. Toxics 2019, 7, 55. [Google Scholar] [CrossRef] [PubMed]
- Jarup, L.; Persson, B.; Elinder, C.G. Decreased glomerular filtration rate in solderers exposed to cadmium. Occup. Environ. Med. 1995, 52, 818–822. [Google Scholar] [CrossRef]
- Honda, R.; Swaddiwudhipong, W.; Nishijo, M.; Mahasakpan, P.; Teeyakasem, W.; Ruangyuttikarn, W.; Satarug, S.; Padungtod, C.; Nakagawa, H. Cadmium induced renal dysfunction among residents of rice farming area downstream from a zinc-mineralized belt in Thailand. Toxicol. Lett. 2010, 198, 26–32. [Google Scholar] [CrossRef]
- Heymsfield, S.B.; Arteaga, C.; McManus, C.; Smith, J.; Moffitt, S. Measurement of muscle mass in humans: Validity of the 24-hour urinary creatinine method. Am. J. Clin. Nutr. 1983, 37, 478–494. [Google Scholar] [CrossRef] [PubMed]
- Weaver, V.M.; Garcia Vargas, G.; Silbergeld, E.K.; Rothenberg, S.J.; Fadrowski, J.J.; Rubio-Andrade, M.; Parsons, P.J.; Steuerwald, A.J.; Navas-Acien, A.; Guallar, E. Impact of urine concentration adjustment method on associations between urine metals and estimated glomerular filtration rates (eGFR) in adolescents. Environ. Res. 2014, 132, 226–232. [Google Scholar] [CrossRef]
- Buser, M.C.; Ingber, S.Z.; Raines, N.; Fowler, D.A.; Scinicariello, F. Urinary and blood cadmium and lead and kidney function: NHANES 2007-2012. Int. J. Hyg. Environ. Health 2016, 219, 261–267. [Google Scholar] [CrossRef]
- Jin, R.; Zhu, X.; Shrubsole, M.J.; Yu, C.; Xia, Z.; Dai, Q. Associations of renal function with urinary excretion of metals: Evidence from NHANES 2003-2012. Environ. Int. 2018, 121, 1355–1362. [Google Scholar] [CrossRef]
- Satarug, S.; Boonprasert, K.; Gobe, G.C.; Ruenweerayut, R.; Johnson, D.W.; Na-Bangchang, K.; Vesey, D.A. Chronic exposure to cadmium is associated with a marked reduction in glomerular filtration rate. Clin. Kidney J. 2018, 12, 468–475. [Google Scholar] [CrossRef] [PubMed]
- Phelps, K.R.; Gosmanova, E.O. A generic method for analysis of plasma concentrations. Clin. Nephrol. 2020, 94, 43–49. [Google Scholar] [CrossRef] [PubMed]
- Levey, A.S.; Stevens, L.A.; Scmid, C.H.; Zhang, Y.; Castro, A.F., III; Feldman, H.I.; Kusek, J.W.; Eggers, P.; Van Lente, F.; Greene, T.; et al. Greene, T.; et al. A new equation to estimate glomerular filtration rate. Ann. Intern. Med. 2009, 150, 604–612. [Google Scholar] [CrossRef]
- Mitch, W.E.; Walser, M. A proposed mechanism for reduced creatinine excretion in severe chronic renal failure. Nephron 1978, 21, 248–254. [Google Scholar] [CrossRef]
- Mitch, W.E.; Collier, V.U.; Walser, M. Creatinine metabolism in chronic renal failure. Clin. Sci. 1980, 58, 327–335. [Google Scholar] [CrossRef] [PubMed]
- Soveri, I.; Berg, U.B.; Bjork, J.; Elinder, C.-G.; Grubb, A.; Mejare, I.; Sterner, G.; Back, S.-E. Measuring GFR: A systematic review. Am. J. Kidney Dis. 2014, 64, 411–424. [Google Scholar] [CrossRef] [PubMed]
- Satarug, S.; Baker, J.R.; Reilly, P.E.B.; Moore, M.R.; Williams, D.J. Cadmium levels in the lung, liver, kidney cortex, and urine samples from Australians without occupational exposure to metals. Arch. Environ. Health 2002, 57, 69–77. [Google Scholar] [CrossRef]
- Akerstrom, M.; Barregard, L.; Lundh, T.; Sallsten, G. The relationship between cadmium in kidney and cadmium in urine and blood in an environmentally exposed population. Toxicol. Appl. Pharmacol. 2013, 268, 286–293. [Google Scholar] [CrossRef]
- Nomiyama, K.; Foulkes, C. Reabsorption of filtered cadmium-metallothionein in the rabbit kidney. Proc. Soc. Exp. Biol. Med. 1977, 156, 97–99. [Google Scholar] [CrossRef] [PubMed]
- Schnaper, H.W. The tubulointerstitial pathophysiology of progressive kidney disease. Adv. Chron. Kidney Dis. 2017, 24, 107–116. [Google Scholar] [CrossRef]
- Simmons, R.W.; Pongsakul, P.; Saiyasitpanich, D.; Klinphoklap, S. Elevated levels of cadmium and zinc in paddy soils and elevated levels of cadmium in rice grain downstream of a zinc mineralized area in Thailand: Implications for public health. Environ. Geochem. Health 2005, 27, 501–511. [Google Scholar] [CrossRef] [PubMed]
- Satarug, S.; Swaddiwudhipong, W.; Ruangyuttikarn, W.; Nishijo, M.; Ruiz, P. Modeling cadmium exposures in low- and high-exposure areas in Thailand. Environ. Health Perspect. 2013, 121, 531–536. [Google Scholar] [CrossRef] [PubMed]
- Swaddiwudhipong, W.; Nguntra, P.; Kaewnate, Y.; Mahasakpan, P.; Limpatanachote, P.; Aunjai, T.; Jeekeeree, W.; Punta, B.; Funkhiew, T.; Phopueng, I. Human health effects from cadmium exposure: Comparison between persons living in cadmium-contaminated and non-contaminated areas in northwestern Thailand. Southeast Asian J. Trop. Med. Public Health 2015, 46, 133–142. [Google Scholar] [PubMed]


| Parameters/Factors | All Subjects | Log[(ECd/Ccr) × 105] | ||
|---|---|---|---|---|
| n = 931 | <2.5, n = 146 | 2.5−3.9, n = 654 | ≥4.0, n = 131 | |
| Age (years) | 44.4 ± 12.5 | 31.9 ± 9.1 | 44.8 ±10.9 | 56.3 ± 10.8 * |
| Age range | 16−87 | 16−53 | 18−87 | 36−83 |
| eGFR (mL/min/1.73 m2) a | 93.8 ± 20.8 | 109.3 ± 12.0 | 95.3 ± 17.7 | 69.1 ± 21.8 * |
| eGFR range | 20−139 | 78−130 | 25−139 | 20−112 |
| eGFR <60 mL/min/1.73 m2 (%) | 7.1 | 0 | 3.5 | 32.8 † |
| Women (%) | 58.5 | 54.1 | 60.4 | 54.2 |
| Smoking (%) | 39.2 | 19.2 | 29.8 | 58.8 † |
| Hypertension (%) | 25.5 | 8.2 | 28.8 | 26.0 † |
| Diabetes mellitus (%) | 1.2 | 0 | 0.6 | 5.3 † |
| Serum creatinine, mg/dL | 0.85 ± 0.26 | 0.80 ± 0.15 | 0.82 ± 0.20 | 1.08 ± 0.42 * |
| Urine creatinine, mg/dL | 81.1 ± 73.7 | 53.9 ± 63.0 | 86.7 ± 76.0 | 91.2 ± 63.9 * |
| Urine Cd, μg/L | 1.52 ± 8.32 | 0.11 ± 0.17 | 1.77 ± 3.98 | 13.57 ± 14.62 * |
| Urine NAG, units/L | 5.48 ± 9.95 | 1.73 ± 1.67 | 6.14 ± 10.70 | 7.19 ± 7.00 * |
| Normalized to Ecr as Ex/Ecr b | ||||
| ECd/Ecr, µg/g creatinine | 1.85 ± 6.23 | 0.19 ± 0.10 | 2.04 ± 2.81 | 14.88 ± 8.05 * |
| ENAG/Ecr, units/g creatinine | 6.40 ± 8.94 | 3.27 ± 2.39 | 6.74 ± 7.63 | 7.88 ± 13.97 * |
| Normalized to Ccr as Ex/Ccr c | ||||
| ECd/Ccr × 100, µg/L | 1.57 ± 7.23 | 0.15 ± 0.07 | 1.67 ± 2.24 | 16.01 ± 11.05 * |
| ENAG/Ccr × 100, units/L | 5.42 ± 9.39 | 2.47 ± 1.93 | 5.47 ± 7.62 | 8.48 ± 15.12 * |
| Independent Variables/Factors | eGFR Levels <60 mL/min/1.73 m2 | ||||
|---|---|---|---|---|---|
| β Coefficients | POR | 95% CI | p | ||
| (SE) | Lower | Upper | Value | ||
| Model 1, n = 736 | |||||
| Age (years) | 0.143 (0.018) | 1.153 | 1.113 | 1.195 | <0.001 |
| Log2 [(ECd/Ecr) × 103], µg/g creatinine | 0.493 (0.137) | 1.637 | 1.252 | 2.141 | <0.001 |
| Log2 [(ENAG/Ecr) × 103], units/g creatinine | 0.435 (0.172) | 1.544 | 1.103 | 2.163 | 0.011 |
| Diabetes | 0.705 (0.884) | 2.023 | 0.358 | 11.434 | 0.425 |
| Gender (women) | −0.014 (0.374) | 0.986 | 0.474 | 2.054 | 0.971 |
| Hypertension | 0.644 (0.341) | 1.903 | 0.976 | 3.711 | 0.059 |
| Smoking | −0.211(0.372) | 0.810 | 0.391 | 1.679 | 0.571 |
| Adjusted R2 | 0.499 | − | − | − | <0.001 |
| Model 2, n = 736 | |||||
| Age (years) | 0.149 (0.021) | 1.160 | 1.113 | 1.210 | <0.001 |
| Log2 [(ECd/Ccr) × 105], µg/L | 0.843 (0.161) | 2.324 | 1.695 | 3.187 | <0.001 |
| Log2 [(ENAG/Ccr) × 104], units/L | 0.985 (0.192) | 2.678 | 1.837 | 3.905 | <0.001 |
| Diabetes | 0.100 (0.961) | 1.105 | 0.168 | 7.264 | 0.917 |
| Gender (women) | 0.459 (0.429) | 1.582 | 0.682 | 3.669 | 0.285 |
| Hypertension | 0.667 (0.395) | 1.949 | 0.898 | 4.229 | 0.091 |
| Smoking | −0.374 (0.414) | 0.688 | 0.305 | 1.549 | 0.366 |
| Adjusted R2 | 0.544 | − | − | − | <0.001 |
| Independent Variables/Factors | eGFR, mL/min/1.73 m2 | |||||
|---|---|---|---|---|---|---|
| Cd Excretion Level 1 | Cd Excretion Level 2 | Cd Excretion Level 3 | ||||
| β | p | β | p | β | p | |
| Model 1, n = 736 | ||||||
| Age (years) | −0.527 | <0.001 | −0.624 | <0.001 | −0.489 | <0.001 |
| Log10[(ECd/Ecr) × 103],µg/g creatinine | −0.077 | 0.422 | −0.088 | 0.023 | −0.138 | 0.061 |
| Log10[(ENAG/Ecr) × 103], unit/g creatinine | 0.368 | <0.001 | 0.068 | 0.060 | 0.056 | 0.456 |
| Gender (women) | −0.261 | 0.026 | −0.053 | 0.166 | −0.030 | 0.715 |
| Smoking | −0.256 | 0.025 | 0.029 | 0.455 | −0.069 | 0.392 |
| Hypertension | 0.266 | 0.013 | 0.049 | 0.139 | 0.127 | 0.091 |
| Diabetes | − | − | 0.041 | 0.201 | 0.135 | 0.069 |
| Adjusted R2 | 0.531 | <0.001 | 0.454 | <0.001 | 0.290 | <0.001 |
| Model 2, n = 736 | ||||||
| Age (years) | −0.640 | <0.001 | −0.548 | <0.001 | −0.483 | 0.001 |
| Log10[(ECd/Ccr) × 105], µg/L | −0.073 | 0.414 | −0.128 | 0.001 | −0.281 | 0.001 |
| Log10[(ENAG/Ccr) × 104], units/L | 0.175 | 0.049 | −0.095 | 0.010 | −0.228 | 0.002 |
| Gender (women) | −0.276 | 0.010 | −0.091 | 0.023 | 0.068 | 0.366 |
| Smoking | −0.109 | 0.276 | −0.040 | 0.335 | 0.002 | 0.980 |
| Hypertension | 0.168 | 0.075 | 0.029 | 0.420 | 0.108 | 0.125 |
| Diabetes | − | − | 0.053 | 0.125 | 0.026 | 0.706 |
| Adjusted R2 | 0.452 | <0.001 | 0.383 | <0.001 | 0.436 | <0.001 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Satarug, S.; Vesey, D.A.; Nishijo, M.; Ruangyuttikarn, W.; Gobe, G.C.; Phelps, K.R. The Effect of Cadmium on GFR Is Clarified by Normalization of Excretion Rates to Creatinine Clearance. Int. J. Mol. Sci. 2021, 22, 1762. https://doi.org/10.3390/ijms22041762
Satarug S, Vesey DA, Nishijo M, Ruangyuttikarn W, Gobe GC, Phelps KR. The Effect of Cadmium on GFR Is Clarified by Normalization of Excretion Rates to Creatinine Clearance. International Journal of Molecular Sciences. 2021; 22(4):1762. https://doi.org/10.3390/ijms22041762
Chicago/Turabian StyleSatarug, Soisungwan, David A. Vesey, Muneko Nishijo, Werawan Ruangyuttikarn, Glenda C. Gobe, and Kenneth R. Phelps. 2021. "The Effect of Cadmium on GFR Is Clarified by Normalization of Excretion Rates to Creatinine Clearance" International Journal of Molecular Sciences 22, no. 4: 1762. https://doi.org/10.3390/ijms22041762
APA StyleSatarug, S., Vesey, D. A., Nishijo, M., Ruangyuttikarn, W., Gobe, G. C., & Phelps, K. R. (2021). The Effect of Cadmium on GFR Is Clarified by Normalization of Excretion Rates to Creatinine Clearance. International Journal of Molecular Sciences, 22(4), 1762. https://doi.org/10.3390/ijms22041762









