Dynamical and Structural Properties of Comb Long-Chain Branched Polymer in Shear Flow
Abstract
1. Introduction
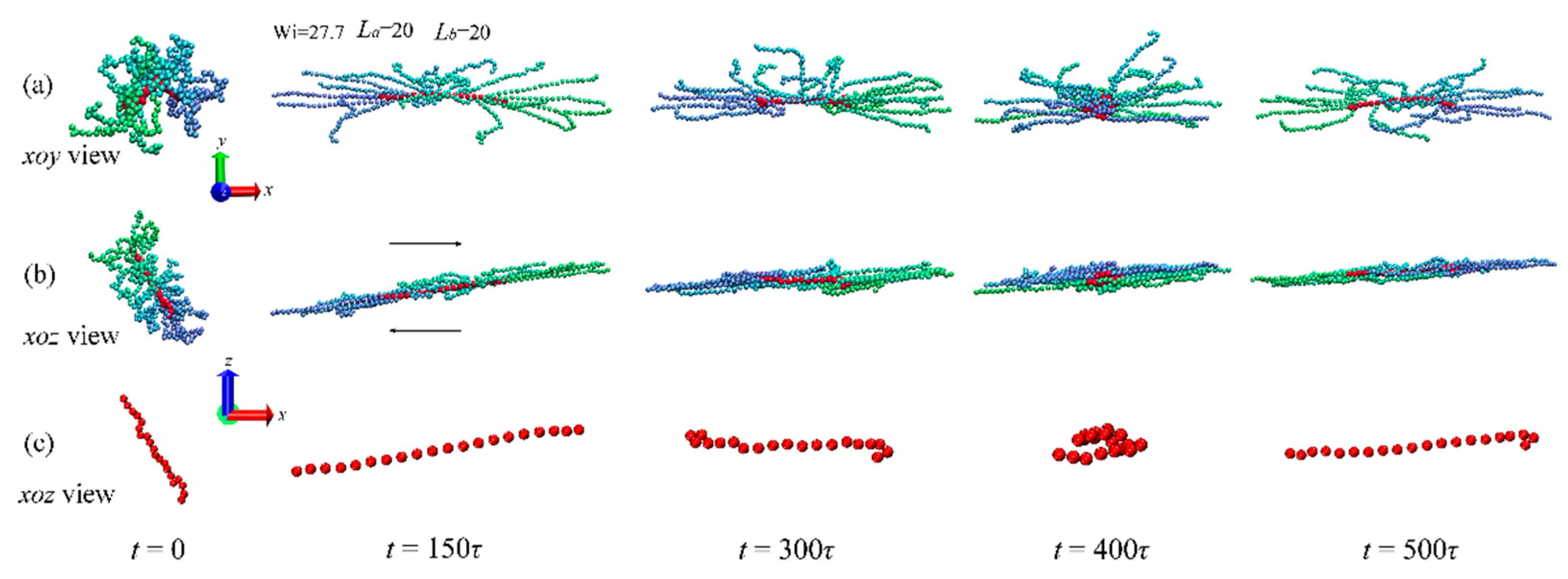
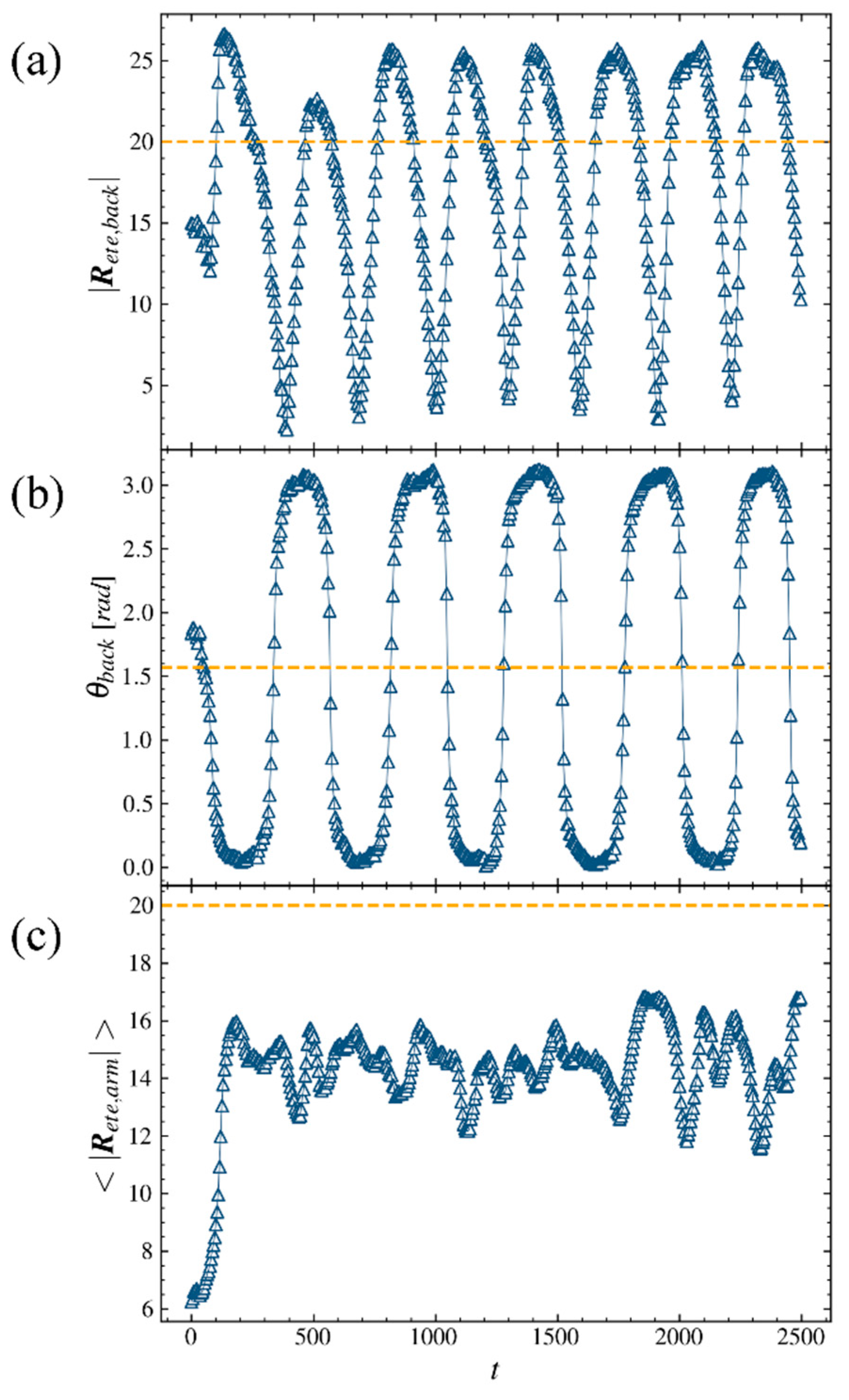
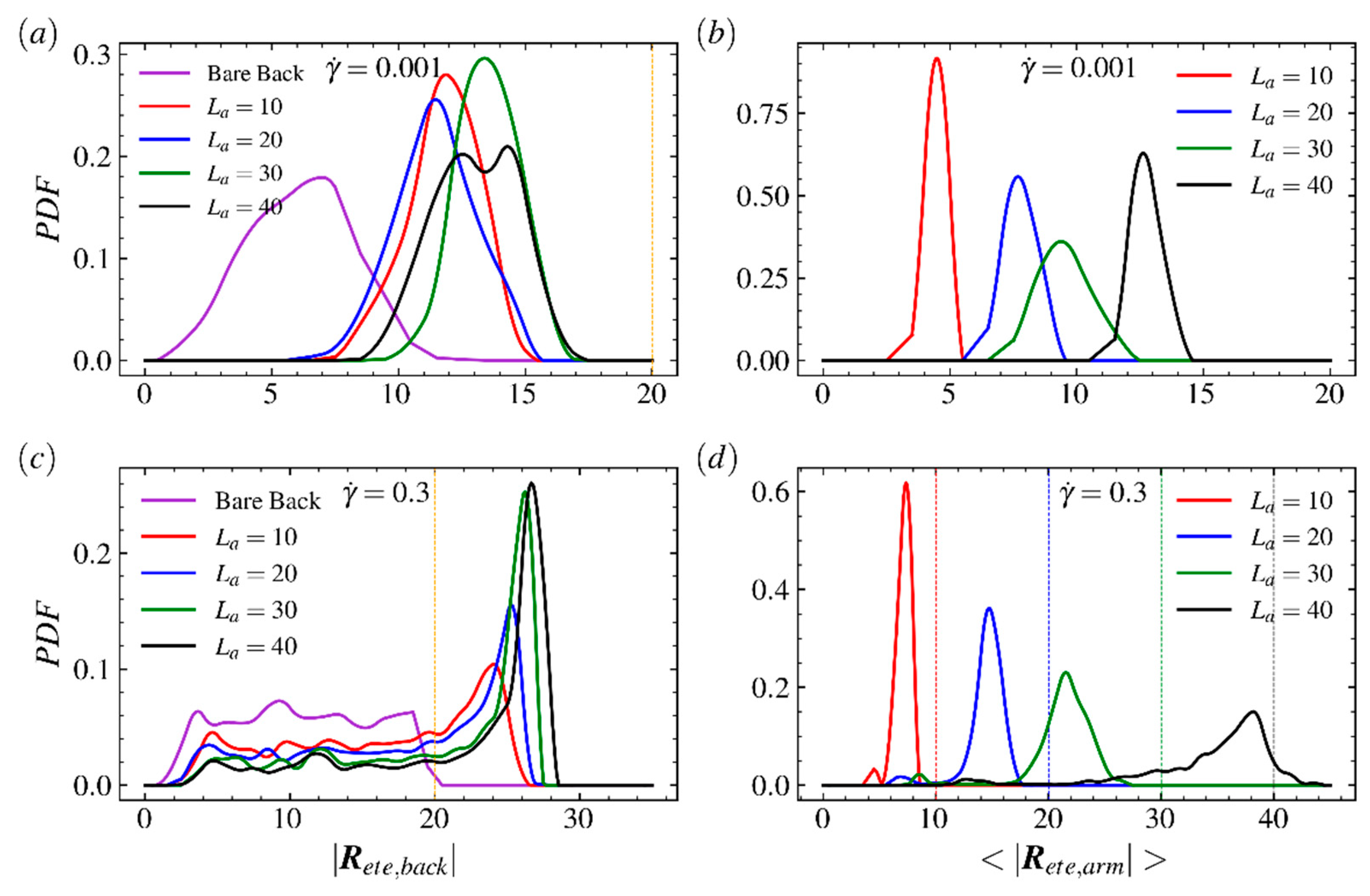
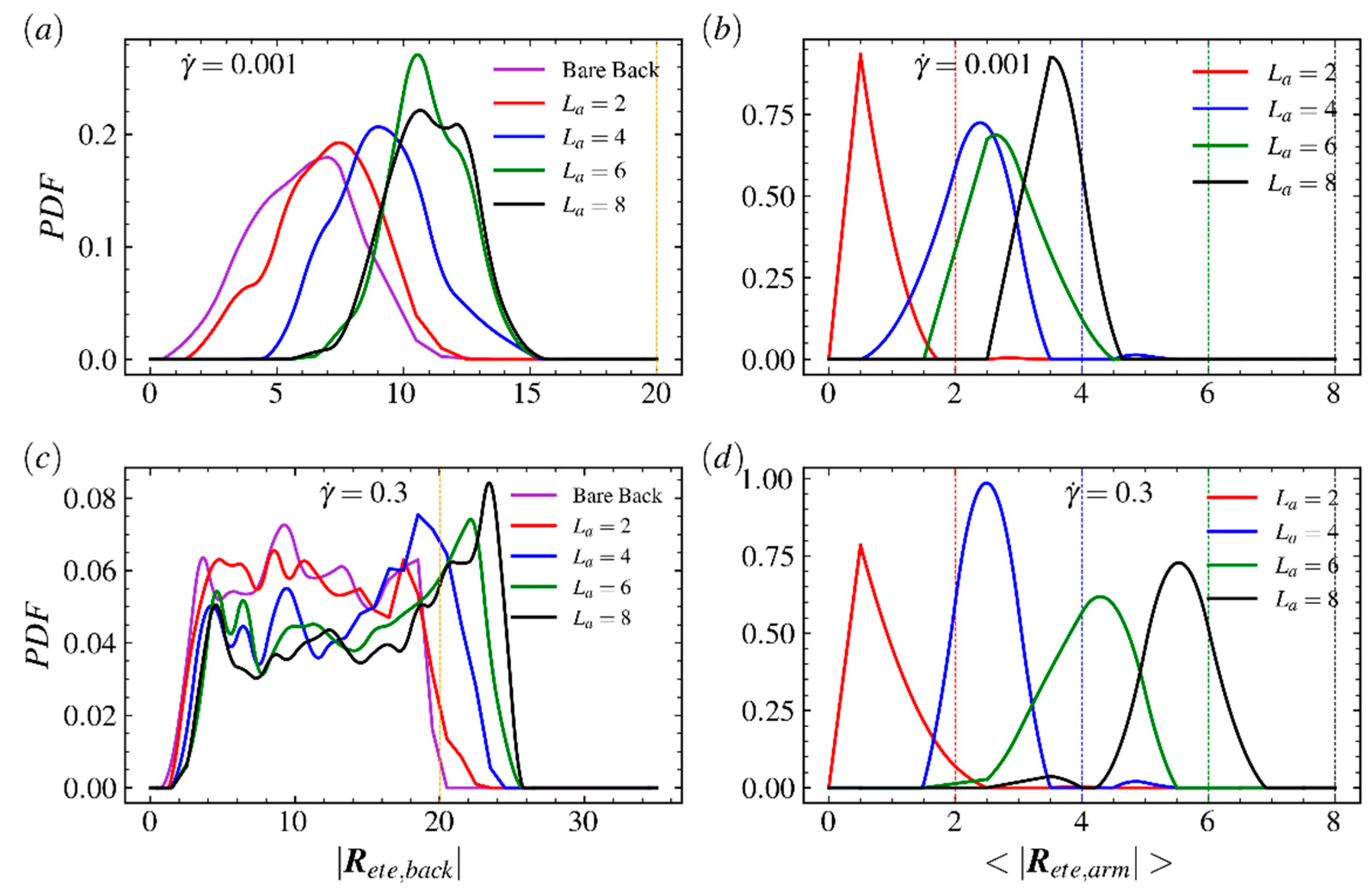
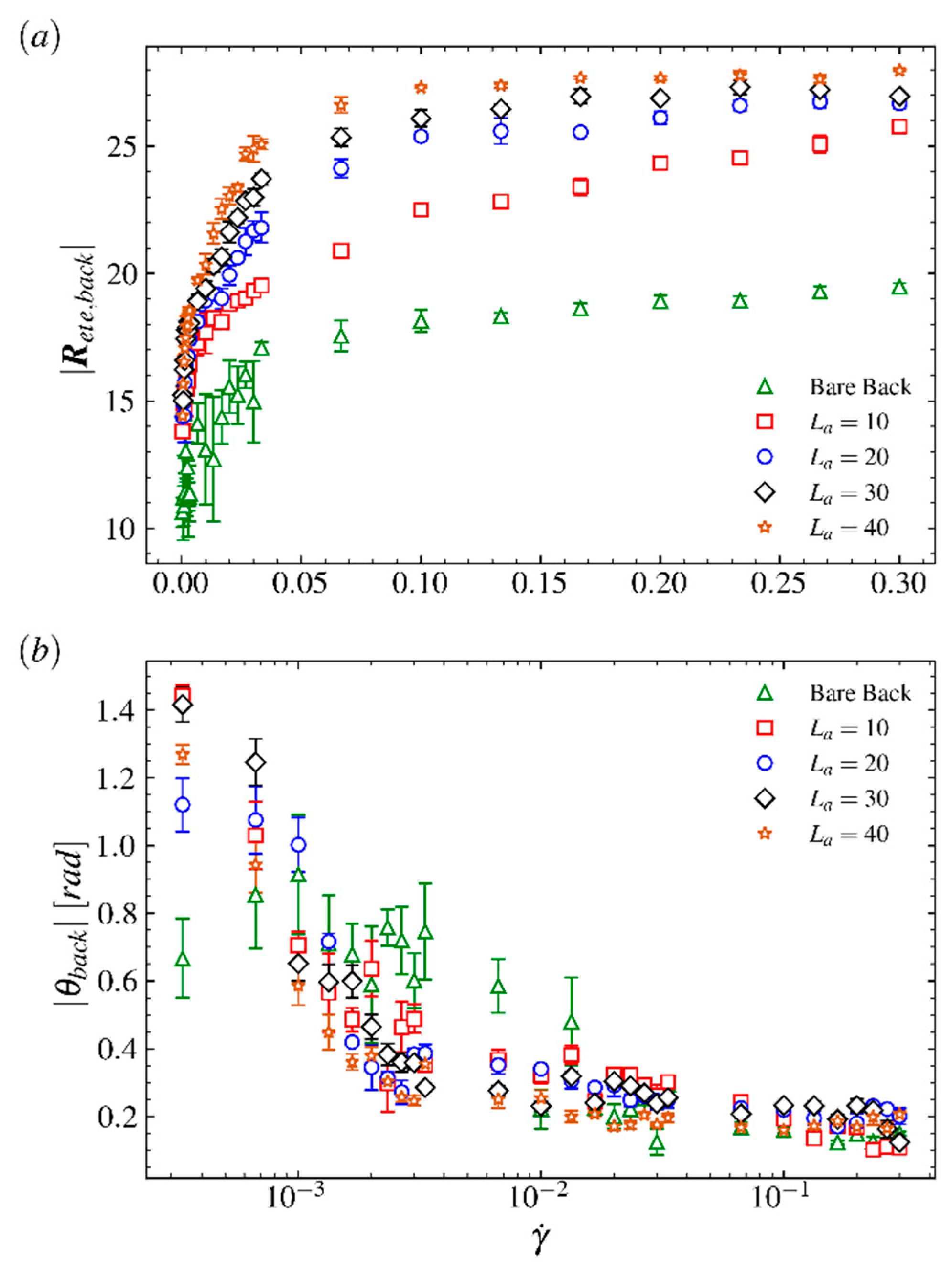
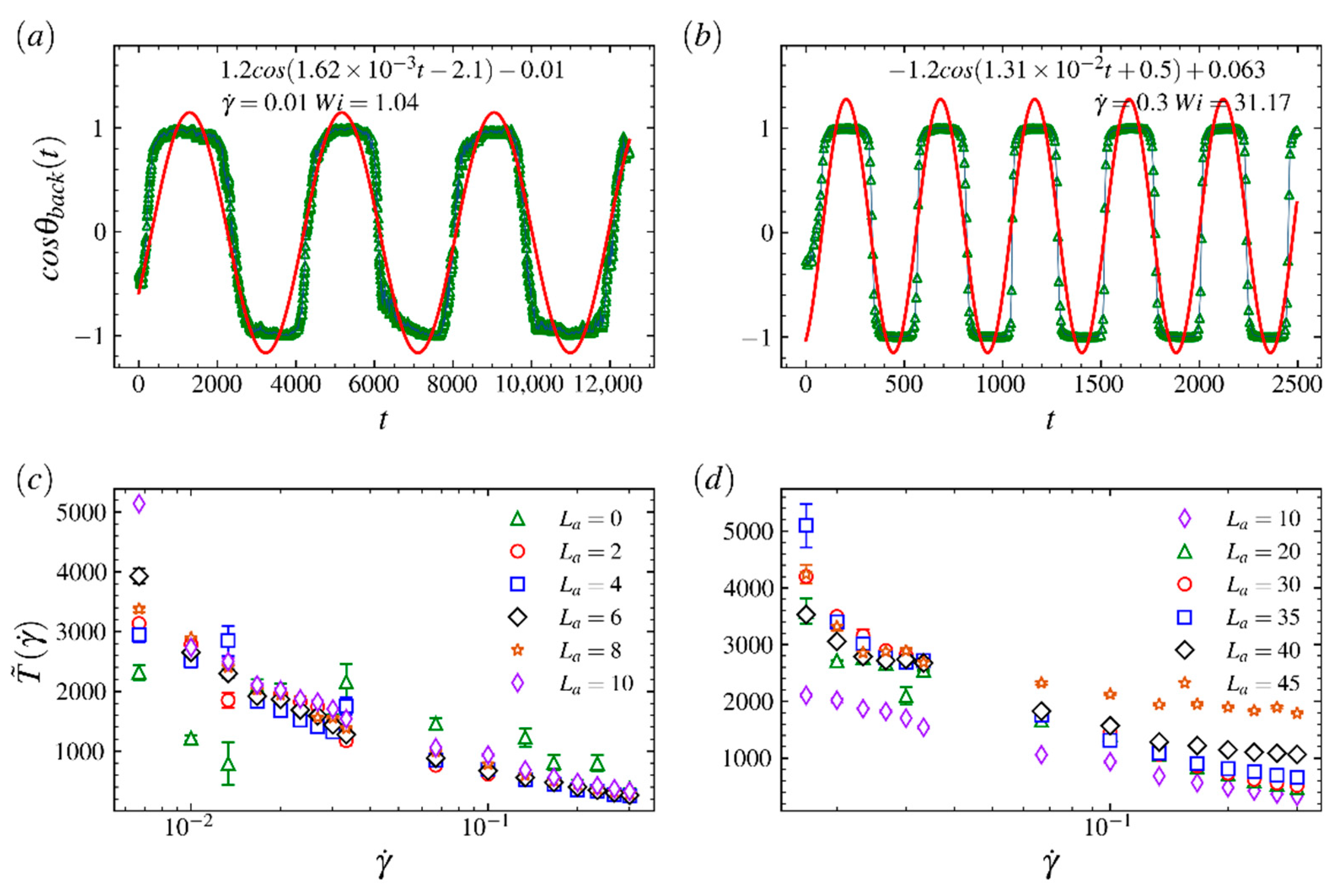
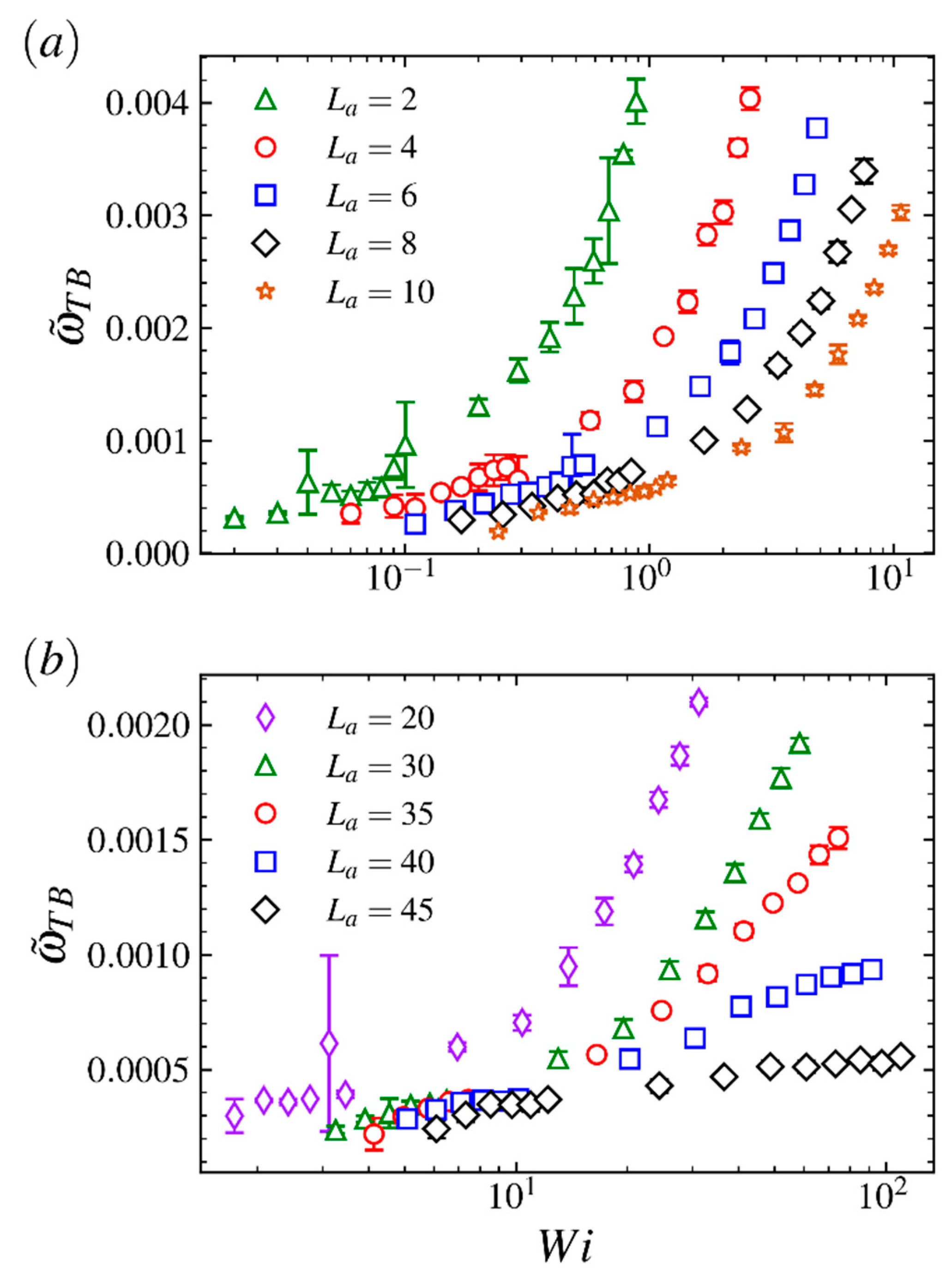
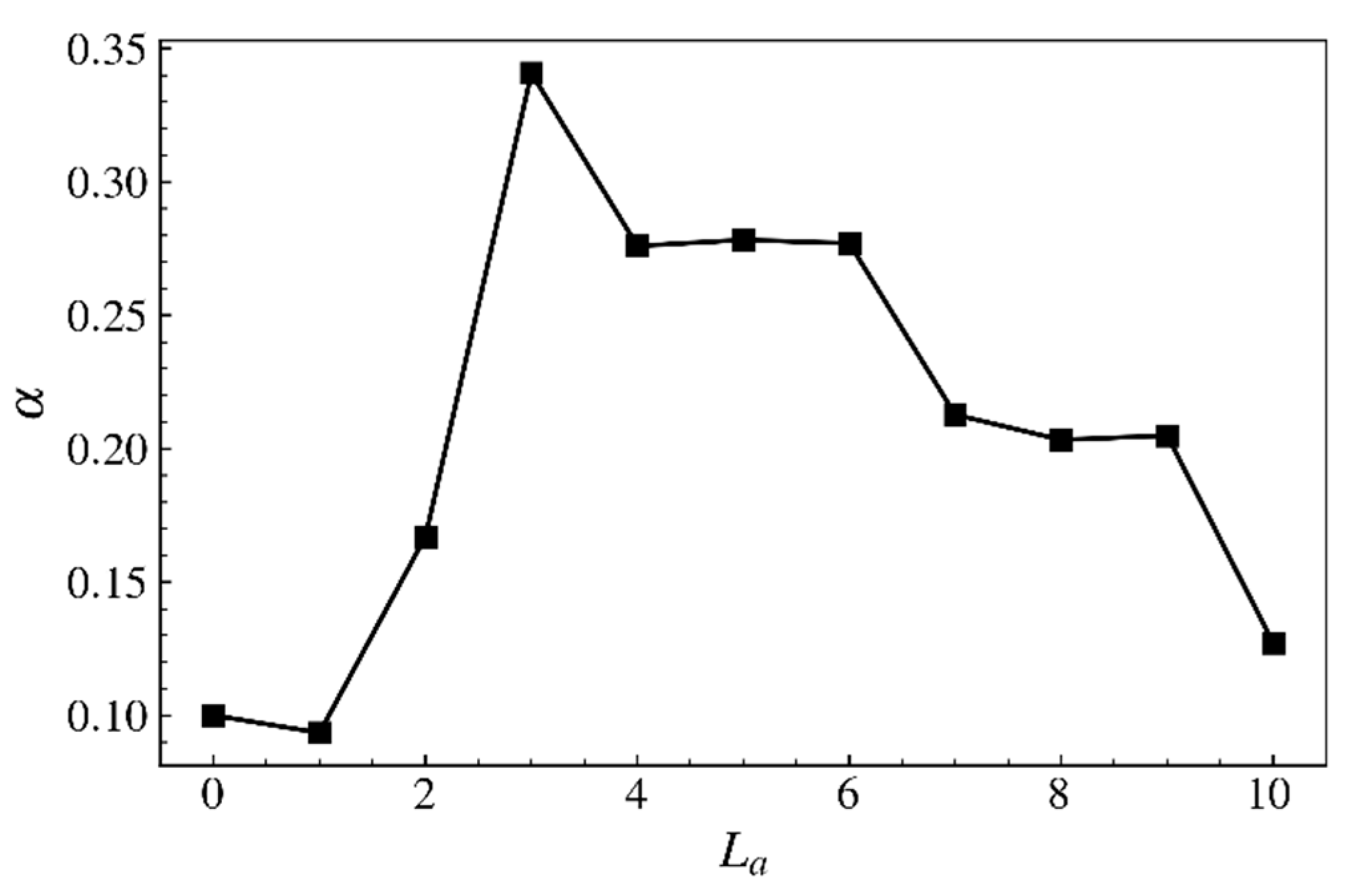
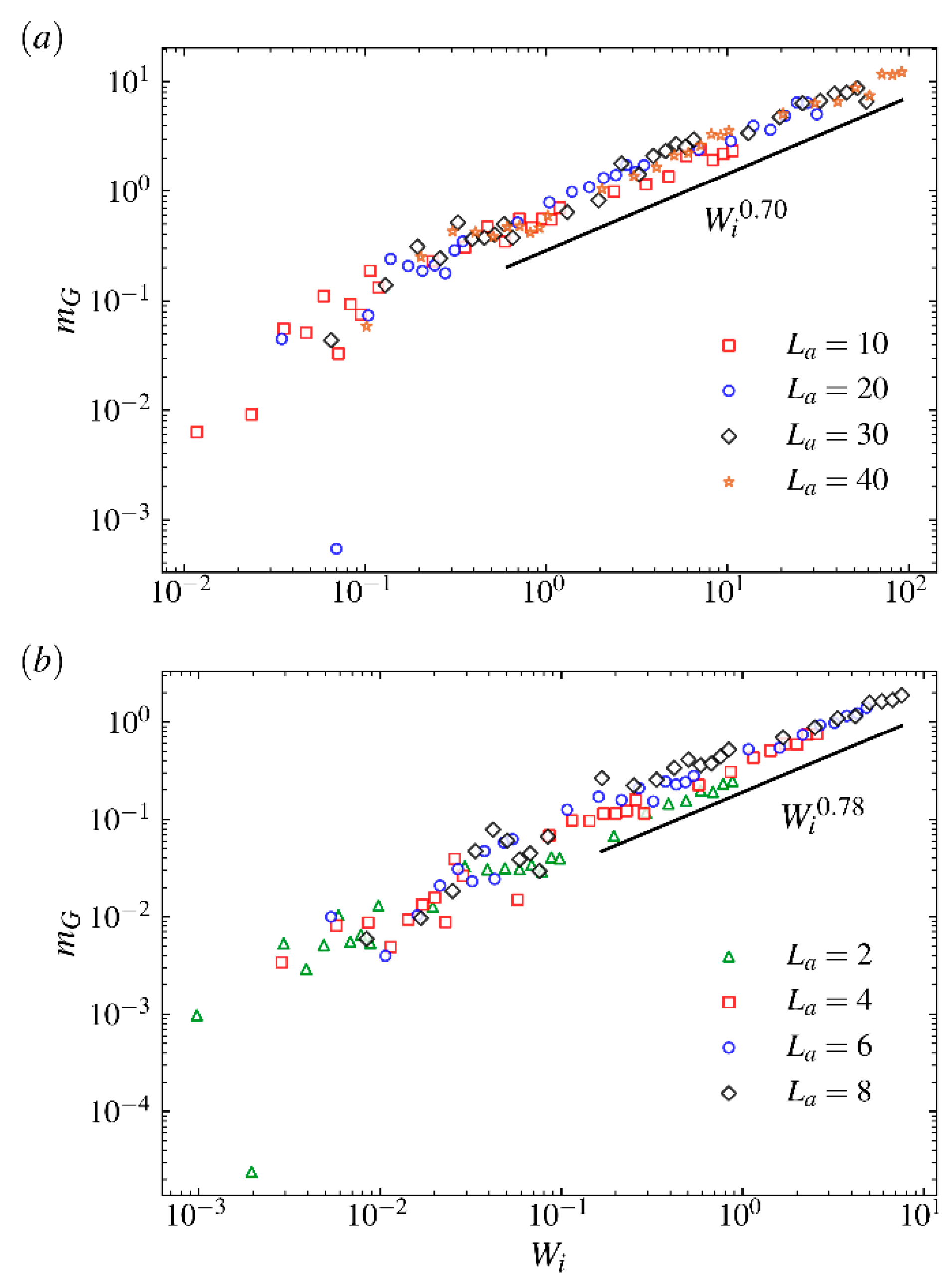
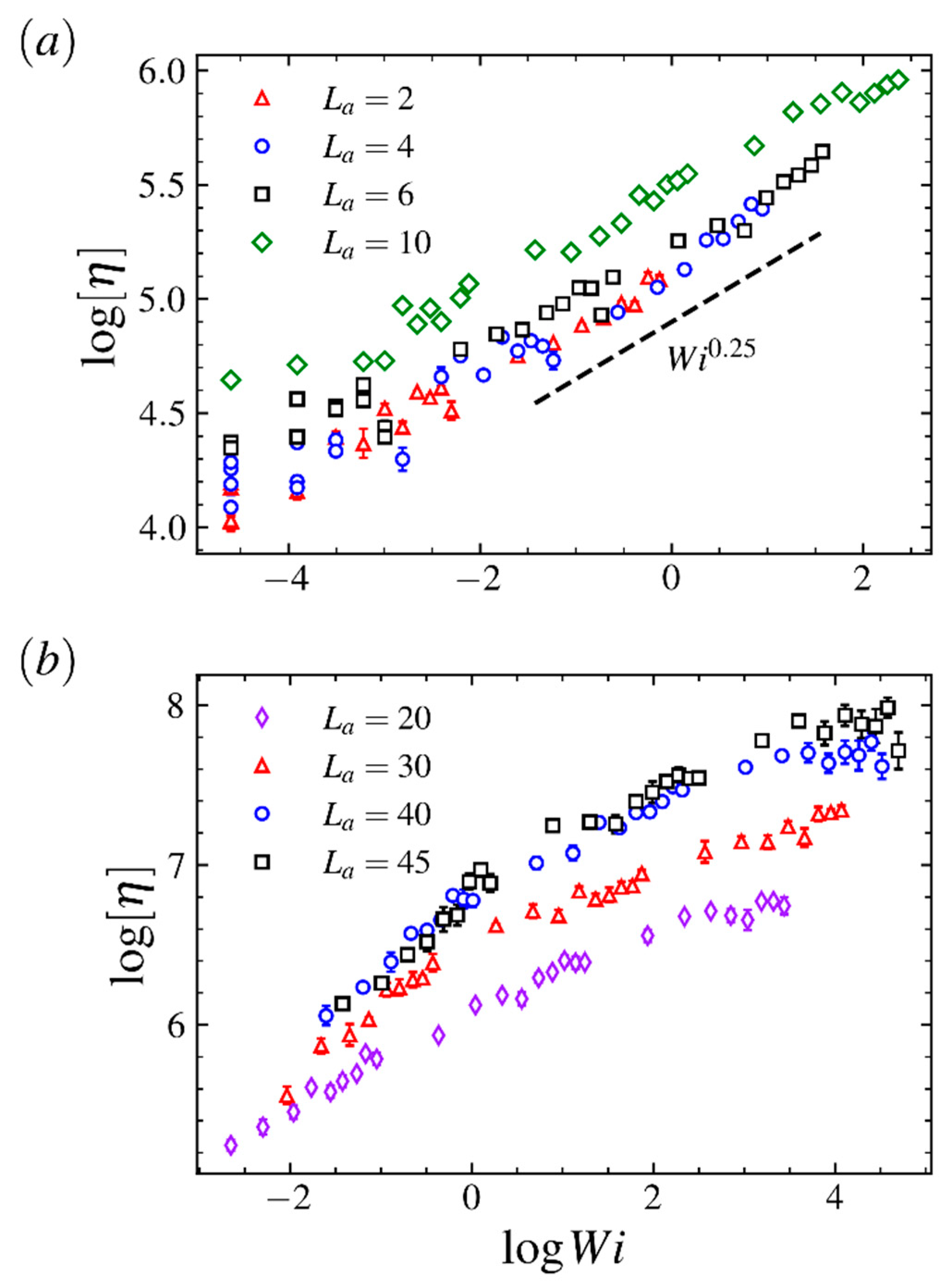
2. Results and Discussion
3. Materials and Methods
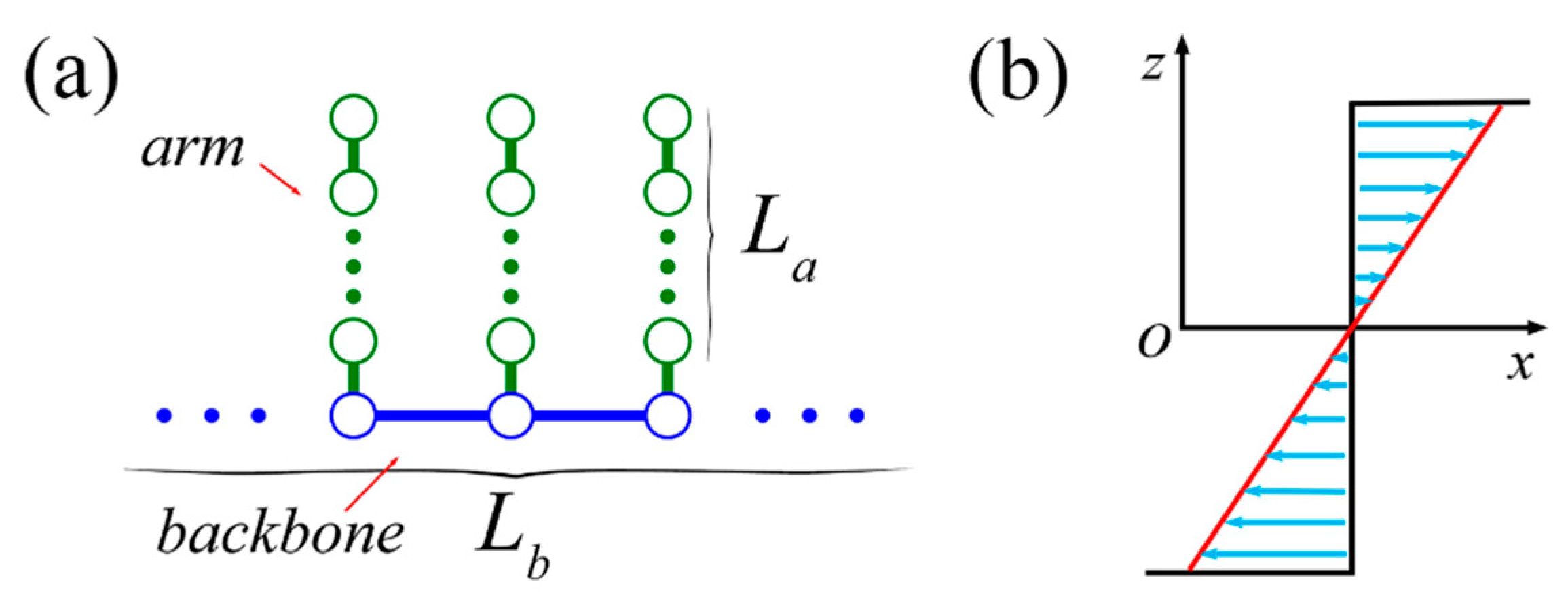
3.1. Comb Polymer
3.2. Multiparticle Collision Dynamics (MPCD)
3.3. Simulation Details
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- LeDuc, P.; Haber, C.; Bao, G.; Wirtz, D. Dynamics of individual flexible polymers in a shear flow. Nature 1999, 399, 564–566. [Google Scholar] [CrossRef]
- Saha Dalal, I.; Albaugh, A.; Hoda, N.; Larson, R.G. Tumbling and deformation of isolated polymer chains in shearing flow. Macromolecules 2012, 45, 9493–9499. [Google Scholar] [CrossRef]
- Chen, W.; Chen, J.; An, L. Tumbling and tank-treading dynamics of individual ring polymers in shear flow. Soft Matter 2013, 9, 4312–4318. [Google Scholar] [CrossRef]
- Delgado-Buscalioni, R. Cyclic motion of a grafted polymer under shear flow. Phys. Rev. Lett. 2006, 96, 088303. [Google Scholar] [CrossRef]
- Ripoll, M.; Winkler, R.; Gompper, G. Star polymers in shear flow. Phys. Rev. Lett. 2006, 96, 188302. [Google Scholar] [CrossRef]
- Tu, M.Q.; Lee, M.; Robertson-Anderson, R.M.; Schroeder, C.M. Direct observation of ring polymer dynamics in the flow-gradient plane of shear flow. Macromolecules 2020, 53, 9406–9419. [Google Scholar] [CrossRef]
- Teixeira, R.E.; Babcock, H.P.; Shaqfeh, E.S.; Chu, S. Shear thinning and tumbling dynamics of single polymers in the flow-gradient plane. Macromolecules 2005, 38, 581–592. [Google Scholar] [CrossRef]
- Aust, C.; Kröger, M.; Hess, S. Structure and dynamics of dilute polymer solutions under shear flow via nonequilibrium molecular dynamics. Macromolecules 1999, 32, 5660–5672. [Google Scholar] [CrossRef]
- Nikoubashman, A.; Likos, C.N. Branched polymers under shear. Macromolecules 2010, 43, 1610–1620. [Google Scholar] [CrossRef][Green Version]
- Singh, S.P.; Gompper, G.; Winkler, R.G. Steady state sedimentation of ultrasoft colloids. J. Chem. Phys. 2018, 148, 084901. [Google Scholar] [CrossRef]
- Smith, D.E.; Babcock, H.P.; Chu, S. Single-polymer dynamics in steady shear flow. Science 1999, 283, 1724–1727. [Google Scholar] [CrossRef]
- Jendrejack, R.M.; Dimalanta, E.T.; Schwartz, D.C.; Graham, M.D.; de Pablo, J.J. DNA dynamics in a microchannel. Phys. Rev. Lett. 2003, 91, 038102. [Google Scholar] [CrossRef]
- Jendrejack, R.M.; Schwartz, D.C.; De Pablo, J.J.; Graham, M.D. Shear-induced migration in flowing polymer solutions: Simulation of long-chain DNA in microchannels. J. Chem. Phys. 2004, 120, 2513–2529. [Google Scholar] [CrossRef]
- Schroeder, C.M.; Teixeira, R.E.; Shaqfeh, E.S.; Chu, S. Characteristic periodic motion of polymers in shear flow. Phys. Rev. Lett. 2005, 95, 018301. [Google Scholar] [CrossRef]
- Ripoll, M.; Winkler, R.; Gompper, G. Hydrodynamic screening of star polymers in shear flow. Eur. Phys. J. E Soft Matter Biol. Phys. 2007, 23, 349–354. [Google Scholar] [CrossRef] [PubMed]
- Winkler, R.G. Semiflexible polymers in shear flow. Phys. Rev. Lett. 2006, 97, 128301. [Google Scholar] [CrossRef] [PubMed]
- Gratton, Y.; Slater, G. Molecular dynamics study of tethered polymers in shear flow. Eur. Phys. J. E Soft Matter Biol. Phys. 2005, 17, 455–465. [Google Scholar] [CrossRef] [PubMed]
- Webster, M.; Yeomans, J. Modeling a tethered polymer in Poiseuille flow. J. Chem. Phys. 2005, 122, 164903. [Google Scholar] [CrossRef] [PubMed]
- Rzehak, R.; Kienle, D.; Kawakatsu, T.; Zimmermann, W. Partial draining of a tethered polymer in flow. Europhys. Lett. 1999, 46, 821. [Google Scholar] [CrossRef]
- Sendner, C.; Netz, R. Single flexible and semiflexible polymers at high shear: Non-monotonic and non-universal stretching response. Eur. Phys. J. E Soft Matter Biol. Phys. 2009, 30, 75–81. [Google Scholar] [CrossRef]
- Sing, C.E.; Alexander-Katz, A. Giant nonmonotonic stretching response of a self-associating polymer in shear flow. Phys. Rev. Lett. 2011, 107, 198302. [Google Scholar] [CrossRef]
- Liebetreu, M.; Ripoll, M.; Likos, C.N. Trefoil knot hydrodynamic delocalization on sheared ring polymers. ACS Macro Lett. 2018, 7, 447–452. [Google Scholar] [CrossRef] [PubMed]
- Chertkov, M.; Kolokolov, I.; Lebedev, V.; Turitsyn, K. Polymer statistics in a random flow with mean shear. J. Fluid Mech. 2005, 531, 251–260. [Google Scholar] [CrossRef]
- Celani, A.; Puliafito, A.; Turitsyn, K. Polymers in linear shear flow: A numerical study. Europhys. Lett. 2005, 70, 464. [Google Scholar] [CrossRef]
- Grest, G.S.; Kremer, K.; Milner, S.T.; Witten, T. Relaxation of self-entangled many-arm star polymers. Macromolecules 1989, 22, 1904–1910. [Google Scholar] [CrossRef]
- Lee, J.H.; Fetters, L.J.; Archer, L.A. Stress relaxation of branched polymers. Macromolecules 2005, 38, 10763–10771. [Google Scholar] [CrossRef]
- Juliani; Archer, L.A. Relaxation dynamics of entangled and unentangled multiarm polymer solutions: Comparisons with theory. Macromolecules 2002, 35, 10048–10053. [Google Scholar] [CrossRef]
- McLeish, T.; Larson, R. Molecular constitutive equations for a class of branched polymers: The pom-pom polymer. J. Rheol. 1998, 42, 81–110. [Google Scholar] [CrossRef]
- McLeish, T.; Allgaier, J.; Bick, D.; Bishko, G.; Biswas, P.; Blackwell, R.; Blottiere, B.; Clarke, N.; Gibbs, B.; Groves, D. Dynamics of entangled H-polymers: Theory, rheology, and neutron-scattering. Macromolecules 1999, 32, 6734–6758. [Google Scholar] [CrossRef]
- Archer, L.A.; Juliani. Linear and nonlinear viscoelasticity of entangled multiarm (pom-pom) polymer liquids. Macromolecules 2004, 37, 1076–1088. [Google Scholar] [CrossRef]
- Juliani; Archer, L.A. Relaxation dynamics of entangled and unentangled multiarm polymer solutions: Experiment. Macromolecules 2002, 35, 6953–6960. [Google Scholar] [CrossRef]
- Islam, M.T.; Juliani, a.; Archer, L.A.; Varshney, S.K. Linear rheology of entangled six-arm and eight-arm polybutadienes. Macromolecules 2001, 34, 6438–6449. [Google Scholar] [CrossRef]
- Kapnistos, M.; Vlassopoulos, D.; Roovers, J.; Leal, L. Linear rheology of architecturally complex macromolecules: Comb polymers with linear backbones. Macromolecules 2005, 38, 7852–7862. [Google Scholar] [CrossRef]
- Jeong, S.H.; Ha, T.Y.; Cho, S.; Roh, E.J.; Kim, J.M.; Baig, C. Melt Rheology of Short-Chain Branched Ring Polymers in Shear Flow. Macromolecules 2021, 54, 10350–10359. [Google Scholar] [CrossRef]
- Li, Z.; Li, Y.; Wang, Y.; Sun, Z.; An, L. Transport of star-branched polymers in nanoscale pipe channels simulated with dissipative particle dynamics simulation. Macromolecules 2010, 43, 5896–5903. [Google Scholar] [CrossRef]
- Jeong, S.; Kim, J.M.; Cho, S.; Baig, C. Effect of short-chain branching on interfacial polymer structure and dynamics under shear flow. Soft Matter 2017, 13, 8644–8650. [Google Scholar] [CrossRef]
- Roh, E.J.; Kim, J.M.; Baig, C. Molecular dynamics study on the structure and relaxation of short-chain branched ring polymer melts. Polymer 2019, 175, 107–117. [Google Scholar] [CrossRef]
- Kim, J.M.; Baig, C. Communication: Role of short chain branching in polymer structure and dynamics. J. Chem. Phys. 2016, 144, 081101. [Google Scholar] [CrossRef]
- Wijesinghe, S.; Perahia, D.; Grest, G.S. Polymer topology effects on dynamics of comb polymer melts. Macromolecules 2018, 51, 7621–7628. [Google Scholar] [CrossRef]
- Delgadillo-Velázquez, O.; Hatzikiriakos, S.; Sentmanat, M. Thermorheological properties of LLDPE/LDPE blends. Rheol. Acta 2008, 47, 19–31. [Google Scholar] [CrossRef]
- Dordinejad, A.K.; Jafari, S.H. Miscibility analysis in LLDPE/LDPE blends via thermorheological analysis: Correlation with branching structure. Polym. Eng. Sci. 2014, 54, 1081–1088. [Google Scholar] [CrossRef]
- Zhu, H.; Wang, Y.; Zhang, X.; Su, Y.; Dong, X.; Chen, Q.; Zhao, Y.; Geng, C.; Zhu, S.; Han, C.C. Influence of molecular architecture and melt rheological characteristic on the optical properties of LDPE blown films. Polymer 2007, 48, 5098–5106. [Google Scholar] [CrossRef]
- Liu, C.; Wang, J.; He, J. Rheological and thermal properties of m-LLDPE blends with m-HDPE and LDPE. Polymer 2002, 43, 3811–3818. [Google Scholar] [CrossRef]
- Ajji, A.; Sammut, P.; Huneault, M. Elongational rheology of LLDPE/LDPE blends. J. Appl. Polym. Sci. 2003, 88, 3070–3077. [Google Scholar] [CrossRef]
- Stange, J.; Uhl, C.; Münstedt, H. Rheological behavior of blends from a linear and a long-chain branched polypropylene. J. Rheol. 2005, 49, 1059–1079. [Google Scholar] [CrossRef]
- McCallum, T.J.; Kontopoulou, M.; Park, C.B.; Muliawan, E.B.; Hatzikiriakos, S.G. The rheological and physical properties of linear and branched polypropylene blends. Polym. Eng. Sci. 2007, 47, 1133–1140. [Google Scholar] [CrossRef]
- Sugimoto, M.; Suzuki, Y.; Hyun, K.; Ahn, K.H.; Ushioda, T.; Nishioka, A.; Taniguchi, T.; Koyama, K. Melt rheology of long-chain-branched polypropylenes. Rheol. Acta 2006, 46, 33–44. [Google Scholar] [CrossRef]
- Schweizer, T. Structure and Rheology of Molten Polymers. Appl. Rheol. 2007, 17, 258–259. [Google Scholar] [CrossRef]
- Bourg, V.; Valette, R.; Moigne, N.L.; Ienny, P.; Guillard, V.; Bergeret, A. Shear and Extensional Rheology of Linear and Branched Polybutylene Succinate Blends. Polymers 2021, 13, 652. [Google Scholar] [CrossRef]
- Doi, M.; Edwards, S.F.; Edwards, S.F. The Theory of Polymer Dynamics; Oxford University Press: Oxford, UK, 1988; p. 73. [Google Scholar]
- Howard, M.P.; Panagiotopoulos, A.Z.; Nikoubashman, A. Efficient mesoscale hydrodynamics: Multiparticle collision dynamics with massively parallel GPU acceleration. Comput. Phys. Commun. 2018, 230, 10–20. [Google Scholar] [CrossRef]
- Anderson, J.A.; Glaser, J.; Glotzer, S.C. HOOMD-blue: A Python package for high-performance molecular dynamics and hard particle Monte Carlo simulations. Comput. Mater. Sci. 2020, 173, 109363. [Google Scholar] [CrossRef]
- Jeong, S.; Cho, S.; Kim, J.M.; Baig, C. Interfacial Molecular Structure and Dynamics of Confined Ring Polymer Melts under Shear Flow. Macromolecules 2018, 51, 4670–4677. [Google Scholar] [CrossRef]
- Jeong, S.H.; Cho, S.; Ha, T.Y.; Roh, E.J.; Baig, C. Structural and Dynamical Characteristics of Short-Chain Branched Ring Polymer Melts at Interface under Shear Flow. Polymers 2020, 12, 3068. [Google Scholar] [CrossRef]
- Navot, Y. Elastic membranes in viscous shear flow. Phys. Fluids 1998, 10, 1819–1833. [Google Scholar] [CrossRef]
- Lu, Y.; An, L.; Wang, Z. Intrinsic viscosity of polymers: General theory based on a partially permeable sphere model. Macromolecules 2013, 46, 5731–5740. [Google Scholar] [CrossRef]
- Bishop, M.; Kalos, M.; Frisch, H. Molecular dynamics of polymeric systems. J. Chem. Phys. 1979, 70, 1299–1304. [Google Scholar] [CrossRef]
- Kremer, K.; Grest, G.S. Dynamics of entangled linear polymer melts: A molecular-dynamics simulation. J. Chem. Phys. 1990, 92, 5057–5086. [Google Scholar] [CrossRef]
- Malevanets, A.; Kapral, R. Solute molecular dynamics in a mesoscale solvent. J. Chem. Phys. 2000, 112, 7260–7269. [Google Scholar] [CrossRef]
- Mussawisade, K.; Ripoll, M.; Winkler, R.; Gompper, G. Dynamics of polymers in a particle-based mesoscopic solvent. J. Chem. Phys. 2005, 123, 144905. [Google Scholar] [CrossRef]
- Malevanets, A.; Kapral, R. Mesoscopic model for solvent dynamics. J. Chem. Phys. 1999, 110, 8605–8613. [Google Scholar] [CrossRef]
- Kapral, R. Multiparticle collision dynamics: Simulation of complex systems on mesoscales. Adv. Chem. Phys. 2008, 140, 89. [Google Scholar]
- Ihle, T.; Kroll, D. Stochastic rotation dynamics. II. Transport coefficients, numerics, and long-time tails. Phys. Rev. E 2003, 67, 066706. [Google Scholar] [CrossRef] [PubMed]
- Huang, C.-C.; Gompper, G.; Winkler, R.G. Hydrodynamic correlations in multiparticle collision dynamics fluids. Phys. Rev. E. 2012, 86, 056711. [Google Scholar] [CrossRef] [PubMed]
- Malevanets, A.; Yeomans, J. Dynamics of short polymer chains in solution. Europhys. Lett. 2000, 52, 231. [Google Scholar] [CrossRef]
- Ripoll, M.; Mussawisade, K.; Winkler, R.; Gompper, G. Low-Reynolds-number hydrodynamics of complex fluids by multi-particle-collision dynamics. Europhys. Lett. 2004, 68, 106. [Google Scholar] [CrossRef]
- Ihle, T.; Kroll, D. Stochastic rotation dynamics: A Galilean-invariant mesoscopic model for fluid flow. Phys. Rev. E 2001, 63, 020201. [Google Scholar] [CrossRef]
- Ihle, T.; Kroll, D.M. Stochastic rotation dynamics. I. Formalism, Galilean invariance, and Green-Kubo relations. Phys. Rev. E 2003, 67, 066705. [Google Scholar] [CrossRef]
- Lees, A.; Edwards, S. The computer study of transport processes under extreme conditions. J. Phys. C Solid State Phys. 1972, 5, 1921. [Google Scholar] [CrossRef]
- Huang, C.-C.; Chatterji, A.; Sutmann, G.; Gompper, G.; Winkler, R.G. Cell-level canonical sampling by velocity scaling for multiparticle collision dynamics simulations. J. Comput. Phys. 2010, 229, 168–177. [Google Scholar] [CrossRef]
- Padding, J.; Louis, A. Hydrodynamic interactions and Brownian forces in colloidal suspensions: Coarse-graining over time and length scales. Phys. Rev. E 2006, 74, 031402. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, D.; Wen, X.; Zhang, D.; Tang, J. Dynamical and Structural Properties of Comb Long-Chain Branched Polymer in Shear Flow. Int. J. Mol. Sci. 2022, 23, 11290. https://doi.org/10.3390/ijms231911290
Wang D, Wen X, Zhang D, Tang J. Dynamical and Structural Properties of Comb Long-Chain Branched Polymer in Shear Flow. International Journal of Molecular Sciences. 2022; 23(19):11290. https://doi.org/10.3390/ijms231911290
Chicago/Turabian StyleWang, Deyin, Xiaohui Wen, Dong Zhang, and Jiajun Tang. 2022. "Dynamical and Structural Properties of Comb Long-Chain Branched Polymer in Shear Flow" International Journal of Molecular Sciences 23, no. 19: 11290. https://doi.org/10.3390/ijms231911290
APA StyleWang, D., Wen, X., Zhang, D., & Tang, J. (2022). Dynamical and Structural Properties of Comb Long-Chain Branched Polymer in Shear Flow. International Journal of Molecular Sciences, 23(19), 11290. https://doi.org/10.3390/ijms231911290






