Changes in Astroglial K+ upon Brief Periods of Energy Deprivation in the Mouse Neocortex
Abstract
:1. Introduction
2. Materials and Methods
2.1. Preparation of Organotypic and Acute Tissue Slices
2.2. Imaging of ATP
2.3. Imaging of Intracellular Na+ and pH
2.4. Measurement of Extracellular K+, Na+, and pH
2.5. Patch-Clamp Recordings
2.6. Data Presentation and Statistics
3. Results
3.1. Effect of Transient Energy Depletion on Intracellular ATP
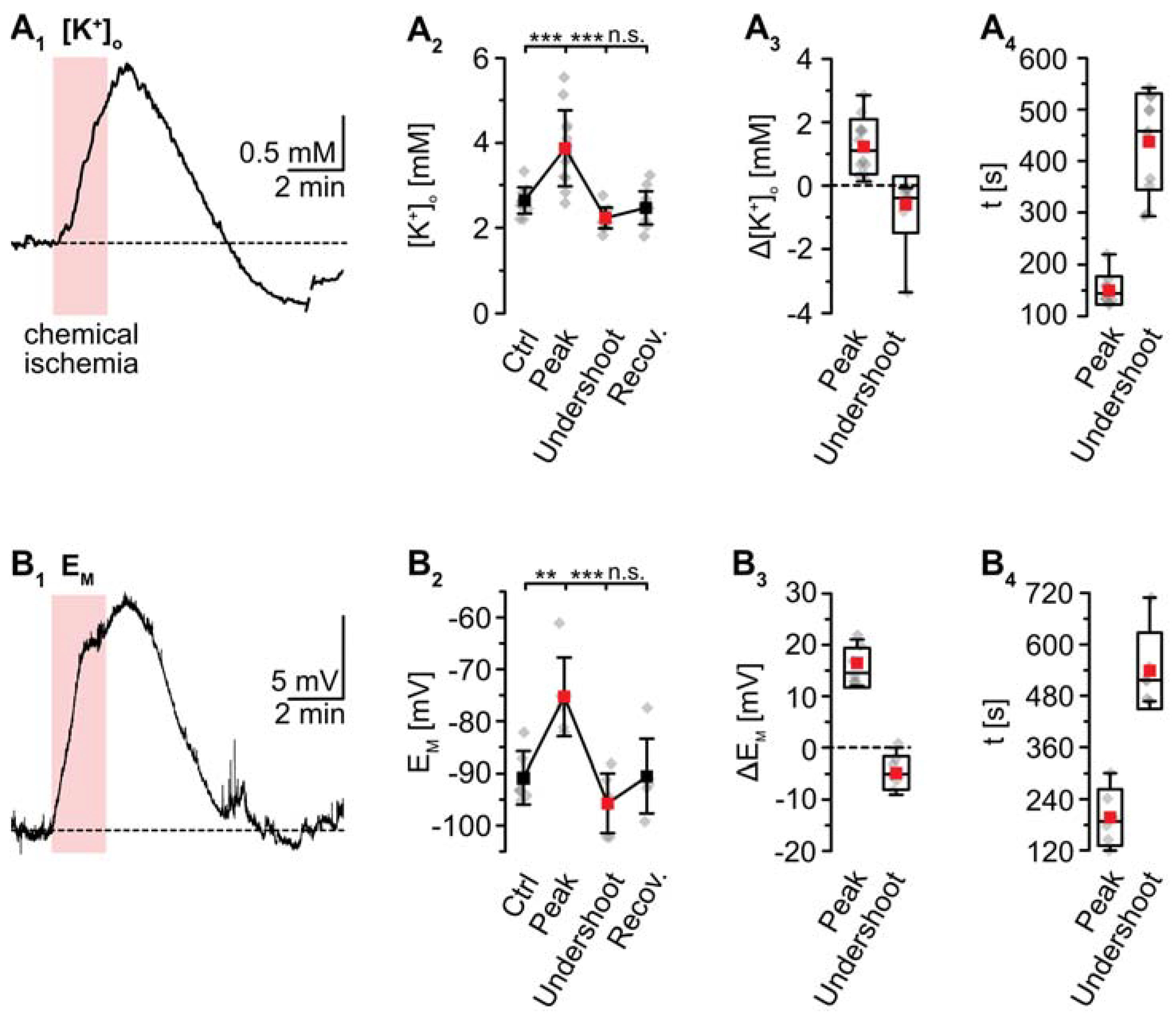
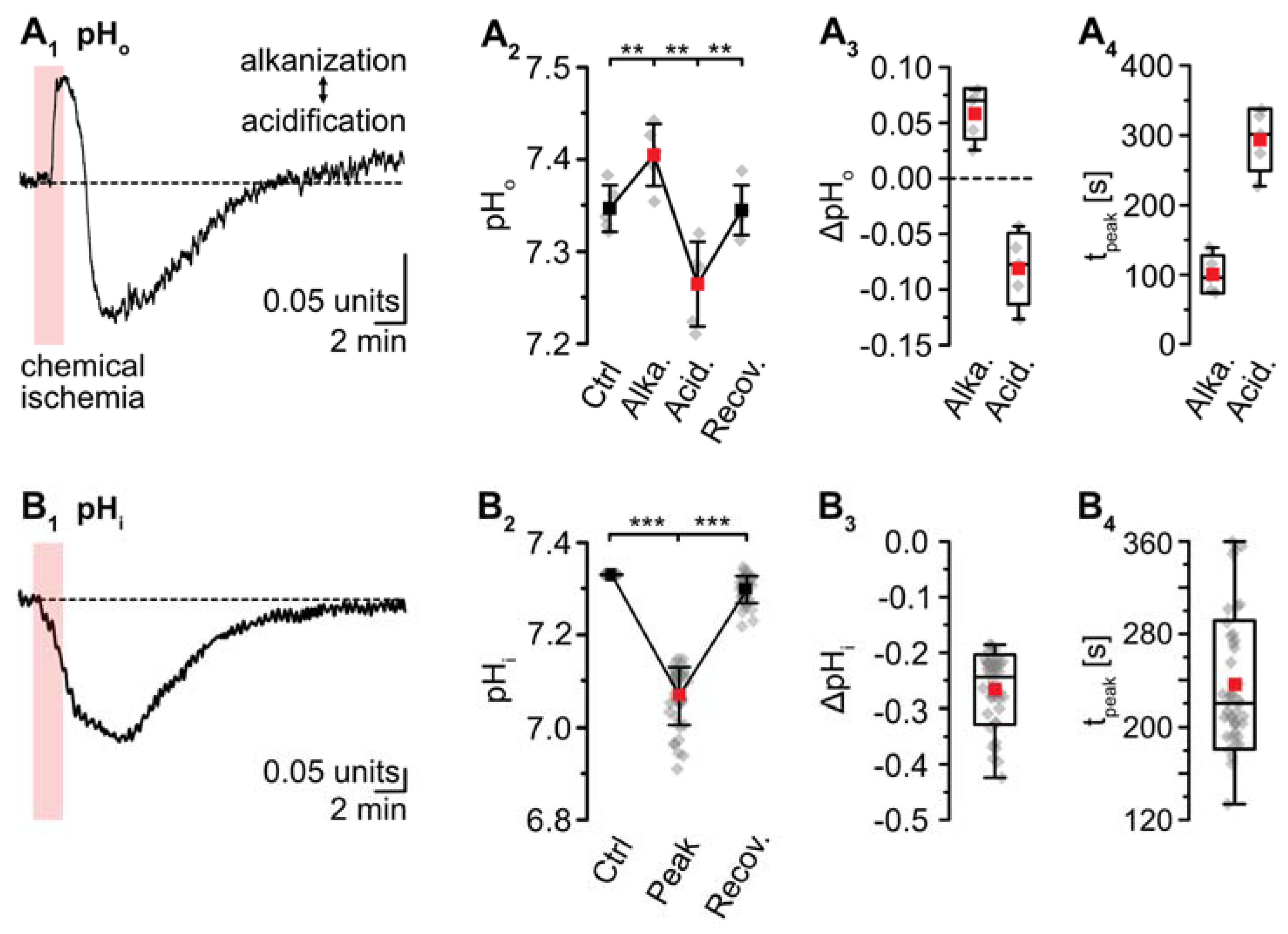
3.2. Changes of Ion Homeostasis and Astrocytic Membrane Potential during Chemical Ischemia
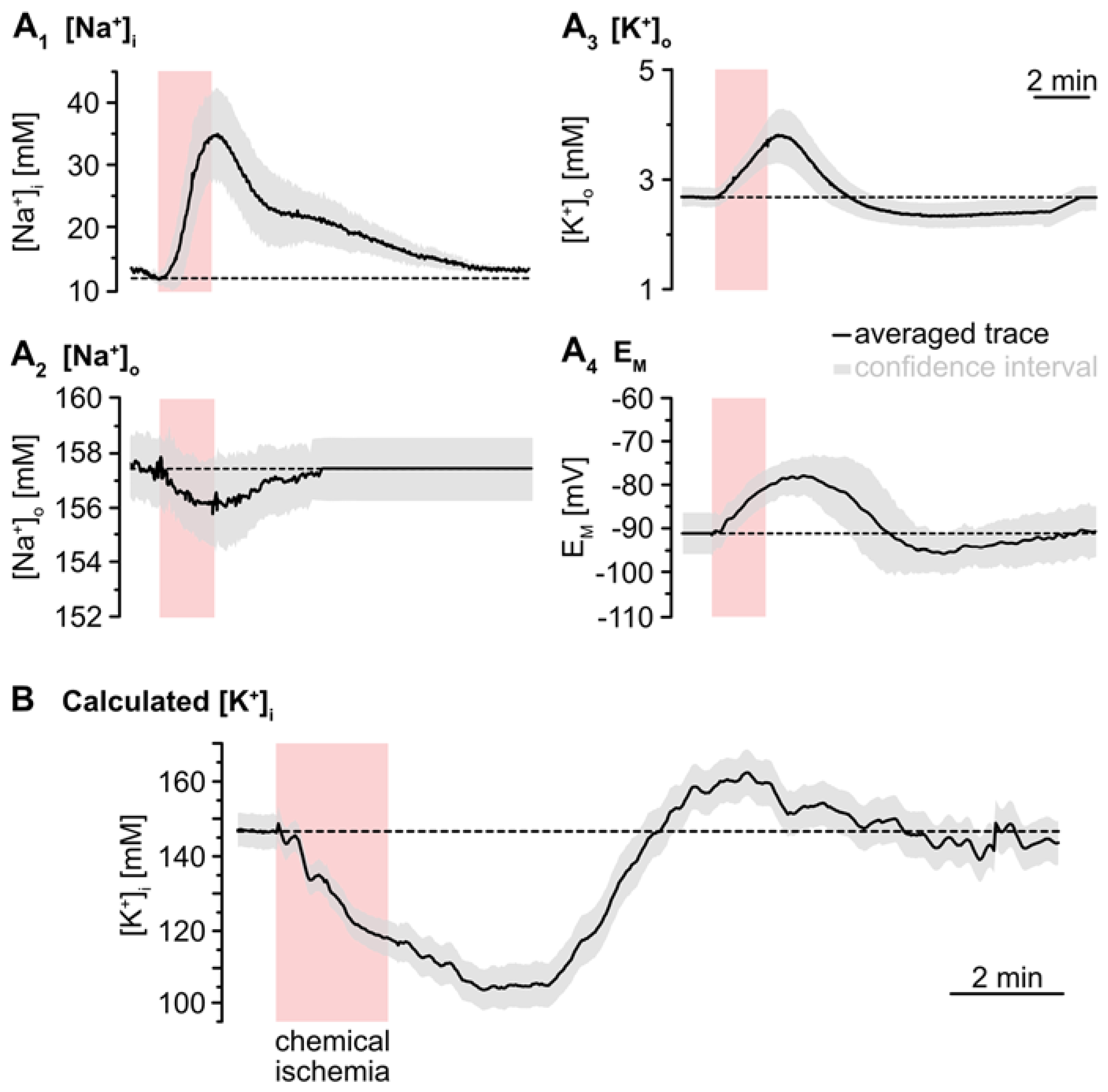
3.3. Simulation of Changes in Astrocytic K+ Concentration upon Transient Chemical Ischemia
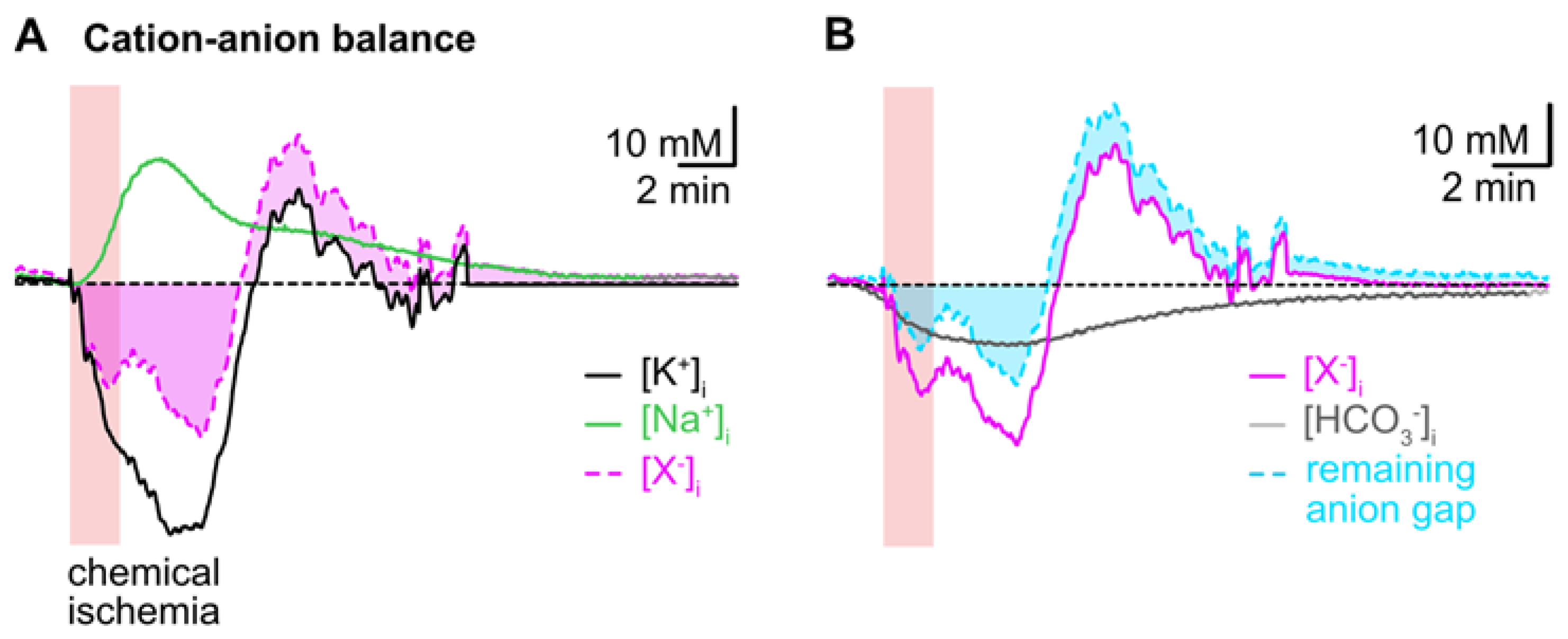
3.4. Cation–Anion Balance during Transient Chemical Ischemia
3.5. Summary of Results
4. Discussion
4.1. Baseline [K+]i of Astrocytes
4.2. Changes in Astroglial [K+]i during Energy Deprivation
4.3. Astroglial Cation–Anion Balance during Energy Deprivation
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Verkhratsky, A.; Nedergaard, M. Physiology of Astroglia. Physiol. Rev. 2018, 98, 239–389. [Google Scholar] [CrossRef] [PubMed]
- Heinemann, U.; Lux, H.D. Ceiling of stimulus induced rises in extracellular potassium concentration in the cerebral cortex of cat. Brain Res. 1977, 120, 231–249. [Google Scholar] [CrossRef]
- Hoppe, D.; Chvatal, A.; Kettenmann, H.; Orkand, R.K.; Ransom, B.R. Characteristics of activity-dependent potassium accumulation in mammalian peripheral nerve in vitro. Brain Res. 1991, 552, 106–112. [Google Scholar] [CrossRef]
- Jendelova, P.; Sykova, E. Role of glia in K+ and pH homeostasis in the neonatal rat spinal cord. Glia 1991, 4, 56–63. [Google Scholar] [CrossRef]
- Somjen, G.G. Ions in the Brain: Normal Function, Seizures, and Stroke; Oxford University Press: New York, NY, USA, 2004. [Google Scholar]
- Hansen, A.J. Effect of anoxia on ion distribution in the brain. Physiol. Rev. 1985, 65, 101–148. [Google Scholar] [CrossRef]
- Kofuji, P.; Newman, E.A. Potassium buffering in the central nervous system. Neuroscience 2004, 129, 1045–1056. [Google Scholar] [CrossRef] [Green Version]
- Bellot-Saez, A.; Kekesi, O.; Morley, J.W.; Buskila, Y. Astrocytic modulation of neuronal excitability through K+ spatial buffering. Neurosci. Biobehav. Rev. 2017, 77, 87–97. [Google Scholar] [CrossRef]
- Larsen, B.R.; Stoica, A.; MacAulay, N. Managing Brain Extracellular K+ during Neuronal Activity: The Physiological Role of the Na+/K+-ATPase Subunit Isoforms. Front. Physiol. 2016, 7, 141. [Google Scholar] [CrossRef] [Green Version]
- Hertz, L.; Song, D.; Xu, J.; Peng, L.; Gibbs, M.E. Role of the Astrocytic Na+, K+-ATPase in K+ Homeostasis in Brain: K+ Uptake, Signaling Pathways and Substrate Utilization. Neurochem. Res. 2015, 40, 2505–2516. [Google Scholar] [CrossRef]
- Leis, J.A.; Bekar, L.K.; Walz, W. Potassium homeostasis in the ischemic brain. Glia 2005, 50, 407–416. [Google Scholar] [CrossRef]
- van Putten, M.J.A.M.; Fahlke, C.; Kafitz, K.W.; Hofmeijer, J.; Rose, C.R. Dysregulation of astrocyte ion homeostasis and its relevance for stroke-induced brain damage. Int. J. Mol. Sci. 2021, 22, 5679. [Google Scholar] [CrossRef] [PubMed]
- Erecinska, M.; Silver, I.A. Ions and energy in mammalian brain. Prog. Neurobiol. 1994, 43, 37–71. [Google Scholar] [CrossRef]
- Du, Y.; Wang, W.; Lutton, A.D.; Kiyoshi, C.M.; Ma, B.; Taylor, A.T.; Olesik, J.W.; McTigue, D.M.; Askwith, C.C.; Zhou, M. Dissipation of transmembrane potassium gradient is the main cause of cerebral ischemia-induced depolarization in astrocytes and neurons. Exp. Neurol. 2018, 303, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Moskowitz, M.A.; Lo, E.H.; Iadecola, C. The science of stroke: Mechanisms in search of treatments. Neuron 2010, 67, 181–198. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pietrobon, D.; Moskowitz, M.A. Chaos and commotion in the wake of cortical spreading depression and spreading depolarizations. Nat. Rev. Neurosci. 2014, 15, 379–393. [Google Scholar] [CrossRef]
- Shuttleworth, C.W.; Andrew, R.D.; Akbari, Y.; Ayata, C.; Balu, R.; Brennan, K.C.; Boutelle, M.; Carlson, A.P.; Dreier, J.P.; Fabricius, M.; et al. Which Spreading Depolarizations Are Deleterious To Brain Tissue? Neurocrit. Care 2020, 32, 317–322. [Google Scholar] [CrossRef]
- Lauritzen, M.; Dreier, J.P.; Fabricius, M.; Hartings, J.A.; Graf, R.; Strong, A.J. Clinical relevance of cortical spreading depression in neurological disorders: Migraine, malignant stroke, subarachnoid and intracranial hemorrhage, and traumatic brain injury. J. Cereb. Blood Flow Metab. 2011, 31, 17–35. [Google Scholar] [CrossRef]
- Gerkau, N.J.; Rakers, C.; Durry, S.; Petzold, G.C.; Rose, C.R. Reverse NCX Attenuates Cellular Sodium Loading in Metabolically Compromised Cortex. Cereb. Cortex 2018, 28, 4264–4280. [Google Scholar] [CrossRef]
- Rakers, C.; Schmid, M.; Petzold, G.C. TRPV4 channels contribute to calcium transients in astrocytes and neurons during peri-infarct depolarizations in a stroke model. Glia 2017, 65, 1550–1561. [Google Scholar] [CrossRef]
- Rossi, D.J.; Brady, J.D.; Mohr, C. Astrocyte metabolism and signaling during brain ischemia. Nat. Neurosci. 2007, 10, 1377–1386. [Google Scholar] [CrossRef]
- Haack, N.; Durry, S.; Kafitz, K.W.; Chesler, M.; Rose, C.R. Double-barreled and concentric microelectrodes for measurement of extracellular ion signals in brain tissue. J. Vis. Exp. 2015, 103, e53058. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rimmele, T.S.; Chatton, J.Y. A novel optical intracellular imaging approach for potassium dynamics in astrocytes. PLoS ONE 2014, 9, e109243. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wellbourne-Wood, J.; Rimmele, T.S.; Chatton, J.Y. Imaging extracellular potassium dynamics in brain tissue using a potassium-sensitive nanosensor. Neurophotonics 2017, 4, 015002. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bischof, H.; Rehberg, M.; Stryeck, S.; Artinger, K.; Eroglu, E.; Waldeck-Weiermair, M.; Gottschalk, B.; Rost, R.; Deak, A.T.; Niedrist, T.; et al. Novel genetically encoded fluorescent probes enable real-time detection of potassium in vitro and in vivo. Nat. Commun. 2017, 8, 1422. [Google Scholar] [CrossRef] [PubMed]
- Shen, Y.; Wu, S.Y.; Rancic, V.; Aggarwal, A.; Qian, Y.; Miyashita, S.I.; Ballanyi, K.; Campbell, R.E.; Dong, M. Genetically encoded fluorescent indicators for imaging intracellular potassium ion concentration. Commun. Biol. 2019, 2, 18. [Google Scholar] [CrossRef] [Green Version]
- Kimelberg, H.K.; Bowman, C.; Biddlecome, S.; Bourke, R.S. Cation transport and membrane potential properties of primary astroglial cultures from neonatal rat brains. Brain Res. 1979, 177, 533–550. [Google Scholar] [CrossRef]
- Dietzel, I.; Heinemann, U. Dynamic variations of the brain cell microenvironment in relation to neuronal hyperactivity. Ann. N. Y. Acad. Sci. 1986, 481, 72–86. [Google Scholar] [CrossRef]
- Kalia, M.; Meijer, H.G.E.; van Gils, S.A.; van Putten, M.J.A.M.; Rose, C.R. Ion dynamics at the energy-deprived tripartite synapse. PLoS Comput. Biol. 2021, 17, e1009019. [Google Scholar] [CrossRef]
- Ullah, G.; Wei, Y.; Dahlem, M.A.; Wechselberger, M.; Schiff, S.J. The Role of Cell Volume in the Dynamics of Seizure, Spreading Depression, and Anoxic Depolarization. PLoS Comput. Biol. 2015, 11, e1004414. [Google Scholar] [CrossRef]
- Halnes, G.; Ostby, I.; Pettersen, K.H.; Omholt, S.W.; Einevoll, G.T. Electrodiffusive model for astrocytic and neuronal ion concentration dynamics. PLoS Comput. Biol. 2013, 9, e1003386. [Google Scholar] [CrossRef] [Green Version]
- Breslin, K.; Wade, J.J.; Wong-Lin, K.; Harkin, J.; Flanagan, B.; Van Zalinge, H.; Hall, S.; Walker, M.; Verkhratsky, A.; McDaid, L. Potassium and sodium microdomains in thin astroglial processes: A computational model study. PLoS Comput. Biol. 2018, 14, e1006151. [Google Scholar] [CrossRef] [PubMed]
- Stoppini, L.; Buchs, P.A.; Muller, D. A simple method for organotypic cultures of nervous tissue. J. Neurosci. Methods 1991, 37, 173–182. [Google Scholar] [CrossRef]
- Lerchundi, R.; Kafitz, K.W.; Winkler, U.; Farfers, M.; Hirrlinger, J.; Rose, C.R. FRET-based imaging of intracellular ATP in organotypic brain slices. J. Neurosci. Res. 2019, 97, 933–945. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kafitz, K.W.; Meier, S.D.; Stephan, J.; Rose, C.R. Developmental profile and properties of sulforhodamine 101-labeled glial cells in acute brain slices of rat hippocampus. J. Neurosci. Methods 2008, 169, 84–92. [Google Scholar] [CrossRef] [PubMed]
- Engels, M.; Kalia, M.; Rahmati, S.; Petersilie, L.; Kovermann, P.; van Putten, M.; Rose, C.R.; Meijer, H.G.E.; Gensch, T.; Fahlke, C. Glial chloride homeostasis under transient ischemic stress. Front. Cell. Neurosci. 2021, 15, 735300. [Google Scholar] [CrossRef]
- Imamura, H.; Huynh Nhat, K.P.; Togawa, H.; Saito, K.; Iino, R.; Kato-Yamada, Y.; Nagai, T.; Noji, H. Visualization of ATP levels inside single living cells with fluorescence resonance energy transfer-based genetically encoded indicators. Proc. Natl. Acad. Sci. USA 2009, 106, 15651–15656. [Google Scholar] [CrossRef] [Green Version]
- Langer, J.; Rose, C.R. Synaptically induced sodium signals in hippocampal astrocytes in situ. J. Physiol. 2009, 587, 5859–5877. [Google Scholar] [CrossRef]
- Kelly, T.; Rose, C.R. Ammonium influx pathways into astrocytes and neurones of hippocampal slices. J. Neurochem. 2010, 115, 1123–1136. [Google Scholar] [CrossRef]
- Mondragao, M.A.; Schmidt, H.; Kleinhans, C.; Langer, J.; Kafitz, K.W.; Rose, C.R. Extrusion versus diffusion: Mechanisms for recovery from sodium loads in mouse CA1 pyramidal neurons. J. Physiol. 2016, 594, 5507–5527. [Google Scholar] [CrossRef] [Green Version]
- Ziemens, D.; Oschmann, F.; Gerkau, N.J.; Rose, C.R. Heterogeneity of activity-induced sodium transients between astrocytes of the mouse hippocampus and neocortex: Mechanisms and consequences. J. Neurosci. 2019, 39, 2620–2634. [Google Scholar] [CrossRef] [Green Version]
- Perkins, K.L. Cell-attached voltage-clamp and current-clamp recording and stimulation techniques in brain slices. J. Neurosci. Methods 2006, 154, 1–18. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Stephan, J.; Haack, N.; Kafitz, K.W.; Durry, S.; Koch, D.; Hochstrate, P.; Seifert, G.; Steinhauser, C.; Rose, C.R. Kir4.1 channels mediate a depolarization of hippocampal astrocytes under hyperammonemic conditions in situ. Glia 2012, 60, 965–978. [Google Scholar] [CrossRef] [PubMed]
- Abdi, H. The Bonferroni and Šidák corrections for multiple comparisons. In Encyclopedia of Measurement and Statistics; Salkind, N., Ed.; Sage Publications: Thousand Oaks, CA, USA, 2007; pp. 103–107. [Google Scholar]
- Lerchundi, R.; Huang, N.; Rose, C.R. Quantitative imaging of changes in astrocytic and neuronal adenosine triphosphate using two different variants of ATeam. Front. Cell. Neurosci. 2020, 14, 80. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Deitmer, J.W.; Rose, C.R. Ion changes and signalling in perisynaptic glia. Brain Res. Rev. 2010, 63, 113–129. [Google Scholar] [CrossRef] [PubMed]
- Majumdar, D.; Bevensee, M.O. Na-coupled bicarbonate transporters of the solute carrier 4 family in the nervous system: Function, localization, and relevance to neurologic function. Neuroscience 2010, 171, 951–972. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Theparambil, S.M.; Ruminot, I.; Schneider, H.P.; Shull, G.E.; Deitmer, J.W. The electrogenic sodium bicarbonate cotransporter NBCe1 is a high-affinity bicarbonate carrier in cortical astrocytes. J. Neurosci. 2014, 34, 1148–1157. [Google Scholar] [CrossRef]
- Kaila, K.; Saarikoski, J.; Voipio, J. Mechanism of action of GABA on intracellular pH and on surface pH in crayfish muscle fibres. J. Physiol. 1990, 427, 241–260. [Google Scholar] [CrossRef] [Green Version]
- Somjen, G.G. Ion regulation in the brain: Implications for pathophysiology. Neuroscientist 2002, 8, 254–267. [Google Scholar] [CrossRef]
- Walz, W.; Mukerji, S. KCl movements during potassium-induced cytotoxic swelling of cultured astrocytes. Exp. Neurol. 1988, 99, 17–29. [Google Scholar] [CrossRef]
- Ballanyi, K.; Grafe, P.; ten Bruggencate, G. Ion activities and potassium uptake mechanisms of glial cells in guinea-pig olfactory cortex slices. J. Physiol. 1987, 382, 159–174. [Google Scholar] [CrossRef]
- Grafe, P.; Ballanyi, K. Cellular mechanisms of potassium homeostasis in the mammalian nervous system. Can. J. Physiol. Pharmacol. 1987, 65, 1038–1042. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kettenmann, H.; Sonnhof, U.; Schachner, M. Exclusive potassium dependence of the membrane potential in cultured mouse oligodendrocytes. J. Neurosci. 1983, 3, 500–505. [Google Scholar] [CrossRef] [PubMed]
- Buhrle, C.P.; Sonnhof, U. Intracellular ion activities and equilibrium potentials in motoneurones and glia cells of the frog spinal cord. Pflug. Arch. 1983, 396, 144–153. [Google Scholar] [CrossRef] [PubMed]
- Zhou, M.; Du, Y.; Aten, S.; Terman, D. On the electrical passivity of astrocyte potassium conductance. J. Neurophysiol. 2021, 126, 1403–1419. [Google Scholar] [CrossRef]
- Ransom, B.R.; Goldring, S. Ionic determinants of membrane potential of cells presumed to be glia in cerebral cortex of cat. J. Neurophysiol. 1973, 36, 855–868. [Google Scholar] [CrossRef]
- Xie, M.; Wang, W.; Kimelberg, H.K.; Zhou, M. Oxygen and glucose deprivation-induced changes in astrocyte membrane potential and their underlying mechanisms in acute rat hippocampal slices. J. Cereb. Blood Flow Metab. 2008, 28, 456–467. [Google Scholar] [CrossRef]
- Walz, W. Role of astrocytes in the clearance of excess extracellular potassium. Neurochem. Int. 2000, 36, 291–300. [Google Scholar] [CrossRef]
- Ransom, C.B.; Ransom, B.R.; Sontheimer, H. Activity-dependent extracellular K+ accumulation in rat optic nerve: The role of glial and axonal Na+ pumps. J. Physiol. 2000, 522 Pt 3, 427–442. [Google Scholar] [CrossRef]
- Sibille, J.; Pannasch, U.; Rouach, N. Astroglial potassium clearance contributes to short-term plasticity of synaptically evoked currents at the tripartite synapse. J. Physiol. 2014, 592, 87–102. [Google Scholar] [CrossRef]
- MacAulay, N. Molecular mechanisms of K+ clearance and extracellular space shrinkage-Glia cells as the stars. Glia 2020, 68, 2192–2211. [Google Scholar] [CrossRef]
- Ekholm, A.; Katsura, K.; Kristian, T.; Liu, M.; Folbergrova, J.; Siesjo, B.K. Coupling of cellular energy state and ion homeostasis during recovery following brain ischemia. Brain Res. 1993, 604, 185–191. [Google Scholar] [CrossRef]
- Murphy, T.H.; Corbett, D. Plasticity during stroke recovery: From synapse to behaviour. Nat. Rev. Neurosci. 2009, 10, 861–872. [Google Scholar] [CrossRef] [PubMed]
- Mies, G.; Paschen, W. Regional changes of blood flow, glucose, and ATP content determined on brain sections during a single passage of spreading depression in rat brain cortex. Exp. Neurol. 1984, 84, 249–258. [Google Scholar] [CrossRef]
- Rose, C.R.; Waxman, S.G.; Ransom, B.R. Effects of glucose deprivation, chemical hypoxia, and simulated ischemia on Na+ homeostasis in rat spinal cord astrocytes. J. Neurosci. 1998, 18, 3554–3562. [Google Scholar] [CrossRef] [Green Version]
- Muller, M.; Somjen, G.G. Na+ and K+ concentrations, extra- and intracellular voltages, and the effect of TTX in hypoxic rat hippocampal slices. J. Neurophysiol. 2000, 83, 735–745. [Google Scholar] [CrossRef]
- Gerkau, N.J.; Lerchundi, R.; Nelson, J.S.E.; Lantermann, M.; Meyer, J.; Hirrlinger, J.; Rose, C.R. Relation between activity-induced intracellular sodium transients and ATP dynamics in mouse hippocampal neurons. J. Physiol. 2019, 597, 5687–5705. [Google Scholar] [CrossRef] [Green Version]
- Wang, F.; Smith, N.A.; Xu, Q.; Fujita, T.; Baba, A.; Matsuda, T.; Takano, T.; Bekar, L.; Nedergaard, M. Astrocytes modulate neural network activity by Ca2+-dependent uptake of extracellular K+. Sci. Signal. 2012, 5, ra26. [Google Scholar] [CrossRef] [Green Version]
- Kintner, D.B.; Wang, Y.; Sun, D. Role of membrane ion transport proteins in cerebral ischemic damage. Front. Biosci. 2007, 12, 762–770. [Google Scholar] [CrossRef] [Green Version]
- Tyurikova, O.; Shih, P.Y.; Dembitskaya, Y.; Savtchenko, L.P.; McHugh, T.J.; Rusakov, D.A.; Semyanov, A. K+ efflux through postsynaptic NMDA receptors suppresses local astrocytic glutamate uptake. Glia 2022, 70, 961–974. [Google Scholar] [CrossRef]
- Wilson, C.S.; Mongin, A.A. The signaling role for chloride in the bidirectional communication between neurons and astrocytes. Neurosci. Lett. 2019, 689, 33–44. [Google Scholar] [CrossRef]
- Woo, J.; Jang, M.W.; Lee, J.; Koh, W.; Mikoshiba, K.; Lee, C.J. The molecular mechanism of synaptic activity-induced astrocytic volume transient. J. Physiol. 2020, 598, 4555–4572. [Google Scholar] [CrossRef] [PubMed]
- Benesova, J.; Rusnakova, V.; Honsa, P.; Pivonkova, H.; Dzamba, D.; Kubista, M.; Anderova, M. Distinct expression/function of potassium and chloride channels contributes to the diverse volume regulation in cortical astrocytes of GFAP/EGFP mice. PLoS ONE 2012, 7, e29725. [Google Scholar] [CrossRef]
- Kahle, K.T.; Simard, J.M.; Staley, K.J.; Nahed, B.V.; Jones, P.S.; Sun, D. Molecular mechanisms of ischemic cerebral edema: Role of electroneutral ion transport. Physiol. (Bethesda) 2009, 24, 257–265. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kimelberg, H.K. Astrocytic swelling in cerebral ischemia as a possible cause of injury and target for therapy. Glia 2005, 50, 389–397. [Google Scholar] [CrossRef]
- Risher, W.C.; Croom, D.; Kirov, S.A. Persistent astroglial swelling accompanies rapid reversible dendritic injury during stroke-induced spreading depolarizations. Glia 2012, 60, 1709–1720. [Google Scholar] [CrossRef] [Green Version]
- Risher, W.C.; Andrew, R.D.; Kirov, S.A. Real-time passive volume responses of astrocytes to acute osmotic and ischemic stress in cortical slices and in vivo revealed by two-photon microscopy. Glia 2009, 57, 207–221. [Google Scholar] [CrossRef] [Green Version]
- Hyzinski-Garcia, M.C.; Rudkouskaya, A.; Mongin, A.A. LRRC8A protein is indispensable for swelling-activated and ATP-induced release of excitatory amino acids in rat astrocytes. J. Physiol. 2014, 592, 4855–4862. [Google Scholar] [CrossRef]
- Pasantes-Morales, H.; Vazquez-Juarez, E. Transporters and channels in cytotoxic astrocyte swelling. Neurochem. Res. 2012, 37, 2379–2387. [Google Scholar] [CrossRef]




| Parameter | Control | Chemical Ischemia | Δ | n | p | |
|---|---|---|---|---|---|---|
| [ATP]o [%] | 100 | 82.7 ± 5.3 | −17.3 ± 5.3 | 27/4/3 | n.a. | |
| [Na+]o [mM] | 157.4 ± 1.5 | 155.5 ± 1.9 | −1.9 ± 1.0 (−1%) | 22/22/11 | 3 × 10−3 | ** |
| [Na+]i [mM] | 12.1 ± 2.9 § | 36.5 ± 7.2 | +24.4 ± 7.2 (+201%) | 34/4/4 | 2 × 10−29 | *** |
| [K+]o [mM] | 2.7 ± 0.3 | 3.9 ± 0.9 | +1.7 ± 0.9 (+46%) | 12/12/9 | 2 × 10−4 | *** |
| [K+]i [mM] | 146.2 | 103.4 | −42.8 (−29%) | n.a. | n.a. | |
| EM [mV] | −90.7 ± 5.1 | −75.2 ± 7.6 | +15.5 ± 3.8 (−17%) | 6/6/4 | 0.003 | ** |
| pHo | 7.35 ± 0.03 | 7.41 ± 0.03 | +0.05 ± 0.03 (+1%) | 5/5/4 | 0.016 | * |
| 7.27 ± 0.05 | −0.08 ± 0.03 (−1%) | 8 × 10−4 | ** | |||
| pHi | 7.33 ± 0.26 | 7.07 ± 0.06 | −0.26 ± 0.06 (−4%) | 42/5/5 | 4 × 10−43 | *** |
| [HCO3−]o [mM] | 23.0 | 25.9 | +2.9 (+13%) | n.a. | n.a. | |
| 19.2 | −3.8 (−17%) | |||||
| [HCO3−]i [mM] | 22.1 | 11.6 | −10.5 (−52%) | n.a. | n.a. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Eitelmann, S.; Stephan, J.; Everaerts, K.; Durry, S.; Pape, N.; Gerkau, N.J.; Rose, C.R. Changes in Astroglial K+ upon Brief Periods of Energy Deprivation in the Mouse Neocortex. Int. J. Mol. Sci. 2022, 23, 4836. https://doi.org/10.3390/ijms23094836
Eitelmann S, Stephan J, Everaerts K, Durry S, Pape N, Gerkau NJ, Rose CR. Changes in Astroglial K+ upon Brief Periods of Energy Deprivation in the Mouse Neocortex. International Journal of Molecular Sciences. 2022; 23(9):4836. https://doi.org/10.3390/ijms23094836
Chicago/Turabian StyleEitelmann, Sara, Jonathan Stephan, Katharina Everaerts, Simone Durry, Nils Pape, Niklas J. Gerkau, and Christine R. Rose. 2022. "Changes in Astroglial K+ upon Brief Periods of Energy Deprivation in the Mouse Neocortex" International Journal of Molecular Sciences 23, no. 9: 4836. https://doi.org/10.3390/ijms23094836
APA StyleEitelmann, S., Stephan, J., Everaerts, K., Durry, S., Pape, N., Gerkau, N. J., & Rose, C. R. (2022). Changes in Astroglial K+ upon Brief Periods of Energy Deprivation in the Mouse Neocortex. International Journal of Molecular Sciences, 23(9), 4836. https://doi.org/10.3390/ijms23094836







