Exploring the Non-Covalent Bonding in Water Clusters
Abstract
1. Introduction
2. Results and Discussion
2.1. Geometric and Energetic Features of Water Clusters
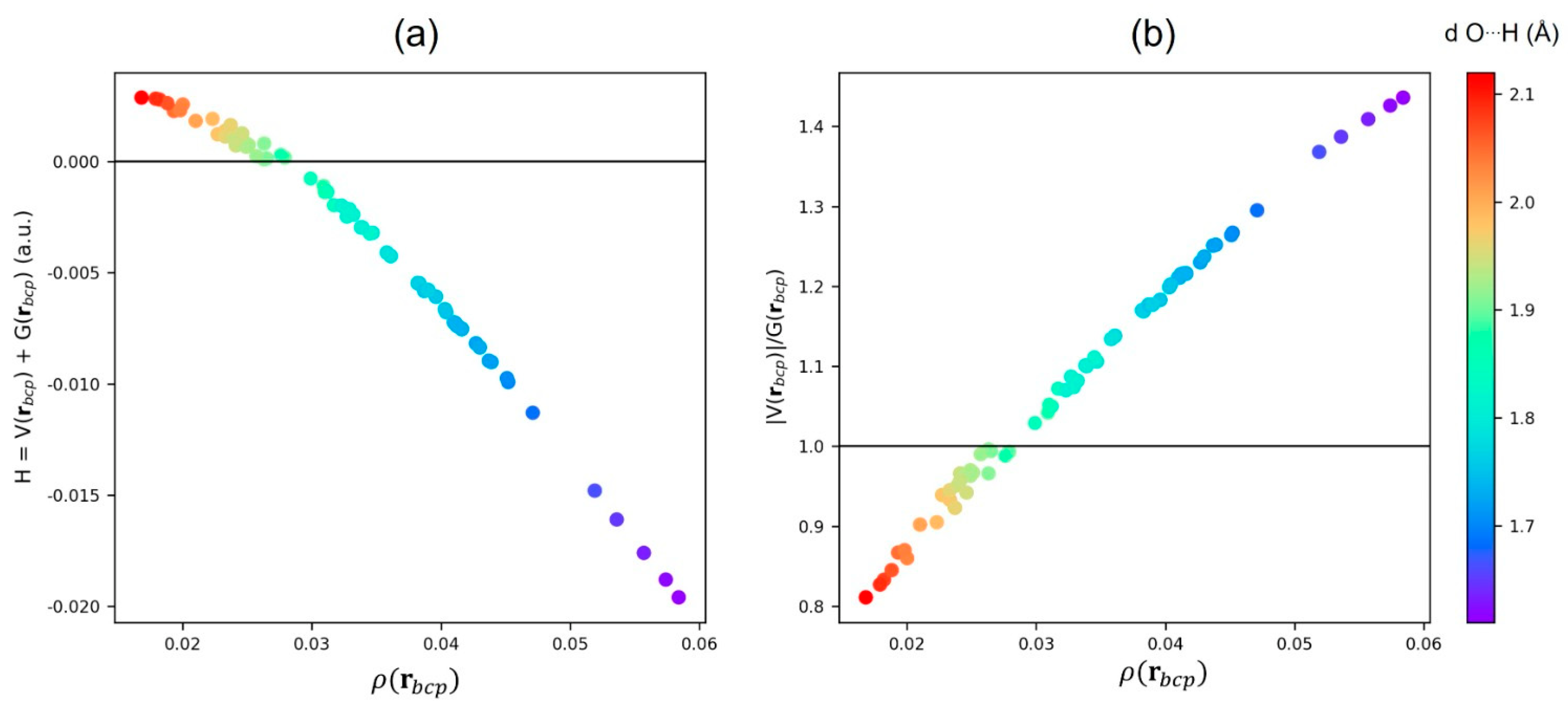
2.2. QTAIM
2.3. Source Function Analysis
3. Models and Methods
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sanderson, R.T. Chemical Bonds and Bond Energy, 2nd ed.; Academic Press: Cambridge, MA, USA, 1976. [Google Scholar]
- Shaik, S. The Lewis legacy: The chemical bond—A territory and heartland of chemistry. J. Comput. Chem. 2006, 28, 51–61. [Google Scholar] [CrossRef] [PubMed]
- Carey, F.A.; Sundberg, R.J. Chemical Bonding and Molecular Structure. In Advanced Organic Chemistry; Springer: Boston, MA, USA, 2007; pp. 1–117. [Google Scholar]
- Westwell, M.S.; Searle, M.S.; Wales, D.J.; Williams, D.H. Empirical Correlations between Thermodynamic Properties and Intermolecular Forces. J. Am. Chem. Soc. 1995, 117, 5013–5015. [Google Scholar] [CrossRef]
- Widom, B. Intermolecular Forces and the Nature of the Liquid State. Science 1967, 157, 375–382. [Google Scholar] [CrossRef] [PubMed]
- Rowlinson, J.S. Intermolecular forces and some properties of matter. Q. Rev. Chem. Soc. 1954, 8, 168–191. [Google Scholar] [CrossRef]
- Liu, G.; Wei, S.-H.; Zhang, C. Review of the Intermolecular Interactions in Energetic Molecular Cocrystals. Cryst. Growth Des. 2020, 20, 7065–7079. [Google Scholar] [CrossRef]
- Kaur, R.; Cavanagh, K.; Rodríguez-Hornedo, N.; Matzger, A.J. Multidrug Cocrystal of Anticonvulsants: Influence of Strong Intermolecular Interactions on Physiochemical Properties. Cryst. Growth Des. 2017, 17, 5012–5016. [Google Scholar] [CrossRef]
- Stone, A. The Theory of Intermolecular Forces, 2nd ed.; Oxford University Press: Oxford, UK, 2013. [Google Scholar]
- Saleh, G.; Gatti, C.; Presti, L.L.; Contreras-Garcia, J. Revealing Non-Covalent Interactions in Molecular Crystals through Their Experimental Electron Densities. Chem. Eur. J. 2012, 18, 15523–15536. [Google Scholar] [CrossRef]
- Beran, G.J.O.; Heit, Y.N.; Hartman, J.D. Noncovalent Interactions in Molecular Crystals. In Non-Covalent Interactions in Quantum Chemistry and Physics; Elsevier: Amsterdam, The Netherlands, 2017; pp. 303–331. [Google Scholar]
- Karshikoff, A. Non-Covalent Interactions in Proteins; Imperial College Press: London, UK, 2006; ISBN 978-1-86094-707-0. [Google Scholar]
- Kollman, P. Chapter 2 Non-Covalent Forces of Importance in Biochemistry. In New Comprehensive Biochemistry; Elsevier: Amsterdam, The Netherlands, 1984; pp. 55–71. [Google Scholar] [CrossRef]
- Oses, M.R.; De Oteyza, D.G.; Fernández-Torrente, I.; Gonzalez-Lakunza, N.; Schmidt-Weber, P.M.; Kampen, T.; Horn, K.; Gourdon, A.; Arnau, A.; Ortega, J.E. Non-Covalent Interactions in Supramolecular Assemblies Investigated with Electron Spectroscopies. ChemPhysChem 2009, 10, 896–900. [Google Scholar] [CrossRef]
- Frieden, E. Non-covalent interactions: Key to biological flexibility and specificity. J. Chem. Educ. 1975, 52, 754–756. [Google Scholar] [CrossRef]
- Weinhold, F.; Klein, R.A. What is a hydrogen bond? Mutually consistent theoretical and experimental criteria for characterizing H-bonding interactions. Mol. Phys. 2012, 110, 565–579. [Google Scholar] [CrossRef]
- Perrin, C.L.; Nielson, J.B. “Strong” Hydrogen Bonds in Chemistry and Biology. Annu. Rev. Phys. Chem. 1997, 48, 511–544. [Google Scholar] [CrossRef]
- Rincón, L.; Almeida, R.; García-Aldea, D.; Riega, H.D.Y. Hydrogen bond cooperativity and electron delocalization in hydrogen fluoride clusters. J. Chem. Phys. 2001, 114, 5552–5561. [Google Scholar] [CrossRef]
- Miriyala, V.M.; Lo, R.; Bouř, P.; Wu, T.; Nachtigallová, D.; Hobza, P. Unexpected Strengthening of the H-Bond Complexes in a Polar Solvent Due to a More Efficient Solvation of the Complex Compared to Isolated Monomers. J. Phys. Chem. A 2022, 126, 7938–7943. [Google Scholar] [CrossRef]
- Aquino, A.J.A.; Tunega, D.; Haberhauer, G.; Gerzabek, M.H.; Lischka, H. Solvent Effects on Hydrogen BondsA Theoretical Study. J. Phys. Chem. A 2002, 106, 1862–1871. [Google Scholar] [CrossRef]
- Brini, E.; Fennell, C.J.; Fernandez-Serra, M.; Hribar-Lee, B.; Lukšič, M.; Dill, K.A. How Water’s Properties Are Encoded in Its Molecular Structure and Energies. Chem. Rev. 2017, 117, 12385–12414. [Google Scholar] [CrossRef]
- Errington, J.R.; Debenedetti, P.G. Relationship between structural order and the anomalies of liquid water. Nature 2001, 409, 318–321. [Google Scholar] [CrossRef]
- Santra, B.; DiStasio, R.A., Jr.; Martelli, F.; Car, R. Local structure analysis in ab initio liquid water. Mol. Phys. 2015, 113, 2829–2841. [Google Scholar] [CrossRef]
- Marx, D.; Tuckerman, M.E.; Hutter, J.; Parrinello, M. The nature of the hydrated excess proton in water. Nature 1999, 397, 601–604. [Google Scholar] [CrossRef]
- Wang, Y.; Babin, V.; Bowman, J.M.; Paesani, F. The Water Hexamer: Cage, Prism, or Both. Full Dimensional Quantum Simulations Say Both. J. Am. Chem. Soc. 2012, 134, 11116–11119. [Google Scholar] [CrossRef]
- Howard, J.C.; Tschumper, G.S. Benchmark Structures and Harmonic Vibrational Frequencies Near the CCSD(T) Complete Basis Set Limit for Small Water Clusters: (H2O)n=2,3,4,5,6. J. Chem. Theory Comput. 2015, 11, 2126–2136. [Google Scholar] [CrossRef]
- Kelkkanen, A.K.; Lundqvist, B.I.; Nørskov, J.K. Density functional for van der Waals forces accounts for hydrogen bond in benchmark set of water hexamers. J. Chem. Phys. 2009, 131, 46102. [Google Scholar] [CrossRef] [PubMed]
- Ansari, N.; Dandekar, R.; Caravati, S.; Sosso, G.; Hassanali, A. High and low density patches in simulated liquid water. J. Chem. Phys. 2018, 149, 204507. [Google Scholar] [CrossRef] [PubMed]
- Gao, Y.; Fang, H.; Ni, K. A hierarchical clustering method of hydrogen bond networks in liquid water undergoing shear flow. Sci. Rep. 2021, 11, 9542. [Google Scholar] [CrossRef] [PubMed]
- Chaplin, M. A proposal for the structuring of water. Biophys. Chem. 2000, 83, 211–221. [Google Scholar] [CrossRef]
- Lozynski, M. Liquid water: The helical perspective of structure. Chem. Phys. 2015, 455, 1–6. [Google Scholar] [CrossRef]
- Koehler, J.E.H.; Saenger, W.; Lesyng, B. Cooperative effects in extended hydrogen bonded systems involving O?H groups.Ab initio studies of the cyclic S4 water tetramer. J. Comput. Chem. 1987, 8, 1090–1098. [Google Scholar] [CrossRef]
- Suhai, S. Cooperative effects in hydrogen bonding: Fourth-order many-body perturbation theory studies of water oligomers and of an infinite water chain as a model for ice. J. Chem. Phys. 1994, 101, 9766–9782. [Google Scholar] [CrossRef]
- Diez Riega, H.; Almeida Rincón, R.L. Interpretación de La Partición de La Energía de Muchos Cuerpos en Agregados de Agua Formados por la Interacción de Enlace de Hidrógeno. Rev. Mexic. Fís. 2006, 52, 204–207. [Google Scholar]
- Xantheas, S.S. Cooperativity and hydrogen bonding network in water clusters. Chem. Phys. 2000, 258, 225–231. [Google Scholar] [CrossRef]
- Ahirwar, M.B.; Gadre, S.R.; Deshmukh, M.M. Direct and Reliable Method for Estimating the Hydrogen Bond Energies and Cooperativity in Water Clusters, Wn, n = 3 to 8. J. Phys. Chem. A 2020, 124, 6699–6706. [Google Scholar] [CrossRef]
- Guevara-Vela, J.M.; Chávez-Calvillo, R.; García-Revilla, M.; Hernández-Trujillo, J.; Christiansen, O.; Francisco, E.; Pendás, M.; Rocha-Rinza, T. Hydrogen-Bond Cooperative Effects in Small Cyclic Water Clusters as Revealed by the Interacting Quantum Atoms Approach. Chem. A Eur. J. 2013, 19, 14304–14315. [Google Scholar] [CrossRef]
- Albrecht, L.; Boyd, R.J. Visualizing Internal Stabilization in Weakly Bound Systems Using Atomic Energies: Hydrogen Bonding in Small Water Clusters. J. Phys. Chem. A 2012, 116, 3946–3951. [Google Scholar] [CrossRef]
- la Vega, A.S.; Rocha-Rinza, T.; Guevara-Vela, J.M. Cooperativity and Anticooperativity in Ion-Water Interactions: Implications for the Aqueous Solvation of Ions. ChemPhysChem 2021, 22, 1269–1285. [Google Scholar] [CrossRef]
- Řezáč, J.; Jurečka, P.; Riley, K.E.; Černý, J.; Valdes, H.; Pluháčková, K.; Berka, K.; Řezáč, T.; Pitoňák, M.; Vondrášek, J.; et al. Quantum Chemical Benchmark Energy and Geometry Database for Molecular Clusters and Complex Molecular Systems: A Users Manual and Examples. Collect. Czechoslov. Chem. Commun. 2008, 73, 1261–1270. [Google Scholar] [CrossRef]
- Rincón, L.; Almeida, R.; Aldea, D.G. Many-body energy decomposition analysis of cooperativity in hydrogen fluoride clusters. Int. J. Quantum Chem. 2004, 102, 443–453. [Google Scholar] [CrossRef]
- Xantheas, S.S.; Burnham, C.J.; Harrison, R.J. Development of transferable interaction models for water. II. Accurate energetics of the first few water clusters from first principles. J. Chem. Phys. 2002, 116, 1493–1499. [Google Scholar] [CrossRef]
- Ghosh, S.R.; Debnath, B.; Jana, A.D. Water dimer isomers: Interaction energies and electronic structure. J. Mol. Model. 2020, 26, 20. [Google Scholar] [CrossRef]
- Narten, A.H.; Thiessen, W.E.; Blum, L. Atom Pair Distribution Functions of Liquid Water at 25 °C from Neutron Diffraction. Science 1982, 217, 1033–1034. [Google Scholar] [CrossRef]
- Eisenberg, D.; Kauzmann, W. The Structure and Properties of Water, 1st ed.; Oxford University Press: New York, NY, USA, 1969. [Google Scholar]
- Hobza, P. The Benchmark Energy & Geometry Database (BEGDB). Available online: http://www.begdb.org/ (accessed on 16 December 2022).
- Bader, R.W. Atoms in Molecules: A Quantum Theory. In International Series of Monographs on Chemistry; Oxford Science: Oxford, UK, 1990; Volume 22. [Google Scholar]
- Mora, A.J.; Belandria, L.M.; Delgado, G.E.; Seijas, L.E.; Lunar, A.; Almeida, R. Non-covalent interactions in the multicomponent crystal of 1-aminocyclopentane carboxylic acid, oxalic acid and water: A crystallographic and a theoretical approach. Acta Crystallogr. Sect. B Struct. Sci. Cryst. Eng. Mater. 2017, 73, 968–980. [Google Scholar] [CrossRef]
- Bruno-Colmenarez, J.; Atencio, R.; Quintero, M.; Seijas, L.; Almeida, R.; Rincón, L. Crystal Structure Analysis and Topological Study of Non-covalent Interactions in 2,2-Biimidazole:Salicylic Acid 2:1 Co-crystal. J. Chem. Crystallogr. 2017, 47, 47–58. [Google Scholar] [CrossRef]
- Guillén, M.; Mora, A.J.; Belandria, L.M.; Seijas, L.E.; Ramírez, J.W.; Burgos, J.L.; Rincón, L.; Delgado, G.E. Two conformational polymorphs of 4-methylhippuric acid. Acta Crystallogr. Sect. B Struct. Sci. Cryst. Eng. Mater. 2020, 76, 1077–1091. [Google Scholar] [CrossRef]
- Seijas, L.E.; Belandria, L.M.; Vivas, P.J.; Guillén, M.; Burgos, J.L.; Fitch, A.N.; Wright, J.; Rincón, L.; Delgado, G.E.; Mora, A.J. Two nickel (II) complexes with side chain isomeric ligands: L-leucine and L-isoleucine to study non-covalent interactions and metal-ligand bonding. J. Mol. Struct. 2022, 1261, 132898. [Google Scholar] [CrossRef]
- Alkorta, I.; Barrios, L.; Rozas, I.; Elguero, J. Comparison of models to correlate electron density at the bond critical point and bond distance. J. Mol. Struct. THEOCHEM 2000, 496, 131–137. [Google Scholar] [CrossRef]
- Knop, O.; Boyd, R.J.; Choi, S.C. Sulfur-sulfur bond lengths, or can a bond length be estimated from a single parameter? J. Am. Chem. Soc. 1988, 110, 7299–7301. [Google Scholar] [CrossRef]
- Knop, O.; Rankin, K.N.; Boyd, R.J. Coming to Grips with N−H⋯N Bonds. 2. Homocorrelations between Parameters Deriving from the Electron Density at the Bond Critical Point. J. Phys. Chem. A 2002, 107, 272–284. [Google Scholar] [CrossRef]
- Espinosa, E.; Alkorta, I.; Elguero, J.; Molins, E. From weak to strong interactions: A comprehensive analysis of the topological and energetic properties of the electron density distribution involving X–H⋯F–Y systems. J. Chem. Phys. 2002, 117, 5529–5542. [Google Scholar] [CrossRef]
- Cremer, D.; Kraka, E. A Description of the Chemical Bond in Terms of Local Properties of Electron Density and Energy. Croat. Chem. Acta 1984, 57, 1259–1281. [Google Scholar]
- Cremer, D.; Kraka, E. Chemical Bonds without Bonding Electron Density ? Does the Difference Electron-Density Analysis Suffice for a Description of the Chemical Bond? Angew. Chem. Int. Ed. 1984, 23, 627–628. [Google Scholar] [CrossRef]
- Kazachenko, A.S.; Issaoui, N.; Sagaama, A.; Malyar, Y.N.; Al-Dossary, O.; Bousiakou, L.G.; Kazachenko, A.S.; Miroshnokova, A.V.; Xiang, Z. Hydrogen bonds interactions in biuret-water clusters: FTIR, X-ray diffraction, AIM, DFT, RDG, ELF, NLO analysis. J. King Saud Univ. Sci. 2022, 34, 102350. [Google Scholar] [CrossRef]
- Fradera, X.; Austen, M.A.; Bader, R.F.W. The Lewis Model and Beyond. J. Phys. Chem. A 1998, 103, 304–314. [Google Scholar] [CrossRef]
- Poater, J.; Fradera, X.; Solà, M.; Duran, M.; Simon, S. On the electron-pair nature of the hydrogen bond in the framework of the atoms in molecules theory. Chem. Phys. Lett. 2003, 369, 248–255. [Google Scholar] [CrossRef]
- Fonseca Guerra, C.; Bickelhaupt, F.M. Orbital Interactions in Strong and Weak Hydrogen Bonds are Essential for DNA Replication. Angew. Chem. Int. Ed. 2002, 41, 2092. [Google Scholar] [CrossRef]
- Bader, R.F.; Gatti, C. A Green’s function for the density. Chem. Phys. Lett. 1998, 287, 233–238. [Google Scholar] [CrossRef]
- Rohman, S.S.; Kashyap, C.; Ullah, S.S.; Mazumder, L.J.; Sahu, P.P.; Kalita, A.; Reza, S.; Hazarika, P.P.; Borah, B.; Guha, A.K. Revisiting ultra-weak metal-metal bonding. Chem. Phys. Lett. 2019, 730, 411–415. [Google Scholar] [CrossRef]
- Gatti, C. The Source Function Descriptor as a Tool to Extract Chemical Information from Theoretical and Experimental Electron Densities. In Electron Density and Chemical Bonding II: Theoretical Charge Density Studies; Stalke, D., Ed.; Springer: Berlin/Heidelberg, Germany, 2012; pp. 193–285. ISBN 978-3-642-30808-6. [Google Scholar]
- Monza, E.; Gatti, C.; Presti, L.L.; Ortoleva, E. Revealing Electron Delocalization through the Source Function. J. Phys. Chem. A 2011, 115, 12864–12878. [Google Scholar] [CrossRef]
- Gatti, C.; Bertini, L. The local form of the source function as a fingerprint of strong and weak intra- and intermolecular interactions. Acta Crystallogr. Sect. A Found. Crystallogr. 2004, 60, 438–449. [Google Scholar] [CrossRef]
- Gatti, C.; Cargnoni, F.; Bertini, L. Chemical information from the source function. J. Comput. Chem. 2003, 24, 422–436. [Google Scholar] [CrossRef]
- Řezáč, J. Non-Covalent Interactions Atlas Benchmark Data Sets 2: Hydrogen Bonding in an Extended Chemical Space. J. Chem. Theory Comput. 2020, 16, 6305–6316. [Google Scholar] [CrossRef]
- Goerigk, L.; Kruse, H.; Grimme, S. Benchmarking Density Functional Methods against the S66 and S66x8 Datasets for Non-Covalent Interactions. ChemPhysChem 2011, 12, 3421–3433. [Google Scholar] [CrossRef]
- Zhao, Y.; Truhlar, D.G. The M06 suite of density functionals for main group thermochemistry, thermochemical kinetics, noncovalent interactions, excited states, and transition elements: Two new functionals and systematic testing of four M06-class functionals and 12 other functionals. Theor. Chem. Acc. 2008, 120, 215–241. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian16 Revision C.01; Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Keith, T.A. AIMAll, Version 19.10.12; TK Gristmill Software: Overland Park, KS, USA, 2019.
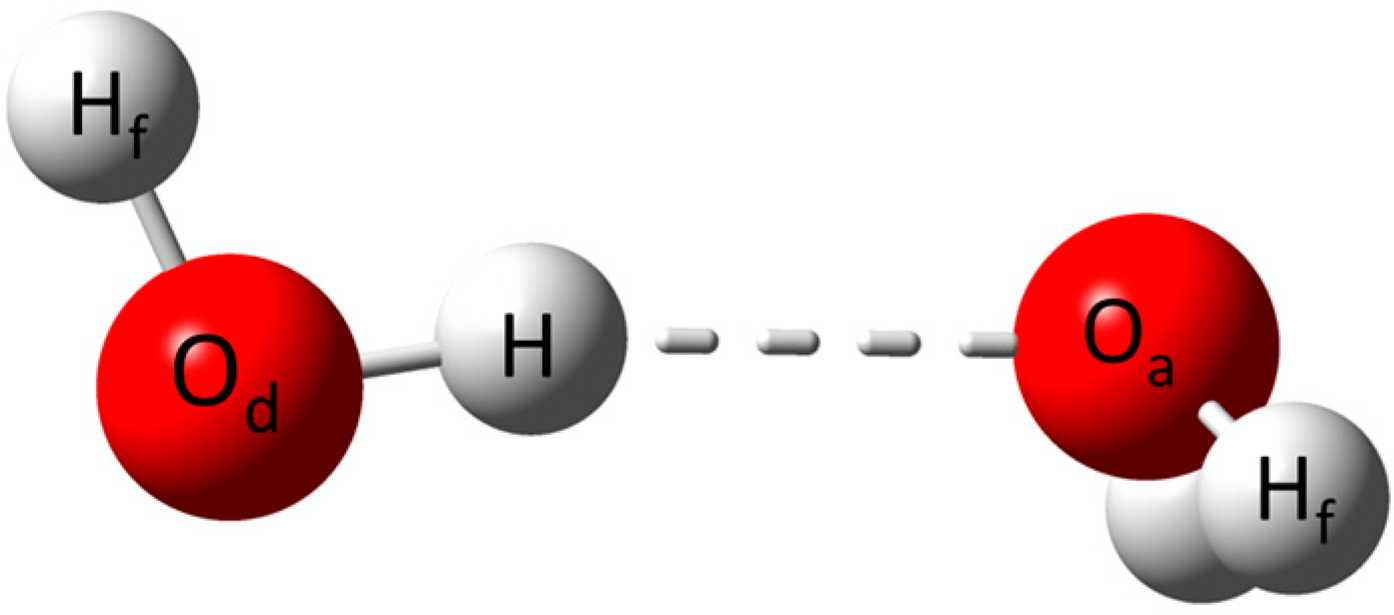

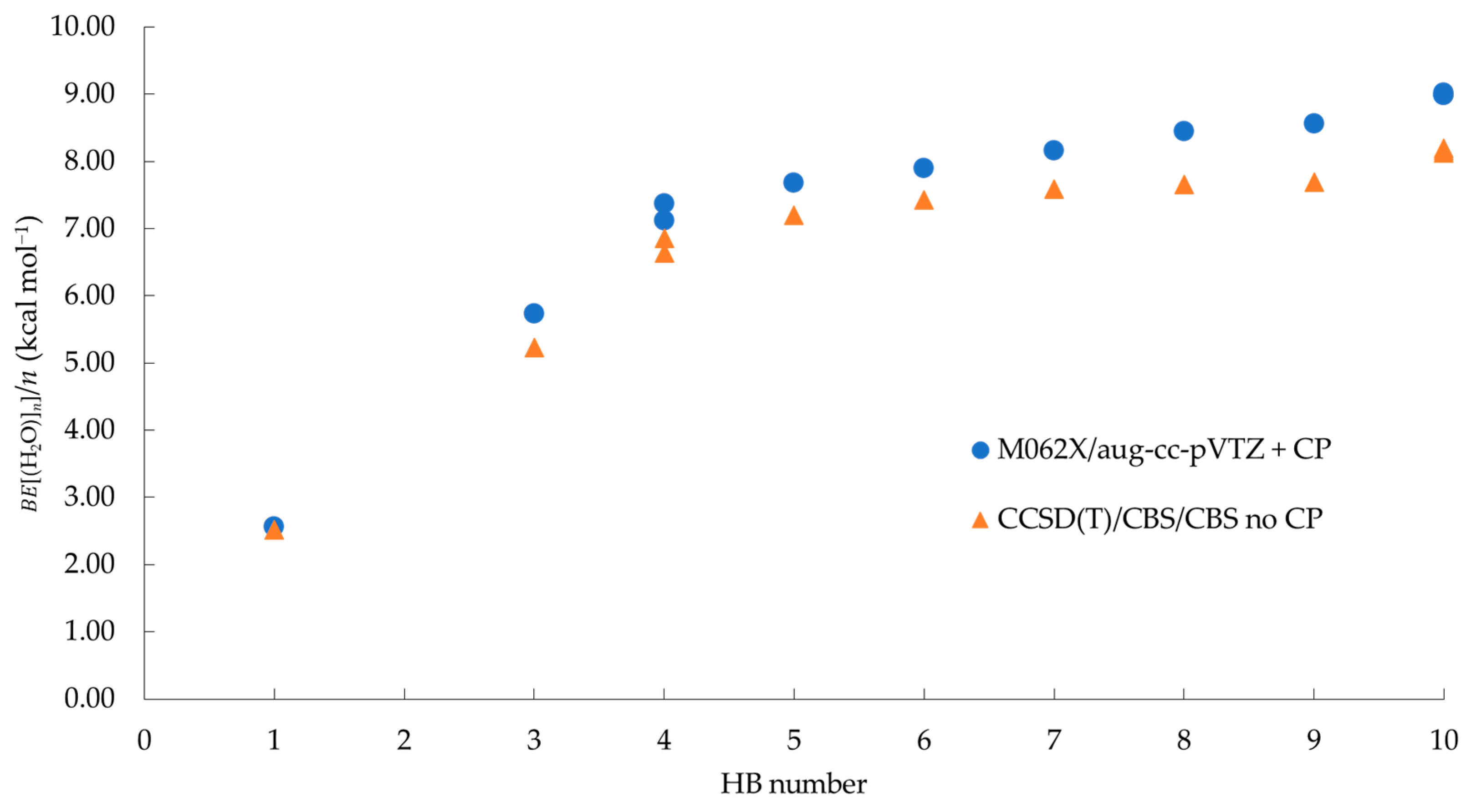




| Cluster (H2O)n | n | Number of HBs | M062X/aug-cc-pVTZ + CP (kcal mol−1) | CCSD(T)/CBS/CBS No CP 1 (kcal mol−1) |
|---|---|---|---|---|
| 2a | 2 | 1 | 2.57 | 2.52 |
| 3a | 3 | 3 | 5.74 | 5.23 |
| 4a | 4 | 4 | 7.12 | 6.65 |
| 4b | 4 | 4 | 7.38 | 6.86 |
| 5a | 5 | 5 | 7.68 | 7.20 |
| 6a | 6 | 6 | 7.90 | 7.43 |
| 6b | 6 | 7 | 8.16 | 7.59 |
| 6c | 6 | 8 | 8.45 | 7.66 |
| 6d | 6 | 9 | 8.56 | 7.69 |
| 7a | 7 | 10 | 9.02 | 8.20 |
| 7b | 7 | 10 | 8.99 | 8.16 |
| 7c | 7 | 10 | 8.99 | 8.13 |
| Cluster 1 (H2O)n | <O⋯O> (Å) | <O⋯H> (Å) | <S%> (H) | <S%> (Od) | <S%> (Oa) | <S%> (H,Od) | <S%> (H,Oa) | S% (Od, H,Oa) | |
|---|---|---|---|---|---|---|---|---|---|
| 2a | 2.904 | 1.947 | 0.966 | −37.96 | 76.10 | 27.35 | 38.14 | −10.71 | 65.49 |
| 3a | 2.783 | 1.892 | 0.982 | −30.63 | 67.30 | 28.93 | 36.67 | −1.70 | 65.60 |
| 4a | 2.737 | 1.774 | 1.154 | −16.79 | 55.49 | 35.82 | 38.70 | 19.03 | 74.52 |
| 4b | 2.728 | 1.764 | 1.169 | −15.55 | 54.41 | 36.18 | 38.86 | 20.63 | 75.04 |
| 5a | 2.717 | 1.737 | 1.206 | −13.15 | 52.47 | 37.66 | 39.32 | 24.51 | 76.98 |
| 6a | 2.709 | 1.734 | 1.216 | −12.37 | 51.88 | 38.24 | 39.51 | 25.87 | 77.75 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Seijas, L.E.; Zambrano, C.H.; Almeida, R.; Alí-Torres, J.; Rincón, L.; Torres, F.J. Exploring the Non-Covalent Bonding in Water Clusters. Int. J. Mol. Sci. 2023, 24, 5271. https://doi.org/10.3390/ijms24065271
Seijas LE, Zambrano CH, Almeida R, Alí-Torres J, Rincón L, Torres FJ. Exploring the Non-Covalent Bonding in Water Clusters. International Journal of Molecular Sciences. 2023; 24(6):5271. https://doi.org/10.3390/ijms24065271
Chicago/Turabian StyleSeijas, Luis E., Cesar H. Zambrano, Rafael Almeida, Jorge Alí-Torres, Luis Rincón, and Fernando Javier Torres. 2023. "Exploring the Non-Covalent Bonding in Water Clusters" International Journal of Molecular Sciences 24, no. 6: 5271. https://doi.org/10.3390/ijms24065271
APA StyleSeijas, L. E., Zambrano, C. H., Almeida, R., Alí-Torres, J., Rincón, L., & Torres, F. J. (2023). Exploring the Non-Covalent Bonding in Water Clusters. International Journal of Molecular Sciences, 24(6), 5271. https://doi.org/10.3390/ijms24065271







