The Effect of Sorbent Composition on Sorption Properties of Materials Based on Ti-Ca-Mg Phosphates
Abstract
:1. Introduction
2. Results and Discussion
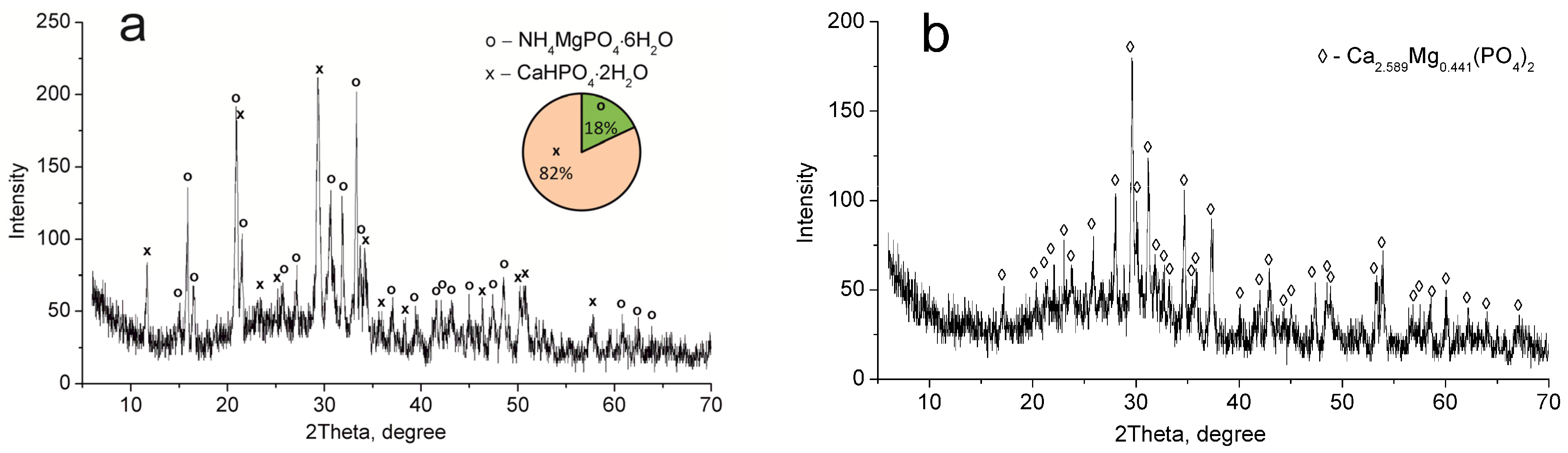
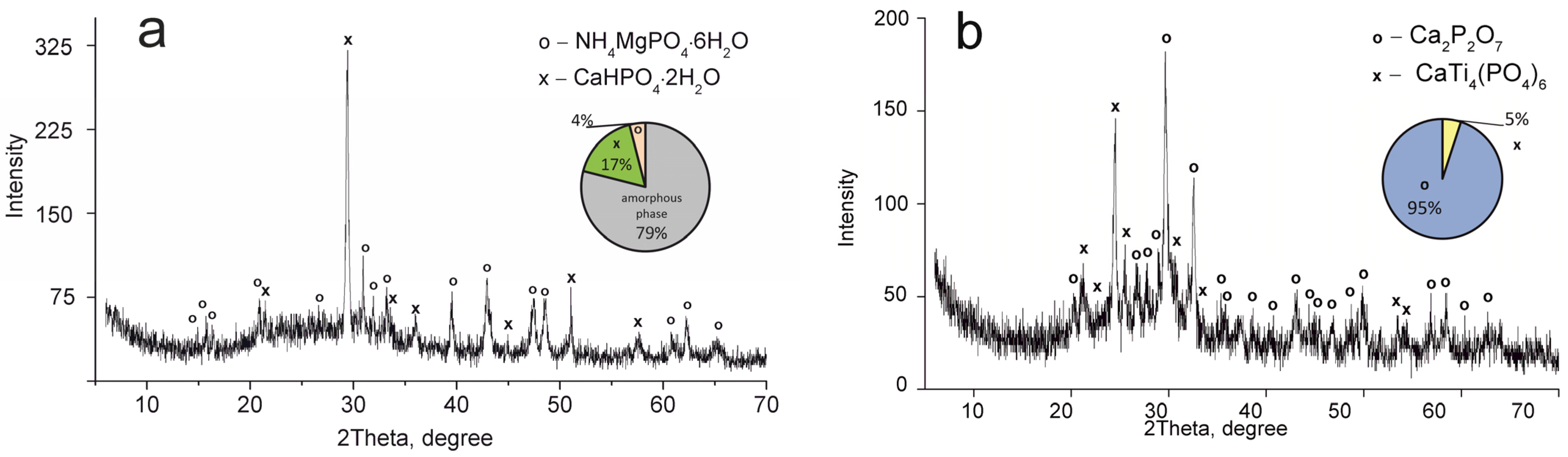
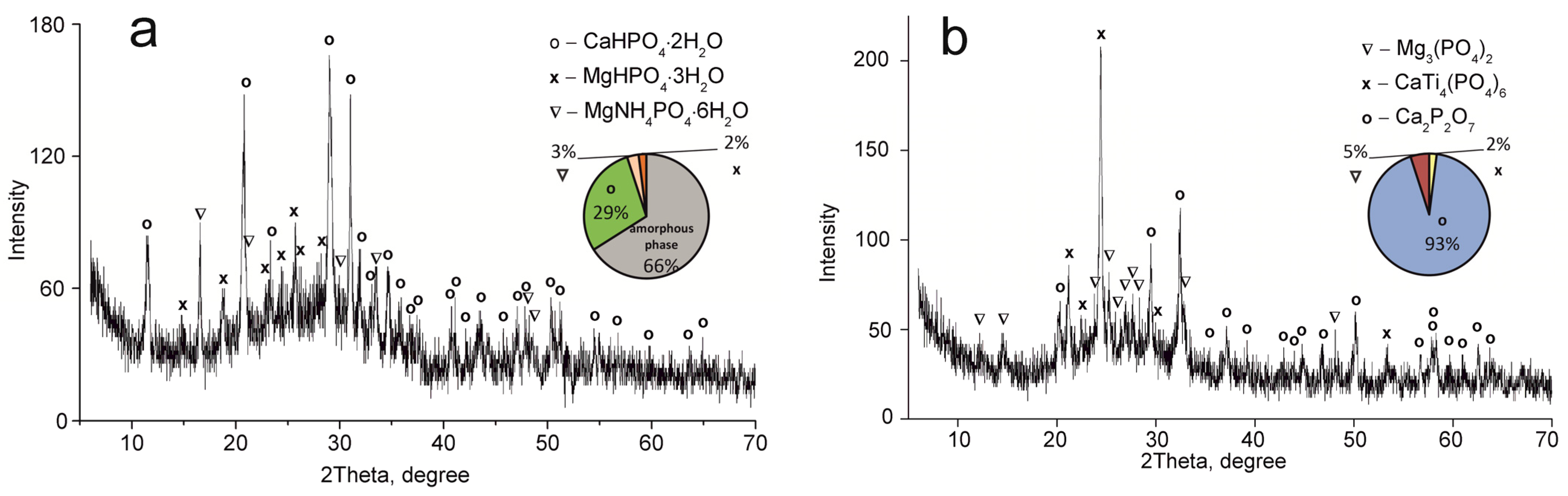
2.1. Characterization
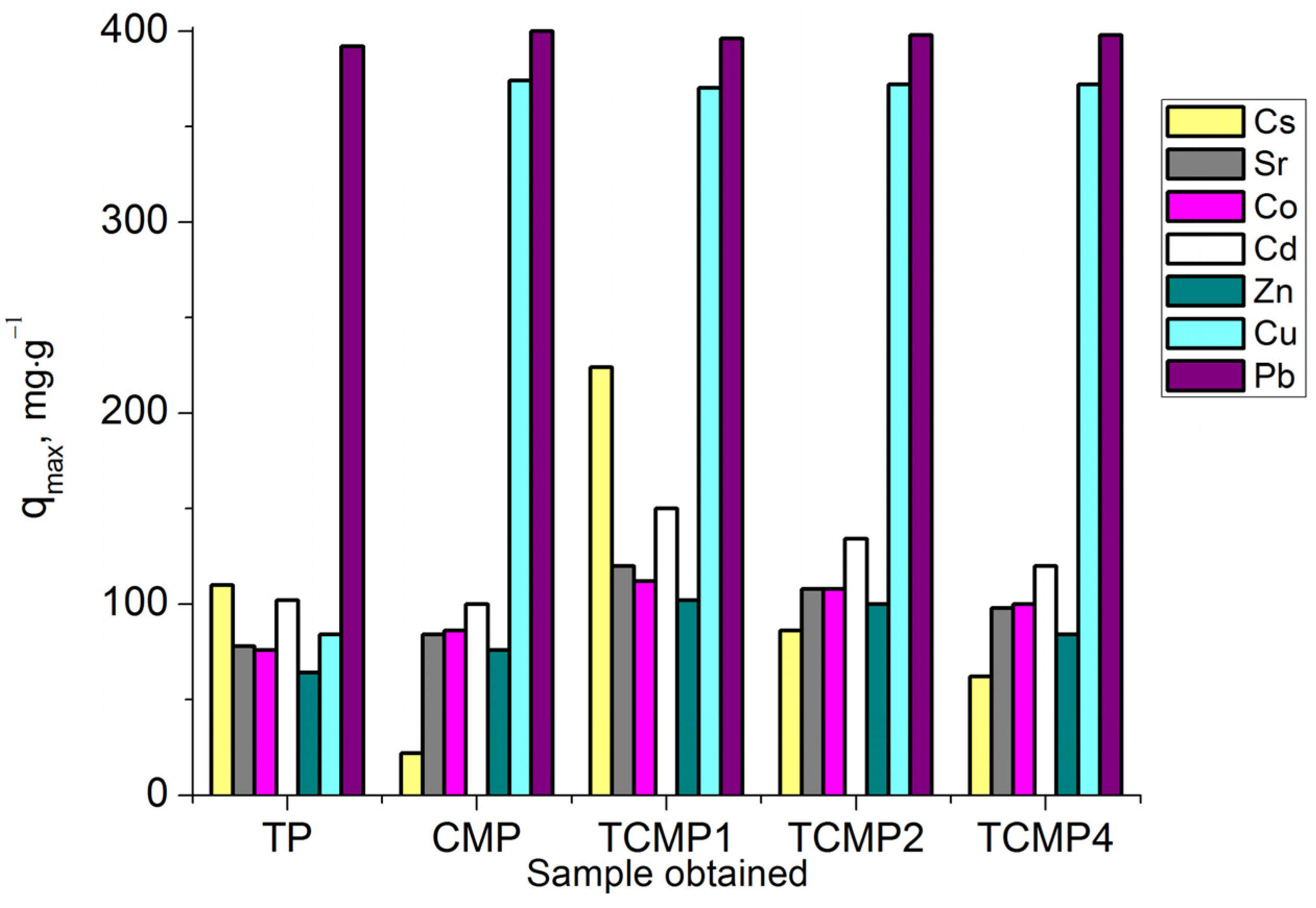
2.2. Sorption Study
2.2.1. Sorption on TP
2.2.2. Sorption on CMP
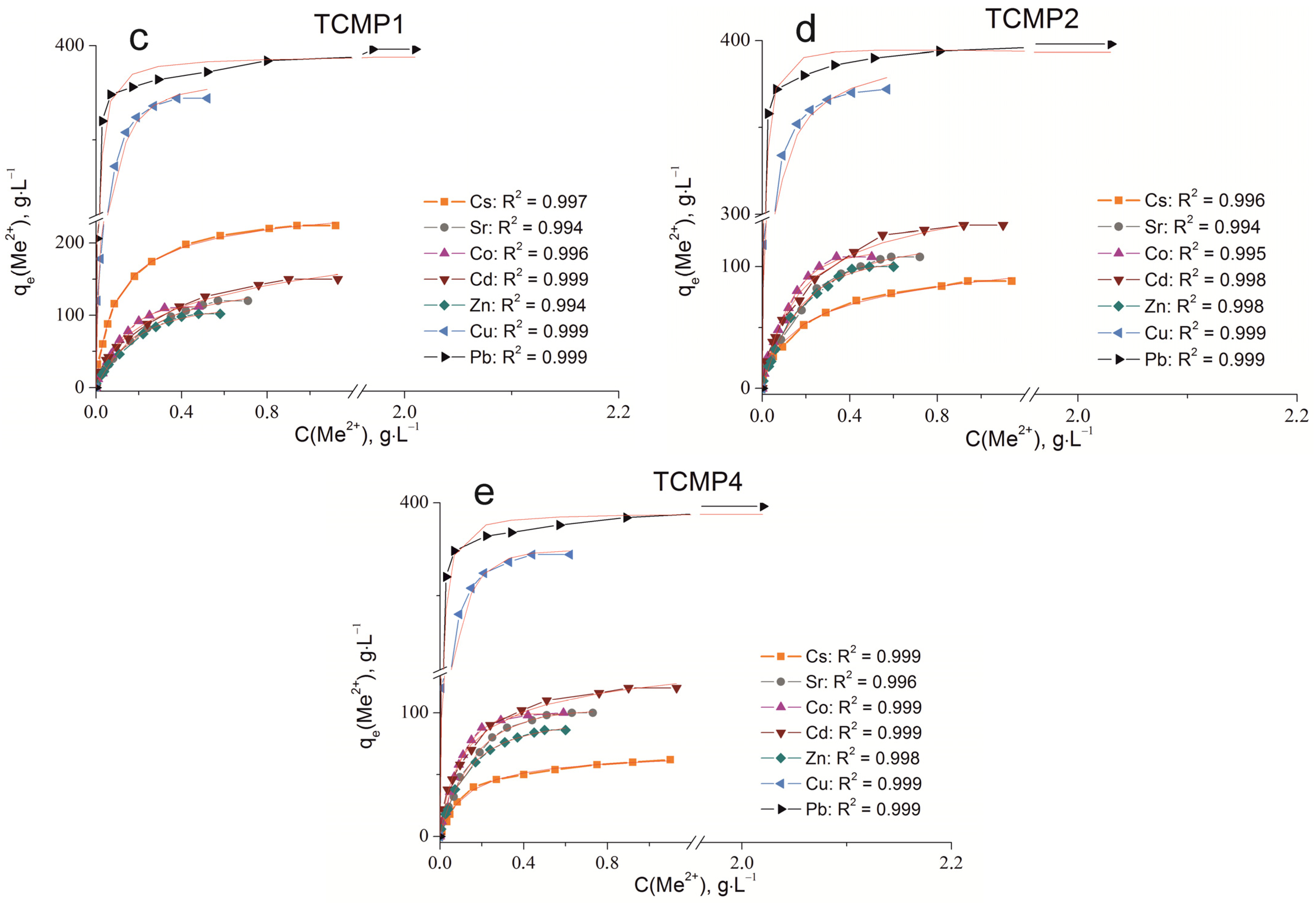
2.2.3. Sorption on TCMPs
2.2.4. Sorption Test
3. Materials and Methods
3.1. Starting Materials
3.2. TP, TCMP1-4, and CMP Synthesis
3.3. Characterization Techniques
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A

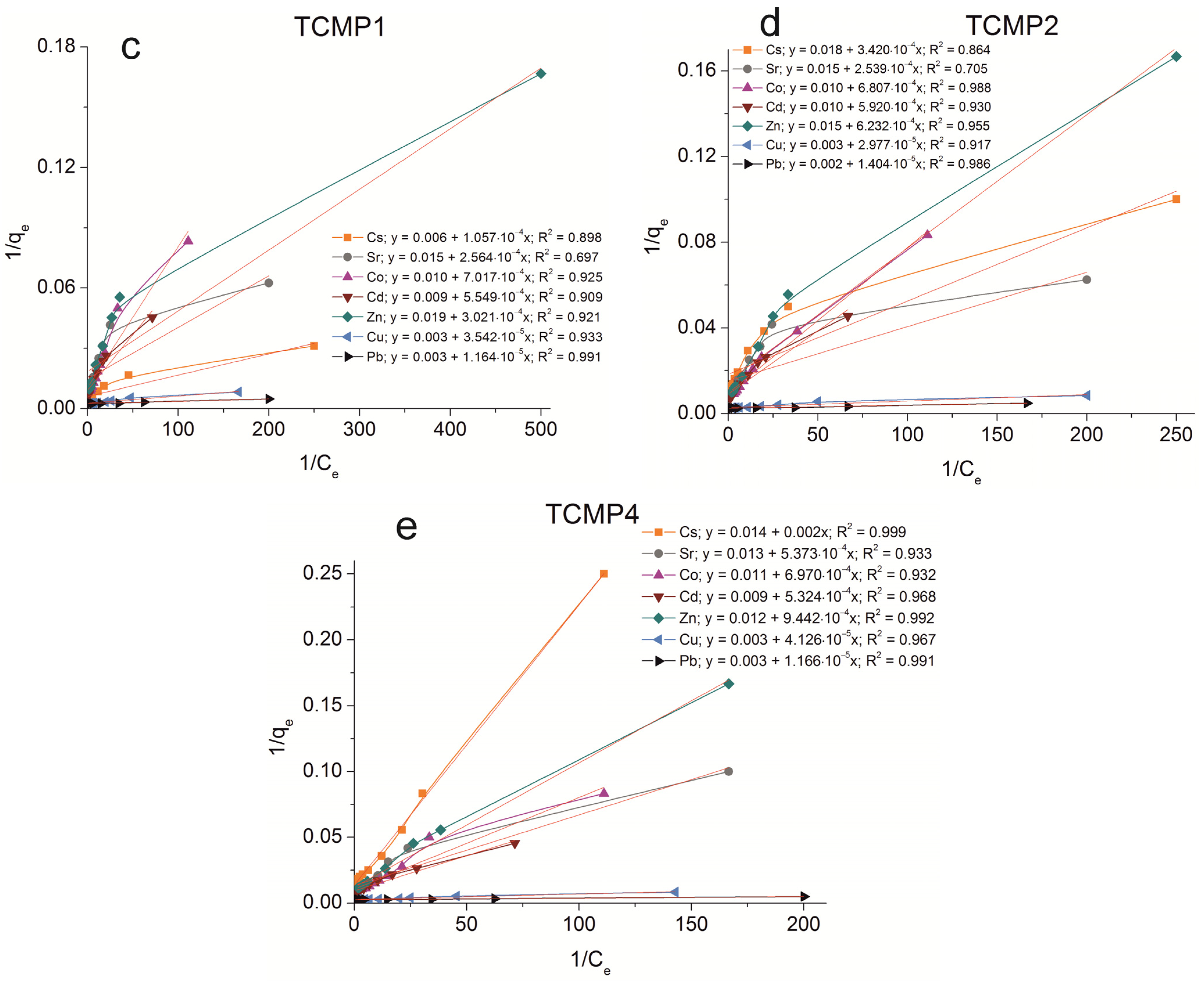
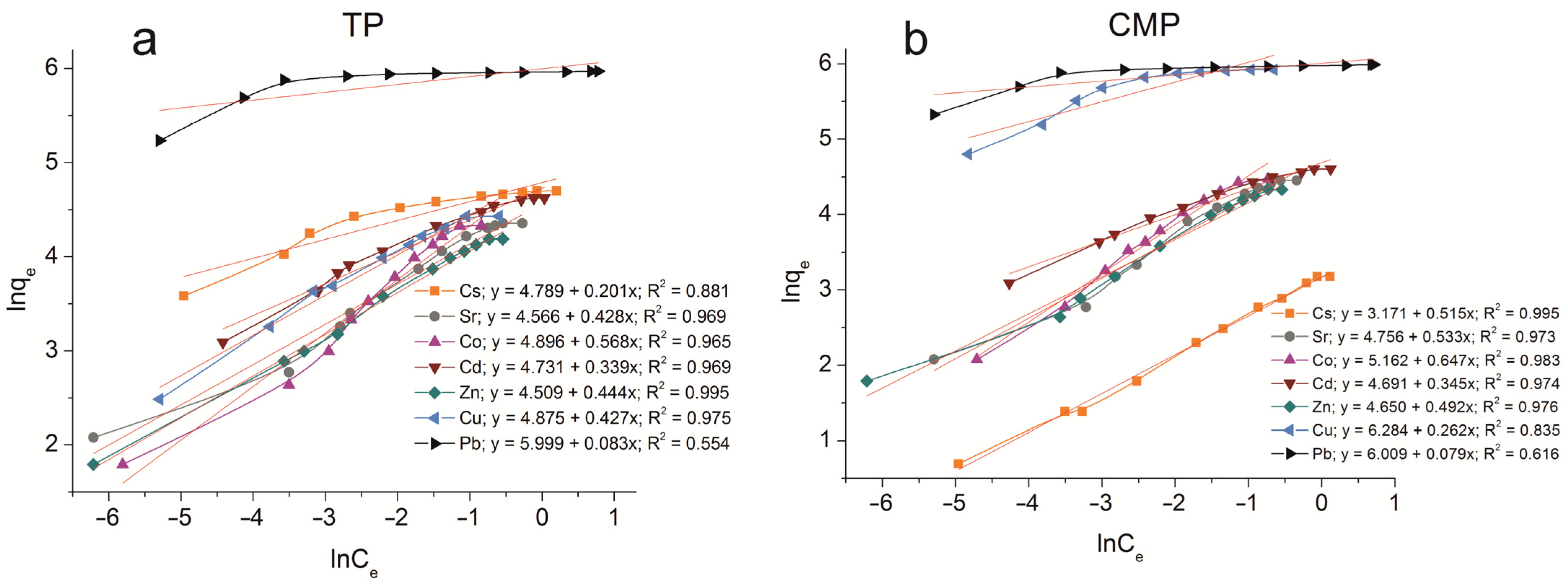
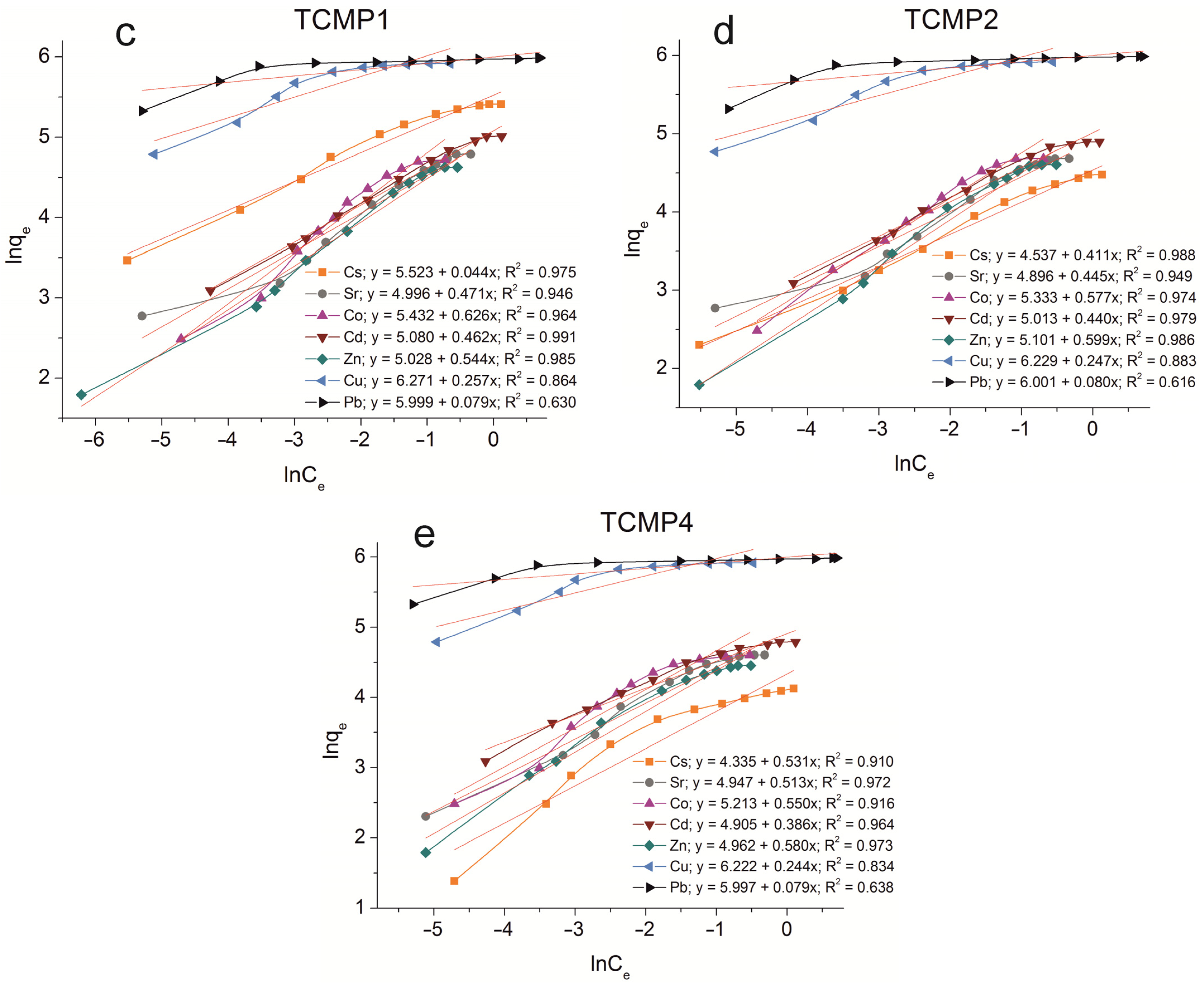
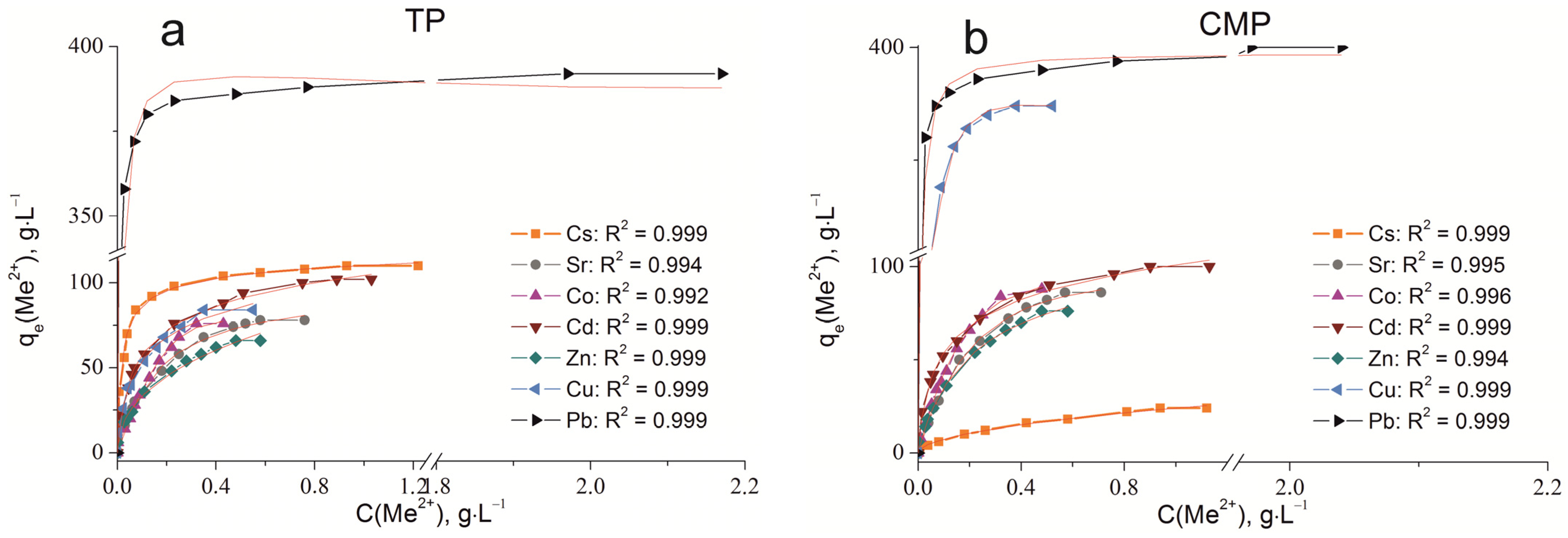
| Cation | KL | qe,calc *, mg·g−1 | qe,exp *, mg·g−1 | R2 |
|---|---|---|---|---|
| TP | ||||
| Cs | 75.30 | 100 | 110 | 0.984 |
| Sr | 104.02 | 46 | 78 | 0.976 |
| Co | 52.08 | 44 | 76 | 0.979 |
| Cd | 27.56 | 84 | 102 | 0.979 |
| Zn | 82.87 | 42 | 66 | 0.983 |
| Cu | 42.38 | 67 | 84 | 0.983 |
| Pb | 217.08 | 334 | 392 | 0.915 |
| CMP | ||||
| Cs | 26.00 | 13 | 24 | 0.975 |
| Sr | 34.38 | 53 | 86 | 0.973 |
| Co | 13.00 | 77 | 88 | 0.982 |
| Cd | 23.78 | 84 | 100 | 0.985 |
| Zn | 86.09 | 40 | 76 | 0.974 |
| Cu | 63.88 | 334 | 374 | 0.928 |
| Pb | 255.10 | 334 | 400 | 0.916 |
| TCMP1 | ||||
| Cs | 56.76 | 167 | 224 | 0.989 |
| Sr | 58.50 | 67 | 120 | 0.974 |
| Co | 14.25 | 100 | 112 | 0.976 |
| Cd | 16.22 | 112 | 150 | 0.982 |
| Zn | 62.89 | 53 | 102 | 0.969 |
| Cu | 84.70 | 334 | 372 | 0.954 |
| Pb | 257.73 | 334 | 398 | 0.916 |
| TCMP2 | ||||
| Cs | 52.63 | 56 | 88 | 0.979 |
| Sr | 59.08 | 67 | 108 | 0.978 |
| Co | 14.69 | 100 | 108 | 0.972 |
| Cd | 16.89 | 100 | 134 | 0.966 |
| Zn | 24.07 | 87 | 100 | 0.999 |
| Cu | 100.77 | 334 | 372 | 0.971 |
| Pb | 142.45 | 500 | 398 | 0.919 |
| TCMP4 | ||||
| Cs | 7.00 | 71 | 62 | 0.991 |
| Sr | 24.20 | 77 | 100 | 0.975 |
| Co | 15.78 | 91 | 100 | 0.961 |
| Cd | 16.90 | 112 | 120 | 0.997 |
| Zn | 12.71 | 83 | 86 | 0.962 |
| Cu | 72.71 | 334 | 372 | 0.938 |
| Pb | 257.29 | 334 | 398 | 0.916 |
| Cation | KF | n | R2 |
|---|---|---|---|
| TP | |||
| Cs | 120.18 | 4.98 | 0.999 |
| Sr | 96.16 | 2.34 | 0.998 |
| Co | 133.75 | 1.76 | 0.997 |
| Cd | 113.41 | 2.95 | 0.999 |
| Zn | 90.83 | 2.25 | 0.999 |
| Cu | 130.97 | 2.34 | 0.999 |
| Pb | 403.02 | 12.05 | 0.999 |
| CMP | |||
| Cs | 23.83 | 1.94 | 0.999 |
| Sr | 116.28 | 1.88 | 0.937 |
| Co | 174.51 | 1.55 | 0.999 |
| Cd | 108.96 | 2.90 | 0.999 |
| Zn | 104.58 | 2.03 | 0.995 |
| Cu | 535.93 | 3.82 | 0.999 |
| Pb | 407.07 | 12.66 | 0.999 |
| TCMP1 | |||
| Cs | 250.38 | 22.73 | 0.936 |
| Sr | 147.82 | 2.12 | 0.996 |
| Co | 228.61 | 1.60 | 0.999 |
| Cd | 160.77 | 2.16 | 0.999 |
| Zn | 152.63 | 1.84 | 0.999 |
| Cu | 529.00 | 3.89 | 0.999 |
| Pb | 403.02 | 12.66 | 0.999 |
| TCMP2 | |||
| Cs | 93.41 | 2.43 | 0.999 |
| Sr | 113.75 | 2.25 | 0.943 |
| Co | 207.06 | 1.73 | 0.999 |
| Cd | 150.35 | 2.27 | 0.999 |
| Zn | 164.19 | 1.67 | 0.999 |
| Cu | 507.25 | 4.05 | 0.999 |
| Pb | 403.83 | 12.50 | 0.999 |
| TCMP4 | |||
| Cs | 76.32 | 1.88 | 0.999 |
| Sr | 140.75 | 1.95 | 0.999 |
| Co | 183.64 | 1.82 | 0.999 |
| Cd | 134.96 | 2.59 | 0.999 |
| Zn | 142.88 | 1.72 | 0.999 |
| Cu | 503.71 | 4.10 | 0.999 |
| Pb | 402.22 | 12.66 | 0.999 |
| Cation | KR | aR | β | R2 |
|---|---|---|---|---|
| TP | ||||
| Cs | 7050 | 62.95 | 0.944 | 0.999 |
| Sr | 584 | 5.92 | 1.003 | 0.994 |
| Co | 407 | 5.24 | 1.683 | 0.992 |
| Cd | 2163 | 19.74 | 0.856 | 0.999 |
| Zn | 4438 | 49.47 | 0.604 | 0.999 |
| Cu | 2162 | 20.70 | 0.838 | 0.999 |
| Pb | 71,106 | 181.26 | 1.011 | 0.999 |
| CMP | ||||
| Cs | 2667 | 110.66 | 0.498 | 0.999 |
| Sr | 440 | 3.84 | 1.177 | 0.995 |
| Co | 526 | 4.81 | 1.316 | 0.996 |
| Cd | 1984 | 18.64 | 0.842 | 0.999 |
| Zn | 583 | 5.49 | 0.919 | 0.994 |
| Cu | 15,912 | 42.17 | 1.058 | 0.999 |
| Pb | 85,160 | 214.04 | 1.001 | 0.999 |
| TCMP1 | ||||
| Cs | 2868 | 11.75 | 0.950 | 0.997 |
| Sr | 650 | 3.90 | 1.040 | 0.994 |
| Co | 730 | 6.17 | 1.464 | 0.996 |
| Cd | 1260 | 7.34 | 0.804 | 0.999 |
| Zn | 606 | 4.43 | 1.158 | 0.994 |
| Cu | 18,301 | 47.33 | 1.022 | 0.999 |
| Pb | 86,965 | 220.29 | 1.000 | 0.999 |
| TCMP2 | ||||
| Cs | 890 | 9.24 | 0.851 | 0.996 |
| Sr | 649 | 4.60 | 1.086 | 0.994 |
| Co | 787 | 6.46 | 1.328 | 0.995 |
| Cd | 1040 | 6.64 | 0.941 | 0.998 |
| Zn | 630 | 4.93 | 1.182 | 0.998 |
| Cu | 21,987 | 55.83 | 0.985 | 0.999 |
| Pb | 72,780 | 183.56 | 1.008 | 0.999 |
| TCMP4 | ||||
| Cs | 548 | 8.07 | 0.989 | 0.999 |
| Sr | 624 | 5.15 | 1.212 | 0.996 |
| Co | 873 | 8.44 | 1.317 | 0.999 |
| Cd | 1528 | 11.60 | 0.907 | 0.999 |
| Zn | 747 | 7.09 | 1.060 | 0.998 |
| Cu | 17,478 | 45.81 | 1.032 | 0.999 |
| Pb | 85,639 | 216.77 | 1.002 | 0.999 |
References
- Hemachandra, C.K.; Pathiratne, A. Assessing Toxicity of Copper, Cadmium and Chromium Levels Relevant to Discharge Limits of Industrial Effluents into Inland Surface Waters Using Common Onion, Allium cepa Bioassay. Bull. Environ. Contam. Toxicol. 2015, 94, 199–203. [Google Scholar] [CrossRef] [PubMed]
- Hussain, A.; Aslam, S.; Javid, A.; Rashid, M.; Hussain, I.; Qazi, J.I. Biphasic Treatment System for the Removal of Toxic and Hazardous Pollutants from Industrial Wastewaters. In Emerging and Eco-Friendly Approaches for Waste Management; Springer: Singapore, 2019; pp. 397–421. [Google Scholar]
- Chandra, R. (Ed.) Advances in Biodegradation and Bioremediation of Industrial Waste; CRC Press: Boca Raton, FL, USA, 2015; ISBN 9780429089251. [Google Scholar]
- Abdelrahman, E.A.; Abou El-Reash, Y.G.; Youssef, H.M.; Kotp, Y.H.; Hegazey, R.M. Utilization of rice husk and waste aluminum cans for the synthesis of some nanosized zeolite, zeolite/zeolite, and geopolymer/zeolite products for the efficient removal of Co(II), Cu(II), and Zn(II) ions from aqueous media. J. Hazard. Mater. 2021, 401, 123813. [Google Scholar] [CrossRef] [PubMed]
- Sekine, Y.; Motokawa, R.; Kozai, N.; Ohnuki, T.; Matsumura, D.; Tsuji, T.; Kawasaki, R.; Akiyoshi, K. Calcium-deficient Hydroxyapatite as a Potential Sorbent for Strontium. Sci. Rep. 2017, 7, 2064. [Google Scholar] [CrossRef] [PubMed]
- Van den Berg, H.H.J.L.; Friederichs, L.; Versteegh, J.F.M.; Smeets, P.W.M.H.; de Roda Husman, A.M. How current risk assessment and risk management methods for drinking water in The Netherlands cover the WHO water safety plan approach. Int. J. Hyg. Environ. Health 2019, 222, 1030–1037. [Google Scholar] [CrossRef] [PubMed]
- Lin, X.; Burns, R.C.; Lawrance, G.A. Heavy Metals in Wastewater: The Effect of Electrolyte Composition on the Precipitation of Cadmium(II) Using Lime and Magnesia. Water Air Soil Pollut. 2005, 165, 131–152. [Google Scholar] [CrossRef]
- Meunier, N.; Drogui, P.; Montané, C.; Hausler, R.; Blais, J.-F.; Mercier, G. Heavy Metals Removal from Acidic and Saline Soil Leachate Using Either Electrochemical Coagulation or Chemical Precipitation. J. Environ. Eng. 2006, 132, 545–554. [Google Scholar] [CrossRef]
- Melville, J.B.; Matijevic, E. Removal of Copper, Lead, and Cadmium Ions by Microflotation. J. Colloid Interface Sci. 1976, 57, 94–103. [Google Scholar] [CrossRef]
- Ipek, U. Removal of Ni(II) and Zn(II) from an Aqueous Solutionby Reverse Osmosis. Desalination 2005, 174, 161–169. [Google Scholar] [CrossRef]
- Wood, D.J.; Law, J.D.; Tullock, P.A. Extraction of Lead and Strontium from Hazardous Waste Streams by Solvent Extraction with 4′,4′,(5′)-di-(t-butyldicyclohexo)-18-crown-6. Solvent Extr. Ion Exch. 1997, 15, 65–78. [Google Scholar] [CrossRef]
- Parida, K.M.; Sahu, B.B.; Das, D.P. A Comparative Study on Textural Characterization: Cation-Exchange and Sorption Properties of Crystalline α-Zirconium(IV), Tin(IV), and Titanium(IV) phosphates. J. Colloid Interface Sci. 2004, 270, 436–445. [Google Scholar] [CrossRef]
- Castaldi, P.; Santona, L.; Enzo, S.; Melis, P. Sorption Processes and XRD Analysis of a Natural Zeolite Exchanged with Pb2+, Cd2+ and Zn2+ Cations. J. Hazard. Mater. 2008, 156, 428–434. [Google Scholar] [CrossRef] [PubMed]
- Sulaymon, A.H.; Faisal, A.A.H.; Khaliefa, Q.M. Simultaneous adsorption–Precipitation Characterization as Mechanisms for Metals Removal from Aqueous Solutions by Cement Kiln Dust (CKD). Desalin. Water Treat. 2016, 57, 819–826. [Google Scholar] [CrossRef]
- Pang, F.M.; Teng, S.P.; Teng, T.T.; Omar, A.K.M. Heavy Metals Removal by Hydroxide Precipitation and Coagulation-Flocculation Methods from Aqueous Solutions. Water Qual. Res. J. 2009, 44, 174–182. [Google Scholar] [CrossRef]
- Renou, S.; Poulain, S.; Givaudan, J.G.; Moulin, P. Treatment Process Adapted to Stabilized Leachates: Lime Precipitation–Prefiltration–Reverse Osmosis. J. Membr. Sci. 2008, 313, 9–22. [Google Scholar] [CrossRef]
- Vipin, A.K.; Ling, S.; Fugetsu, B. Sodium Cobalt Hexacyanoferrate Encapsulated in Alginate Vesicle with CNT for Both Cesium and Strontium Removal. Carbohydr. Polym. 2014, 111, 477–484. [Google Scholar] [CrossRef]
- Amer, M.W.; Awwad, A.M. Removal of Zn(II), Cd(II) and Cu(II) Ions from Aqueous Solution by Nano-Structured Kaolinite. Asian J. Chem. 2017, 29, 965–969. [Google Scholar] [CrossRef]
- Isinkaralar, K. High-Efficiency Removal of Benzene Vapor Using Activated Carbon from Althaea officinalis L. Biomass as a Lignocellulosic Precursor. Environ. Sci. Pollut. Res. 2022, 29, 66728–66740. [Google Scholar] [CrossRef]
- Isinkaralar, K. Theoretical Removal Study of Gas BTEX onto Activated Carbon Produced from Digitalis purpurea L. Biomass. Biomass Convers. Biorefinery 2022, 12, 4171–4181. [Google Scholar] [CrossRef]
- Li, Z.; Nagashima, M.; Ikeda, K. Treatment Technology of Hazardous Water Contaminated with Radioisotopes with Paper Sludge Ash-Based Geopolymer—Stabilization of Immobilization of Strontium and Cesium by Mixing Seawater. Materials 2018, 11, 1521. [Google Scholar] [CrossRef]
- Simsek, E.B.; Duranoglu, D.; Beker, U. Heavy Metal Adsorption by Magnetic Hybrid-Sorbent: An Experimental and Theoretical Approach. Sep. Sci. Technol. 2012, 47, 1334–1340. [Google Scholar] [CrossRef]
- Park, Y.; Lee, Y.-C.; Shin, W.S.; Choi, S.-J. Removal of Cobalt, Strontium and Cesium from Radioactive Laundry Wastewater by Ammonium Molybdophosphate–polyacrylonitrile (AMP–PAN). Chem. Eng. J. 2010, 162, 685–695. [Google Scholar] [CrossRef]
- Lou, Z.; Cao, Z.; Xu, J.; Zhou, X.; Zhu, J.; Liu, X.; Ali Baig, S.; Zhou, J.; Xu, X. Enhanced Removal of As(III)/(V) from Water by Simultaneously Supported and Stabilized Fe-Mn Binary Oxide Nanohybrids. Chem. Eng. J. 2017, 322, 710–721. [Google Scholar] [CrossRef]
- Mahmoud, A.; Hoadley, A.F.A. An Evaluation of a Hybrid Ion Exchange Electrodialysis Process in the Recovery of Heavy Metals from Simulated Dilute Industrial Wastewater. Water Res. 2012, 46, 3364–3376. [Google Scholar] [CrossRef] [PubMed]
- Lertlapwasin, R.; Bhawawet, N.; Imyim, A.; Fuangswasdi, S. Ionic Liquid Extraction of Heavy Metal Ions by 2-aminothiophenol in 1-butyl-3-methylimidazolium Hexafluorophosphate and Their Association Constants. Sep. Purif. Technol. 2010, 72, 70–76. [Google Scholar] [CrossRef]
- Samiey, B.; Cheng, C.-H.; Wu, J. Organic-Inorganic Hybrid Polymers as Adsorbents for Removal of Heavy Metal Ions from Solutions: A Review. Materials 2014, 7, 673–726. [Google Scholar] [CrossRef]
- Fernane, F.; Mecherri, M.O.; Sharrock, P.; Hadioui, M.; Lounici, H.; Fedoroff, M. Sorption of Cadmium and Copper Ions on Natural and Synthetic Hydroxylapatite Particles. Mater. Charact. 2008, 59, 554–559. [Google Scholar] [CrossRef]
- Núñez, D.; Serrano, J.A.; Mancisidor, A.; Elgueta, E.; Varaprasad, K.; Oyarzún, P.; Cáceres, R.; Ide, W.; Rivas, B.L. Heavy Metal Removal from Aqueous Systems Using Hydroxyapatite Nanocrystals Derived from Clam Shells. RSC Adv. 2019, 9, 22883–22890. [Google Scholar] [CrossRef]
- Ivanets, A.I.; Kitikova, N.V.; Shashkova, I.L.; Oleksiienko, O.V.; Levchuk, I.; Sillanpää, M. Using of Phosphatized Dolomite for Treatment of Real Mine Water from Metal Ions. J. Water Process Eng. 2016, 9, 246–253. [Google Scholar] [CrossRef]
- Zou, X.; Zhao, Y.; Zhang, Z. Preparation of Hydroxyapatite Nanostructures with Different Morphologies and Adsorption Behavior on Seven Heavy Metals Ions. J. Contam. Hydrol. 2019, 226, 103538. [Google Scholar] [CrossRef]
- Elkady, M.F.; Mahmoud, M.M.; Abd-El-Rahman, H.M. Kinetic approach for cadmium sorption using microwave synthesized nano-hydroxyapatite. J. Non-Cryst. Solids 2011, 357, 1118–1129. [Google Scholar] [CrossRef]
- Maslova, M.; Mudruk, N.; Ivanets, A.; Shashkova, I.; Kitikova, N. A Novel Sorbent Based on Ti-Ca-Mg Phosphates: Synthesis, Characterization, and Sorption Properties. Environ. Sci. Pollut. Res. 2020, 27, 3933–3949. [Google Scholar] [CrossRef] [PubMed]
- Gerasimova, L.G.; Maslova, M.V.; Shchukina, E.S. The Technology of Sphene Concentrate Treatment to Obtain Titanium Salts. Theor. Found. Chem. Eng. 2009, 43, 464–467. [Google Scholar] [CrossRef]
- Maslova, M.; Mudruk, N.; Ivanets, A.; Shashkova, I.; Kitikova, N. The Effect of pH on Removal of Toxic Metal Ions from Aqueous Solutions Using Composite Sorbent Based on Ti-Ca-Mg Phosphates. J. Water Process Eng. 2021, 40, 101830. [Google Scholar] [CrossRef]
- Ivanets, A.; Kitikova, N.; Shashkova, I.; Radkevich, A.; Stepanchuk, T.; Maslova, M.; Mudruk, N. One-Stage Adsorption Treatment of Liquid Radioactive Wastes with Complex Radionuclide Composition. Water Air Soil Pollut. 2020, 231, 144. [Google Scholar] [CrossRef]
- Ivanets, A.I.; Srivastava, V.; Kitikova, N.V.; Shashkova, I.L.; Sillanpää, M. Non-Apatite Ca-Mg Phosphate Sorbent for Removal of Toxic Metal Ions from Aqueous Solutions. J. Environ. Chem. Eng. 2017, 5, 2010–2017. [Google Scholar] [CrossRef]

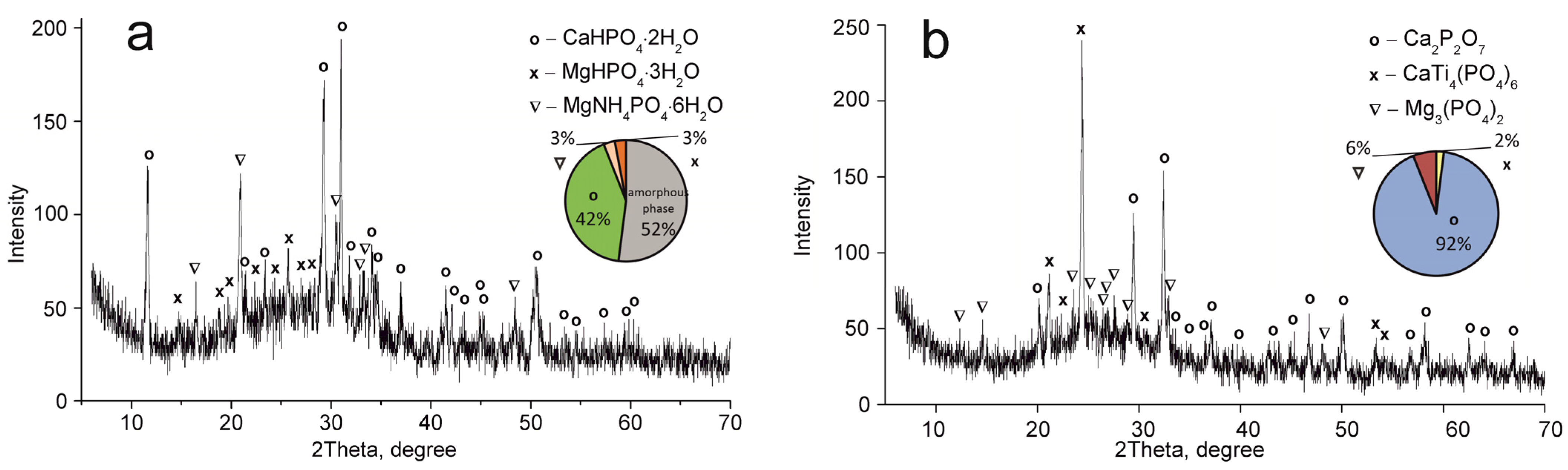
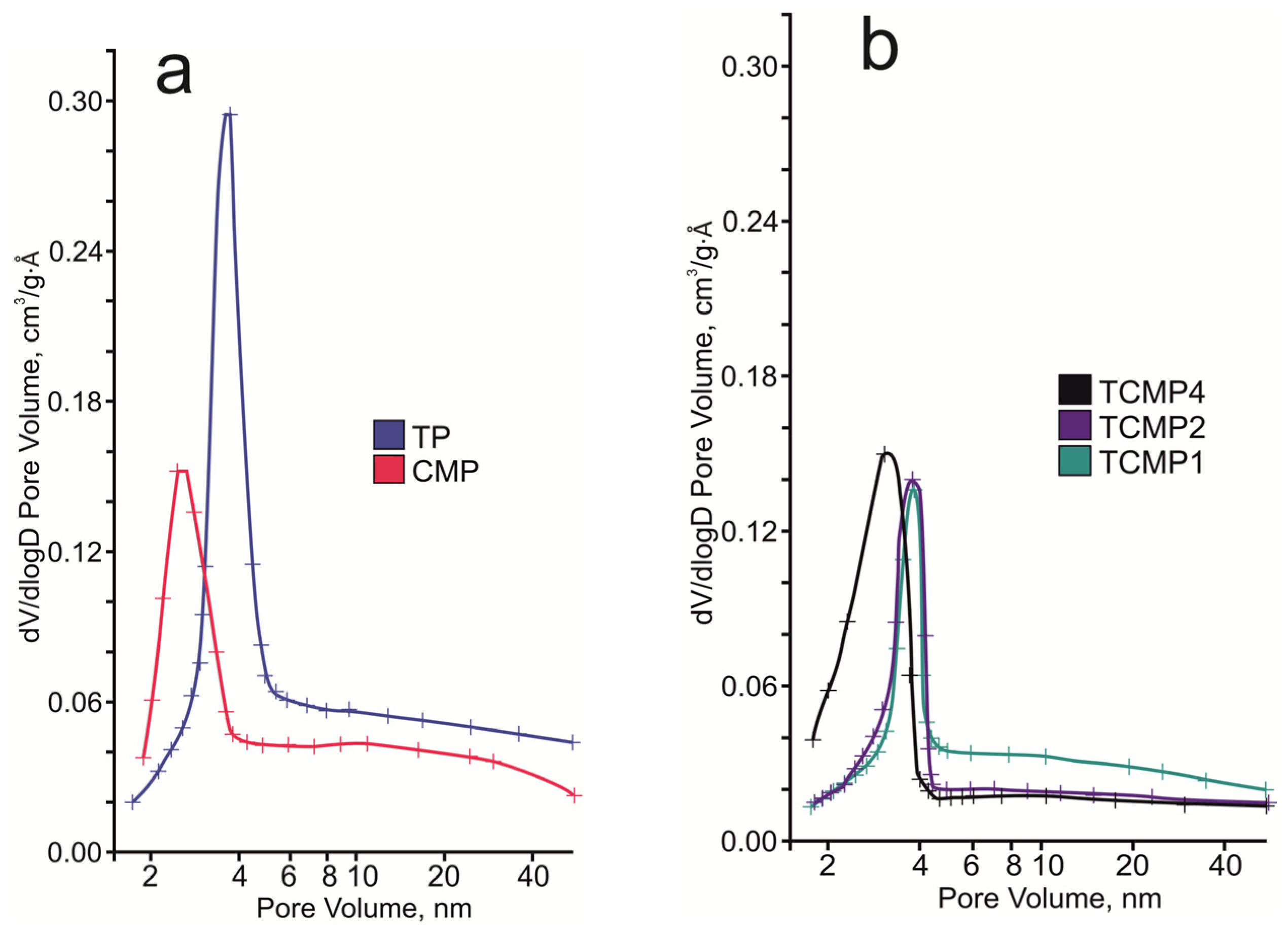
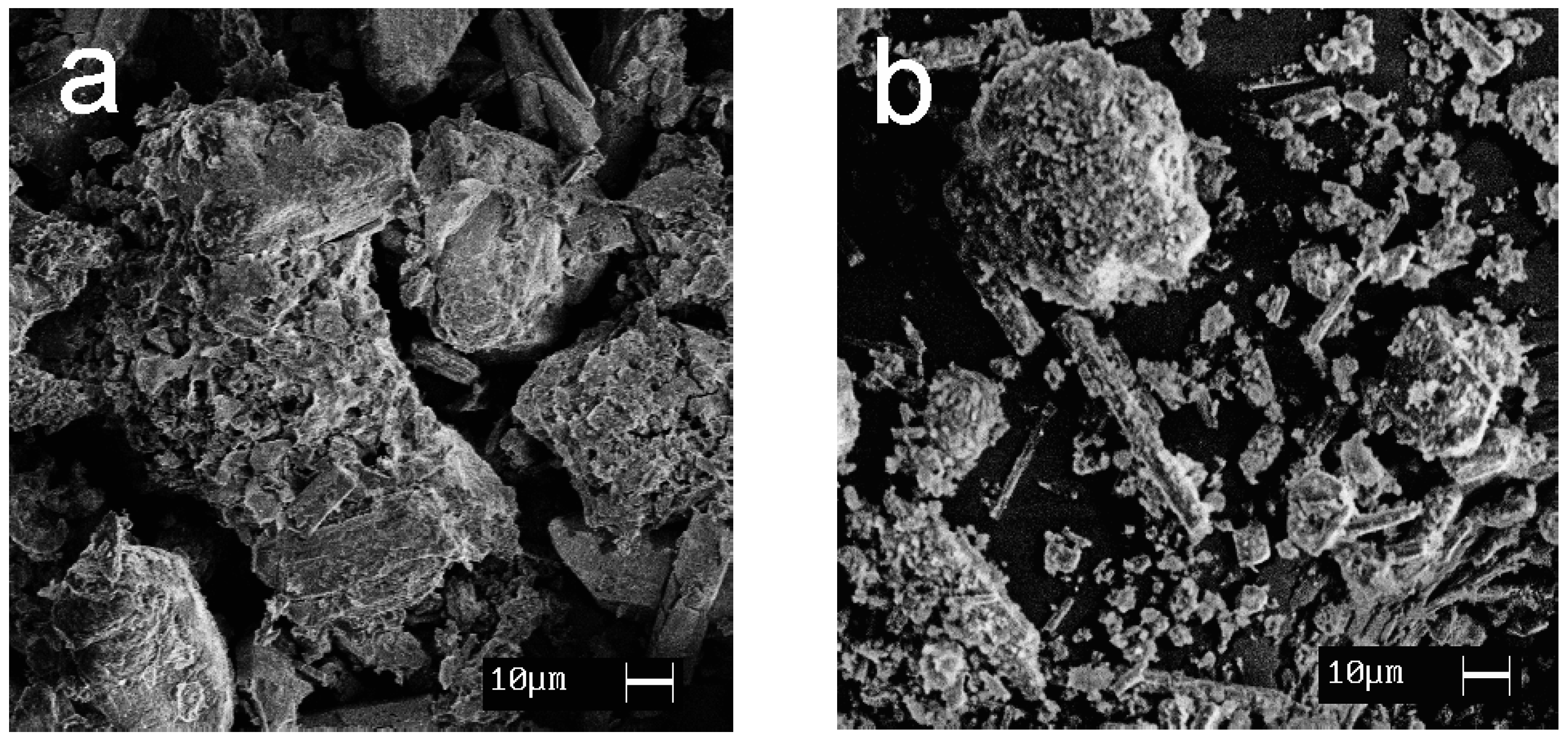

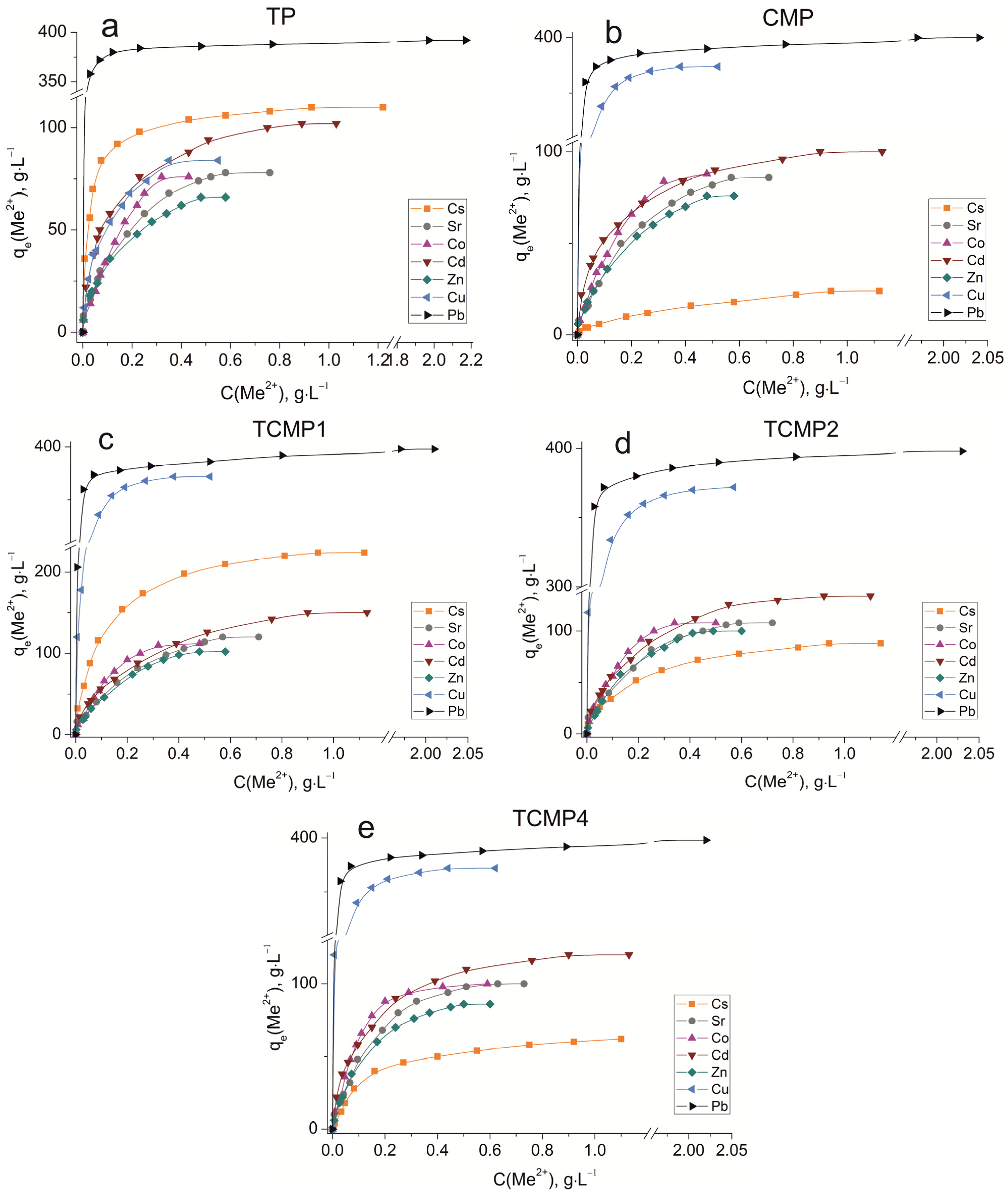
| Sample | Component, mas. % | Degree of Conversion, % | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Ti | P | NH4+ | S | Ca | Mg | Ti | Ca | Mg | |
| TP | 21.90 | 14.82 | 1.98 | 0.02 | – | – | 99.9 | – | – |
| TCMP1 | 11.00 | 15.33 | 0.12 | 0.14 | 7.12 | 1.76 | 99.8 | 98.3 | 96.2 |
| TCMP2 | 7.42 | 15.45 | 0.21 | 0.35 | 9.73 | 2.38 | 99.8 | 98.4 | 96.3 |
| TCMP4 | 4.39 | 15.80 | 0.22 | 0.28 | 11.60 | 2.78 | 99.8 | 98.5 | 96.3 |
| CMP | – | 16.15 | – | 0.34 | 14.81 | 3.77 | – | 98.9 | 97.1 |
| Sample | S, m2·g−1 | Pore Volume, cm3·g−1 | Pore Diameter, nm |
|---|---|---|---|
| TP | 124.48 | 0.41 | 11.23 |
| TCMP1 | 66.88 | 0.24 | 14.15 |
| TCMP2 | 51.35 | 0.13 | 12.58 |
| TCMP4 | 45.78 | 0.10 | 8.52 |
| CMP | 34.27 | 0.09 | 5.68 |
| Formula | Ksp | Formula | pH of the Initial Stage of Hydroxide Precipitation |
|---|---|---|---|
| Cd3(PO4)2 | 2.5·10−33 | Cd(OH)2 | 8.3 |
| Co3(PO4)2 | 2.1·10−35 | Co(OH)2 | 8.2 |
| Cs3PO4 | 1.1·10−7 | CsOH | 13.0 |
| Cu3(PO4)2 | 1.4·10−37 | Cu(OH)2 | 5.2 |
| Pb3(PO4)2 | 7.9·10−43 | Pb(OH)2 | 6.4 |
| Sr3(PO4)2 | 1.0·10−31 | Sr(OH)2 | 8.5 |
| Zn3(PO4)2 | 9.1·10−33 | Zn(OH)2 | 6.4 |
| CaHPO4 | 2.7·10−7 | ||
| MgHPO4 | 0.2·10−5 | ||
| MgNH4PO4 | 2.5·10−13 |
| Sample | Activity of Radionuclides Aeq, 105 Bq·L−1 | Distribution Coefficient Kd, 105 mL·g−1 | Removal Efficiency S, % | ||||||
|---|---|---|---|---|---|---|---|---|---|
| 137Cs | 90Sr | 60Co | 137Cs | 90Sr | 60Co | 137Cs | 90Sr | 60Co | |
| TP | 3.20·10−2 | 4.67·10−2 | 2.76·10−2 | 3.52 | 2.15 | 0.754 | 99.93 | 99.88 | 99.67 |
| TCMP1 | 1.80·10−2 | 3.94·10−2 | 2.52·10−2 | 6.25 | 2.55 | 0.826 | 99.96 | 99.90 | 99.70 |
| TCMP2 | 4.35 | 3.52·10−2 | 9.31·10−3 | 2.34·10−2 | 2.85 | 2.24 | 90.34 | 99.91 | 99.89 |
| TCMP4 | 20.46 | 1.28·10−2 | 1.11·10−3 | 3.00·10−3 | 7.84 | 18.80 | 54.55 | 99.97 | 99.99 |
| CMP | 41.71 | 5.16·10−3 | 5.45·10−4 | 2.65·10−4 | 19.46 | 38.30 | 9.58 | 99.99 | 99.99 |
| Sample | Ti:P (Molar) | (Ca+Mg):P (Molar) | Ti:(Ca+Mg) (Molar) | Time, h |
|---|---|---|---|---|
| TP | 1:1 | – | – | 5 |
| TCMP4 | 1:1 | 1:1 | 1:4 | 5 + 3 * |
| TCMP2 | 1:1 | 1:1 | 1:2 | 5 + 3 * |
| TCMP1 | 1:1 | 1:1 | 1:1 | 5 + 3 * |
| CMP | – | 1:1 | – | 3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mudruk, N.; Maslova, M. The Effect of Sorbent Composition on Sorption Properties of Materials Based on Ti-Ca-Mg Phosphates. Int. J. Mol. Sci. 2023, 24, 7903. https://doi.org/10.3390/ijms24097903
Mudruk N, Maslova M. The Effect of Sorbent Composition on Sorption Properties of Materials Based on Ti-Ca-Mg Phosphates. International Journal of Molecular Sciences. 2023; 24(9):7903. https://doi.org/10.3390/ijms24097903
Chicago/Turabian StyleMudruk, Natalia, and Marina Maslova. 2023. "The Effect of Sorbent Composition on Sorption Properties of Materials Based on Ti-Ca-Mg Phosphates" International Journal of Molecular Sciences 24, no. 9: 7903. https://doi.org/10.3390/ijms24097903
APA StyleMudruk, N., & Maslova, M. (2023). The Effect of Sorbent Composition on Sorption Properties of Materials Based on Ti-Ca-Mg Phosphates. International Journal of Molecular Sciences, 24(9), 7903. https://doi.org/10.3390/ijms24097903





