Length Effect on the Stress Detection of Prestressed Steel Strands Based on Electromagnetic Oscillation Method
Abstract
1. Introduction
1.1. Ultrasound Guided Wave Method
1.2. Embedded Fiber Sensors or Magnetoelastic Devices
1.3. Electromagnetic Oscillation Method
2. Theories and Models
2.1. Principle of LC Electromagnetic Oscillation
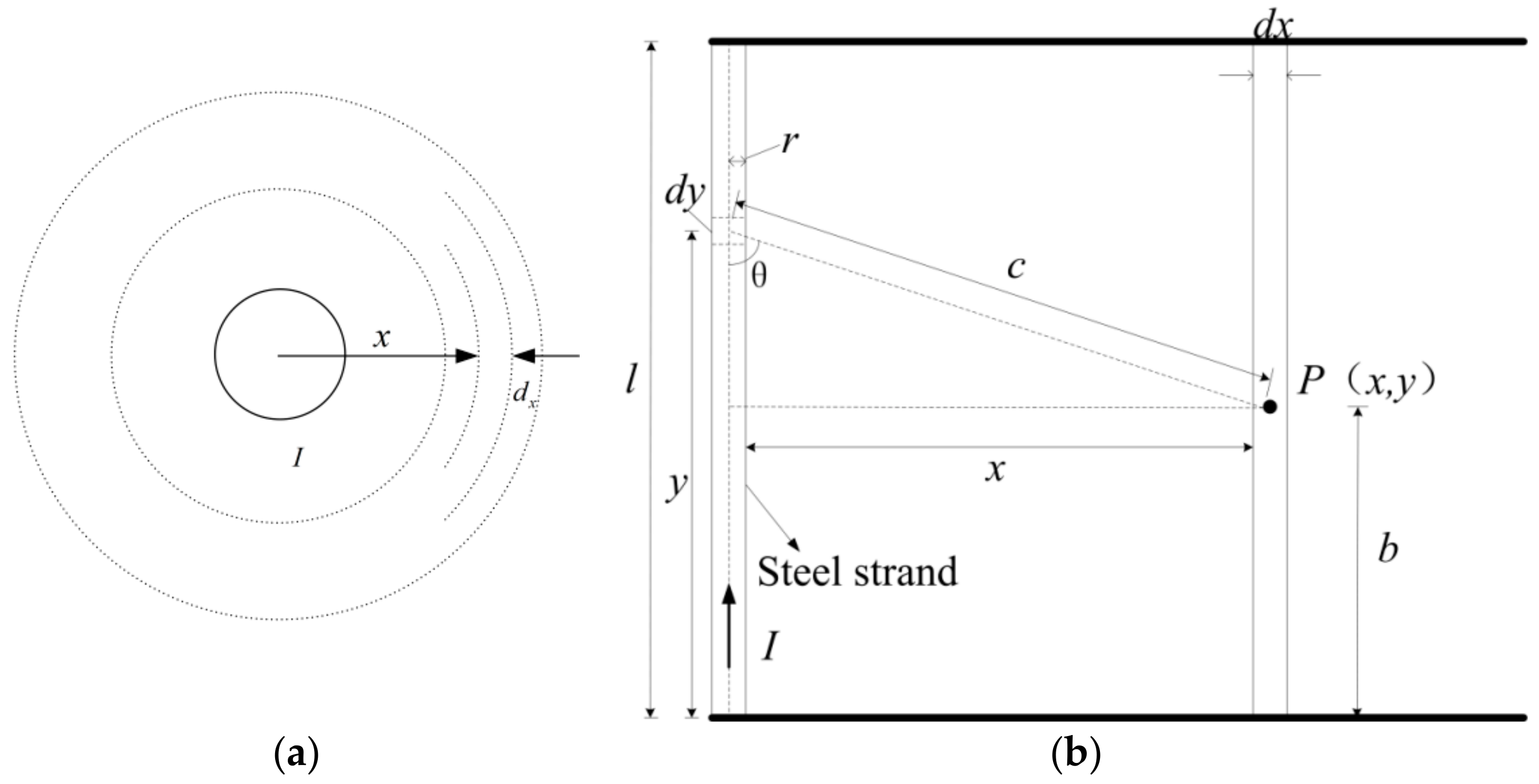
2.2. Inductance Model of Segment Wire
2.2.1. Modeling
2.2.2. Simulation Result
2.3. Inductance Model of Spiral Coil
2.3.1. Modeling
2.3.2. Simulation Result
3. Experimental Studies
3.1. Parameters of Steel Strand
3.2. Experimental Systems
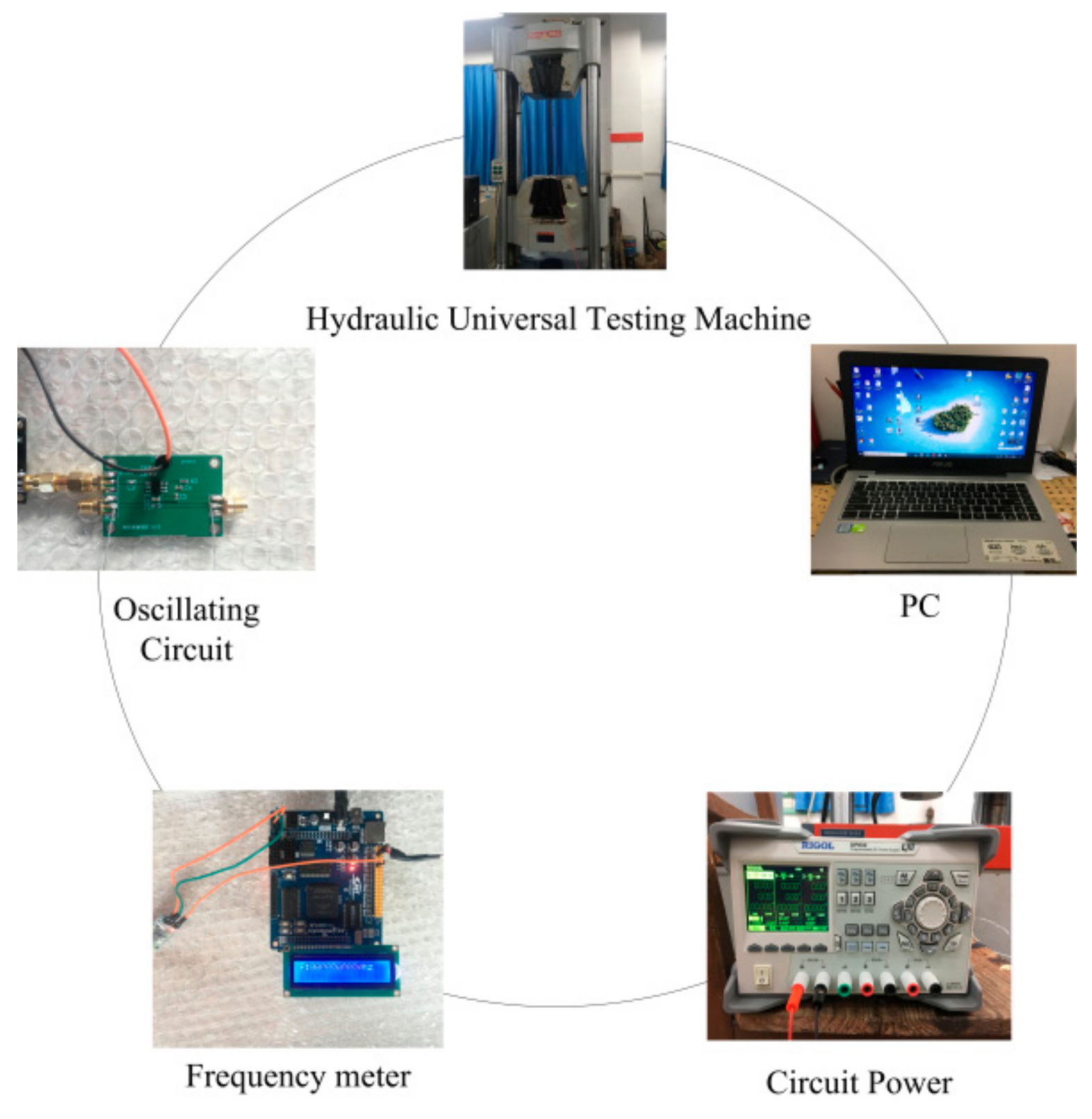
3.2.1. Experimental Devices and Procedure of Short Steel Strand (1.2 m)
3.2.2. Experimental Devices and Procedure of Long Steel Strand (5 m, 10 m and 15 m)
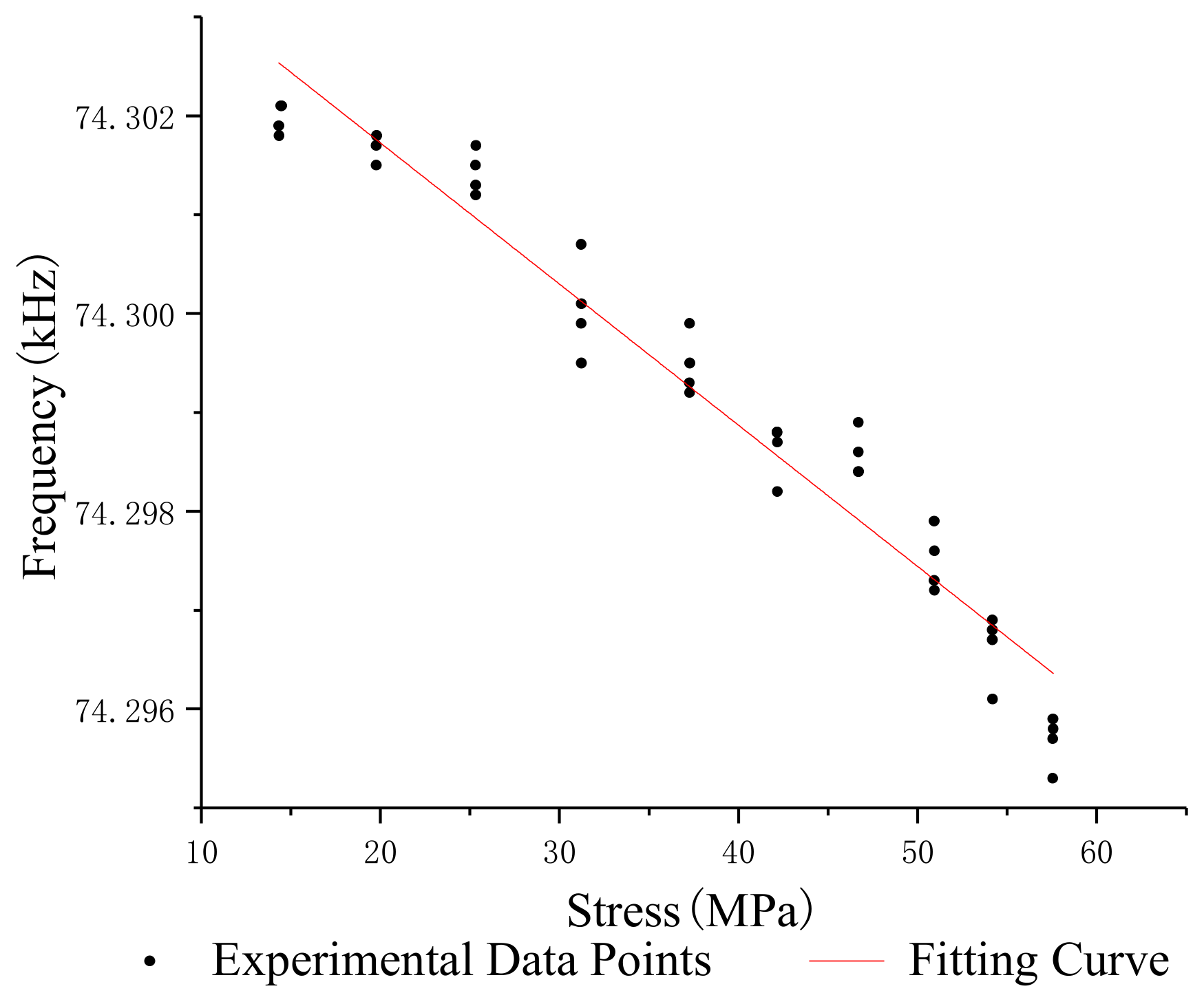
3.3. Experimental Data
- (1)
- The repeatability error of the 1.2 m steel strand test data does not exceed 0.023%, and that of the 5 m, 10 m and 15 m steel strands test data are less than 0.025%, 0.05% and 0.05%.
- (2)
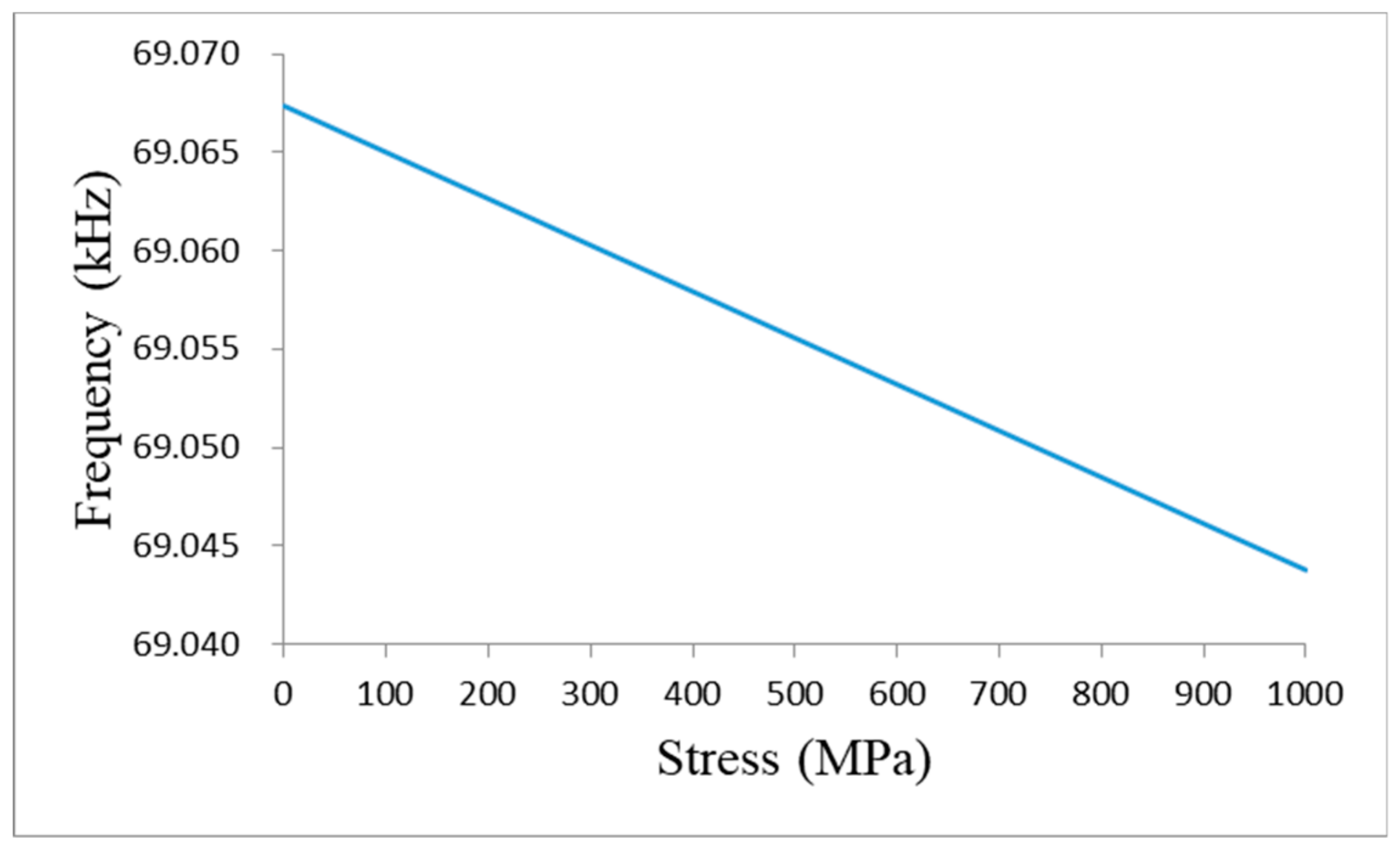
- Different lengths of steel strands have different stress-frequency trends. The data analysis of the tensile test of the 1.2 m steel strand shows that the frequency decreases with the increase of stress; while the test analysis of 5 m, 10 m and 15 m steel strands shows that the frequency increases with the increase of stress. It can be seen that the 1.2 m steel strands mainly exhibit the inductance characteristics of the segment wire, while the 5 m, 10 m and 15 m steel strands exhibit the inductance characteristics of the spiral coil.
- (3)
- The linearity of stress and frequency fitting curves of different lengths of strands is diversity. The correlation of the stress-frequency fitting curves of the 5 m, 10 m and 15 m strands are 0.8569, 0.9221 and 0.9801. With the increase in the length of the steel strand, the more concentrated the experimental data of stress-frequency is, and the better linear correlation of the curves.
- (4)
- In summary, the steel strands exhibit different inductance characteristics in the LC electromagnetic oscillation circuit, and the length of steel strand is the main factor.
4. Results and Discussion
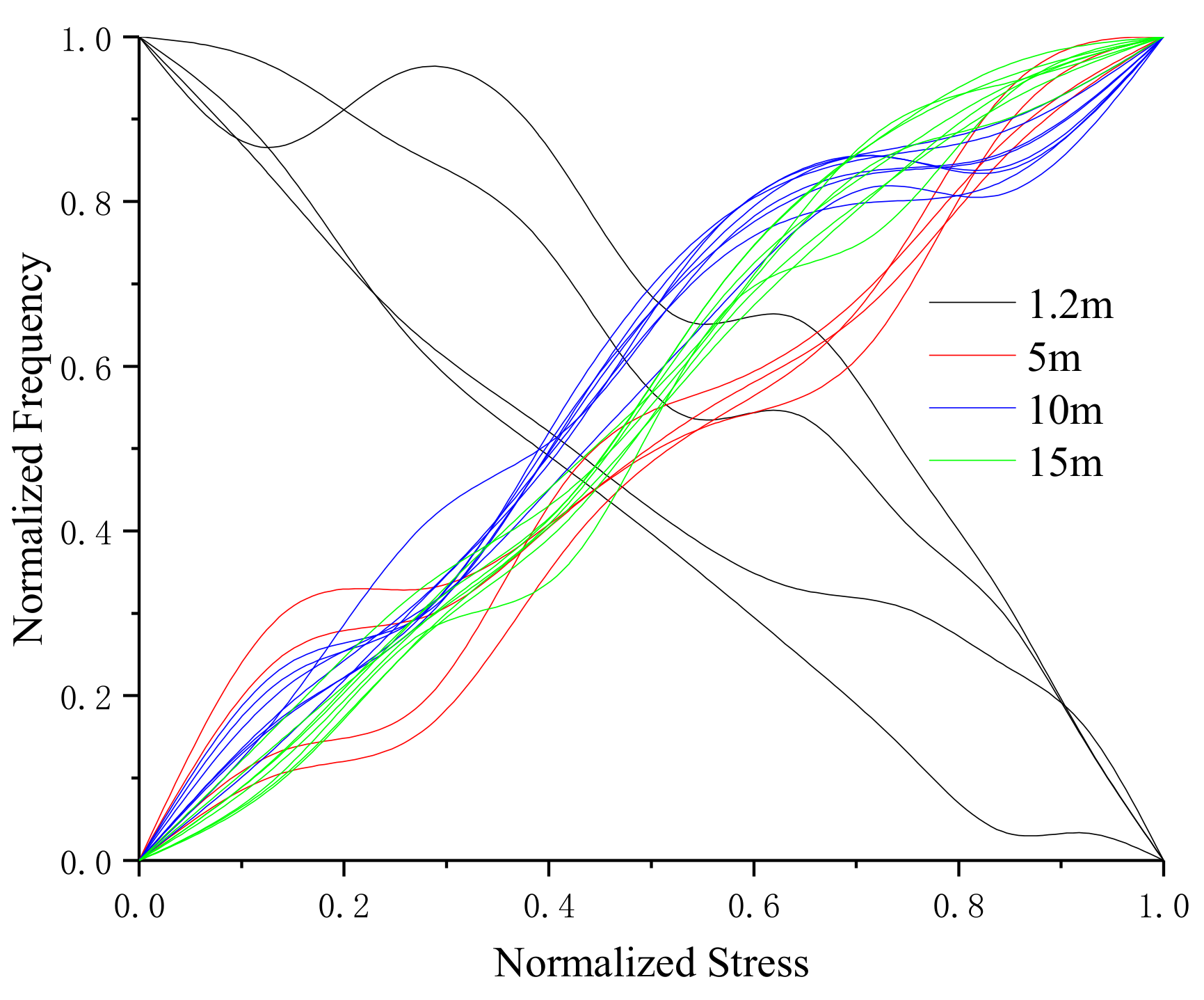
4.1. Analysis of Length Effect
- (1)
- The variation trend of stress-frequency curve of the four steel strands are different. The stress-frequency of the 1.2 m steel strand is negatively correlated, while the other three length are positively correlated.
- (2)
- The stress-frequency variation trends of 1.2 m and 10 m steel strands coincided with the results of the author’s previous research. What is more, the force–frequency curve of the 1.2 m steel strand shows large dispersion, and the general variation trend is that the oscillation frequency decreases with the increase of the stress. Compared with the 1.2 m steel strand, the stress-frequency curves of 5 m, 10 m and 15 m steel strands have smaller dispersion and better repeatability. The general variation trend is that the resonant frequency increases with the increase of stress.
- (3)
- The stress-frequency variation rule of steel strands transitions from a negative correlation of 1.2 m to a positive correlation of 5 m, indicating that the critical length of the long and short steel strands is within the range of (1.2 m, 5 m). Therefore, the critical length can be obtained by fitting the relationship between and in the stress-frequency fitting curve obtained from the experimental data of each length.
4.2. Analysis and Discussion
- (1)
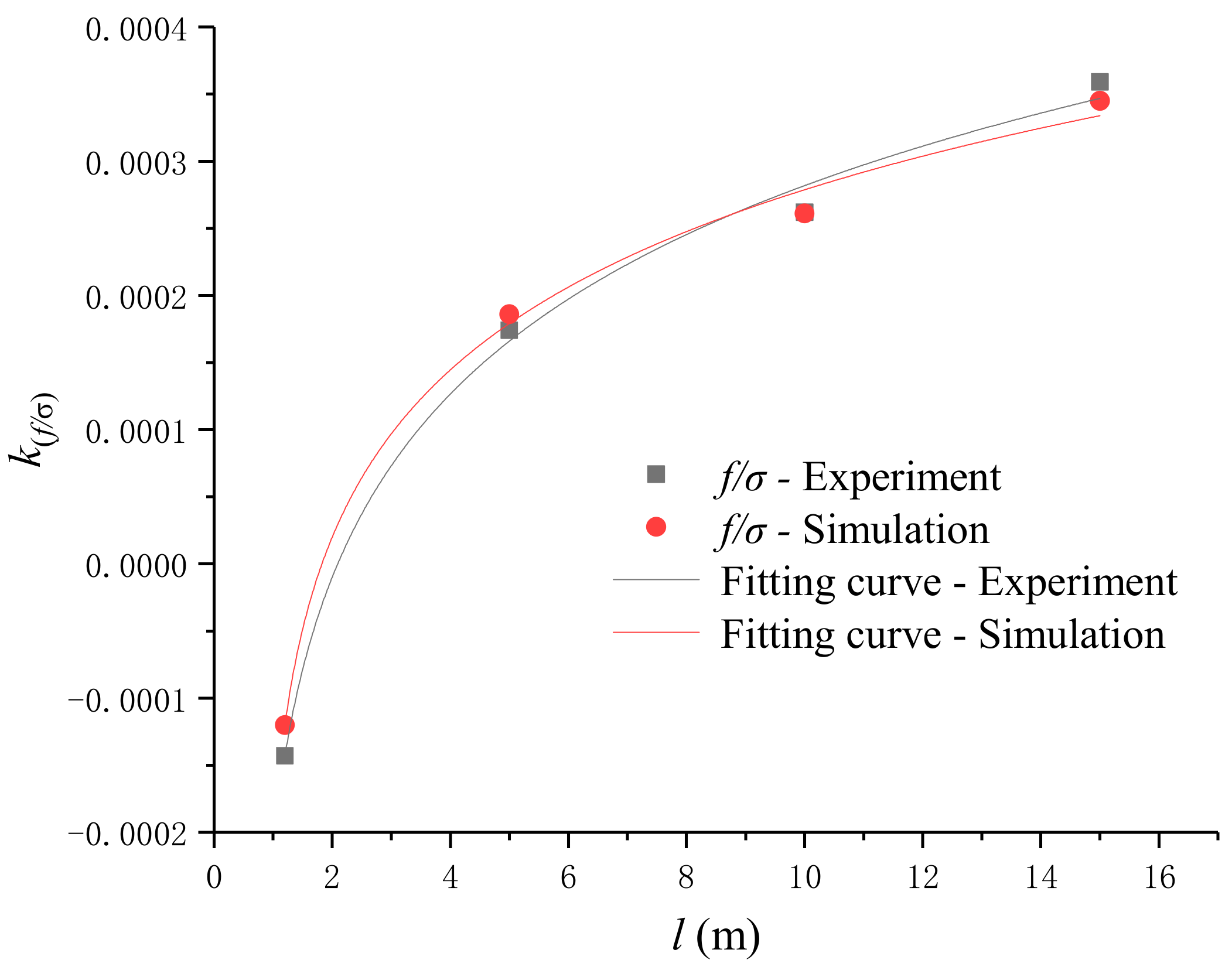
- The experimental length effect curves and Equation (31) show that the stress-frequency variation trend of steel strand is related to the length. The critical length for distinguishing long or short steel strands is . When , the oscillation frequency decreases with the increase of stress; when , the oscillation frequency increases with the increase of stress.
- (2)
- Similar to the analysis results of experimental length effect, the stress-frequency variation trend of simulation length effect is also related to the length of steel strand. The critical length for distinguishing long or short steel strands is . When , the oscillation frequency decreases with the increase of stress; when , the oscillation frequency increases with the increase of stress.
- (3)
- The error of critical length between experimental length effect and simulation length effect is 8.46%, which meets the requirement of application.
- (4)
- It can be inferred from the trend of the length effect curve that when the length of steel strand is longer than 15 m, the oscillation frequency increases with the increase of stress.
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Qian, J.; Chen, X.; Sun, L.; Yao, G.; Wang, X. Numerical and experimental identification of seven-wire strand tensions using scale energy entropy spectra of ultrasonic guided waves. Shock Vib. 2018, 2018, 6905073. [Google Scholar] [CrossRef]
- Niederleithinger, E.; Herbrand, M.; Muller, M. Monitoring of shear tests on prestressed concrete continuous beams using ultrasound and coda wave interferometry. Bauingenieur 2017, 92, 474–481. [Google Scholar]
- Feng, H.; Liu, X.; Wu, B.; Han, Q.; He, C. Design of a miniaturised ultrasonic guided wave inspection instrument for steel strand flaw detection. Insight 2017, 59, 17–23. [Google Scholar]
- Xu, J.; Wu, X.; Sun, P. Detecting broken-wire flaws at multiple locations in the same wire of prestressing strands using guided waves. Ultrasonics 2013, 53, 150–156. [Google Scholar] [CrossRef] [PubMed]
- Farhidzadeh, A.; Salamone, S. Reference-free corrosion damage diagnosis in steel strands using guided ultrasonic waves. Ultrasonics 2015, 57, 198–208. [Google Scholar] [CrossRef] [PubMed]
- Chaki, S.; Bourse, G. Guided ultrasonic waves for non-destructive monitoring of the stress levels in prestressed steel strands. Ultrasonics 2009, 49, 162–171. [Google Scholar] [CrossRef] [PubMed]
- Rizzo, P. Ultrasonic wave propagation in progressively loaded multi-wire strands. Exp. Mech. 2006, 46, 297–306. [Google Scholar] [CrossRef]
- Kim, J.M.; Kim, H.W.; Park, Y.H.; Yang, I.H.; Kim, Y.S. FGB sensors encapsulated into 7-wire steel strand for tension monitoring of a prestressing tendon. Adv. Struct. Eng. 2012, 15, 907–917. [Google Scholar] [CrossRef]
- Perry, M.; Yan, Z.; Sun, Z.; Zhang, L.; Niewczas, P.; Johnston, M. High stress monitoring of prestressing tendons in nuclear concrete vessels using fibre-optic sensors. Nucl. Eng. Des. 2014, 268, 35–40. [Google Scholar] [CrossRef]
- Leung, C.K.Y.; Wan, K.; Inaudi, D.; Bao, X.; Habel, W.; Zhou, Z.; Ou, J.; Ghandehari, M.; Wu, H.; Imai, M. Review: Optical fiber sensors for civil engineering applications. Mater. Struct. 2015, 48, 871–906. [Google Scholar] [CrossRef]
- Huynh, T.C.; Kim, J.T. FOS-Based Prestress Force Monitoring and Temperature Effect Estimation in Unbonded Tendons of PSC Girders. J. Aerosp. Eng. 2017, 30, B4016005. [Google Scholar] [CrossRef]
- Kim, T.M.; Kim, D.H.; Kim, M.K.; Lim, Y.M. Fiber Bragg grating-based long-gauge fiber optic sensor for monitoring of a 60 m full-scale prestressed concrete girder during lifting and loading. Sensor. Actuators A-Phys. 2016, 252, 134–145. [Google Scholar] [CrossRef]
- Lan, C.; Zhou, Z.; Ou, J. Monitoring of structural prestress loss in RC beams by inner distributed Brillouin and fiber Bragg grating sensors on a single optical fiber. Struct. Control Health 2014, 21, 317–330. [Google Scholar] [CrossRef]
- Militzer, M. A synchrotron look at steel. Science 2002, 298, 975–976. [Google Scholar] [CrossRef] [PubMed]
- Duan, Y.; Zhang, R.; Zhao, Y.; Or, S.W.; Fan, K.; Tang, Z. Steel stress monitoring sensor based on elasto-magnetic effect and using magneto-electric laminated composite. J. Appl. Phys. 2012, 111, 07E516. [Google Scholar] [CrossRef]
- Ricken, W.; Schoenekess, H.C.; Becker, W.-J. Improved multi-sensor for force measurement of pre-stressed steel cables by means of the eddy current technique. Sens. Actuators 2006, 129, 80–85. [Google Scholar] [CrossRef]
- Guo, T.; Chen, Z.; Lu, S.; Yao, R. Monitoring and analysis of long-term prestress losses in post-tensioned concrete beams. Measurement 2018, 122, 573–581. [Google Scholar] [CrossRef]
- Chen, Z.; Zhang, S. EM-Based monitoring and probabilistic analysis of prestress loss of bonded tendons in PSC beams. Adv. Civ. Eng. 2018, 4064362. [Google Scholar] [CrossRef]
- Kim, J.; Kim, J.W.; Lee, C.; Park, S. Development of Embedded EM Sensors for Estimating Tensile Forces of PSC Girder Bridges. Sensors 2017, 17, 1989. [Google Scholar] [CrossRef]
- Yim, J.; Wang, M.; Shin, S. Field application of elasto-magnetic stress sensors for monitoring of cable tension force in cable-stayed bridges. Smart Struct. Syst. 2013, 12, 465–482. [Google Scholar] [CrossRef]
- Chen, D.; Zhang, B.; Li, X.; Tu, C.; Yuan, C.; Li, W.; Zhou, Z.; Liang, Z. A stress measurement method for steel strands based on LC oscillation. Adv. Mater. Sci. Eng. 2018, 2018, 1584903. [Google Scholar] [CrossRef]
- Li, X.; Zhang, B.; Yuan, C.; Tu, C.; Chen, D.; Chen, Z.; Li, Y. An electromagnetic oscillation method for stress measurement of steel strands. Measurement 2018, 125, 330–335. [Google Scholar] [CrossRef]

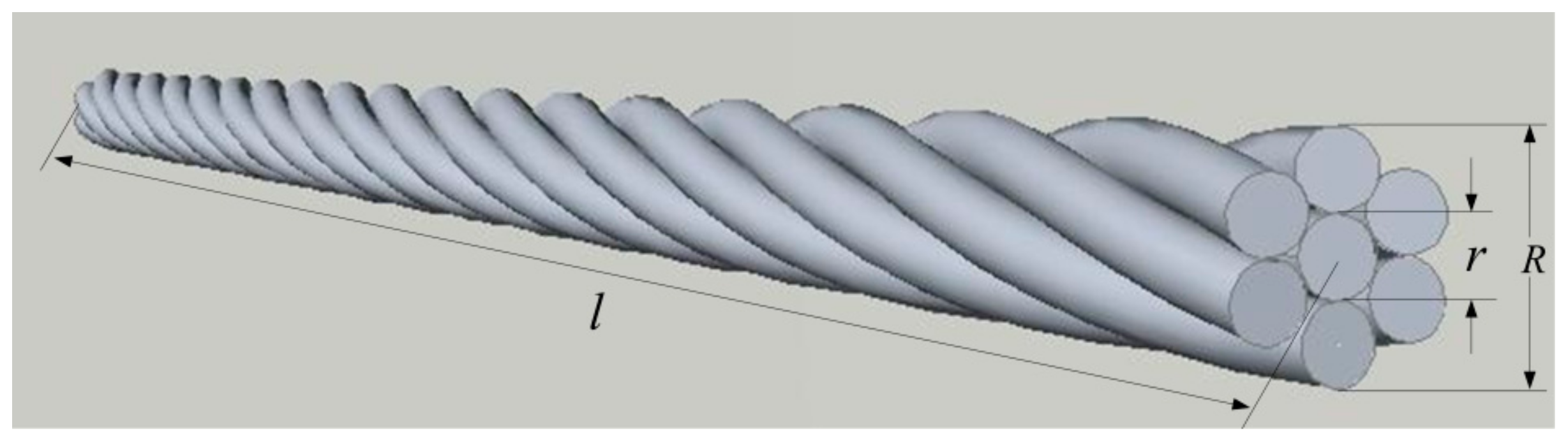

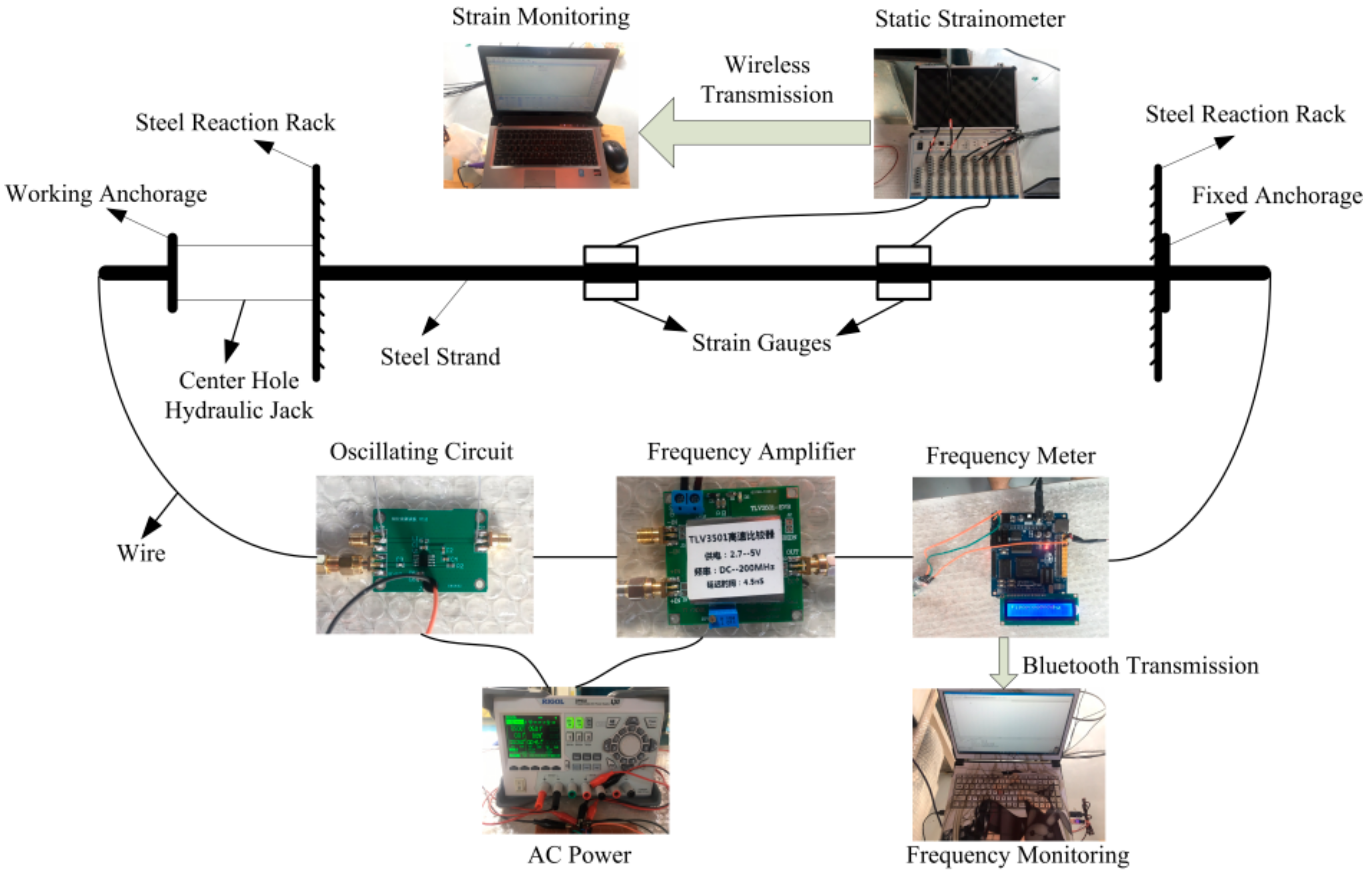



| Structure of Steel Strand | Length of Steel Strand m | Nominal Area of Steel Strand S mm2 | Nominal Diameter of Steel Strand D mm | Ultimate Tensile Strength Rm MPa No Less than | Maximum Tension Fm kN No Less than | Maximum Elongation AGT % No Less than |
|---|---|---|---|---|---|---|
| 1 × 7 | 1.2, 5, 10 and 15 | 139 | 15.2 | 1860 | 260 | 3.5 |
| Average Stress /MPa | Measurement Times | SD | RE | MF /kHz | |||
|---|---|---|---|---|---|---|---|
| Loading 1 /kHz | Loading 2 /kHz | Loading 3 /kHz | Loading 4 /kHz | ||||
| 14.389 | 74.3009 | 74.2940 | 74.2748 | 74.2677 | 0.01566 | 0.0211% | 74.2844 |
| 19.784 | 74.3018 | 74.2926 | 74.2746 | 74.2669 | 0.01604 | 0.0216% | 74.2840 |
| 25.324 | 74.3018 | 74.2911 | 74.2740 | 74.2656 | 0.01636 | 0.0220% | 74.2831 |
| 31.223 | 74.3010 | 74.2900 | 74.2733 | 74.2647 | 0.01633 | 0.0220% | 74.2823 |
| 37.266 | 74.2995 | 74.2889 | 74.2720 | 74.2639 | 0.01610 | 0.0217% | 74.2811 |
| 42.158 | 74.2995 | 74.2883 | 74.2720 | 74.2632 | 0.01626 | 0.0219% | 74.2808 |
| 46.691 | 74.2984 | 74.2881 | 74.2712 | 74.2625 | 0.01621 | 0.0218% | 74.2801 |
| 50.935 | 74.2972 | 74.2875 | 74.2705 | 74.2619 | 0.01600 | 0.0215% | 74.2793 |
| 54.173 | 74.2951 | 74.2869 | 74.2696 | 74.2619 | 0.01528 | 0.0206% | 74.2784 |
| 57.554 | 74.2951 | 74.2855 | 74.2687 | 74.2617 | 0.01528 | 0.0206% | 74.2778 |
| Average Stress /MPa | Measurement Times | SD | RE | MF /kHz | |||
|---|---|---|---|---|---|---|---|
| Loading 1 /kHz | Loading 2 /kHz | Loading 3 /kHz | Loading 4 /kHz | ||||
| 203 | 178.534 | 178.572 | 178.630 | 178.615 | 0.04345 | 0.0243% | 178.5878 |
| 301 | 178.589 | 178.609 | 178.599 | 178.626 | 0.01578 | 0.0088% | 178.6058 |
| 402 | 178.596 | 178.613 | 178.607 | 178.632 | 0.01508 | 0.0084% | 178.6120 |
| 498 | 178.614 | 178.625 | 178.639 | 178.655 | 0.01775 | 0.0099% | 178.6333 |
| 600 | 178.629 | 178.641 | 178.652 | 178.671 | 0.01784 | 0.0100% | 178.6483 |
| 706 | 178.643 | 178.656 | 178.667 | 178.687 | 0.01863 | 0.0104% | 178.6633 |
| 792 | 178.678 | 178.677 | 178.687 | 178.711 | 0.01582 | 0.0089% | 178.6883 |
| 905 | 178.695 | 178.696 | 178.703 | 178.719 | 0.01109 | 0.0062% | 178.7033 |
| Average Stress /MPa | Measurement Sequence | SD | RE | MF /kHz | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Cycle 1 /kHz Loading | Cycle 1 /kHz Unloading | Cycle 2 /kHz Loading | Cycle 2 /kHz Unloading | Cycle 3 /kHz Loading | Cycle 3 /kHz Unloading | Cycle 4 /kHz Loading | Cycle 4 /kHz Unloading | ||||
| 205 | 125.992 | 126.005 | 126.040 | 126.053 | 126.108 | 126.122 | 126.139 | 126.155 | 0.06241 | 0.0495% | 126.0768 |
| 310 | 126.031 | 126.047 | 126.049 | 126.083 | 126.136 | 126.133 | 126.193 | 126.162 | 0.05999 | 0.0476% | 126.1043 |
| 408 | 126.087 | 126.088 | 126.075 | 126.087 | 126.161 | 126.146 | 126.228 | 126.177 | 0.05543 | 0.0439% | 126.1311 |
| 511 | 126.135 | 126.112 | 126.097 | 126.113 | 126.190 | 126.158 | 126.227 | 126.185 | 0.04571 | 0.0362% | 126.1521 |
| 605 | 126.156 | 126.141 | 126.119 | 126.139 | 126.220 | 126.229 | 126.264 | 126.228 | 0.05407 | 0.0429% | 126.1870 |
| 714 | 126.174 | 126.171 | 126.155 | 126.196 | 126.281 | 126.221 | 126.298 | 126.263 | 0.05474 | 0.0434% | 126.2199 |
| 803 | 126.151 | 126.169 | 126.195 | 126.213 | 126.267 | 126.284 | 126.272 | 126.284 | 0.05403 | 0.0428% | 126.2294 |
| 912 | 126.192 | 126.192 | 126.219 | 126.219 | 126.296 | 126.296 | 126.313 | 126.313 | 0.05427 | 0.0430% | 126.2550 |
| Average Stres /MPa | Measurement Sequence | SD | RE | MF /kHz | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Cycle 1 /kHz Loading | Cycle 1 /kHz Unloading | Cycle 2 /kHz Loading | Cycle 2 /kHz Unloading | Cycle 3 /kHz Loading | Cycle 3 /kHz Unloading | Cycle 4 /kHz Loading | Cycle 4 /kHz Unloading | ||||
| 204 | 117.170 | 117.182 | 117.190 | 117.201 | 117.215 | 117.219 | 117.254 | 117.250 | 0.03049 | 0.0260% | 117.2101 |
| 308 | 117.204 | 117.240 | 117.195 | 117.219 | 117.224 | 117.231 | 117.289 | 117.288 | 0.03524 | 0.0301% | 117.2363 |
| 401 | 117.259 | 117.314 | 117.244 | 117.251 | 117.253 | 117.258 | 117.304 | 117.321 | 0.03172 | 0.0270% | 117.2755 |
| 512 | 117.297 | 117.359 | 117.278 | 117.342 | 117.275 | 117.315 | 117.359 | 117.364 | 0.03722 | 0.0317% | 117.3236 |
| 604 | 117.370 | 117.403 | 117.305 | 117.389 | 117.302 | 117.425 | 117.401 | 117.412 | 0.04747 | 0.0404% | 117.3759 |
| 700 | 117.421 | 117.438 | 117.340 | 117.410 | 117.408 | 117.443 | 117.387 | 117.438 | 0.03424 | 0.0292% | 117.4106 |
| 803 | 117.447 | 117.459 | 117.403 | 117.421 | 117.444 | 117.478 | 117.467 | 117.478 | 0.02681 | 0.0228% | 117.4496 |
| 907 | 117.471 | 117.471 | 117.438 | 117.438 | 117.472 | 117.472 | 117.491 | 117.491 | 0.02038 | 0.0174% | 117.4680 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, B.; Tu, C.; Li, X.; Cui, H.; Zheng, G. Length Effect on the Stress Detection of Prestressed Steel Strands Based on Electromagnetic Oscillation Method. Sensors 2019, 19, 2782. https://doi.org/10.3390/s19122782
Zhang B, Tu C, Li X, Cui H, Zheng G. Length Effect on the Stress Detection of Prestressed Steel Strands Based on Electromagnetic Oscillation Method. Sensors. 2019; 19(12):2782. https://doi.org/10.3390/s19122782
Chicago/Turabian StyleZhang, Benniu, Chong Tu, Xingxing Li, Hongmei Cui, and Gang Zheng. 2019. "Length Effect on the Stress Detection of Prestressed Steel Strands Based on Electromagnetic Oscillation Method" Sensors 19, no. 12: 2782. https://doi.org/10.3390/s19122782
APA StyleZhang, B., Tu, C., Li, X., Cui, H., & Zheng, G. (2019). Length Effect on the Stress Detection of Prestressed Steel Strands Based on Electromagnetic Oscillation Method. Sensors, 19(12), 2782. https://doi.org/10.3390/s19122782




