Alternative Approaches to Vibration Measurement Due to the Blasting Operation: A Pilot Study
Abstract
:1. Introduction
2. Methods
2.1. Seismic Equipment BRS32
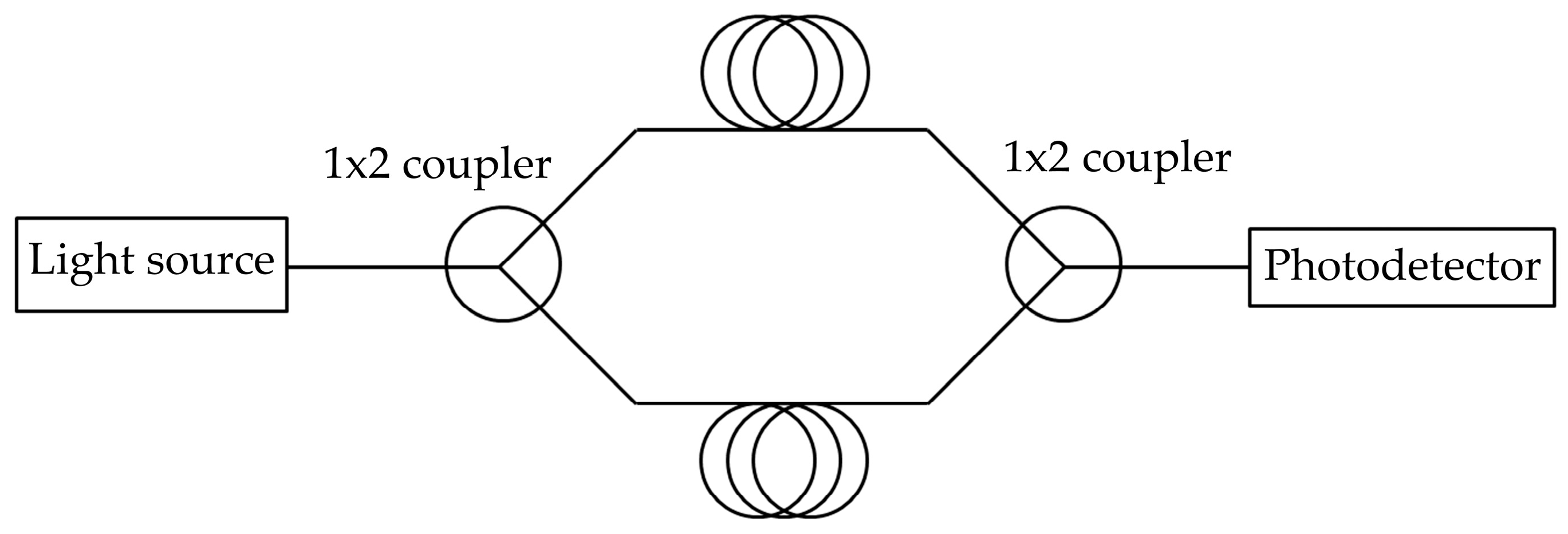
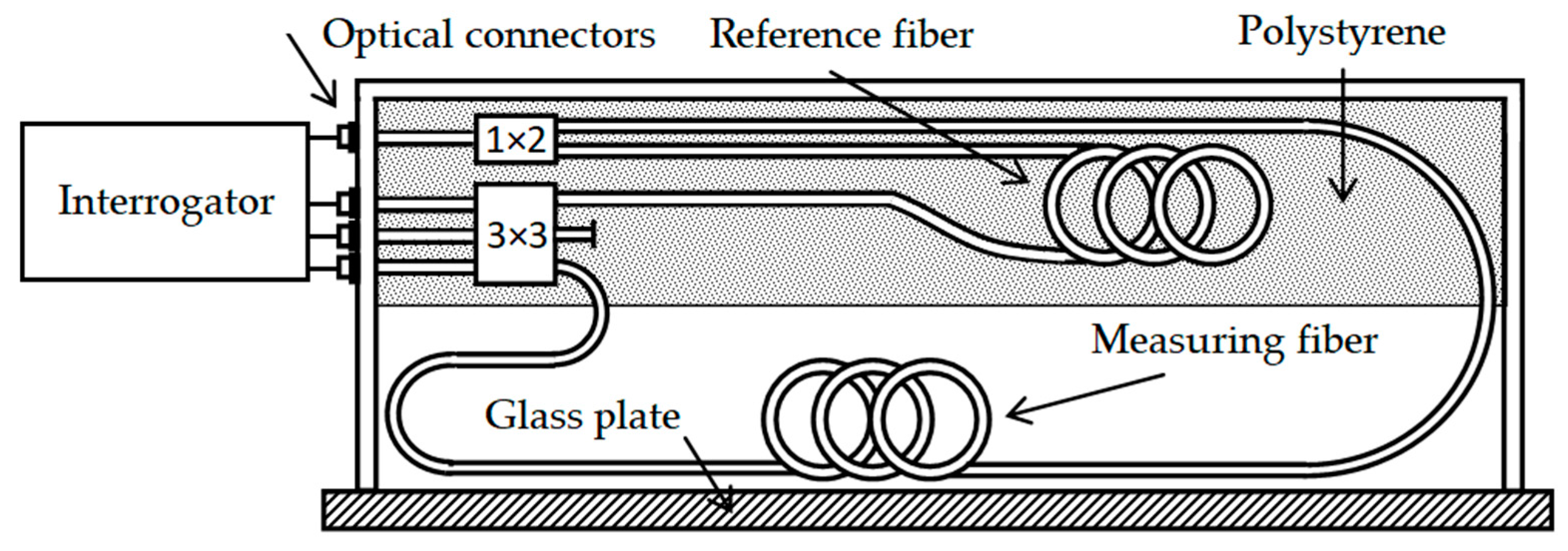
2.2. Experimentally Developed Fiber-Optic Interferometric Sensor
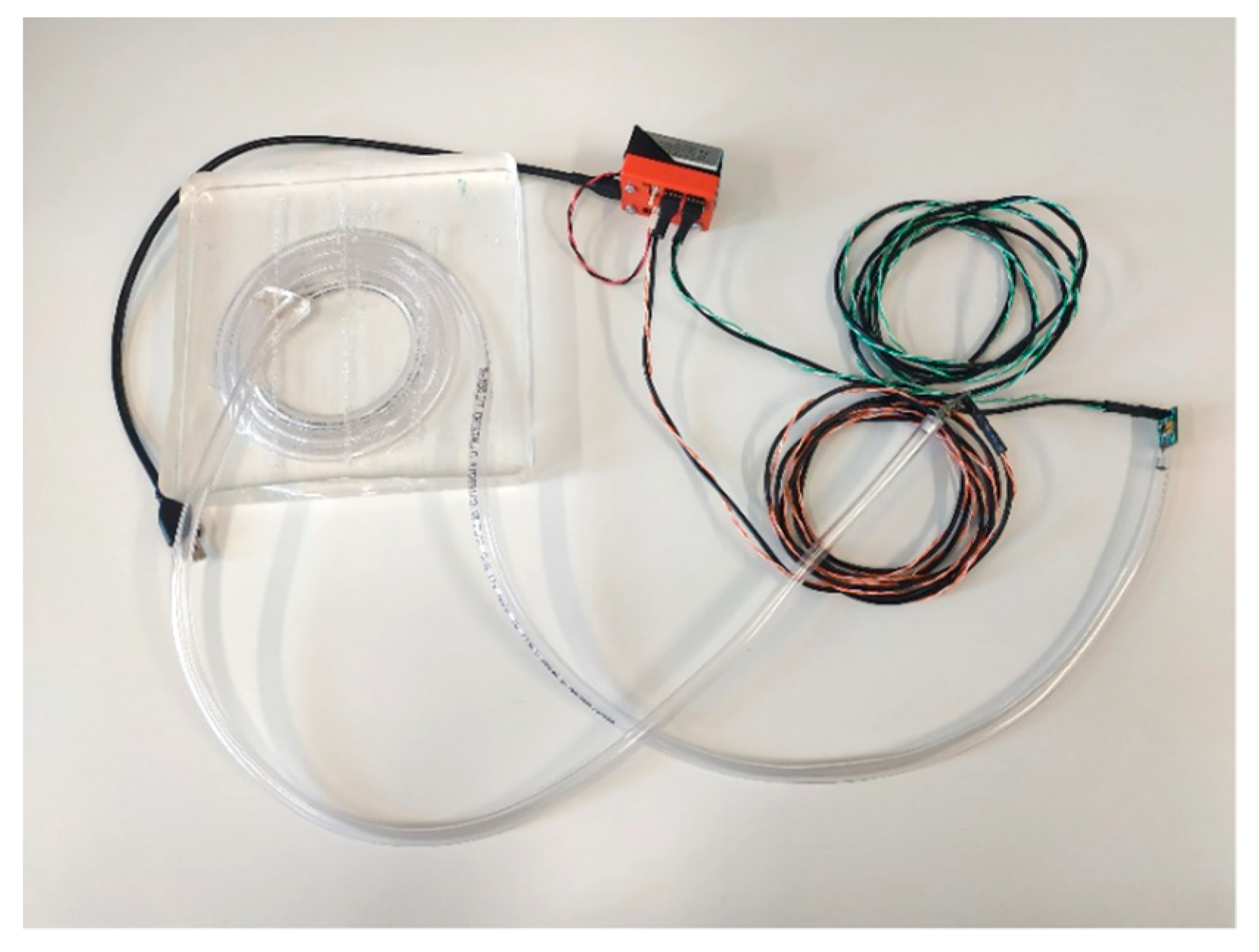
2.3. Experimentally Developed Acoustic Sensor
- Electret microphone model PUM-5250L-R;
- Low-noise operational amplifier OPA172;
- Microcontroller unit (MCU) STM32F373;
- Sampling rate 5 kHz;
- Polydimethylsiloxane (type Sylgard 184, weight 750 g);
- Acoustic tube length of 1.5 m (PVC material).
3. Experimental Setup
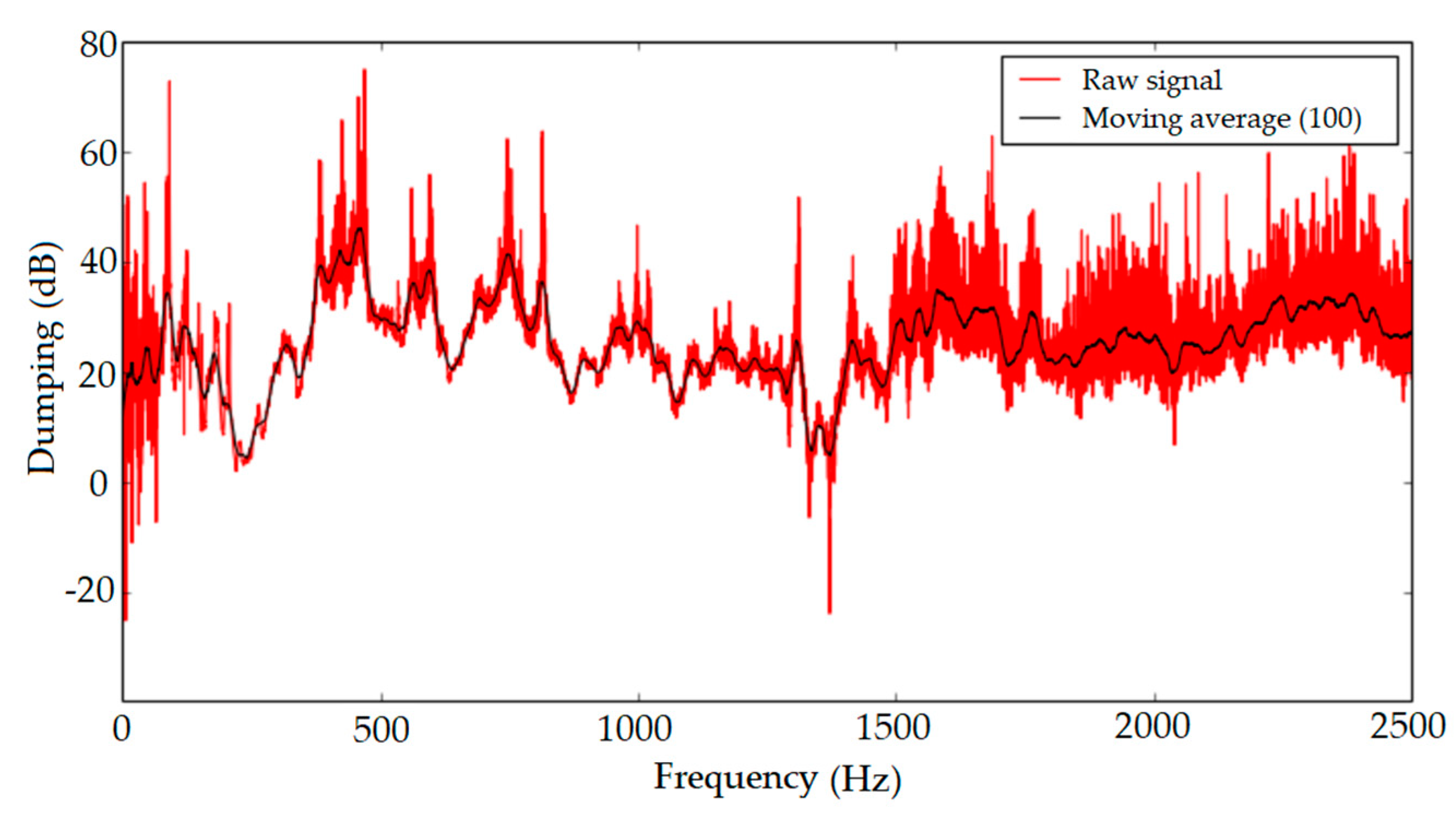
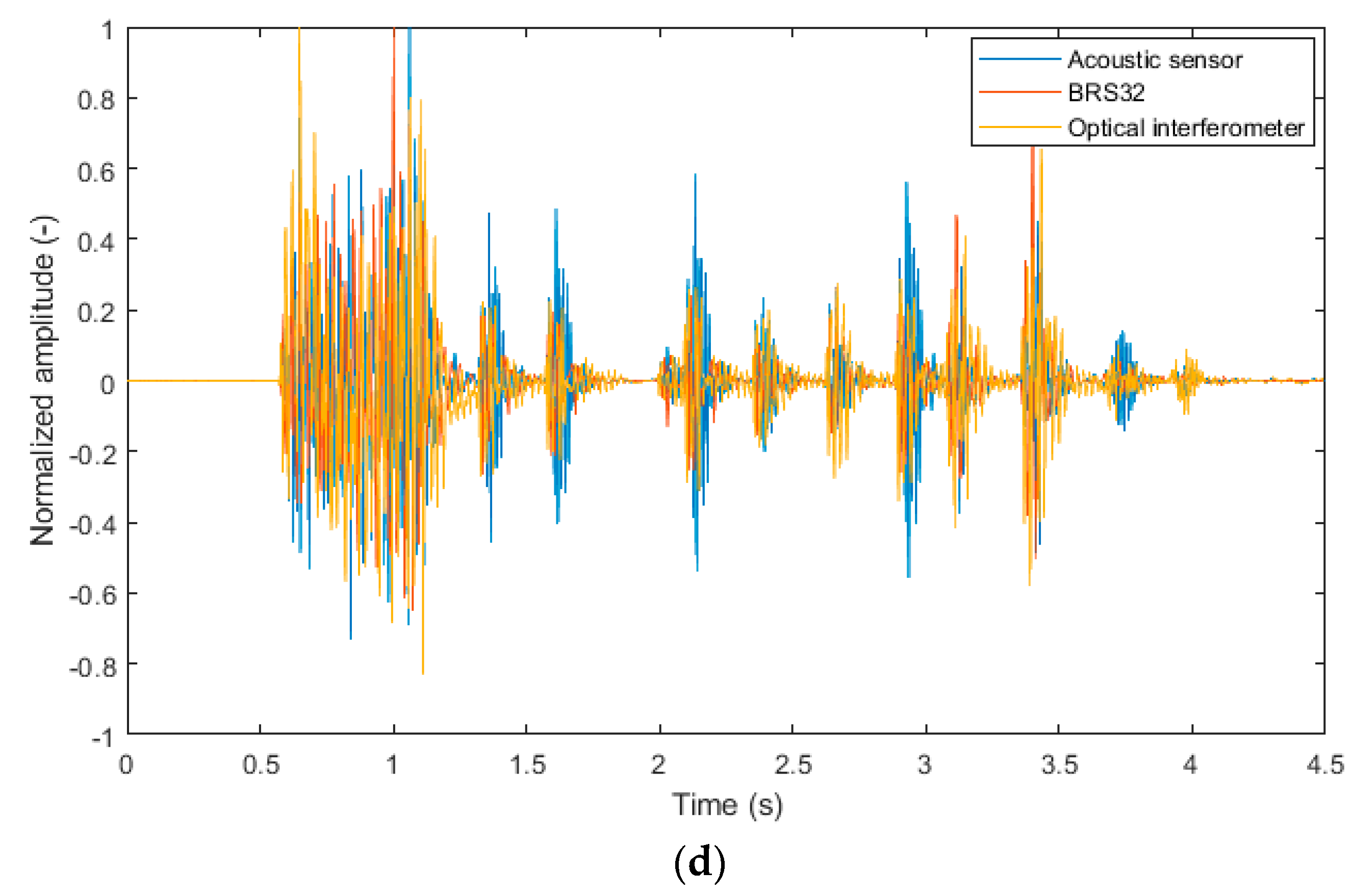
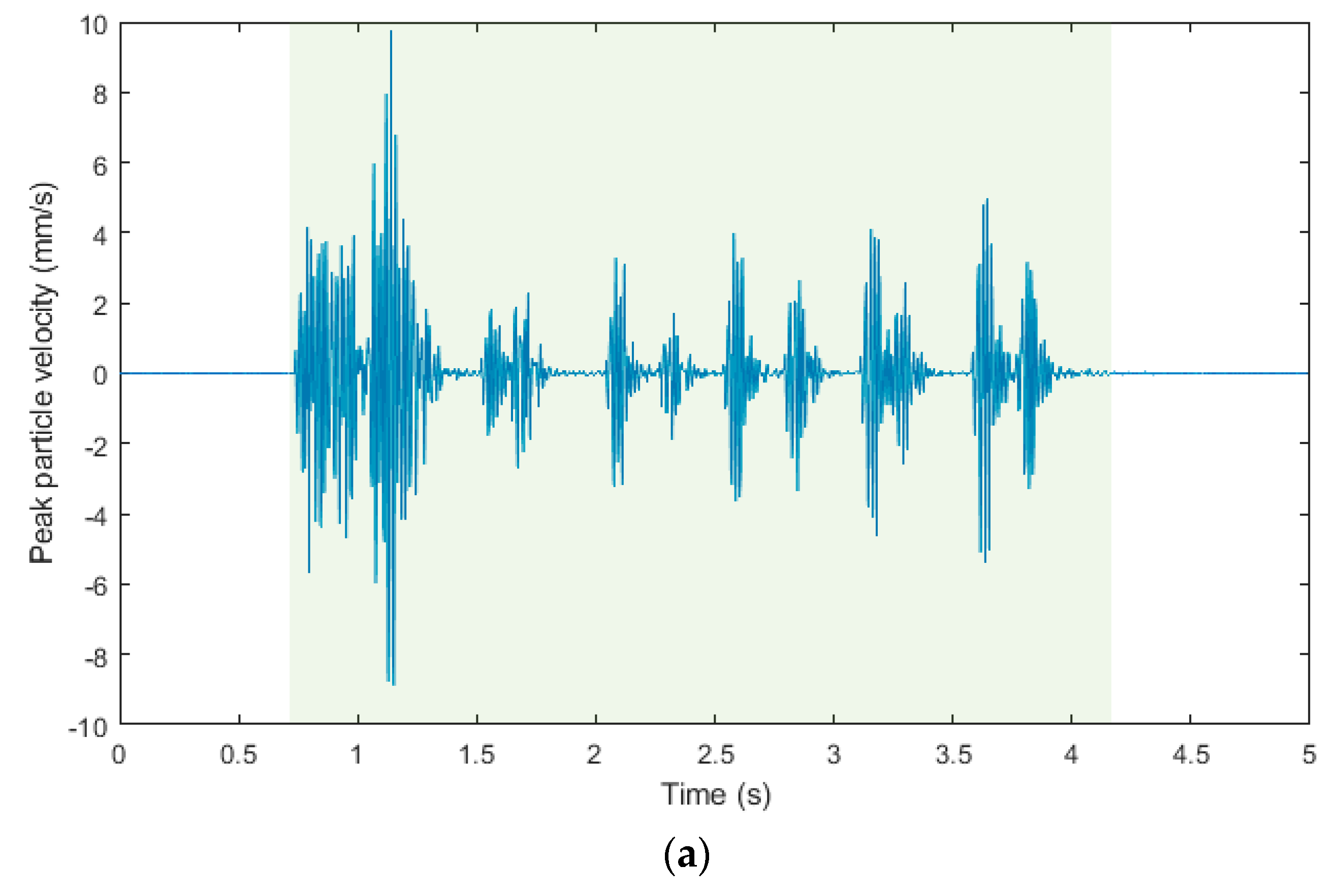
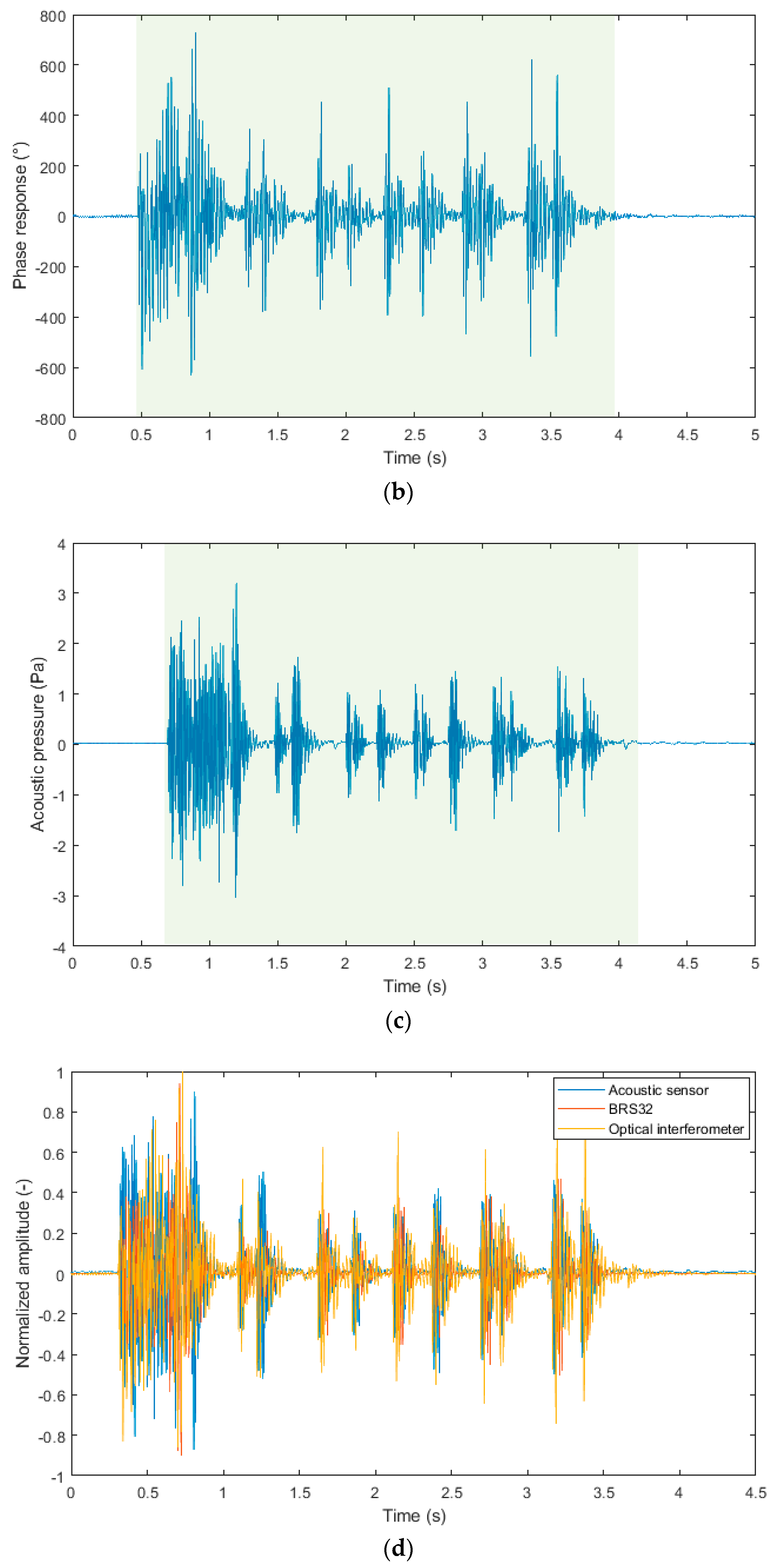
Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Bongiovanni, G. Experimental studies of vibrations caused by blasting for tunnel excavations. In Earthquake, Blast and Impacts: Measurements and Effects of Vibration; Elsevier Applied Science: Amsterdam, The Netherlands, 1991; pp. 201–210. [Google Scholar]
- Jiang, N.; Gao, T.; Zhou, C.; Luo, X. Effect of excavation blasting vibration on adjacent buried gas pipeline in a metro tunnel. Tunn. Undergr. Space Technol. 2018, 81, 590–601. [Google Scholar] [CrossRef]
- Jiang, J.; Li, H.; Hong, S.; Liu, Y.; Xia, X.; Zuo, H.; Zeng, Y. Monitoring and analysis of blasting vibration in tunnel excavation of nuclear power plant. Vibroeng. Procedia 2019, 22, 135–139. [Google Scholar] [CrossRef]
- Lai, J.; Fan, H.; Chen, J.; Qiu, J.; Wang, K. Blasting vibration monitoring of undercrossing railway tunnel using wireless sensor network. Int. J. Distrib. Sens. Netw. 2015. [Google Scholar] [CrossRef]
- Huang, D.; Cui, S.; Li, X. Wavelet packet analysis of blasting vibration signal of mountain tunnel. Soil Dyn. Earthq. Eng. 2019, 117, 72–80. [Google Scholar] [CrossRef]
- Kiehl, J.; Werfling, J. Measurements and analyses of blasting vibrations. In Proceedings of the 10th ISRM Congress, Sandton, South Africa, 8–12 September 2003; International Society for Rock Mechanics and Rock Engineering: Lisabon, Portugal, 2003; pp. 635–640. [Google Scholar]
- BSI Group. BS ISO 4866:2010 Mechanical Vibration and Shock, Vibration of Fixed Structures, Guidelines for the Measurement of Vibrations and Evaluation of Their Effects on Structures; BSI Group: London, UK, 2010. [Google Scholar]
- Regulation No. 272/2011—Coll. on Health Protection against Adverse Effects of Noise and Vibration. Available online: https://www.zakonyprolidi.cz/cs/2011-272 (accessed on 21 September 2019). (In Czech).
- Scheeper, P.; Gulløv, J.O.; Kofoed, L.M. A piezoelectric triaxial accelerometer. J. Micromech. Microeng. 1996, 6, 131–133. [Google Scholar] [CrossRef]
- Kaláb, Z.; Pandula, B.; Stolárik, M.; Kondela, J. Contribution to measurements of vibration during driving of tunnels. EGRSE J. 2011, XVIII/3, 62–71. [Google Scholar]
- Lopez-Higuera, J.M. Handbook of Optical Fiber Sensing Technology; Wiley: Hoboken, NY, USA, 2002; 828p, ISBN 978-0-471-82053-6. [Google Scholar]
- Nesterov, V.V.; Nasonkin, V.A. Characteristics of orientation of long-baseline laser interferometry seismic deformographs. Geophys. J. 2001, 16, 561–568. [Google Scholar]
- Papp, B.; Donno, D.; Martin, J.E.; Hartog, A.H. Fundamentals of vibration sensing with distributed fiber optic sensors through downscaled experiments. In Proceedings of the 76th European Association of Geoscientists and Engineers Conference and Exhibition 2014: Experience the Energy—Incorporating SPE EUROPEC, Amsterdam, The Netherlands, 16–19 June 2014; p. 622, ISBN 978-163266694-9. [Google Scholar]
- Schreiber, K.U.; Stedmam, G.E.; Igel, H.; Flaws, A. Ring laser gyroscopes as rotation sensors for seismic wave studies. In Earthquake Source Asymmetry, Structural Media and Rotation Effects; Teisseyre, R., Takeo, M., Majewski, E., Eds.; Springer: Berlin/Heidelberg, Germany, 2006; pp. 377–390. [Google Scholar]
- Grigorie, L.T.; Edu, I.R.; Abochiei, F.C. Trends in the development of miniature optical accelerometers. In Proceedings of the International Multidisciplinary Scientific Geoconference surveying Geology and Mining Ecology Management, SGEM, Albena, Bulgaria, 17–26 June 2014; pp. 197–204. [Google Scholar]
- Zumberge, M.A.; Berger, J.; Hatfield, W.; Wielandt, E. A three-component borehole optical seismic and geodetic sensor. Bull. Seism. Soc. Am. 2018, 108, 2022–2031. [Google Scholar] [CrossRef]
- Beben, D.; Anigacz, W. Dynamic testing of railway metal culvert using geodetic methods. In Proceedings of the MATEC Web of Conferences, Oravsky Haj-Trstena, Slovakia, 21–25 May 2017. [Google Scholar] [CrossRef]
- Äraya, Ä.; Takamori, A.; Morii, W.; Mioyo, K.; Ohashi, M.; Hayama, K.; Uchiyama, T.; Miyoki, S.; Saito, Y. Design and operation of a 1500-m laser strainmeter installed at an underground site in Kamioka, Japan. Earthplanets Space 2017, 69. [Google Scholar] [CrossRef] [Green Version]
- Papp, B.; Donno, D.; Martin, J.E.; Hartog, A.H. A study of the geophysical response of distributed fiberoptic acoustic sensors through laboratory-scale experiments. Geophys. Prospect. 2017, 65, 1186–1204. [Google Scholar] [CrossRef]
- Nedoma, J.; Zboril, O.; Fajkus, M.; Zavodny, P.; Kepak, S.; Bednarek, L.; Martinek, R.; Vasinek, V. Fiber optic system design for vehicle detection and analysis. In Proceedings of the SPIE Photonics Europe, Brussels, Belgium, 4–7 April 2016. [Google Scholar] [CrossRef]
- Stolarik, M.; Pinka, M.; Zabka, S.; Novak, M. Dynamic effect of harmonic vibrations: Various approaches to monitor. In Proceedings of the SPIE Security + Defence, Berlin, Germany, 10–13 September 2018. [Google Scholar] [CrossRef]
- Nedoma, J.; Stolarik, M.; Fajkus, M.; Pinka, M.; Hejduk, S. Use of fiber-optic sensors for the detection of the rail vehicles and monitoring of the rock mass dynamic response due to railway rolling stock for the civil engineering needs. Appl. Sci. 2019, 9, 134. [Google Scholar] [CrossRef]
- Cao, Y.; Chen, H. Experiment of surrounding rock blasting vibration safety criterion based on time-energy density. Jiefangjun Ligong Daxue Xuebao/J. Plan. Univ. Sci. Technol. 2014, 15, 264–269. [Google Scholar]
- Lai, J.; Qiu, J.; Fan, H.; Zhang, Q.; Hu, Z.; Wang, J.; Chen, J. Fiber Bragg Grating Sensors-Based In Situ Monitoring and Safety Assessment of Loess Tunnel. J. Sens. 2016, 2016, 8658290, Corrigendum in J. Sens. 2019, 2019, 2972178. [Google Scholar] [CrossRef]
- Zhang, X.; Liu, X.; Zhang, F.; Sun, Z.; Min, L.; Li, S.; Jiang, S.; Li, M.; Wang, C.; Ni, J. Reliable high sensitivity FBG geophone for low frequency seismic acquisition. Meas. J. Int. Meas. Confed. 2018, 129, 62–67. [Google Scholar] [CrossRef]
- Lai, J.; Qiu, J.; Fan, H.; Chen, J.; Hu, Z.; Zhang, Q.; Wang, J. Structural Safety Assessment of Existing Multiarch Tunnel: A Case Study. Adv. Mater. Sci. Eng. 2017. [Google Scholar] [CrossRef]
- Schroeck, M.; Ecke, W.; Graupner, A. Strain monitoring in steel rock bolts using FBG sensor array. Spie Int. Soc. Opt. Eng. 2000, 4074, 298–304. [Google Scholar]
- Fajkus, M.; Nedoma, J.; Mec, P.; Hrubesova, E.; Martinek, R.; Vasinek, V. Analysis of the highway tunnels monitoring using an optical fiber implemented into primary lining. J. Electr. Eng. 2017, 68, 364–370. [Google Scholar] [CrossRef] [Green Version]
- Wang, F.; Huang, H.; Zhang, D.; Zhang, W.; Xu, R. Deformation sensing method of shield tunnel based on optical fiber sensing technology of BOTDA. Yanshilixue Yu Gongcheng Xuebao/Chin. J. Rock Mech. Eng. 2013, 32, 1901–1908. [Google Scholar]
- Song, S.; Wu, Z.; Yang, C.; Wan, C.; Shen, S. Deformation monitoring of shield tunnel based on distributed optical fiber strain sensing technology. In Structural Health Monitoring 2011: Condition-Based Maintenance and Intelligent Structures, Proceedings of the 8th International Workshop on Structural Health Monitoring, Stanford, CA, USA, 13–15 September 2011; Destech Publications: Lancaster, PA, USA, 2011; Volume 2, pp. 2058–2066. [Google Scholar]
- Moffat, R.A.; Beltran, J.F.; Herrera, R. Applications of BOTDR fiber optics to the monitoring of underground structures. Geomech. Eng. 2015, 9, 397–414. [Google Scholar] [CrossRef]
- Reed, J.W.; Colquitt, R. Acoustic Pulse Transfer System for Event Counting. U.S. Patent No. 7,022,925, 4 April 2006. [Google Scholar]
- Newmeyer, R.A. Sensor for Vehicular Traffic Data Accumulating Systems. U.S. Patent No. 3,949,355, 6 April 1976. [Google Scholar]
- Tyburski, R.M. Roadway Sensor Systems. U.S. Patent No. 5,450,077, 12 September 1995. [Google Scholar]
- Enoksson, P.; Göran, S.; Erik, S. Fluid density sensor based on resonance vibration. Sens. Actuators A Phys. 1995, 47, 327–331. [Google Scholar] [CrossRef]
- Krantz, W.B.; Bilodeau, R.R.; Voorhees, M.E.; Elgas, R.J. Use of axial membrane vibrations to enhance mass transfer in a hollow tube oxygenator. J. Membr. Sci. 1997, 124, 283–299. [Google Scholar] [CrossRef]
- Whitmore, S.A.; Matthew, D.W. Wiener deconvolution for reconstruction of pneumatically attenuated pressure signals. AIAA J. 2011, 49, 890–897. [Google Scholar] [CrossRef]
- Broz, M.; Strunc, J.; Malek, J.; Linda, M. New generation seismological datalogger BRS32-USB and its application in induced seismicity monitoring. EGRSE J. 2014, XXI/1, 35–47. [Google Scholar]
- Fang, Z.; Chin, K.; QU, R.; Cai, H. Fundamentals of Optical Fiber Sensors; Wiley: Hoboken, NJ, USA, 2012; ISBN 9780470575406. [Google Scholar]
- Todd, M.D.; Johnson, G.A.; Chány, C.C. Passive, light intensity-independent interferometric method for fibre Bragg grating interrogation. Electron. Lett. 1999, 35, 1970–1971. [Google Scholar] [CrossRef]
- Jones, D.I.G. Handbook of Viscoelastic Vibration Damping; J. Wiley: Hoboken, NJ, USA, 2001; ISBN 0471492485. [Google Scholar]
- Her, S.-C.; Tsai, C.-Y. Strain measurement of fiber optic sensor surface bonding on host material. Trans. Nonferrous Met. Soc. China 2009, 19, 143–149. [Google Scholar] [CrossRef]
- Bartolo, R.E.; Tveten, A.B.; Dandridge, A. Thermal phase noise measurements in optical fiber interferometers. IEEE J. Quantum Electron. 2012, 48, 720–727. [Google Scholar] [CrossRef]
- Kersey, A.D. A review of recent developments in fiber optic sensor technology. Opt. Fiber Technol. 1996, 2, 291–317. [Google Scholar] [CrossRef]
- Stolarik, M.; Kalab, Z. Jablunkov Rail Tunnels: Experimental Seismological Measurement. In Proceedings of the XIV Danube-European Conference on Geotechnical Engineering, Bratislava, Slovak Republic, 2–4 June 2010; p. 241. [Google Scholar]
- Stolarik, M.; Kalab, Z. Seismic experimental measurements in the near zone on IV. railway corridor tunnels. In Proceedings of the 5th International Conference on the Dynamics of Civil Engineering and Transport Structures and Wind Engineering, Zilina, Slovakia, 30 May–2 June 2011; pp. 67–70. [Google Scholar]
- Stolarik, M. Experimental Seismic Measurement in Tunnel—Influence of Sensor Locating on Quality of Rekord. Geotechnika 2011, 1–2, 34–37. [Google Scholar]
- Sushil, B. Engineering Rock Blasting Operation; A.A.Balkema: Rotterdam, The Netherlands, 1997; p. 375. [Google Scholar]
- CSN 73 0040—Loads of Technical Structures by Technical Seismicity; Czech Office for Standards, Metrology and Testing: Prague, Czech Republic, 1996. (In Czech)
- SN 640 312a—Erschütterungen einwirkungen auf Bauwerke; Schweizerische Normenvereinigung: Zurich, Switzerland, 1992; Available online: http://www.kgkissling.ch/dokumente/Erschuetterungsmessungen_Norm_SN_640_312a.pdf (accessed on 21 September 2019). (In German)
- DIN 4150—Erschütterungen im Bauwesen; DIN Standards Committee Printing and Graphic Technology: Berlin, Germany, 2016; Available online: https://www.din.de/en/getting-involved/standards-committees/ndr/standards/wdc-beuth:din21:262430160 (accessed on 21 September 2019). (In German)
- Pandula, B.; Kondela, J. Blasting Seismic Methodology. Slovenská společnost pre trhacie a vŕtacie práce—ZSVTS: Bratislava, Slovakia, 2010; p. 156. (In Slovak) [Google Scholar]
- Jayasinghe, B.; Zhao, Z.; Teck Chee, A.G.; Zhou, H.; Gui, Y. Attenuation of rock blasting induced ground vibration in rock-soil interface. J. Rock Mech. Geotech. Eng. 2019. [Google Scholar] [CrossRef]










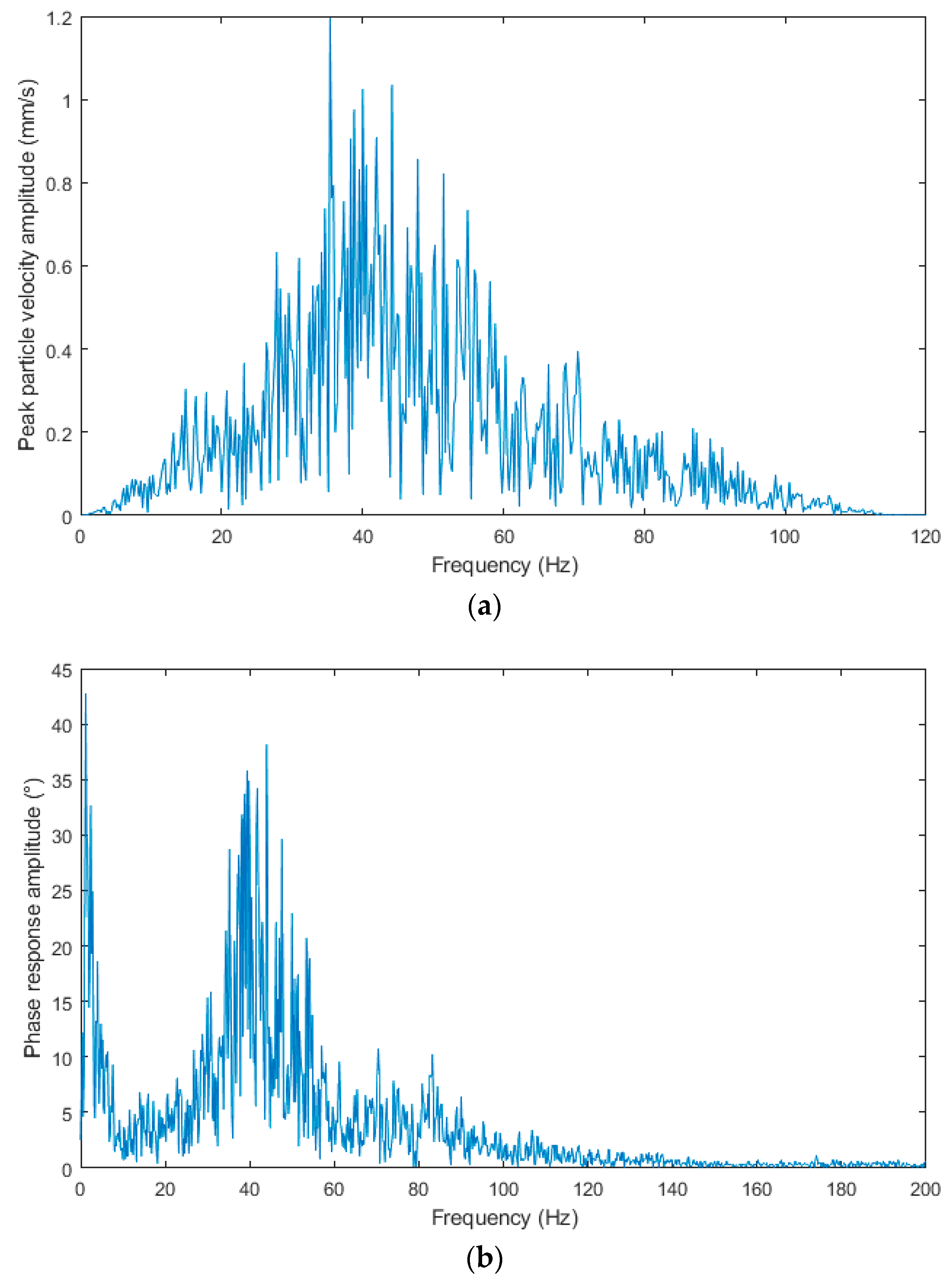

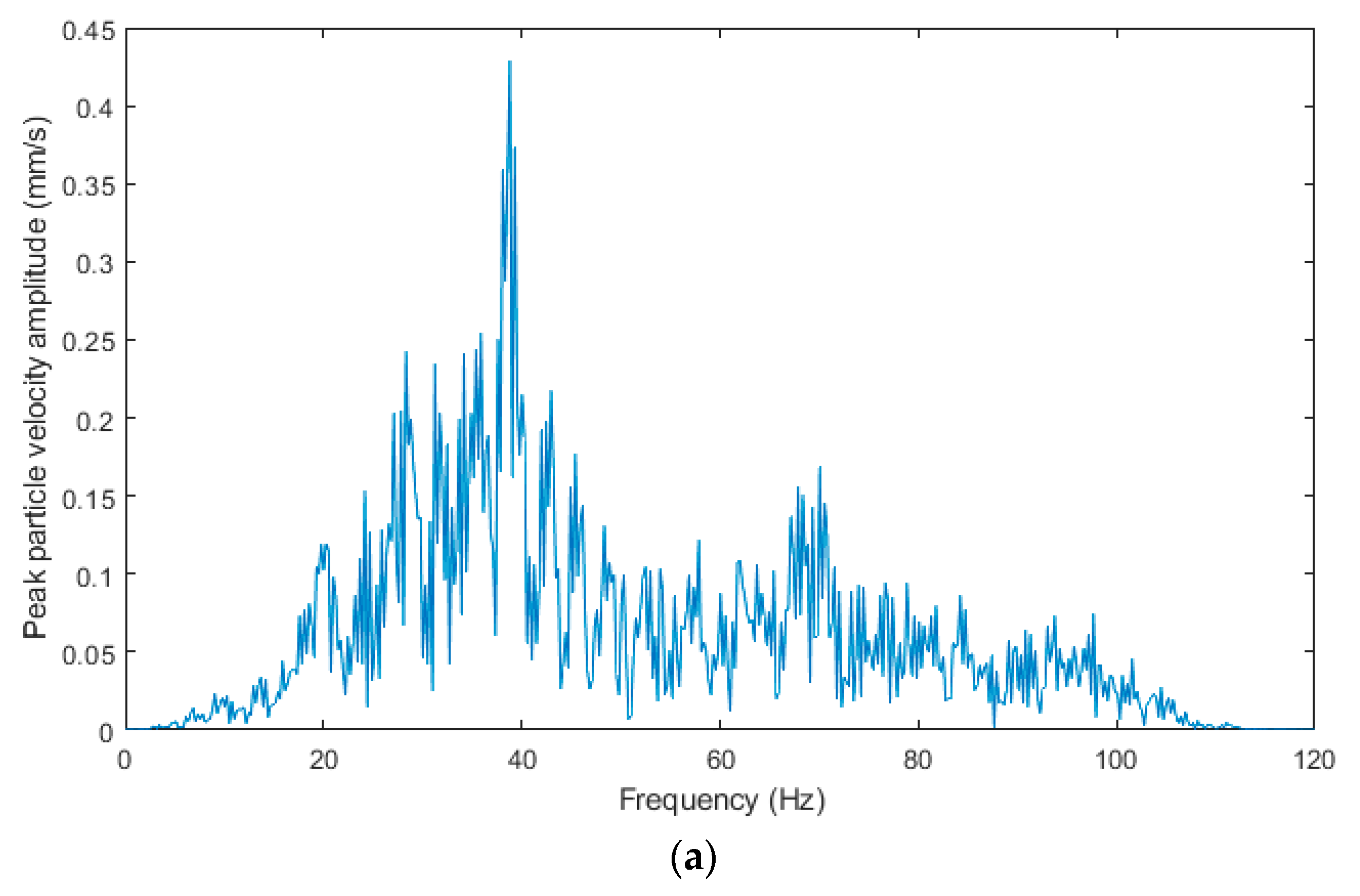
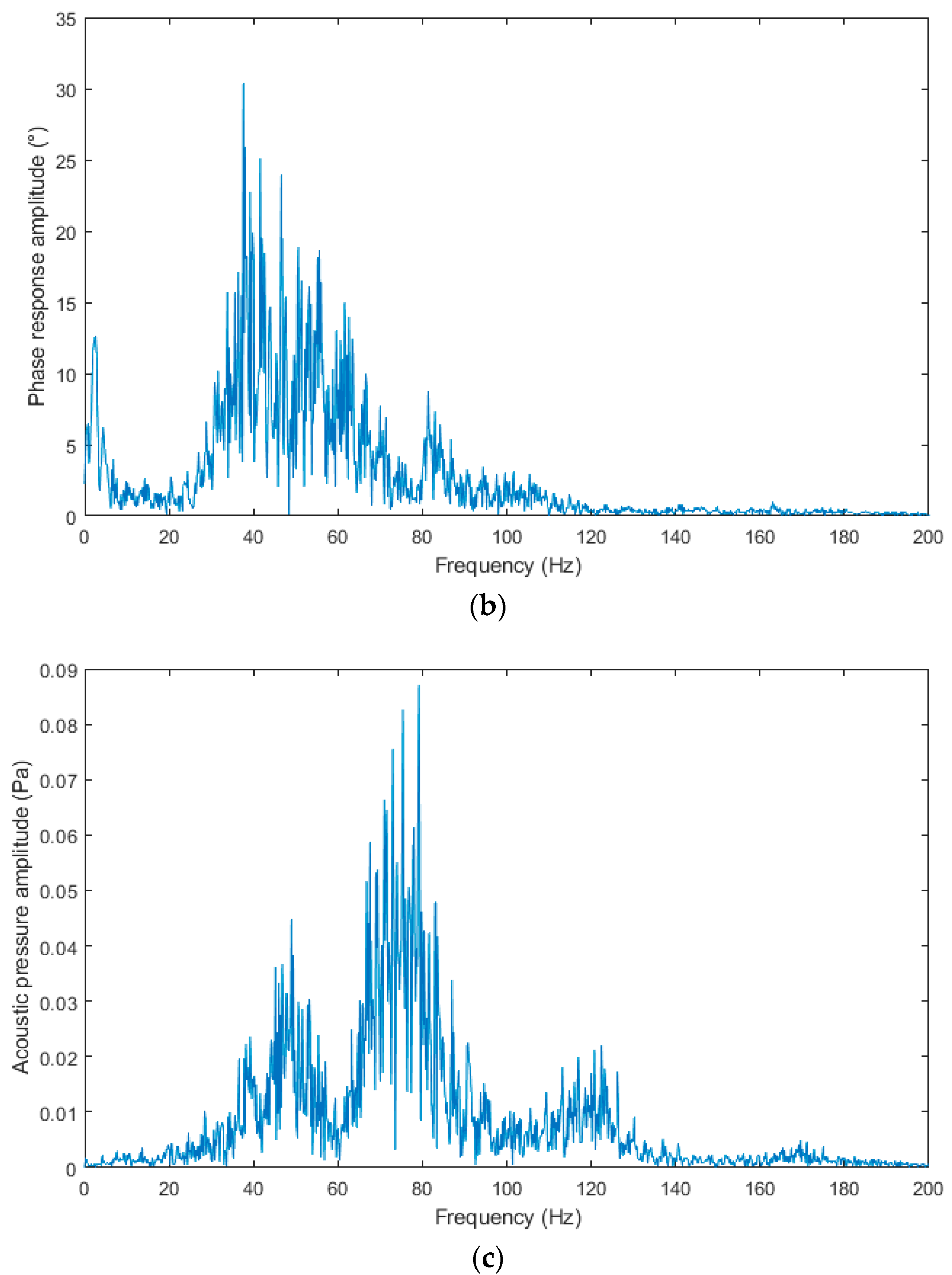
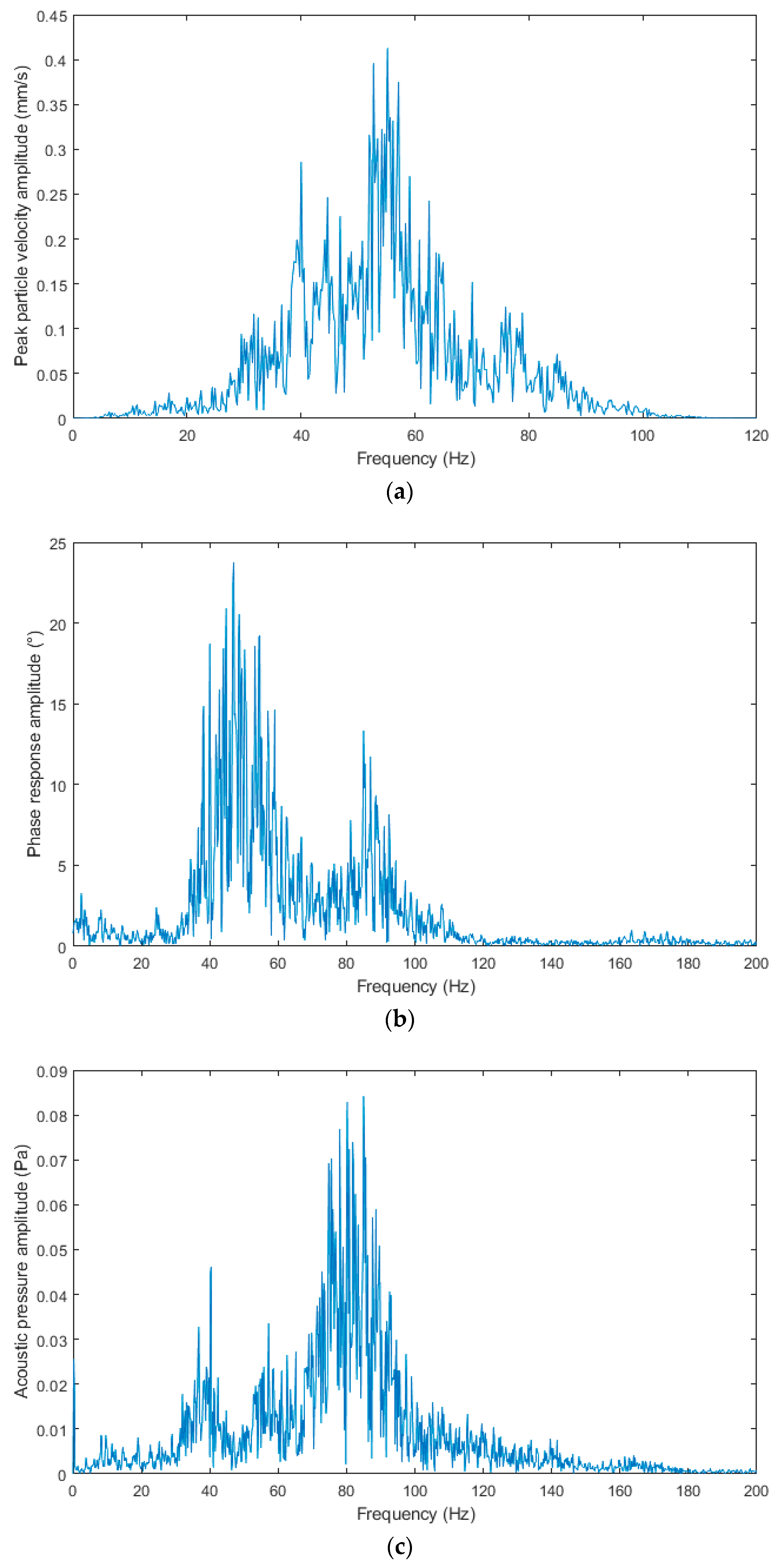
| Date | Bandwidth (Hz) | Dominant Component (Hz) | ||||
|---|---|---|---|---|---|---|
| BRS 32 | INTS | ACOS | BRS 32 | INTS | ACOS | |
| 11 March 2019 | 30–60 | 30–58 | 30–65 | 35 | 45 | 55 |
| 8 April 2019 | 39–75 | 30–70 | 30–80 | 48 | 41 | 75 |
| 26 April 2019 | 30–79 | 35–95 | 35–98 | 52 | 49 | 80 |
| Type of Sensor | Bandwidth (Hz) | Sampling Frequency (Hz) | Size (mm) | Weight (kg) | Price (USD) |
|---|---|---|---|---|---|
| BRS 32 | 4.5–100 | 125–500 | 270 × 245 × 130 | 4.6 | 3100 |
| INTS | 2–5000 | 10,000 | 500 × 500 × 350 | 8 | 1700 |
| ACOS | 20–18,000 | 5000–50,000 | 80 × 80 × 30 | 0.3 | 1500 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kepak, S.; Stolarik, M.; Nedoma, J.; Martinek, R.; Kolarik, J.; Pinka, M. Alternative Approaches to Vibration Measurement Due to the Blasting Operation: A Pilot Study. Sensors 2019, 19, 4084. https://doi.org/10.3390/s19194084
Kepak S, Stolarik M, Nedoma J, Martinek R, Kolarik J, Pinka M. Alternative Approaches to Vibration Measurement Due to the Blasting Operation: A Pilot Study. Sensors. 2019; 19(19):4084. https://doi.org/10.3390/s19194084
Chicago/Turabian StyleKepak, Stanislav, Martin Stolarik, Jan Nedoma, Radek Martinek, Jakub Kolarik, and Miroslav Pinka. 2019. "Alternative Approaches to Vibration Measurement Due to the Blasting Operation: A Pilot Study" Sensors 19, no. 19: 4084. https://doi.org/10.3390/s19194084
APA StyleKepak, S., Stolarik, M., Nedoma, J., Martinek, R., Kolarik, J., & Pinka, M. (2019). Alternative Approaches to Vibration Measurement Due to the Blasting Operation: A Pilot Study. Sensors, 19(19), 4084. https://doi.org/10.3390/s19194084






