Study on Strain Characterization and Failure Location of Rock Fracture Process Using Distributed Optical Fiber under Uniaxial Compression
Abstract
:1. Introduction
2. The Basic Principle of BOTDR Optical Fiber Testing Technology
3. Experimental Process of Uniaxial Compression
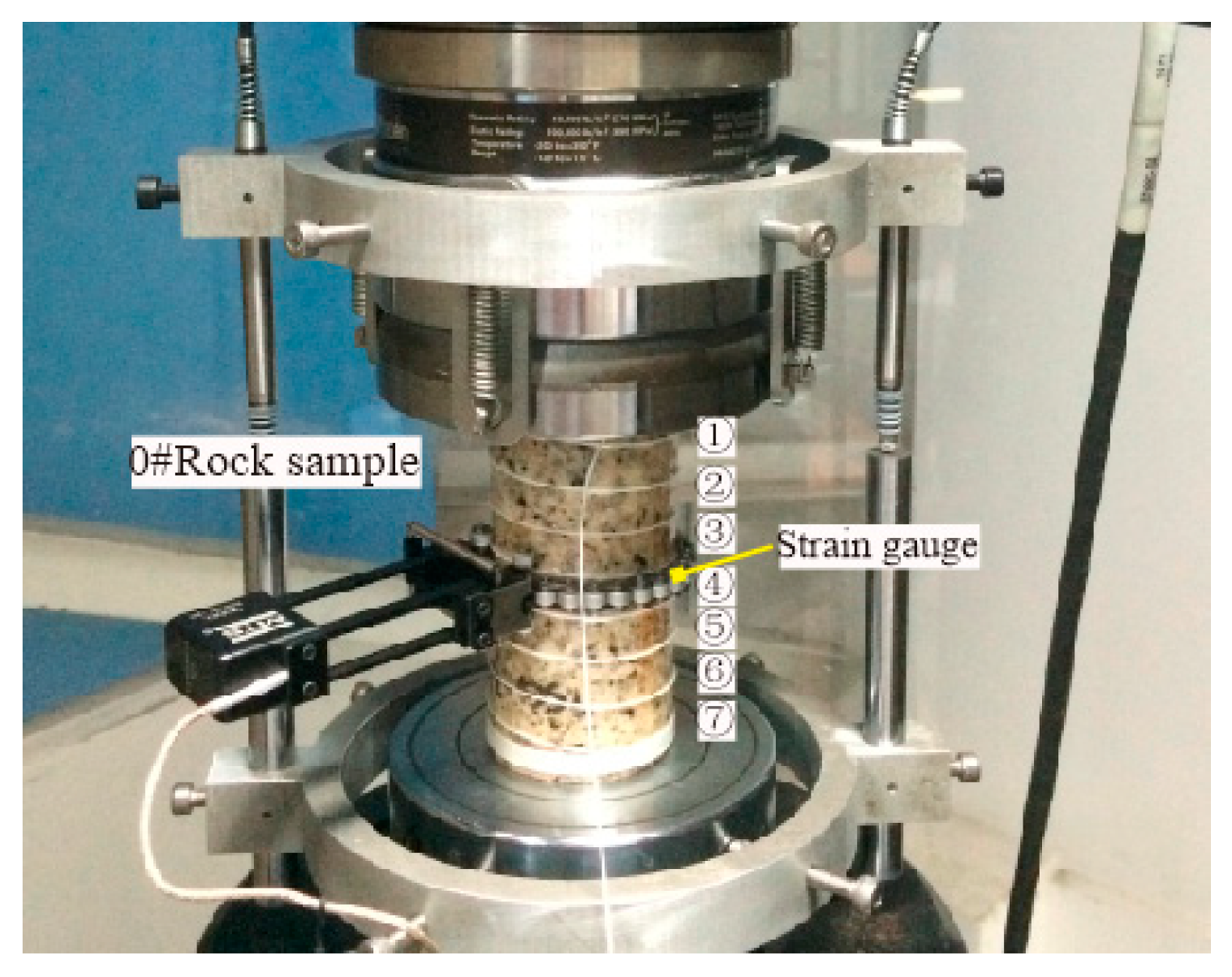
3.1. Sample Preparation
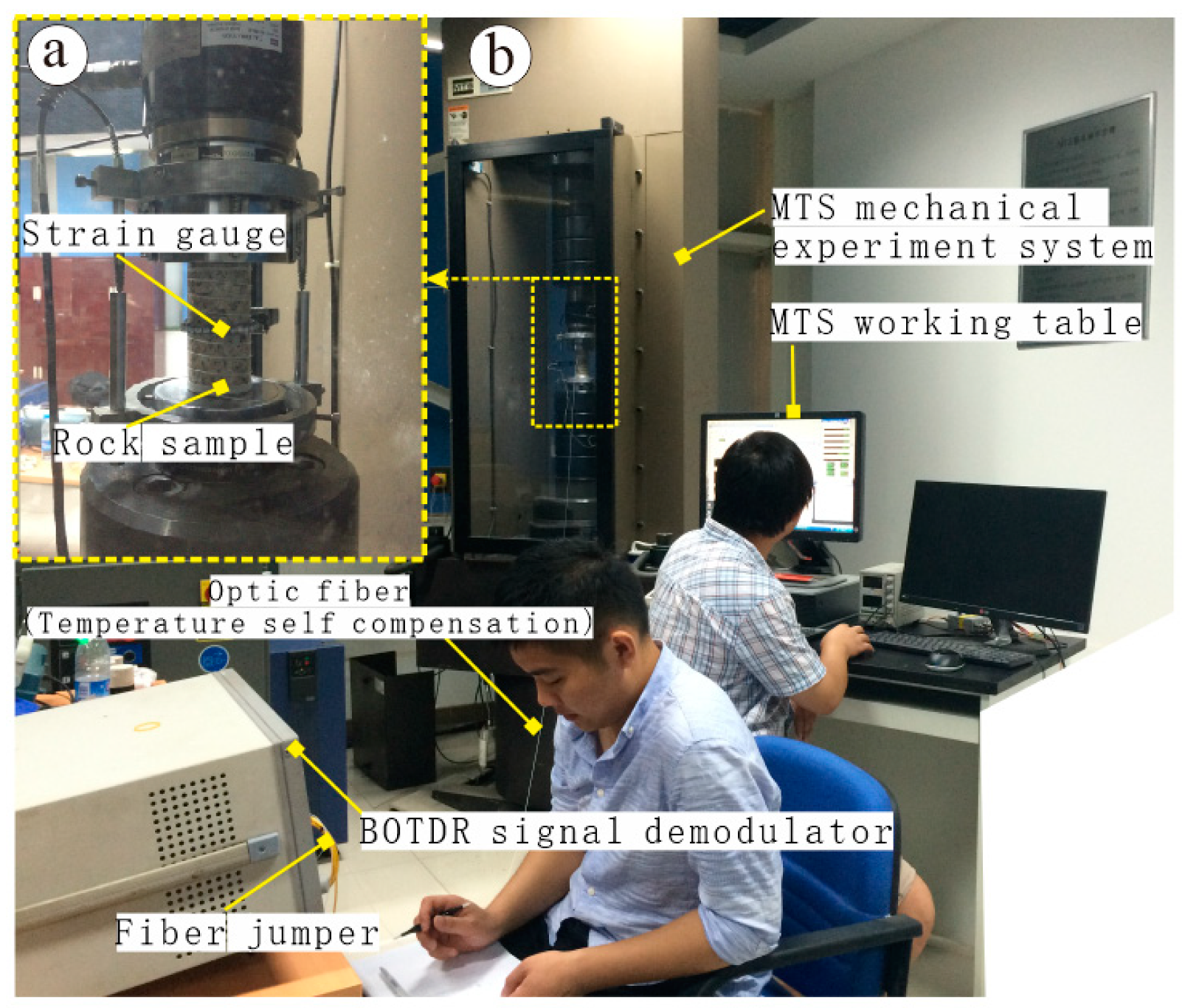
3.2. Testing Instrument
3.3. Arrangement of Optical Fiber Sensors
3.4. Arrangement of Optical Fiber Sensors
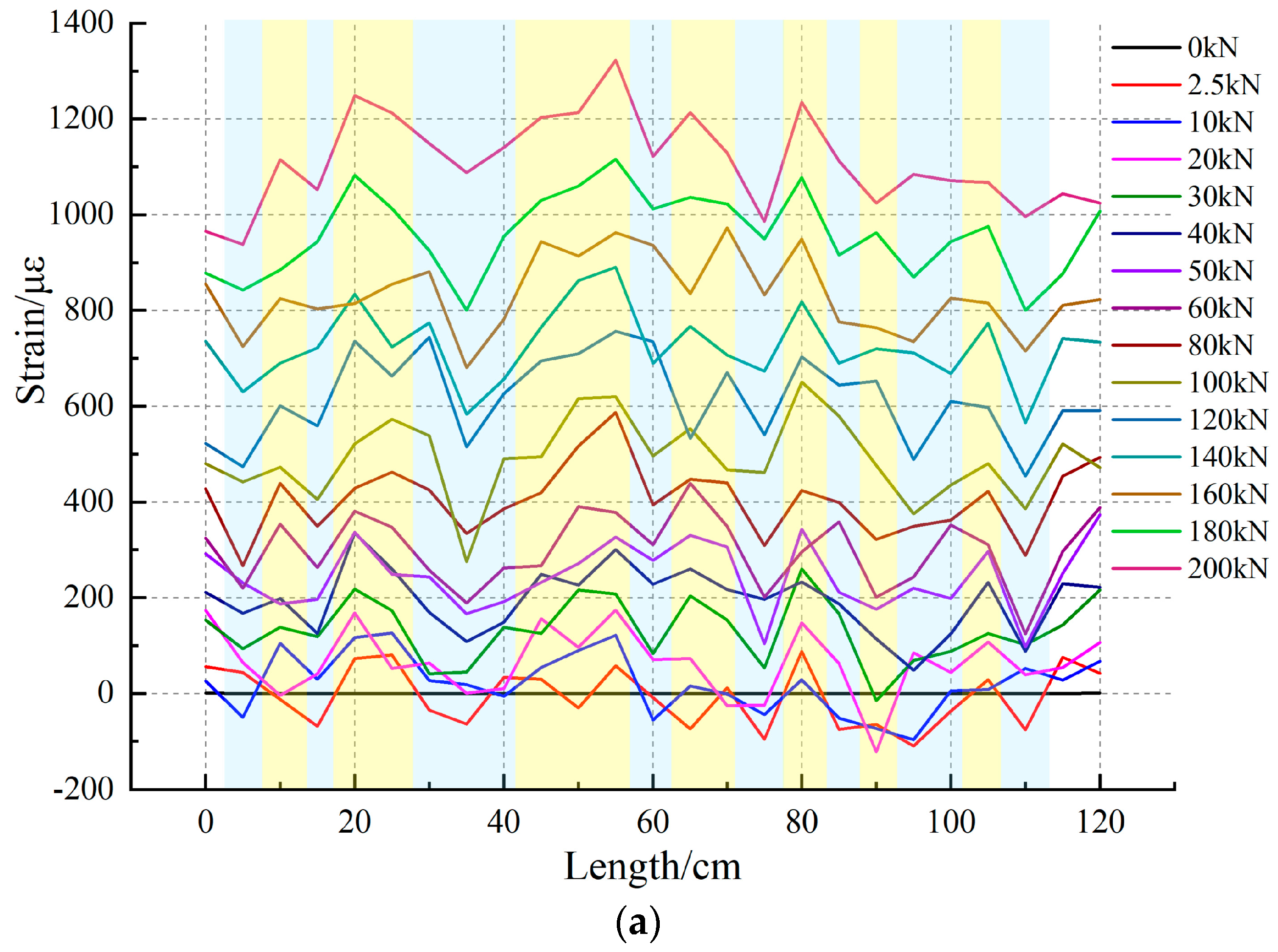
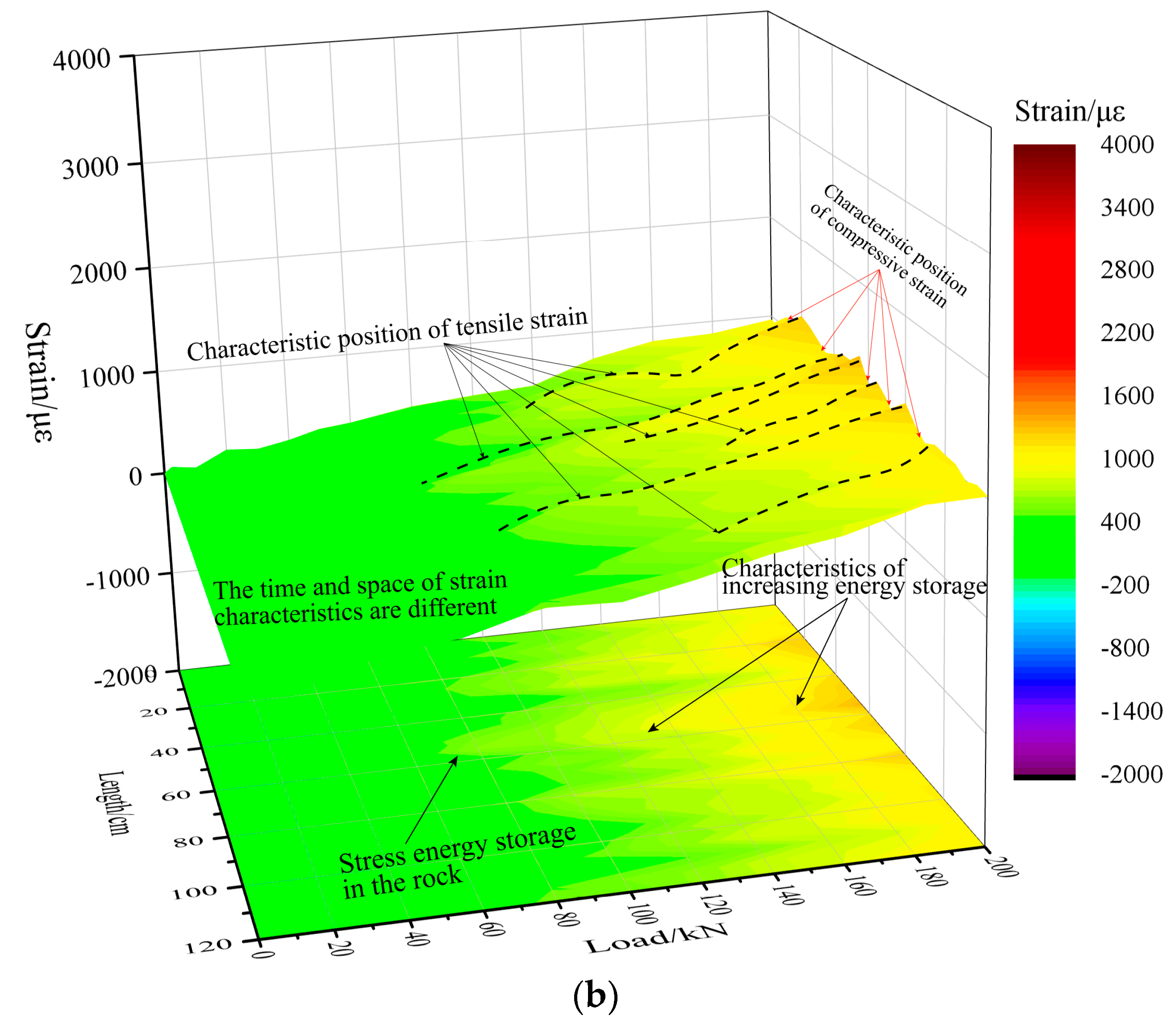
4. Test Results and Discussion
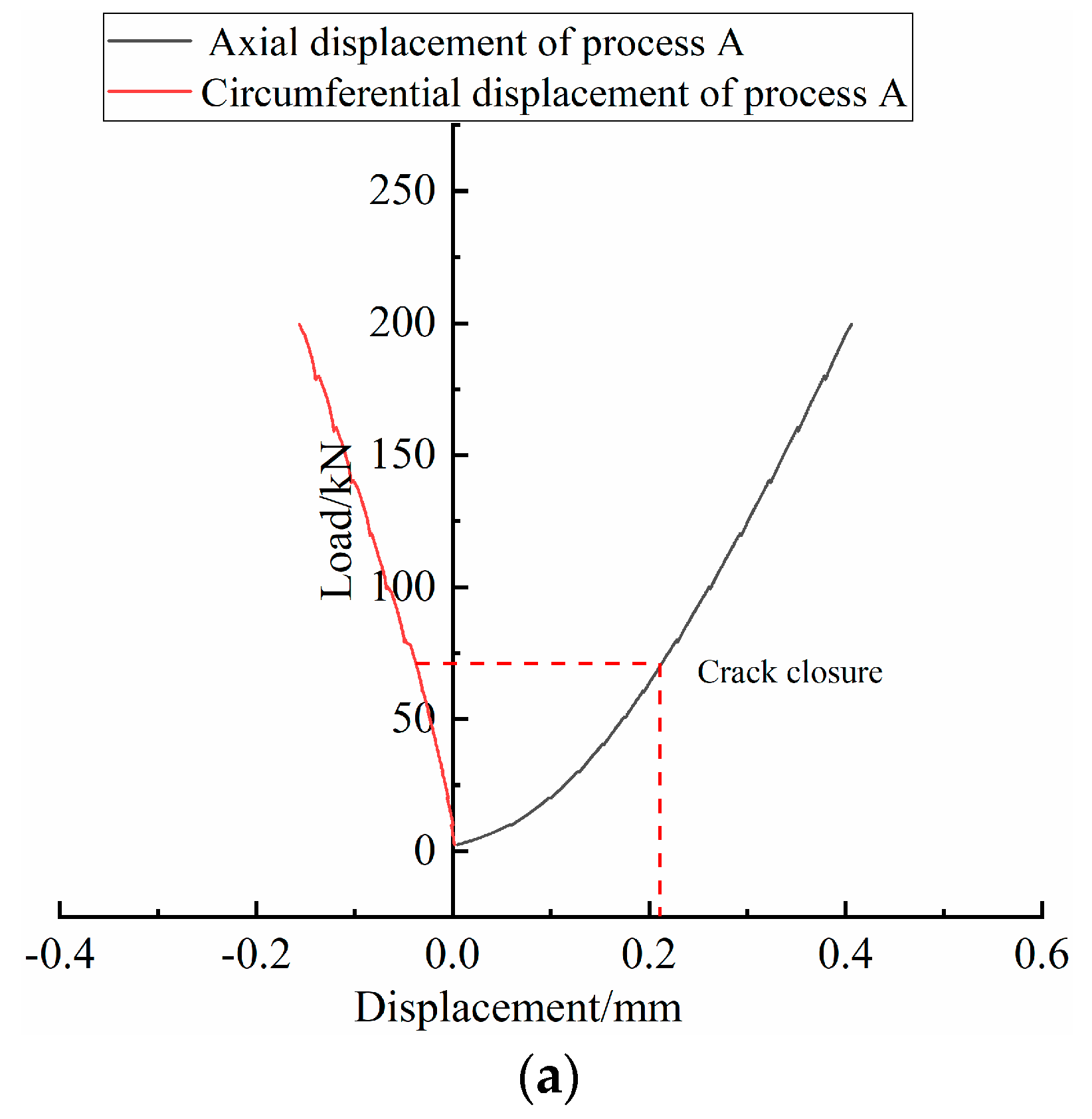
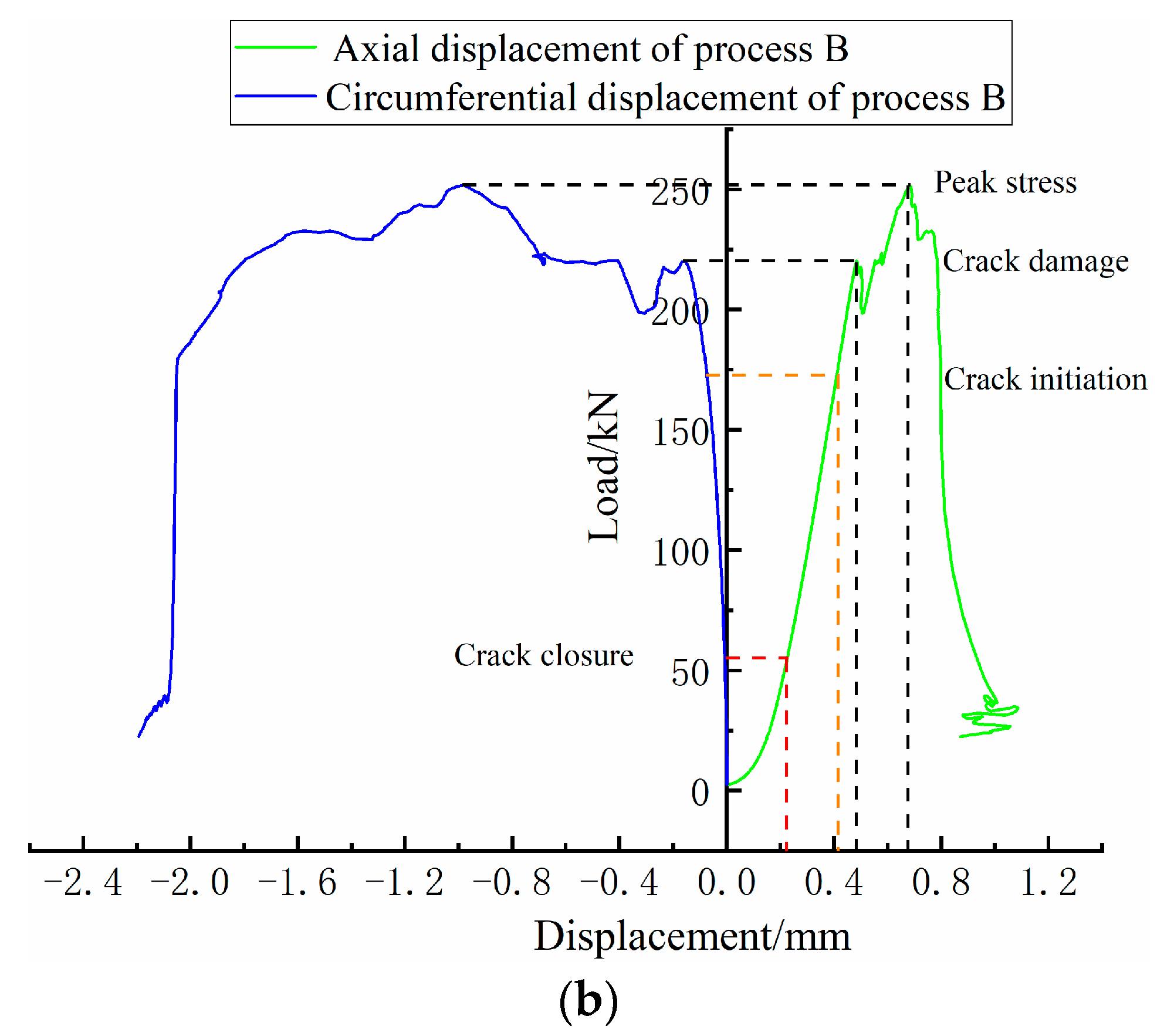
4.1. Analysis of The Deformation Process of the Rock Sample
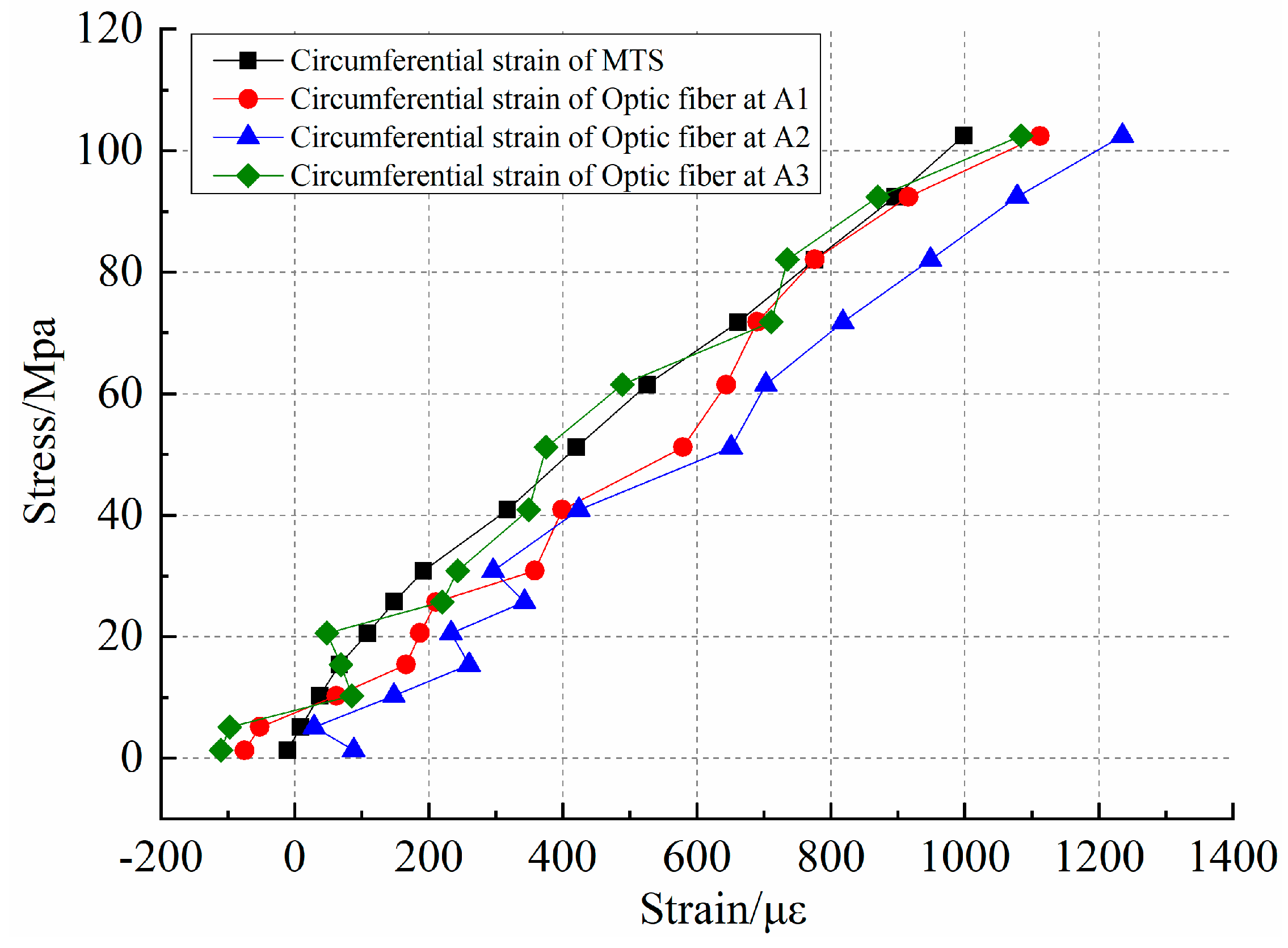
4.2. Optical Fiber Test Analysis of Process A
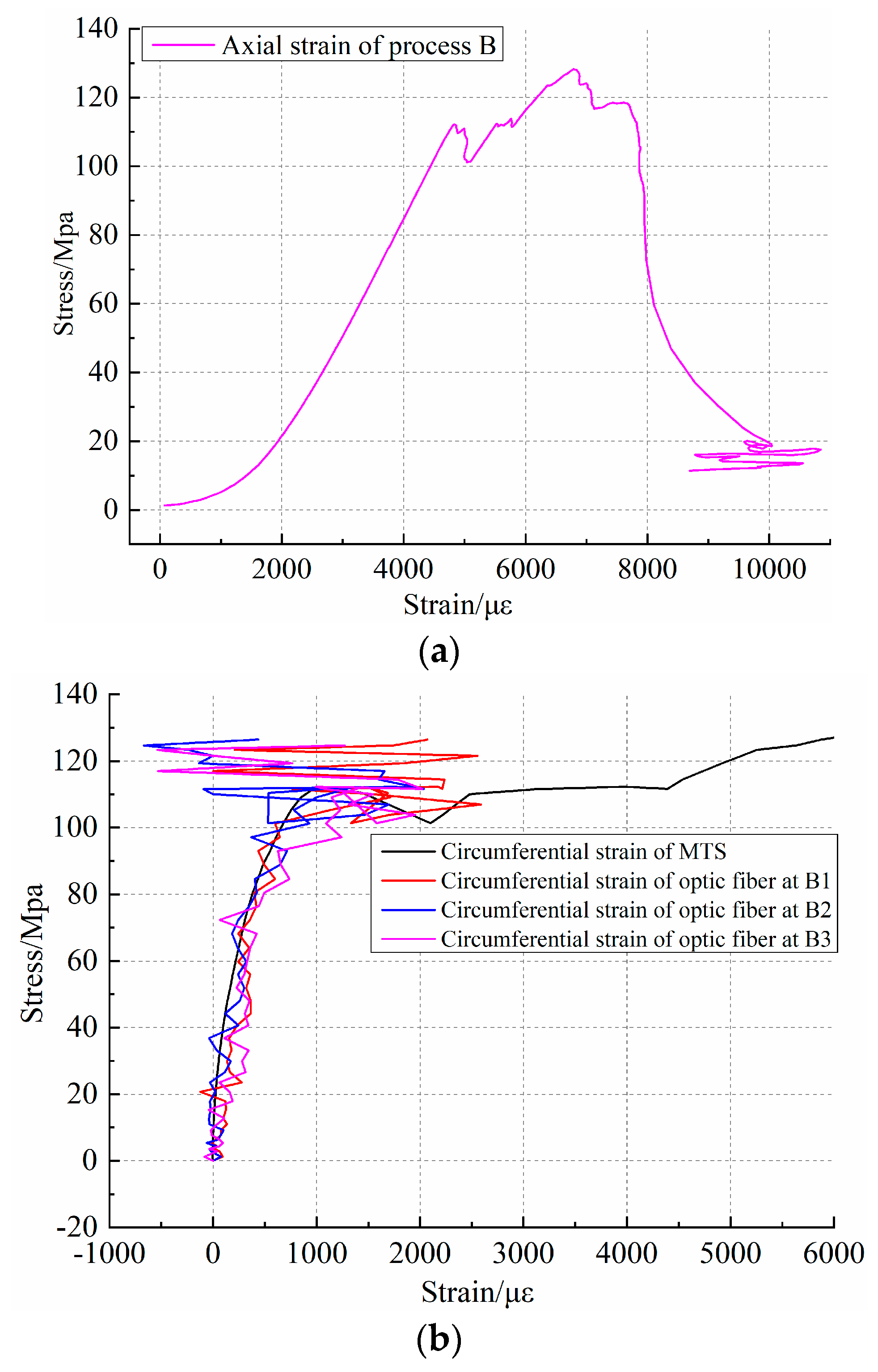
4.3. Optical Fiber Test Analysis of Process B
5. Conclusions
- (1)
- Distributed optical fiber is used to observe strain during the rock fracturing process under uniaxial compression. The optical fiber testing technology has good consistency with the MTS test results in terms of its circumferential strain response. During the testing process, the minimum relative error of local points in the circumferential position reaches 1.27%. BOTDR distributed optical fiber technology introduces a new testing method for rock deformation and failure observation and provides basic data for underground engineering applications.
- (2)
- Distributed optical fiber testing has a threshold value for rock fracture observation. Generally, rock failure occurs when a tensile strain reaches 600~800 με, which can be better reflected in the early and middle stages of the testing process. The process of micro-deformation of rock and surface strain before failure can be captured, and thus the precursor information of rock failure can be distinguished, and the disaster prevention and warning ability of the project can be improved. The test results of the No.0 and No.3 rock samples also indicate that the thresholds for optical fiber testing are different for different rocks.
- (3)
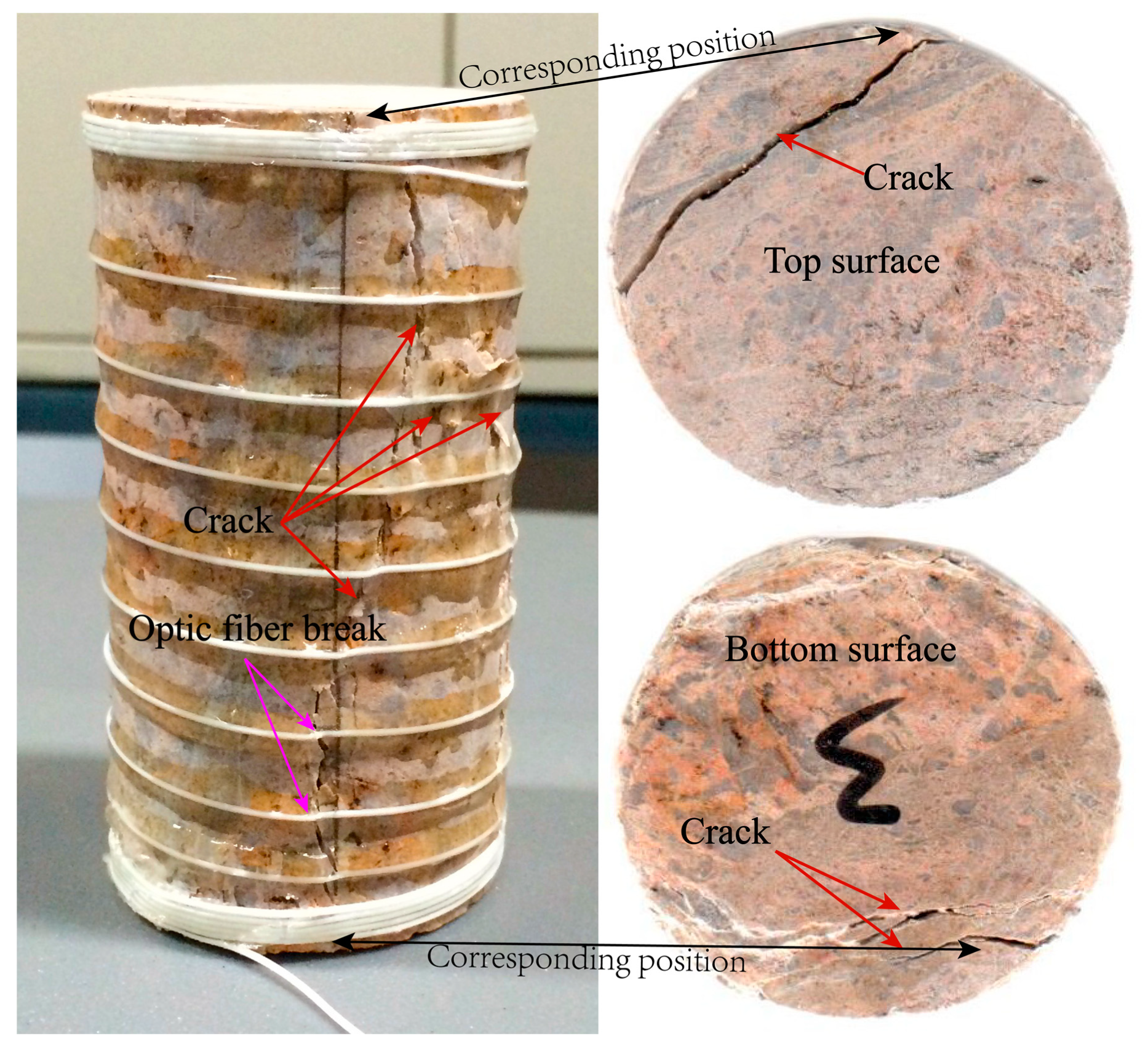
- Distributed optical fiber testing technology can identify the time of rock fracture development, reflect the tension and shear damage zone of surface strain before rock sample fracture, and delineate the location of failure in microscopical view. In this experiment, the morphological imaging of tissue is incomplete. In practical engineering applications, the space and time scales of rock and soil deformation are larger than those of the test. A reasonable scheme design and optical fiber arrangement are helpful for distributed optical fiber testing technology to capture the process of rock mass deformation and destruction and to analyze the evolution law of rock mass.
- (4)
- In this study, two groups of rock samples are used for fracturing tests; the comparison shows that the collection method of distributed optical fiber data, the mechanical properties of optical fiber, and the consolidation of the cementing agent will all have an impact on the test data. Improving the optical fiber performance and coupling treatment between the optical fiber and rock mass are key and these are difficult points that must be explored in the rock mass monitoring of distributed optical fiber testing technology in the future.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Deere, D.U. Technical description of rock cores for engineering purposes. Rock Mech. Eng. Geol. 1964, 1, 17–22. [Google Scholar]
- Priest, S.D.; Hudson, J.A. Discontinuity spacings in rock. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1976, 13, 135–148. [Google Scholar] [CrossRef]
- Dershowitz, W.S.; Einstein, H.H. Characterizing rock joint geometry with joint system model. Rock Mech. Rock Eng. 1988, 21, 21–51. [Google Scholar] [CrossRef]
- Huang, R.Q.; Dong, X.J. Application of three-dimensional laser scanning and surveying it geological investigation of high rock slope. J. China Univ. Geosci. 2008, 19, 184–190. [Google Scholar]
- Zhou, W.; Shi, X.; Lu, X.; Qi, C.; Luan, B.; Liu, F. The mechanical and microstructural properties of refuse mudstone-GGBS-red mud based geopolymer composites made with sand. Constr. Build. Mater. 2020, 253, 119193. [Google Scholar] [CrossRef]
- Dey, T.N.; Wang, C.Y. Some mechanisms of microcrack growth and Interaction in compressive rock failure. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1981, 18, 199–209. [Google Scholar] [CrossRef]
- Bobet, A. Modelling of crack initiation, propagation and coalescence in uniaxial compression. Rock Mech. Rock Eng. 2000, 33, 119–139. [Google Scholar] [CrossRef]
- Knill, J.L.; Franklin, J.A.; Malone, A.W. A study of acoustic emission from stressed rock. Int. J. Rock Mech. Min. Sci. 1968, 5, 87–121. [Google Scholar] [CrossRef]
- Zhao, Y.; Huang, J.; Wang, R. Real-time SEM observations of the microfracturing process in rock during a compression test. Int. J. Rock Mech. Min. Sci. Geomech. 1993, 30, 643–652. [Google Scholar] [CrossRef]
- Zhao, Y.; Huang, J.; Wang, R. Real-time observation of microfracturing process in rock during compression test. Chin. J. Rock Mech. Eng. 1992, 11, 284–294. [Google Scholar] [CrossRef]
- Liu, D.M.; Cai, M.F.; Zhou, Y.B.; Chen, Z.Y. Dynamic monitoring on developing process of rock cracks. Chin. J. Rock Mech. Eng. 2006, 25, 467–472. [Google Scholar] [CrossRef]
- Kawakata, H.; Cho, A.; Yanagidani, T.; Shimada, M. The observations of faulting in Westerly granite under triaxial compression by X Tay CT scan. Int. J Rock Mech. Min. Si. 1997, 34, 151–162. [Google Scholar] [CrossRef]
- Ge, X.R.; Ren, J.X.; Pu, Y.; Ma, W.; Zhu, Y. A real-in-time CT triaxial testing study of meso-damage evolution law of coal. Chin. J. Rock Mech. Eng. 1999, 18, 497–502. [Google Scholar] [CrossRef]
- Wei, S.M.; Chai, J. Strain transfer analysis of rock deformation based on FBG Sensing. J. Exp. Mech. 2010, 25, 445–450. [Google Scholar] [CrossRef]
- Cheng-Kai, F.; Yan-Kun, S.; Qi, L.; Hai-Feng, L.; Zhi-Yong, N.; Xia-Ying, L. Testing Technology of fiber Bragg grating in the shale damage experiments under uniaxial compression conditions. Rock Soil Mech. 2017, 38, 2456–2464. [Google Scholar]
- Munoz, H.; Taheri, A.; Chanda, E.K. Pre-Peak and Post-Peak Rock Strain Characteristics During Uniaxial Compression by 3D Digital Image Correlation. Rock Mech. Rock Eng. 2016, 49, 2541–2554. [Google Scholar] [CrossRef]
- Munoz, H.; Taheri, A. Local Damage and Progressive Localization in Porous Sandstone During Cyclic Loading. Rock Mech. Rock Eng. 2017, 50, 3253–3259. [Google Scholar] [CrossRef]
- Munoz, H.; Taheri, A. Specimen aspect ratio and progressive field strain development of sandstone under uniaxial compression by three-dimensional digital image correlation. J. Rock Mech. Geotech. Eng. 2020, 9, 599–610. [Google Scholar] [CrossRef]
- Munoz, H.; Taheri, A.; Chanda, E.K. Fracture Energy-Based Brittleness Index Development and Brittleness Quantification by Pre-peak Strength Parameters in Rock Uniaxial Compression. Rock Mech. Rock Eng. 2016, 49, 4587–4606. [Google Scholar] [CrossRef]
- Taheri, A.; Zhang, Y.; Munoz, H. Performance of rock crack stress thresholds determination criteria and investigating strength and confining pressure effects. Constr. Build Mater. 2020, 243, 118263. [Google Scholar] [CrossRef]
- He, T.M.; Liu, Z.Y.; Li, S.Y. Experimental Studies of Ultrasonic Tomography on Fangshan Granite Under Uniaxial Compression. CT Theory Appl. 2015, 24, 657–665. (In Chinese) [Google Scholar] [CrossRef]
- Yong-Shang, M.A.; Wei-Zhong, C.H.E.N.; Dian-Sen, Y.; Jian-Ping, Y.; Zhe, G. Experimental study of brittle rock failure based on three-dimensional digital image correlation technique. Rock Soil Mech. 2017, 38, 117–123. [Google Scholar] [CrossRef]
- Zhang, Y.B.; Wu, W.R.; Yao, X.L.; Liang, P.; Tian, B.Z.; Huang, Y.L.; Liang, J.L. Acoustic emission-infrared characteristics and damage evolution of granite under uniaxial compression. Rock Soil Mech. 2020, 41, 1–8. [Google Scholar] [CrossRef]
- Wu, Z.S.; Zhang, H.; Yang, C.Q. Development and performance evaluation of no-slippage optical fiber as Brillouin scattering based distributed sensors. Struct. Health Monit. 2010, 9, 413–431. [Google Scholar] [CrossRef]
- Shi, B.; Xu, H.Z.; Zhang, D.; Ding, Y.; Cui, H.L.; Gao, J.Q.; Chen, B. A Study on BOTDR application in monitoring deformation of a tunnel. In Proceedings of the First International Conference on Structural Health Monitoring and Intelligent Infrastructure, Tokyo, Japan, 13–15 November 2003; pp. 1025–1030. [Google Scholar]
- Liu, J.; Shi, B.; Zhang, D.; Sui, H.B.; Suo, W.B. The experimental study of foundation pit deformation distribution based on BOTDR. Rock Soil Mech. 2006, 265, 125–127. [Google Scholar] [CrossRef]
- Mendez, A.; Morse, T.F. Applications of embedded optical fiber sensors in reinforced concrete buildings and structures. In Proceedings of the SPIE-The International Society for Optical, Boston, MA, USA, 5–7 September 1989; pp. 60–67. [Google Scholar] [CrossRef]
- Fuhr, P.L.; Huston, D.R.; Nelson, M.; Nelson, O.; Hu, J.; Mowat, E.; Spammer, S.; Tamm, W. Fiber optic sensing of a bridge in Waterbury. Intell. Mater. Syst. Struct. 1999, 10, 293–303. [Google Scholar] [CrossRef]
- Inaudi, D.; del Grosso, A.E.; Lanata, F. Analysis of long-term deformation data from the San Giorgio harbor pier in Genoa. SPIE Proc. 2001, 4337, 459–465. [Google Scholar] [CrossRef]
- Zhang, D.; Zhang, P.S.; Shi, B.; Wang, H.X.; Li, C.S. Monitoring and analysis of overburden deformation and failure using distributed fiber’ optic sensing. Chin. J. Geotech. Eng. 2015, 37, 952–957. [Google Scholar] [CrossRef]
- Zhang, P.S.; Xu, S.A.; Guo, L.Q.; Wu, R.X. Prospect and progress of deformation and failure monitoring technology of surrounding rock in stope. Coal Sci. Technol. 2020, 48, 14–35. [Google Scholar] [CrossRef]
- Wei, S.M.; Chai, J. Study of application of optical fiber Bragg grating sensing to uniaxial compression experiments of rock. Rock Soil Mech. 2008, 29, 3174–3177. [Google Scholar] [CrossRef]
- Chai, J.; Du, W.; Yuan, Q.; Zhang, D. Analysis of test method for physical model test of mining based on optical fiber sensing technology detection. Opt. Fiber Technol. 2019, 48, 84–94. [Google Scholar] [CrossRef]
- Bao, X.; Zhou, D.P.; Baker, C.; Chen, L. Recent development in the distributed fiber optic acoustic and ultrasonic detection. J. Lightwave Technol. 2017, 35, 3256–3267. [Google Scholar] [CrossRef]
- Lanciano, C.; Salvini, R. Monitoring of Strain and Temperature in an Open Pit Using Brillouin Distributed Optical Fiber Sensors. Sensors 2020, 20, 1924. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, D.; Shi, B.; Cui, H.L.; Xu, H.Z. Improvement of spatial resolution of Brillouin optical time domain reflectometer using spectral decomposition. Opt. Appl. 2004, 34, 291–302. [Google Scholar]
- Zhang, D.; Shi, B.; Wu, Z.S.; Shen, S.; Zhang, Y. Distributed optical fiber sensor based on BOTDR and its application to structural health monitoring. China Civ. Eng. J. 2003, 36, 83–87. [Google Scholar] [CrossRef]
- Wang, X.; Shi, B.; Wei, G.Q.; Cheng, G.; Zgang, C. A Novel Technique for Civil and Geotechnical Engineering Monitoring: Performance and Characteristics of BOFDA. J. Dis. Prev. Mitig. Eng. 2015, 35, 763–768. [Google Scholar] [CrossRef]
- Dan, Z.; Bin, S.H.I.; Hong-Zhong, X.; Junqi, G.; Hong, Z. Experimental study on the deformation monitoring of reinforced concrete T-beam using BOTDR. J. Southeast Univ. (Nat. Sci. Ed.) 2004, 34, 480–484. [Google Scholar] [CrossRef]
- Horiguchi, T.; Kurashima, T.; Tateda, M. Tensile strain dependence of Brillouin frequency shift in silica optical fibers. IEEE Photonics Technol. Lett. 1989, 1, 107–108. [Google Scholar] [CrossRef]
- Fairhurst, C.E.; Hudson, J.A. Draft ISRM suggested method for the complete stress-strain curve for intact rock in uniaxial compression. Int. J. Rock Mech. Min. Sci. 1999, 36, 279–289. [Google Scholar] [CrossRef]
- Sun, Y.; Li, Q.; Yang, D.; Fan, C.; Sun, A. Investigation of the dynamic strain responses of sandstone using multichannel fiber-optic sensor arrays. Eng. Geol. 2016, 213, 1–10. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhang, Z.; Xue, S.; Wang, R.; Xiao, M. Stability analysis of a typical landslide mass in the Three Gorges Reservoir under varying reservoir water levels. Environ. Earth Sci. 2020, 79. [Google Scholar] [CrossRef]
- Zhu, H.H.; Shi, B.; Yan, J.F.; Zhang, J.; Wang, J. Investigation of the evolutionary process of a reinforced model slope using a fiber-optic monitoring network. Eng. Geol. 2015, 186, 34–43. [Google Scholar] [CrossRef]
- Zhang, L.F.; Yang, D.X.; Chen, Z.H.; Liu, A.C. Deformation and failure characteristics of sandstone under uniaxial compression using distributed fiber optic strain sensing. J. Rock Mech. Geotech. Eng. 2020, 12, 427–666. [Google Scholar] [CrossRef]




| Number | Collection Location | Diameter/mm | Length/mm |
|---|---|---|---|
| 0# | Wenchuan | 49.83 | 100.14 |
| 3# | Langfang | 49.75 | 100.23 |
| Load/kN | /με | /με | /% | |
|---|---|---|---|---|
| 2.5 | −96 | −10.9 | −85.1 | 783.17 |
| 10 | −14 | 8.6 | −22.6 | 262.19 |
| 20 | 25 | 36.7 | −11.7 | 31.96 |
| 30 | 53 | 66.8 | −13.8 | 20.71 |
| 40 | 196 | 108.7 | 87.3 | 80.36 |
| 50 | 104 | 149 | −45 | 30.18 |
| 60 | 201 | 191.9 | 9.1 | 4.74 |
| 80 | 309 | 317.1 | −8.1 | 2.55 |
| 100 | 461 | 419.7 | 41.3 | 9.84 |
| 120 | 540 | 525.7 | 14.3 | 2.73 |
| 140 | 673 | 661.8 | 11.2 | 1.69 |
| 160 | 832 | 775.9 | 56.1 | 7.23 |
| 180 | 949 | 895.8 | 53.2 | 5.94 |
| 200 | 986 | 998 | −12 | 1.21 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, S.; Wang, S.; Zhang, P.; Yang, D.; Sun, B. Study on Strain Characterization and Failure Location of Rock Fracture Process Using Distributed Optical Fiber under Uniaxial Compression. Sensors 2020, 20, 3853. https://doi.org/10.3390/s20143853
Xu S, Wang S, Zhang P, Yang D, Sun B. Study on Strain Characterization and Failure Location of Rock Fracture Process Using Distributed Optical Fiber under Uniaxial Compression. Sensors. 2020; 20(14):3853. https://doi.org/10.3390/s20143853
Chicago/Turabian StyleXu, Shiang, Shuangming Wang, Pingsong Zhang, Duoxing Yang, and Binyang Sun. 2020. "Study on Strain Characterization and Failure Location of Rock Fracture Process Using Distributed Optical Fiber under Uniaxial Compression" Sensors 20, no. 14: 3853. https://doi.org/10.3390/s20143853
APA StyleXu, S., Wang, S., Zhang, P., Yang, D., & Sun, B. (2020). Study on Strain Characterization and Failure Location of Rock Fracture Process Using Distributed Optical Fiber under Uniaxial Compression. Sensors, 20(14), 3853. https://doi.org/10.3390/s20143853





