Object Distance Estimation Using a Single Image Taken from a Moving Rolling Shutter Camera
Abstract
:1. Introduction
2. Distance Estimation Using Rolling Shutter Effect
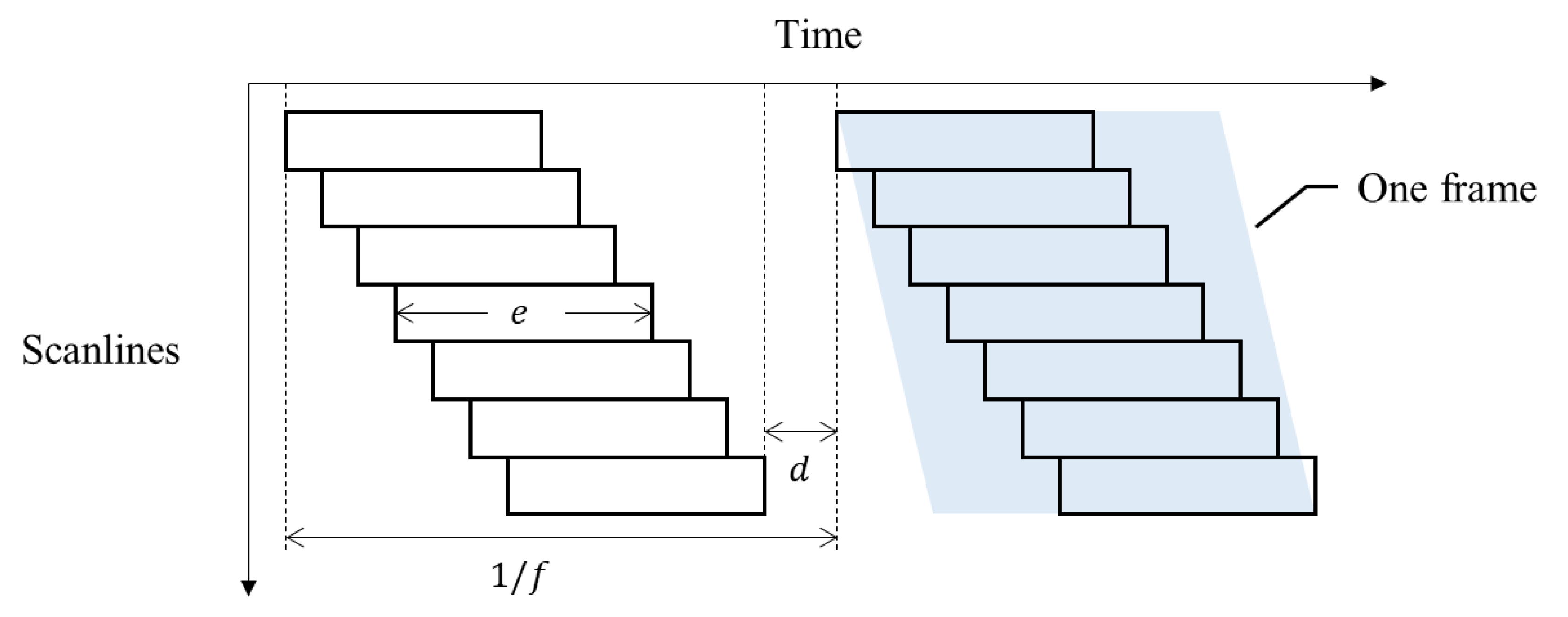
2.1. Rolling Shutter Camera and Rolling Shutter Effect (RSE)
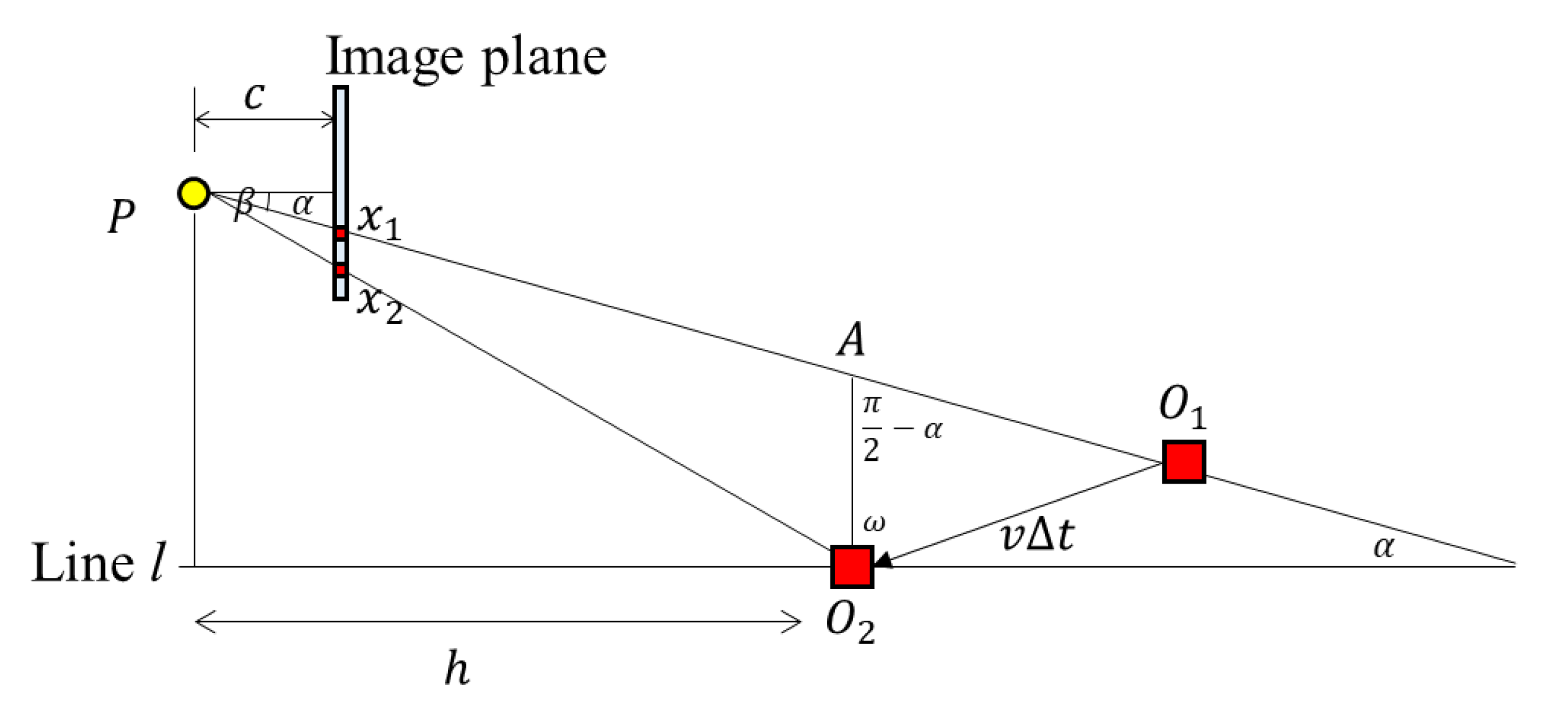
2.2. Derivation of Distance Estimation Equation Using the RSE
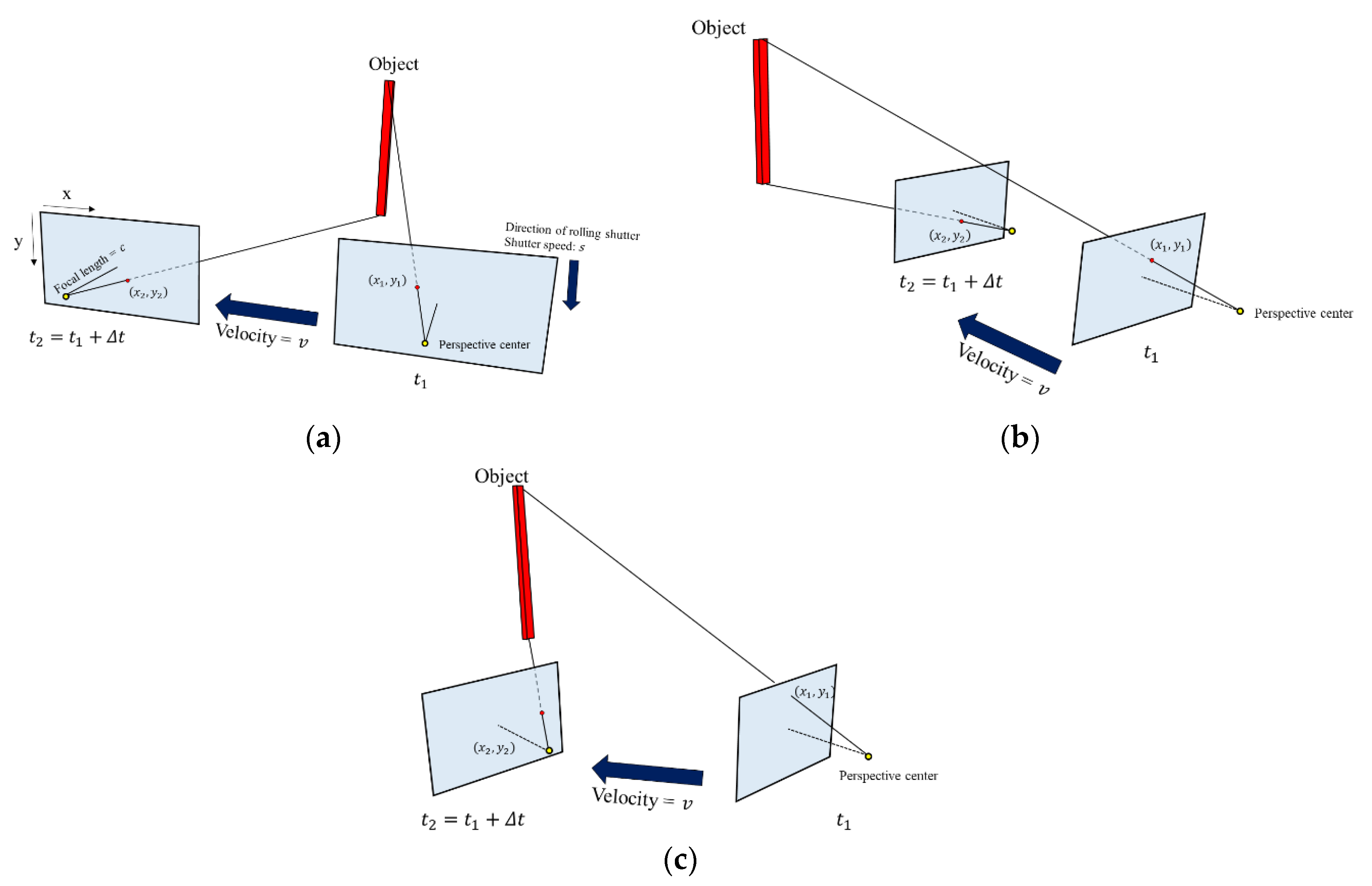
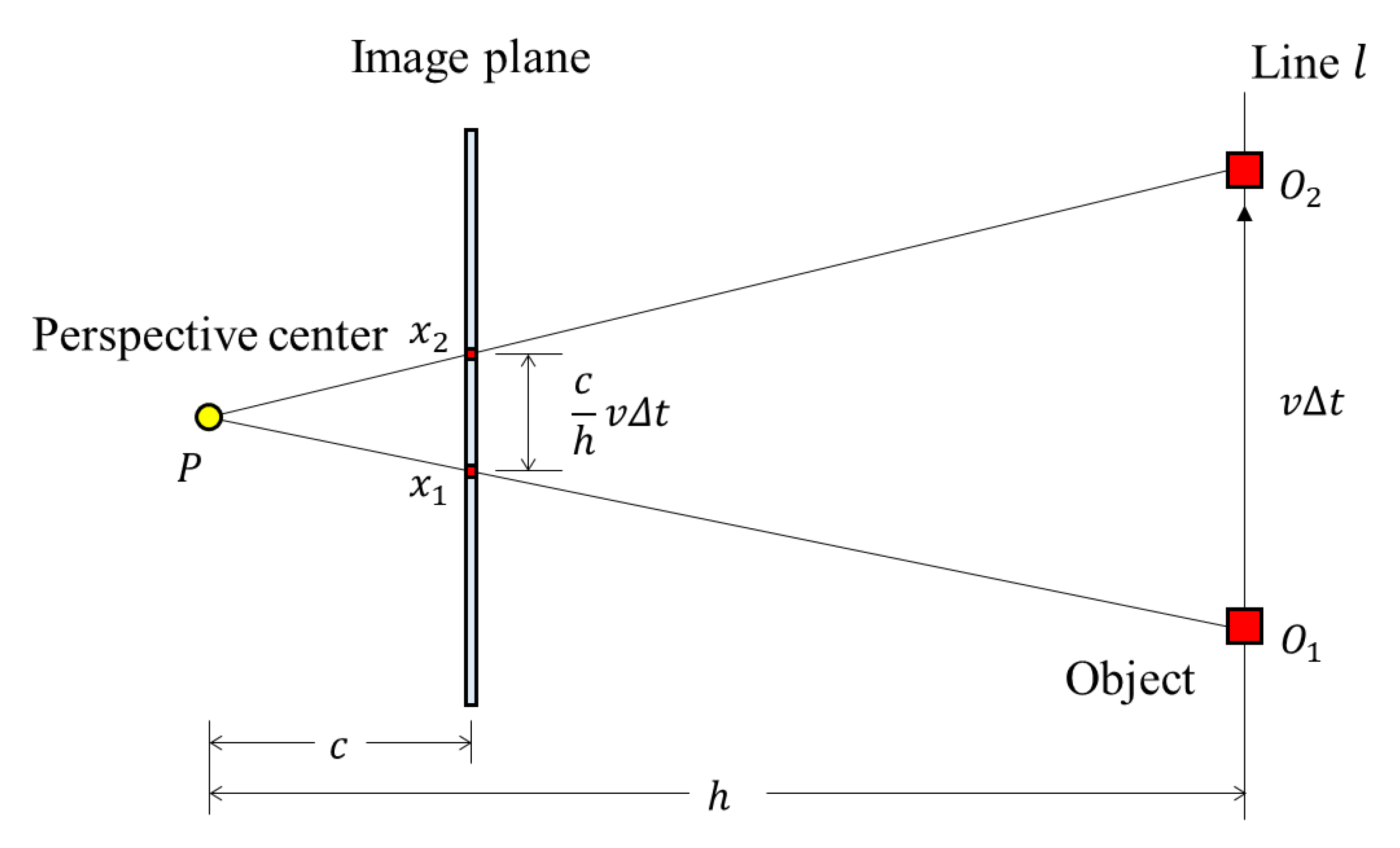
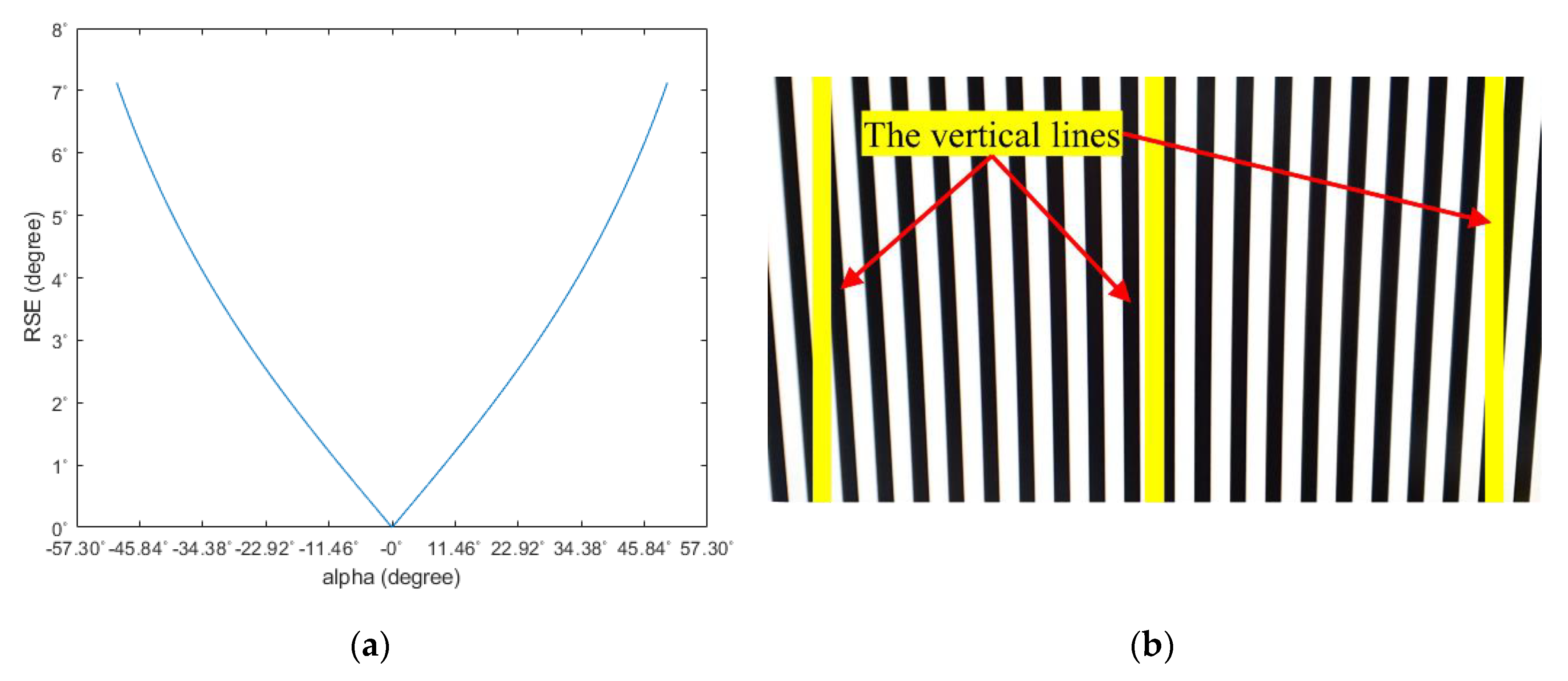
2.2.1. Derivation of the RSE Equation: The Movement of the Object Parallel to the Image Plane
2.2.2. Derivation of the RSE Equation: The Movement of the Object is Perpendicular to the Image Plane
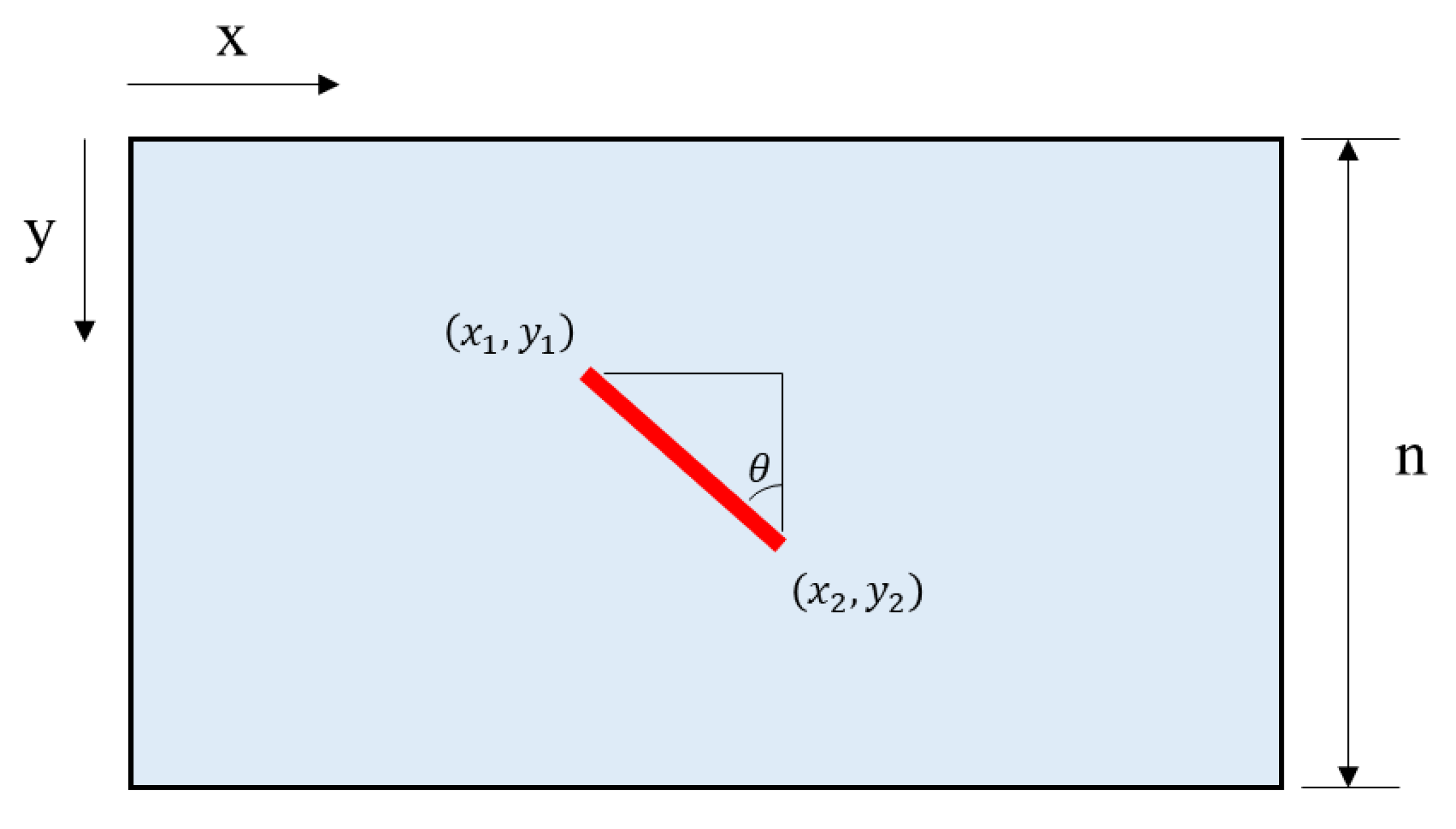
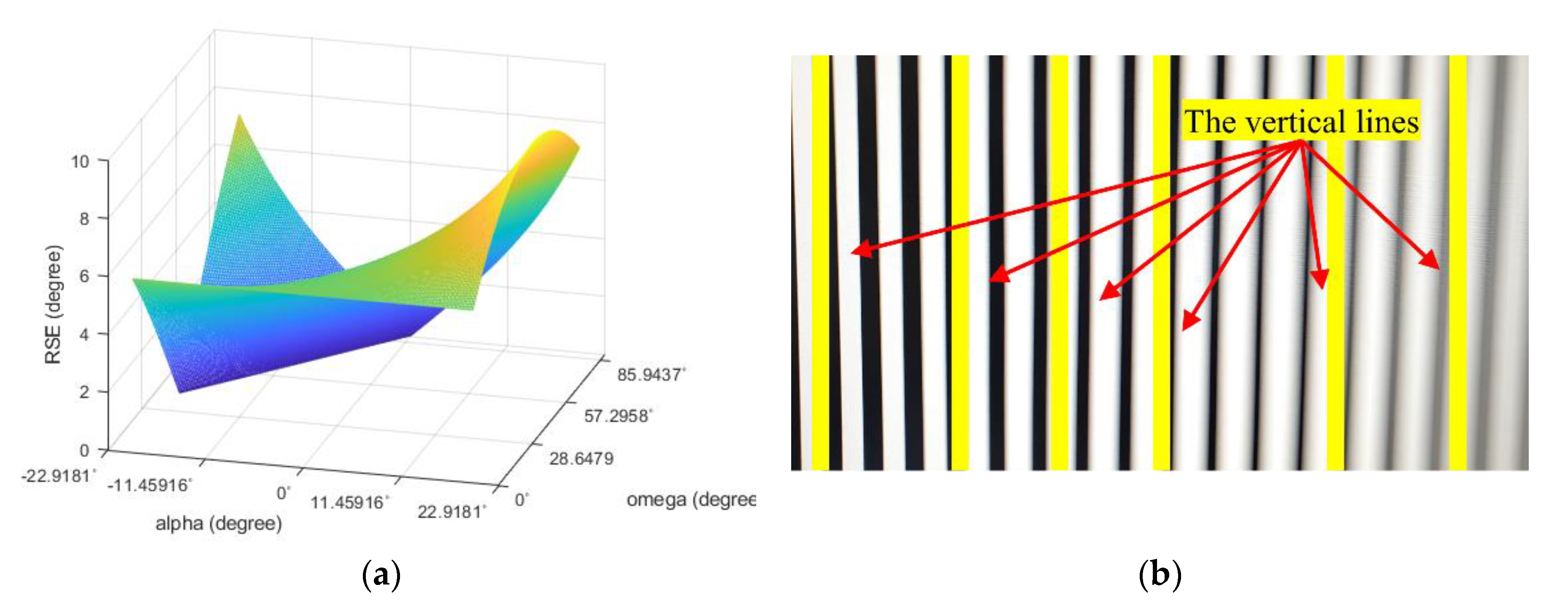
2.2.3. Derivation of the RSE Equation: The Movement of the Object has a Certain Angle to the Image Plane
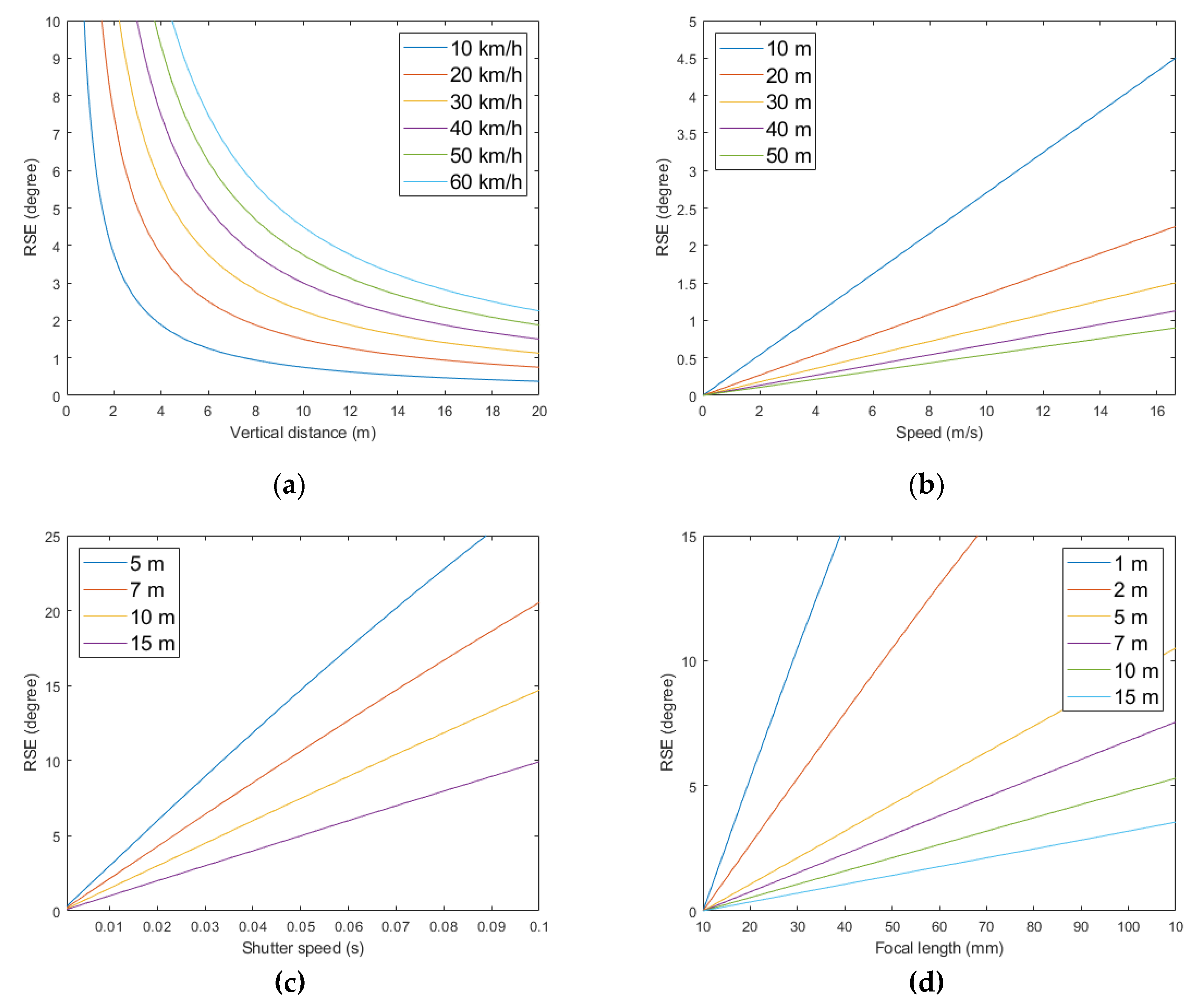
3. Experimental Result and Discussion
3.1. Sensors and Simulation Result
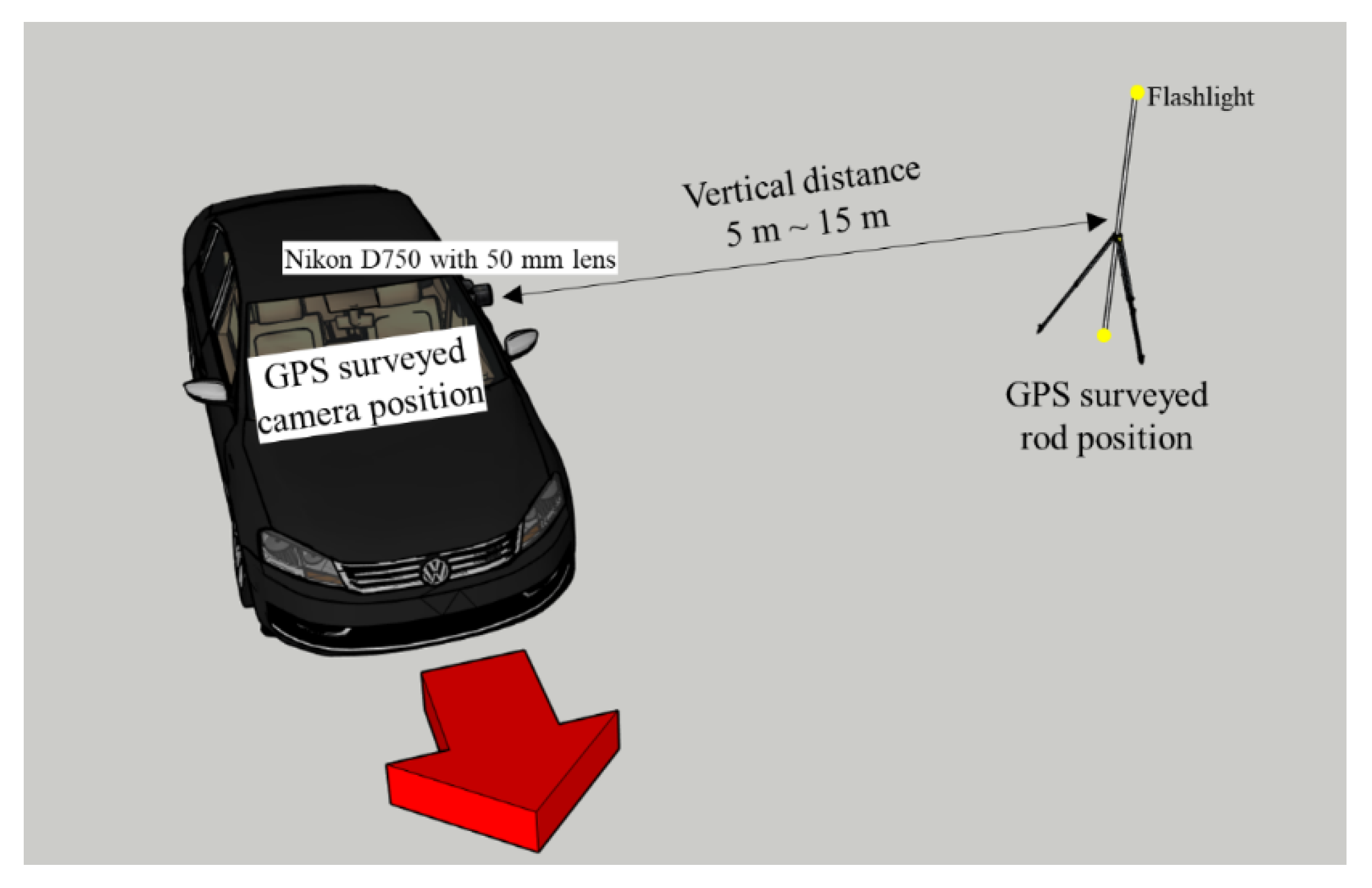
3.2. Field Test
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Forssen, P.-E.; Ringaby, E. Rectifying rolling shutter video from hand-held devices. In Proceedings of the 2010 IEEE Computer Society Conference on Computer Vision and Pattern Recognition, San Francisco, CA, USA, 13–18 June 2010; pp. 507–514. [Google Scholar] [CrossRef]
- Meingast, M.; Geyer, C.; Sastry, S. Geometric models of rolling-shutter cameras. arXiv 2005, arXiv:cs/0503076. Available online: https://arxiv.org/abs/cs/0503076 (accessed on 8 July 2020).
- Yoon, H.; Hoskere, V.; Park, J.-W.; Spencer, B.F. Cross-correlation-based structural system identification using unmanned aerial vehicles. Sensors 2017, 17, 2075. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kerl, C.; Stuckler, J.; Cremers, D. Dense continuous-time tracking and mapping with rolling shutter RGB-D cameras. In Proceedings of the IEEE International Conference on Computer Vision, Santiago, Chile, 7–13 December 2015; pp. 2264–2272. [Google Scholar] [CrossRef]
- Lovegrove, S.; Patron-Perez, A.; Sibley, G. Spline fusion: A continuous-time representation for visual-inertial fusion with application to rolling shutter cameras. In Proceedings of the BMVC, Bristol, UK, 9–13 September 2013; Volume 2, p. 8. [Google Scholar] [CrossRef] [Green Version]
- Ait-Aider, O.; Andreff, N.; Lavest, J.M.; Martinet, P. Simultaneous object pose and velocity computation using a single view from a rolling shutter camera. In European Conference on Computer Vision; Springer: Cham, Switzerland, 2006; pp. 56–68. [Google Scholar] [CrossRef]
- Ait-Aider, O.; Berry, F. Structure and kinematics triangulation with a rolling shutter stereo rig. In Proceedings of the 2009 IEEE 12th International Conference on Computer Vision, Kyoto, Japan, 29 September–2 October 2009; pp. 1835–1840. [Google Scholar] [CrossRef]
- Hedborg, J.; Forssen, P.-E.; Felsberg, M.; Ringaby, E. Rolling shutter bundle adjustment. In Proceedings of the 2012 IEEE Conference on Computer Vision and Pattern Recognition, Providence, RI, USA, 16–21 June 2012; pp. 1434–1441. [Google Scholar] [CrossRef] [Green Version]
- Saurer, O.; Koser, K.; Bouguet, J.-Y.; Pollefeys, M. Rolling shutter stereo. In Proceedings of the 2013 IEEE International Conference on Computer Vision, Sydney, NSW, Australia, 1–8 December 2013; pp. 465–472. [Google Scholar] [CrossRef] [Green Version]
- Kim, J.-H.; Cadena, C.; Reid, I. Direct semi-dense SLAM for rolling shutter cameras. In Proceedings of the 2016 IEEE International Conference on Robotics and Automation (ICRA), Stockholm, Sweden, 16–21 May 2016; pp. 1308–1315. [Google Scholar] [CrossRef]
- Vautherin, J.; Rutishauser, S.; Schneider-Zapp, K.; Choi, H.F.; Chovancova, V.; Glass, A.; Strecha, C. Photogrammetric accuracy and modeling of rolling shutter cameras. ISPRS Ann. Photogramm. Remote Sens. Spat. Inf. Sci. 2016, 3, 139–146. [Google Scholar] [CrossRef]
- Zhou, Y.; Daakir, M.; Rupnik, E.; Pierrot-Deseilligny, M. A two-step approach for the correction of rolling shutter distortion in UAV photogrammetry. ISPRS J. Photogramm. Remote Sens. 2020, 160, 51–66. [Google Scholar] [CrossRef]
- Wang, K.; Fan, B.; Dai, Y. Relative pose estimation for stereo rolling shutter cameras. arXiv 2020, arXiv:2006.07807. Available online: https://arxiv.org/abs/2006.07807 (accessed on 8 July 2020).
- Albl, C.; Kukelova, Z.; Larsson, V.; Pajdla, T. Rolling shutter camera absolute pose. IEEE Trans. Pattern Anal. Mach. Intell. 2019, 42, 1439–1452. [Google Scholar] [CrossRef]
- Kukelova, Z.; Albl, C.; Sugimoto, A.; Schindler, K.; Pajdla, T. Minimal rolling shutter absolute pose with unknown focal length and radial distortion. arXiv 2020, arXiv:2004.14052. Available online: https://arxiv.org/abs/2004.14052 (accessed on 8 July 2020).
- Schubert, D.; Demmel, N.; von Stumberg, L.; Usenko, V.; Cremers, D. Rolling-shutter modelling for direct visual-inertial odometry. arXiv 2019, arXiv:1911.01015. Available online: https://arxiv.org/abs/1911.01015 (accessed on 8 July 2020).
- Taketomi, T.; Uchiyama, H.; Ikeda, S. Visual SLAM algorithms: A survey from 2010 to 2016. IPSJ Trans. Comput. Vis. Appl. 2017, 9, 16. [Google Scholar] [CrossRef]
- Oth, L.; Furgale, P.; Kneip, L.; Siegwart, R. Rolling shutter camera calibration. In Proceedings of the 2013 IEEE Conference on Computer Vision and Pattern Recognition, Portland, OR, USA, 23–28 June 2013; pp. 1360–1367. [Google Scholar] [CrossRef] [Green Version]
- Wan, L.; Zhang, Y.; Jia, P.; Xu, J. Modeling and rectification of rolling shutter effect in CMOS aerial cameras. J. Harbin Inst. Technol. 2017, 24, 71–77. [Google Scholar] [CrossRef]
- Ringaby, E.; Forssén, P.-E. Efficient video rectification and stabilization for cellphones. Int. J. Comput. Vis. 2012, 96, 335–352. [Google Scholar] [CrossRef] [Green Version]
- Lee, C.-R.; Yoon, J.H.; Yoon, K.-J. Calibration and noise identification of a rolling shutter camera and a low-cost inertial measurement unit. Sensors 2018, 18, 2345. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chen, T.; Zhu, Z.; Shamir, A.; Hu, S.-M.; Cohen-Or, D. 3-Sweep. ACM Trans. Graph. 2013, 32, 1–10. [Google Scholar] [CrossRef]
- Kholgade, N.; Simon, T.; Efros, A.; Sheikh, Y. 3D object manipulation in a single photograph using stock 3D models. ACM Trans. Graph. 2014, 33, 1–12. [Google Scholar] [CrossRef]
- Huang, Q.; Wang, H.; Koltun, V. Single-view reconstruction via joint analysis of image and shape collections. ACM Trans. Graph. 2015, 34, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Liang, C.-K.; Chang, L.-W.; Chen, H.H. Analysis and compensation of rolling shutter effect. IEEE Trans. Image Process. 2008, 17, 1323–1330. [Google Scholar] [CrossRef] [PubMed]
- Patel, S.; Deb, K. Study of Active earth pressure behind a vertical retaining wall subjected to rotation about the base. Int. J. Geomech. 2020, 20. [Google Scholar] [CrossRef]
- Duane, C.B. Close-range camera calibration. Photogramm. Eng. 1971, 37, 855–866. [Google Scholar]
- Konolige, K.; Augenbraun, J.; Donaldson, N.; Fiebig, C.; Shah, P. A low-cost laser distance sensor. In Proceedings of the 2008 IEEE International Conference on Robotics and Automation, Pasadena, CA, USA, 19–23 May 2008; pp. 3002–3008. [Google Scholar] [CrossRef]
- Chen, X.; Zhao, M.; Xiang, L.; Sugai, F.; Yaguchi, H.; Okada, K.; Inaba, M. Development of a low-cost ultra-tiny line laser range sensor. In Proceedings of the 2016 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Daejeon, Korea, 9–14 October 2016; pp. 111–116. [Google Scholar] [CrossRef]

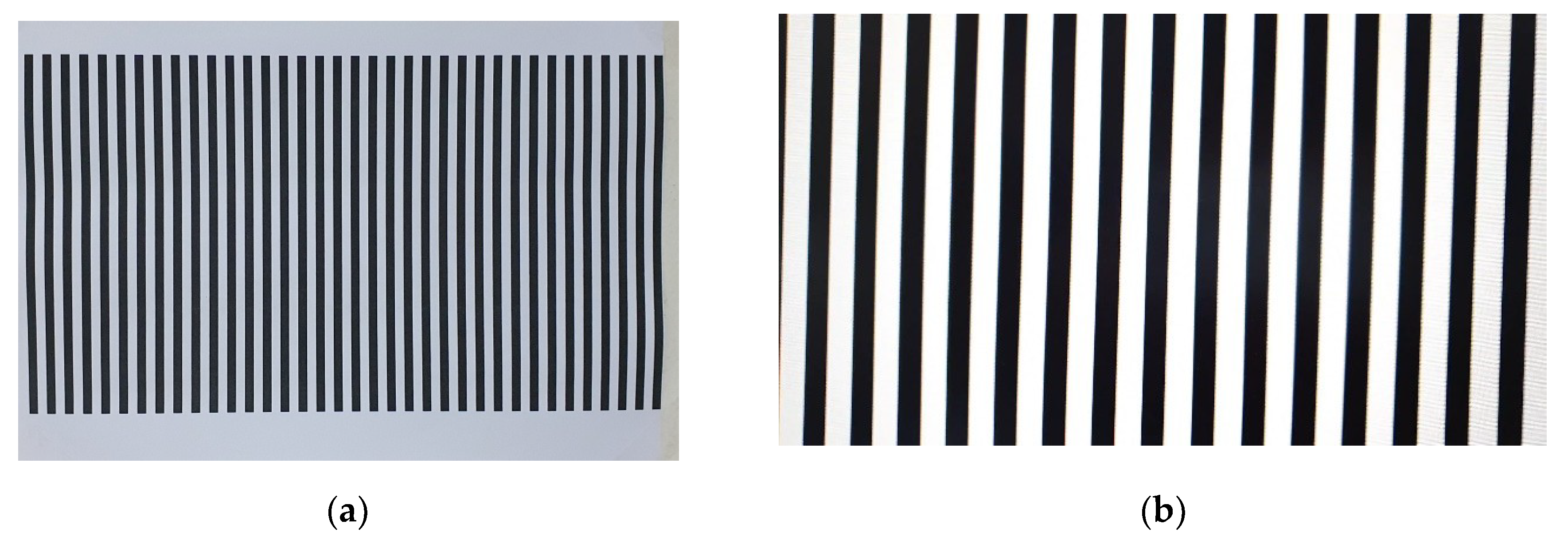




| Nikon D750 with AF-S Nikkor 50 mm f/1.8 G (Video Mode) | ||
|---|---|---|
| Specifications | ||
| Sensor Size | 35.9× 24 mm | |
| Image resolution | 6010 × 4010 | |
| Video resolution | 1920 × 1080 | |
| Pixel size | 5.98 μm | |
| Parameters | Value | |
| Focal length | fcx | 54.230267 mm |
| fcy | 54.448138 mm | |
| Principal point | pcx | 3132.23992 pixels |
| pcy | 1706.34214 pixels | |
| Skew | 0.0000 | |
| Radial distortion parameters | [−0.12818 1.21296] | |
| Tangential distortion parameters | [−0.00898 0.00315] | |
| Case | Speed (m/s) | Distance (m) | RSE (°) | Estimated Distance (m) | Error (m) |
|---|---|---|---|---|---|
| Case 1 (30 km/h, 5 m) | 8.0591 | 5.2054 | 4.17 | 5.2191 | 0.0137 |
| 8.0564 | 5.4532 | 4.03 | 5.4179 | 0.0353 | |
| 8.0676 | 4.8304 | 4.62 | 4.7169 | 0.1135 | |
| 8.0637 | 4.9168 | 4.30 | 5.0627 | 0.1459 | |
| 8.0221 | 5.3246 | 4.09 | 5.2952 | 0.0294 | |
| Case 2 (40 km/h, 5 m) | 11.2101 | 5.4408 | 5.48 | 5.5200 | 0.0792 |
| 11.2115 | 4.9365 | 5.91 | 5.1153 | 0.1788 | |
| 11.1760 | 5.3313 | 5.76 | 5.2365 | 0.0948 | |
| 11.1636 | 5.1477 | 5.99 | 5.0356 | 0.1121 | |
| 11.0520 | 5.3982 | 5.46 | 5.4553 | 0.0571 | |
| Case 3 (50 km/h, 5 m) | 13.5850 | 4.9982 | 7.28 | 5.0206 | 0.0224 |
| 13.5862 | 5.2270 | 6.71 | 5.4563 | 0.2293 | |
| 13.5744 | 5.1882 | 7.03 | 5.2008 | 0.0126 | |
| 13.5815 | 5.2039 | 7.22 | 5.0662 | 0.1377 | |
| 13.4091 | 5.1584 | 6.75 | 5.3463 | 0.1879 | |
| Case 4 (40 km/h, 7 m) | 10.8343 | 6.8436 | 4.40 | 6.9635 | 0.1199 |
| 11.0844 | 6.7237 | 4.50 | 6.6481 | 0.0756 | |
| 11.0032 | 6.8947 | 4.25 | 6.9898 | 0.0951 | |
| 11.1035 | 7.3434 | 4.00 | 7.4994 | 0.1560 | |
| 10.9582 | 7.0984 | 4.26 | 6.9435 | 0.1549 | |
| Case 5 (40 km/h, 10 m) | 10.9093 | 9.703 | 3.01 | 9.9355 | 0.2325 |
| 10.7693 | 10.2543 | 2.79 | 10.4228 | 0.1685 | |
| 11.1222 | 10.3869 | 2.83 | 10.6349 | 0.248 | |
| 11.1384 | 9.8542 | 3.13 | 9.6141 | 0.2401 | |
| 11.0771 | 10.1456 | 2.87 | 10.4352 | 0.2896 | |
| Case 6 (40 km/h, 15 m) | 11.0834 | 15.4933 | 1.86 | 16.1324 | 0.6391 |
| 11.0470 | 16.6647 | 1.89 | 15.8734 | 0.7913 | |
| 11.0691 | 15.4279 | 1.86 | 16.1166 | 0.6887 | |
| 11.0768 | 16.2025 | 1.85 | 15.3739 | 0.8286 | |
| 11.0527 | 16.1976 | 1.88 | 15.9551 | 0.2425 | |
| Case 7 (shutter speed 1/25 s) | 11.0357 | 5.2785 | 11.06 | 5.3222 | 0.0437 |
| 10.8491 | 5.5469 | 10.46 | 5.6047 | 0.0578 | |
| 10.9340 | 4.8003 | 12.15 | 4.7480 | 0.0523 | |
| 11.1712 | 4.9649 | 11.98 | 5.2230 | 0.2581 | |
| 11.0971 | 5.1576 | 11.52 | 5.2688 | 0.1112 | |
| Case 8 (shutter speed 1/60 s) | 11.3816 | 5.6948 | 4.61 | 5.5492 | 0.1456 |
| 11.0655 | 5.3171 | 4.74 | 5.2525 | 0.0646 | |
| 11.1952 | 5.9502 | 4.29 | 5.8793 | 0.0709 | |
| 11.1869 | 5.0344 | 4.85 | 5.1891 | 0.1547 | |
| 11.4898 | 5.4387 | 4.73 | 5.4663 | 0.0276 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, N.; Bae, J.; Kim, C.; Park, S.; Sohn, H.-G. Object Distance Estimation Using a Single Image Taken from a Moving Rolling Shutter Camera. Sensors 2020, 20, 3860. https://doi.org/10.3390/s20143860
Kim N, Bae J, Kim C, Park S, Sohn H-G. Object Distance Estimation Using a Single Image Taken from a Moving Rolling Shutter Camera. Sensors. 2020; 20(14):3860. https://doi.org/10.3390/s20143860
Chicago/Turabian StyleKim, Namhoon, Junsu Bae, Cheolhwan Kim, Soyeon Park, and Hong-Gyoo Sohn. 2020. "Object Distance Estimation Using a Single Image Taken from a Moving Rolling Shutter Camera" Sensors 20, no. 14: 3860. https://doi.org/10.3390/s20143860
APA StyleKim, N., Bae, J., Kim, C., Park, S., & Sohn, H.-G. (2020). Object Distance Estimation Using a Single Image Taken from a Moving Rolling Shutter Camera. Sensors, 20(14), 3860. https://doi.org/10.3390/s20143860





