Temperature Field Boundary Conditions and Lateral Temperature Gradient Effect on a PC Box-Girder Bridge Based on Real-Time Solar Radiation and Spatial Temperature Monitoring
Abstract
:1. Introduction
2. Experimental Instrumentation and Data Acquisition
3. Solar Radiation and Heat Transfer Theory
3.1. Direct Solar Radiation
3.2. Scattered Solar Radiation
3.3. Ground Reflected Radiation
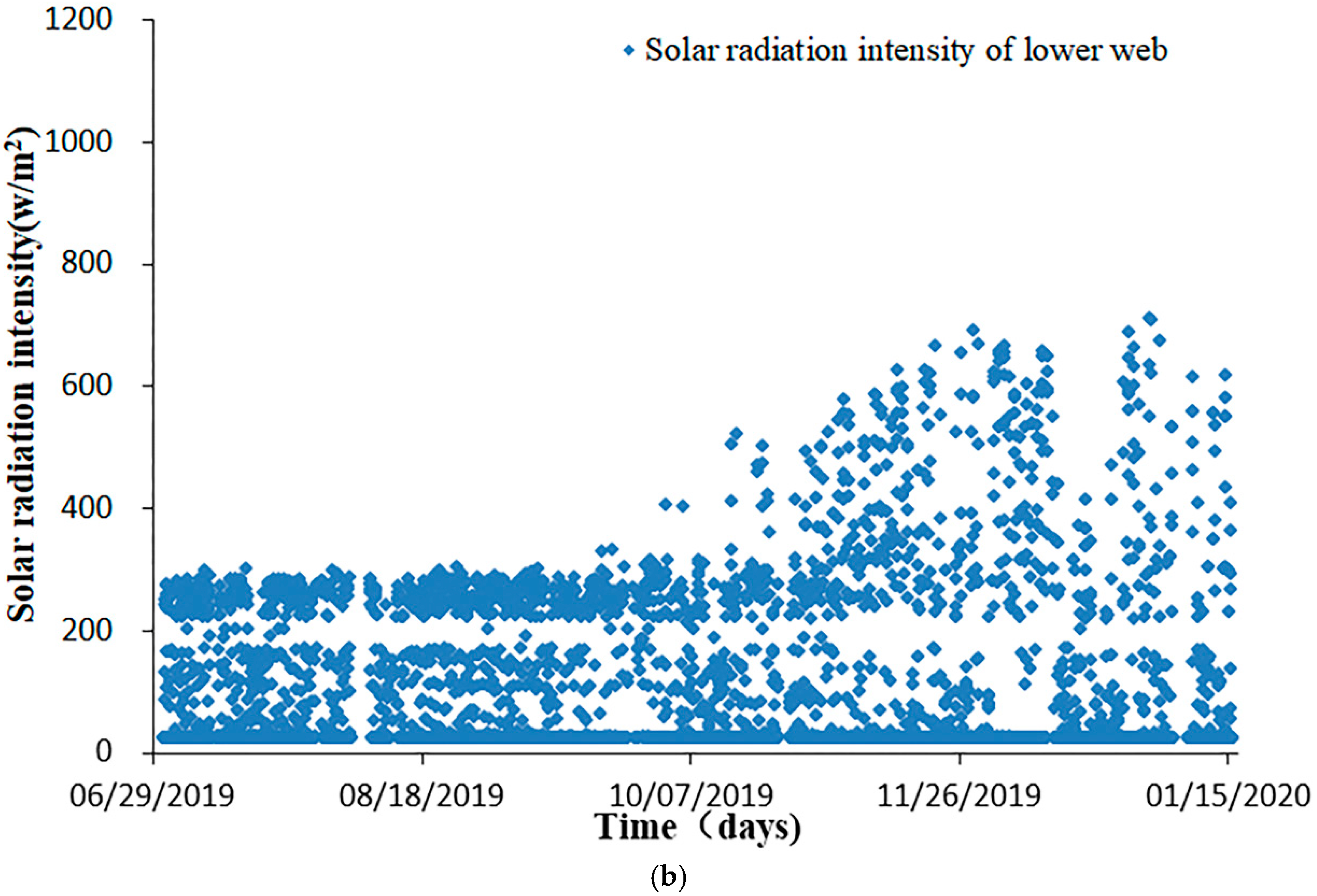
4. Theoretical and Measured Solar Radiation Intensity
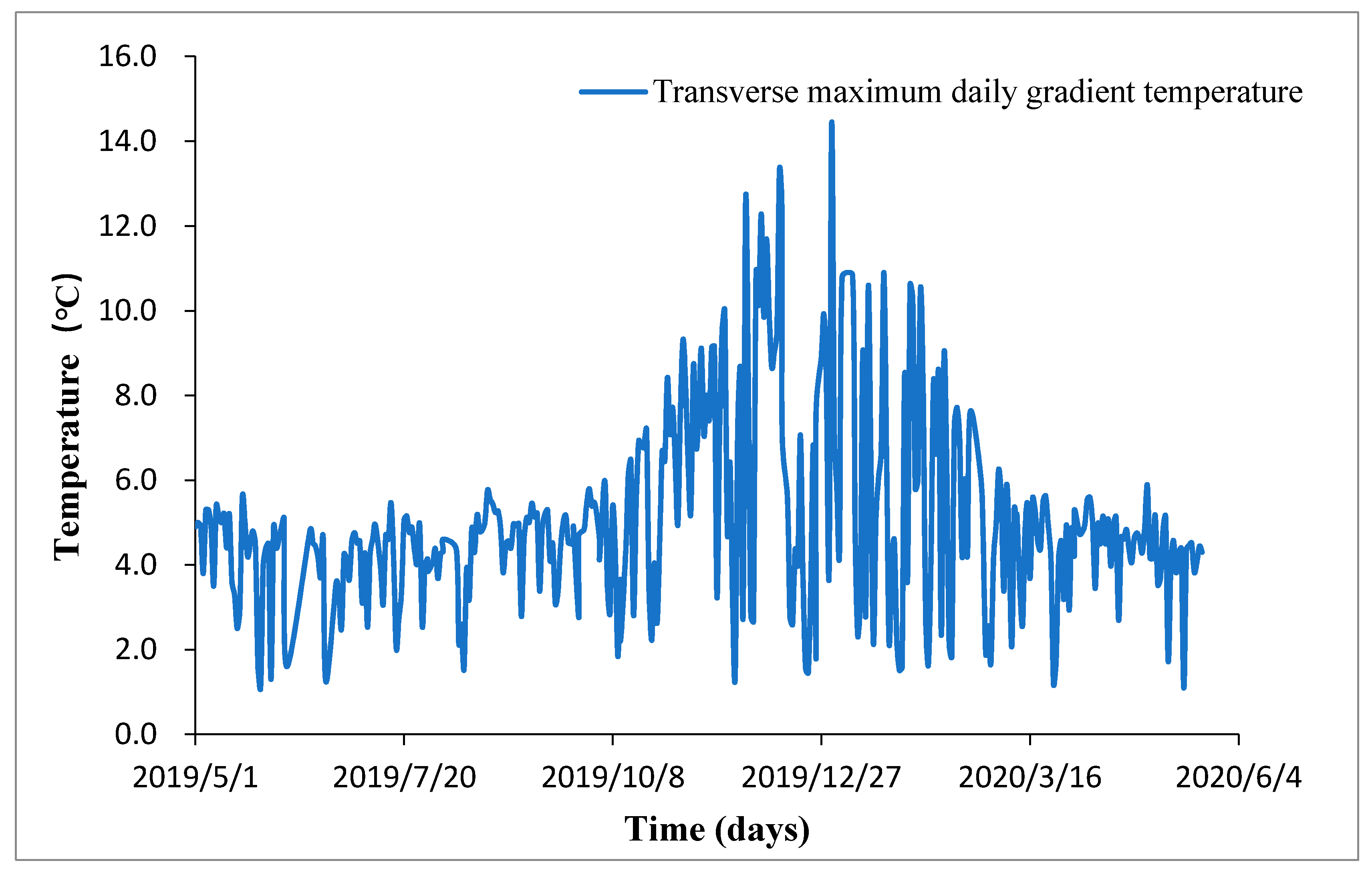
5. Solar Radiation and Temperature Gradient Relationship
6. Simulation of Temperature Field with Finite Element Method
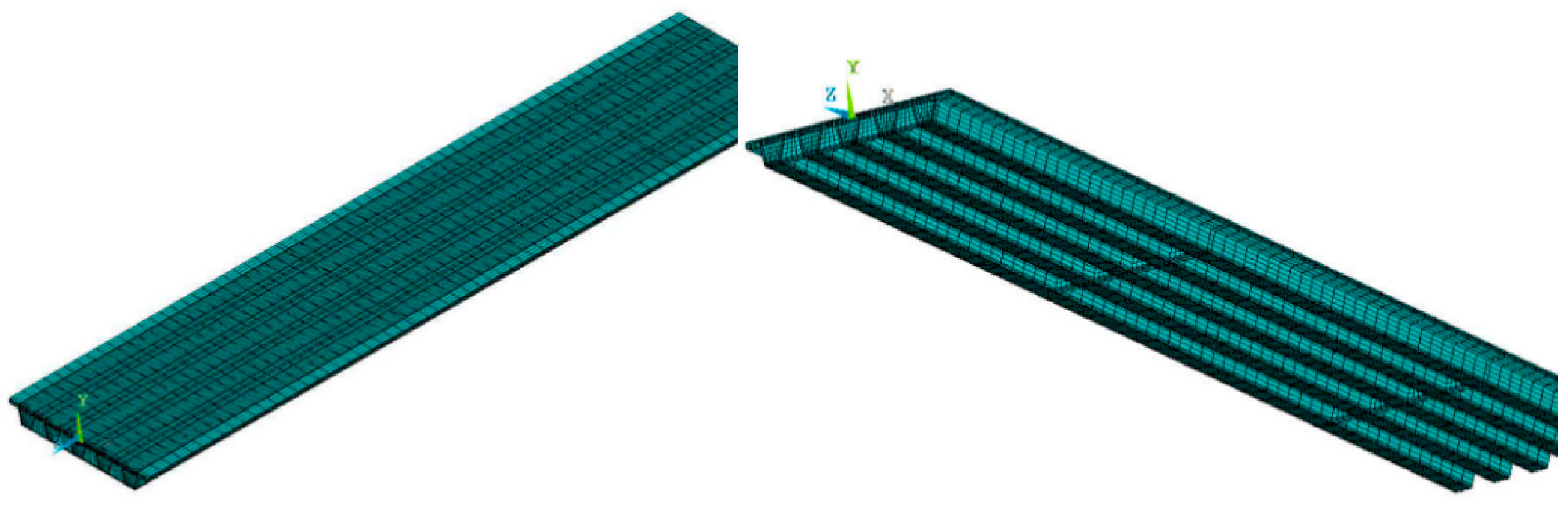
6.1. Finite Element Model
6.2. Parameters Used in Finite Element Modeling
6.2.1. The Thermo-Physical Properties of the Box Girder
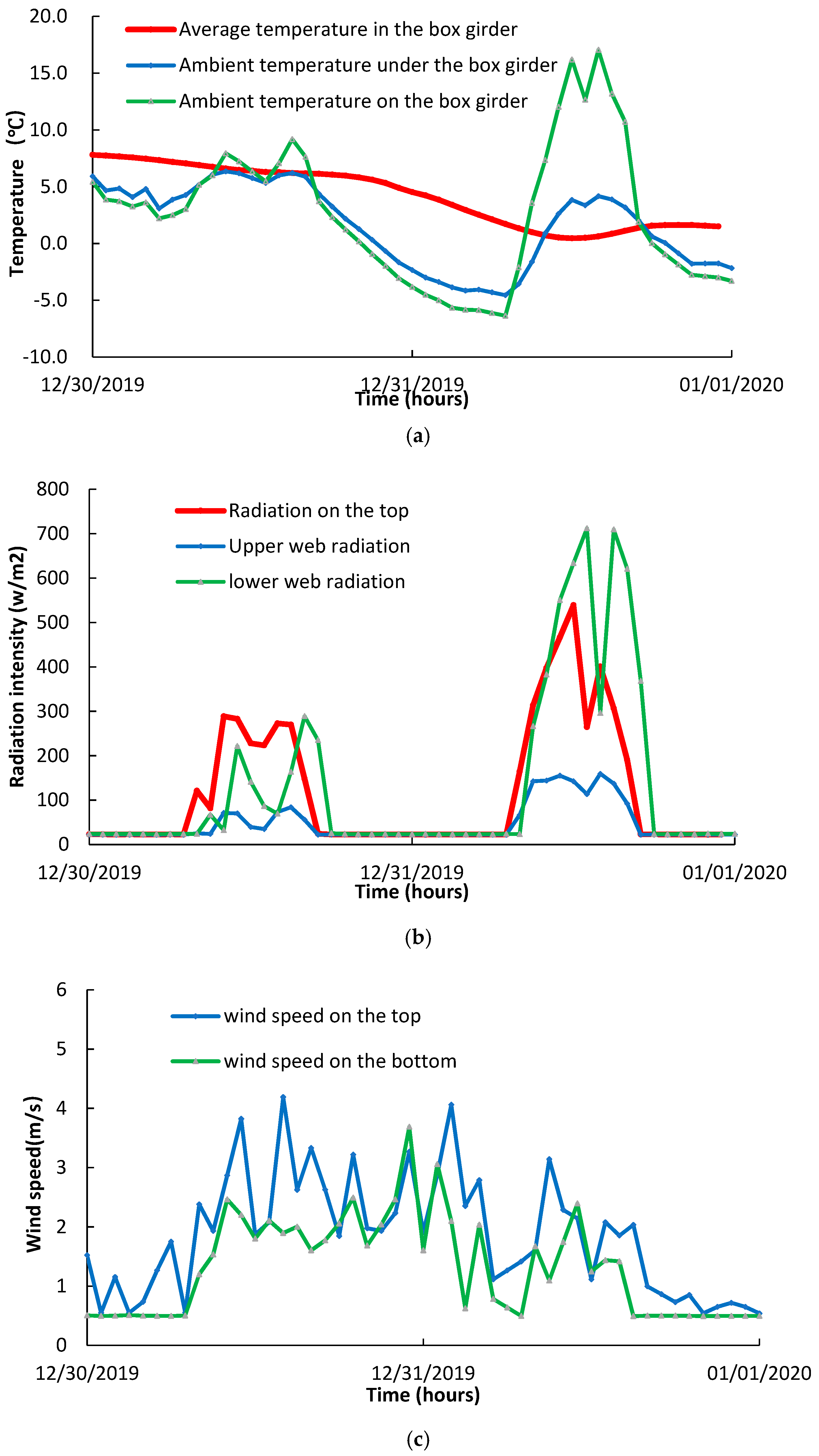
6.2.2. Boundary Conditions
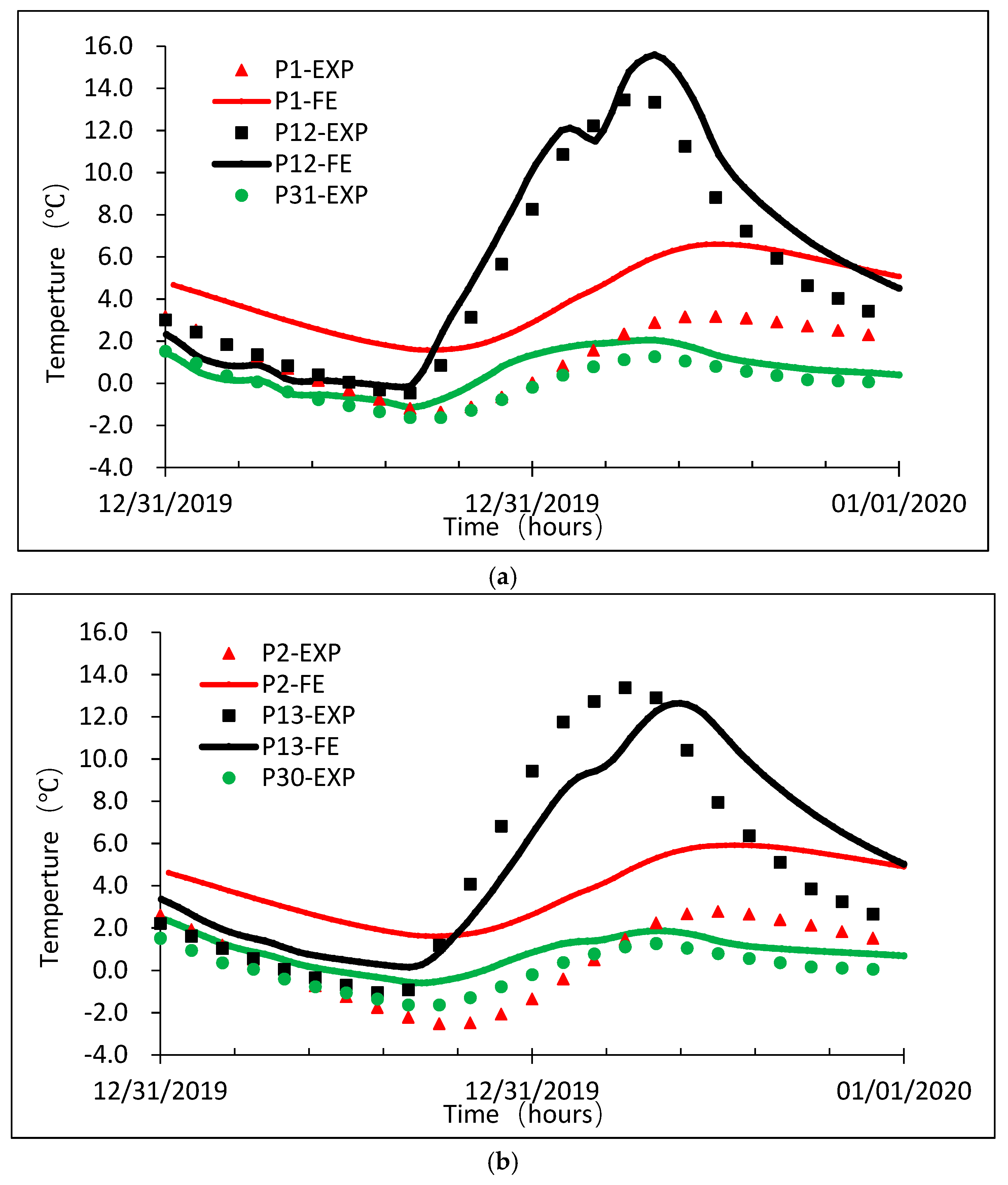
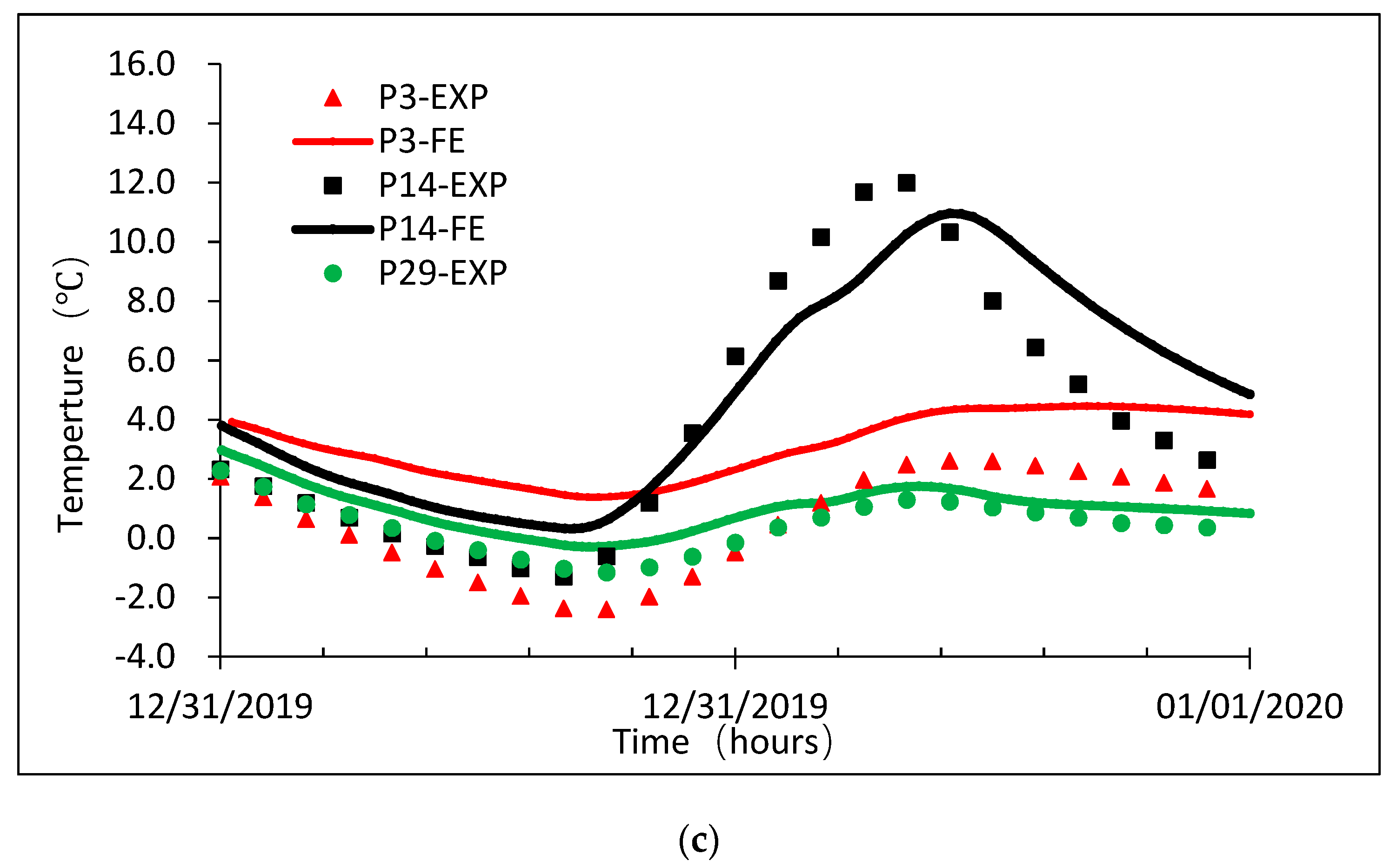
6.2.3. Comparison between the FE Analysis and Experimental Results
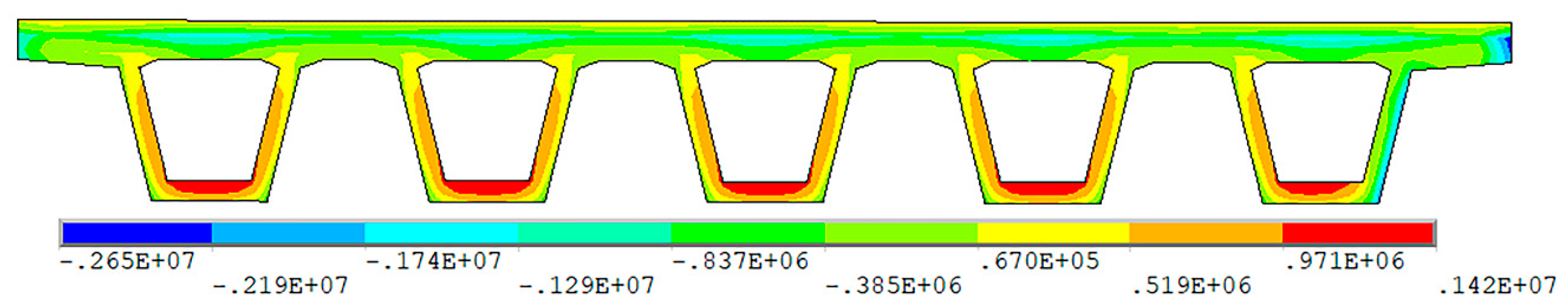
6.2.4. Stresses Caused by the Spatial Temperature Gradients in FE Analysis
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Hossain, T.; Segura, S.; Okeil, A.M. Structural effects of temperature gradient on a continuous prestressed concrete girder bridge: Analysis and field measurements. Struct. Infrastruct. Eng. 2020, 1–12. [Google Scholar] [CrossRef]
- Maguire, M.; Roberts-Wollmann, C.L.; Cousins, T. Live-load testing and long-term monitoring of the Varina-Enon Bridge: Investigating thermal distress. J. Bridge Eng. 2018, 23, 04018003. [Google Scholar] [CrossRef]
- Lee, J. Investigation of extreme environmental conditions and design thermal gradients during construction for prestressed concrete bridge girders. J. Bridge Eng. 2012, 17, 547–556. [Google Scholar] [CrossRef]
- Wang, Y.; Zhan, Y.; Zhao, R. Analysis of thermal behavior on concrete box-girder arch bridges under convection and solar radiation. Adv. Struct. Eng. 2016, 19, 1043–1059. [Google Scholar] [CrossRef]
- Abid, S.R.; Taysi, N.; Ozakca, M. Experimental analysis of temperature gradients in concrete box-girders. Constr. Build. Mater. 2016, 106, 523–532. [Google Scholar] [CrossRef]
- Abid, S.R.; Mussa, F.; Taysi, N.; Özakça, M. Experimental and finite element investigation of temperature distributions in concrete-encased steel girders. Struct. Control Health Monit. 2018, 25, e2042. [Google Scholar] [CrossRef]
- Taysi, N.; Abid, S.R. Temperature distributions and variations in concrete box-girder bridges: Experimental and finite element parametric studies. Adv. Struct. Eng. 2015, 18, 469–486. [Google Scholar] [CrossRef]
- Tian, Y.; Zhang, N.; Xia, H. Temperature effect on service performance of high-speed railway concrete bridges. Adv. Struct. Eng. 2017, 20, 865–883. [Google Scholar] [CrossRef]
- Lawson, L.; Ryan, K.L.; Buckle, I.G. Bridge Temperature Profiles Revisited: Thermal Analyses Based on Recent Meteorological Data from Nevada. J. Bridge Eng. 2020, 25, 04019124. [Google Scholar] [CrossRef]
- Hagedorn, R.; Martivargas, J.R.; Dang, C.N.; Hale, W.M.; Floyd, R.W. Temperature Gradients in Bridge Concrete I-Girders under Heat Wave. J. Bridge Eng. 2019, 24, 04019077. [Google Scholar] [CrossRef]
- Rodriguez, L.E.; Barr, P.J.; Halling, M.W. Temperature Effects on a Box-Girder Integral-Abutment Bridge. J. Perform. Constr. Facil. 2014, 28, 583–591. [Google Scholar] [CrossRef]
- Kehlbeck, F. Effect of Solar Radiation on Bridge Structure; Liu, X., Translator; Railway Publishing House: Beijing, China, 1981. [Google Scholar]
- Ghali, A.; Favre, R.; Elbadry, M. Concrete Structures: Stresses and Deformation, 3rd ed.; E & FN Spon: London, UK, 2002. [Google Scholar]
- Lei, X.; Jiang, H.; Wang, J. Temperature effects on horizontally curved concrete box-girder bridges with single-column piers. J. Aerosp. Eng. 2019, 32, 04019008. [Google Scholar] [CrossRef]
- Liu, X.F. Temperature Induced Stress Analysis for Concrete Structures; Communications Press: Beijing, China, 1991. [Google Scholar]
- Elbadry, M.; Ghali, A. Temperature Variations in Concrete Bridges. J. Struct. Eng. 1983, 109, 2355–2374. [Google Scholar] [CrossRef]
- Jain, P.C. A method for diffuse and global irradiation of horizontal surfaces. Solar Energy 1990, 44, 301–308. [Google Scholar] [CrossRef]
- Page, J.K. The estimation of monthly means values of daily total short-wave radiation oil vertical and inclined surfaces from sunshine records for latitudes 40° N–40° S. In Proceedings of the UN Conference on News Sources of Energy, Rome, Italy, 16 May 1961; pp. 1–16. [Google Scholar]
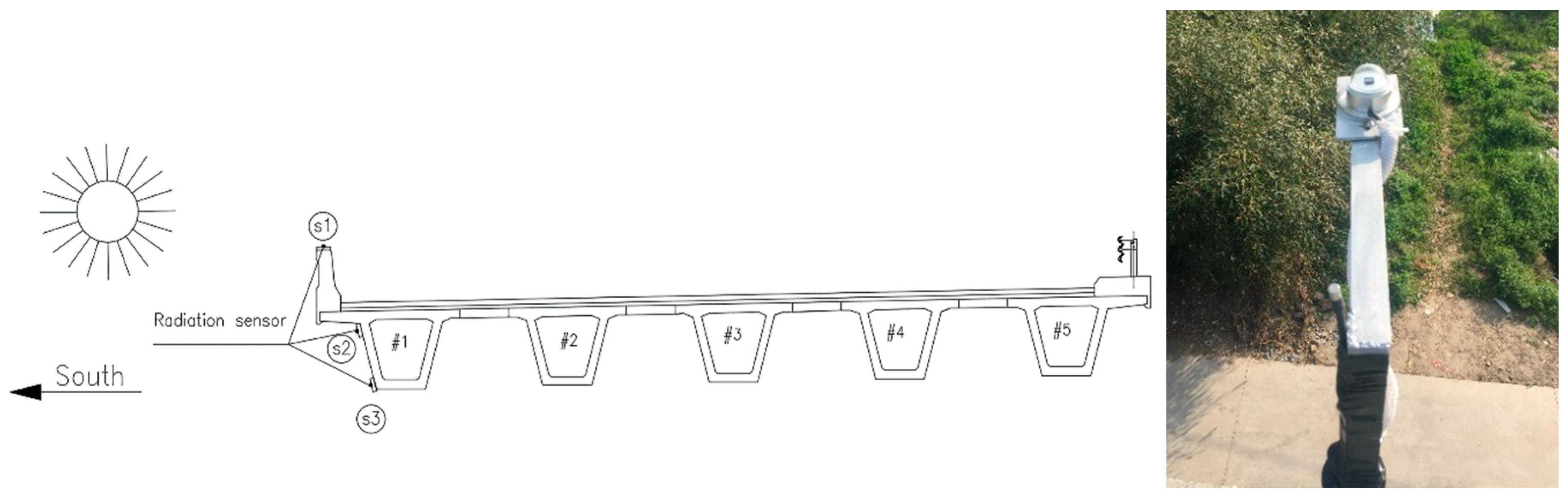

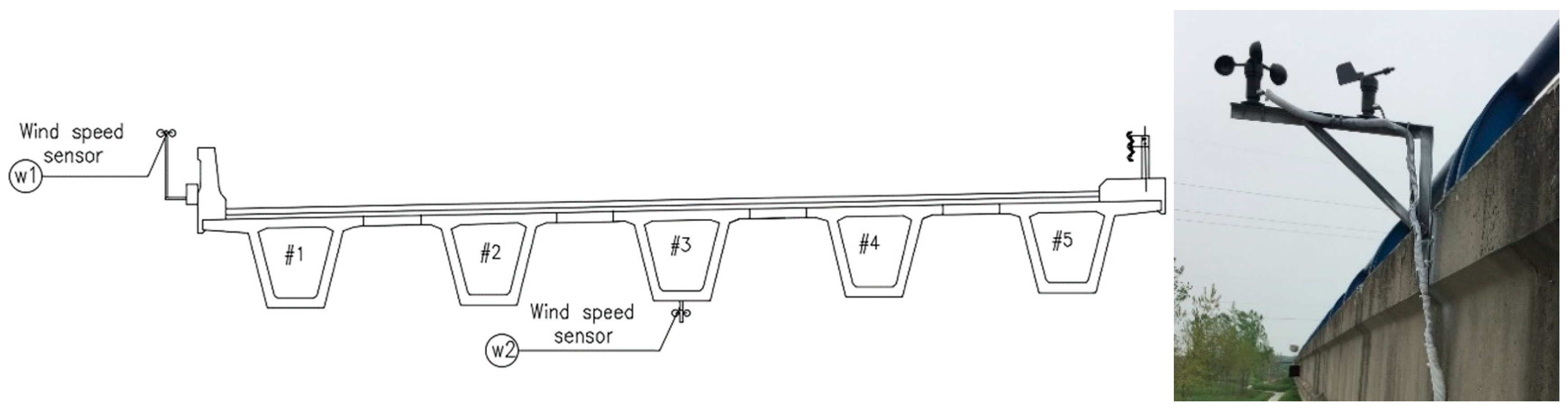





| Item | Daily Maximum Solar Radiation | ||
|---|---|---|---|
| Position | Top slab (s1) | Upper web (s2) | Lower web (s3) |
| Pearson correlation coefficients | 0.124 | 0.276 | 0.879 |
| Material | Density (kg/m3) | Specific Capacity (J/kg °C) | Heat Thermal Conductivity (W/m °C) | Absorption Rate |
|---|---|---|---|---|
| Concrete | 2500 | 880 | 2.5 | 0.4 |
| Asphalt concrete | 1700 | 1000 | 1.403 | 0.8 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lei, X.; Fan, X.; Jiang, H.; Zhu, K.; Zhan, H. Temperature Field Boundary Conditions and Lateral Temperature Gradient Effect on a PC Box-Girder Bridge Based on Real-Time Solar Radiation and Spatial Temperature Monitoring. Sensors 2020, 20, 5261. https://doi.org/10.3390/s20185261
Lei X, Fan X, Jiang H, Zhu K, Zhan H. Temperature Field Boundary Conditions and Lateral Temperature Gradient Effect on a PC Box-Girder Bridge Based on Real-Time Solar Radiation and Spatial Temperature Monitoring. Sensors. 2020; 20(18):5261. https://doi.org/10.3390/s20185261
Chicago/Turabian StyleLei, Xiao, Xutao Fan, Hanwan Jiang, Kunning Zhu, and Hanyu Zhan. 2020. "Temperature Field Boundary Conditions and Lateral Temperature Gradient Effect on a PC Box-Girder Bridge Based on Real-Time Solar Radiation and Spatial Temperature Monitoring" Sensors 20, no. 18: 5261. https://doi.org/10.3390/s20185261
APA StyleLei, X., Fan, X., Jiang, H., Zhu, K., & Zhan, H. (2020). Temperature Field Boundary Conditions and Lateral Temperature Gradient Effect on a PC Box-Girder Bridge Based on Real-Time Solar Radiation and Spatial Temperature Monitoring. Sensors, 20(18), 5261. https://doi.org/10.3390/s20185261





