Wearable Cardiorespiratory Monitoring Employing a Multimodal Digital Patch Stethoscope: Estimation of ECG, PEP, LVET and Respiration Using a 55 mm Single-Lead ECG and Phonocardiogram
Abstract
:1. Introduction
1.1. Clinical Background and State-of-the-Art
1.2. Scope of the Presented Work
2. Materials and Methods
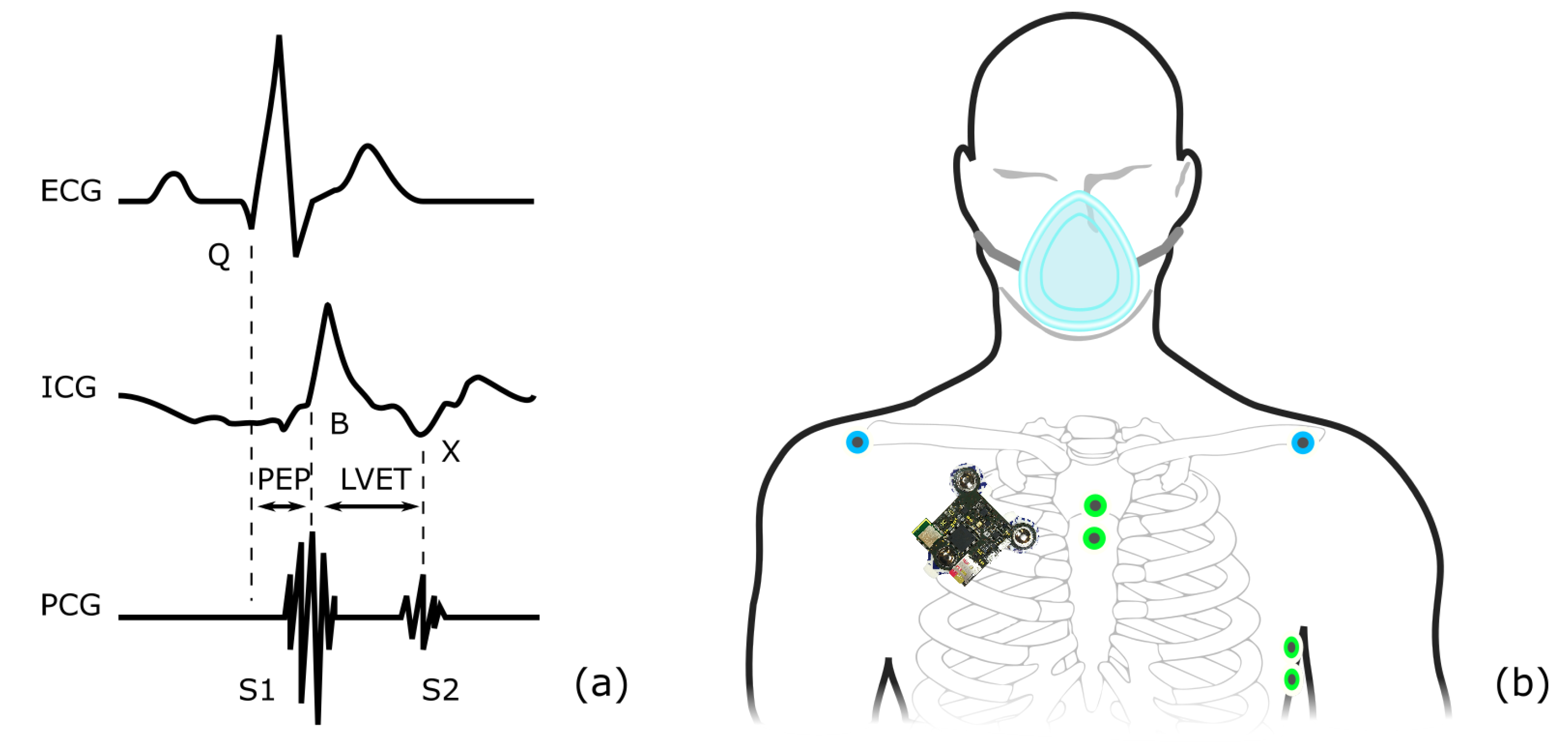
2.1. Data Acquisition
2.2. Regression Models and Cross-Validation
2.3. Performance Metrics
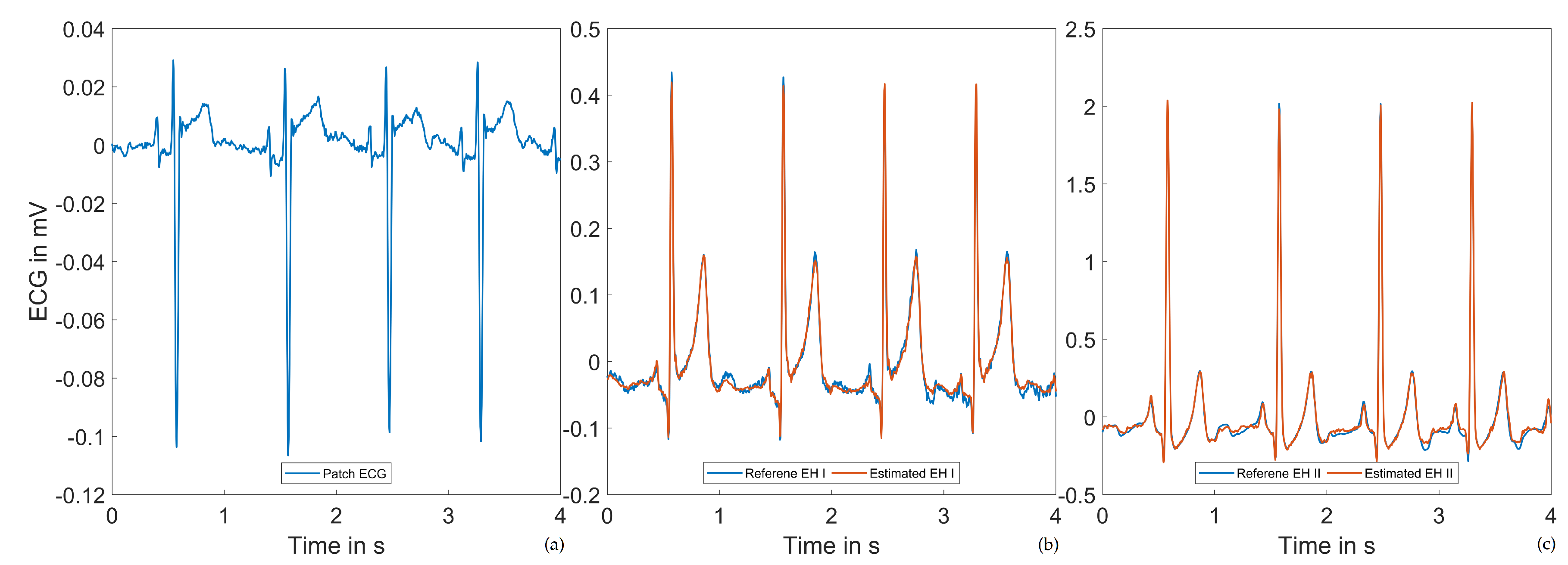
2.4. Einthoven Lead Estimation
2.5. PEP and LVET Estimation
2.6. Electrocardiogram- and Phonocardiogram-Derived Respiration
3. Results
3.1. Einthoven Lead Estimation
3.2. PEP and LVET Estimation
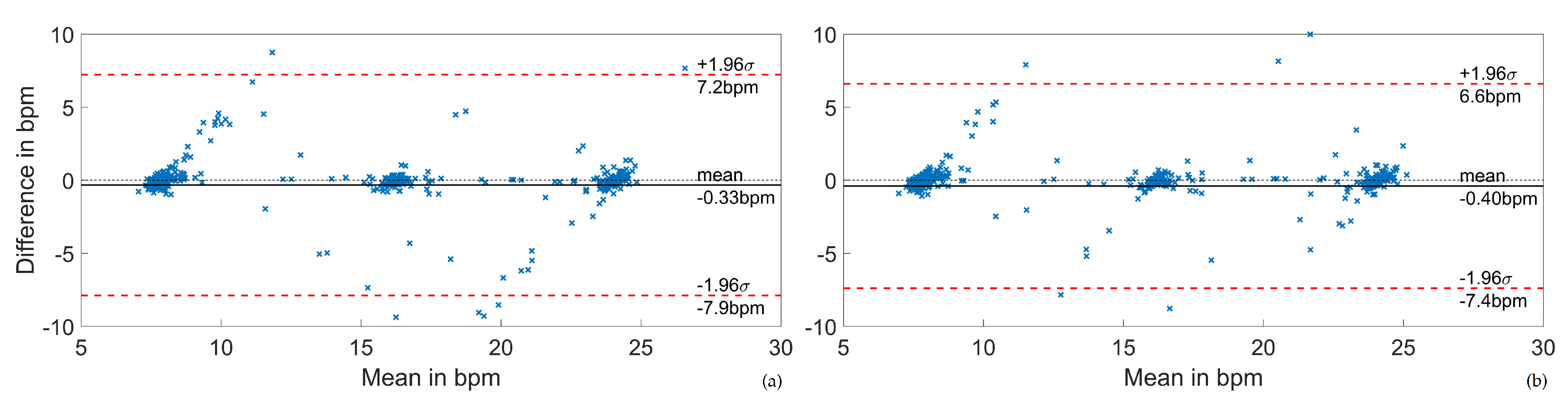
3.3. Electrocardiogram- and Phonocardiogram-Derived Respiration
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| B2B | Beat-to-beat |
| BIC | Bayesian information criterion |
| bpm | Breaths per minute |
| CVD | Cardiovascular disease |
| ECG | Electrocardiogram |
| EDR | Electrocardiogram-derived respiration |
| FFT | Fast Fourier transform |
| ICG | Impedance cardiography |
| ICS | Intercostal space |
| LVET | Left ventricular ejection time |
| MDPI | Multidisciplinary Digital Publishing Institute |
| MAE | Mean absolute error |
| MAPE | Mean absolute percentage error |
| MARG | Magnetic, angular rate, and gravity |
| ME | Mean error |
| MERS | Middle East respiratory syndrome |
| MLP | Multi-layer perceptron |
| NMSE | Normalized mean squared error |
| PCA | Principal component analysis |
| PCB | Printed circuit boards |
| PCG | Phonocardiogram |
| PDR | Phonocardiogram-derived respiration |
| PEP | Pre-ejection period |
| PPG | Photoplethysmogram |
| RR | Respiratory rate |
| SARS | Severe acute respiratory syndrome |
| SCG | Seismocardiogram |
| SSE | Sum of squared errors |
| STI | Systolic time intervals |
| TDNN | Time-delay neural network |
References
- World Health Organization. Global Health Estimates 2016: Deaths by Cause, Age, Sex, by Country and by Region, 2000–2016; World Health Organization: Geneva, Switzerland, 2018. [Google Scholar]
- Marin, J.M.; Agusti, A.; Villar, I.; Forner, M.; Nieto, D.; Carrizo, S.J.; Barbé, F.; Vicente, E.; Wei, Y.; Nieto, F.J.; et al. Association between treated and untreated obstructive sleep apnea and risk of hypertension. JAMA 2012, 307, 2169–2176. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cadby, G.; McArdle, N.; Briffa, T.; Hillman, D.R.; Simpson, L.; Knuiman, M.; Hung, J. Severity of OSA is an independent predictor of incident atrial fibrillation hospitalization in a large sleep-clinic cohort. Chest 2015, 148, 945–952. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Benjafield, A.V.; Ayas, N.T.; Eastwood, P.R.; Heinzer, R.; Ip, M.S.; Morrell, M.J.; Nunez, C.M.; Patel, S.R.; Penzel, T.; Pépin, J.L.; et al. Estimation of the global prevalence and burden of obstructive sleep apnoea: A literature based analysis. Lancet Respir. Med. 2019, 7, 687–698. [Google Scholar] [CrossRef] [Green Version]
- Broens, S.J.; He, X.; Evley, R.; Olofsen, E.; Niesters, M.; Mahajan, R.P.; Dahan, A.; van Velzen, M. Frequent respiratory events in postoperative patients aged 60 years and above. Ther. Clin. Risk Manag. 2017, 13, 1091. [Google Scholar] [CrossRef] [Green Version]
- Sun, Z.; Sessler, D.I.; Dalton, J.E.; Devereaux, P.; Shahinyan, A.; Naylor, A.J.; Hutcherson, M.T.; Finnegan, P.S.; Tandon, V.; Darvish-Kazem, S.; et al. Postoperative hypoxemia is common and persistent: A prospective blinded observational study. Anesth. Analg. 2015, 121, 709. [Google Scholar] [CrossRef] [Green Version]
- Fernandez-Bustamante, A.; Frendl, G.; Sprung, J.; Kor, D.J.; Subramaniam, B.; Ruiz, R.M.; Lee, J.W.; Henderson, W.G.; Moss, A.; Mehdiratta, N.; et al. Postoperative pulmonary complications, early mortality, and hospital stay following noncardiothoracic surgery: A multicenter study by the perioperative research network investigators. JAMA Surg. 2017, 152, 157–166. [Google Scholar] [CrossRef]
- World Health Organization. Coronavirus Disease 2019 (COVID-19): Situation Report, 51; World Health Organization: Geneva, Switzerland, 2020. [Google Scholar]
- Bai, L.; Yang, D.; Wang, X.; Tong, L.; Zhu, X.; Bai, C.; Powell, C.A. Chinese experts’ consensus on the Internet of Things-aided diagnosis and treatment of coronavirus disease 2019. Clin. eHealth 2020, 3, 7–15. [Google Scholar] [CrossRef]
- Majumder, S.; Mondal, T.; Deen, M.J. Wearable sensors for remote health monitoring. Sensors 2017, 17, 130. [Google Scholar] [CrossRef]
- Dias, D.; Paulo Silva Cunha, J. Wearable health devices—vital sign monitoring, systems and technologies. Sensors 2018, 18, 2414. [Google Scholar] [CrossRef] [Green Version]
- Zeng, W.; Shu, L.; Li, Q.; Chen, S.; Wang, F.; Tao, X.M. Fiber based wearable electronics: A review of materials, fabrication, devices, and applications. Adv. Mater. 2014, 26, 5310–5336. [Google Scholar] [CrossRef]
- Khan, Y.; Ostfeld, A.E.; Lochner, C.M.; Pierre, A.; Arias, A.C. Monitoring of vital signs with flexible and wearable medical devices. Adv. Mater. 2016, 28, 4373–4395. [Google Scholar] [CrossRef] [PubMed]
- Harmon, K.G.; Zigman, M.; Drezner, J.A. The effectiveness of screening history, physical exam, and ECG to detect potentially lethal cardiac disorders in athletes: A systematic review/meta-analysis. J. Electrocardiol. 2015, 48, 329–338. [Google Scholar] [CrossRef] [PubMed]
- Greenland, P.; Alpert, J.S.; Beller, G.A.; Benjamin, E.J.; Budoff, M.J.; Fayad, Z.A.; Foster, E.; Hlatky, M.A.; Hodgson, J.M.; Kushner, F.G.; et al. 2010 ACCF/AHA guideline for assessment of cardiovascular risk in asymptomatic adults: A report of the American College of Cardiology Foundation/American Heart Association task force on practice guidelines developed in collaboration with the American Society of Echocardiography, American Society of Nuclear Cardiology, Society of Atherosclerosis Imaging and Prevention, Society for Cardiovascular Angiography and Interventions, Society of Cardiovascular Computed Tomography, and Society for Cardiovascular Magnetic Resonance. J. Am. Coll. Cardiol. 2010, 56, e50–e103. [Google Scholar] [PubMed] [Green Version]
- Amsterdam, E.A.; Kirk, J.D.; Bluemke, D.A.; Diercks, D.; Farkouh, M.E.; Garvey, J.L.; Kontos, M.C.; McCord, J.; Miller, T.D.; Morise, A.; et al. Testing of Low-Risk Patients Presenting to the Emergency Department With Chest Pain. Circulation 2010, 122, 1756–1776. [Google Scholar] [CrossRef] [Green Version]
- Calkins, H.; Hindricks, G.; Cappato, R.; Kim, Y.H.; Saad, E.B.; Aguinaga, L.; Akar, J.G.; Badhwar, V.; Brugada, J.; Camm, J.; et al. 2017 HRS/EHRA/ECAS/APHRS/SOLAECE expert consensus statement on catheter and surgical ablation of atrial fibrillation. EP Eur. 2017, 20, e1–e160. [Google Scholar] [CrossRef]
- Tomašić, I.; Trobec, R. Electrocardiographic systems with reduced numbers of Leads—synthesis of the 12-lead ECG. IEEE Rev. Biomed. Eng. 2013, 7, 126–142. [Google Scholar] [CrossRef]
- Hansen, I.H.; Hoppe, K.; Gjerde, A.; Kanters, J.K.; Sorensen, H.B. Comparing twelve-lead electrocardiography with close-to-heart patch based electrocardiography. In Proceedings of the 2015 37th Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBC), Milan, Italy, 25–29 August 2015; pp. 330–333. [Google Scholar]
- Lee, H.J.; Lee, D.S.; Kwon, H.B.; Kim, D.Y.; Park, K.S. Reconstruction of 12-lead ECG Using a Single-patch Device. Methods Inf. Med. 2017, 56, 319–327. [Google Scholar] [CrossRef]
- Tomasic, I.; Rashkovska, A.; Trobec, R.; Lindén, M. The Implications of the Lead Theory on the Patch ECG Devices Positioning and Measurement. In World Congress on Medical Physics and Biomedical Engineering 2018; Springer: Singapore, 2019; pp. 693–696. [Google Scholar]
- Reed, T.R.; Reed, N.E.; Fritzson, P. Heart sound analysis for symptom detection and computer-aided diagnosis. Simul. Model. Pract. Theory 2004, 12, 129–146. [Google Scholar] [CrossRef]
- Gavrovska, A.; Zajić, G.; Reljin, I.; Reljin, B. Classification of prolapsed mitral valve versus healthy heart from phonocardiograms by multifractal analysis. Comput. Math. Methods Med. 2013, 2013, 376152. [Google Scholar] [CrossRef]
- Potes, C.; Parvaneh, S.; Rahman, A.; Conroy, B. Ensemble of feature based and deep learning based classifiers for detection of abnormal heart sounds. In Proceedings of the 2016 Computing in Cardiology Conference (CinC), Vancouver, BC, Canada, 11–14 September 2016; pp. 621–624. [Google Scholar]
- Pinto, C.; Pereira, D.; Ferreira-Coimbra, J.; Português, J.; Gama, V.; Coimbra, M. A comparative study of electronic stethoscopes for cardiac auscultation. In Proceedings of the 2017 39th Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBC), Seogwipo, Korea, 11–15 July 2017; pp. 2610–2613. [Google Scholar]
- Swarup, S.; Makaryus, A.N. Digital stethoscope: Technology update. Med. Devices (Auckland, NZ) 2018, 11, 29. [Google Scholar] [CrossRef] [Green Version]
- Tavakolian, K. Systolic time intervals and new measurement methods. Cardiovasc. Eng. Technol. 2016, 7, 118–125. [Google Scholar] [CrossRef] [PubMed]
- Carvalho, P.; Paiva, R.; Couceiro, R.; Henriques, J.; Antunes, M.; Quintal, I.; Muehlsteff, J.; Aubert, X. Comparison of systolic time interval measurement modalities for portable devices. In Proceedings of the 2010 Annual International Conference of the IEEE Engineering in Medicine and Biology, Buenos Aires, Argentina, 31 August–4 September 2010; pp. 606–609. [Google Scholar]
- Kh Dehkordi, P.; Khosrow-Khavar, F.; Di Rienzo, M.; Inan, O.T.; Schmidt, S.E.; Blaber, A.; Sørensen, K.; Struijk, J.J.; Zakeri, V.; Lombardi, P.; et al. Comparison of Different Methods for Estimating Cardiac Timings: A Comprehensive Multimodal Echocardiography Investigation. Front. Physiol. 2019, 10, 1057. [Google Scholar] [CrossRef] [PubMed]
- Paiva, R.; Carvalho, P.; Couceiro, R.; Henriques, J.; Antunes, M.; Quintal, I.; Muehlsteff, J. Beat-to-beat systolic time-interval measurement from heart sounds and ECG. Physiol. Meas. 2012, 33, 177. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.F.; Chou, Y.S.; Wang, J.Y. PEP and LVET Detection from PCG and ECG. In International Conference on Biomedical and Health Informatics; Springer: Cham, Switzerland, 2019; pp. 363–370. [Google Scholar]
- Inan, O.T. Wearable Sensing of Left Ventricular Function. In Mobile Health; Springer: Cham, Switzerland, 2017; pp. 265–287. [Google Scholar]
- Berry, R.B.; Brooks, R.; Gamaldo, C.E.; Harding, S.M.; Marcus, C.; Vaughn, B.V. The AASM manual for the scoring of sleep and associated events. Rules Terminol. Tech. Specif. Darien Illinois Am. Acad. Sleep Med. 2020, 176, 2012. [Google Scholar]
- Kapur, V.K.; Auckley, D.H.; Chowdhuri, S.; Kuhlmann, D.C.; Mehra, R.; Ramar, K.; Harrod, C.G. Clinical practice guideline for diagnostic testing for adult obstructive sleep apnea: An American Academy of Sleep Medicine clinical practice guideline. J. Clin. Sleep Med. 2017, 13, 479–504. [Google Scholar] [CrossRef] [PubMed]
- Charlton, P.H.; Birrenkott, D.A.; Bonnici, T.; Pimentel, M.A.; Johnson, A.E.; Alastruey, J.; Tarassenko, L.; Watkinson, P.J.; Beale, R.; Clifton, D.A. Breathing rate estimation from the electrocardiogram and photoplethysmogram: A review. IEEE Rev. Biomed. Eng. 2017, 11, 2–20. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Klum, M.; Minn, T.; Tigges, T.; Pielmus, A.G.; Orglmeister, R. Minimally spaced electrode positions for multi-functional chest sensors: ECG and respiratory signal estimation. Curr. Dir. Biomed. Eng. 2016, 2, 695–699. [Google Scholar] [CrossRef]
- Massaroni, C.; Nicolò, A.; Lo Presti, D.; Sacchetti, M.; Silvestri, S.; Schena, E. Contact based methods for measuring respiratory rate. Sensors 2019, 19, 908. [Google Scholar] [CrossRef] [Green Version]
- Liu, H.; Allen, J.; Zheng, D.; Chen, F. Recent development of respiratory rate measurement technologies. Physiol. Meas. 2019, 40, 07TR01. [Google Scholar] [CrossRef] [Green Version]
- Taebi, A.; Mansy, H.A. Grouping similar seismocardiographic signals using respiratory information. In Proceedings of the 2017 IEEE Signal Processing in Medicine and Biology Symposium (SPMB), Philadelphia, PA, USA, 2 December 2017; pp. 1–6. [Google Scholar]
- Skoric, J.; D’Mello, Y.; Lortie, M.; Gagnon, S.; Plant, D.V. Effect of Static Respiratory Volume on the Waveform of Cardiac-induced Sternal Vibrations. In Proceedings of the 2019 41st Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBC), Berlin, Germany, 23–27 July 2019; pp. 4917–4921. [Google Scholar]
- Zakeri, V.; Akhbardeh, A.; Alamdari, N.; Fazel-Rezai, R.; Paukkunen, M.; Tavakolian, K. Analyzing seismocardiogram cycles to identify the respiratory phases. IEEE Trans. Biomed. Eng. 2016, 64, 1786–1792. [Google Scholar] [CrossRef]
- Alamdari, N.; Tavakolian, K.; Zakeri, V.; Fazel-Rezai, R.; Akhbardeh, A. A morphological approach to detect respiratory phases of seismocardiogram. In Proceedings of the 2016 38th Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBC), Orlando, FL, USA, 16–20 August 2016; pp. 4272–4275. [Google Scholar]
- Choudhary, T.; Bhuyan, M.; Sharma, L. Effect of Respiratory Effort Levels on SCG Signals. In Proceedings of the 2019 IEEE Region 10 Symposium (TENSYMP), Kolkata, India, 7–9 June 2019; pp. 143–146. [Google Scholar]
- Choudhary, T.; Bhuyan, M.; Bora, K.; Sharma, L. Design of Breathing-states Detector for m-Health Platform using Seismocardiographic Signal. arXiv 2020, arXiv:2002.10510. [Google Scholar]
- Taebi, A.; Solar, B.E.; Bomar, A.J.; Sandler, R.H.; Mansy, H.A. Recent advances in seismocardiography. Vibration 2019, 2, 5. [Google Scholar] [CrossRef] [Green Version]
- Di Rienzo, M.; Vaini, E.; Castiglioni, P.; Merati, G.; Meriggi, P.; Parati, G.; Faini, A.; Rizzo, F. Wearable seismocardiography: Towards a beat-by-beat assessment of cardiac mechanics in ambulant subjects. Auton. Neurosci. 2013, 178, 50–59. [Google Scholar] [CrossRef] [PubMed]
- Zanetti, J.M.; Tavakolian, K. Seismocardiography: Past, present and future. In Proceedings of the 2013 35th Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBC), Osaka, Japan, 3–7 July 2013; pp. 7004–7007. [Google Scholar]
- Lam, T.; Nagappa, M.; Wong, J.; Singh, M.; Wong, D.; Chung, F. Continuous pulse oximetry and capnography monitoring for postoperative respiratory depression and adverse events: A systematic review and meta-analysis. Anesth. Analg. 2017, 125, 2019–2029. [Google Scholar] [CrossRef] [PubMed]
- Edgerton, J.R.; Mahoney, C.; Mack, M.J.; Roper, K.; Herbert, M.A. Long-term monitoring after surgical ablation for atrial fibrillation: How much is enough? J. Thorac. Cardiovasc. Surg. 2011, 142, 162–165. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- De Oliveira, A.C.T.; Martinez, D.; Vasconcelos, L.F.T.; Gonçalves, S.C.; do Carmo Lenz, M.; Fuchs, S.C.; Gus, M.; de Abreu-Silva, E.O.; Moreira, L.B.; Fuchs, F.D. Diagnosis of obstructive sleep apnea syndrome and its outcomes with home portable monitoring. Chest 2009, 135, 330–336. [Google Scholar] [CrossRef] [Green Version]
- Klum, M.; Leib, F.; Oberschelp, C.; Martens, D.; Pielmus, A.G.; Tigges, T.; Penzel, T.; Orglmeister, R. Wearable Multimodal Stethoscope Patch for Wireless Biosignal Acquisition and Long-Term Auscultation. In Proceedings of the 2019 41st Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBC), Berlin, Germany, 23–27 July 2019; pp. 5781–5785. [Google Scholar]
- Pan, J.; Tompkins, W.J. A real-time QRS detection algorithm. IEEE Trans. Biomed. Eng. 1985, BME-32, 230–236. [Google Scholar] [CrossRef]
- Varghees, V.N.; Ramachandran, K. Effective heart sound segmentation and murmur classification using empirical wavelet transform and instantaneous phase for electronic stethoscope. IEEE Sens. J. 2017, 17, 3861–3872. [Google Scholar] [CrossRef]
- Langley, P.; Bowers, E.J.; Murray, A. Principal component analysis as a tool for analyzing beat-to-beat changes in ECG features: Application to ECG-derived respiration. IEEE Trans. Biomed. Eng. 2009, 57, 821–829. [Google Scholar] [CrossRef] [Green Version]
- Klum, M.; Tigges, T.; Pielmus, A.; Feldheiser, A.; Orglmeister, R. Impedance Plethysmography for Respiratory Flow and Rate Estimation using Multilayer Perceptrons. Int. J. Bioelectromagn. 2019, 21, 34–47. [Google Scholar]
- Selvaraj, N. Long-term remote monitoring of vital signs using a wireless patch sensor. In Proceedings of the 2014 IEEE Healthcare Innovation Conference (HIC), Seattle, WA, USA, 8–10 October 2014; pp. 83–86. [Google Scholar]











| Feature Class | Source Signals | Feature Symbol | Description |
|---|---|---|---|
| Timing | ECG | Heart rate | |
| Timing | PCG | Left ventricular ejection time | |
| Timing | ECG, PCG | Pre-ejection period | |
| Area | ECG | QRS complex area | |
| Area | PCG | S1 area | |
| Area | PCG | S2 area | |
| Amplitude | ECG | QRS complex amplitude | |
| Amplitude | PCG | S1 amplitude | |
| Amplitude | PCG | S2 amplitude | |
| Morphology | ECG | Morphological variations of the QRS complex | |
| Morphology | PCG | Morphological variations of the S1 peak | |
| Morphology | PCG | Morphological variations of the S2 peak |
| Target ECG Lead | Model | MEV | MAEV | r | NMSE% |
|---|---|---|---|---|---|
| Einthoven I | Poly | ||||
| Einthoven I | MLP | ||||
| Einthoven I | TDNN | ||||
| Einthoven II | Poly | ||||
| Einthoven II | MLP | ||||
| Einthoven II | TDNN |
| Position | STI | MEms | MAEms | MAPE% |
|---|---|---|---|---|
| Lateral | PEP | |||
| LVET |
| Position | rflow | MEbpm | MAEbpm | rRR | MAPE% | Outliers% |
|---|---|---|---|---|---|---|
| supine | ||||||
| lateral | ||||||
| prone | ||||||
| all |
| Position | rflow | MEbpm | MAEbpm | rRR | MAPE% | Outliers% |
|---|---|---|---|---|---|---|
| supine | ||||||
| lateral | ||||||
| prone | ||||||
| all |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Klum, M.; Urban, M.; Tigges, T.; Pielmus, A.-G.; Feldheiser, A.; Schmitt, T.; Orglmeister, R. Wearable Cardiorespiratory Monitoring Employing a Multimodal Digital Patch Stethoscope: Estimation of ECG, PEP, LVET and Respiration Using a 55 mm Single-Lead ECG and Phonocardiogram. Sensors 2020, 20, 2033. https://doi.org/10.3390/s20072033
Klum M, Urban M, Tigges T, Pielmus A-G, Feldheiser A, Schmitt T, Orglmeister R. Wearable Cardiorespiratory Monitoring Employing a Multimodal Digital Patch Stethoscope: Estimation of ECG, PEP, LVET and Respiration Using a 55 mm Single-Lead ECG and Phonocardiogram. Sensors. 2020; 20(7):2033. https://doi.org/10.3390/s20072033
Chicago/Turabian StyleKlum, Michael, Mike Urban, Timo Tigges, Alexandru-Gabriel Pielmus, Aarne Feldheiser, Theresa Schmitt, and Reinhold Orglmeister. 2020. "Wearable Cardiorespiratory Monitoring Employing a Multimodal Digital Patch Stethoscope: Estimation of ECG, PEP, LVET and Respiration Using a 55 mm Single-Lead ECG and Phonocardiogram" Sensors 20, no. 7: 2033. https://doi.org/10.3390/s20072033
APA StyleKlum, M., Urban, M., Tigges, T., Pielmus, A. -G., Feldheiser, A., Schmitt, T., & Orglmeister, R. (2020). Wearable Cardiorespiratory Monitoring Employing a Multimodal Digital Patch Stethoscope: Estimation of ECG, PEP, LVET and Respiration Using a 55 mm Single-Lead ECG and Phonocardiogram. Sensors, 20(7), 2033. https://doi.org/10.3390/s20072033






