The Preisach model, originally developed in 1935 to describe hysteresis in ferromagnetism, was named after its author [
1]. This model represents the mapping of the input data functions into the output data function. It is based on the elementary nonlinear hysteresis operator
Gα,β, which is a discontinuous operator with local memory. However, by superposing these operators within the domain
Γ, the Preisach hysteresis operator
is formed as a continuous system of infinitely many elementary operators connected in parallel (or in series):
where
represents the Preisach weight function, according to which the elementary operators are arranged, and
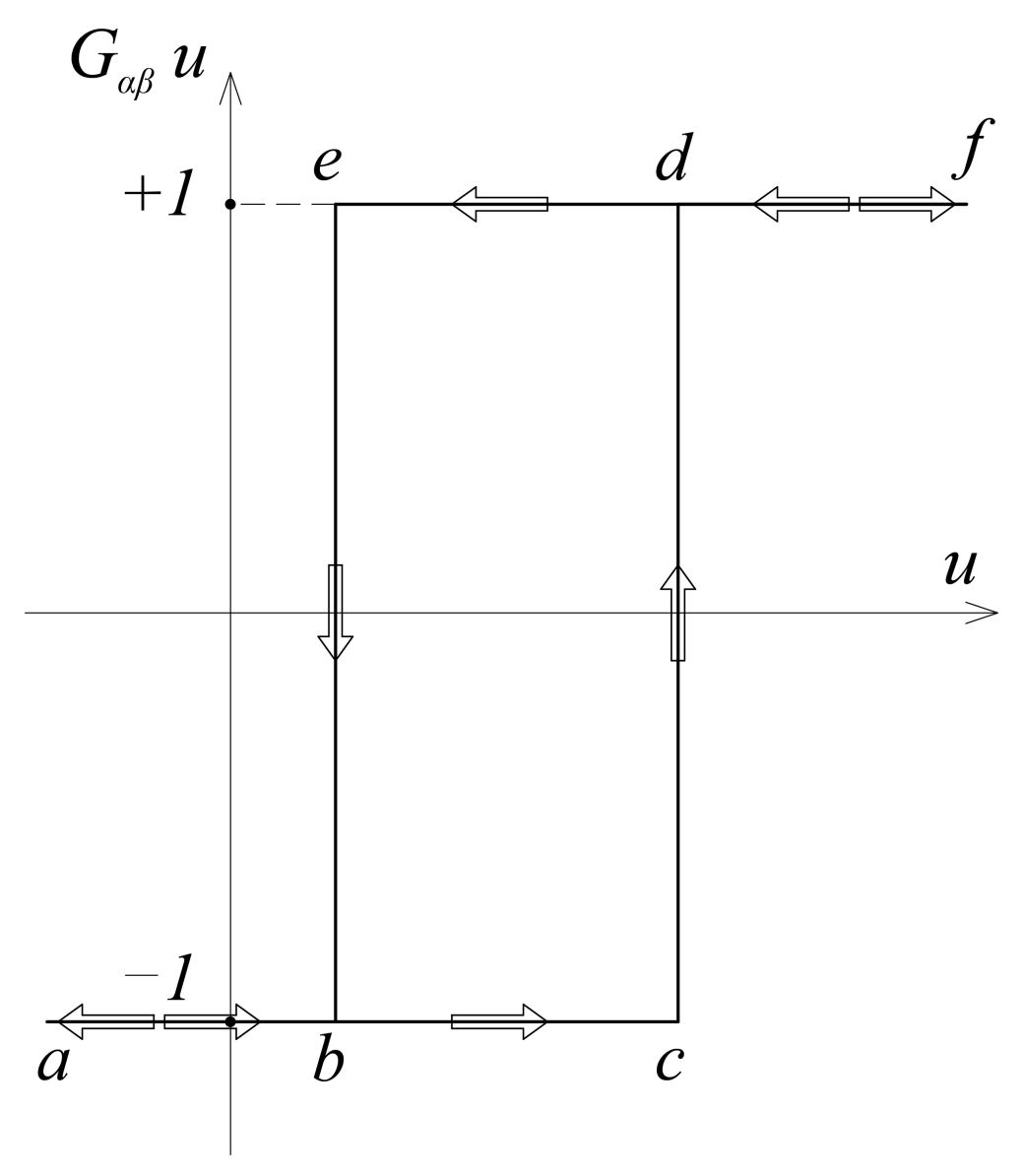
Gα,β is the elementary hysteresis operator shown in
Figure 1.
Structural mild steel behavior under monotonic loading is characterized by a phenomenon called the Lüders band, whose main feature is the development of a horizontal yield plateau. Those phenomena occur during the transition from the elastic region to the region of nonlinear plastic hardening.
This local instability is characteristic only of monotonic loading, while the horizontal yield plateau disappears due to reversible loading.
2.1. Single Crystal Preisach Model
In this paper, a new type of Preisach model for response of structural mild steel under constant cyclic loading will be developed. Its basis is a model that describes the behavior of this type of steel under monotonic loading [
7].
The behavior of the steel S275, under monotonic loading, is characterized by an unstable elastoplastic transition which reduces their workability and ductility. This phenomenon is a result of the separation of the free atoms (usually carbon or nickel) and their incorporation at the sites of existing and newly formed dislocations within the lattice formed by atoms of iron [
12].
The initial part of the stress–strain diagram of these steel is linear and proportional to the modulus of elasticity
E until the stress
σuy is reached This limit is called the upper yield stress. Reaching a given value is succeeded by a sudden drop in stress to the value of the lower yield point
σly. This is followed by the formation of a yield plateau, with an approximate magnitude of 1–3% of the total dilatation, according to [
13]. The cause of this behavior of the mentioned steel lies in the microstructure and atomic lattice. Free carbon and nitrogen atoms surround the dislocation, causing a high level of stress required to move it within the crystal lattice
σuy. After initiating the dislocation, its motion continues relatively easily, forming a yield plateau, until a new regrouping of atoms within the crystal lattice occurs.
This phenomenon is called the Lüders band, and the length of the yield plateau is the Lüder dilatation
εL. The Lüders band represents a local, inhomogeneous deformation, which causes the development of a yield plateau until the Lüders band spreads over the entire sample, followed by material hardening and homogeneous deformation (
Figure 2).
The upper yield stress value σuy, of the mild construction steel, is very sensitive to small stress concentrations, alignment of the sample inside the machine jaws, as well as to other parameters. For this reason, the upper yield strength is neglected, and the value of the stress at which the transition from the elastic to the plastic region occurs is taken to be the value of the lower yield strength σly.
The basic principle in modeling elastoplastic material behavior is based on defining an analog mechanical model, determined by an appropriate set of algebraic and/or differential equations. A material model, describing the response of the subject steel under monotonic axial stress, is constructed by modifying the existing three-element model [
3,
4,
5,
6]. By introducing a delay element, the delay of material hardening is achieved after reaching the yield strength
Y =
σly.
The concavity of the
σ-
ε curve in the hardening zone is achieved by its approximation with three lines, which makes the working diagram quintuple linear. This is achieved by introducing additional elements into the three linear mechanical model, as shown in the
Figure 3.
The material properties of the mechanical model, shown in
Figure 3b, are defined by Equation (2).
It is possible to define a new hysteresis mechanical model based on the working diagram shown in
Figure 3, which describes the behavior of a structural mild steel single crystal under monotonic loading:
And its appropriate Preisach function:
The formation of a horizontal yield plateau and a phenomenon called the Lüders band represent the characteristic behavior of mild steels under monotonic loading [
14]. Under the first load direction switch, a vanishing of the yield plateau occurs, with a complete transformation of the material behavior into an ideal elastoplastic behavior with hardening.
The cyclic behavior of the examined steel types is characterized by the formation of regular hysteresis loops, with no flattening. A new hysteresis model is established by excluding the yield plateau and modifying the material parameters.
A satisfactory approximation of hysteresis loops is possible using a three-element model [
3]. In order to better match the real response of the material, a five-element model with a trilinear working diagram, shown in
Figure 4, was used in this paper.
The material properties of the mechanical model, shown in
Figure 4b, are defined by Equation (2), where substitution should be made:
It is possible to define a new hysteresis mechanical model based on the working diagram shown in
Figure 4, which describes the behavior of a structural mild steel single crystal under cyclic loading:
By switching to the single integral, where the integration of the first part on the right hand side of Equation (6) takes part along line
, second along line
, and third along line
Equation (6) for stress becomes:
The first integral of Equation (7) represents the stress due to elastic deformation. The second and third addends describe the behavior of the material after reaching the yield strengths and , respectively.
2.2. Polycrystal Preisach Model
To model the real polycrystalline material, such as mild steel S275, parallel or series connections of infinitely many single crystal elements (
Figure 5) are exploited as in [
15]. In this paper we are using parallel connections of monocrystalline elements with the range of the yield stress
Yimin ≤ Yi ≤ Yimax. Then the stress in the polycrystalline element reads:
In Equation (8)
p(Yi) is the yield strength probability density function. Assuming that the yield limits
Y1 are the same in all parallel-connected individual units and defining that the distribution functions of other
Yi values are uniform, as in papers [
3,
4,
5,
6,
7].
The total stress, due to strain as an input, becomes:
Since the first addend of Equation (10) does not depend on
, on the basis of the second it is
, and on the basis of the third
. The term
β can be reintroduced into the equation, with shifts
, and
, where the negative sign of the shift is lost due to the change of integration boundaries within triangles:
The integration domains in Equation (11) represent the areas of the bands between the corresponding lines in a bounded triangle (
Figure 6), because the Preisach function exists only in these domains and otherwise is zero. Domain A′ represents the area between the lines
, and
, while domain B′ represents the area between the lines
and
.
The Preisach function for polycrystalline internal hysteresis loops is defined as:
The geometric interpretation of Equation (12) represents the Preisach triangle shown in
Figure 6.
2.3. Wiping Out and Congruency Properties of Preisach Model
Using the geometric interpretation of the Preisach model, the calculation of the double integral in Equation (11) can be avoided. Determination of the integral value comes down to the estimation of the area inside the Preisach triangle. Observing the elementary operators values, it can be stated that at any time, the Preisach triangle consists of points at which elementary operators are at a “switch on” position and points at which they are at a “switch off” position. It can be noticed that the value of the output, at some arbitrary moment, depends on the division of the boundary triangle into positive and negative sets A+ and A−. Increasing and decreasing of the input leads to a change in this redistribution and the formation of a staircase line L(t), which represents the boundary between these sets.
The vertices of the staircase line are the extreme values of the input data so the L(t) line represents the memory of this operator. The memory formation in Preisach model is achieved by changing the shape of the staircase line L(t).
The wiping-out property of Preisach model can be described through its geometric interpretation. This phenomenon characteristic is that each local maximum erases the vertices of the line
L(t) whose
α coordinates are below that maximum, and each local minimum erases vertices whose
β coordinates are above that minimum (
Figure 7). Only the alternative values of the dominant extremes of the input data are stored in the Preisach model, while all others are deleted.
The Preisach model, therefore, possesses selective memory. The elementary hysteresis operator (
Figure 1) has local memory, but by combining a large number of these operators, non-local model memory is achieved. This memory does not depend on the entire load history, but only on the dominant values of the input extremes, due to the wiping-out property.
Congruence is another fundamental property of the Preisach model, which can be easily demonstrated through the geometric interpretation of the model (
Figure 8). If we observe two states, defined by Preisach triangles
T1 and
T2, in which the input varies in the same range, it can be noticed that the changes in the areas of the triangles are equal in both cases (Δ
T1 = Δ
T2), regardless of previous load histories. It follows that the output increments for two states with different previous load histories are mutually equal Δ
f1 = Δ
f2. The consequence of this property is that all inner loops, created by varying the input between the same dominant extremes, have the same shape and size, but a different position within the main loop, which depends on the previous load history. The complete matching of these loops could be achieved by their translation in the
f-axis direction.
The wiping-out and congruence properties represent necessary and sufficient conditions for hysteresis nonlinearity to be defined by the Preisach model [
16]. These phenomena are essential for further consideration of energy losses achieved during random load histories.
2.4. Heat Loses
The term hysteresis is mainly related to the appearance of the hysteresis loop. However, hysteresis is also associated with loss of energy manifested through energy losses. Energy losses accomplished during the formation of hysteresis loops were first considered in electromagnetism. The hysteresis energy losses in magnetism are defined by Charles Proteus Steinmetz as the surface of the hysteresis loop [
17].
Thanks to this observation, the determination of hysteresis losses at cyclic loads is based on the principle of energy conservation. The analysis of the energy consumed in the formation of hysteresis is important for the field of continuum mechanics.
Determination of energy losses during the formation of hysteresis loops, for an arbitrary history of excitation, can be performed on the basis of Preisach hysteresis operator and its weight function
μ(α, β). The general solution was defined by Mayergoyz [
16], while the analysis of energy dissipation in the plastic domain for a three-element model of materials is presented in the paper [
3].
The overall plastic work
Wp, under cyclic loading, is spent on thermal changes
S, and the energy remains trapped inside the material [
4], called locked-in energy
WL.
If the heat losses
S are defined using the Preisach hysteresis model, the total loss can be obtained as the sum of the losses in elementary hysteresis operators. The realized loss in one elementary hysteresis operator
Gα,β, at full cycle, as shown in
Figure 1, is:
while the energy loss during one input change (switch up or switch down) is:
Since the product
μ(
α,
β) ×
Gα,
β can be considered as a rectangular loop with an output value ±
μ (
α,
β), the energy loss in such a loop, during one input change, is defined as the product
μ(
α,
β) (
α −
β). Whereas the Preisach hysteresis model is a set of a large (infinite) number of elementary hysteresis operators, the total energy losses are obtained by summing the energy losses of all elementary hysteresis operators. This is achieved by integration over the domain Ω where the value of the input within the Preisach triangle has changed. Energy losses at the cross-sectional level are then defined as:
The double integral in Equation (16) is determined as the volume with basis area Ω in the
α −
β plane and height in the z-direction with the value of (
α −
β). From Equation (16), to find the fraction of the plastic work dissipated into heat, one should integrate the Preisach function for a chosen model within the area of the limiting triangle. Following the procedure explained in [
3] for a parallel connection of infinitely many slip and sliding elements connected in series one obtains:
For mild steel material constants that are used in the above equation are: E = 210 GPa, Ymin = 16 KN/cm2, σ = Ymax = 2 Ymin = 32 KN/cm2 and εp = 1.25%.
Then from Equation (14) it is obtained for one monotonic cycle:
One can easily calculate, following the procedure given in [
3], the amount of plastic work
Wp as:
Finally, the difference is:
This represents the locked-in energy stored during the primary loading from initial, zero state stress to the state of stress Ymin ≤ σ ≤ Ymax.