Locating Ships Using Time Reversal and Matrix Pencil Method by Their Underwater Acoustic Signals
Abstract
:1. Introduction
2. Background
2.1. Matrix Pencil Method
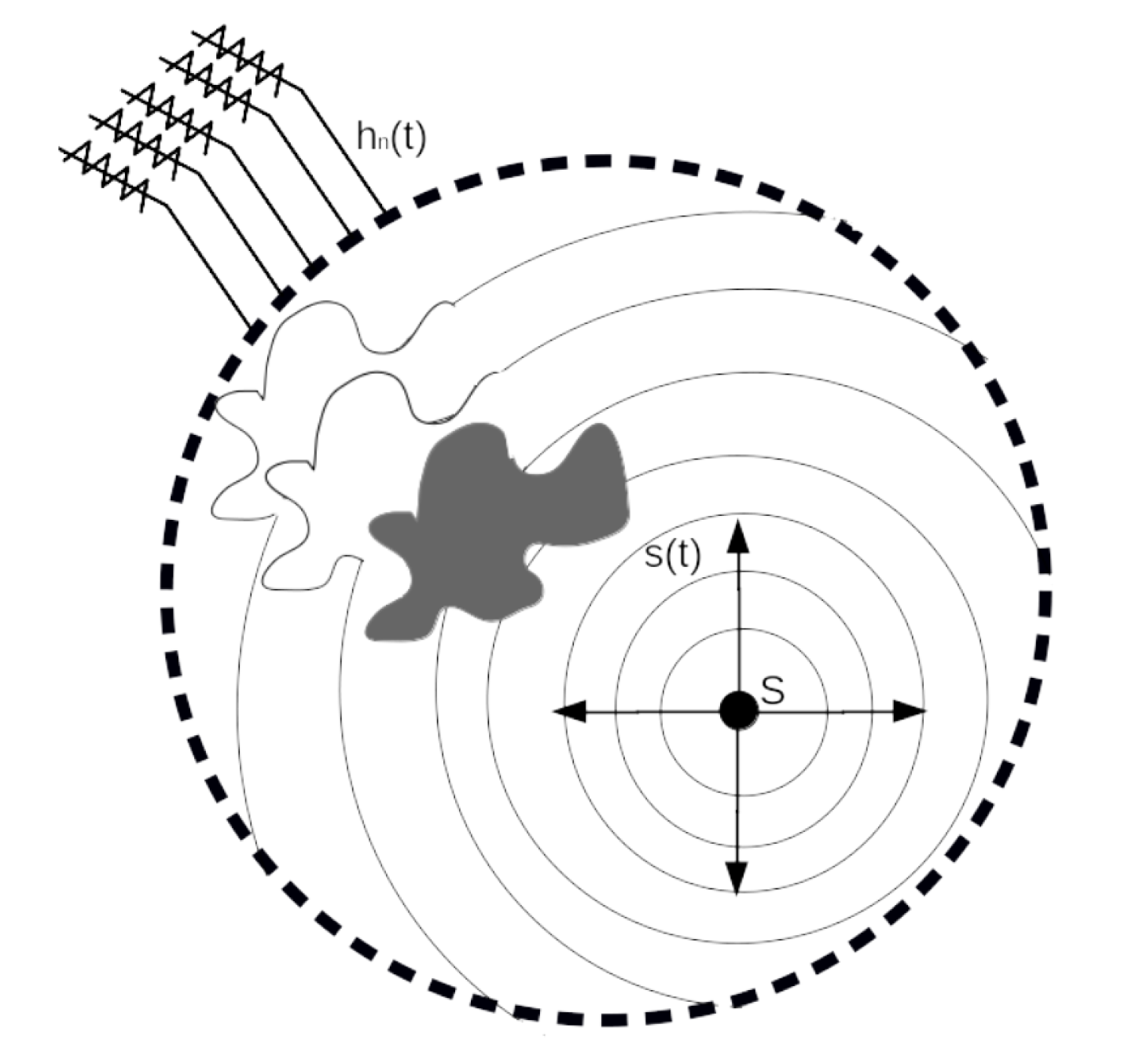
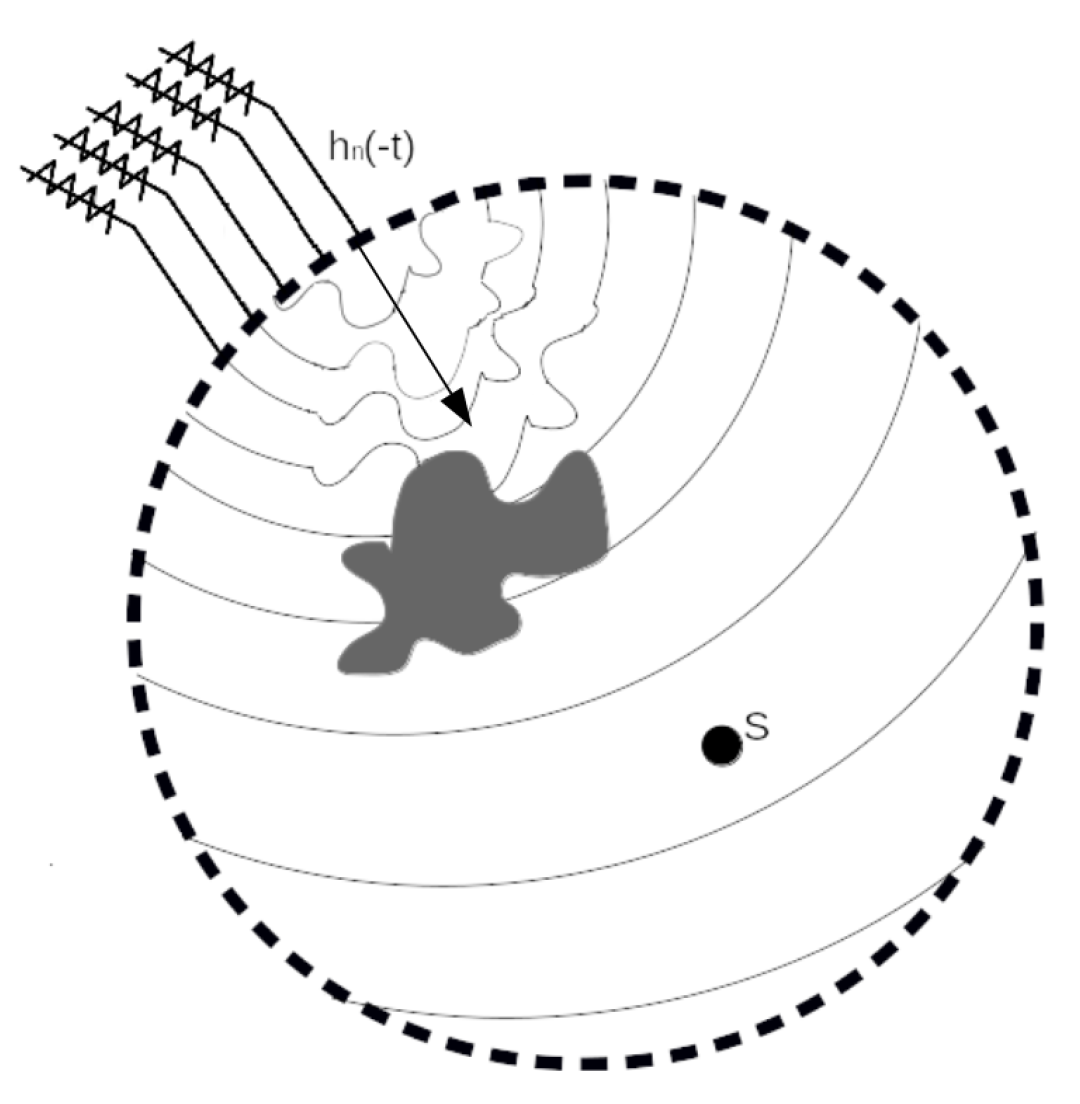
2.2. Time Reversal Method
3. Proposed Method
3.1. Matrix Pencil Method
3.1.1. Signal Test
3.1.2. Definition of M
3.2. Time Reversal Method
4. Method Validation
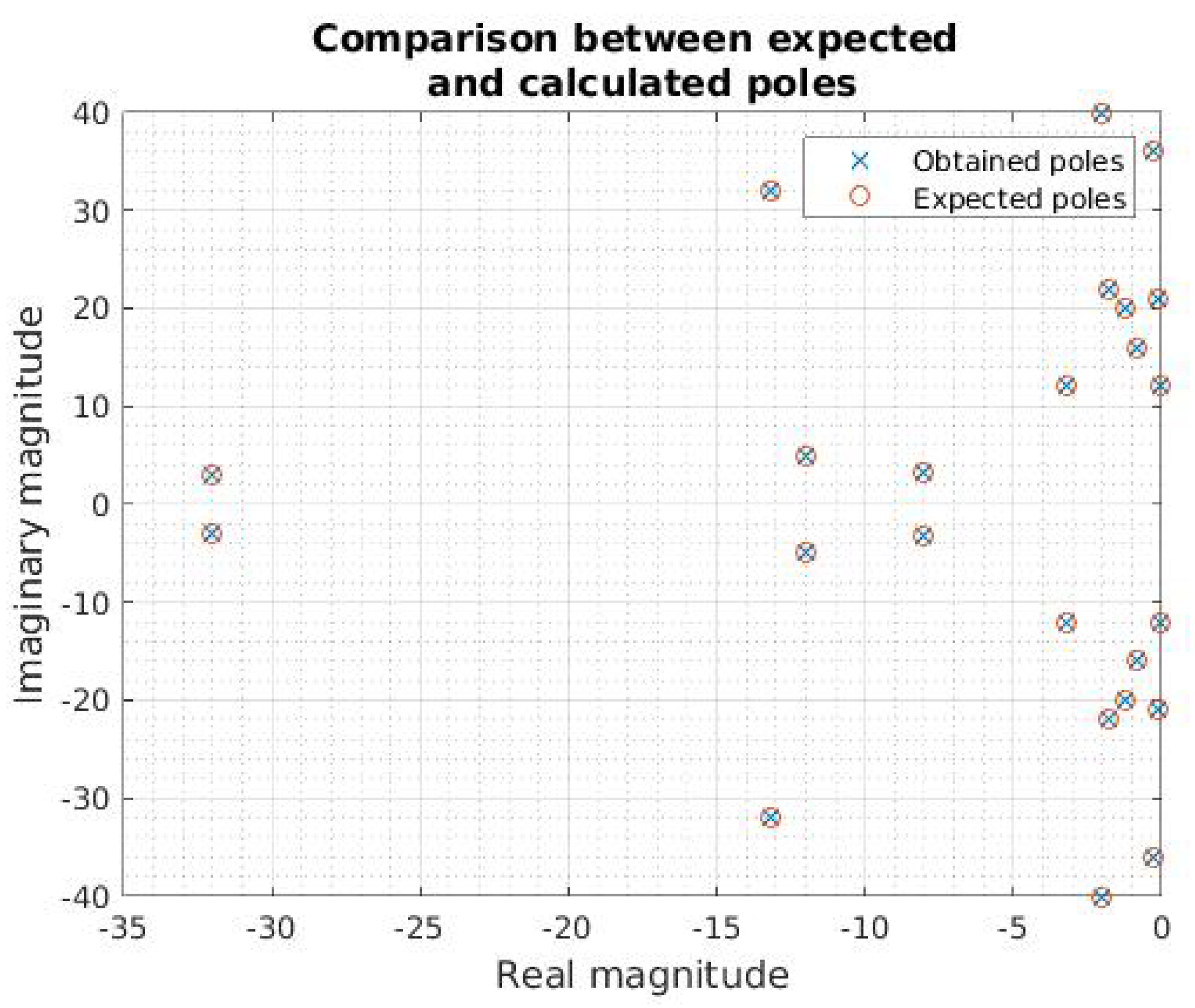
4.1. Data Reduction Produced by MPM
4.2. Location Simulation Process
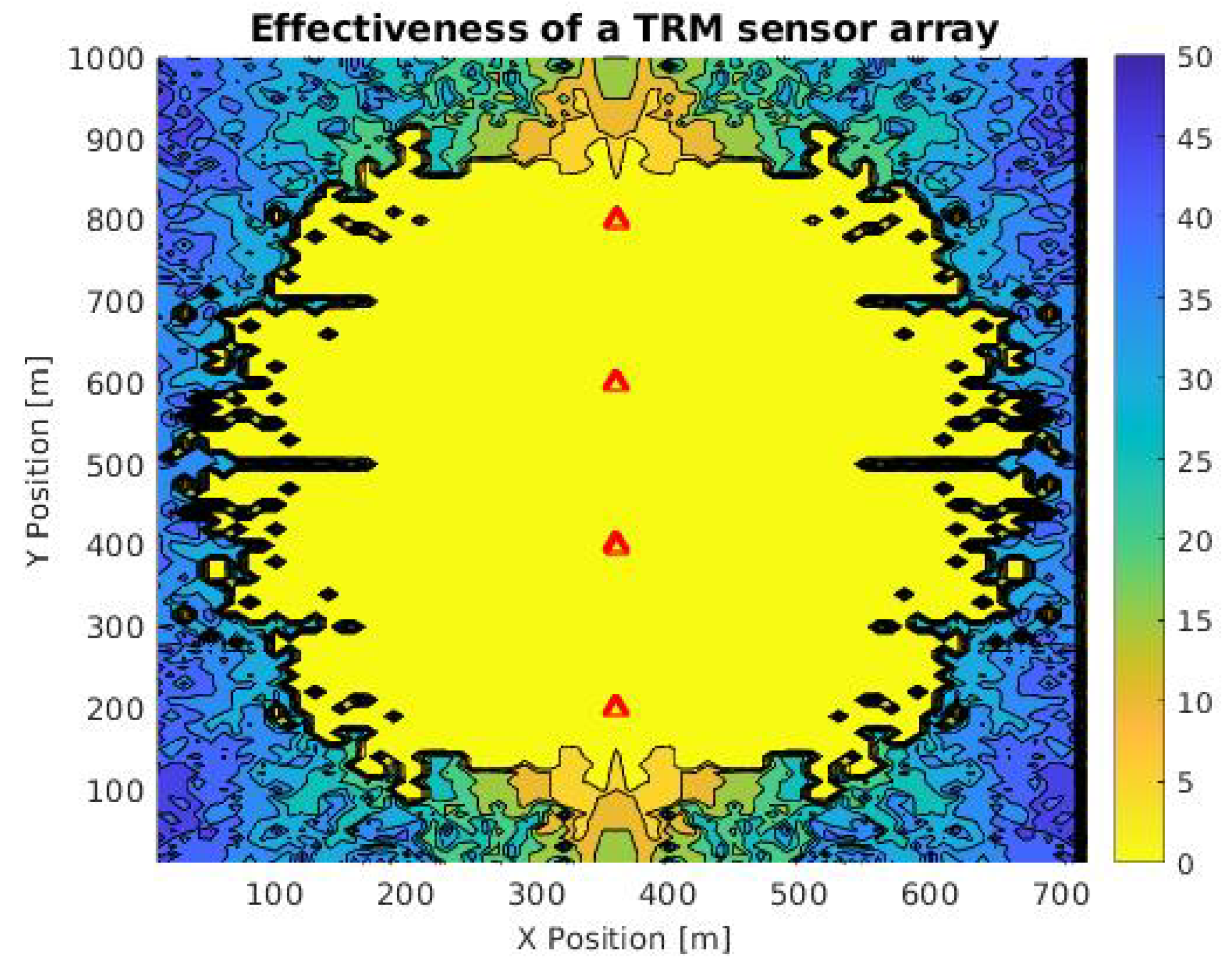
4.2.1. Simulation Analysis Derived by the Change in the Distribution of the Sensors
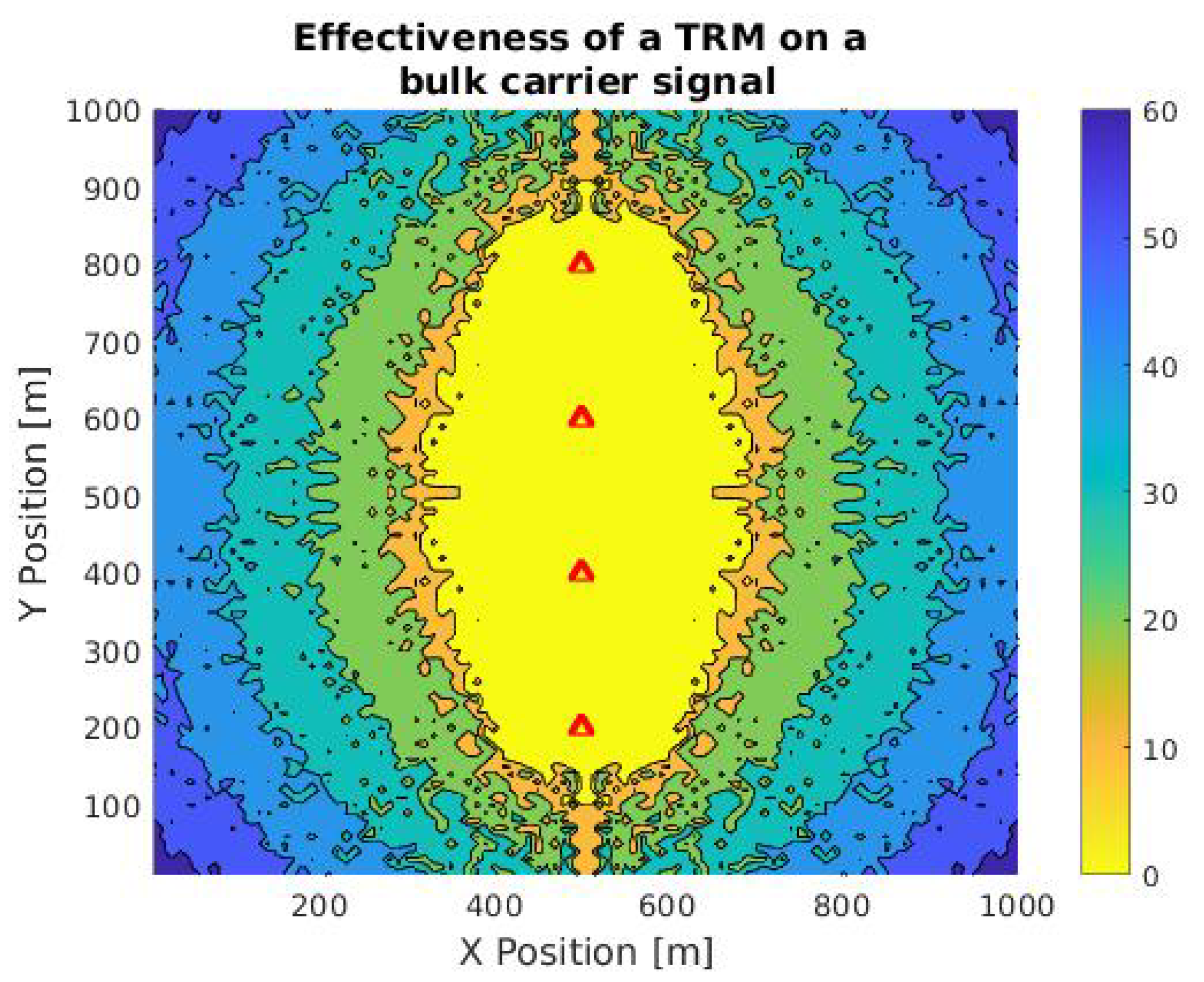
4.2.2. Simulation Analysis Derived by the Change in Signal of Interest
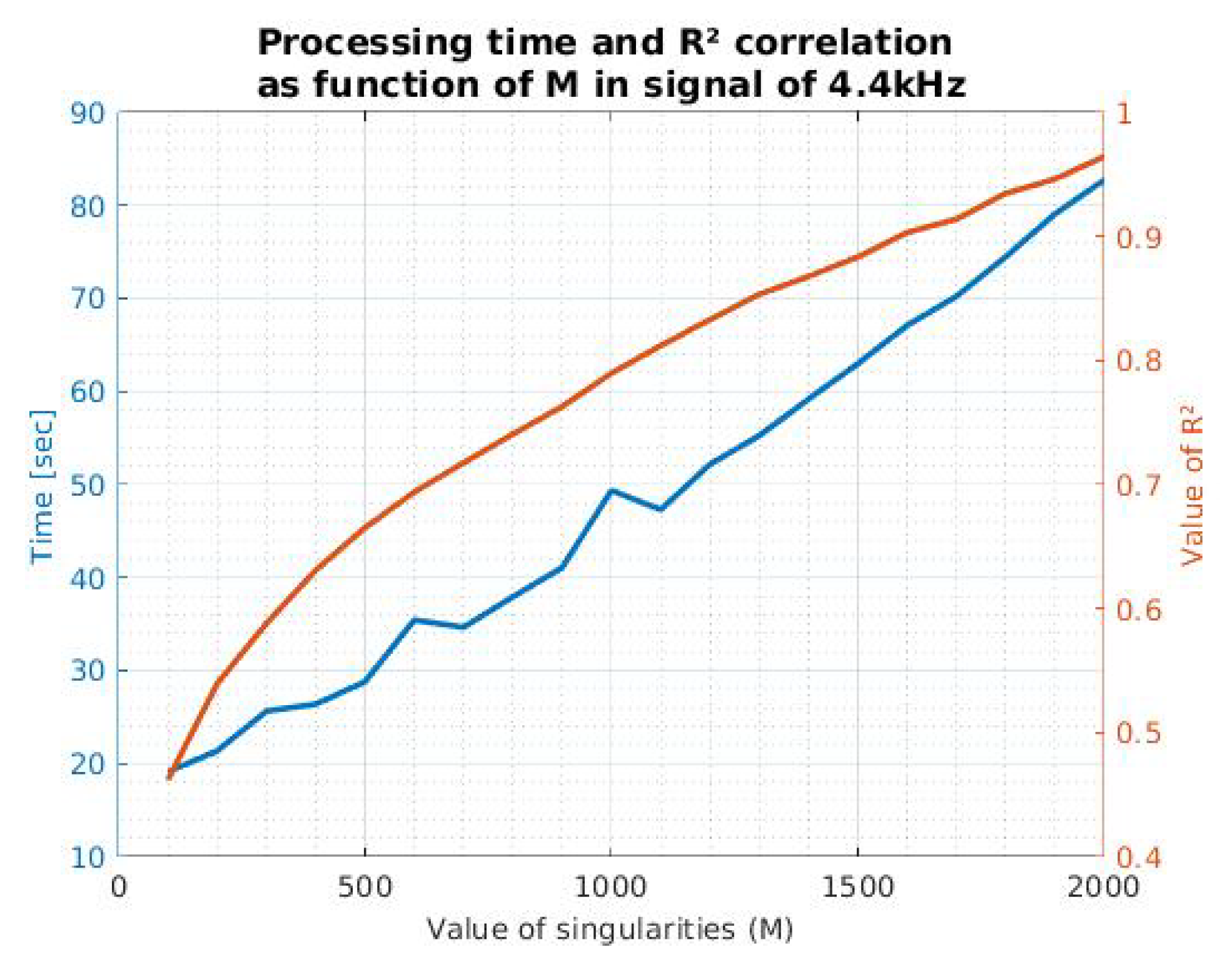
4.2.3. Simulation Analysis of the Signals Treated with MPM
4.2.4. Simulation Analysis of Signals Treated with MPM, Including Noise Levels
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Baum, C.E. The Singularity Expansion Method: Background and Develompents. IEEE Antennas Propag. Soc. Newsl. 1986, 28, 14–23. [Google Scholar] [CrossRef]
- Sarkar, T.K.; Pereira, O. Using the Matrix Pencil Method to Estimate the Parameters of a Sum of Complex Exponentials. IEEE Antennas Propag. Mag. 1995, 37. [Google Scholar] [CrossRef] [Green Version]
- Caboussat, A.; Miers, G.K. Numerical approximation of electromagnetic signals arising in the evaluation of geological formations. Comput. Math. Appl. 2010, 59, 338–351. [Google Scholar] [CrossRef] [Green Version]
- Lee, W.; Sarkar, T.K.; Moon, H.; Salazar-Palma, M. Identification of multiple objects using their natural resonant frequencies. IEEE Antennas Wirel. Propag. Lett. 2013, 12, 54–57. [Google Scholar] [CrossRef]
- Bannawat, L.; Boonpoonga, A.; Burintramart, S. Performance evaluation of target identification using matrix pencil method in different frequency range. In Proceedings of the 2014 International Electrical Engineering Congress (iEECON), Pattaya, Thailand, 19–21 March 2014; pp. 1–4. [Google Scholar]
- Ma, X.; Zhou, Z.; Liu, K.; Zhang, J.; Raza, W. Poles Extraction of Underwater Targets Based on Matrix Pencil Method. IEEE Access 2020, 8, 103007–103019. [Google Scholar] [CrossRef]
- Fink, M.; Prada, C.; Wu, F.; Cassereau, D. Self focusing in inhomogeneous media with ’time reversal’ acoustic mirrors. Ultrason. Symp. Proc. 1989, 2, 681–686. [Google Scholar] [CrossRef]
- Mora, N.; Rachidi, F.; Rubinstein, M. Application of the time reversal of electromagnetic fi elds to locate lightning discharges. Atmos. Res. 2012, 117, 78–85. [Google Scholar] [CrossRef]
- Panagiotopoulos, C.G.; Stavroulakis, G.E. A Numerical Study on Computational Time Reversal for Structural Health Monitoring. Signals 2021, 2, 225–244. [Google Scholar] [CrossRef]
- Hamill, D.C. Time Reversal Duality Between Linear Networks. IEEE Trans. Circuits Syst. I Fundam. Theory Appl. 1996, 43, 63–65. [Google Scholar] [CrossRef]
- Arriba-ruiz, I.D.; Muñoz-ferreras, J.M.; Pérez-martínez, F. Multipath mitigation techniques based on time reversal concept and superresolution algorithms for inverse synthetic aperture radar imaging. IET Radar Sonar Navig. 2013, 7, 413–421. [Google Scholar] [CrossRef]
- Li, C.X.; Xu, W.; Member, S.; Li, J.L.; Gong, X.Y. Time-Reversal Detection of Multidimensional Signals in Underwater Acoustics. IEEE J. Ocean. Eng. 2011, 36, 61–71. [Google Scholar] [CrossRef]
- Zhao, A.; Zeng, C.; Hui, J.; Wang, K.; Tang, K. Study on time reversal maximum ratio combining in underwater acoustic communications. Appl. Sci. 2021, 11, 1509. [Google Scholar] [CrossRef]
- Fu, Y.; Yu, Z. A Low SNR and Fast Passive Location Algorithm Based on Virtual Time Reversal. IEEE Access 2021, 9, 29303–29311. [Google Scholar] [CrossRef]
- Eaton, R.S.; German, S.; Balasuriya, A. Maritime Border Security using Sensors, Processing, and Platforms to Detect Dark Vessels. In Proceedings of the 2018 IEEE International Symposium on Technologies for Homeland Security, HST 2018, Woburn, MA, USA, 23–24 October 2018. [Google Scholar] [CrossRef]
- Lin, Y.C.; Chen, H.J.; Chung, W.H.; Lee, T.S. Geometric approach to passive ranging in underwater localization systems. IEEE Access 2018, 6, 54018–54032. [Google Scholar] [CrossRef]
- Frasier, K.E.; Garrison, L.P.; Soldevilla, M.S.; Wiggins, S.M.; Hildebrand, J.A. Cetacean distribution models based on visual and passive acoustic data. Sci. Rep. 2021, 11, 1–16. [Google Scholar] [CrossRef]
- Zurek, E.; Gamarra, M.R.; Escorcia, J.R.; Gutierrez, C.; Bayona, H.; Pérez, R.P.; García, X. Using Artificial Neural Networks. Polibits 2014, 1, 69–73. [Google Scholar] [CrossRef]
- Golen, E.F.; Mishra, S.; Shenoy, N. An underwater sensor allocation scheme for a range dependent environment. Comput. Netw. 2010, 54, 404–415. [Google Scholar] [CrossRef]
- Samuel, F.; Bhavani, R.G. GPS based system for detection and control of maritime boundary intruding boats. Midwest Symp. Circuits Syst. 2016, 16–19. [Google Scholar] [CrossRef]
- Hu, L.; Wang, X.; Wang, S. Decentralized Underwater Target Detection and Localization. IEEE Sens. J. 2021, 21, 2385–2399. [Google Scholar] [CrossRef]
- Satheesh, C.; Kamal, S.; Mujeeb, A.; Supriya, M.H. Passive Sonar Target Classification Using Deep Generative β-VAE. IEEE Signal Process. Lett. 2021, 28, 808–812. [Google Scholar] [CrossRef]
- Mazurek, G.; Kulpa, K.; Malanowski, M.; Droszcz, A. Experimental seaborne passive radar. Sensors 2021, 21, 2171. [Google Scholar] [CrossRef]
- Hotkani, M.M.; Seyedin, S.A. Estimating the Position of Underwater Targets Based on the Emission of Noise Caused by Spinning the Propeller of Surface Vessels. In Proceedings of the 2019 6th Iranian Conference on Radar and Surveillance Systems, ICRSS 2019, Isfahan, Iran, 20–22 November 2019; pp. 2–6. [Google Scholar] [CrossRef]
- Jamalabdollahi, M.; Zekavat, S.A.; Tech, M. Positioning in Inhomogeneous Media. In Handbook of Position Location; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2018; Chapter 10; pp. 343–366. [Google Scholar] [CrossRef]
- Chaparro-arce, D.; Gallego, A.; Albarracin-Vargas, F.; Gutierrez, C.; Vega, F.; Pedraza, C. Matrix Pencil Method applied to the compression of audio data in naval operations. In Proceedings of the IEEE International Conference on Computational Electromagnetics, Singapore, 24–26 August 2020; pp. 1–3. [Google Scholar]
- Sarkar, T.K.; Salazar-Palma, M.; Mokole, E.L. Application of the principle of analytic continuation to interpolate/extrapolate system responses resulting in reduced computations-Part A: Parametric methods. IEEE J. Multiscale Multiphysics Comput. Tech. 2016, 1, 48–59. [Google Scholar] [CrossRef]
- Hua, Y.; Sarkar, T.K. Matrix Pencil Method for Estimating Parameters of exponetially damped. IEEE Trans. Acoust. Speech Signal Process. 1990, 38, 814–824. [Google Scholar] [CrossRef] [Green Version]
- Fink, M. Time Reversal of Ultrasonic Fields-Part. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 1992, 39, 555–566. [Google Scholar] [CrossRef]
- Zhang, D. A Coefficient of Determination for Generalized Linear Models. Am. Stat. 2016, 71, 1–20. [Google Scholar] [CrossRef]
- McKenna, M.F.; Ross, D.; Wiggins, S.M.; Hildebrand, J.A. Underwater radiated noise from modern commercial ships. J. Acoust. Soc. Am. 2012, 131, 92–103. [Google Scholar] [CrossRef] [Green Version]
- Cabrera, F.; Molina, N.; Tichavska, M.; Araña, V. Automatic Identification System modular receiver for academic purposes. Radio Sci. 2016, 51, 1038–1047. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.; Zhang, M.; Li, L.; Li, G.; Chen, Y.; Zhang, X. Research on the real-time positioning method of vector buoys in deep sea. J. Phys. Conf. Ser. 2021, 1812, 012031. [Google Scholar] [CrossRef]
- Chaparro-arce, D.; Gutierrez, S.; Gallego, A.; Gutierrez, C.; Vega, F.; Pedraza, C. Extraction of complex natural resonances of ships acoustic signals using Matrix Pencil Method. In Proceedings of the IEEE Colombian Conference on Comunications and Computing, Cali, Colombia, 7–8 August 2020; pp. 1–4. [Google Scholar]




| M | Compression Percentage (%) | Correlation Coefficient () |
|---|---|---|
| 10 | 99.09 | 0.25 |
| 50 | 97.72 | 0.39 |
| 80 | 96.36 | 0.441 |
| 100 | 95.45 | 0.4715 |
| 200 | 90.909 | 0.5638 |
| 300 | 86.363 | 0.6318 |
| 400 | 81.81 | 0.6922 |
| 500 | 77.305 | 0.756 |
| 600 | 72.727 | 0.803 |
| 700 | 68.182 | 0.842 |
| 800 | 63.687 | 0.8812 |
| 900 | 59.14 | 0.9312 |
| 1000 | 54.54 | 0.955 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chaparro-Arce, D.; Gutierrez, S.; Gallego, A.; Pedraza, C.; Vega, F.; Gutierrez, C. Locating Ships Using Time Reversal and Matrix Pencil Method by Their Underwater Acoustic Signals. Sensors 2021, 21, 5065. https://doi.org/10.3390/s21155065
Chaparro-Arce D, Gutierrez S, Gallego A, Pedraza C, Vega F, Gutierrez C. Locating Ships Using Time Reversal and Matrix Pencil Method by Their Underwater Acoustic Signals. Sensors. 2021; 21(15):5065. https://doi.org/10.3390/s21155065
Chicago/Turabian StyleChaparro-Arce, Daniel, Sergio Gutierrez, Andres Gallego, Cesar Pedraza, Felix Vega, and Carlos Gutierrez. 2021. "Locating Ships Using Time Reversal and Matrix Pencil Method by Their Underwater Acoustic Signals" Sensors 21, no. 15: 5065. https://doi.org/10.3390/s21155065
APA StyleChaparro-Arce, D., Gutierrez, S., Gallego, A., Pedraza, C., Vega, F., & Gutierrez, C. (2021). Locating Ships Using Time Reversal and Matrix Pencil Method by Their Underwater Acoustic Signals. Sensors, 21(15), 5065. https://doi.org/10.3390/s21155065







