Terahertz Time-Domain Spectroscopic Study of Tricalcium Silicate
Abstract
:1. Introduction
2. Materials and Sample Preparation
2.1. C3S and PE
2.2. Sample Preparation
3. THz-TDS System and Data Analysis
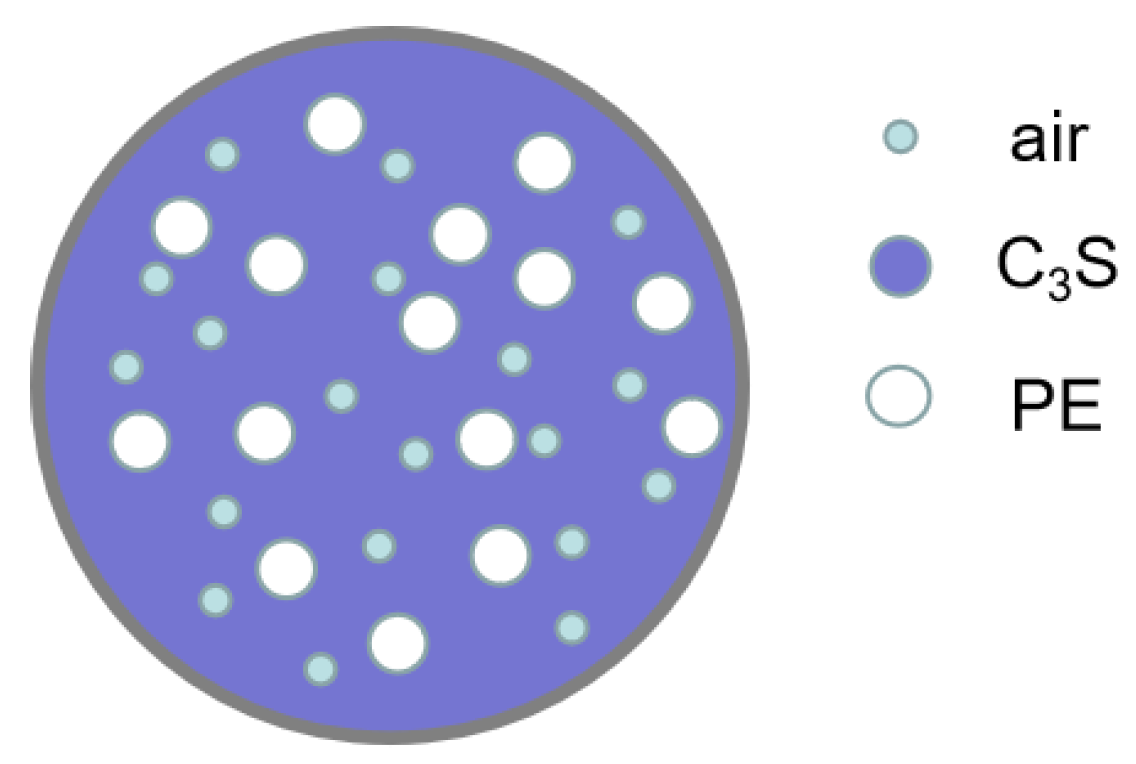
4. Effective Medium Theory
4.1. Maxwell–Garnett (MG) Model
4.2. Bruggeman (BM) Model
4.3. Landau–Lifshitz–Loovenga (LLL) Model
5. Results and Discussions
5.1. THz Absorption Spectra of Different Samples
5.2. THz Dielectric Spectra of Two-Phase Composite Samples
5.3. THz Dielectric Spectra of Three-Phase Composite Samples
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ge, H.; Jiang, Y.; Lian, F.; Zhang, Y.; Xia, S. Characterization of Wheat Varieties Using Terahertz Time-Domain Spectroscopy. Sensors 2015, 15, 12560–12572. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Garg, D.; Bandyopadhyay, A.; Sengupta, A. Critical spectroscopic considerations towards reliable detection of material using terahertz time-domain spectroscopy. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2022, 268, 120632. [Google Scholar] [CrossRef] [PubMed]
- Xie, L.; Yao, Y.; Ying, Y. The application of terahertz spectroscopy to protein detection: A review. Appl. Spectrosc. Rev. Int. J. Princ. Methods Appl. 2014, 49, 448–461. [Google Scholar] [CrossRef]
- Sakai, S.; Yang, D.; Yasuda, T.; Akiyama, K.; Kuga, T.; Kano, A.; Shiraishi, F.; Amekawa, S.; Ohtsuka, S.; Nakaguchi, K.; et al. Pulsed Terahertz Radiation for Sensitive Quantification of Carbonate Minerals. ACS Omega 2019, 4, 2702–2707. [Google Scholar] [CrossRef] [PubMed]
- Hao, S.; Huang, H.; Ma, Y.; Zhang, Z.; Zheng, Z. Sensitive characterizations of natural dolomite by terahertz time-domain spectroscopy. Opt. Commun. 2020, 456, 124524. [Google Scholar] [CrossRef]
- Zhao, Y.; Liu, X.; Chen, B.; Yang, F.; Zhang, Y.; Wang, P.; Robinson, I. Three-Dimensional Characterization of Hardened Paste of Hydrated Tricalcium Silicate by Serial Block-Face Scanning Electron Microscopy. Materials 2019, 12, 1882. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dolado, J.S.; Goracci, G.; Duque, E.; Martauz, P.; Zuo, Y.; Ye, G. THz Fingerprints of Cement-Based Materials. Materials 2020, 13, 4194. [Google Scholar] [CrossRef] [PubMed]
- Ray, S.; Devi, N.; Dash, J.; Sasmal, S.; Pesala, B. Effect of Nano-Silica Incorporation on Cement Hydration Dynamics Studied Using Terahertz Spectroscopy. In Proceedings of the International Conference on Fibre Optics and Photonics, Kanpur, India, 4–8 December 2016; Optica Publishing Group: Washington, DC, USA, 2016; p. Th3A.88. [Google Scholar]
- Dash, J.; Ray, S.; Nallappan, K.; Sasmal, S.; Pesala, B. Terahertz spectroscopy of concrete for evaluating the critical hydration level. In Terahertz, RF, Millimeter, and Submillimeter-Wave Technology and Applications VII; SPIE: Bellingham, USA, 2014; pp. 79–85. [Google Scholar]
- Li, Z.; Lian, F.Y. Research on Scattering in the THz Quantitative Analysis. Guang Pu Xue Yu Guang Pu Fen Xi 2016, 36, 1666–1669. [Google Scholar] [PubMed]
- Bardon, T.; May, R.K.; Taday, P.F.; Strlic, M. Influence of Particle Size on Optical Constants from Pellets Measured with Terahertz Pulsed Spectroscopy. IEEE Trans. Terahertz Sci. Technol. 2016, 6, 408–413. [Google Scholar] [CrossRef] [Green Version]
- Namkung, H.; Kim, J.; Chung, H.; Arnold, M.A. Impact of pellet thickness on quantitative terahertz spectroscopy of solid samples in a polyethylene matrix. Anal. Chem. 2013, 85, 3674–3681. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.P.; Fan, L.J.; Cui, B.; Chen, G.X.; Zhang, Z.W.; Zhang, C.L. The Study on the Far-FTIR and THz Spectra of Azitromycin Drugs with Different Physical Forms. Guang Pu Xue Yu Guang Pu Fen Xi 2015, 35, 3054–3057. [Google Scholar] [PubMed]
- Calvo-de la Rosa, J.; Locquet, A.; Bouscaud, D.; Berveiller, S.; Citrin, D. Terahertz Permittivity of Pressed ZnO and CuO Powder in Polyethylene Pellets: Effect of Porosity. IEEE Trans. Terahertz Sci. Technol. 2021, 11, 402–407. [Google Scholar] [CrossRef]
- Zhou, J.W.; Arbab, M.H. Effective Debye relaxation models for binary solutions of polar liquids at terahertz frequencies. Phys. Chem. Chem. Phys. 2021, 23, 4426–4436. [Google Scholar] [CrossRef] [PubMed]
- Im, J.; Goo, T.; Kim, J.; Choi, S.; Hong, S.J.; Bahk, Y.M. Detection of Microplastic in Salts Using Terahertz Time-Domain Spectroscopy. Sensors 2021, 21, 3161. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.G. Terahertz Spectroscopic Study of Hardened Cement Paste. Master’s Thesis, Taiyuan University of Technology, Taiyuan, China, 2021. [Google Scholar]
- Scheller, M.; Wietzke, S.; Jansen, C.; Jordens, C.; Lehnhardt, M.; Koch, M. Applications for effective medium theories in the terahertz regime. In Proceedings of the 2009 34th International Conference on Infrared, Millimeter, and Terahertz Waves, Busan, Korea, 21–25 September 2009; IEEE: Piscataway, USA, 2009; pp. 1–2. [Google Scholar]
- Markel, V.A. Introduction to the Maxwell Garnett approximation: Tutorial. J. Opt. Soc. Am. A 2016, 33, 1244–1256. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Scheller, M.; Wietzke, S.; Jansen, C.; Koch, M. Modelling heterogeneous dielectric mixtures in the terahertz regime: A quasi-static effective medium theory. J. Phys. D Appl. Phys. 2009, 42, 065415. [Google Scholar] [CrossRef]
- Su, H.-X.; Zhang, Z.-H.; Zhao, X.-Y.; Li, Z.; Yan, F.; Zhang, H.J.S.; Analysis, S. The Lambert-Beer’s law characterization of formal analysis in Terahertz spectrum quantitative testing. Spectrosc. Spectr. Anal. 2013, 33, 3180–3186. [Google Scholar]
- Qu, F.; Lin, L.; Cai, C.; Dong, T.; He, Y.; Nie, P. Molecular Characterization and Theoretical Calculation of Plant Growth Regulators Based on Terahertz Time-Domain Spectroscopy. Appl. Sci. 2018, 8, 420. [Google Scholar] [CrossRef] [Green Version]
- Qu, F.; Pan, Y.; Lin, L.; Cai, C.; Dong, T.; He, Y.; Nie, P. Experimental and Theoretical Study on Terahertz Absorption Characteristics and Spectral De-noising of Three Plant Growth Regulators. J. Infrared Millim. Terahertz Waves 2018, 39, 1015–1027. [Google Scholar] [CrossRef]
- Sun, P.; Zou, Y.J.O.; Electronics, Q. Complex dielectric properties of anhydrous polycrystalline glucose in the terahertz region. Opt. Quantum Electron. 2016, 48, 1–10. [Google Scholar] [CrossRef]

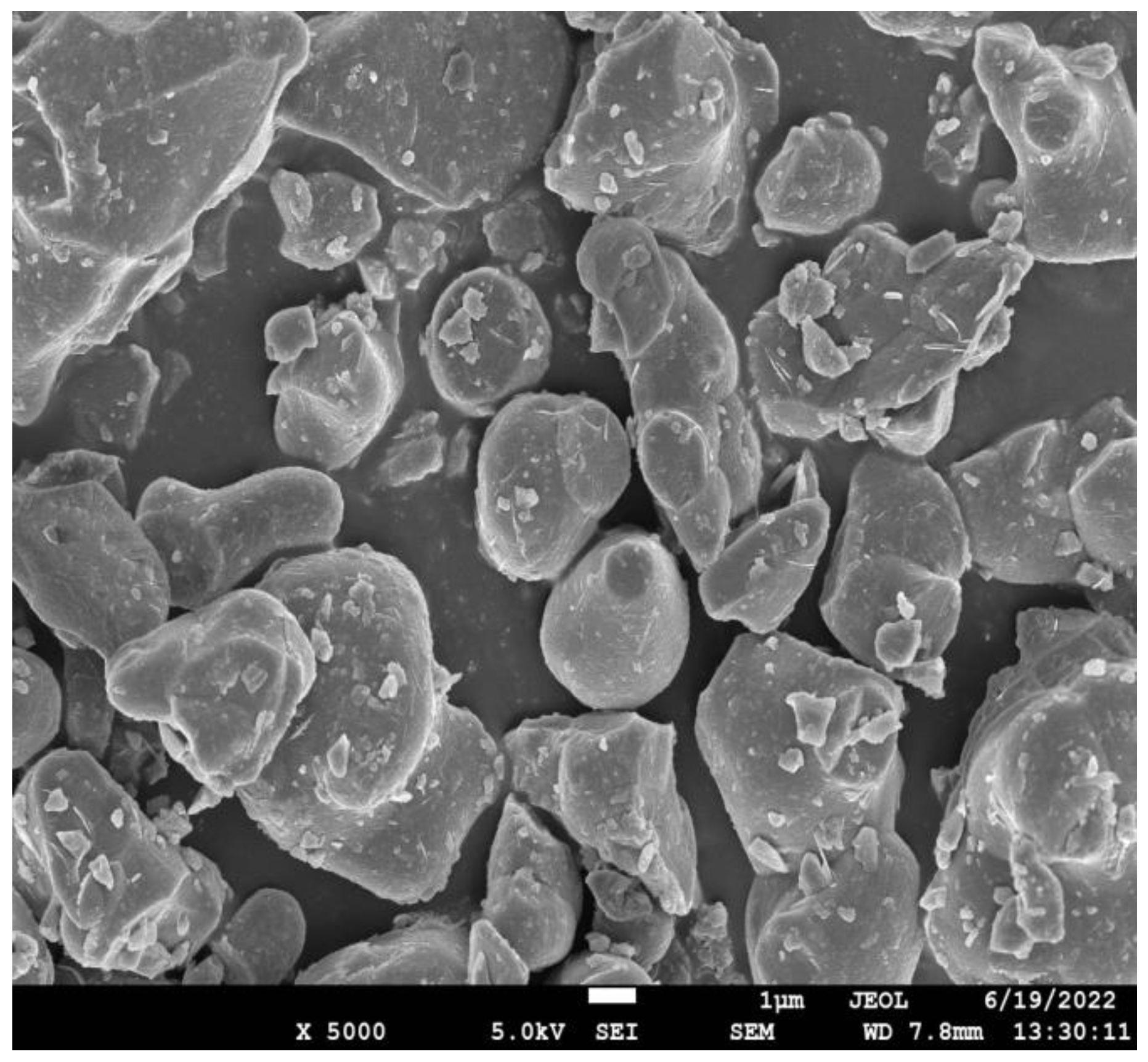


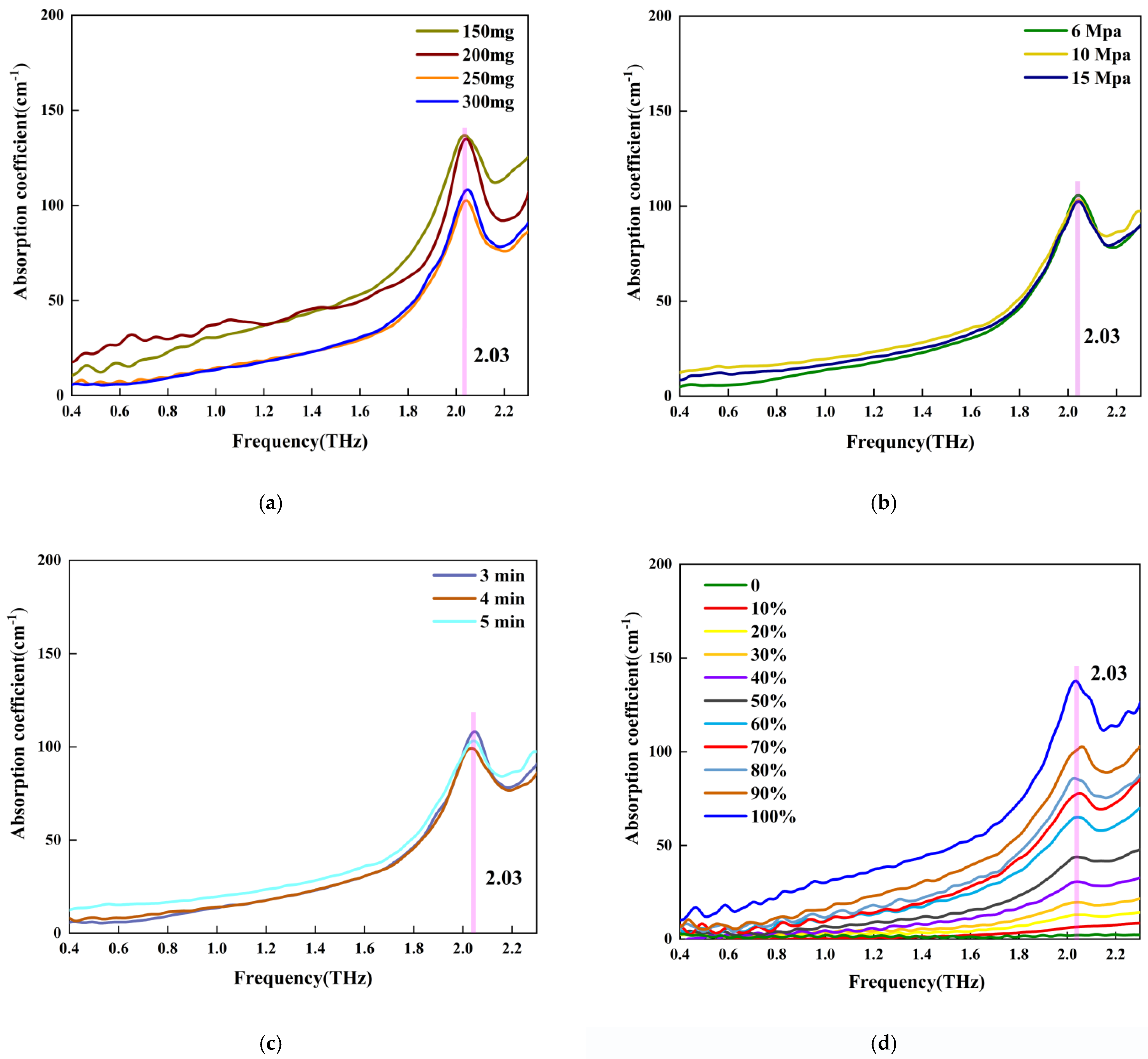
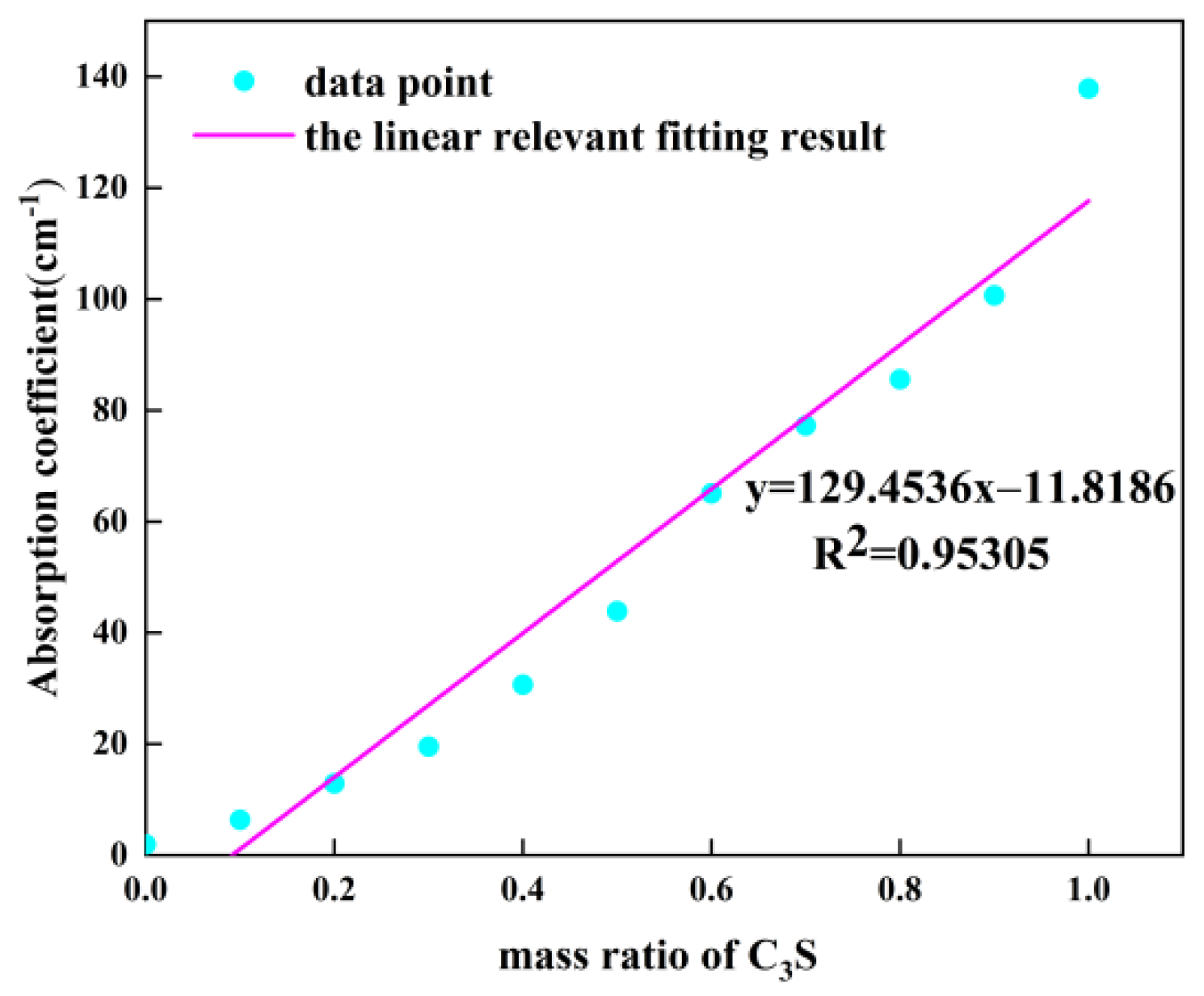

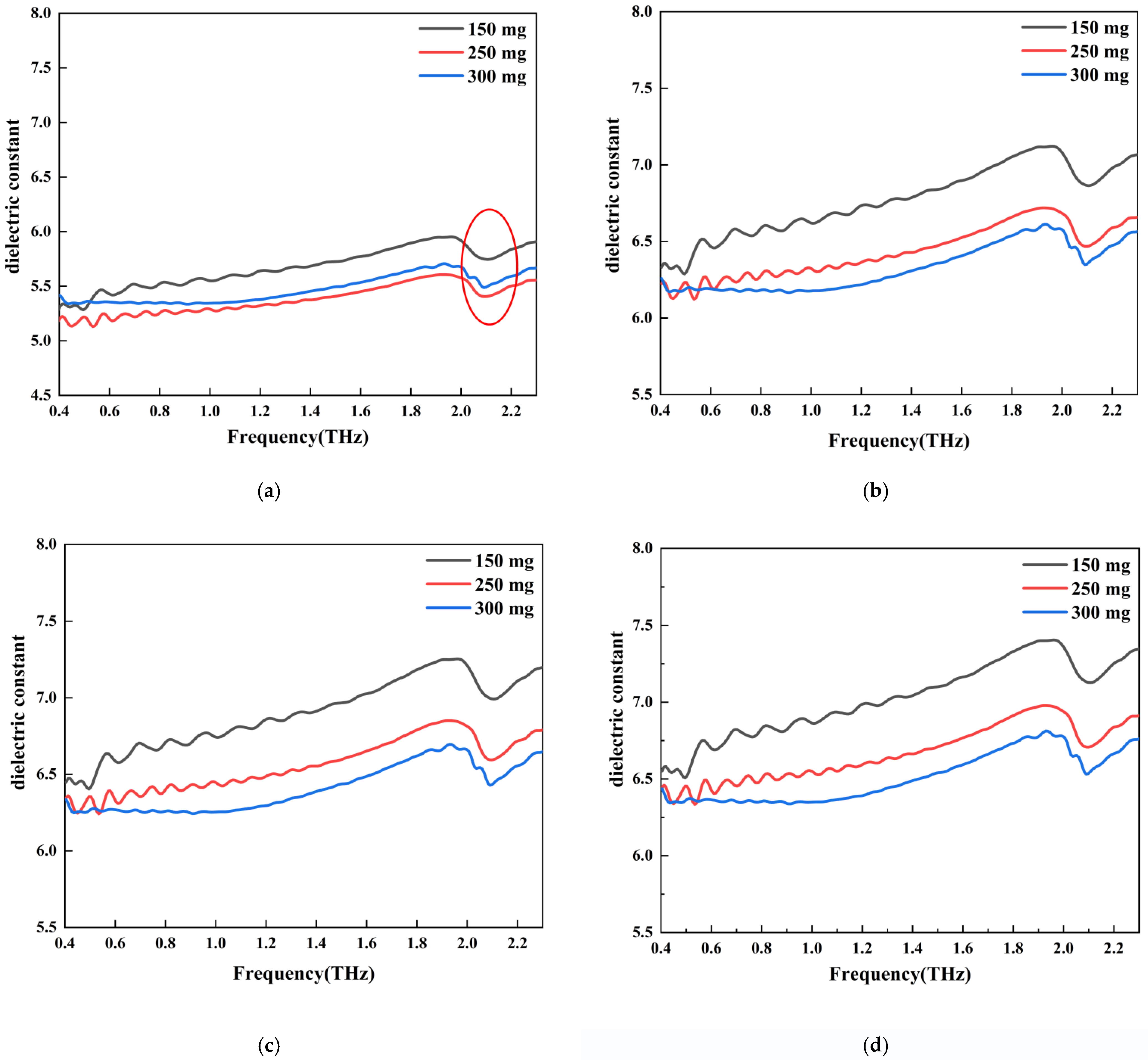



| Concentration | Span | D [4, 3] | D [3, 2] | d (0.1) | d (0.5) | d (0.9) |
|---|---|---|---|---|---|---|
| 0.0074% | 2.864 | 13.156 μm | 3.009 μm | 0.81 μm | 9.972 μm | 29.526 μm |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, W.; Chen, Y.; Tian, L.; Wang, L.; Li, X. Terahertz Time-Domain Spectroscopic Study of Tricalcium Silicate. Sensors 2022, 22, 9354. https://doi.org/10.3390/s22239354
Zhang W, Chen Y, Tian L, Wang L, Li X. Terahertz Time-Domain Spectroscopic Study of Tricalcium Silicate. Sensors. 2022; 22(23):9354. https://doi.org/10.3390/s22239354
Chicago/Turabian StyleZhang, Wentao, Ya Chen, Lidan Tian, Linhao Wang, and Xiangyu Li. 2022. "Terahertz Time-Domain Spectroscopic Study of Tricalcium Silicate" Sensors 22, no. 23: 9354. https://doi.org/10.3390/s22239354
APA StyleZhang, W., Chen, Y., Tian, L., Wang, L., & Li, X. (2022). Terahertz Time-Domain Spectroscopic Study of Tricalcium Silicate. Sensors, 22(23), 9354. https://doi.org/10.3390/s22239354





