Achieving Long Distance Sensing Using Semiconductor Laser with Optical Feedback by Operating at Switching Status
Abstract
1. Introduction
2. A Semiconductor Laser with Optical Feedback (SLOF) at a Switching Status
3. SLOF at Switching Status for Distance Sensing
4. Experiments
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Taimre, T.; Nikolić, M.; Bertling, K.; Lim, Y.L.; Bosch, T.; Rakić, A.D. Laser feedback interferometry: A tutorial on the self-mixing effect for coherent sensing. Adv. Opt. Photonics 2015, 7, 570–631. [Google Scholar] [CrossRef]
- Sciamanna, M.; Shore, K.A. Physics and applications of laser diode chaos. Nat. Photonics 2015, 9, 151–162. [Google Scholar] [CrossRef]
- Chen, Z.; Yu, Y.; Ruan, Y.; Nie, B.; Xi, J.; Guo, Q.; Tong, J. Dual-frequency doppler LiDAR based on external optical feedback effect in a laser. Sensors 2020, 20, 6303. [Google Scholar] [CrossRef] [PubMed]
- Donati, S. Developing self-mixing interferometry for instrumentation and measurements. Laser Photonics Rev. 2012, 6, 393–417. [Google Scholar] [CrossRef]
- Xue, C.; Chang, D.; Fan, Y.; Ji, S.; Zhang, Z.; Lin, H.; Spencer, P.S.; Hong, Y. Characteristics of microwave photonic signal generation using vertical-cavity surface-emitting lasers with optical injection and feedback. J. Opt. Soc. Am. B 2020, 37, 1394–1400. [Google Scholar] [CrossRef]
- Zhuang, J.-P.; Li, X.-Z.; Li, S.-S.; Chan, S.-C. Frequency-modulated microwave generation with feedback stabilization using an optically injected semiconductor laser. Opt. Lett. 2016, 41, 5764–5767. [Google Scholar] [CrossRef]
- Tykalewicz, B.; Goulding, D.; Hegarty, S.; Huyet, G.; Erneux, T.; Kelleher, B.; Viktorov, E. Emergence of resonant mode-locking via delayed feedback in quantum dot semiconductor lasers. Opt. Express 2016, 24, 4239–4246. [Google Scholar] [CrossRef]
- Nie, B.; Ruan, Y.; Yu, Y.; Guo, Q.; Xi, J.; Tong, J. Period-one microwave photonic sensing by a laser diode with optical feedback. J. Lightwave Technol. 2020, 38, 5423–5429. [Google Scholar] [CrossRef]
- Xue, C.; Ji, S.; Hong, Y.; Jiang, N.; Li, H.; Qiu, K. Numerical investigation of photonic microwave generation in an optically injected semiconductor laser subject to filtered optical feedback. Opt. Express 2019, 27, 5065–5082. [Google Scholar] [CrossRef]
- Qi, X.Q.; Liu, J.M. Photonic Microwave Applications of the Dynamics of Semiconductor Lasers. IEEE J. Sel. Top. Quantum Electron. 2011, 17, 1198–1211. [Google Scholar] [CrossRef]
- Li, L.; Yi, X.; Chew, S.X.; Song, S.; Nguyen, L.; Minasian, R.A. Double-pass microwave photonic sensing system based on low-coherence interferometry. Opt. Lett. 2019, 44, 1662–1665. [Google Scholar] [CrossRef] [PubMed]
- Li, S.-S.; Zou, X.; Wang, L.; Wang, A.; Pan, W.; Yan, L. Stable period-one oscillations in a semiconductor laser under optical feedback from a narrowband fiber Bragg grating. Opt. Express 2020, 28, 21286–21299. [Google Scholar] [CrossRef] [PubMed]
- Cui, C.; Chan, S.-C. Performance analysis on using period-one oscillation of optically injected semiconductor lasers for radio-over-fiber uplinks. IEEE J. Quantum Electron. 2012, 48, 490–499. [Google Scholar] [CrossRef]
- Chan, S.-C.; Hwang, S.-K.; Liu, J.-M. Period-one oscillation for photonic microwave transmission using an optically injected semiconductor laser. Opt. Express 2007, 15, 14921–14935. [Google Scholar] [CrossRef] [PubMed]
- Fan, L.; Wu, Z.-M.; Deng, T.; Wu, J.-G.; Tang, X.; Chen, J.-J.; Mao, S.; Xia, G.-Q. Subharmonic microwave modulation stabilization of tunable photonic microwave generated by period-one nonlinear dynamics of an optically injected semiconductor laser. J. Lightwave Technol. 2014, 32, 4660–4666. [Google Scholar] [CrossRef]
- Zhou, P.; Li, N.; Pan, S. Photonic microwave harmonic down-converter based on stabilized period-one nonlinear dynamics of semiconductor lasers. Opt. Lett. 2019, 44, 4869–4872. [Google Scholar] [CrossRef]
- Sciamanna, M.; Virte, M.; Masoller, C.; Gavrielides, A. Hopf bifurcation to square-wave switching in mutually coupled semiconductor lasers. Phys. Rev. E 2012, 86, 016218. [Google Scholar] [CrossRef]
- Dong, J.-X.; Zhuang, J.-P.; Chan, S.-C. Tunable switching between stable and periodic states in a semiconductor laser with feedback. Opt. Lett. 2017, 42, 4291–4294. [Google Scholar] [CrossRef]
- Zhong, Z.; Chang, D.; Jin, W.; Lee, M.; Wang, A.; Jiang, S.; He, J.; Tang, J.; Hong, Y. Intermittent dynamical state switching in discrete-mode semiconductor lasers subject to optical feedback. Photonics Res. 2021, 9, 1336–1342. [Google Scholar] [CrossRef]
- Li, S.S.; Li, X.Z.; Zhuang, J.P.; Mezosi, G.; Sorel, M.; Chan, S.C. Square-wave oscillations in a semiconductor ring laser subject to counter-directional delayed mutual feedback. Opt. Lett. 2016, 41, 812–815. [Google Scholar] [CrossRef]
- Kaplan, A.M.; Agrawal, G.P.; Maywar, D.N. Optical Square-Wave Clock Generation Based on an All-Optical Flip-Flop. IEEE Photonics Technol. Lett. 2010, 22, 489–491. [Google Scholar] [CrossRef]
- Zhang, X.; Gu, C.; Chen, G.; Sun, B.; Xu, L.; Wang, A.; Ming, H. Square-wave pulse with ultra-wide tuning range in a passively mode-locked fiber laser. Opt. Lett. 2012, 37, 1334. [Google Scholar] [CrossRef] [PubMed]
- Duan, Z.; Yu, Y.; Gao, B.; Jiang, C. Absolute distance measurement based on multiple self-mixing interferometry. Opt. Commun. 2017, 389, 270–274. [Google Scholar] [CrossRef]
- Guo, D.; Wang, M. Self-mixing interferometry based on a double-modulation technique for absolute distance measurement. Appl. Opt. 2007, 46, 1486–1491. [Google Scholar] [CrossRef] [PubMed]
- Gouaux, F.; Servagent, N.; Bosch, T. Absolute distance measurement with an optical feedback interferometer. Appl. Opt. 1998, 37, 6684–6689. [Google Scholar] [CrossRef]
- Zhao, Y.; Wang, C.; Zhao, Y.; Zhu, D.; Lu, L. An All-Fiber Self-Mixing Range Finder With Tunable Fiber Ring Cavity Laser Source. J. Lightwave Technol. 2021, 39, 4217–4224. [Google Scholar] [CrossRef]
- Lang, R.; Kobayashi, K. External optical feedback effects on semiconductor injection laser properties. IEEE J. Quantum Electron. 1980, 16, 347–355. [Google Scholar] [CrossRef]
- Dong, J.-X.; Ruan, J.; Zhang, L.; Zhuang, J.-P.; Chan, S.-C. Stable-unstable switching dynamics in semiconductor lasers with external cavities. Phys. Rev. A 2021, 103, 053524. [Google Scholar] [CrossRef]
- Murakami, A.; Ohtsubo, J.; Liu, Y. Stability analysis of semiconductor laser with phase-conjugate feedback. IEEE J. Quantum Electron. 1997, 33, 1825–1831. [Google Scholar] [CrossRef][Green Version]
- Ohtsubo, J. Semiconductor Lasers: Stability, Instability and Chaos; Springer: Berlin/Heidelberg, Germany, 2012; Volume 111. [Google Scholar]

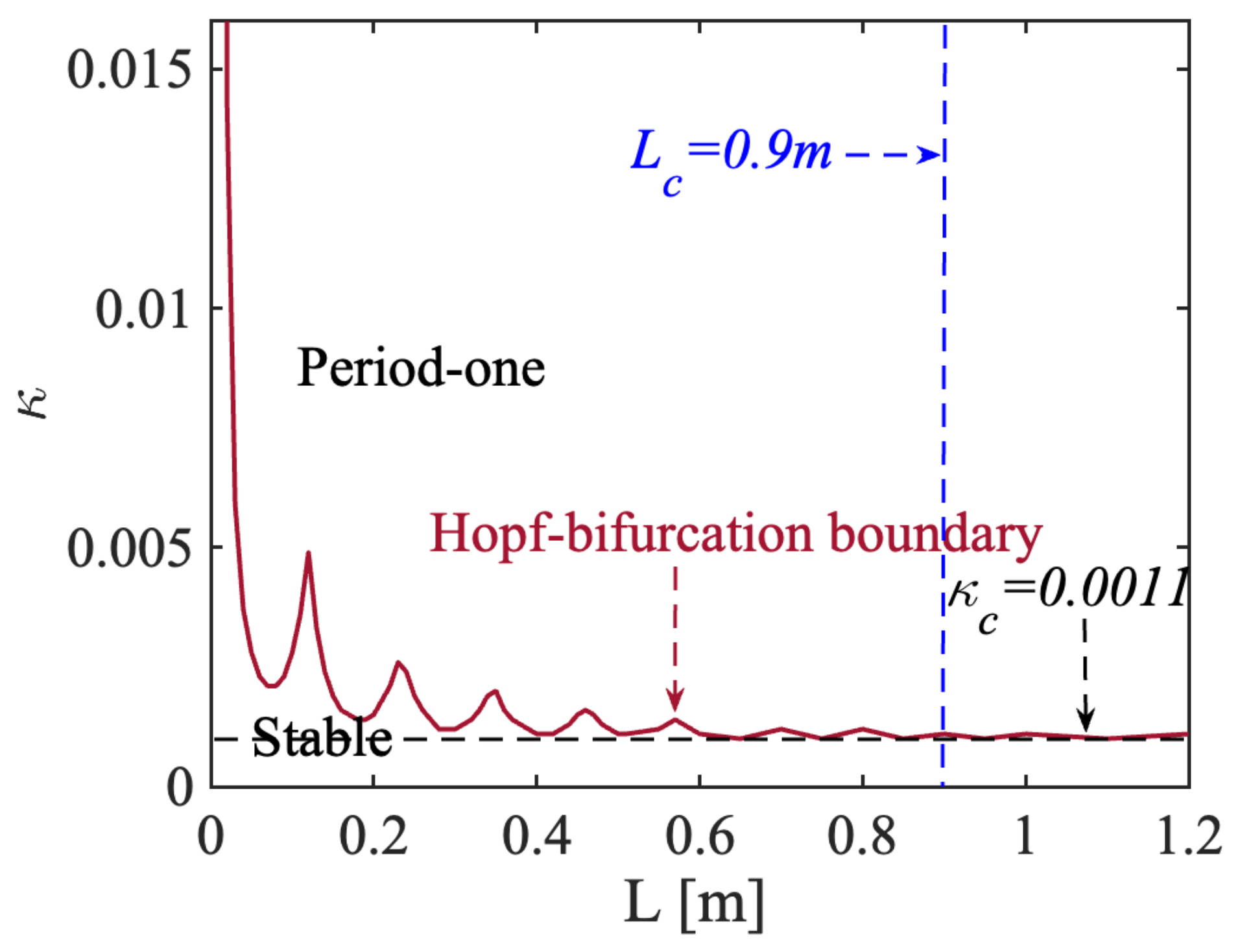

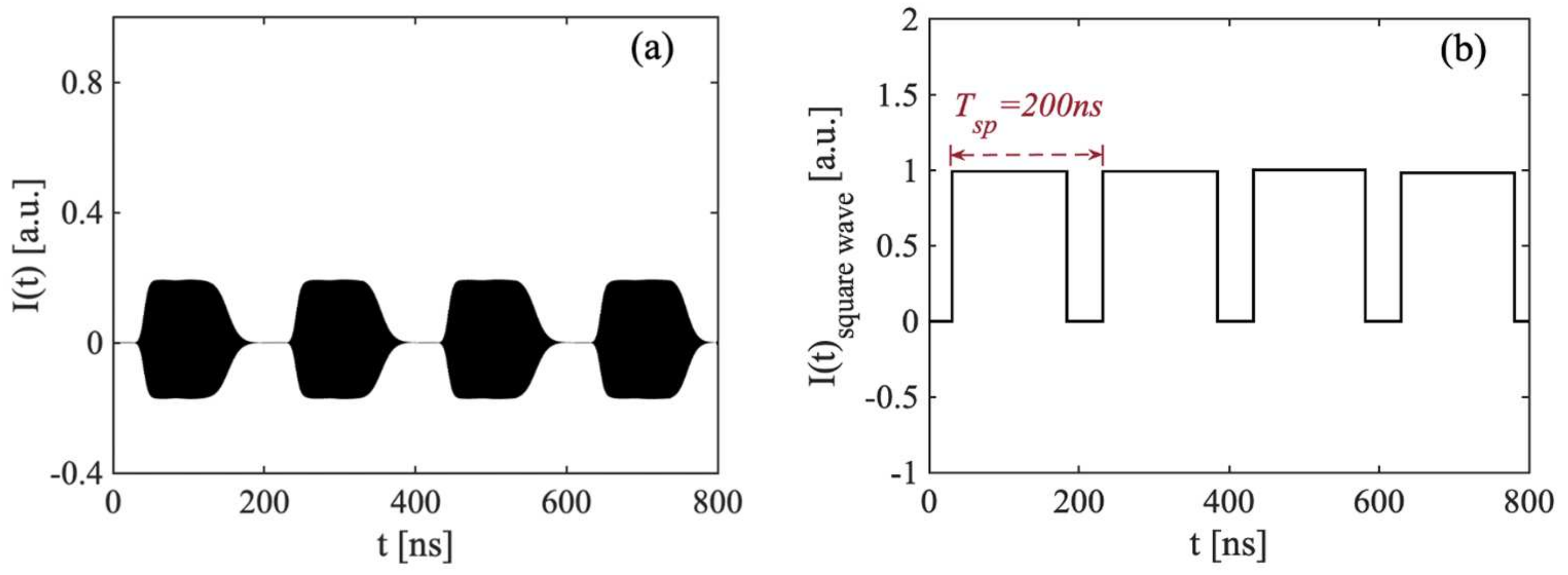

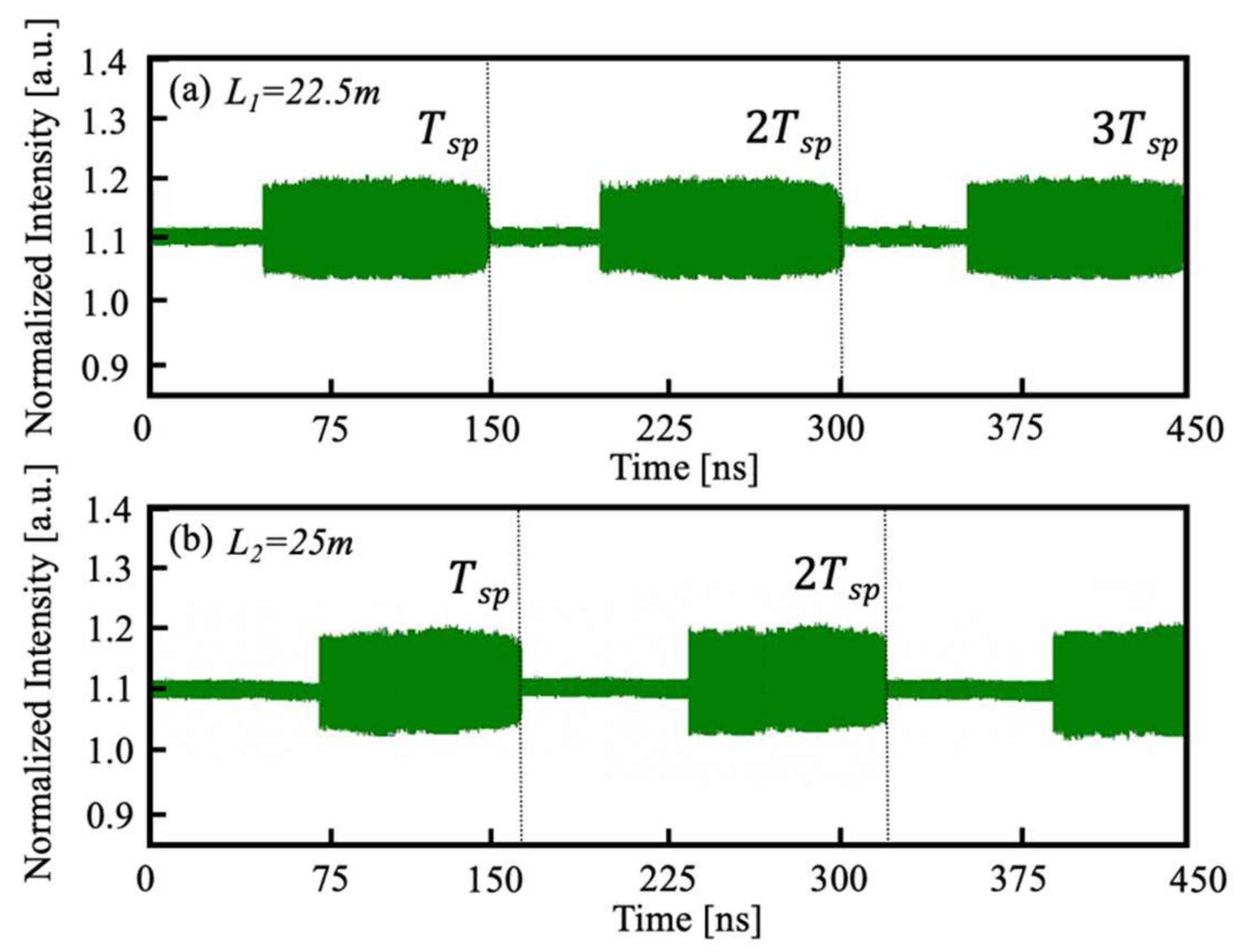
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nie, B.; Ruan, Y.; Yu, Y.; Guo, Q.; Fang, C.; Xi, J.; Tong, J.; Du, H. Achieving Long Distance Sensing Using Semiconductor Laser with Optical Feedback by Operating at Switching Status. Sensors 2022, 22, 963. https://doi.org/10.3390/s22030963
Nie B, Ruan Y, Yu Y, Guo Q, Fang C, Xi J, Tong J, Du H. Achieving Long Distance Sensing Using Semiconductor Laser with Optical Feedback by Operating at Switching Status. Sensors. 2022; 22(3):963. https://doi.org/10.3390/s22030963
Chicago/Turabian StyleNie, Bairun, Yuxi Ruan, Yanguang Yu, Qinghua Guo, Can Fang, Jiangtao Xi, Jun Tong, and Haiping Du. 2022. "Achieving Long Distance Sensing Using Semiconductor Laser with Optical Feedback by Operating at Switching Status" Sensors 22, no. 3: 963. https://doi.org/10.3390/s22030963
APA StyleNie, B., Ruan, Y., Yu, Y., Guo, Q., Fang, C., Xi, J., Tong, J., & Du, H. (2022). Achieving Long Distance Sensing Using Semiconductor Laser with Optical Feedback by Operating at Switching Status. Sensors, 22(3), 963. https://doi.org/10.3390/s22030963








