A Preliminary Study for Reference RF Coil at 11.7 T MRI: Based on Electromagnetic Field Simulation of Hybrid-BC RF Coil According to Diameter and Length at 3.0, 7.0 and 11.7 T
Abstract
:1. Introduction
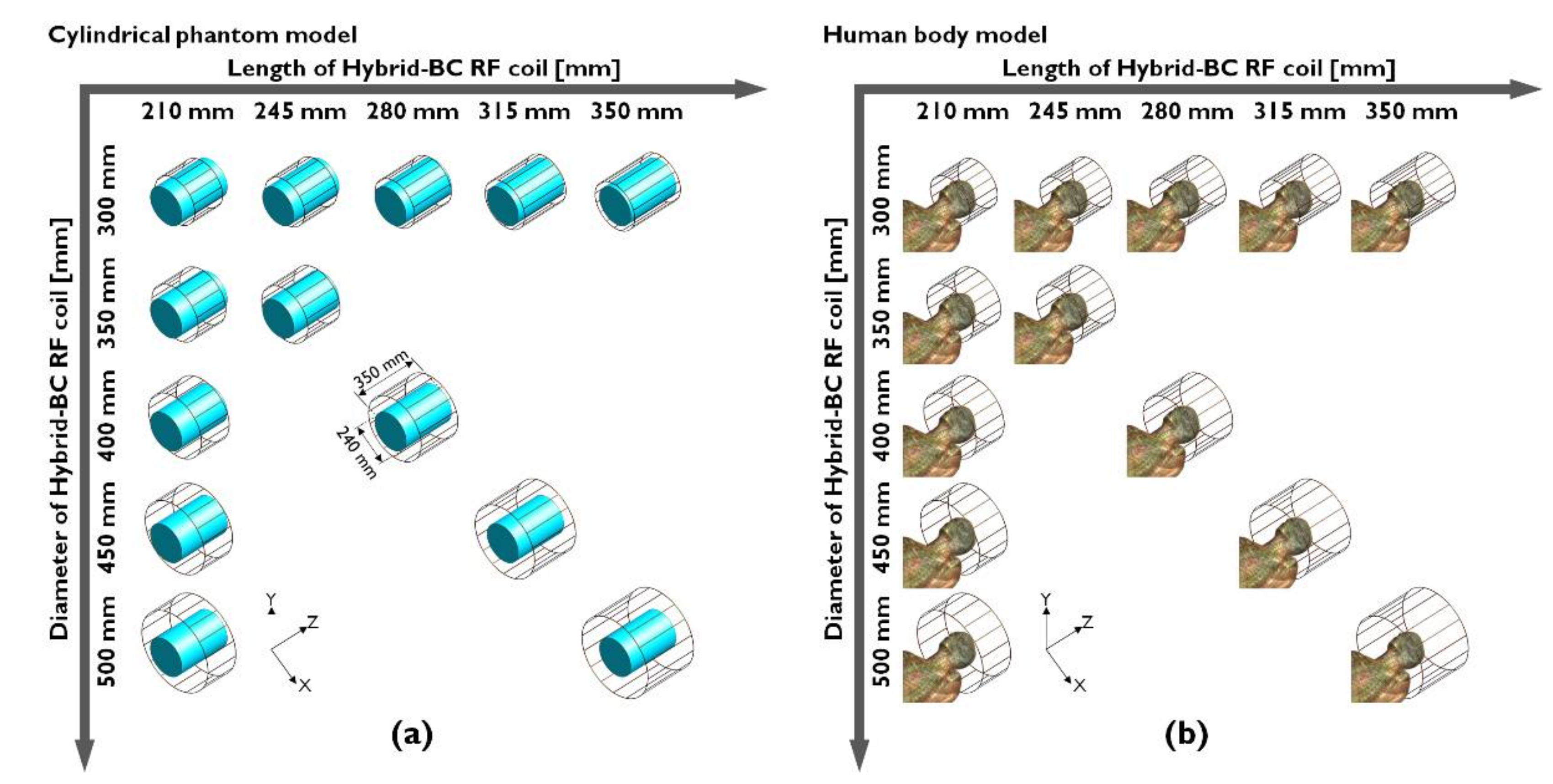
2. Materials and Methods
2.1. EM-Field Simulation Setup
2.2. Analysis
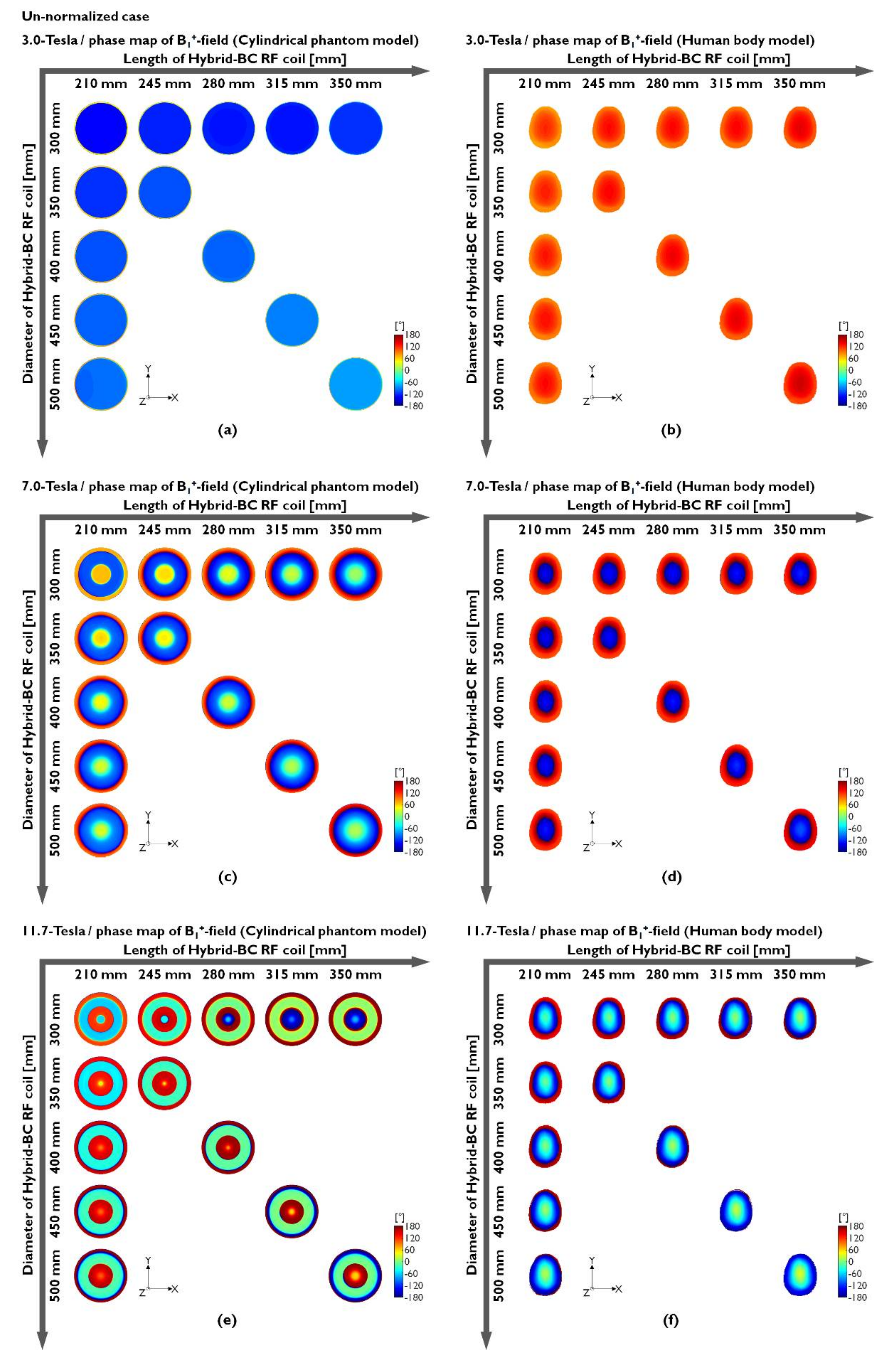
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
List of Symbols
| λ | Larmor frequencies |
| εr | Relative permittivity |
| σ | Conductivity |
| x-component of magnetic flux density field | |
| y-component of magnetic flux density field | |
| z-component of magnetic flux density field | |
| Transverse component of magnetic flux density field | |
| Phase of magnetic flux density field | |
| Absolute value | |
| Power loss | |
| Conduction current | |
| Time-harmonic electric field phasor | |
| Conjugate transpose of time-harmonic electric field phasor | |
| Locations of calculation | |
| Mass density | |
| Temperature |
Abbreviations
| MRI | Magnetic resonance imaging |
| T | Tesla (A derived unit of the magnetic B-field strength) |
| RF | Radiofrequency |
| B0-field | Magnetic field |
| B1-field | Magnetic flux density field |
| |B1|-field | Magnitude map of magnetic flux density field |
| B1+-field | RF transmission field |
| |B1+|-field | Magnitude map of RF transmission field |
| B1--field | RF reception field |
| |B1-|-field | Magnitude map of RF reception field |
| E-field | Electric field |
| |E|-field | Magnitude map of electric field |
| BC | Birdcage coil |
| LP-BC | Low-pass-type birdcage coil |
| HP-BC | High-pass-type birdcage coil |
| Hybrid-BC | Hybrid-type birdcage coil (Band-pass-type birdcage coil) |
| LF | Low-field |
| HF | High-field |
| UHF | Ultra-high-field |
| EUHF | Extremely-ultra-high-field |
| SAR | Specific absorption rate |
| D | Diameter |
| L | Length |
| D/L-ratio | Diameter-to-length ratio |
| EM-field | Electromagnetic field |
| CP | left-point |
| STD | Standard deviation |
| GUGMC | Gachon University Gil medical left |
| FDTD | Finite-difference time-domain |
| IT’IS | The foundation for research on information technologies in society |
| GPU | Graphics processing unit |
| CPU | Central processing unit |
| CUDA | Compute unified device architecture |
References
- Hayes, C.E.; Edelstein, W.A.; Schenck, J.F.; Mueller, O.M.; Eash, M. An efficient, highly homogeneous radiofrequency coil for whole-body NMR imaging at 1.5T. J. Magn. Reson. 1985, 63, 622–628. [Google Scholar] [CrossRef]
- Leifer, M.C. Resonant modes of the birdcage coil. J. Magn. Reson. 1997, 124, 51–60. [Google Scholar] [CrossRef]
- Li, S.; Yang, Q.X.; Smith, M.B. RF coil optimization: Evaluation of B1 field homogeneity using field histograms and finite element calculations. Magn. Reson. Imaging 1994, 12, 1079–1087. [Google Scholar] [CrossRef]
- Caverly, R.H. RF Aspects of High-Field Magnetic Resonance Imaging (HF-MRI): Recent Advances. IEEE J. Electromagn. RF Microw. Med. Biol. 2019, 3, 111–119. [Google Scholar] [CrossRef]
- Seo, J.H.; Ryu, Y.; Han, S.-D.; Song, H.; Kim, H.-K.; Kim, K.-N. Influence of biological subject, shielding cage, and resonance frequency on radio wave propagation in a birdcage coil. Electron. Lett. 2016, 52, 801–803. [Google Scholar] [CrossRef]
- Heo, P.; Seo, J.-H.; Han, S.-D.; Ryu, Y.; Byun, J.-D.; Kim, K.-N.; Lee, J.H. Multi-port-driven birdcage coil for multiple-mouse MR imaging at 7 T. Scanning 2016, 38, 747–756. [Google Scholar] [CrossRef] [Green Version]
- Cheng, T.; Magill, A.W.; Comment, A.; Gruetter, R.; Lei, H. Ultra-high field birdcage coils: A comparison study at 14.1T. In Proceedings of the 36th Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBS 2014), Chicago, IL, USA, 26–30 August 2014; pp. 2360–2363. [Google Scholar]
- Platt, T.; Ladd, M.E.; Paech, D. 7 Tesla and beyond: Advanced methods and clinical applications in magnetic resonance imaging. Investig. Radiol. 2021, 56, 705–725. [Google Scholar] [CrossRef]
- Seo, J.-H.; Lee, J.J.; Kim, K.-N. Surface coil with an inductively coupled wireless surface and volume coil for improving the magnetic field sensitivity at 400-MHz MRI. J. Magn. 2018, 23, 192–195. [Google Scholar] [CrossRef]
- Ohliger, M.A.; Sodickson, D.K. An introduction to coil array design for parallel MR. NMR Biomed. 2006, 19, 300–315. [Google Scholar] [CrossRef]
- Fujita, H.; Braum, W.O.; Morich, M.A. Novel quadrature birdcage coil for a vertical B0 field open MRI system. Magn. Reson. Med. 2000, 44, 633–640. [Google Scholar] [CrossRef]
- Gulsen, G.; Muftuler, L.T.; Nalcioglu, O. A double end-cap birdcage RF coil for small animal whole body imaging. J. Magn. Reson. 2002, 156, 309–312. [Google Scholar] [CrossRef]
- Golestanirad, L.; Rahsepar, A.A.; Kirsch, J.E.; Suwa, K.; Collins, J.C.; Angelone, L.M.; Keil, B.; Passman, R.S.; Bonmassar, G.; Serano, P.; et al. Changes in the specific absorption rate (SAR) of radiofrequency energy in patients with retained cardiac leads during MRI at 1.5 T and 3 T. Magn. Reson. Med. 2019, 81, 653–669. [Google Scholar] [CrossRef] [PubMed]
- Oh, S.; Webb, A.G.; Neuberger, T.; Park, B.; Collins, C.M. Experimental and numerical assessment of MRI-induced temperature change and SAR distributions in phantoms and in vivo. Magn. Reson. Med. 2010, 63, 218–223. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Giovannetti, G.; Francesconi, R.; Landini, L.; Viti, V.; Santarelli, M.F.; Positano, V.; Benassi, A. A quadrature lowpass birdcage coil for a vertical low field MRI scanner. Concepts Magn. Reson. Part B Magn. Reson. Eng. 2004, 22B, 1–6. [Google Scholar] [CrossRef]
- Mispelter, J.; Lupu, M.; Briguet, A. NMR Probeheads for Biophysical and Biomedical Experiments, 2nd ed.; Imperial College Press: London, UK, 2015; pp. 527–541. [Google Scholar]
- Qian, C.; Masad, I.S.; Rosenberg, J.T.; Elumalai, M.; Brey, W.W.; Grant, S.C.; Gor’kov, P.L. A volume birdcage coil with an adjustable sliding tuner ring for neuroimaging in high field vertical magnets: Ex and in vivo applications at 21.1 T. J. Magn. Reson. 2012, 221, 110–116. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Barberi, E.A.; Gati, J.S.; Rutt, B.K.; Menon, R.S. A transmit-only/receive-only (TORO) RF system for high-field MRI/MRS applications. Magn. Reson. Med. 2000, 43, 284–289. [Google Scholar] [CrossRef]
- Kim, Y.C.; Kim, H.D.; Yun, B.-J.; Ahmad, S.F. A simple analytical solution for the designing of the birdcage RF coil used in NMR imaging applications. Appl. Sci. 2020, 10, 2242. [Google Scholar] [CrossRef] [Green Version]
- Giovannetti, G. Birdcage coils: Equivalent capacitance and equivalent inductance. Concepts Magn. Reson. 2014, 44, 32–38. [Google Scholar] [CrossRef]
- Vaughan, J.T.; Griffiths, J.R. RF Coils for MRI; WILEY: New York, NY, USA, 2012; pp. 123–136. [Google Scholar]
- Puddu, C.; Fanti, A.; Curreli, N.; Mazzarella, G. Challenging the lumped birdcage coil model for high-field MRI. In Proceedings of the IEEE 2014 Loughborough Antennas & Propagation Conference (LAPC), Loughborough, Leicestershire, UK, 10–11 November 2014; pp. 308–311. [Google Scholar]
- Runge, V.M.; Heverhagen, J.T. The clinical utility of magnetic resonance imaging according to field strength, specifically addressing the breadth of current state-of-the-art systems, which include 0.55 T, 1.5 T, 3 T, and 7 T. Investig. Radiol. 2022, 57, 1–12. [Google Scholar] [CrossRef]
- Ibrahim, T.S.; Lee, R.; Abduljialil, A.M.; Baertlein, B.A.; Robitaille, P.M. Dielectric resonances and B1 field inhomogeneity in UHF MRI: Computational analysis and experimental findings. Magn. Reson. Imaging 2001, 19, 219–226. [Google Scholar] [CrossRef]
- Jin, J.M.; Chen, J.; Chew, W.C.; Gan, H.; Magin, R.L.; Dimbylow, P.J. Computation of electromagnetic fields for high-frequency magnetic resonance imaging applications. Phys. Med. Biol. 1996, 41, 2719–2738. [Google Scholar] [CrossRef] [PubMed]
- Kim, K.-N.; Seo, J.-H.; Han, S.-D.; Song, H.; Ryu, Y. B1 propagation properties of combined circularly polarised mode in ultra-high-field MRI. Electron. Lett. 2016, 52, 591–592. [Google Scholar] [CrossRef]
- Seo, J.H.; Han, S.D.; Kim, K.N. Design of crisscrossed double-layer birdcage coil for improving B1+ field homogeneity for small-animal magnetic resonance imaging at 300 MHz. J. Magn. 2015, 20, 308–311. [Google Scholar] [CrossRef] [Green Version]
- Seo, J.H.; Song, H.; Kim, H.J.; Han, S.-D.; Heo, P.; Kim, D.; Ryu, Y.; Kim, K.-N. Helmholtz transceiver array for improving the |B1|-field homogeneity at 7-T magnetic resonance imaging. Phys. Wave Phen. 2017, 25, 147–150. [Google Scholar] [CrossRef]
- Kaneko, Y.; Soutome, Y.; Habara, H.; Bito, Y.; Ochi, H. B1-control receive array coil (B-RAC) for reducing B1+ inhomogeneity in abdominal imaging at 3T-MRI. J. Magn. Reson. 2018, 287, 25–32. [Google Scholar] [CrossRef]
- Park, B.S.; Ma, G.; Koch, W.T.; Rajan, S.S.; Mastromanolis, M.; Lam, J.; Sung, K.; McCright, B. Improvement of 19F MR image uniformity in a mouse model of cellular therapy using inductive coupling. Magn. Reson. Mater. Phys. Biol. Med. 2019, 32, 15–23. [Google Scholar] [CrossRef]
- Boehmert, L.; Kuehne, A.; Waiczies, H.; Wenz, D.; Eigentler, T.W.; Funk, S.; von Knobelsdorff-Brenkenhoff, F.; Schulz-Menger, J.; Nagel, A.M.; Seeliger, E.; et al. Cardiorenal sodium MRI at 7.0 Tesla using a 4/4 channel 1H/23Na radiofrequency antenna array. Magn. Reson. Med. 2019, 82, 2343–2356. [Google Scholar] [CrossRef]
- Destruel, A.; Fuentes, M.; Weber, E.; O’Brien, K.; Jin, J.; Liu, F.; Barth, M.; Crozier, S. A numerical and experimental study of RF shimming in the presence of hip prostheses using adaptive SAR at 3 T. Magn. Reson. Med. 2019, 81, 3826–3839. [Google Scholar] [CrossRef]
- Krikken, E.; Steensma, B.R.; Voogt, I.J.; Luijten, P.R.; Klomp, D.W.J.; Raaijmakers, A.J.E.; Wijnen, J.P. Homogeneous B1+ for bilateral breast imaging at 7 T using a five dipole transmit array merged with a high density receive loop array. NMR Biomed. 2019, 32, e4039. [Google Scholar] [CrossRef]
- Kim, K.N.; Hernandez, D.; Seo, J.H.; Noh, Y.; Han, Y.; Ryu, Y.C.; Chung, J.Y. Quantitative assessment of phased array coils with different numbers of receiving channels in terms of signal-to-noise ratio and spatial noise variation in magnetic resonance imaging. PLoS ONE 2019, 14, e0219407. [Google Scholar] [CrossRef]
- Park, B.S.; Rajan, S.S.; McCright, B. Sensitivity and uniformity improvement of phased array MR images using inductive coupling and RF detuning circuits. Magn. Reson. Mater. Phys. Biol. Med. 2020, 33, 725–733. [Google Scholar] [CrossRef] [PubMed]
- Elabyad, I.A.; Terekhov, M.; Stefanescu, M.R.; Lohr, D.; Fischer, M.; Schreiber, L.M. Design of a novel antisymmetric coil array for parallel transmit cardiac MRI in pigs at 7 T. J. Magn. Reson. 2019, 305, 195–208. [Google Scholar] [CrossRef] [PubMed]
- Puddu, C.; Rao, M.; Xu, X.; Deppe, M.H.; Collier, G.; Maunder, A.; Chan, H.F.; De Zanche, N.; Robb, F.; Wild, J.M. An asymmetrical whole-body birdcage RF coil without RF shield for hyperpolarized (129) Xe lung MR imaging at 1.5 T. Magn. Reson. Med. 2021, 86, 3373–3381. [Google Scholar] [CrossRef] [PubMed]
- Li, M.; Weber, E.; Jin, J.; Hugger, T.; Tesiram, Y.; Ullmann, P.; Stark, S.; Fuentes, M.; Junge, S.; Liu, F.; et al. Radial magnetic resonance imaging (MRI) using a rotating radiofrequency (RF) coil at 9.4 T. NMR Biomed. 2018, 31, e3860. [Google Scholar] [CrossRef]
- Kim, K.N.; Han, S.D.; Seo, J.H.; Heo, P.; Yoo, D.; Im, G.H.; Lee, J.H. An asymmetric birdcage coil for small-animal MR imaging at 7T. Magn. Reson. Med. Sci. 2017, 16, 253–258. [Google Scholar] [CrossRef] [Green Version]
- Koreshin, E.; Efimtcev, A.; Gulko, A.; Popov, S.; Orlov, I.; Trufanov, G.; Zubkov, M. Design of a RF-resonant set improving locally the B1+ efficiency. Applications for clinical MRI in andrology and urology. J. Magn. Reson. 2020, 317, 106774. [Google Scholar] [CrossRef]
- Rietsch, S.H.G.; Orzada, S.; Maderwald, S.; Brunheim, S.; Philips, B.W.J.; Scheenen, T.W.J.; Ladd, M.E.; Quick, H.H. 7T ultra-high field body MR imaging with an 8-channel transmit/32-channel receive radiofrequency coil array. Med. Phys. 2018, 45, 2978–2990. [Google Scholar] [CrossRef]
- Kim, K.-N.; Seo, J.-H.; Han, S.-D.; Heo, P.; Im, G.H.; Lee, J.H. Development of double-layer coupled coil for improving S/N in 7 T small-animal MRI. Scanning. 2015, 37, 361–371. [Google Scholar] [CrossRef]
- Seo, J.-H.; Han, S.-D.; Kim, K.-N. Improvements in magnetic field intensity and uniformity for small-animal MRI through a high-permittivity material attachment. Electron. Lett. 2016, 52, 898–900. [Google Scholar] [CrossRef]
- Kim, K.N.; Ryu, Y.; Seo, J.H.; Kim, Y.B. Magnetic field sensitivity at 7-T using dual-helmholtz transmit-only coil and 12-channel receive-only bended coil. Scanning 2016, 38, 515–524. [Google Scholar] [CrossRef] [Green Version]
- Yang, Q.X.; Rupprecht, S.; Luo, W.; Sica, C.; Herse, Z.; Wang, J.; Cao, Z.; Vesek, J.; Lanagan, M.T.; Carluccio, G.; et al. Radiofrequency field enhancement with high dielectric constant (HDC) pads in a receive array coil at 3.0T. J. Magn. Reson. Imaging 2013, 38, 435–440. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ibrahim, T.S.; Lee, R.; Baertlein, B.A.; Robitaille, P.M. B1 field homogeneity and SAR calculations for the birdcage coil. Phys. Med. Biol. 2001, 46, 609–619. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Eryaman, Y.; Akin, B.; Atalar, E. Reduction of implant RF heating through modification of transmit coil electric field. Magn. Reson. Med. 2011, 65, 1305–1313. [Google Scholar] [CrossRef] [PubMed]
- Wolf, S.; Diehl, D.; Gebhardt, M.; Mallow, J.; Speck, O. SAR simulations for high-field MRI: How much detail, effort, and accuracy is needed? Magn. Reson. Med. 2013, 69, 1157–1168. [Google Scholar] [CrossRef]
- Destruel, A.; O’Brien, K.; Jin, J.; Liu, F.; Barth, M.; Crozier, S. Adaptive SAR mass-averaging framework to improve predictions of local RF heating near a hip implant for parallel transmit at 7 T. Magn. Reson. Med. 2019, 81, 615–627. [Google Scholar] [CrossRef] [Green Version]
- Krishnamurthy, N.; Santini, T.; Wood, S.; Kim, J.; Zhao, T.; Aizenstein, H.J.; Ibrahim, T.S. Computational and experimental evaluation of the Tic-Tac-Toe RF coil for 7 Tesla MRI. PLoS ONE 2019, 14, e0209663. [Google Scholar] [CrossRef] [Green Version]
- Kazemivalipour, E.; Keil, B.; Vali, A.; Rajan, S.; Elahi, B.; Atalar, E.; Wald, L.L.; Rosenow, J.; Pilitsis, J.; Golestanirad, L. Reconfigurable MRI technology for low-SAR imaging of deep brain stimulation at 3T: Application in bilateral leads, fully-implanted systems, and surgically modified lead trajectories. Neuroimage 2019, 199, 18–29. [Google Scholar] [CrossRef]
- Tang, M.; Okamoto, K.; Haruyama, T.; Yamamoto, T. Electromagnetic simulation of RF burn injuries occurring at skin-skin and skin-bore wall contact points in an MRI scanner with a birdcage coil. Phys. Med. 2021, 82, 219–227. [Google Scholar] [CrossRef]
- Chen, H.; Guo, L.; Li, M.; Destruel, A.; Liu, C.; Weber, E.; Liu, F.; Crozier, S. Metamaterial-inspired radiofrequency (RF) shield with reduced specific absorption rate (SAR) and improved transmit efficiency for UHF MRI. IEEE Trans. Biomed. Eng. 2021, 68, 1178–1189. [Google Scholar] [CrossRef]
- Nikulin, A.V.; Vignaud, A.; Avdievich, N.I.; Berrahou, D.; de Rosny, J.; Ourir, A. Open birdcage coil for head imaging at 7T. Magn. Reson. Med. 2021, 86, 2290–2300. [Google Scholar] [CrossRef]
- Fiedler, T.M.; Orzada, S.; Flöser, M.; Rietsch, S.H.G.; Schmidt, S.; Stelter, J.K.; Wittrich, M.; Quick, H.H.; Bitz, A.K.; Ladd, M.E. Performance and safety assessment of an integrated transmit array for body imaging at 7 T under consideration of specific absorption rate, tissue temperature, and thermal dose. NMR Biomed. 2021, 28, e4656. [Google Scholar] [CrossRef] [PubMed]
- Malik, S.J.; Hand, J.W.; Satnarine, R.; Price, A.N.; Hajnal, J.V. Specific absorption rate and temperature in neonate models resulting from exposure to a 7T head coil. Magn. Reson. Med. 2021, 86, 1299–1313. [Google Scholar] [CrossRef] [PubMed]
- Seo, J.H.; Han, S.D.; Kim, K.-N. Investigation of the B1 field distribution and RF power deposition in a birdcage coil as functions of the number of coil legs at 4.7 T, 7.0 T, and 11.7 T. J. Korean Phys. Soc. 2015, 66, 1822–1826. [Google Scholar] [CrossRef]
- Yee, K.S. Numerical solution of initial boundary value problems involving Maxwell’s equations in isotropic media. IEEE Trans. Antennas Propag. 1996, 14, 302–307. [Google Scholar]
- Hasgall, P.A.; Di Gennaro, F.; Baumgartner, C.; Neufeld, E.; Lloyd, B.; Gosselin, M.C.; Payne, D.; Klingenböck, A.; Kuster, N. IT’IS Database for Thermal and Electromagnetic Parameters of Biological Tissues; Version 4.0; IT’IS Foundation: Zurich, Switzerland, 15 May 2018. [Google Scholar] [CrossRef]
- Hoult, D.I. The principle of reciprocity in signal strength calculations—A mathematical guide. Concepts Magn. Reson. 2000, 12, 173–187. [Google Scholar] [CrossRef]
- Herrmann, T.; Liebig, T.; Mallow, J.; Bruns, C.; Stadler, J.; Mylius, J.; Brosch, M.; Svedja, J.T.; Chen, Z.; Rennings, A.; et al. Metamaterial-based transmit and receive system for whole-body magnetic resonance imaging at ultra-high magnetic fields. PLoS ONE 2018, 13, e0191719. [Google Scholar] [CrossRef] [Green Version]
- Tang, L.; Hue, Y.-K.; Ibrahim, T.S. Studies of RF shimming techniques with minimization of RF power deposition and their associated temperature changes. Concepts Magn. Reson. Part B Magn. Reson. Eng. 2011, 39B, 11–25. [Google Scholar] [CrossRef] [Green Version]
- Morrell, G.R. A phase-sensitive method of flip angle mapping. Magn. Reson. Med. 2008, 60, 889–894. [Google Scholar] [CrossRef]
- Collins, C.M.; Wang, Z. Calculation of radiofrequency electromagnetic fields and their effects in MRI of human subjects. Magn. Reson. Med. 2011, 65, 1470–1482. [Google Scholar] [CrossRef]
- Yang, R.; Zheng, J.; Wang, Y.; Guo, R.; Kainz, W.; Chen, J. Impact of electrode structure on RF-induced heating for an AIMD implanted lead in a 1.5-Tesla MRI system. IEEE J. Electromagn. RF Microw. Med. Biol. 2019, 3, 247–253. [Google Scholar] [CrossRef]
- Yu, Z.; Xin, X.; Collins, C.M. Potential for high-permittivity materials to reduce local SAR at a pacemaker lead tip during MRI of the head with a body transmit coil at 3 T. Magn. Reson. Med. 2016, 78, 383–386. [Google Scholar] [CrossRef]
- Wang, J.; Yang, Q.X.; Zhang, X.; Collins, C.M.; Smith, M.B.; Zhu, X.-H.; Adriany, G.; Ugurbil, K.; Chen, W. Polarization of the RF field in a human head at high field: A study with a quadrature surface coil at 7.0 T. Magn. Reson. Med. 2002, 48, 362–369. [Google Scholar] [CrossRef] [PubMed]
- Wenz, D.; Gruetter, R. Dipole-fed rectangular dielectric resonator antennas for magnetic resonance imaging at 7 T: The impact of quasi-transverse electric modes on transmit field distribution. Front. Phys. 2021, 9, 675509. [Google Scholar] [CrossRef]
- Polimeni, J.R.; Renvall, V.; Zaretskaya, N.; Fischl, B. Analysis strategies for high-resolution UHF-fMRI data. NeuroImage 2018, 168, 296–320. [Google Scholar] [CrossRef]
- Burkett, B.J.; Fagan, A.J.; Felmlee, J.P.; Black, D.F.; Lane, J.I.; Port, J.D.; Rydberg, C.H.; Welker, K.M. Clinical 7-T MRI for neuroradiology: Strengths, weaknesses, and ongoing challenges. Neuroradiology 2021, 63, 167–177. [Google Scholar] [CrossRef] [PubMed]
- Ladd, M.E.; Bachert, P.; Meyerspeer, M.; Moser, E.; Nagel, A.M.; Norris, D.G.; Schmitter, S.; Speck, O.; Straub, S.; Zaiss, M. Pros and cons of ultra-high-field MRI/MRS for human application. Prog. Nucl. Magn. Reson. Spectrosc. 2018, 109, 1–50. [Google Scholar] [CrossRef]
- Vachha, B.; Huang, S.Y. MRI with ultrahigh field strength and high-performance gradients: Challenges and opportunities for clinical neuroimaging at 7 T and beyond. Eur. Radiol. Exp. 2021, 5, 35. [Google Scholar] [CrossRef]
- Terekhov, M.; Elabyad, I.A.; Schreiber, L.M. Global optimization of default phases for parallel transmit coils for ultra-high-field cardiac MRI. PLoS ONE 2021, 16, e0255341. [Google Scholar] [CrossRef]
- Gandji, N.P.; Sica, C.T.; Lanagan, M.T.; Woo, M.-K.; DelaBarre, L.; Radder, J.; Zhang, B.; Lattanzi, R.; Adriany, G.; Ugurbil, K.; et al. Displacement current distribution on a high dielectric constant helmet and its effect on RF field at 10.5 T (447 MHz). Magn. Reson. Med. 2021, 86, 3292–3303. [Google Scholar] [CrossRef]
- Quettier, L.; Aubert, G.; Belorgey, J.; Berriaud, C.; Bredy, P.; Dilasser, G.; Dubois, O.; Gilgrass, G.; Guihard, Q.; Jannot, V.; et al. Commissioning completion of the iseult whole body 11.7 T MRI system. IEEE Trans. Appl. Supercond. 2020, 30, 1–5. [Google Scholar] [CrossRef]
- Ahmad, S.F.; Kim, Y.C.; Choi, I.C.; Kim, H.D. Recent progress in birdcage RF coil technology for MRI system. Diagnostics 2020, 10, 1017. [Google Scholar] [CrossRef] [PubMed]
- Kaggie, J.D.; Lanz, T.; McLean, M.A.; Riemer, F.; Schulte, R.F.; Benjamin, A.J.V.; Kessler, D.A.; Sun, C.; Gilbert, F.J.; Graves, M.J.; et al. Combined 23Na and 13C imaging at 3.0 Tesla using a single-tuned large FOV birdcage coil. Magn. Reson. Med. 2021, 86, 1734–1745. [Google Scholar] [CrossRef] [PubMed]
- van Houtum, Q.Q.; Mohamed Hoesein, F.F.A.A.; Verhoeff, J.J.J.C.; van Rossum, P.P.S.N.; van Lindert, A.A.S.R.; van der Velden, T.T.A.; van der Kemp, W.W.J.M.; Klomp, D.D.W.J.; Arteaga de Castro, C.C.S. Feasibility of 31P spectroscopic imaging at 7 T in lung carcinoma patients. NMR Biomed. 2021, 34, e4204. [Google Scholar] [PubMed] [Green Version]
- Celik, A.A.; Choi, C.H.; Tellmann, L.; Rick, C.; Shah, N.J.; Felder, J. Design and construction of a PET-compatible double-tuned 1H/31P MR head coil. IEEE Trans. Med. Imaging 2021, 40, 2015–2022. [Google Scholar] [CrossRef] [PubMed]
- Shan, K.; Duan, Y. Rapid four-ring birdcage coil analysis: Design optimization for high efficiency, low interference, and improved body loading tolerance. Magn. Reson. Imaging 2020, 66, 30–35. [Google Scholar] [CrossRef]
- Bonmassar, G.; Serano, P. MRI-induced heating of coils for microscopic magnetic stimulation at 1.5 Tesla: An initial study. Front Hum. Neurosci. 2020, 14, 53. [Google Scholar] [CrossRef]
- Ha, Y.; Choi, C.H.; Worthoff, W.A.; Shymanskaya, A.; Schöneck, M.; Willuweit, A.; Felder, J.; Shah, N.J. Design and use of a folded four-ring double-tuned birdcage coil for rat brain sodium imaging at 9.4 T. J. Magn. Reson. 2018, 286, 110–114. [Google Scholar] [CrossRef]
- Zhang, Y.; Gao, Y.; Fang, K.; Ye, J.; Ruan, Y.; Yang, X.; Zhang, Y.; Thompson, G.; Chen, G.; Zhang, X. Proton/deuterium magnetic resonance imaging of rodents at 9.4T using birdcage coils. Bioelectromagnetics 2022, 43, 40–46. [Google Scholar] [CrossRef]
- Chi, Z.; Yi, Y.; Wang, Y.; Wu, M.; Wang, L.; Zhao, X.; Meng, Y.; Zheng, Z.; Zhao, Q.; Zhou, J. Adaptive cylindrical wireless metasurfaces in clinical magnetic resonance imaging. Adv. Mater. 2021, 33, 2102469. [Google Scholar] [CrossRef]
- Lu, M.; Yang, Z.; Wang, F.; Drake, G.; Chen, L.M.; Gore, J.C.; Yan, X. Optimization of a quadrature birdcage coil for functional imaging of squirrel monkey brain at 9.4T. Magn. Reson. Imaging 2021, 79, 45–51. [Google Scholar] [CrossRef]
- Vít, M.; Buriana, M.; Berková, Z.; Lacik, J.; Sedlacek, O.; Hoogenboom, R.; Raida, Z.; Jirak, D. A broad tuneable birdcage coil for mouse 1H/19F MR applications. J. Magn. Reson. 2021, 329, 107023. [Google Scholar] [CrossRef] [PubMed]
- Giovannetti, G.; Flori, A.; Santarelli, M.F.; Positano, V.; Martini, N.; Francischello, R.; Schulte, R.F.; Ardenkjaer-Larsen, J.H.; Menichetti, L.; Aquaro, G.D.; et al. Radio frequency coils for hyperpolarized 13C magnetic resonance experiments with a 3T MR clinical scanner: Experience from a cardiovascular Lab. Electronics 2021, 10, 366. [Google Scholar] [CrossRef]
- Svenningsen, S.; McIntosh, M.; Ouriadov, A.; Matheson, A.M.; Konyer, N.B.; Eddy, R.L.; McCormack, D.G.; Noseworthy, M.D.; Nair, P.; Parraga, G. Reproducibility of hyperpolarized 129Xe MRI ventilation defect percent in severe asthma to evaluate clinical trial feasibility. Acad. Radiol. 2021, 28, 817–826. [Google Scholar] [CrossRef] [PubMed]
- Valkovič, L.; Dragonu, I.; Almujayyaz, S.; Batzakis, A.; Young, L.A.J.; Purvis, L.A.B.; Clarke, W.T.; Wichmann, T.; Lanz, T.; Neubauer, S.; et al. Using a whole-body 31P birdcage transmit coil and 16-element receive array for human cardiac metabolic imaging at 7T. PLoS ONE 2017, 12, e0187153. [Google Scholar] [CrossRef] [PubMed]
- Lakshmanan, K.; Dehkharghani, S.; Madelin, G.; Brown, R. A dual-tuned 17O/1H head array for direct brain oximetry at 3 Tesla. Magn. Reson. Med. 2020, 83, 1512–1518. [Google Scholar] [CrossRef] [PubMed]




| Cylindrical Phantom Model (Distilled Water) | |||||||||||
| L (mm) | Center-Point Values [×10−6 μT] | Mean Values [×10−6 μT] | |||||||||
| D (mm) | 210 | 245 | 280 | 315 | 350 | 210 | 245 | 280 | 315 | 350 | |
| 3.0 T | 300 | 0.681 | 0.827 | 0.903 | 0.956 | 0.106 | 0.269 | 0.334 | 0.371 | 0.397 | 0.444 |
| 350 | 0.507 | 0.625 | 0.201 | 0.252 | |||||||
| 400 | 0.384 | 0.518 | 0.153 | 0.212 | |||||||
| 450 | 0.300 | 0.429 | 0.120 | 0.177 | |||||||
| 500 | 0.239 | 0.400 | 0.096 | 0.165 | |||||||
| 7.0 T | 300 | 0.248 | 0.273 | 0.299 | 0.328 | 0.373 | 0.061 | 0.074 | 0.092 | 0.111 | 0.134 |
| 350 | 0.142 | 0.167 | 0.045 | 0.056 | |||||||
| 400 | 0.100 | 0.158 | 0.038 | 0.058 | |||||||
| 450 | 0.082 | 0.176 | 0.033 | 0.067 | |||||||
| 500 | 0.071 | 0.205 | 0.029 | 0.081 | |||||||
| 11.7 T | 300 | 0.551 | 0.556 | 0.357 | 0.422 | 0.465 | 0.280 | 0.365 | 0.359 | 0.349 | 0.310 |
| 350 | 0.175 | 0.124 | 0.289 | 0.331 | |||||||
| 400 | 0.218 | 0.102 | 0.250 | 0.232 | |||||||
| 450 | 0.259 | 0.165 | 0.204 | 0.163 | |||||||
| 500 | 0.292 | 0.339 | 0.171 | 0.146 | |||||||
| Human Body Model (Duke Phantom) | |||||||||||
| L (mm) | Center-Point Values [×10−6 μT] | Mean Values [×10−6 μT] | |||||||||
| D (mm) | 210 | 245 | 280 | 315 | 350 | 210 | 245 | 280 | 315 | 350 | |
| 3.0 T | 300 | 0.071 | 0.082 | 0.083 | 0.083 | 0.093 | 0.069 | 0.077 | 0.077 | 0.077 | 0.086 |
| 350 | 0.054 | 0.064 | 0.053 | 0.060 | |||||||
| 400 | 0.043 | 0.053 | 0.041 | 0.049 | |||||||
| 450 | 0.034 | 0.044 | 0.033 | 0.041 | |||||||
| 500 | 0.028 | 0.042 | 0.026 | 0.039 | |||||||
| 7.0 T | 300 | 0.060 | 0.070 | 0.075 | 0.080 | 0.092 | 0.051 | 0.058 | 0.062 | 0.066 | 0.076 |
| 350 | 0.047 | 0.057 | 0.040 | 0.047 | |||||||
| 400 | 0.039 | 0.055 | 0.033 | 0.046 | |||||||
| 450 | 0.033 | 0.057 | 0.028 | 0.047 | |||||||
| 500 | 0.030 | 0.063 | 0.025 | 0.053 | |||||||
| 11.7 T | 300 | 0.079 | 0.093 | 0.088 | 0.083 | 0.088 | 0.070 | 0.084 | 0.081 | 0.077 | 0.083 |
| 350 | 0.070 | 0.081 | 0.059 | 0.070 | |||||||
| 400 | 0.061 | 0.069 | 0.050 | 0.058 | |||||||
| 450 | 0.056 | 0.063 | 0.044 | 0.051 | |||||||
| 500 | 0.053 | 0.072 | 0.041 | 0.057 | |||||||
| Cylindrical Phantom Model (Distilled Water) | |||||||||||
| L (mm) | Normalization Factors [a.u.] | STD of Normalized |B1+|-field [× 10−6 μT] | |||||||||
| D (mm) | 210 | 245 | 280 | 315 | 350 | 210 | 245 | 280 | 315 | 350 | |
| 3.0 T | 300 | 2.876 | 2.368 | 2.168 | 2.048 | 1.846 | 0.215 | 0.210 | 0.207 | 0.204 | 0.202 |
| 350 | 3.861 | 3.132 | 0.215 | 0.211 | |||||||
| 400 | 5.101 | 3.776 | 0.214 | 0.208 | |||||||
| 450 | 6.539 | 4.562 | 0.213 | 0.206 | |||||||
| 500 | 8.195 | 4.897 | 0.211 | 0.204 | |||||||
| 7.0 T | 300 | 7.894 | 7.168 | 6.543 | 5.974 | 5.239 | 0.138 | 0.131 | 0.126 | 0.122 | 0.131 |
| 350 | 13.812 | 11.722 | 0.121 | 0.116 | |||||||
| 400 | 19.572 | 12.360 | 0.118 | 0.118 | |||||||
| 450 | 23.983 | 11.108 | 0.120 | 0.128 | |||||||
| 500 | 27.488 | 9.541 | 0.138 | 0.136 | |||||||
| 11.7 T | 300 | 3.552 | 3.518 | 5.486 | 4.634 | 4.214 | 0.187 | 0.246 | 0.373 | 0.306 | 0.241 |
| 350 | 11.193 | 15.750 | 0.645 | 1.042 | |||||||
| 400 | 8.982 | 19.221 | 0.448 | 0.866 | |||||||
| 450 | 7.552 | 11.861 | 0.312 | 0.354 | |||||||
| 500 | 6.692 | 5.777 | 0.237 | 0.143 | |||||||
| Human Body Model (Duke Phantom) | |||||||||||
| L (mm) | Normalization Factors [a.u.] | STD of Normalized |B1+|-field [× 10−6 μT] | |||||||||
| D (mm) | 210 | 245 | 280 | 315 | 350 | 210 | 245 | 280 | 315 | 350 | |
| 3.0 T | 300 | 27.556 | 24.006 | 23.683 | 23.597 | 21.015 | 0.018 | 0.015 | 0.016 | 0.017 | 0.019 |
| 350 | 35.973 | 30.773 | 0.017 | 0.014 | |||||||
| 400 | 45.852 | 37.297 | 0.016 | 0.014 | |||||||
| 450 | 57.364 | 44.273 | 0.015 | 0.016 | |||||||
| 500 | 70.429 | 46.176 | 0.013 | 0.017 | |||||||
| 7.0 T | 300 | 32.437 | 27.853 | 26.087 | 24.407 | 21.199 | 0.056 | 0.060 | 0.062 | 0.063 | 0.060 |
| 350 | 41.781 | 34.551 | 0.059 | 0.058 | |||||||
| 400 | 50.575 | 35.516 | 0.057 | 0.057 | |||||||
| 450 | 58.789 | 34.435 | 0.056 | 0.057 | |||||||
| 500 | 65.341 | 31.205 | 0.054 | 0.055 | |||||||
| 11.7 T | 300 | 24.685 | 21.103 | 22.141 | 23.708 | 22.149 | 0.067 | 0.066 | 0.066 | 0.065 | 0.062 |
| 350 | 28.169 | 24.172 | 0.063 | 0.061 | |||||||
| 400 | 31.964 | 28.335 | 0.067 | 0.063 | |||||||
| 450 | 35.028 | 31.294 | 0.069 | 0.066 | |||||||
| 500 | 36.804 | 27.399 | 0.071 | 0.066 | |||||||
| Human Body Model (Duke Phantom) | |||||||||||
| L (mm) | Unnormalized Peak SAR [W/kg] | Normalized Peak SAR [W/kg] | |||||||||
| D (mm) | 210 | 245 | 280 | 315 | 350 | 210 | 245 | 280 | 315 | 350 | |
| 3.0 T | 300 | 0.007 | 0.010 | 0.010 | 0.011 | 0.013 | 0.203 | 0.239 | 0.240 | 0.248 | 0.280 |
| 350 | 0.005 | 0.007 | 0.183 | 0.217 | |||||||
| 400 | 0.003 | 0.006 | 0.143 | 0.204 | |||||||
| 450 | 0.002 | 0.004 | 0.117 | 0.169 | |||||||
| 500 | 0.001 | 0.003 | 0.081 | 0.128 | |||||||
| 7.0 T | 300 | 0.012 | 0.017 | 0.019 | 0.021 | 0.028 | 0.341 | 0.398 | 0.446 | 0.492 | 0.590 |
| 350 | 0.007 | 0.010 | 0.247 | 0.317 | |||||||
| 400 | 0.006 | 0.011 | 0.251 | 0.400 | |||||||
| 450 | 0.004 | 0.010 | 0.199 | 0.454 | |||||||
| 500 | 0.003 | 0.013 | 0.207 | 0.595 | |||||||
| 11.7 T | 300 | 0.030 | 0.040 | 0.038 | 0.035 | 0.042 | 0.822 | 0.967 | 0.908 | 0.816 | 0.879 |
| 350 | 0.020 | 0.031 | 0.714 | 0.943 | |||||||
| 400 | 0.016 | 0.022 | 0.752 | 0.821 | |||||||
| 450 | 0.013 | 0.018 | 0.732 | 0.816 | |||||||
| 500 | 0.013 | 0.025 | 0.914 | 1.155 | |||||||
| B0-Field | Numerical Phantom | Length Increase | Diameter Increase | Diameter and Length Increase |
|---|---|---|---|---|
| 3.0 T | Cylindrical phantom model | 35.813% | −184.944% | −70.2712% |
| Human body model | 23.737% | −155.585% | −67.5715% | |
| 7.0 T | Cylindrical phantom model | 33.633% | −248.214% | −56.574% |
| Human body model | 34.645% | −101.44% | −9.492 | |
| 11.7 T | Cylindrical phantom model | −54.448% | −215.118% | −441.132 |
| Human body model | 14.510% | −49.0946% | −26.773 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Seo, J.-H.; Chung, J.-Y. A Preliminary Study for Reference RF Coil at 11.7 T MRI: Based on Electromagnetic Field Simulation of Hybrid-BC RF Coil According to Diameter and Length at 3.0, 7.0 and 11.7 T. Sensors 2022, 22, 1512. https://doi.org/10.3390/s22041512
Seo J-H, Chung J-Y. A Preliminary Study for Reference RF Coil at 11.7 T MRI: Based on Electromagnetic Field Simulation of Hybrid-BC RF Coil According to Diameter and Length at 3.0, 7.0 and 11.7 T. Sensors. 2022; 22(4):1512. https://doi.org/10.3390/s22041512
Chicago/Turabian StyleSeo, Jeung-Hoon, and Jun-Young Chung. 2022. "A Preliminary Study for Reference RF Coil at 11.7 T MRI: Based on Electromagnetic Field Simulation of Hybrid-BC RF Coil According to Diameter and Length at 3.0, 7.0 and 11.7 T" Sensors 22, no. 4: 1512. https://doi.org/10.3390/s22041512
APA StyleSeo, J.-H., & Chung, J.-Y. (2022). A Preliminary Study for Reference RF Coil at 11.7 T MRI: Based on Electromagnetic Field Simulation of Hybrid-BC RF Coil According to Diameter and Length at 3.0, 7.0 and 11.7 T. Sensors, 22(4), 1512. https://doi.org/10.3390/s22041512






