A Preliminary Exploration of the Placental Position Influence on Uterine Electromyography Using Fractional Modelling
Abstract
:1. Introduction
2. Materials and Methods
3. Results
4. Discussion and Conclusions
- The mentioned remodelling could be in favour of a decreased myometrial impedance in cases where the placenta is anterior as a result of large uteroplacental vessels implantation, allowing increased placental blood flow [63]. This is in accordance with the Nyquist plot results in Figure 7, whereas the impedance range variation for both the resistance and reactance for the anterior placental case is lower compared to the non-anterior placental case.
- Regarding the intriguing increase in the frequency for the peak impedance value (Figure 8) in the anterior placenta case (0.261 Hz), relative to the non-anterior placenta case (0.246 Hz), further studies are required in this respect. The herein presented fractional circuit model may be beneficial for the understanding of this behaviour.
- Lower energy levels for anterior placenta, as reported in Figure 2 and Figure 5, may be due to local hormonal inhibitory influence of the placenta that blocks the propagation of uterine contractile activity [10]. Kanda et al. [64] concluded that, in rats, the muscular activity in the placental region is significantly inhibited until the last stage of pregnancy.
- Low energy levels identified in our study may also be a result of blocked propagation of electrical activity from the cells of the non-placental region to the placental region [10] presumably to avoid placenta abruption.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Marque, C.K.; Terrien, J.; Rihana, S.; Germain, G. Preterm Labour Detection by Use of a Biophysical Marker: The Uterine Electrical Activity. BMC Pregnancy Childbirth 2007, 7, S5. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Selvaraju, V.; Karthick, P.A.; Swaminathan, R. Analysis of Frequency Bands of Uterine Electromyography Signals for the Detection of Preterm Birth. In Public Health and Informatics: Proceedings of Medical Informatics Europe 2021; IOS Press: Athens, Greece, 2021; Volume 281, pp. 283–287. ISBN 9781643681856. [Google Scholar]
- Hadar, E.; Biron-Shental, T.; Gavish, O.; Raban, O.; Yogev, Y. A Comparison between Electrical Uterine Monitor, Tocodynamometer and Intra Uterine Pressure Catheter for Uterine Activity in Labor. J. Matern. Neonatal Med. 2015, 28, 1367–1374. [Google Scholar] [CrossRef] [PubMed]
- Euliano, T.Y.; Nguyen, M.T.; Darmanjian, S.; McGorray, S.P.; Euliano, N.; Onkala, A.; Gregg, A.R. Monitoring Uterine Activity during Labor: A Comparison of 3 Methods. Am. J. Obstet. Gynecol. 2013, 208, 66.e1–66.e6. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vlemminx, M.W.C.; Thijssen, K.M.J.; Bajlekov, G.I.; Dieleman, J.P.; Der Hout-Van Der Jagt, V.; Beatrijs, M.; Oei, S.G. Could Electrohysterography Be the Solution for External Uterine Monitoring in Obese Women? J. Perinatol. 2018, 38, 580–586. [Google Scholar] [CrossRef] [PubMed]
- Duchene, J.; Devedeux, D.; Mansour, S.; Marque, C. Analyzing Uterine EMG: Tracking Instantaneous Burst Frequency. IEEE Eng. Med. Biol. Mag. 1995, 14, 125–132. [Google Scholar] [CrossRef]
- Aina-Mumuney, A.; Hwang, K.; Sunwoo, N.; Burd, I.; Blakemore, K. The Impact of Maternal Body Mass Index and Gestational Age on the Detection of Uterine Contractions by Tocodynamometry. Reprod. Sci. 2016, 23, 638–643. [Google Scholar] [CrossRef] [Green Version]
- Hiersch, L.; Salzer, L.; Aviram, A.; Ben-Haroush, A.; Ashwal, E.; Yogev, Y. Factors Affecting Uterine Electrical Activity during the Active Phase of Labor prior to Rupture of Membranes. J. Matern. Neonatal Med. 2015, 28, 1633–1636. [Google Scholar] [CrossRef]
- Mc Dermott, I.; Breslin, E.; Saade, G.; Garfield, R.; Thornton, S. Comparison of Electromyographic Recordings during Labor in Women with an Anterior or Posterior Placenta. Am. J. Perinatol. 2010, 27, 325–326. [Google Scholar] [CrossRef]
- Kavšek, G.; Pajntar, M.; Leskošek, B. Electromyographic Activity of the Uterus Above the Placental Implantation Site. Gynecol. Obstet. Investig. 1999, 48, 81–84. [Google Scholar] [CrossRef]
- Fele-Žorž, G.; Kavšek, G.; Novak-Antolič, Ž.; Jager, F. A Comparison of Various Linear and Non-Linear Signal Processing Techniques to Separate Uterine EMG Records of Term and Pre-Term Delivery Groups. Med. Biol. Eng. Comput. 2008, 46, 911–922. [Google Scholar] [CrossRef]
- Grgic, O.; Matijevic, R.; Vasilj, O. Placental Site Does Not Change Background Uterine Electromyographic Activity in the Middle Trimester of Pregnancy. Eur. J. Obs. Gynecol. Reprod. Biol. 2006, 127, 209–212. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Avis, N.J.; Lindow, S.W.; Kleinermann, F. In Vitro Multifrequency Electrical Impedance Measurements and Modelling of the Cervix in Late Pregnancy. Physiol. Meas. 1996, 17 (Suppl. 4A), A97. [Google Scholar] [CrossRef] [PubMed]
- Gandhi, S.V.; Walker, D.; Milnes, P.; Mukherjee, S.; Brown, B.H.; Anumba, D.O.C. Electrical Impedance Spectroscopy of the Cervix in Non-Pregnant and Pregnant Women. Eur. J. Obs. Gynecol. Reprod. Biol. 2006, 129, 145–149. [Google Scholar] [CrossRef] [PubMed]
- Kilbas, A.; Srivastava, H.; Trujillo, J. Theory and Applications of Fractional Differential Equations, 1st ed.; Elsevier Science Inc.: New York, NY, USA, 2006; ISBN 9780444518323. [Google Scholar]
- Monje, C.A.; Chen, Y.; Vinagre, B.M.; Xue, D.; Feliu, V. Fractional-Order Systems and Controls. In Advances in Industrial Control, 1st ed.; Springer: London, UK, 2010; ISBN 978. [Google Scholar]
- Ortigueira, M.D. Fractional Calculus for Scientists and Engineers. In Lecture Notes in Electrical Engineering, 1st ed.; Springer: Dordrecht, The Netherlands, 2011; ISBN 978. [Google Scholar]
- Podlubny, I. Fractional Differential Equations-An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solutions and Some of Their Applications, 1st ed.; Academic Press: San Diego, CA, USA, 1998. [Google Scholar]
- Herrmann, R. Fractional Calculus An Introduction for Physicists, 2nd ed.; World Scientific Publishing: Singapore, 2014; ISBN 978/981/3274/57/0. [Google Scholar]
- Samko, S.G.; Kilbas, A.A.; Marichev, O.I. Fractional Integrals and Derivatives, 1st ed.; Gordon and Breach Science Publishers: New York, NY, USA, 1993. [Google Scholar]
- Tenreiro Machado, J.A.; Silva, M.F.; Barbosa, R.S.; Jesus, I.S.; Reis, C.M.; Marcos, M.G.; Galhano, A.F. Some Applications of Fractional Calculus in Engineering. Math. Probl. Eng. 2010, 2010, 639801. [Google Scholar] [CrossRef] [Green Version]
- Martynyuk, V.; Ortigueira, M.; Fedula, M.; Savenko, O. Fractional Model of the Electrochemical Capacitor Relaxation Phenomenon. Bull. Polish Acad. Sci. Tech. Sci. 2018, 66, 441–448. [Google Scholar] [CrossRef]
- Muresan, C.I.; Birs, I.R.; Dulf, E.H. Event-Based Implementation of Fractional Order IMC Controllers for Simple FOPDT Processes. Mathematics 2020, 8, 1378. [Google Scholar] [CrossRef]
- Dulf, E.-H.; Vodnar, D.C.; Danku, A.; Muresan, C.-I.; Crisan, O. Fractional-Order Models for Biochemical Processes. Fractal Fract. 2020, 4, 12. [Google Scholar] [CrossRef] [Green Version]
- Freeborn, T.J.; Maundy, B.; Elwakil, A.S. Measurement of Supercapacitor Fractional-Order Model Parameters From Voltage-Excited Step Response. IEEE J. Emerg. Sel. Top. Circuits Syst. 2013, 3, 367–376. [Google Scholar] [CrossRef]
- Freeborn, T.J.; Maundy, B.; Elwakil, A.S. Fractional-Order Models of Supercapacitors, Batteries and Fuel Cells: A Survey. Mater. Renew. Sustain. Energy 2015, 4, 9. [Google Scholar] [CrossRef] [Green Version]
- Lewandowski, M.; Orzyłowski, M. Fractional-Order Models: The Case Study of the Supercapacitor Capacitance Measurement. Bull. Polish Acad. Sci. Tech. Sci. 2017, 65, 449–457. [Google Scholar] [CrossRef] [Green Version]
- Martynyuk, V.; Ortigueira, M. Fractional Model of an Electrochemical Capacitor. Signal Processing 2015, 107, 355–360. [Google Scholar] [CrossRef]
- Elwakil, A. Fractional-Order Circuits and Systems: An Emerging Interdisciplinary Research Area. IEEE Circuits Syst. Mag. 2010, 10, 40–50. [Google Scholar] [CrossRef]
- Cole, K.S.; Cole, R.H. Dispersion and Absorption in Dielectrics II. Direct Current Characteristics. J. Chem. Phys. 1942, 10, 98–105. [Google Scholar] [CrossRef]
- Fouda, M.E.; Khorshid, A.E.; Alquaydheb, I.; Eltawil, A.; Kurdahi, F. Extracting the Cole-Cole Model Parameters of Tissue-Mimicking Materials. In Proceedings of the 2018 IEEE Biomedical Circuits and Systems Conference (BioCAS), Cleveland, OH, USA, 17–19 October 2018; pp. 1–4. [Google Scholar]
- Freeborn, T.J.; Maundy, B.; Elwakil, A. Improved Cole-Cole Parameter Extraction from Frequency Response Using Least Squares Fitting. In Proceedings of the 2012 IEEE International Symposium on Circuits and Systems, Seoul, Korea, 20–23 May 2012; Volume 2, pp. 337–340. [Google Scholar]
- Freeborn, T.J.; Maundy, B.; Elwakil, A.S. Least Squares Estimation Technique of Cole-Cole Parameters from Step Response. Electron. Lett. 2012, 48, 752. [Google Scholar] [CrossRef]
- Freeborn, T.J. A Survey of Fractional-Order Circuit Models for Biology and Biomedicine. IEEE J. Emerg. Sel. Top. Circuits Syst. 2013, 3, 416–424. [Google Scholar] [CrossRef]
- Elwakil, A.S.; Maundy, B. Extracting the Cole-Cole Impedance Model Parameters without Direct Impedance Measurement. Electron. Lett. 2010, 46, 1367. [Google Scholar] [CrossRef]
- McRae, D.A.; Esrick, M.A.; Mueller, S.C. Changes in the Noninvasive, in Vivo Electrical Impedance of Three Xenografts during the Necrotic Cell-Response Sequence. Int. J. Radiat. Oncol. 1999, 43, 849–857. [Google Scholar] [CrossRef]
- Eldarrat, A.H.; Wood, D.J.; Kale, G.M.; High, A.S. Age-Related Changes in Ac-Impedance Spectroscopy Studies of Normal Human Dentine. J. Mater. Sci. Mater. Med. 2007, 18, 1203–1210. [Google Scholar] [CrossRef]
- Sezdi, M.; Bayik, M.; Ulgen, Y. Storage Effects on the Cole-Cole Parameters of Erythrocyte Suspensions. Physiol. Meas. 2006, 27, 623–635. [Google Scholar] [CrossRef]
- Ionescu, C.M.; Machado, J.A.T.; De Keyser, R. Modeling of the Lung Impedance Using a Fractional-Order Ladder Network With Constant Phase Elements. IEEE Trans. Biomed. Circuits Syst. 2011, 5, 83–89. [Google Scholar] [CrossRef]
- Copot, D.; De Keyser, R.; Derom, E.; Ortigueira, M.; Ionescu, C.M. Reducing Bias in Fractional Order Impedance Estimation for Lung Function Evaluation. Biomed. Signal Process. Control 2018, 39, 74–80. [Google Scholar] [CrossRef]
- Guermazi, M.; Kanoun, O.; Derbel, N. Investigation of Long Time Beef and Veal Meat Behavior by Bioimpedance Spectroscopy for Meat Monitoring. IEEE Sens. J. 2014, 14, 3624–3630. [Google Scholar] [CrossRef]
- Westerlund, S.; Ekstam, L. Capacitor Theory. IEEE Trans. Dielectr. Electr. Insul. 1994, 1, 826–839. [Google Scholar] [CrossRef]
- Lazarević, M.; Cajić, M.; Đurović, N. Biomechanical Modelling and Simulation of Soft Tissues Using Fractional Memristive Elements. In Proceedings of the Electronic 8th GRACM International Congress in Computational Mechanics, Volos, Greece, 12–15 July 2015; pp. 1–10. [Google Scholar]
- Rigaud, B.; Hamzaoui, L.; Frikha, M.R.; Chauveau, N.; Morucci, J.P. In Vitro Tissue Characterization and Modelling Using Electrical Impedance Measurements in the 100 Hz-10 MHz Frequency Range. Physiol. Meas. 1995, 16, A15–A28. [Google Scholar] [CrossRef] [PubMed]
- Alexandersson, A.; Steingrimsdottir, T.; Terrien, J.; Marque, C.; Karlsson, B. The Icelandic 16-Electrode Electrohysterogram Database. Sci. Data 2015, 2, 150017. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Esgalhado, F.; Batista, A.G.; Mouriño, H.; Russo, S.; Palma dos Reis, C.R.; Serrano, F.; Vassilenko, V.; Ortigueira, M. Uterine Contractions Clustering Based on Electrohysterography. Comput. Biol. Med. 2020, 123, 103897. [Google Scholar] [CrossRef]
- Batista, A.G.; Cebola, R.; Esgalhado, F.; Russo, S.; dos Reis, C.R.P.; Serrano, F.; Vassilenko, V.; Ortigueira, M. The Contractiongram: A Method for the Visualization of Uterine Contraction Evolution Using the Electrohysterogram. Biomed. Signal Process. Control 2021, 67, 102531. [Google Scholar] [CrossRef]
- Esgalhado, F.; Batista, A.G.; Mouriño, H.; Russo, S.; dos Reis, C.R.P.; Serrano, F.; Vassilenko, V.; Duarte Ortigueira, M. Automatic Contraction Detection Using Uterine Electromyography. Appl. Sci. 2020, 10, 7014. [Google Scholar] [CrossRef]
- Batista, A.G.; Najdi, S.; Godinho, D.M.; Martins, C.; Serrano, F.C.; Ortigueira, M.D.; Rato, R.T. A Multichannel Time–frequency and Multi-Wavelet Toolbox for Uterine Electromyography Processing and Visualisation. Comput. Biol. Med. 2016, 76, 178–191. [Google Scholar] [CrossRef]
- Russo, S.; Batista, A.; Esgalhado, F.; Palma dos Reis, C.R.; Serrano, F.; Vassilenko, V.; Ortigueira, M. Alvarez Waves in Pregnancy: A Comprehensive Review. Biophys. Rev. 2021, 13, 563–574. [Google Scholar] [CrossRef]
- Marquel, C.; Gondty, J.; Rossi, J.; Baaklini, N. Surveillance Des Grossesses À Risque Par Électromyographie Uterine. RBM-News 1995, 17, 25–31. [Google Scholar] [CrossRef]
- Roberts, W.F.; Perry, K.G.; Naef, R.W.; Washburne, J.F.; Morrison, J.C. The Irritable Uterus: A Risk Factor for Preterm Birth? Am. J. Obstet. Gynecol. 1995, 172, 138–142. [Google Scholar] [CrossRef]
- Welch, P. The Use of Fast Fourier Transform for the Estimation of Power Spectra: A Method Based on Time Averaging over Short, Modified Periodograms. IEEE Trans. Audio Electroacoust. 1967, 15, 70–73. [Google Scholar] [CrossRef] [Green Version]
- Ulgen, Y.; Sezdi, M. Hematocrit Dependence of the Cole-Cole Parameters of Human Blood. In Proceedings of the 1998 2nd International Conference Biomedical Engineering Days, Istanbul, Turkey, 22–22 May 1998; pp. 71–74. [Google Scholar]
- Levenberg, K. A Method for the Solution of Certain Non-Linear Problems in Least Squares. Q. Appl. Math. 1944, 2, 164–168. [Google Scholar] [CrossRef] [Green Version]
- Marquardt, D.W.; Marquardtt, D.W. An Algorithm for Least-Squares Estimation of Nonlinear Parameters. J. Soc. Ind. Appl. Math. 1963, 11, 431–441. [Google Scholar] [CrossRef]
- Brosens, I.; Robertson, W.B.; Dixon, H.G. The Physiological Response of the Vessels of the Placental Bed to Normal Pregnancy. J. Pathol. Bacteriol. 1967, 93, 569–579. [Google Scholar] [CrossRef]
- Medicine, S.; George, S. Uteroplacental Arterial Changes Related to Interstitial Trophoblast Migration in Early Human Pregnancy. Placenta 1983, 4, 397–413. [Google Scholar]
- Blankenship, T.N.; Enders, A.C.; King, B.F. Trophoblastic Invasion and Modification of Uterine Veins during Placental Development in Macaques. Cell Tissue Res. 1993, 274, 135–144. [Google Scholar] [CrossRef]
- Ziekenhuis, A. Trophoblastic Invasion of Human Decidua From 8 to 18 Weeks of Pregnancy. Placenta 1980, 1, 3–19. [Google Scholar]
- Sheppard, B.L.; Bonnar, J. the ultrastructure of the arterial supply of the human placenta in pregnancy complicated by fetal growth retardation. BJOG Int. J. Obstet. Gynaecol. 1976, 83, 948–959. [Google Scholar] [CrossRef]
- Khong, T.Y.; Wolf, F.; Robertson, W.B.; Brosens, I. Inadequate Maternal Vascular Response to Placentation in Pregnancies Complicated by Pre-Eclampsia and by Small-for-Gestational Age Infants. BJOG Int. J. Obstet. Gynaecol. 1986, 93, 1049–1059. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Zhao, S. Chapter 2: Placental Blood Circulation. In Vascular Biology of the Placenta; Morgan & Claypool Life Sciences: San Rafael, CA, USA, 2010; pp. 1–5. [Google Scholar]
- Kanda, B.Y.S.; Kuriyama, H. Specific Features of Smooth Muscle Cells Recorded from the Placental Region of the Myometrium of Pregnant Rats. J. Physiol. 1980, 50, 127–144. [Google Scholar] [CrossRef] [PubMed] [Green Version]
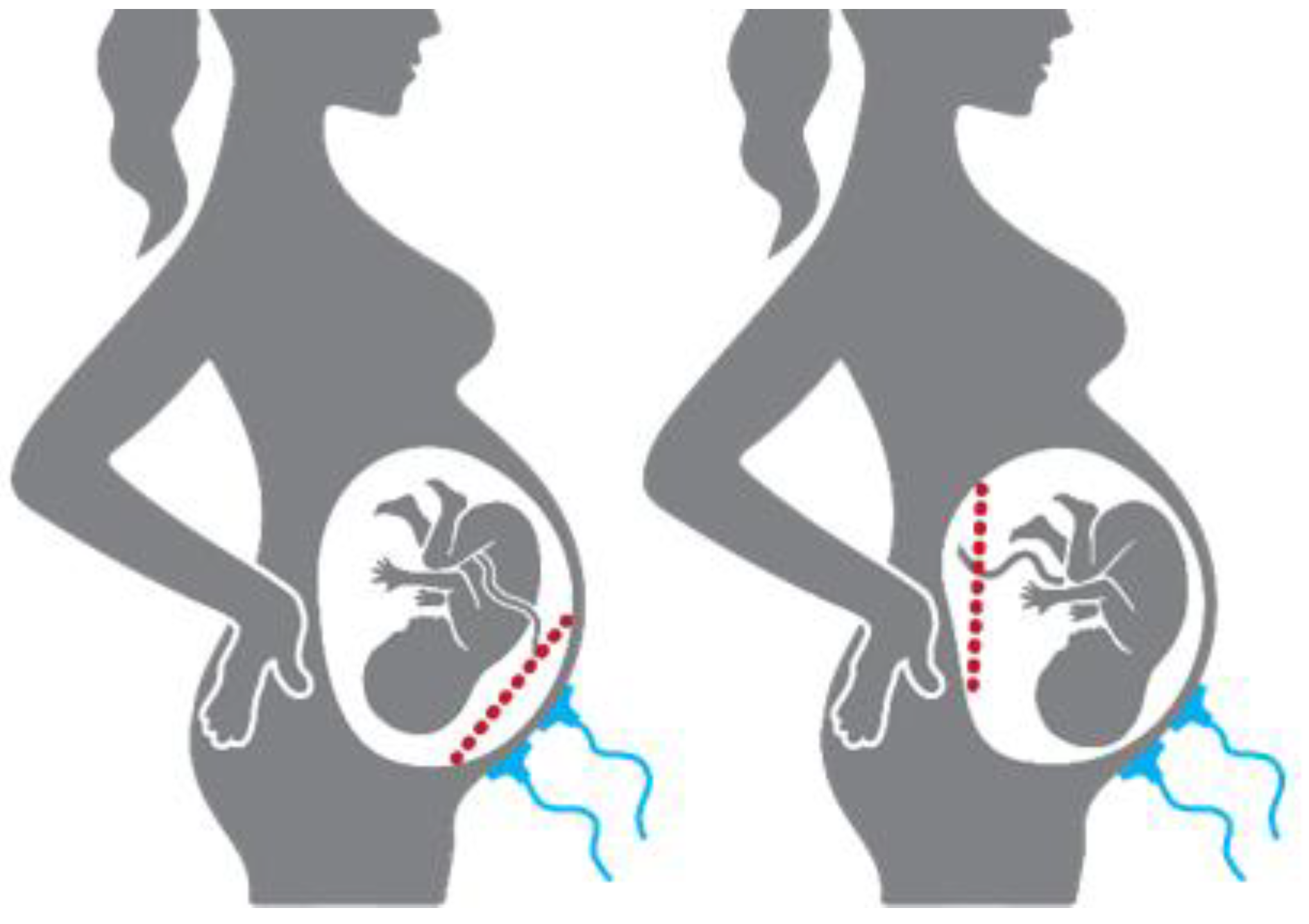
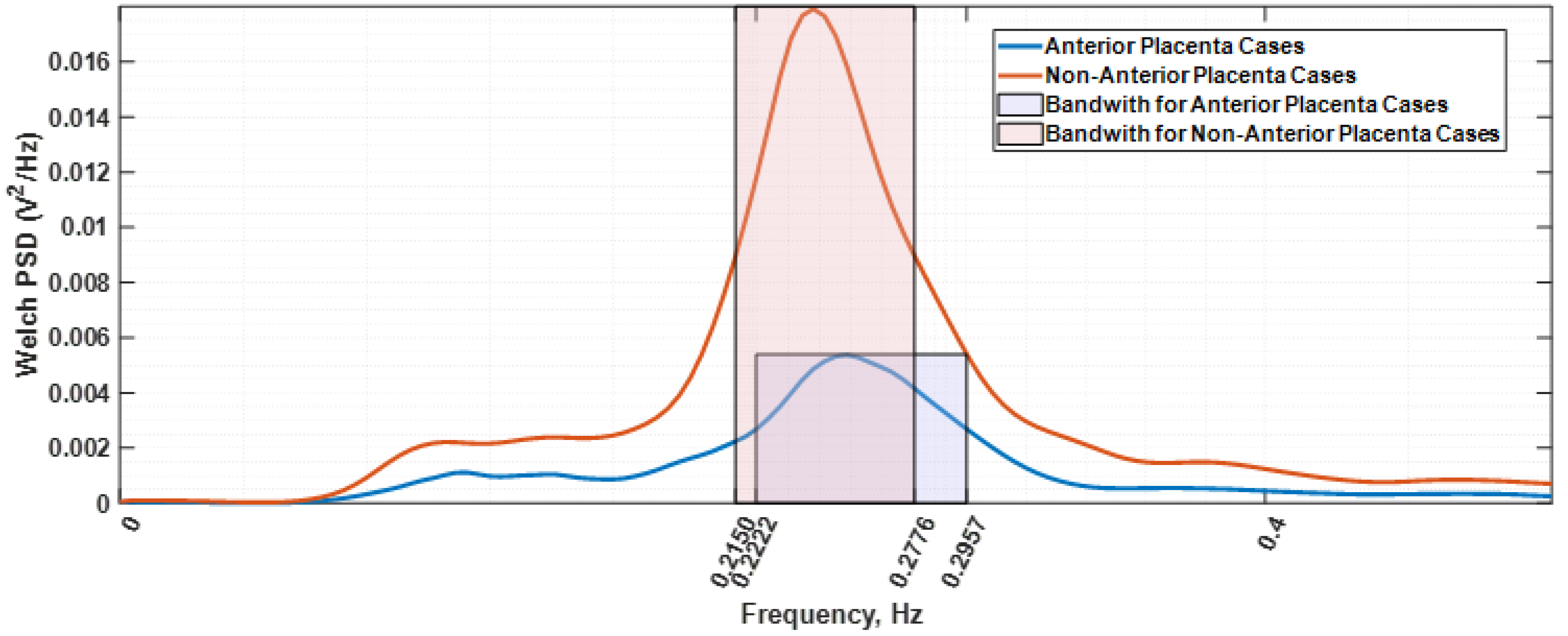




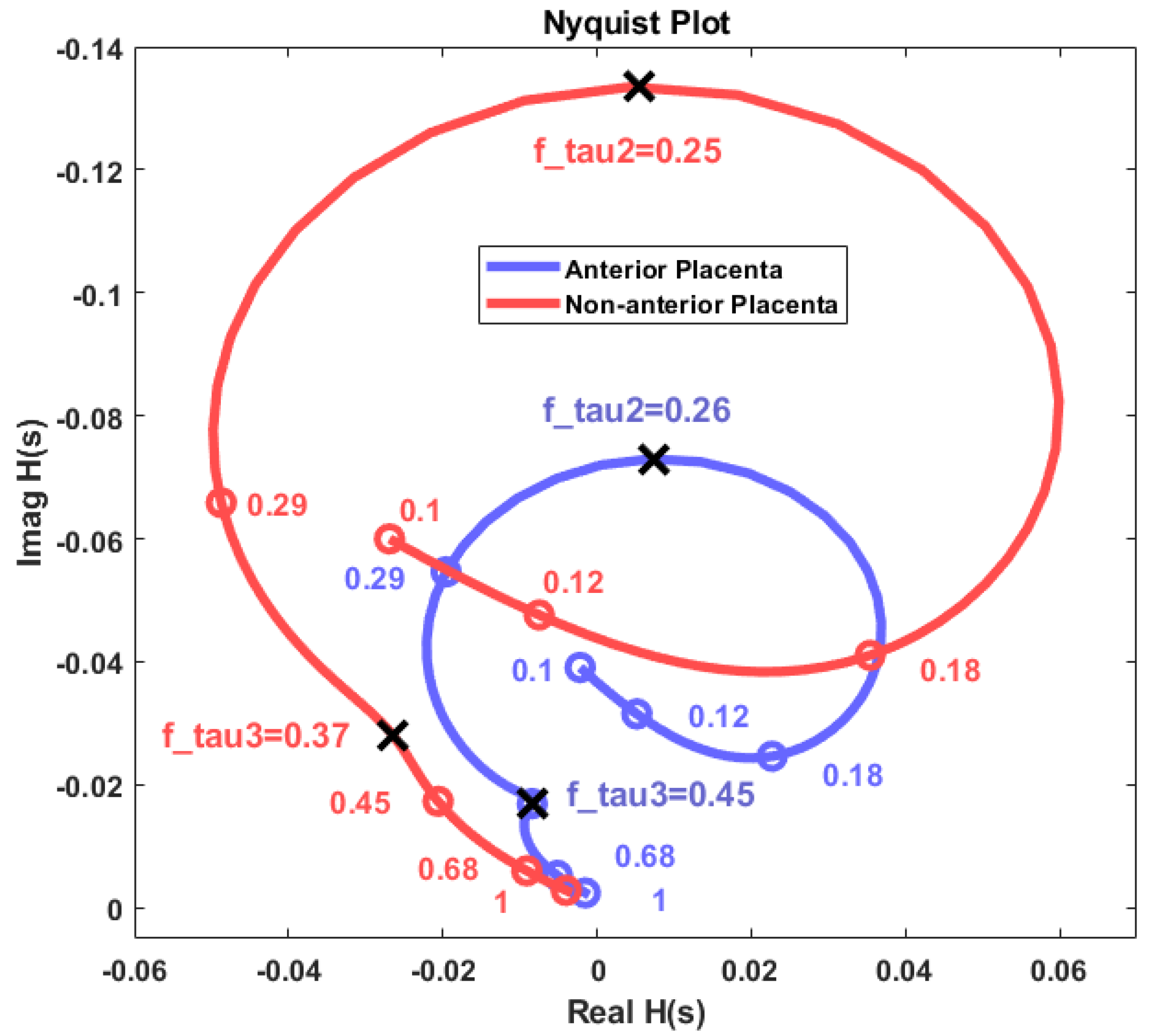
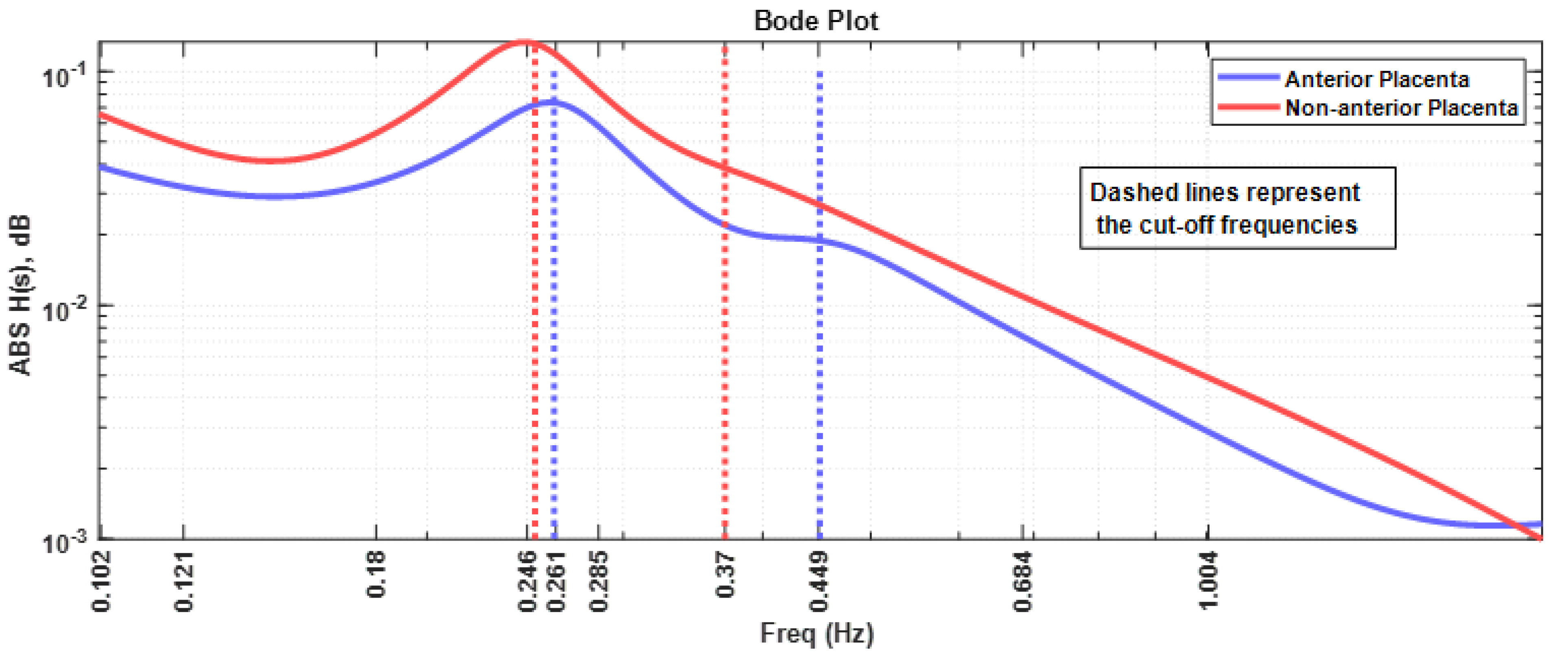
| Parameters | Mean Standard Deviation |
|---|---|
| Maternal age (years) | 28.87 5.63 |
| Gestational age at delivery (weeks) | 39.76 1.40 |
| Pre-gestational BMI | 25.82 4.80 |
| Gravidity | 2.62 1.45 |
| Parity | 0.91 0.92 |
| RMS (mV) | 0.04 0.02 |
| Minimum RMS (mV) | 0.02 |
| Maximum RMS (mV) | 0.13 |
| Anterior Placenta | 24 subjects (70 recordings) |
| Non-Anterior Placenta | 21 subjects (51 recordings) |
| Case | R0 (Ω) | R1 (Ω) | R2 (Ω) | R3 (Ω) | C1 (F/ ) | C2 (F/ ) | C3 (F/ ) | α1 | α2 | α3 |
|---|---|---|---|---|---|---|---|---|---|---|
| Anterior Placenta | 1.00 × 10−3 | 5.00 × 103 | 27.30 × 10−3 | 5.10 × 10−3 | 22.61 | 16.38 | 46.37 | 1.548 | 1.850 | 1.694 |
| Non-Anterior Placenta | 1.80 × 10−3 | 4.93 × 103 | 15.70 × 10−3 | 3.20 × 10−3 | 40.30 | 25.51 | 50.00 | 1.387 | 1.838 | 1.764 |
| Anterior Placenta | Non-Anterior Placenta | |
|---|---|---|
| τ1 | 6.60 × 103 | 1.83 × 103 |
| τ2 | 0.609 | 0.648 |
| τ3 | 0.356 | 0.431 |
| Cut-off freq. for τ1 (Hz) | 2.41 × 10−5 | 8.66 × 10−5 |
| Cut-off freq. for τ2 (Hz) | 0.26 | 0.25 |
| Cut-off freq. for τ3 (Hz) | 0.45 | 0.37 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Şan, M.; Batista, A.; Russo, S.; Esgalhado, F.; dos Reis, C.R.P.; Serrano, F.; Ortigueira, M. A Preliminary Exploration of the Placental Position Influence on Uterine Electromyography Using Fractional Modelling. Sensors 2022, 22, 1704. https://doi.org/10.3390/s22051704
Şan M, Batista A, Russo S, Esgalhado F, dos Reis CRP, Serrano F, Ortigueira M. A Preliminary Exploration of the Placental Position Influence on Uterine Electromyography Using Fractional Modelling. Sensors. 2022; 22(5):1704. https://doi.org/10.3390/s22051704
Chicago/Turabian StyleŞan, Müfit, Arnaldo Batista, Sara Russo, Filipa Esgalhado, Catarina R. Palma dos Reis, Fátima Serrano, and Manuel Ortigueira. 2022. "A Preliminary Exploration of the Placental Position Influence on Uterine Electromyography Using Fractional Modelling" Sensors 22, no. 5: 1704. https://doi.org/10.3390/s22051704
APA StyleŞan, M., Batista, A., Russo, S., Esgalhado, F., dos Reis, C. R. P., Serrano, F., & Ortigueira, M. (2022). A Preliminary Exploration of the Placental Position Influence on Uterine Electromyography Using Fractional Modelling. Sensors, 22(5), 1704. https://doi.org/10.3390/s22051704







