Various Types of Light Guides for Use in Lossy Mode Resonance-Based Sensors
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Socorro, A.B.; Del Villar, I.; Corres, J.M.; Arregui, F.J.; Matias, I.R. Tapered single-mode optical fiber pH sensor based on lossy mode resonances generated by a polymeric thin-film. IEEE Sens. J. 2012, 12, 2598–2603. [Google Scholar] [CrossRef]
- Cheng, X.; Bonefacino, J.; Guan, B.O.; Tam, H.Y. All-polymer fiber-optic pH sensor. Opt. Express 2018, 26, 14610–14616. [Google Scholar] [CrossRef] [Green Version]
- Gu, B.; Yin, M.-J.; Zhang, A.P.; Qian, J.-W.; He, S. Low-cost high-performance fiber-optic pH sensor based on thin-core fiber modal interferometer. Opt. Express 2009, 17, 22296–22302. [Google Scholar] [CrossRef]
- Socorro, A.B.; Corres, J.M.; Del Villar, I.; Arregui, F.J.; Matias, I.R. Fiber-optic biosensor based on lossy mode resonance. Sens. Actuators B 2012, 174, 263–269. [Google Scholar] [CrossRef]
- Zeni, L.; Perri, C.; Cennamo, N.; Arcadio, F.; D’Agostino, G.; Salmona, M.; Beeg, M.; Gobbi, M. A portable optical-fibre-based surface plasmon resonance biosensor for the detection of therapeutic antibodies in human serum. Sci. Rep. 2020, 10, 11154. [Google Scholar] [CrossRef]
- Leitão, C.; Pereira, S.O.; Marques, C.; Cennamo, N.; Zeni, L.; Shaimerdenova, M.; Ayupova, T.; Tosi, D. Cost-effective fiber optic solutions for biosensing. Biosensors 2022, 12, 575. [Google Scholar] [CrossRef]
- Wang, Y.; Shen, C.; Lou, W.; Shentu, F.; Zhong, C.; Dong, X.; Tong, L. Fiber optic relative humidity sensor based on the tilted fiber Bragg grating coated with graphene oxide. Appl. Phys. Lett. 2016, 109, 031107. [Google Scholar] [CrossRef]
- Zhang, E.; Lu, D.; Zhang, S.; Gui, X.; Guan, H.; Zhang, Z.; Lin, Y.; Ming, J.; Hong, J.; Dong, J.; et al. High-sensitivity fiber-optic humidity sensor based on microfiber overlaid with niobium disulfide. J. Mater. Sci. 2020, 55, 16576–16587. [Google Scholar] [CrossRef]
- Limodehi, H.E.; Légaré, F. Fiber optic humidity sensor using water vapor condensation. Opt. Express 2017, 25, 15313–15321. [Google Scholar] [CrossRef]
- Sanchez, P.; Mendizabal, D.; Zamarreño, C.R.; Matias, I.R.; Arregui, F.J. Indium-Tin-Oxide-Coated optical fibers for temperature- viscosity sensing applications in synthetic lubricant oil. Proc. SPIE 2015, 9634, 1024–1027. [Google Scholar] [CrossRef]
- Hu, T.; Zhao, Y.; Song, A. Fiber optic SPR sensor for refractive index and temperature measurement based on MMF-FBG-MMF structure. Sens. Actuators B Chem. 2016, 237, 521–525. [Google Scholar] [CrossRef]
- Gao, S.; Ji, C.; Ning, Q.; Chen, W.; Li, J. High-sensitive Mach-Zehnder interferometric temperature fiber-optic sensor based on core-offset splicing technique. Opt. Fiber Technol. 2020, 56, 102202. [Google Scholar] [CrossRef]
- Yin, M.-J.; Gu, B.; An, Q.-F.; Yang, C.; Guan, Y.L.; Yong, K.-T. Recent development of fiber-optic chemical sensors and biosensors: Mechanisms, materials, micro/nano-fabrications and applications. Coord. Chem. Rev. 2018, 376, 348–392. [Google Scholar] [CrossRef]
- Devendiran, S.; Priya, A.K.; Sastikumar, D. Design of aluminium oxide (Al2O3) fiber optic gas sensor based on detection of refracted light in evanescent mode from the side-polished modified clad region. Sens. Actuators B Chem. 2022, 361, 131738. [Google Scholar] [CrossRef]
- Vitoria, I.; Ruiz Zamarreño, C.; Ozcariz, A.; Matias, I.R. Fiber optic gas sensors based on lossy mode resonances and sensing materials used therefor: A comprehensive review. Sensors 2021, 21, 731. [Google Scholar] [CrossRef] [PubMed]
- Yan, A.; Huang, S.; Li, S.; Chen, R.; Ohodnicki, P.; Chen, K. Fiber optical methane sensors using functional metal oxide nanomaterials. In Proceedings of the Asia-Pacific Optical Sensors Conference, Shanghai, China, 11–14 October 2016; p. W4A.12. [Google Scholar] [CrossRef]
- Gordon, J.D.; Lowder, T.L.; Selfridge, R.H.; Schultz, S.M. Optical D-fiber-based volatile organic compound sensor. Appl. Opt. 2007, 46, 7805–7810. [Google Scholar] [CrossRef]
- Naku, W.; Nambisan, A.K.; Roman, M.; Zhu, C.; Gerald, R.E., II; Huang, J. Identification of volatile organic liquids by combining an array of fiber-optic sensors and machine learning. ACS Omega 2023, 8, 4597–4607. [Google Scholar] [CrossRef]
- Ascorbe, J.; Corres, J.M.; Matias, I.R.; Arregui, F.J. High sensitivity humidity sensor based on cladding-etched optical fiber and lossy mode resonances. Sens. Actuators B 2016, 233, 7–16. [Google Scholar] [CrossRef] [Green Version]
- Zhu, S.; Pang, F.; Huang, S.; Zou, F.; Dong, Y.; Wang, T. High sensitivity refractive index sensor based on adiabatic tapered optical fiber deposited with nanofilm by ALD. Opt. Express 2015, 23, 13880–13888. [Google Scholar] [CrossRef]
- Ozcariz, A.; Dominik, M.; Smietana, M.; Zamarreño, C.R.; Del Villar, I.; Arregui, F.J. Lossy mode resonance optical sensors based on indium-gallium-zinc oxide thin film. Sens. Actuators A 2019, 290, 20–27. [Google Scholar] [CrossRef]
- Rees, N.D.; James, S.W.; Tatam, R.P.; Ashwell, G.J. Optical fiber long-period gratings with Langmuir Blodgett thin-film overlays. Opt. Lett. 2002, 27, 686–688. [Google Scholar] [CrossRef]
- Del Villar, I.; Matías, I.; Arregui, F.; Lalanne, P. Optimization of sensitivity in Long Period Fiber Gratings with overlay deposition. Opt. Express 2005, 13, 56–69. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zou, F.; Liu, Y.; Deng, C.; Dong, Y.; Zhu, S.; Wang, T. Refractive index sensitivity of nano-film coated longperiod fiber gratings. Opt. Express 2015, 23, 1114–1124. [Google Scholar] [CrossRef] [Green Version]
- Zheltikov, A.M. Ray-optic analysis of the (bio)sensing ability of ring-cladding hollow waveguides. Appl. Opt. 2008, 47, 474–479. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.; Pang, F.; Dong, Y.; Wen, J.; Chen, Z.; Wang, T. Refractive index sensitivity enhancement of optical fiber cladding mode by depositing nanofilm via ALD technology. Opt. Express 2013, 21, 26136–26143. [Google Scholar] [CrossRef] [PubMed]
- Del Villar, I.; Zamarreño, C.R.; Hernáez, M.; Arregui, F.J.; Matías, I.R. Lossy mode resonance generation with Indium Tin Oxide coated optical fibers for sensing applications. J. Light. Technol. 2010, 28, 111–117. [Google Scholar] [CrossRef] [Green Version]
- Paliwal, N.; John, J. Lossy Mode Resonance (LMR) Based Fiber Optic Sensors: A Review. IEEE Sens. J. 2015, 15, 5361–5371. [Google Scholar] [CrossRef]
- Del Villar, I.; Arregui, F.J.; Zamarreño, C.R.; Corres, J.M.; Bariain, C.; Goicoechea, J.; Elosua, C.; Hernaez, M.; Rivero, P.J.; Socorro, A.B.; et al. Optical sensors based on lossy-mode resonances. Sens. Actuators B 2017, 240, 174–185. [Google Scholar] [CrossRef]
- Wang, Q.; Zhao, W.-M. A comprehensive review of lossy mode resonance-based fber optic sensors. Opt. Lasers Eng. 2018, 100, 47–60. [Google Scholar] [CrossRef]
- Savelyev, E. Sensitivity of lossy mode resonance-based optical fiber sensors as a function of the coating material refractive index. Eur. Phys. J. D 2021, 75, 285. [Google Scholar] [CrossRef]
- Zamarreno, C.R.; Sanchez, P.; Hernaez, M.; Del Villar, I.; Fernandez-Valdivielso, C.; Matias, I.R.; Arregui, F.J. Sensing properties of indium oxide coated optical fiber devices based on lossy mode resonances. IEEE Sens. J. 2012, 12, 151–155. [Google Scholar] [CrossRef]
- Del Villar, I.; Socorro, A.B.; Hernaez, M.; Corres, J.M.; Zamarreño, C.R.; Sanchez, P.; Arregui, F.J.; Matias, I.R. Sensors based on thin-film coated cladding removed multimode optical Fiber and single-mode multimode single-mode Fiber: A comparative study. J. Sens. 2015, 2015, 763762. [Google Scholar] [CrossRef] [Green Version]
- Prasanth, A.; Meher, S.R.; Alex, Z.C. Experimental analysis of SnO2 coated LMR based fiber optic sensor for ethanol detection. Opt. Fiber Technol. 2021, 65, 102618. [Google Scholar] [CrossRef]
- Zubiate, P.; Zamarreño, C.R.; Del Villar, I.; Matias, I.R.; Arregui, F.J. High sensitive refractometers based on lossy mode resonances (LMRs) supported by ITO coated D-shaped optical fibers. Opt. Express 2015, 23, 8045–8050. [Google Scholar] [CrossRef] [Green Version]
- Śmietana, M.; Sobaszek, M.; Michalak, B.; Niedziałkowski, P.; Białobrzeska, W.; Koba, M.; Sezemsky, P.; Stranak, V.; Karczewski, J.; Ossowski, T.; et al. Optical monitoring of electrochemical processes with ITO-based lossy-mode resonance optical fiber sensor applied as an electrode. J. Lightwave Technol. 2018, 36, 954–960. [Google Scholar] [CrossRef]
- Sanchez, P.; Zamarreño, C.R.; Hernaez, M.; Matias, I.R.; Arregui, F.J. Optical fiber refractometers based on Lossy Mode Resonances by means of SnO2 sputtered coatings. Sens. Actuators B 2014, 202, 154–159. [Google Scholar] [CrossRef]
- Kuznetsov, P.I.; Sudas, D.P.; Savelyev, E.A. Fiber optic lossy mode resonance based sensor for aggressive liquids. Sens. Actuators A Phys. 2021, 321, 112576. [Google Scholar] [CrossRef]
- Sudas, D.P.; Zakharov, L.Y.; Jitov, V.A.; Golant, K.M. Silicon oxynitride thin film coating to lossy mode resonance fiber-optic refractometer. Sensors 2022, 22, 3665. [Google Scholar] [CrossRef]
- Pezzotti, G.; Marin, E.; Adachi, T.; Lerussi, F.; Rondinella, A.; Boschetto, F.; Zhu, W.; Kitajima, T.; Inada, K.; McEntire, B.J.; et al. Incorporating Si3N4 into PEEK to Produce Antibacterial, Osteocondutive, and Radiolucent Spinal Implants. Macromol. Biosci. 2018, 18, e1800033. [Google Scholar] [CrossRef]
- Pezzotti, G.; McEntire, B.J.; Bock, R.; Boffelli, M.; Zhu, W.; Vitale, E.; Puppulin, L.; Adachi, T.; Yamamoto, T.; Kanamura, N.; et al. Silicon Nitride: A synthetic mineral for vertebrate biology. Sci. Rep. 2016, 6, 31717. [Google Scholar] [CrossRef] [Green Version]
- Doering, R.; Nishi, Y. (Eds.) Handbook of Semiconductor Manufacturing Technology, 2nd ed.; CRC Press, Inc.: Boca Raton, FL, USA, 2008. [Google Scholar] [CrossRef]
- Ko, S.; Lee, J.; Koo, J.; Joo, B.S.; Gu, M.; Lee, J.H. Chemical wet etching of an optical fiber using a hydrogen fluoride-free solution for a saturable absorber based on the evanescent field interaction. J. Light. Technol. 2016, 34, 3776. [Google Scholar] [CrossRef]
- Kuznetsov, P.I.; Sudas, D.P.; Yapaskurt, V.O.; Savelyev, E.A. Lossy mode resonance fiber-optic sensors based on niobium pentoxide thin film. Opt. Mater. Express 2021, 11, 2650–2664. [Google Scholar] [CrossRef]
- Kovalev, V.; Kuznetzov, P.; Zhitov, V.; Zakharov, L.; Rukovishnikov, A.; Khomich, A.; Yakushcheva, G.; Gaponenko, S. Spectral ellipsometry of multilayer ZnS/ZnSe heterostructures. J. Appl. Spectrosc. 2002, 69, 298–304. [Google Scholar] [CrossRef]
- Savelyev, E.A.; Sudas, D.P.; Kuztestov, P.I. Using lossy mode resonance for in situ measurement of the refractive index of a layer deposited on an optical fiber lateral surface. Opt. Lett. 2022, 47, 361–364. [Google Scholar] [CrossRef] [PubMed]
- Marciniak, M.; Grzegorzewski, J.; Szustakowski, M. Analysis of lossy mode cut-off conditions in planar waveguides with semiconductor guiding layer. IEE Proc. J. Optoelectron. 1993, 140, 247. [Google Scholar] [CrossRef]
- Golant, E.I.; Pashkovskii, A.B.; Golant, K.M. Lossy mode resonance in an etched-out optical fiber taper covered by a thin ITO layer. Appl. Opt. 2020, 59, 9254. [Google Scholar] [CrossRef]
- Roussey, M.; Descrovi, E.; Häyrinen, M.; Angelini, A.; Kuittinen, M.; Honkanen, S. One-dimensional photonic crystals with cylindrical geometry. Opt. Express 2014, 22, 27236–27241. [Google Scholar] [CrossRef]
- Villatoro, J.; Monzón-Hernández, D.; Mejía, E. Fabrication and modeling of uniform-waist single-mode tapered optical fiber sensors. Appl. Opt. 2003, 42, 2278–2283. [Google Scholar] [CrossRef]
- Yariv, A.; Yeh, P. Photonics: Optical Electronics in Modern Communications. In The Oxford Series in Electrical and Computer Engineering, 6th ed.; Oxford University Press: Oxford, UK, 2006; ISBN 978-0195179460. [Google Scholar]
- Kokubun, Y. Lightwave Engineering (Optical Science and Engineering), 1st ed.; CRC Press, Inc.: Boca Raton, FL, USA, 2012; ISBN 978-1420046489. [Google Scholar]
- Kuznetsov, P.I.; Sudas, D.P.; Savelyev, E.A. A study of the lossy mode resonances during the synthesis process of zinc telluride films. Sensors 2022, 22, 8108. [Google Scholar] [CrossRef] [PubMed]



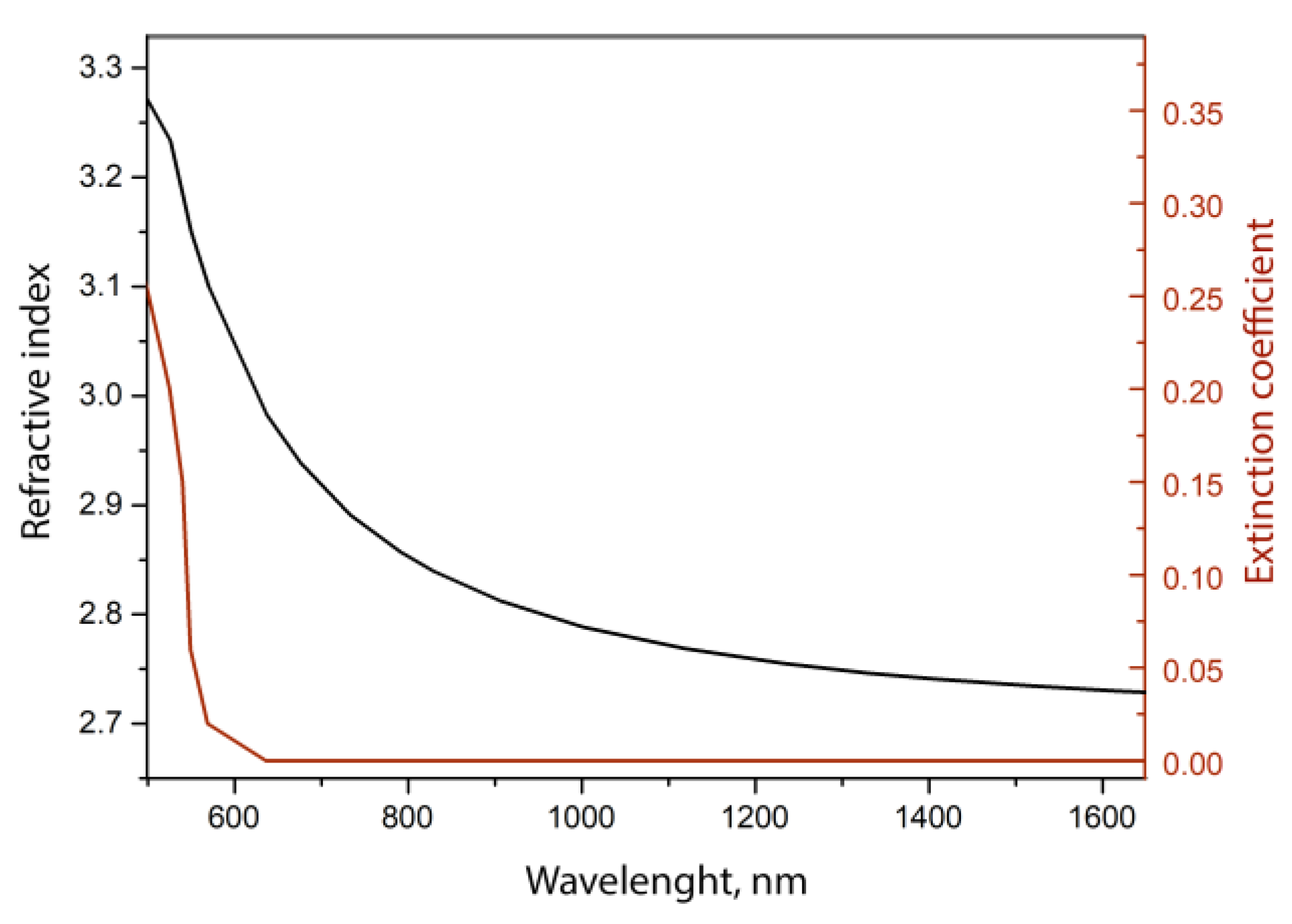
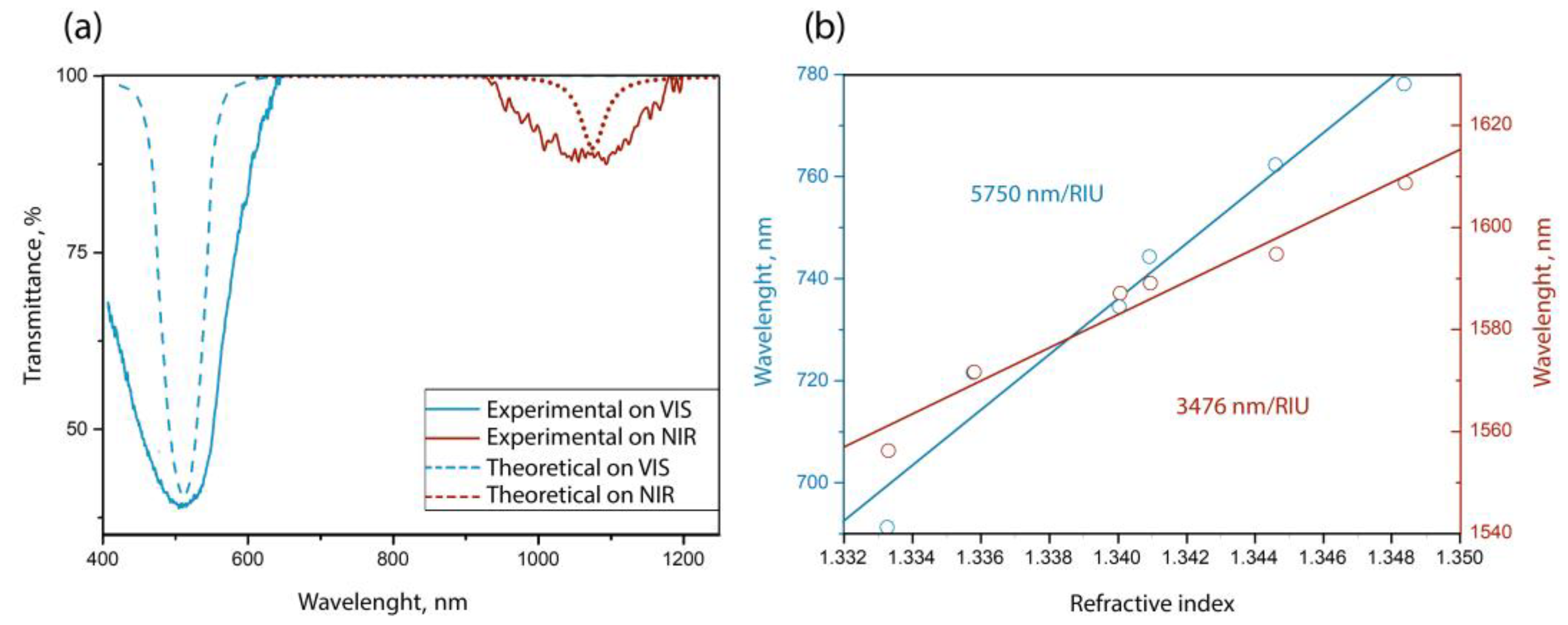
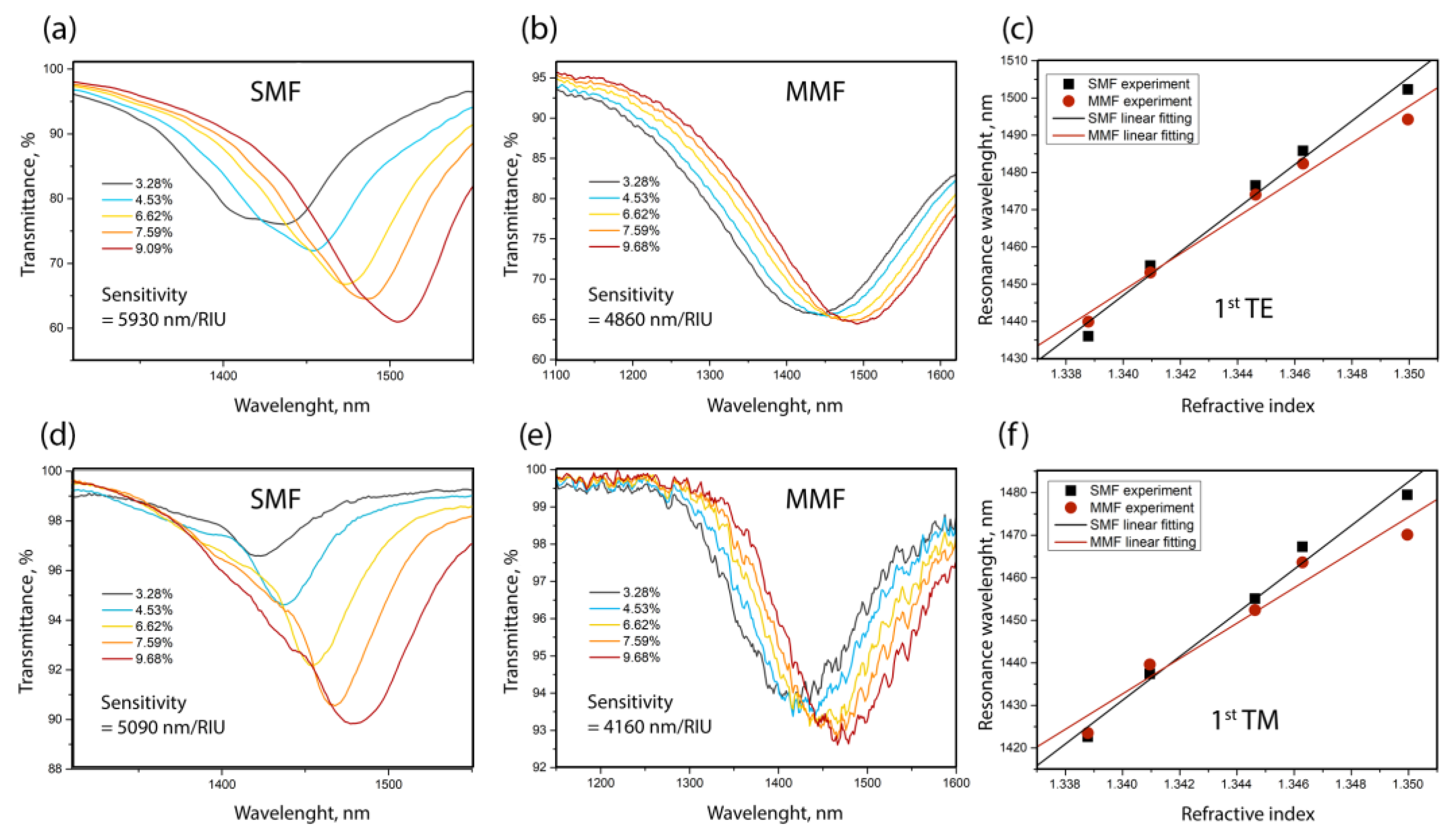

| Fiber Type | Etched Diameter (µm) | Etched Fiber | Coated Fiber | ||
|---|---|---|---|---|---|
| RMS (nm) | Roughness (nm) | RMS (nm) | Roughness (nm) | ||
| SMF | 26.3 | 10.514 | 7.651 | 19.597 | 11.557 |
| MMF | 99.5 | 3.410 | 2.521 | 4.936 | 3.035 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sudas, D.P.; Jitov, V.A.; Kuznetsov, P.I. Various Types of Light Guides for Use in Lossy Mode Resonance-Based Sensors. Sensors 2023, 23, 6049. https://doi.org/10.3390/s23136049
Sudas DP, Jitov VA, Kuznetsov PI. Various Types of Light Guides for Use in Lossy Mode Resonance-Based Sensors. Sensors. 2023; 23(13):6049. https://doi.org/10.3390/s23136049
Chicago/Turabian StyleSudas, Dmitriy P., Viktor A. Jitov, and Petr I. Kuznetsov. 2023. "Various Types of Light Guides for Use in Lossy Mode Resonance-Based Sensors" Sensors 23, no. 13: 6049. https://doi.org/10.3390/s23136049
APA StyleSudas, D. P., Jitov, V. A., & Kuznetsov, P. I. (2023). Various Types of Light Guides for Use in Lossy Mode Resonance-Based Sensors. Sensors, 23(13), 6049. https://doi.org/10.3390/s23136049




