Center of Pressure Behavior in Response to Unexpected Base of Support Shifting: A New Objective Tool for Dynamic Balance Assessment
Abstract
:1. Introduction
2. Methods
2.1. Subjects
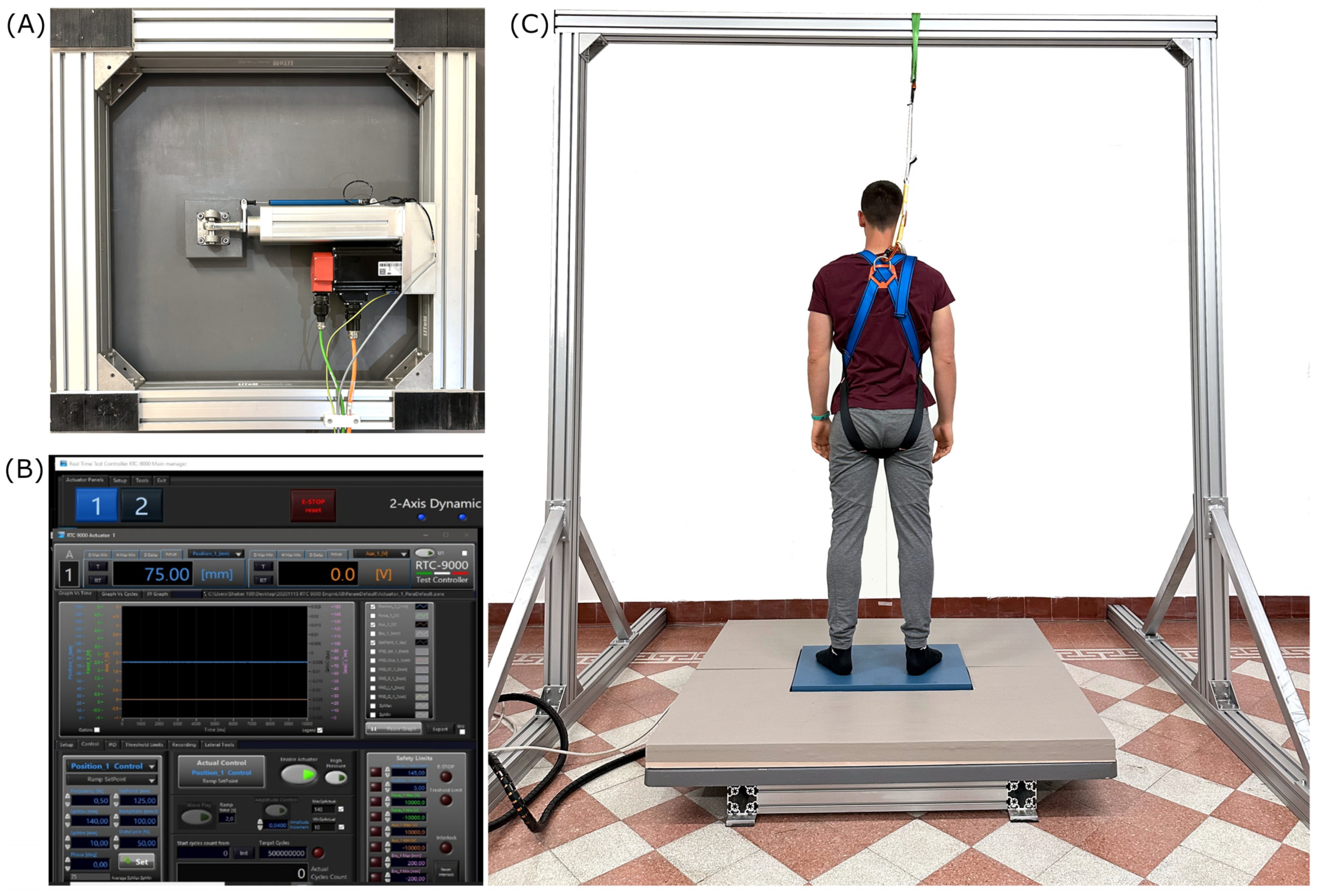
2.2. Experimental Design
2.3. Data Analysis
2.4. Statistical Analysis
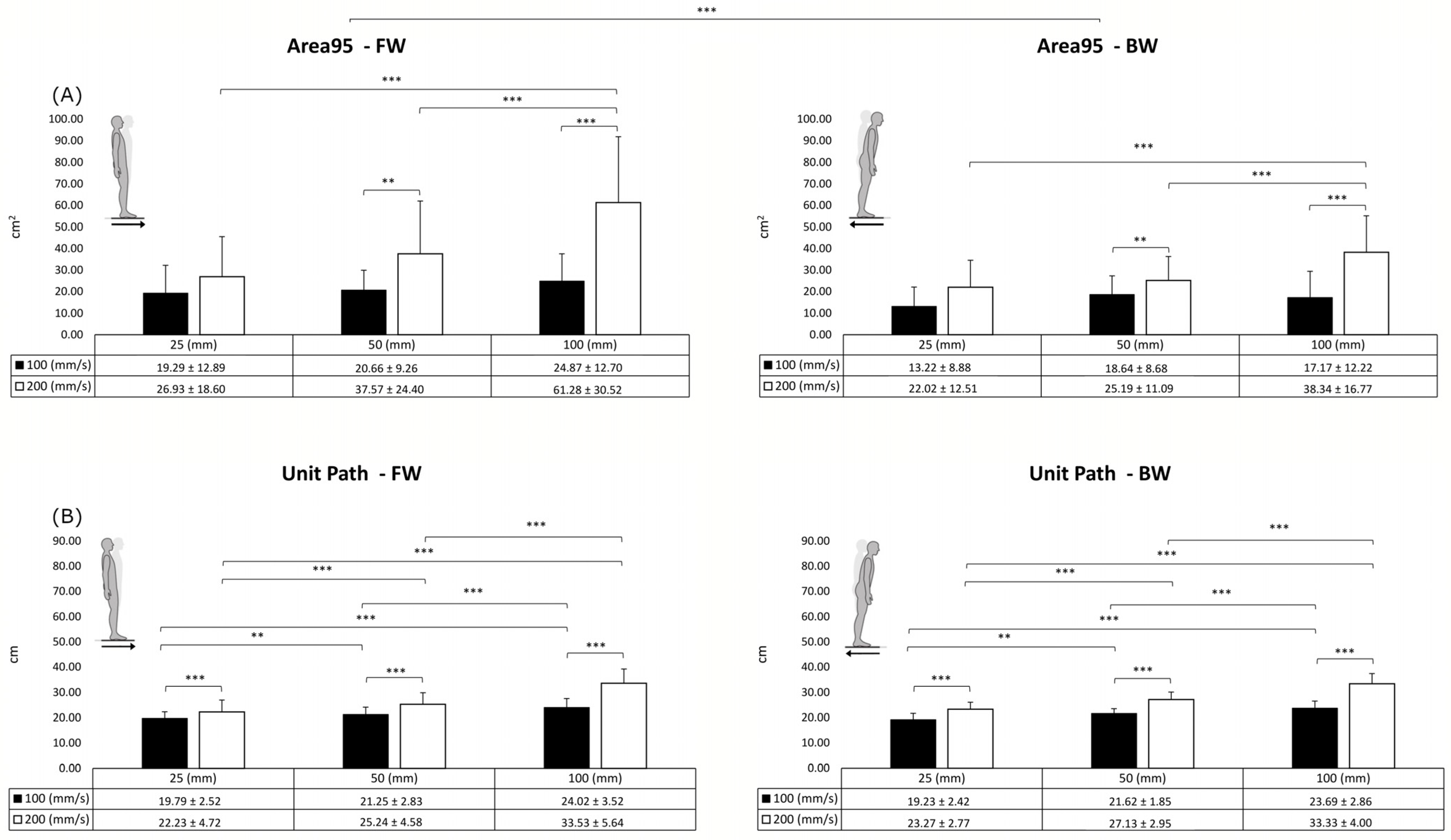
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Takakusaki, K.; Takahashi, M.; Obara, K.; Chiba, R. Neural Substrates Involved in the Control of Posture. Adv. Robot. 2017, 31, 2–23. [Google Scholar] [CrossRef]
- Karnath, H.O.; Ferber, S.; Dichgans, J. The Neural Representation of Postural Control in Humans. Proc. Natl. Acad. Sci. USA 2000, 97, 13931–13936. [Google Scholar] [CrossRef] [PubMed]
- Piirtola, M.; Era, P. Force Platform Measurements as Predictors of Falls among Older People—A Review. Gerontology 2006, 52, 1–16. [Google Scholar] [CrossRef] [PubMed]
- Pirauá, A.L.T.; Cavalcante, B.R.; de Oliveira, V.M.A.; Beltrão, N.B.; de Amorim Batista, G.; Pitangui, A.C.R.; Behm, D.; de Araújo, R.C. Effect of 24-Week Strength Training on Unstable Surfaces on Mobility, Balance, and Concern about Falling in Older Adults. Scand. J. Med. Sci. Sport. 2019, 29, 1805–1812. [Google Scholar] [CrossRef] [PubMed]
- Blake, A.J.; Morgan, K.; Bendall, M.J.; Dallosso, H.; Ebrahim, S.B.J.; Arie, T.H.D.; Fentem, P.H.; Bassey, E.J. Falls by Elderly People at Home: Prevalence and Associated Factors. Age Ageing 1988, 17, 365–372. [Google Scholar] [CrossRef]
- Rubenstein, L.Z. Falls in Older People: Epidemiology, Risk Factors and Strategies for Prevention. Age Ageing 2006, 35, ii37–ii41. [Google Scholar] [CrossRef] [Green Version]
- Zemková, E. Sport-Specific Balance. Sport. Med. 2014, 44, 579–590. [Google Scholar] [CrossRef]
- Paillard, T. Relationship between Sport Expertise and Postural Skills. Front. Psychol. 2019, 10, 1428. [Google Scholar] [CrossRef] [Green Version]
- Paillard, T. Plasticity of the Postural Function to Sport and/or Motor Experience. Neurosci. Biobehav. Rev. 2017, 72, 129–152. [Google Scholar] [CrossRef]
- Rizzato, A.; Paoli, A.; Andretta, M.; Vidorin, F.; Marcolin, G. Are Static and Dynamic Postural Balance Assessments Two Sides of the Same Coin? A Cross-Sectional Study in the Older Adults. Front. Physiol. 2021, 12, 681370. [Google Scholar] [CrossRef]
- Petró, B.; Papachatzopoulou, A.; Kiss, R.M. Devices and Tasks Involved in the Objective Assessment of Standing Dynamic Balancing—A Systematic Literature Review. PLoS ONE 2017, 12, e0185188. [Google Scholar] [CrossRef] [Green Version]
- Kapteyn, T.S.; Bles, W.; Njiokiktjien, C.J.; Kodde, L.; Massen, C.H.; Mol, J.M. Standardization in Platform Stabilometry Being a Part of Posturography. Agressol. Rev. Int. Physio-Biol. Pharmacol. Appl. Eff. Agression 1983, 24, 321–326. [Google Scholar] [CrossRef]
- Scoppa, F.; Capra, R.; Gallamini, M.; Shiffer, R. Clinical Stabilometry Standardization. Basic Definitions—Acquisition Interval—Sampling Frequency. Gait Posture 2013, 37, 290–292. [Google Scholar] [CrossRef] [PubMed]
- Paillard, T.; Noé, F. Techniques and Methods for Testing the Postural Function in Healthy and Pathological Subjects. Biomed. Res. Int. 2015, 2015. [Google Scholar] [CrossRef] [Green Version]
- Yelnik, A.; Bonan, I. Clinical Tools for Assessing Balance Disorders. Neurophysiol. Clin. 2008, 38, 439–445. [Google Scholar] [CrossRef]
- Leirós-Rodríguez, R.; Romo-Pérez, V.; García-Soidán, J.L.; Soto-Rodríguez, A. Identification of Body Balance Deterioration of Gait in Women Using Accelerometers. Sustainability 2020, 12, 1222. [Google Scholar] [CrossRef] [Green Version]
- Leirós-Rodríguez, R.; Romo-Pérez, V.; García-Soidán, J.L. Validity and Reliability of a Tool for Accelerometric Assessment of Static Balance in Women. Eur. J. Physiother. 2017, 19, 243–248. [Google Scholar] [CrossRef]
- Horak, F.B.; Henry, S.M.; Shumway-Cook, A. Postural Perturbations: New Insights for Treatment of Balance Disorders. Phys. Ther. 1997, 77, 517–533. [Google Scholar] [CrossRef]
- Rinaldin, C.D.P.; Ávila de Oliveira, J.; Coelho, D.B.; Scheeren, E.M.; Teixeira, L.A. Instantaneous Interjoint Rescaling and Adaptation to Balance Perturbation under Muscular Fatigue. Eur. J. Neurosci. 2020, 51, 1478–1490. [Google Scholar] [CrossRef]
- Jacob, D.; Unnsteinsdóttir Kristensen, I.S.; Aubonnet, R.; Recenti, M.; Donisi, L.; Ricciardi, C.; Svansson, H.Á.R.; Agnarsdóttir, S.; Colacino, A.; Jónsdóttir, M.K.; et al. Towards Defining Biomarkers to Evaluate Concussions Using Virtual Reality and a Moving Platform (BioVRSea). Sci. Rep. 2022, 12, 8996. [Google Scholar] [CrossRef]
- Mileti, I.; Taborri, J.; Rodriguez-Cianca, D.; Torricelli, D.; Rossi, S.; Patanè, F. Feasibility and Application of the B.E.A.T. Testbed for Assessing the Effects of Lower Limb Exoskeletons on Human Balance. Robotics 2022, 11, 151. [Google Scholar] [CrossRef]
- Runge, C.F.; Shupert, C.L.; Horak, F.B.; Zajac, F.E. Ankle and Hip Postural Strategies Defined by Joint Torques. Gait Posture 1999, 10, 161–170. [Google Scholar] [CrossRef] [PubMed]
- Ghulyan, V.; Paolino, M.; Lopez, C.; Dumitrescu, M.; Lacour, M. A New Translational Platform for Evaluating Aging or Pathology-Related Postural Disorders. Acta Otolaryngol. 2005, 125, 607–617. [Google Scholar] [CrossRef]
- Zemková, E.; Kováčiková, Z.; Jeleň, M.; Neumannová, K.; Janura, M. Postural and Trunk Responses to Unexpected Perturbations Depend on the Velocity and Direction of Platform Motion. Physiol. Res. 2016, 65, 769–776. [Google Scholar] [CrossRef]
- Horak, F.B.; Diener, H.C.; Nashner, L.M. Influence of Central Set on Human Postural Responses. J. Neurophysiol. 1989, 62, 841–853. [Google Scholar] [CrossRef] [PubMed]
- Brown, L.A.; Jensen, J.L.; Korff, T.; Woollacott, M.H. The Translating Platform Paradigm: Perturbation Displacement Waveform Alters the Postural Response. Gait Posture 2001, 14, 256–263. [Google Scholar] [CrossRef]
- Jacobs, J.V.; Horak, F.B. Cortical Control of Postural Responses. J. Neural Transm. 2007, 114, 1339–1348. [Google Scholar] [CrossRef] [Green Version]
- Nashner, L.M.; Cordo, P.J. Relation of Automatic Postural Responses and Reaction-Time Voluntary Movements of Human Leg Muscles. Exp. Brain Res. 1981, 43, 395–405. [Google Scholar] [CrossRef]
- Morasso, P.; Cherif, A.; Zenzeriid, J. Quiet Standing: The Single Inverted Pendulum Model Is Not so Bad after All. PLoS ONE 2019, 14, e0213870. [Google Scholar] [CrossRef] [Green Version]
- Nardone, A.; Godi, M.; Artuso, A.; Schieppati, M. Balance Rehabilitation by Moving Platform and Exercises in Patients with Neuropathy or Vestibular Deficit. Arch. Phys. Med. Rehabil. 2010, 91, 1869–1877. [Google Scholar] [CrossRef]
- Maki, B.E.; McIlroy, W.E. Postural Control in the Older Adult. Clin. Geriatr. Med. 1996, 12, 635–658. [Google Scholar] [CrossRef] [PubMed]
- Lord, S.R.; Close, J.C.T. New Horizons in Falls Prevention. Age Ageing 2018, 47, 492–498. [Google Scholar] [CrossRef] [PubMed]


| Area95 | Unit Path | |||
|---|---|---|---|---|
| Cohen’s d | 95% CI | Cohen’s d | 95% CI | |
| Direction | ||||
| FW vs. BW | −0.63 | [−16.19, −2.48] | −0.16 | [−0.66, 1.40] |
| Ramp rate (mm/s) | ||||
| 100 vs. 200 | −2.37 | [−19.45, −13.03] | −3.01 | [−6.76, −4.94] |
| Displacement (mm) | ||||
| 25 vs. 50 | −0.47 | [−11.17, 0.87] | −1.25 | [−3.87, −1.48] |
| 25 vs. 100 | −1.39 | [−21.07, −9.02] | −3.52 | [−8.70, −6.32] |
| 50 vs. 100 | −0.92 | [−15.92, −3.87] | −2.27 | [−6.02, −3.64] |
| FP | ΔCoPMax | PPV | ||||
|---|---|---|---|---|---|---|
| Cohen’s d | 95% CI | Cohen’s d | 95% CI | Cohen’s d | 95% CI | |
| Direction | ||||||
| FW vs. BW | 1.54 | [1.22, 2.29] | 0.82 | [0.66, 2.39] | 0.39 | [−0.03, 0.41] |
| Ramp rate (mm/s) | ||||||
| 100 vs. 200 | −2.98 | [−2.37, −1.73] | −1.27 | [−2.65, −1.22] | −1.14 | [−0.62, −0.25] |
| Displacement (mm) | ||||||
| 25 vs. 50 | −0.93 | [−1.61, −0.40] | −0.68 | [−1.90, −0.19] | −1.12 | [−0.61, −0.20] |
| 25 vs. 100 | −1.19 | [−1.90, −0.69] | −0.57 | [−1.72, −0.01] | −1.99 | [−0.93, −0.52] |
| 50 vs. 100 | −0.26 | [−0.89, 0.31] | 0.11 | [−0.68, 1.30] | −0.86 | [−052, −0.11] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rizzato, A.; Bozzato, M.; Zullo, G.; Paoli, A.; Marcolin, G. Center of Pressure Behavior in Response to Unexpected Base of Support Shifting: A New Objective Tool for Dynamic Balance Assessment. Sensors 2023, 23, 6203. https://doi.org/10.3390/s23136203
Rizzato A, Bozzato M, Zullo G, Paoli A, Marcolin G. Center of Pressure Behavior in Response to Unexpected Base of Support Shifting: A New Objective Tool for Dynamic Balance Assessment. Sensors. 2023; 23(13):6203. https://doi.org/10.3390/s23136203
Chicago/Turabian StyleRizzato, Alex, Matteo Bozzato, Giuseppe Zullo, Antonio Paoli, and Giuseppe Marcolin. 2023. "Center of Pressure Behavior in Response to Unexpected Base of Support Shifting: A New Objective Tool for Dynamic Balance Assessment" Sensors 23, no. 13: 6203. https://doi.org/10.3390/s23136203
APA StyleRizzato, A., Bozzato, M., Zullo, G., Paoli, A., & Marcolin, G. (2023). Center of Pressure Behavior in Response to Unexpected Base of Support Shifting: A New Objective Tool for Dynamic Balance Assessment. Sensors, 23(13), 6203. https://doi.org/10.3390/s23136203








