Transmission Lines in Capacitance Measurement Systems: An Investigation of Receiver Structures
Abstract
:1. Introduction
- A low-input impedance circuit based on a current to voltage converter [20]. This configuration is optimal for a direct connection of the sensor and circuitry, as it shunts parasitic capacitances. We refer to this as a low-Z receiver in this work.
- In [30], a resonant measurement circuit was proposed. This approach provides low input impedance and additional amplification due to a resonance. We refer to this as LCR receiver.
- An impedance-matched front-end design is proposed in [31], in which the input impedance of the receiver is matched to the wave impedance of the line. We refer to this as matched receiver.
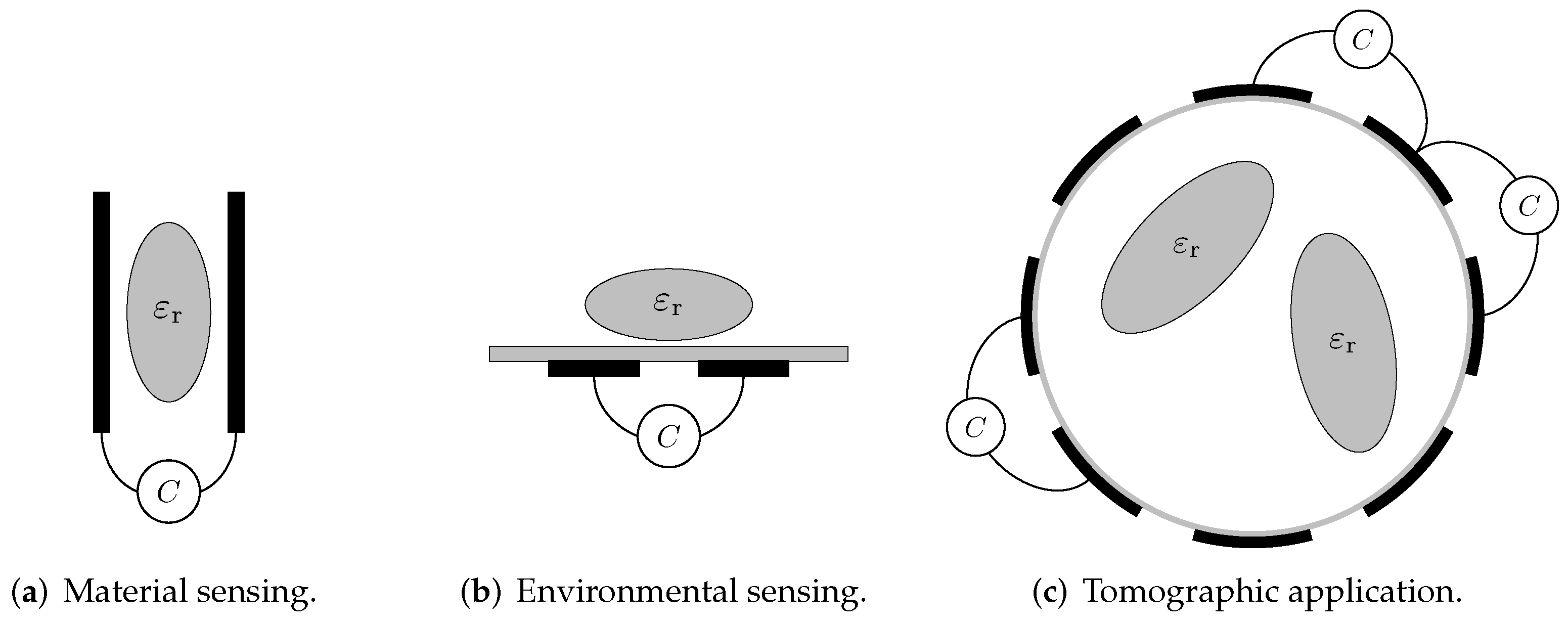
- Holistic system analysis by considering the interaction between the sensor and electronics.
- Investigation of a directly attached sensor and electronics assembly as well as a spatial dislocated arrangement by means of the use of transmission lines.
- Simulation-based assessments of the achievable signal-to-noise ratio (SNR) and quantitative comparison of the topologies, considering the environmental impacts.
- Suggestion of established receiver structures and investigation of their applicability for spectroscopic applications.
2. Overview of Possible Receiver Structures
2.1. Low-Z and Matched Receiver
2.2. LCR Receiver
2.3. Outline of the Further Analysis
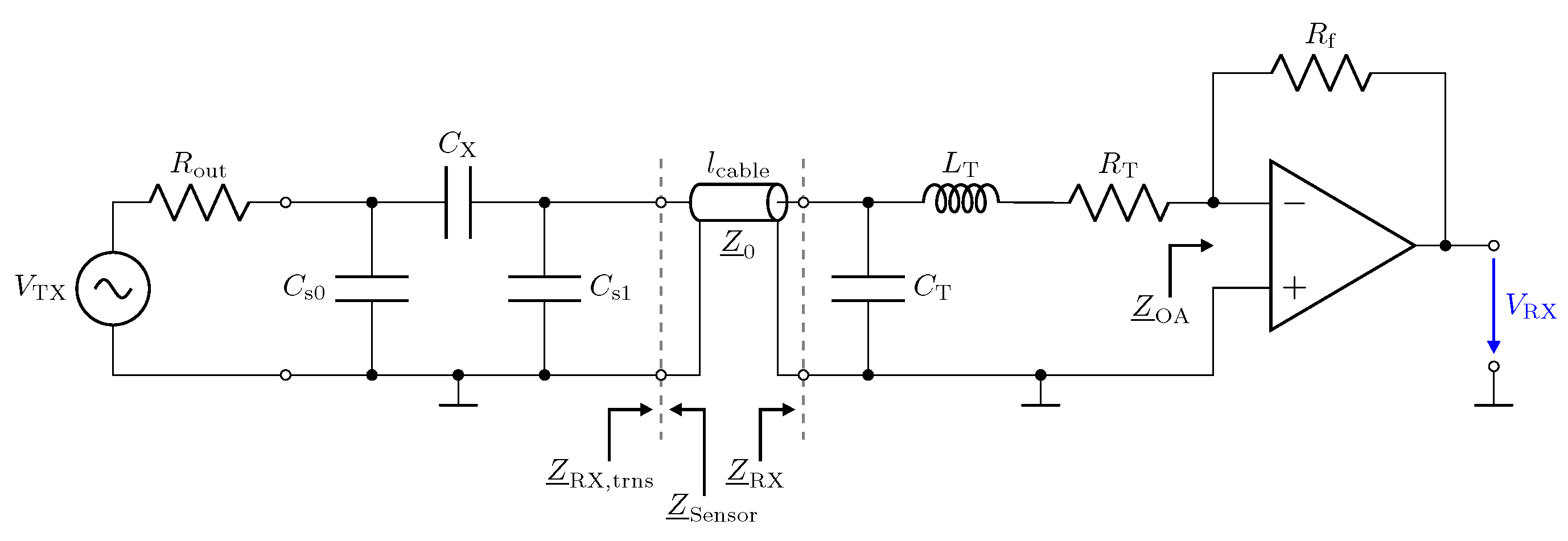
3. Low-Z Receiver
3.1. Low-Z Receiver: Behaviour of and
3.2. Low-Z Receiver: Output Behaviour
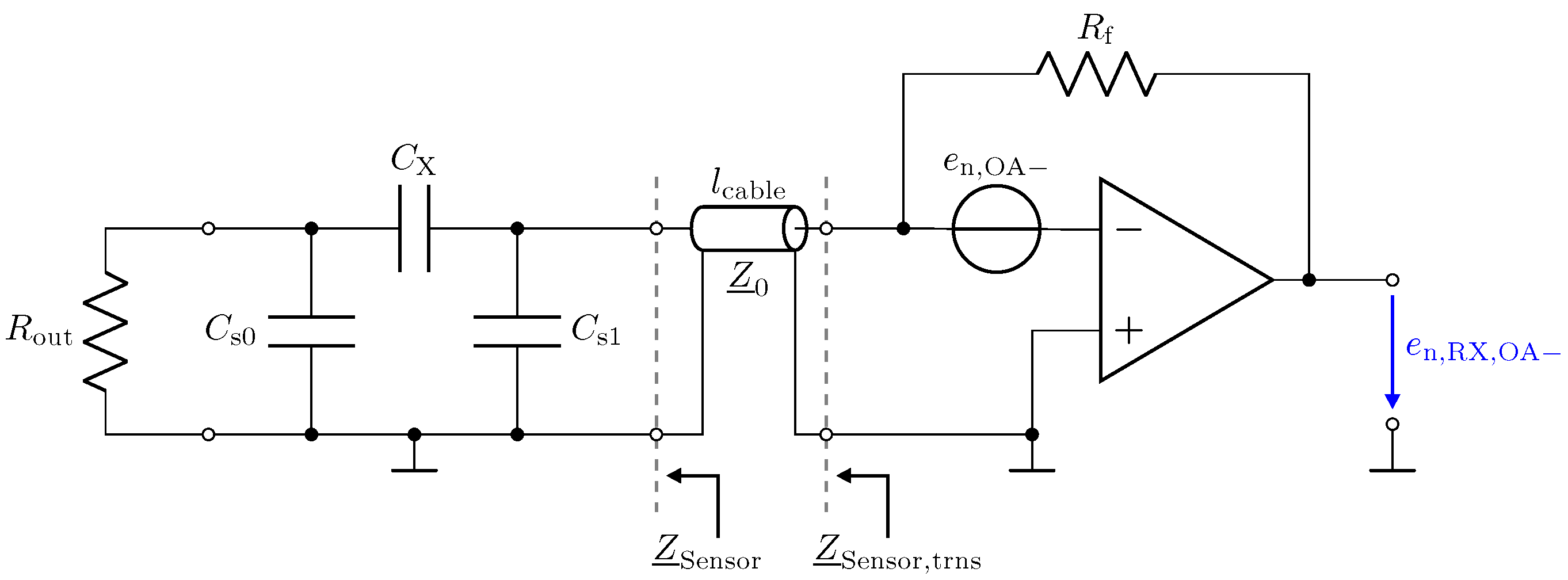
3.3. Low-Z Receiver: Noise Characteristics
4. Matched Receiver
4.1. Matched Receiver: Behaviour of and Output Behaviour
4.2. Matched Receiver: Noise Characteristics
5. LCR Receiver
5.1. Design of a Matched LCR Receiver Input Stage
5.2. LCR Receiver: Behaviour of
5.3. LCR Receiver: Output Behaviour
5.4. Resonant LCR Receiver: Noise Characteristics
6. Receiver Structure Assessment
6.1. Receiver Operation Comparison
6.1.1. Low-Z Receiver
- Simple realization feasibility of the circuits possible with moderate effort.
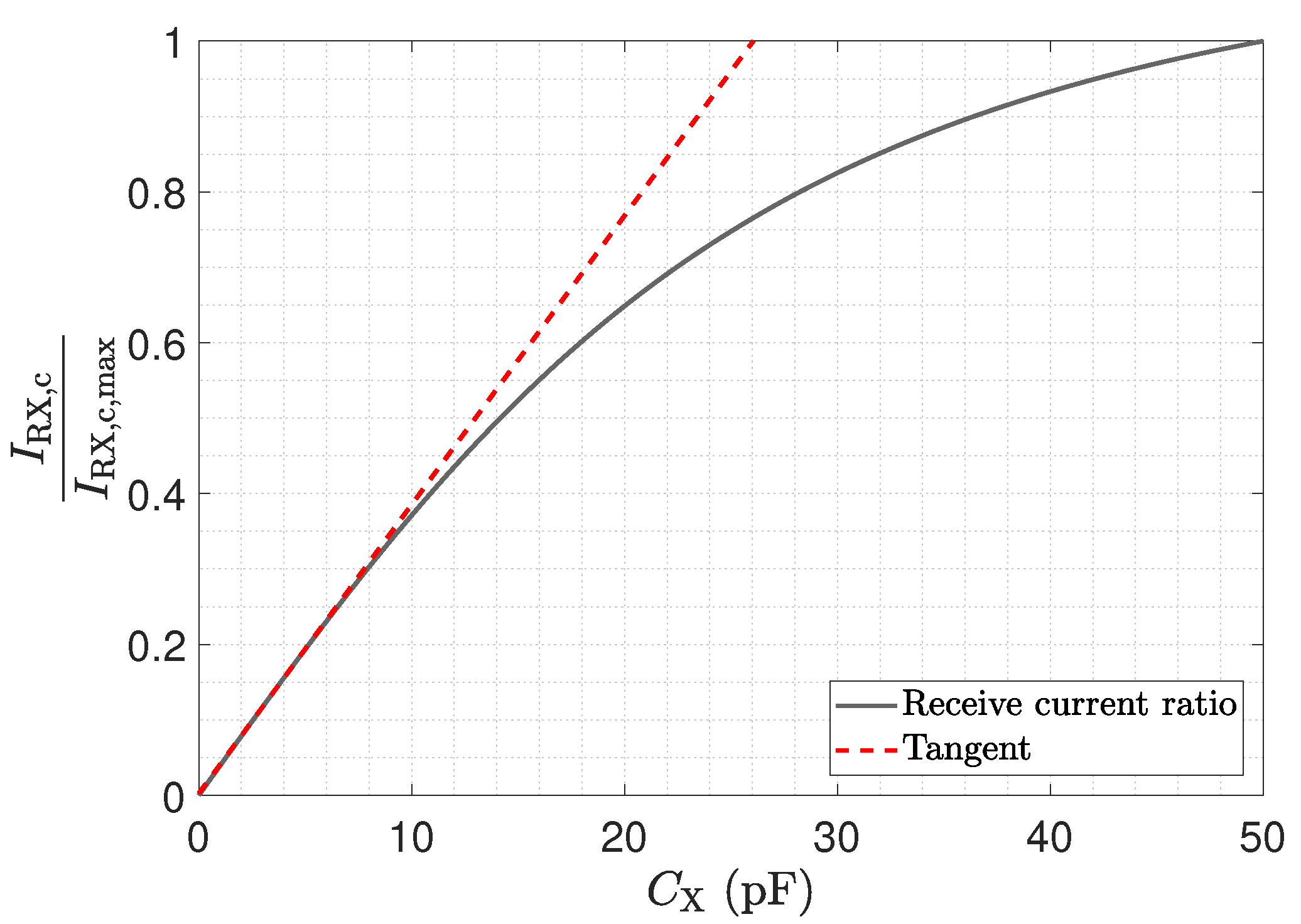
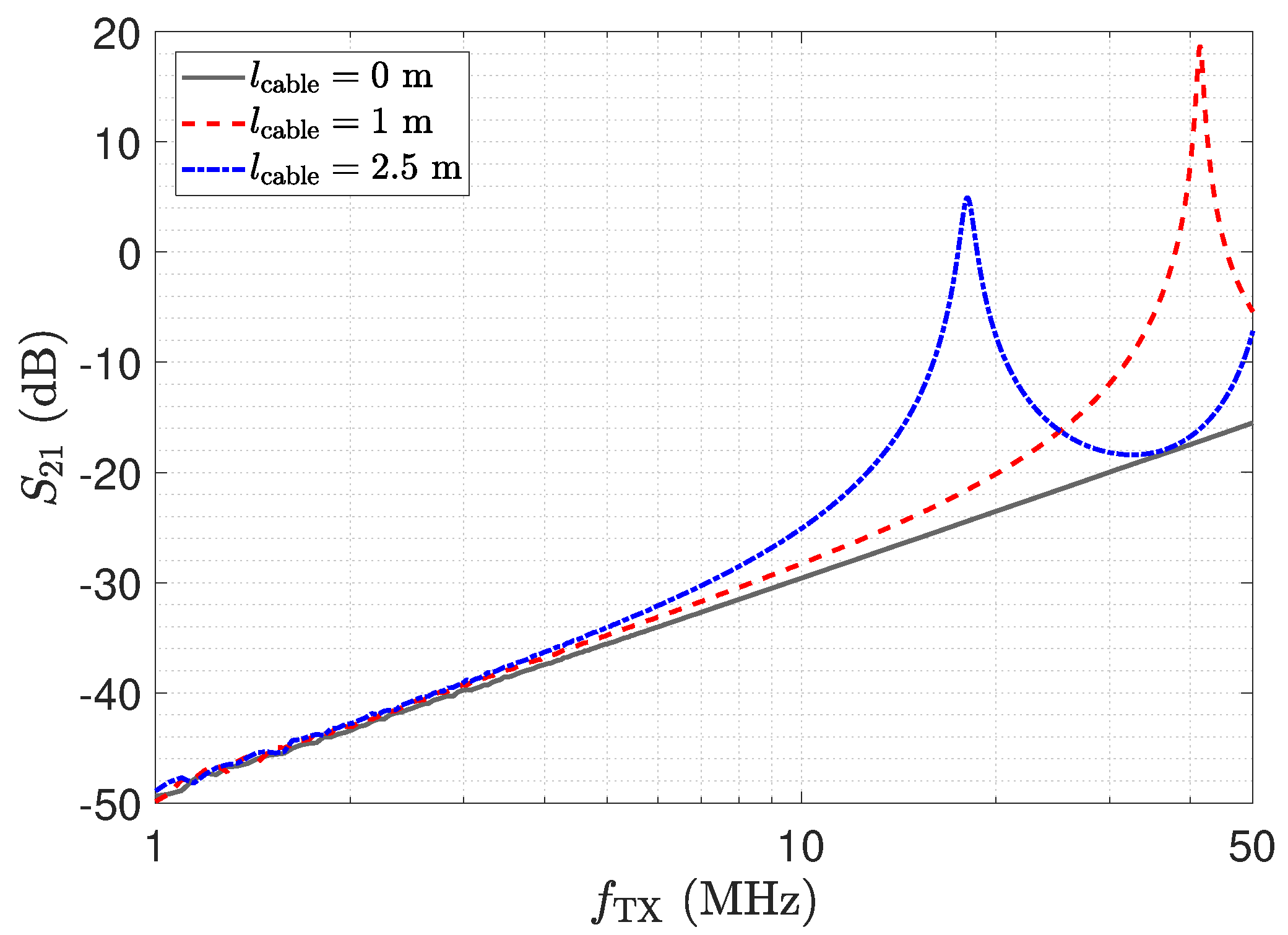
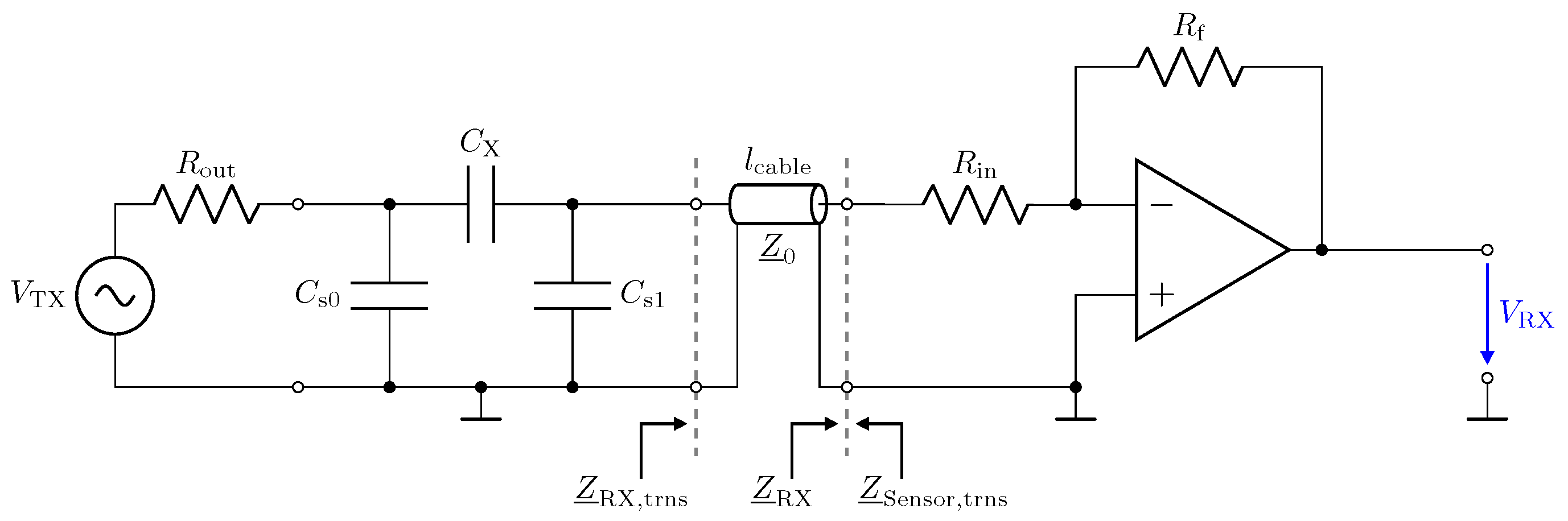
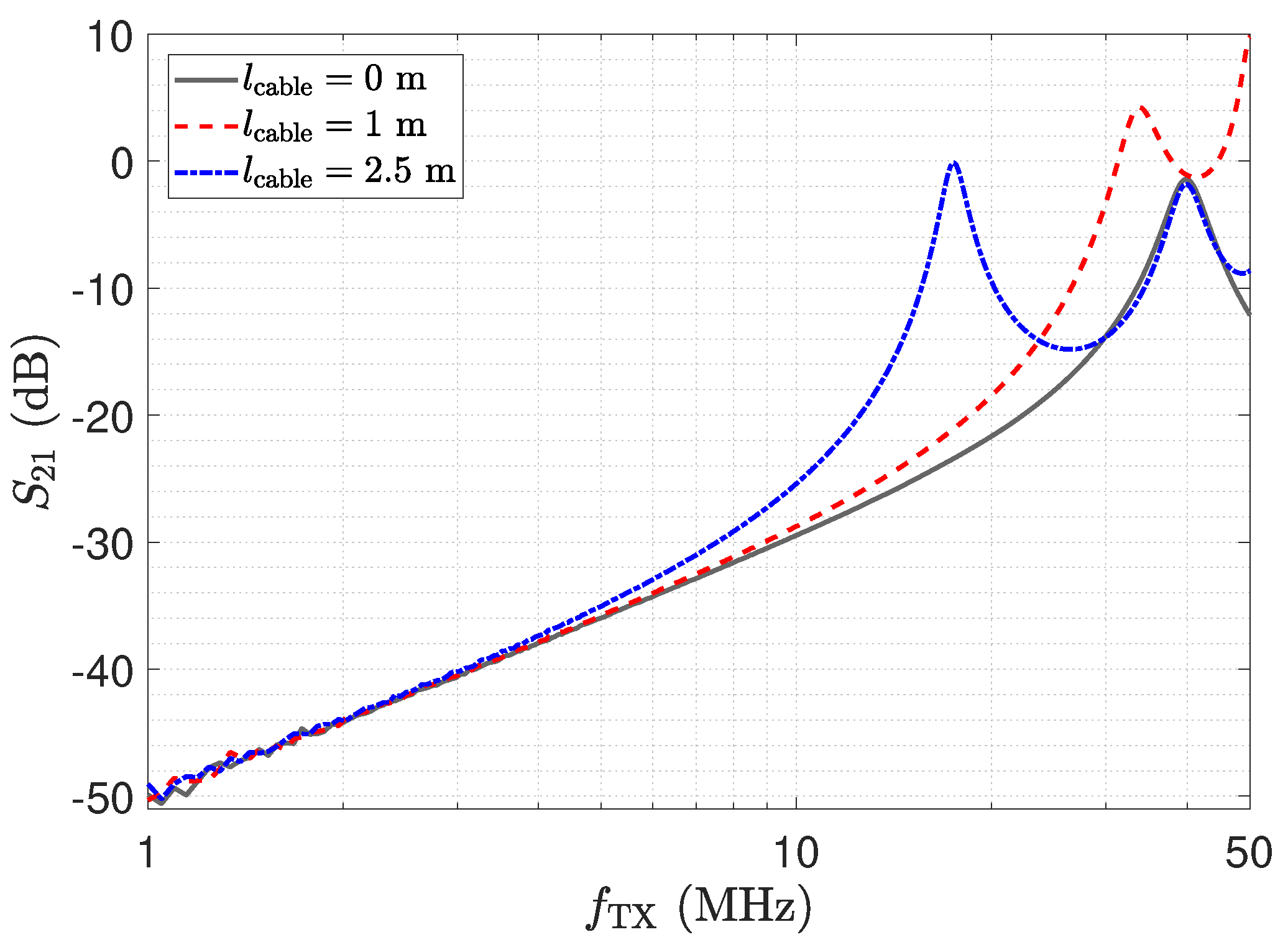
- Even small transmission line lengths show a transformation of to higher input impedances. This also increases the cross-sensitivity with respect to parasitic capacitances.
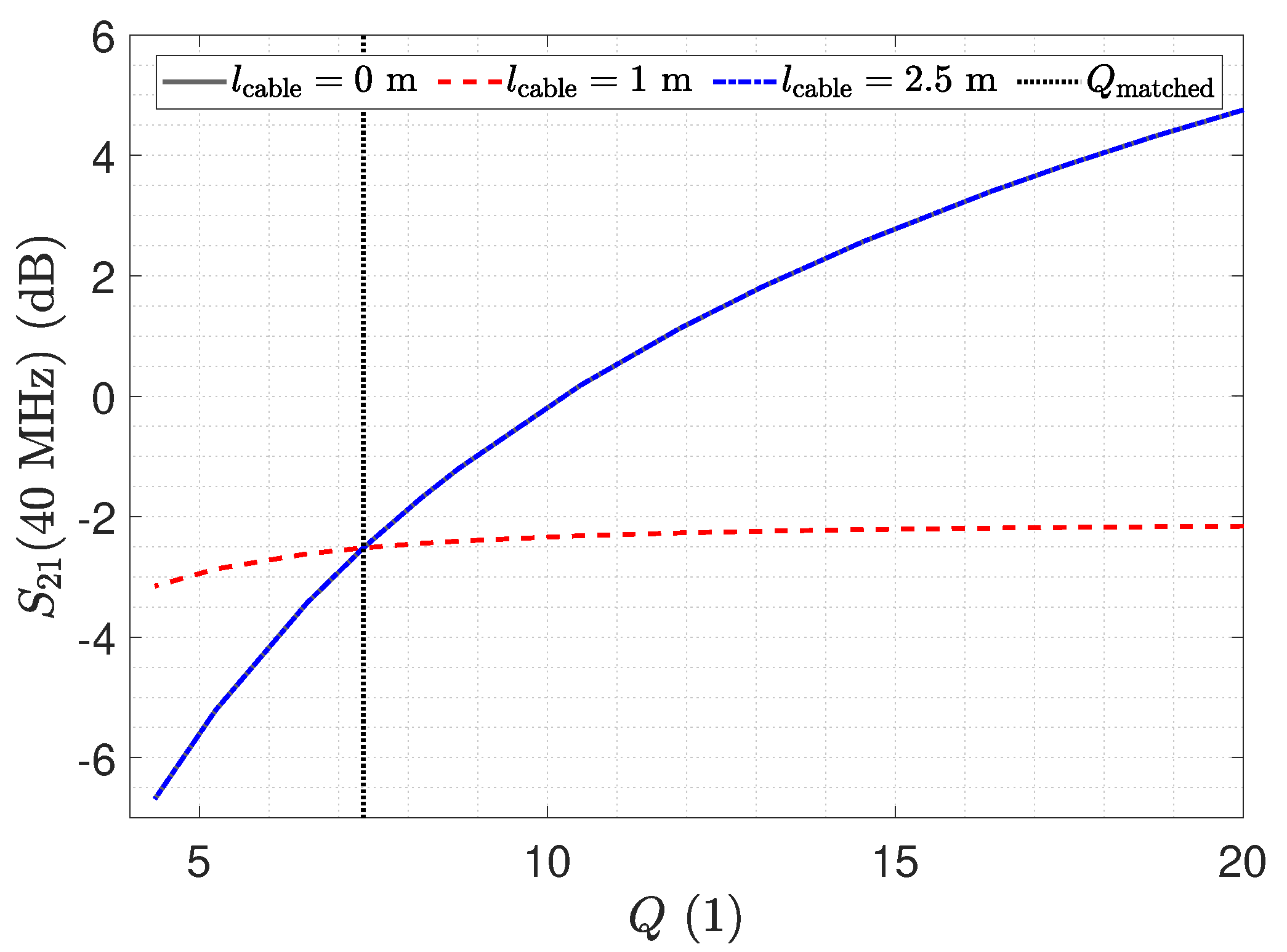
- Selection of frequency and transmission line length: the frequency and the transmission line length have to be matched to obtain a transformer. In this case, the circuit behaves as for a direct connection except for the attenuation of the line. The attenuation can be calibrated.
- A setup has to be avoided. Although it leads to high output signals, it creates undefined signal propagation and harmful operating conditions for the opamp
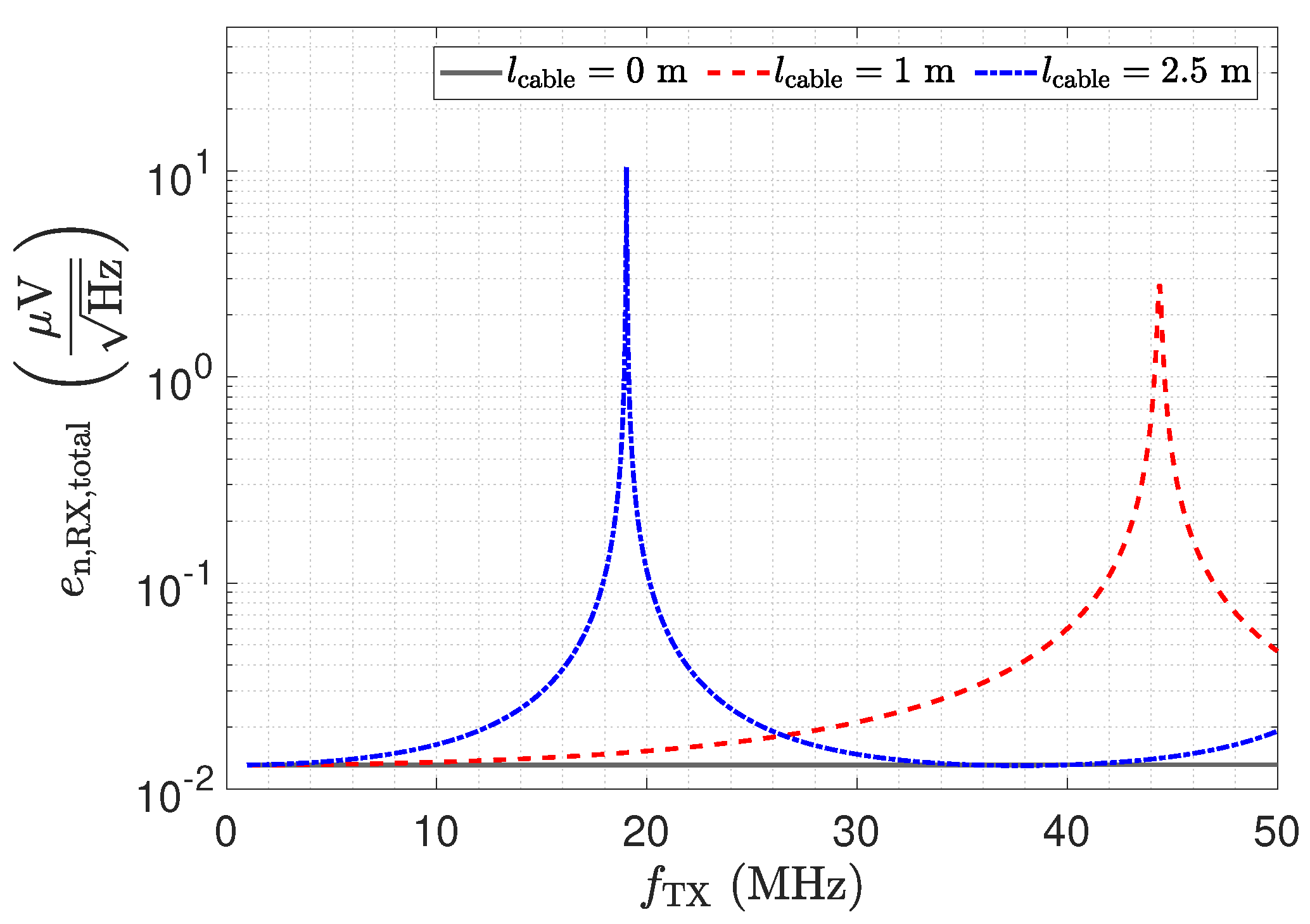
- Noise: Same noise floor as with direct connection for transformer. Significant elevation of noise floor towards the setup.
6.1.2. Matched Receiver
- Simple realization feasibility of the circuits possible with moderate effort. Variations of in the transmission line remain a minor source of deviations in the circuit behaviour.
- Selection of frequency and transmission line length. Due to the matched setup, the frequency can be selected independently from the transmission line length. The receiver provides a constant input impedance.
- Noise: the receiver structure shows an almost constant noise floor over the whole frequency range.
6.1.3. LCR-Receiver
- Realization requires tuning of the input stage. The tuning is sensitive towards parasitic effects of the assembly and the opamp. Furthermore, a relevant sensitivity with respect to temperature changes was observed.
- Selection of frequency and transmission line length: the excitation frequency is a design parameter. If the input stage is tuned to , the line length can be arbitrary. Otherwise, again, a transformer setup should be used.
- Noise: the noise floor is also shaped by the LCR input stage. In a direct connection setup or a setup, the noise floor increases towards the selected resonance frequency. Different line lengths can lead to a spectral shift of the rise in the noise floor.
6.1.4. Summary of Relevant Signal-Propagation and Noise Effects
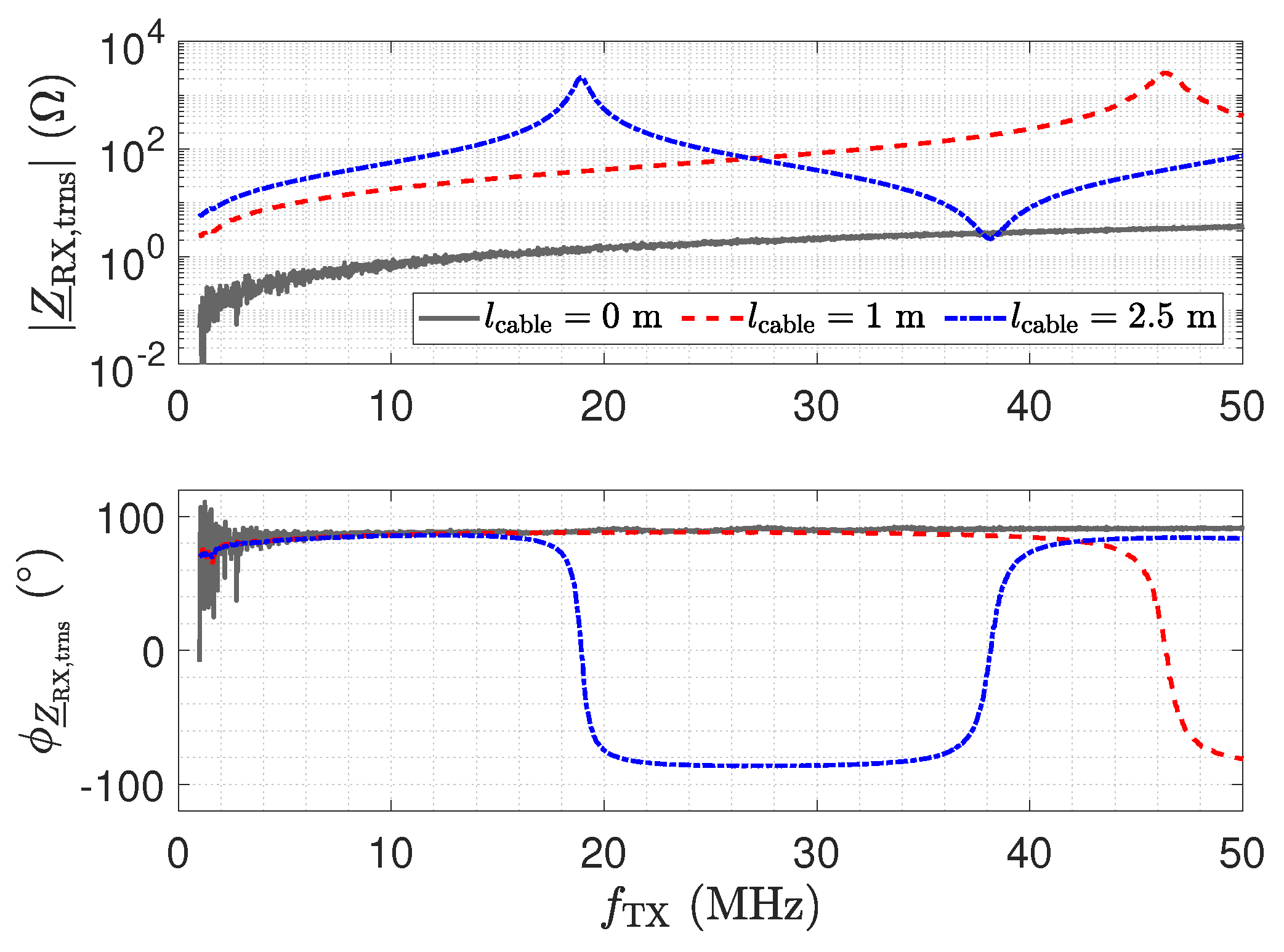
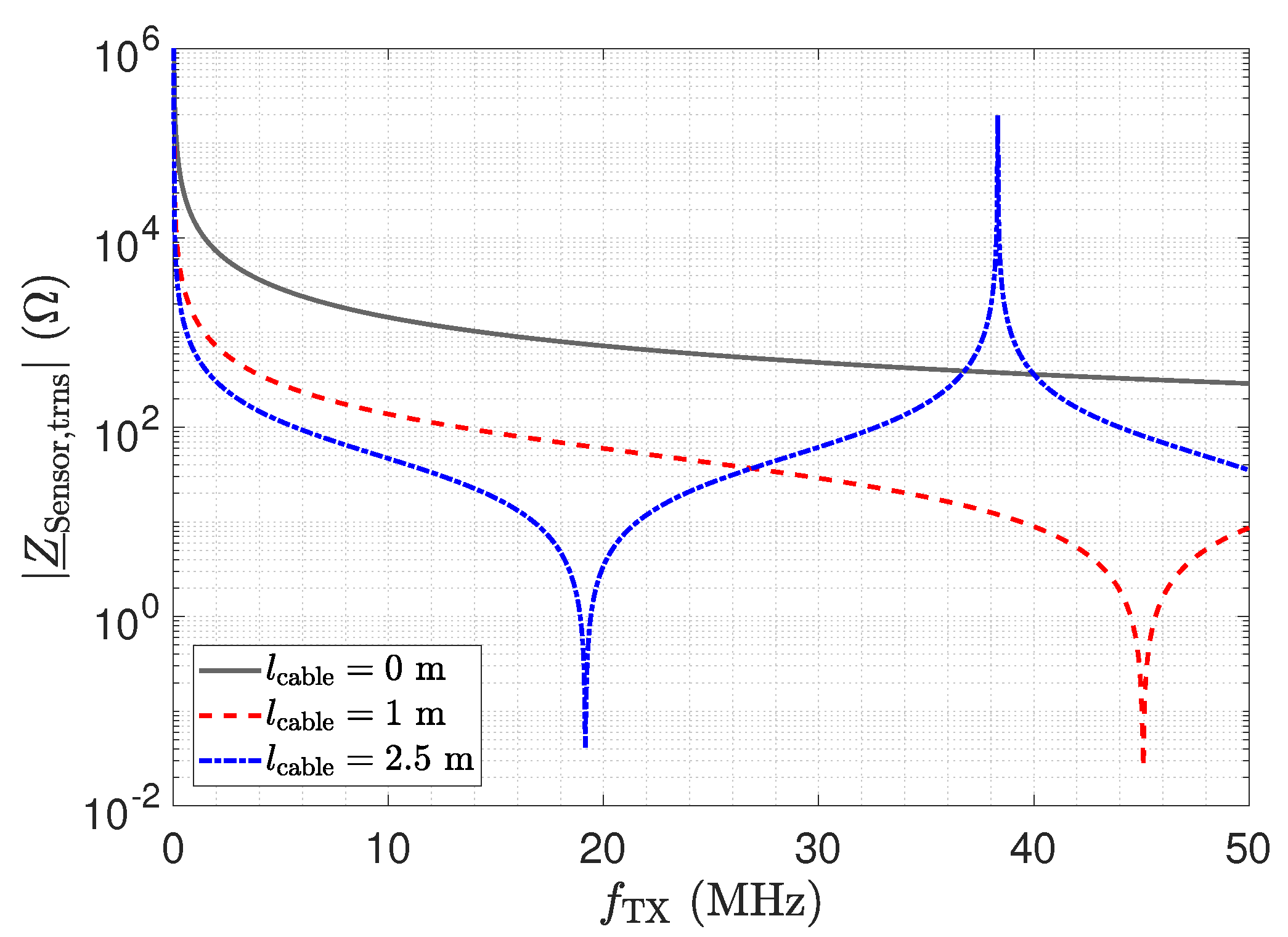
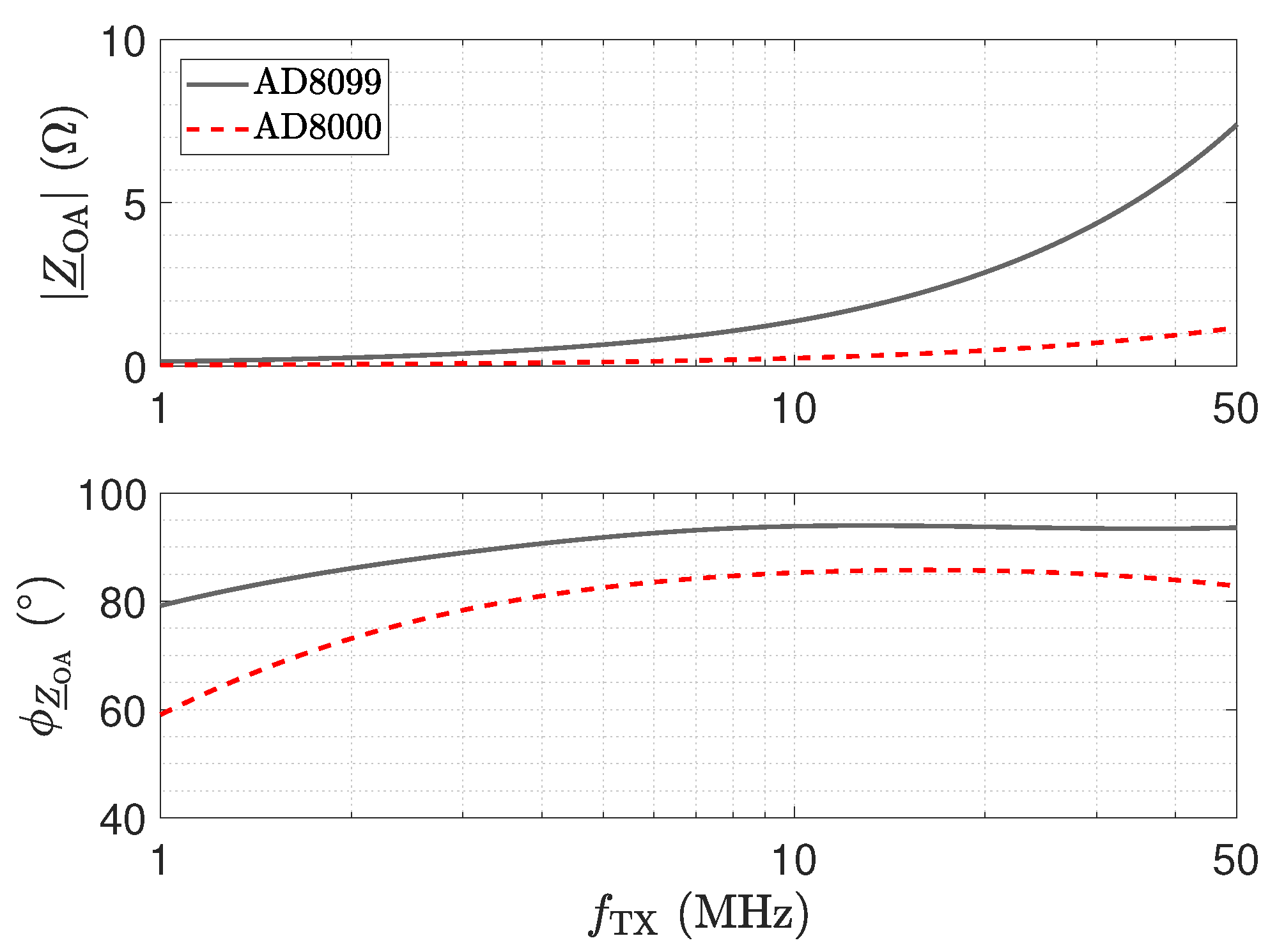
- The impedance depicted in Figure 11 equals the input impedance of the low-Z amplifier for a direct connection. Figure 13: The input–output behaviour of the circuit with the transmission line equals a direct connection. Figure 12: The impedance with the transmission line equals the impedance for a direct connection.
- Figure 13: The peak in the transmission behaviour is due to an impedance transformation. This behaviour is not suitable for measurements.
6.2. SNR Comparison
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| BPF | Band-pass filter |
| CF | Carrier frequency |
| ECT | Electrical capacitance tomography |
| GND | System ground |
| High-Z | High-impedance |
| IF | Intermediate frequency |
| LCR | Inductor-capacitor-resistor |
| low-Z | Low-impedance |
| PCB | Printed circuit board |
| RX | Receiver |
| RMS | Root mean square |
| SMD | Surface mounted devices |
| SNR | Signal-to-noise-ratio |
| TIA | Transimpedance-amplifier |
| TOSM | Through-open-short-match |
| TX | Transmitter |
References
- Kremer, F.; Schönhals, A. Broadband Dielectric Measurement Techniques (106 Hz to 1012 Hz). In Broadband Dielectric Spectroscopy, 1st ed.; Kremer, F., Schönhals, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2003; Chapter 2; pp. 35–57. [Google Scholar] [CrossRef]
- Nelson, S.O. Agricultural applications of dielectric measurements. IEEE Trans. Dielectr. Electr. Insul. 2006, 13, 688–702. [Google Scholar] [CrossRef]
- El Khaled, D.; Castellano, N.N.; Gázquez, J.A.; Perea-Moreno, A.J.; Manzano-Agugliaro, F. Dielectric Spectroscopy in Biomaterials: Agrophysics. Materials 2016, 9, 310. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nelson, S.O. Dielectric Spectroscopy of Fresh Fruits and Vegetables. In Proceedings of the IEEE Instrumentationand Measurement Technology Conference Proceedings, Ottawa, ON, Canada, 17–19 May 2005; Volume 1, pp. 360–364. [Google Scholar] [CrossRef]
- Liu, Y.; Li, D.; Qian, J.; Di, B.; Zhang, G.; Ren, Z. Electrical impedance spectroscopy (EIS) in plant roots research: A review. Plant Methods 2021, 17, 1–25. [Google Scholar] [CrossRef]
- Serrano-Finetti, E.; Mata, N.; Cerrillo, M. Sedimentation monitoring of the active biomass in bioreactors by electrical impedance spectroscopy. In Proceedings of the IEEE International Instrumentation and Measurement Technology Conference (I2MTC), Dubrovnik, Croatia, 25–28 May 2020; pp. 1–5. [Google Scholar] [CrossRef]
- Tiitta, M.; Tiitta, V.; Heikkinen, J.; Lappalainen, R.; Tomppo, L. Classification of Wood Chips Using Electrical Impedance Spectroscopy and Machine Learning. Sensors 2020, 20, 1076. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bretterklieber, T.; Neumayer, M.; Flatscher, M.; Becke, A.; Brasseur, G. Model based monitoring of ice accretion on overhead power lines. In Proceedings of the 2016 IEEE International Instrumentation and Measurement Technology Conference Proceedings, Taipei, Taiwan, 23–26 May 2016; pp. 1–6. [Google Scholar] [CrossRef]
- Flatscher, M.; Neumayer, M.; Bretterklieber, T. Maintaining critical infrastructure under cold climate conditions: A versatile sensing and heating concept. Sens. Actuators Phys. 2017, 267, 538–546. [Google Scholar] [CrossRef]
- Flatscher, M.; Neumayer, M.; Bretterklieber, T.; Moser, M.J.; Zangl, H. De-icing system with integrated ice detection and temperature sensing for meteorological devices. In Proceedings of the 2015 IEEE Sensors Applications Symposium (SAS), Zadar, Croatia, 13–15 April 2015; pp. 1–6. [Google Scholar] [CrossRef]
- Flatscher, M.; Neumayer, M.; Bretterklieber, T. Field sensor analysis for electrical impedance spectroscopy based ice detection. In Proceedings of the IEEE SENSORS, Glassboro, NJ, USA, 13–15 March 2017; pp. 477–479. [Google Scholar] [CrossRef]
- Jiang, Y.; Soleimani, M. Capacitively Coupled Resistivity Imaging for Biomaterial and Biomedical Applications. IEEE Access 2018, 6, 27069–27079. [Google Scholar] [CrossRef]
- Li, Y.; Soleimani, M. Imaging conductive materials with high frequency electrical capacitance tomography. Measurement 2013, 46, 3355–3361. [Google Scholar] [CrossRef]
- Jiang, Y.D.; Soleimani, M. Capacitively Coupled Electrical Impedance Tomography for Brain Imaging. IEEE Trans. Med. Imaging 2019, 38, 2104–2113. [Google Scholar] [CrossRef]
- Darnajou, M.; Dupré, A.; Dang, C.; Ricciardi, G.; Bourennane, S.; Bellis, C. On the Implementation of Simultaneous Multi-Frequency Excitations and Measurements for Electrical Impedance Tomography. Sensors 2019, 19, 3679. [Google Scholar] [CrossRef] [Green Version]
- Neumayer, M.; Steiner, G.; Watzenig, D. Electrical Capacitance Tomography: Current sensors/algorithms and future advances. In Proceedings of the 2012 IEEE International Instrumentation and Measurement Technology Conference Proceedings, Graz, Austria, 13–16 May 2012; pp. 929–934. [Google Scholar] [CrossRef]
- Neumayer, M.; Bretterklieber, T.; Flatscher, M.; Puttinger, S. PCA based state reduction for inverse problems using prior information. COMPEL— Int. J. Comput. Math. Electr. Electron. Eng. 2017, 36, 1430–1441. [Google Scholar] [CrossRef]
- Neumayer, M.; Flatscher, M.; Bretterklieber, T. Coaxial Probe for Dielectric Measurements of Aerated Pulverized Materials. IEEE Trans. Instrum. Meas. 2019, 68, 1402–1411. [Google Scholar] [CrossRef]
- Neumayer, M.; Bretterklieber, T.; Flatscher, M. Signal Processing for Capacitive Ice Sensing: Electrode Topology and Algorithm Design. IEEE Trans. Instrum. Meas. 2019, 68, 1458–1466. [Google Scholar] [CrossRef]
- Baxter, L.K. Capacitive Sensors: Design and Applications, 1st ed.; Wiley-IEEE Press: Hoes Lane: Piscataway, NJ, USA, 1996. [Google Scholar]
- Wilcoxon, R. Does a 10 °C Increase in Temperature Really Reduce the Life of Electronics by Half? Electron. Cool. 2017, 9, 6–7. [Google Scholar]
- Webber, A. Application Report SPRABX4B: Calculating Useful Lifetimes of Embedded Processors. 2020. Available online: https://www.ti.com/lit/an/sprabx4b/sprabx4b.pdf (accessed on 19 January 2022).
- Patel, M.R. De-Rating Parts for Reliability. In The International Handbook of Space Technology, 1st ed.; Macdonald, M., Badescu, V., Eds.; Springer: Berlin/Heidelberg, Germany, 2014; Chapter 10.3.3; p. 266. [Google Scholar] [CrossRef]
- Çengel, Y.A. Cooling of Electronic Equipment. In Heat Transfer: A Practical Approach, 2nd ed.; McGraw-Hill: New York, NY, USA, 2002; Chapter 15; p. 787. [Google Scholar]
- Sohel Murshed, S.; Nieto de Castro, C. A critical review of traditional and emerging techniques and fluids for electronics cooling. Renew. Sustain. Energy Rev. 2017, 78, 821–833. [Google Scholar] [CrossRef]
- Flatscher, M.; Neumayer, M.; Bretterklieber, T.; Wegleiter, H. Front-end circuit modeling for low-Z capacitance measurement applications. In Proceedings of the IEEE International Instrumentation and Measurement Technology Conference Proceedings, Taipei, Taiwan, 23–26 May 2016; pp. 1400–1405. [Google Scholar] [CrossRef]
- Dreike, P.; Fleetwood, D.; King, D.; Sprauer, D.; Zipperian, T. An overview of high-temperature electronic device technologies and potential applications. IEEE Trans. Compon. Packag. Manuf. Technol. Part 1994, 17, 594–609. [Google Scholar] [CrossRef]
- Watson, J.; Castro, G. A review of high-temperature electronics technology and applications. J. Mater. Sci. Mater. Electron. 2015, 26, 9226–9235. [Google Scholar] [CrossRef]
- Yang, W. Design of electrical capacitance tomography sensors. Meas. Sci. Technol. 2010, 21, 042001. [Google Scholar] [CrossRef]
- Wegleiter, H.; Fuchs, A.; Holler, G.; Kortschak, B. Analysis of hardware concepts for electrical capacitance tomography applications. In Proceedings of the IEEE SENSORS, Irvine, CA, USA, 30 October–3 November 2005; pp. 688–691. [Google Scholar] [CrossRef]
- Flatscher, M.; Neumayer, M.; Bretterklieber, T. Impedance matched electrical capacitance tomography system: Front-end design and system analysis. Meas. Sci. Technol. 2019, 30, 104002. [Google Scholar] [CrossRef]
- Flatscher, M.; Neumayer, M.; Bretterklieber, T. Impedance Matched Front-End Circuitry for Electrical Capacitance Tomography Systems. In Proceedings of the 9th World Congress on Industrial Process Tomography, Bath, UK, 2–6 September 2018; pp. 537–545. [Google Scholar]
- Kerö, N.; Sauter, T. Design of an integrated angular sensor system. In Proceedings of the IMTC 18th IEEE Instrumentation and Measurement Technology Conference, Budapest, Hungary, 21–23 May 2001; Volume 1, pp. 433–436. [Google Scholar] [CrossRef]
- Brasseur, G. A capacitive finger-type angular-position and angular-speed sensor. In Proceedings of the IMTC/98 Conference Proceedings. IEEE Instrumentation and Measurement Technology Conference, St. Paul, MN, USA, 18–21 May 1998; Volume 2, pp. 967–972. [Google Scholar] [CrossRef]
- Brasseur, G. Modeling of the front end of a new capacitive finger-type angular-position sensor. IEEE Trans. Instrum. Meas. 2001, 50, 111–116. [Google Scholar] [CrossRef]
- Wegleiter, H.; Fuchs, A.; Holler, G.; Kortschak, B. Development of a displacement current-based sensor for electrical capacitance tomography applications. Flow Meas. Instrum. 2008, 19, 241–250. [Google Scholar] [CrossRef]
- Scherz, P.; Monk, S. Parallel-Resonant Circuits. In Practical Electronics for Inventors, 3rd ed.; McGraw-Hill Education: New York, NY, USA, 2013; Chapter 2.30.6; pp. 196–202. [Google Scholar]
- Neumayer, M.; Flatscher, M.; Bretterklieber, T. Front End Instrumentation Modeling of Electrical Tomography Systems. In Proceedings of the 9th World Congress on Industrial Process Tomography, Bath, UK, 2–6 September 2018; pp. 423–432. [Google Scholar]
- Horowitz, P.; Hill, W. Sinewave Oscillators. In The Art of Electronics, 3rd ed.; Cambridge University Press: New York, NY, USA, 2015; Chapter 7.1.5; pp. 435–443. [Google Scholar]
- Abdul Rahim, R.; Tee, Z.C.; Fazalul Rahiman, M.H.; Pusppanathan, J. A Low Cost and High Speed Electrical Capacitance Tomography System Design. Sensors Transducers J. 2010, 114, 83–101. [Google Scholar]
- Williams, P.; York, T. Evaluation of Integrated Electrodes for Electrical Capacitance Tomography. In Proceedings of the 1st World Congress on Industrial Process Tomography, Buxton, UK, 14–17 April 1999; pp. 370–376. [Google Scholar]
- Mohamad, E.J.; Rahim, R.A.; Ling, L.P.; Rahiman, M.H.F.; Bin Marwah, O.M.F.; Ayob, N.M.N. Segmented Capacitance Tomography Electrodes: A Design and Experimental Verifications. IEEE Sens. J. 2012, 12, 1589–1598. [Google Scholar] [CrossRef]
- Da Silva, M.J.; Hampel, U. A field-focusing imaging sensor for fast visualization of multiphase flows. Meas. Sci. Technol. 2009, 20, 104009. [Google Scholar] [CrossRef]
- Kryszyn, J.; Wróblewski, P.; Stosio, M.; Wanta, D.; Olszewski, T.; Smolik, W. Architecture of EVT4 data acquisition system for electrical capacitance tomography. Measurement 2017, 101, 28–39. [Google Scholar] [CrossRef]
- Yang, Y.; Peng, L.; Jia, J. A novel multi-electrode sensing strategy for electrical capacitance tomography with ultra-low dynamic range. Flow Meas. Instrum. 2017, 53, 67–79. [Google Scholar] [CrossRef] [Green Version]
- Huang, A.; Cao, Z.; Sun, S.; Lu, F.; Xu, L. An Agile Electrical Capacitance Tomography System With Improved Frame Rates. IEEE Sens. J. 2019, 19, 1416–1425. [Google Scholar] [CrossRef]
- Analog Devices. AD8000: 1.5 GHz, Ultrahigh Speed Op Amp. Available online: https://www.analog.com/media/en/technical-documentation/data-sheets/AD8000.pdf (accessed on 19 January 2022).
- Horowitz, P.; Hill, W. Inverting Amplifier. In The Art of Electronics, 3rd ed.; Cambridge University Press: New York, NY, USA, 2015; Chapter 4.2.1; pp. 225–226. [Google Scholar]
- Rohde & Schwarz. ZVL3 Vector Network Analyze. Available online: https://www.rohde-schwarz.com/product/zvl-productstartpage_63493-9014.html;https://scdn.rohde-schwarz.com/ur/pws/dl_downloads/dl_common_library/dl_brochures_and_datasheets/pdf_1/ZVL_dat-sw_en_5213-8150-22_v1200.pdf (accessed on 19 January 2022).
- Yu, H.; Grundler, D. VNA Calibration. In Spin Wave Confinement: Propagating Waves, 2nd ed.; Demokritov, S.O., Ed.; Pan Stanford Publishing Pte. Ltd.: Temasek Boulevard, Singapore, 2017; Chapter 7.4.2; p. 206. [Google Scholar] [CrossRef]
- Pozar, D.M. The Scattering Matrix. In Microwave Engineering, 4th ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2011; Chapter 4.3; pp. 178–188. [Google Scholar]
- Pozar, D.M. Transmission Line Theory. In Microwave Engineering, 4th ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2011; Chapter 2; pp. 48–94. [Google Scholar]
- Pro-Power. RG174A/U—Coaxial Cable. Part Number: PP000620. Available online: http://www.farnell.com/datasheets/2076340.pdf?_ga=2.123439243.182990577.1560099811-1902511469.1560099811 (accessed on 19 January 2022).
- Cheng, D.K. Wave Characteristics on Finite Transmission Lines. In Field and Wave Electromagnetics, 2nd ed.; CRC Press: Reading, MA, USA, 1989; Chapter 9.4; pp. 449–471. [Google Scholar]
- Cartwright, K.V.; Joseph, E.; Kaminsky, E.J. Finding the Exact Maximum Impedance Resonant Frequency of a Practical Parallel Resonant Circuit without Calculus. Technol. Interface Internat. J. 2010, 11, 26–36. [Google Scholar]
- Boylestad, R.L. Resonance. In Introductory Circuit Analysis, 13th ed.; Pearson: Harlow, UK, 2016; Chapter 21; pp. 921–967. [Google Scholar]
- Analog Devices. AD8099: Ultralow Distortion, High Speed, 0.95nV/ Voltage Noise Op Amp. Available online: https://www.analog.com/media/en/technical-documentation/data-sheets/AD8099.pdf (accessed on 19 January 2022).
- Wegleiter, H.; Fuchs, A.; Watzenig, D.; Zangl, H.; Steiner, G. Phase Sensitive Demodulation Front-End for Electrical Capacitance Tomography Applications. In Proceedings of the 5th World Congress on Industrial Process Tomography, Bergen, Norway, 3–6 September 2007; pp. 196–201. [Google Scholar]
- Rosa, E.B.; Grover, F.W. Self and Mutual Inductance of Linear Conductors. In Formulas and Tables for the Calculation of Mutual and Self-Inductance; Bulletin of the Bureau of Standards: Ann Arbor, MI, USA, 1912; Volume 8.1, Chapter 8; pp. 150–166. Available online: https://nvlpubs.nist.gov/nistpubs/bulletin/08/nbsbulletinv8n1p1_A2b.pdf (accessed on 19 January 2022).
- Horowitz, P.; Hill, W. PCB traces. In The Art of Electronics: The X-Chapters, 1st ed.; Cambridge University Press: New York, NY, USA, 2020; Chapter 1x.1.4; pp. 7–9. [Google Scholar]
- Grubmüller, M.; Schweighofer, B.; Wegleiter, H. Characterization of a resistive voltage divider design for wideband power measurements. In Proceedings of the SENSORS, IEEE, Valencia, Spain, 2–5 November 2014; pp. 1332–1335. [Google Scholar] [CrossRef]
- Tietze, U.; Schenk, C.; Gamm, E. High-frequency equivalent circuits. In Electronic Circuits, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2008; Chapter 26.1; pp. 1283–1289. [Google Scholar] [CrossRef]



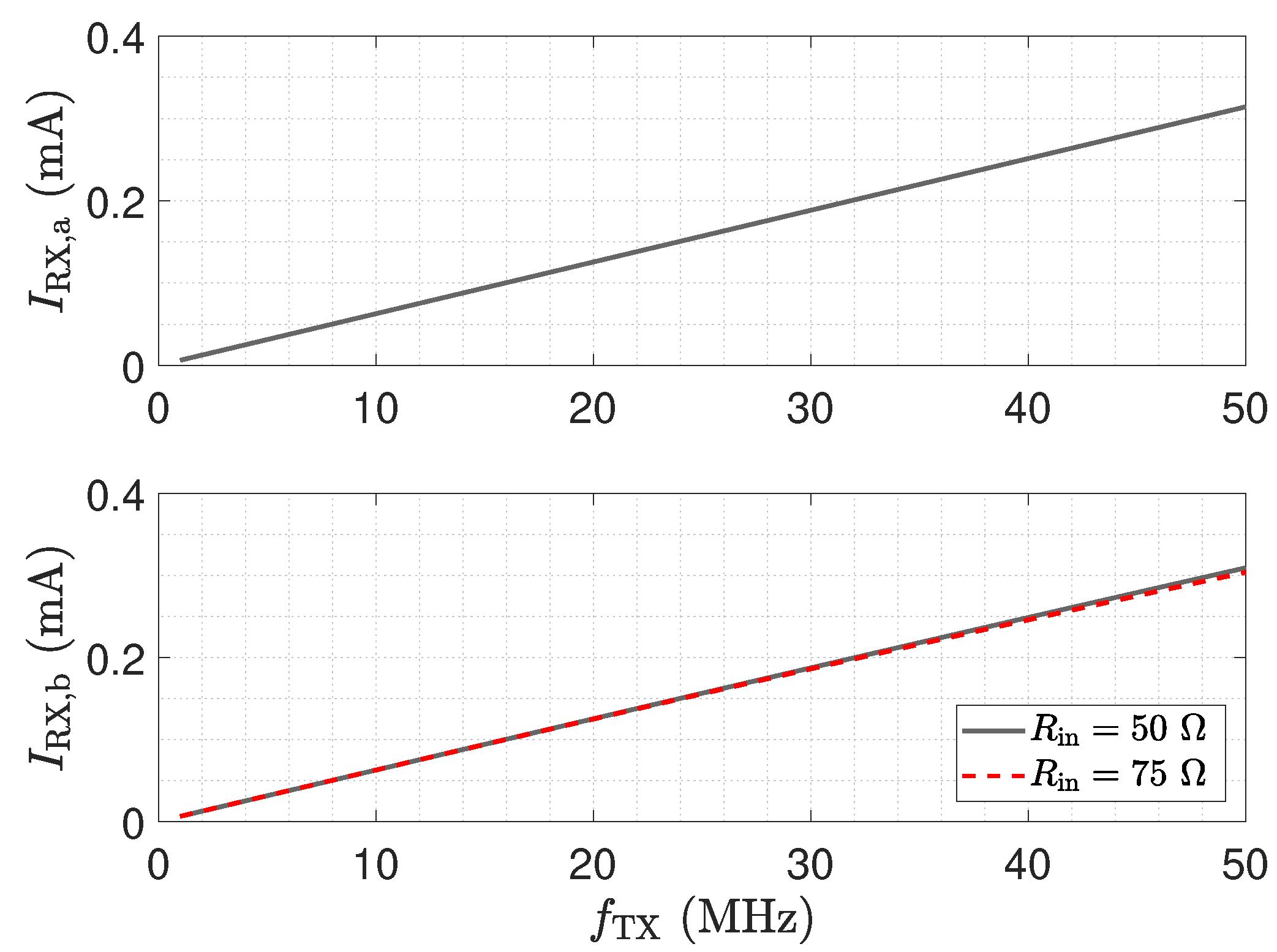
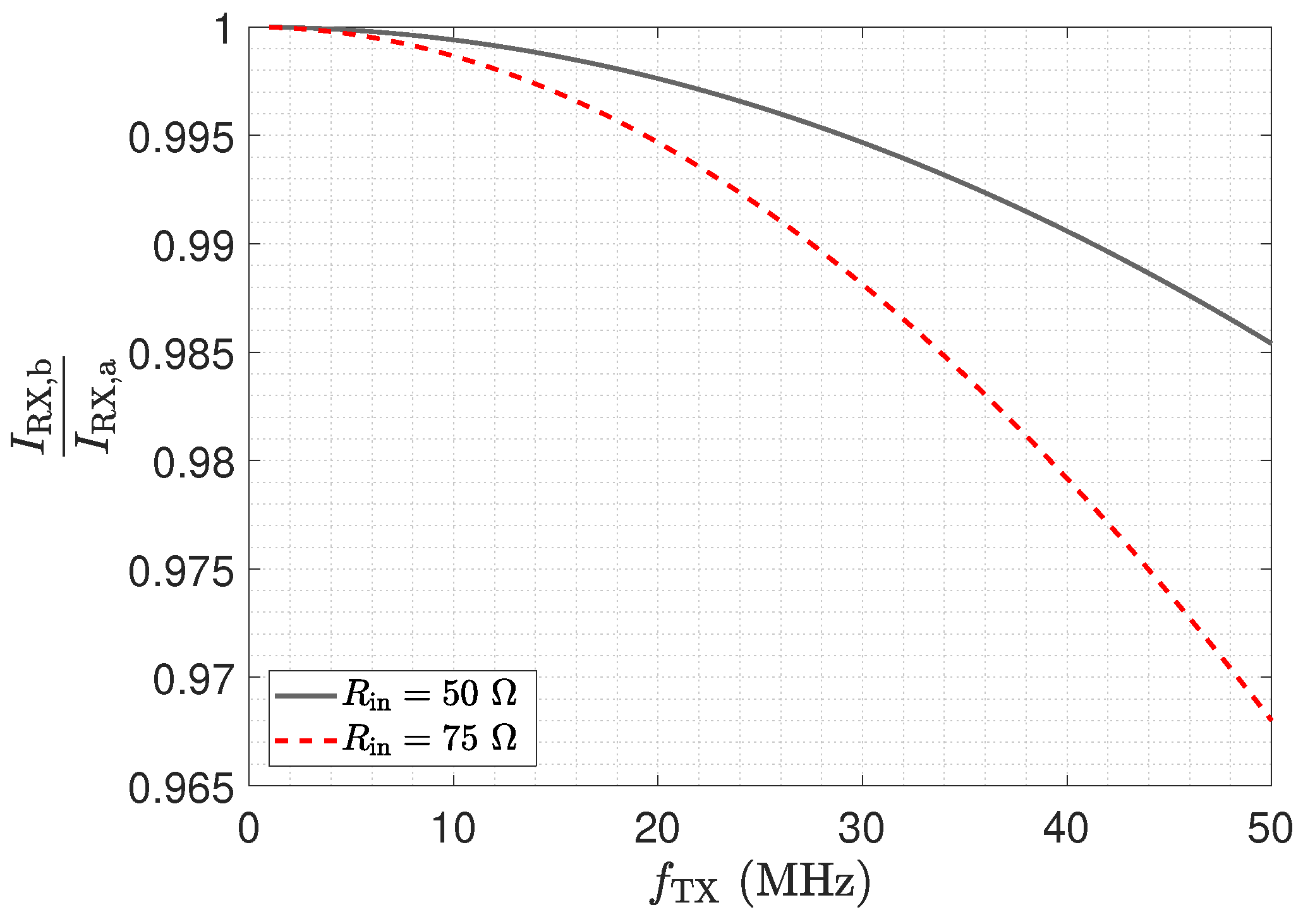
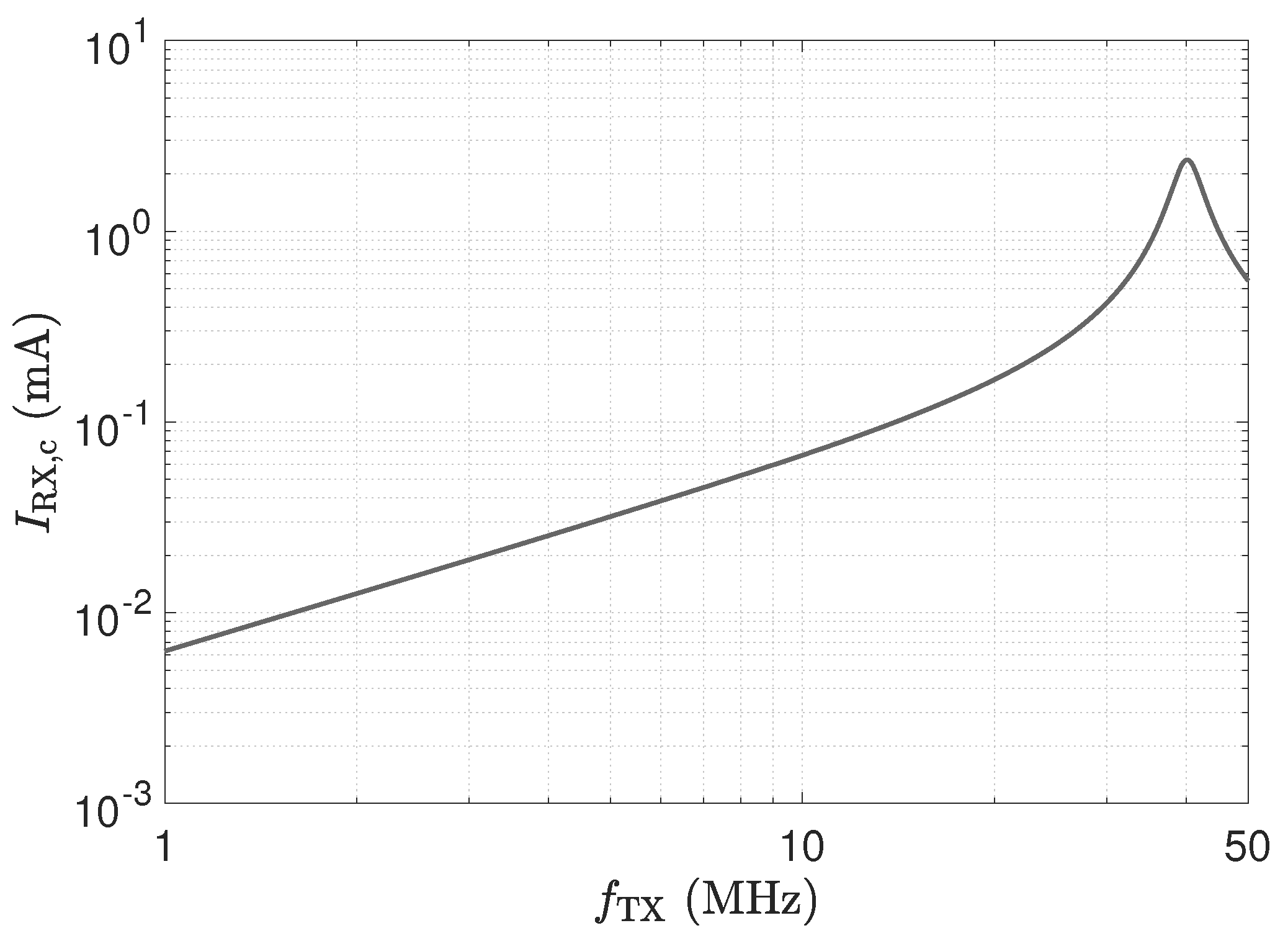

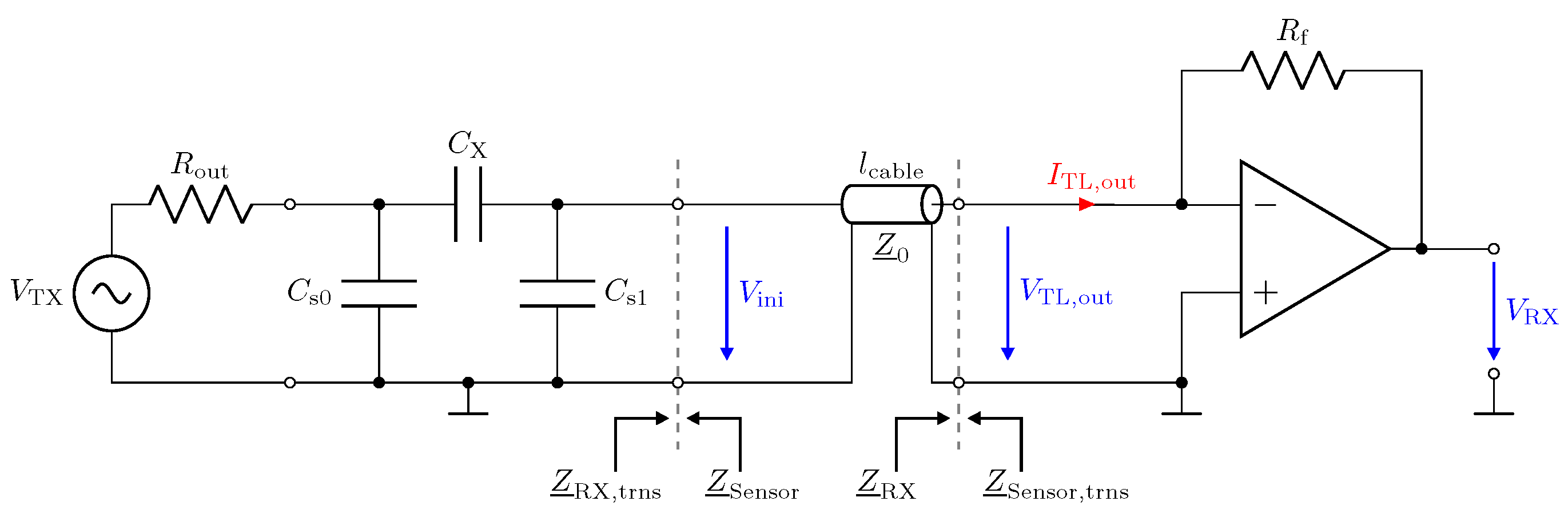










| Nr. | Description | Measurement | Simulation |
|---|---|---|---|
| 1 | low-Z Rec.: effect | Figure 11, Figure 13 | Figure 12 |
| 2 | low-Z Rec.: effect; noise | Figure 11 | Figure 16, Figure 12, Equation (17) |
| 3 | low-Z Rec.: effect | Figure 13 | - |
| 4 | low-Z Rec.: effect; noise | Figure 11 | Figure 16, Figure 12, Equation (17) |
| 5 | Matched Rec. | Figure 19, Figure 18 | - |
| 6 | Matched Rec.: noise | Figure 18 | Figure 20, Equation (17) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Flatscher, M.; Neumayer, M.; Bretterklieber, T.; Wegleiter, H. Transmission Lines in Capacitance Measurement Systems: An Investigation of Receiver Structures. Sensors 2023, 23, 1148. https://doi.org/10.3390/s23031148
Flatscher M, Neumayer M, Bretterklieber T, Wegleiter H. Transmission Lines in Capacitance Measurement Systems: An Investigation of Receiver Structures. Sensors. 2023; 23(3):1148. https://doi.org/10.3390/s23031148
Chicago/Turabian StyleFlatscher, Matthias, Markus Neumayer, Thomas Bretterklieber, and Hannes Wegleiter. 2023. "Transmission Lines in Capacitance Measurement Systems: An Investigation of Receiver Structures" Sensors 23, no. 3: 1148. https://doi.org/10.3390/s23031148
APA StyleFlatscher, M., Neumayer, M., Bretterklieber, T., & Wegleiter, H. (2023). Transmission Lines in Capacitance Measurement Systems: An Investigation of Receiver Structures. Sensors, 23(3), 1148. https://doi.org/10.3390/s23031148







