LED Junction Temperature Measurement: From Steady State to Transient State
Abstract
1. Introduction
2. Steady-State LED Junction Temperature Detection Techniques
2.1. Micro-Thermocouple Method
2.2. Liquid Crystal Thermography (LCT) Method
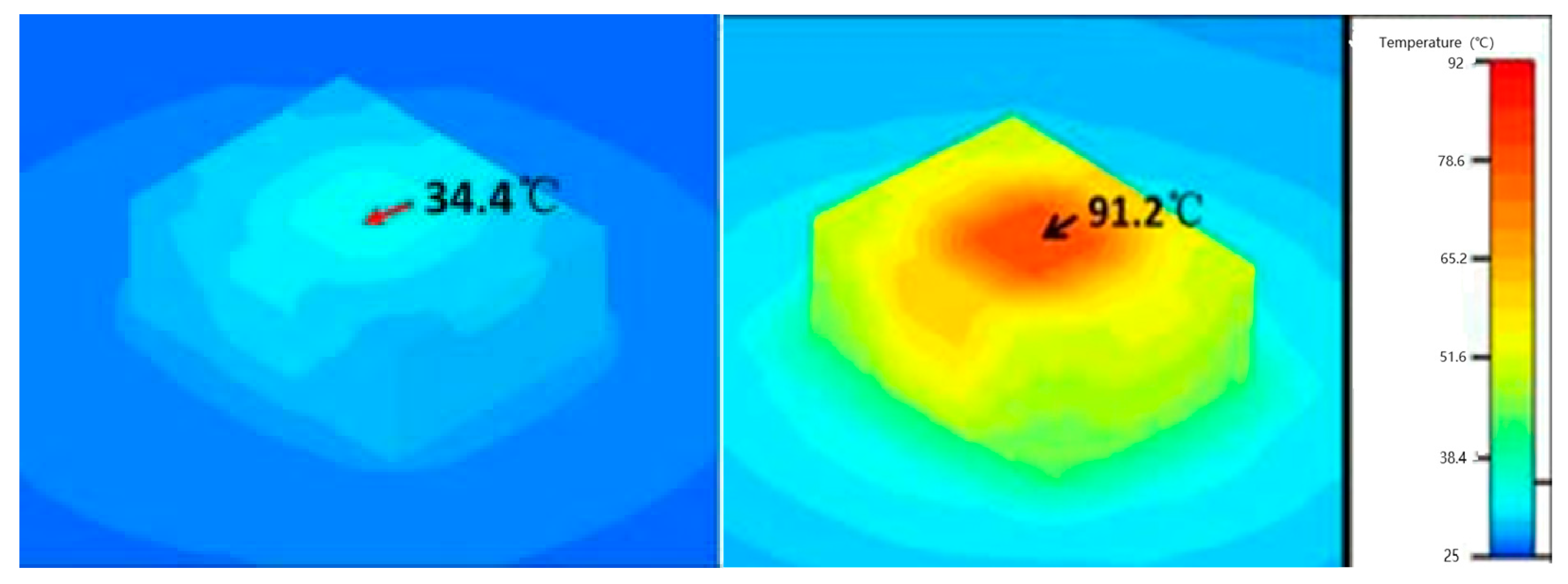
2.3. Infrared (IR) Thermography Method
2.4. Temperature Sensitive Optical Parameters (TSOPs)
2.5. Heterodyne Method
3. Transient LED Junction Temperature Detection Techniques
3.1. Transient Thermal Tester Method (T3ster)
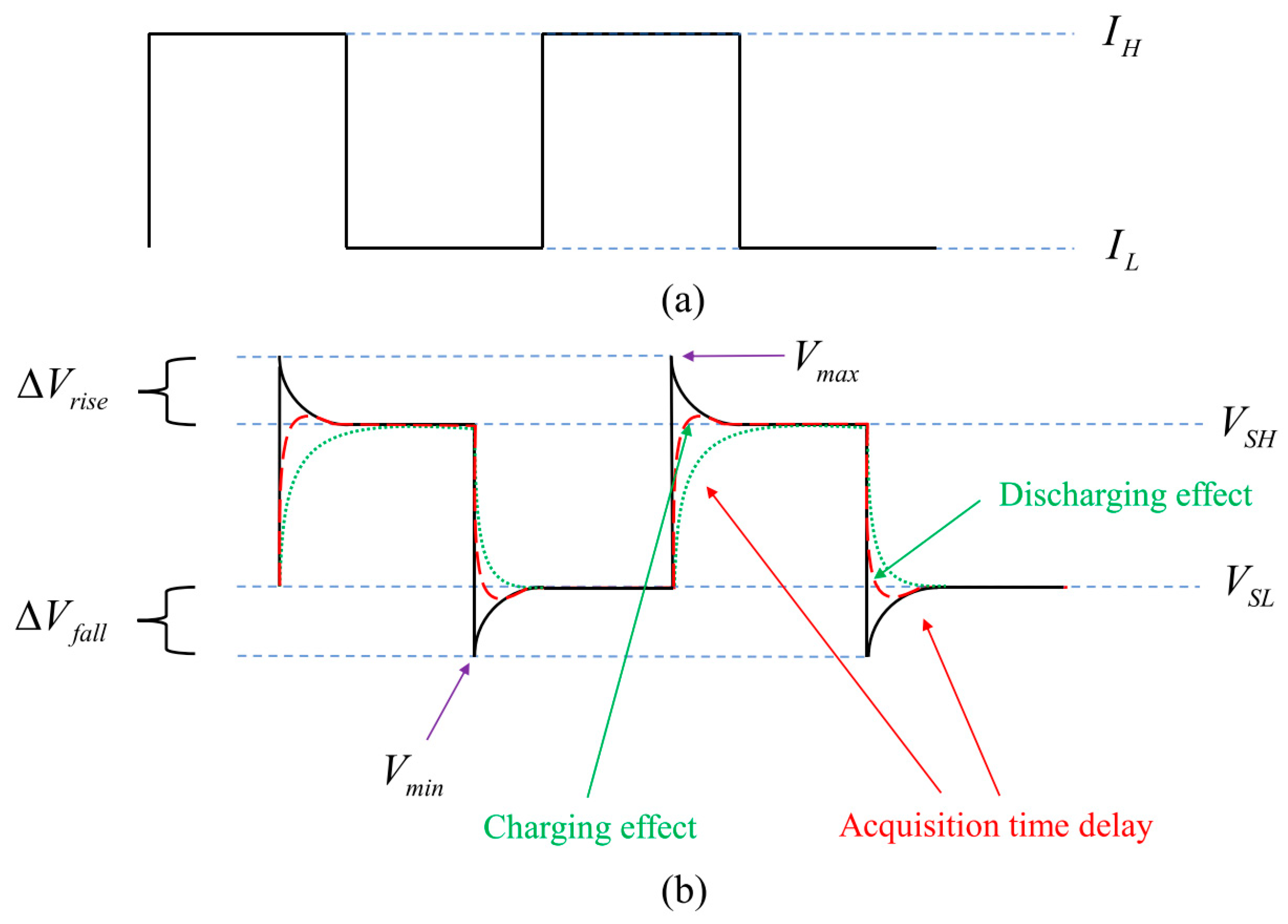
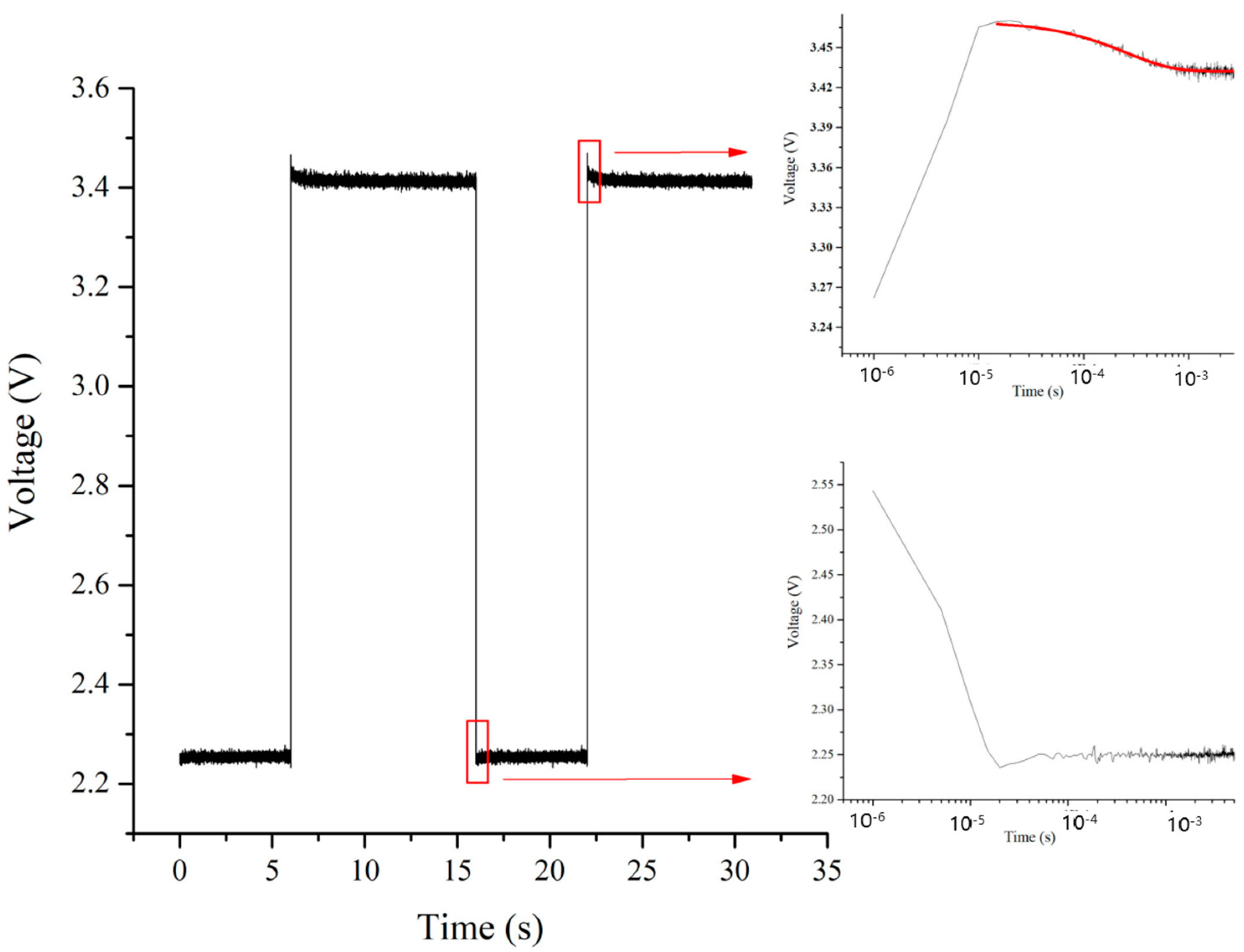
3.2. Continuous Rectangular-Wave Method (CRWM)
3.3. Micro-Raman Spectroscopy

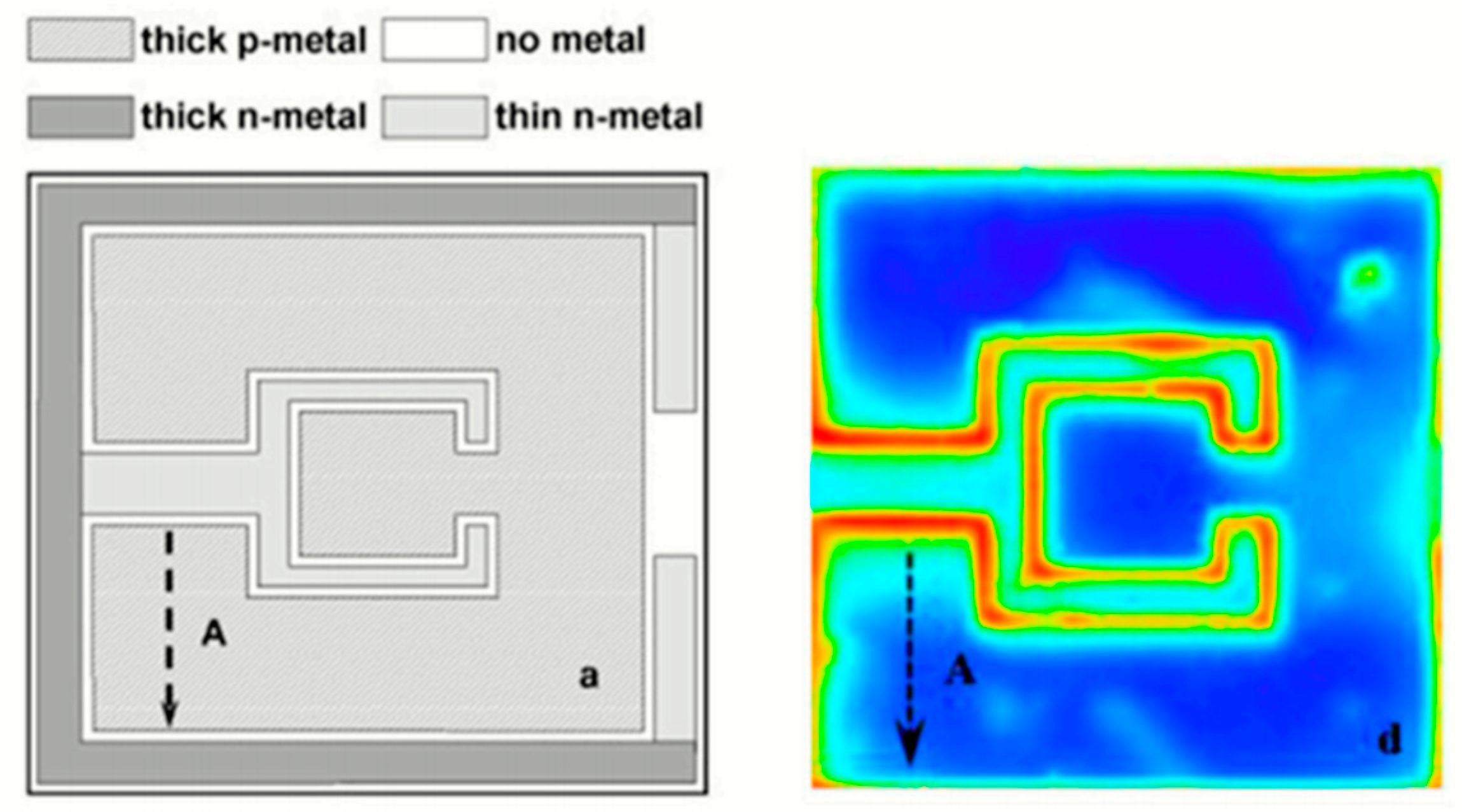
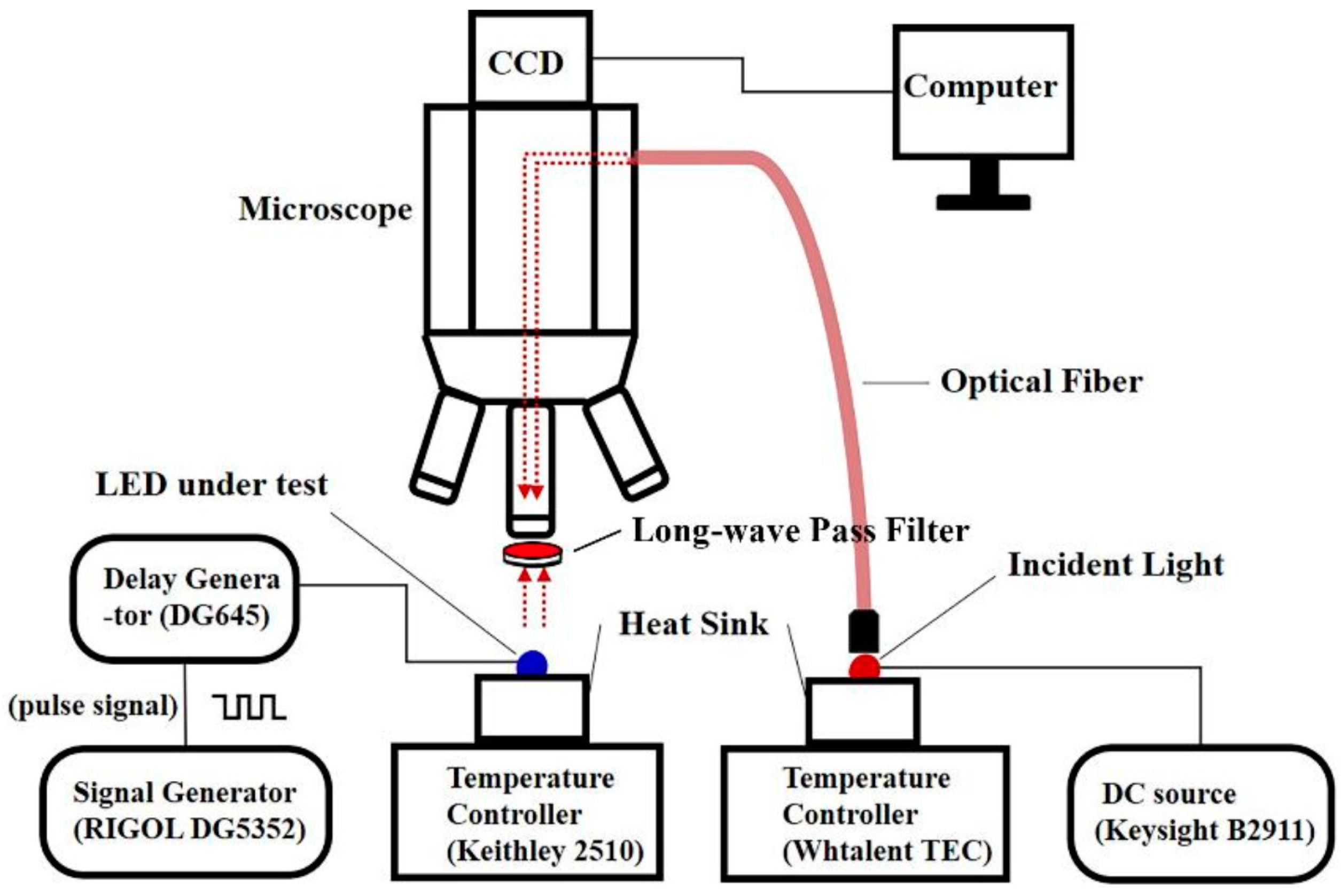
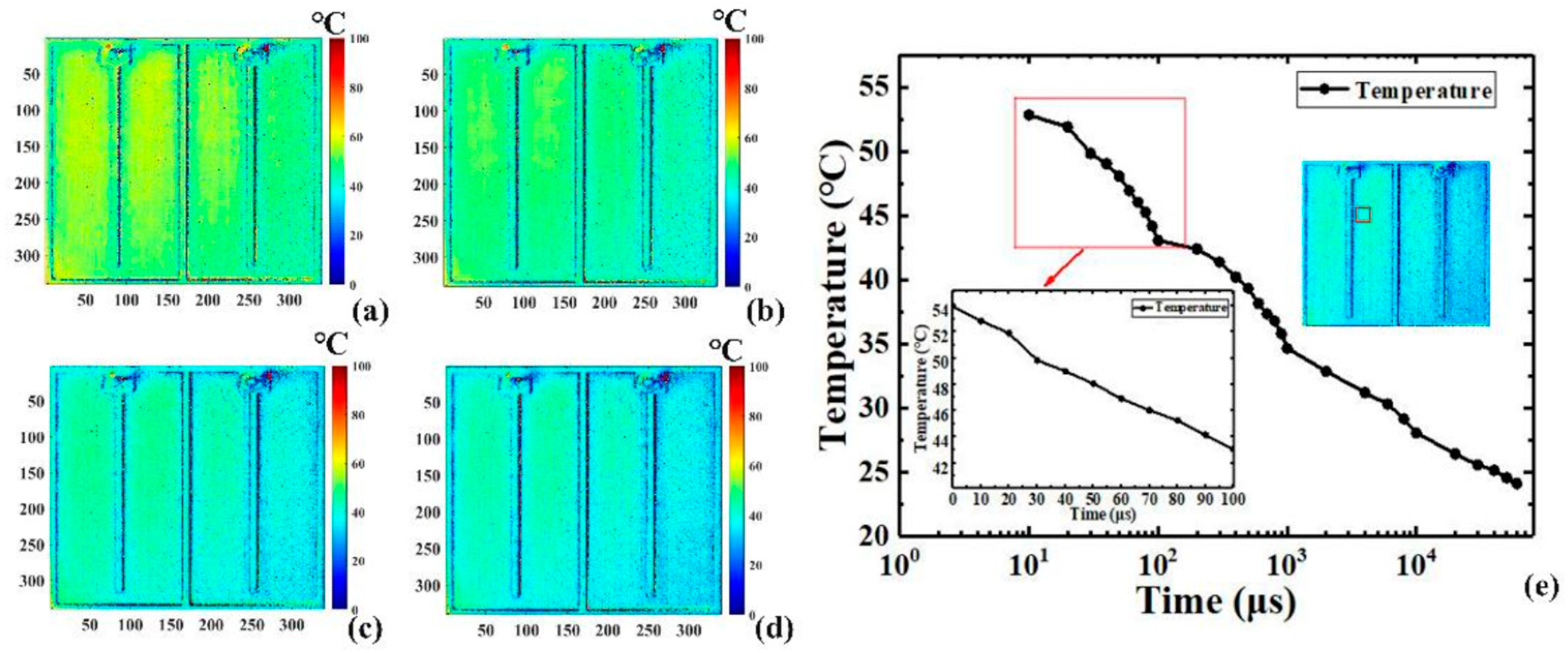
3.4. Thermal Reflection Imaging Method
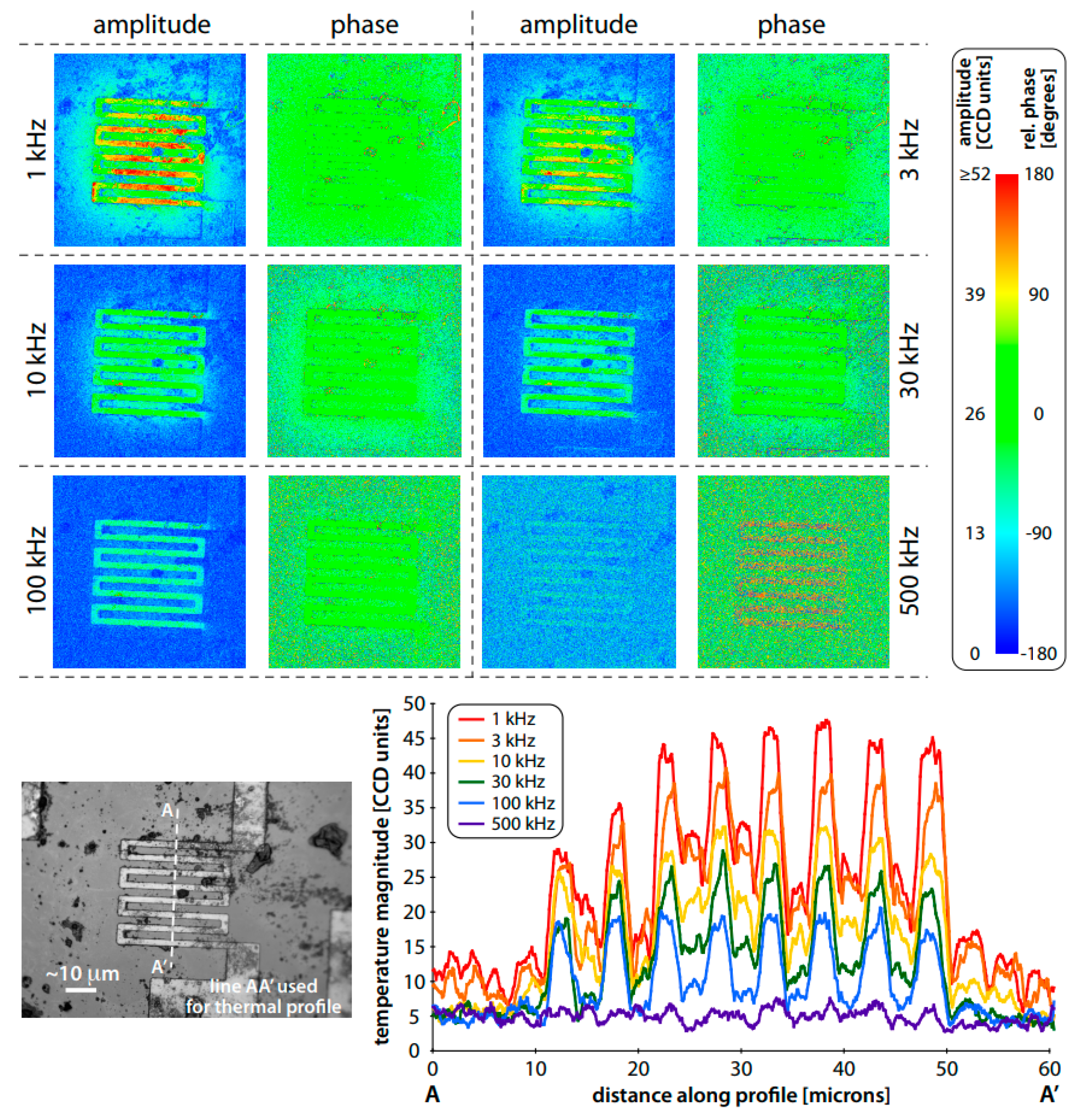
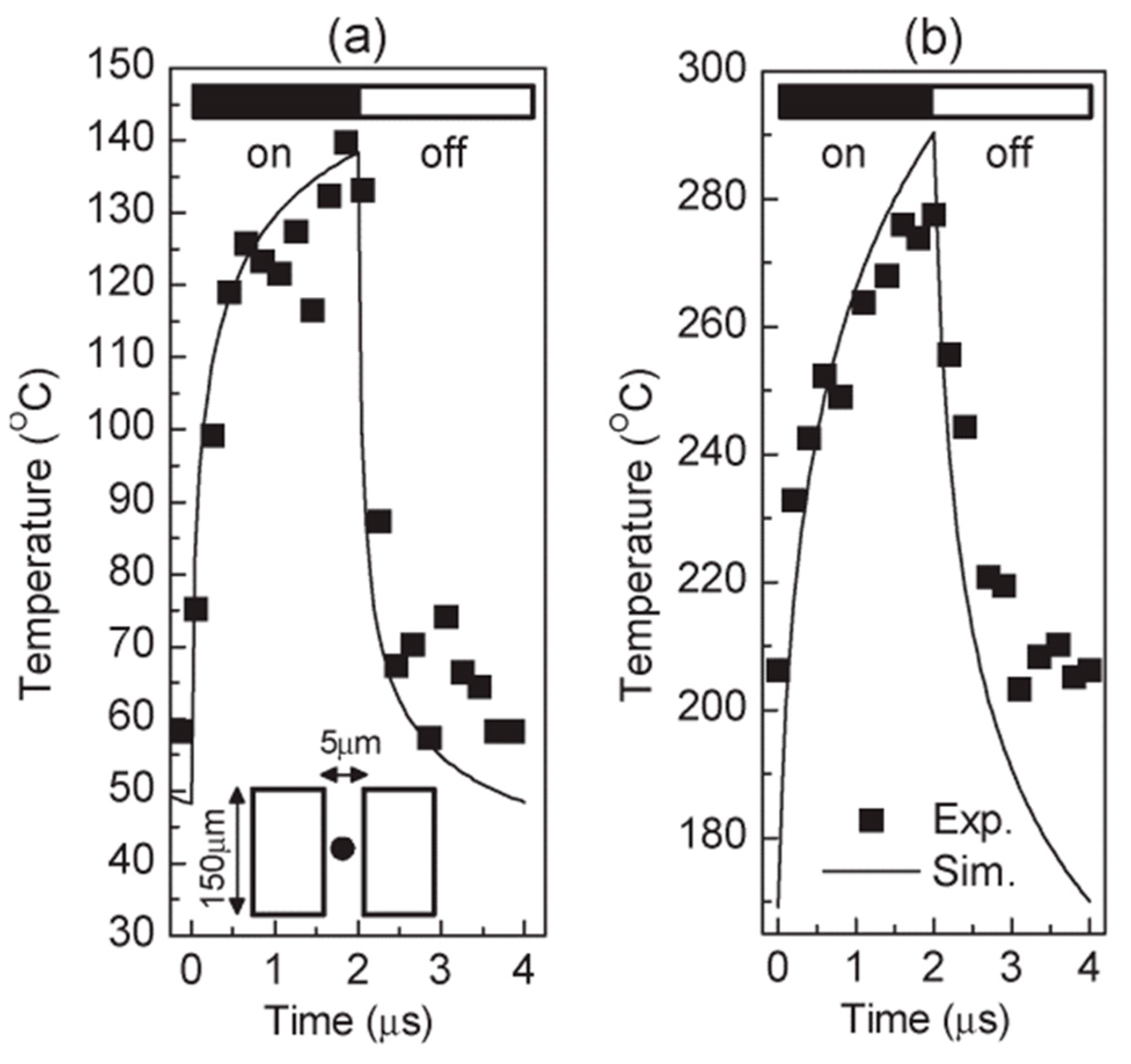
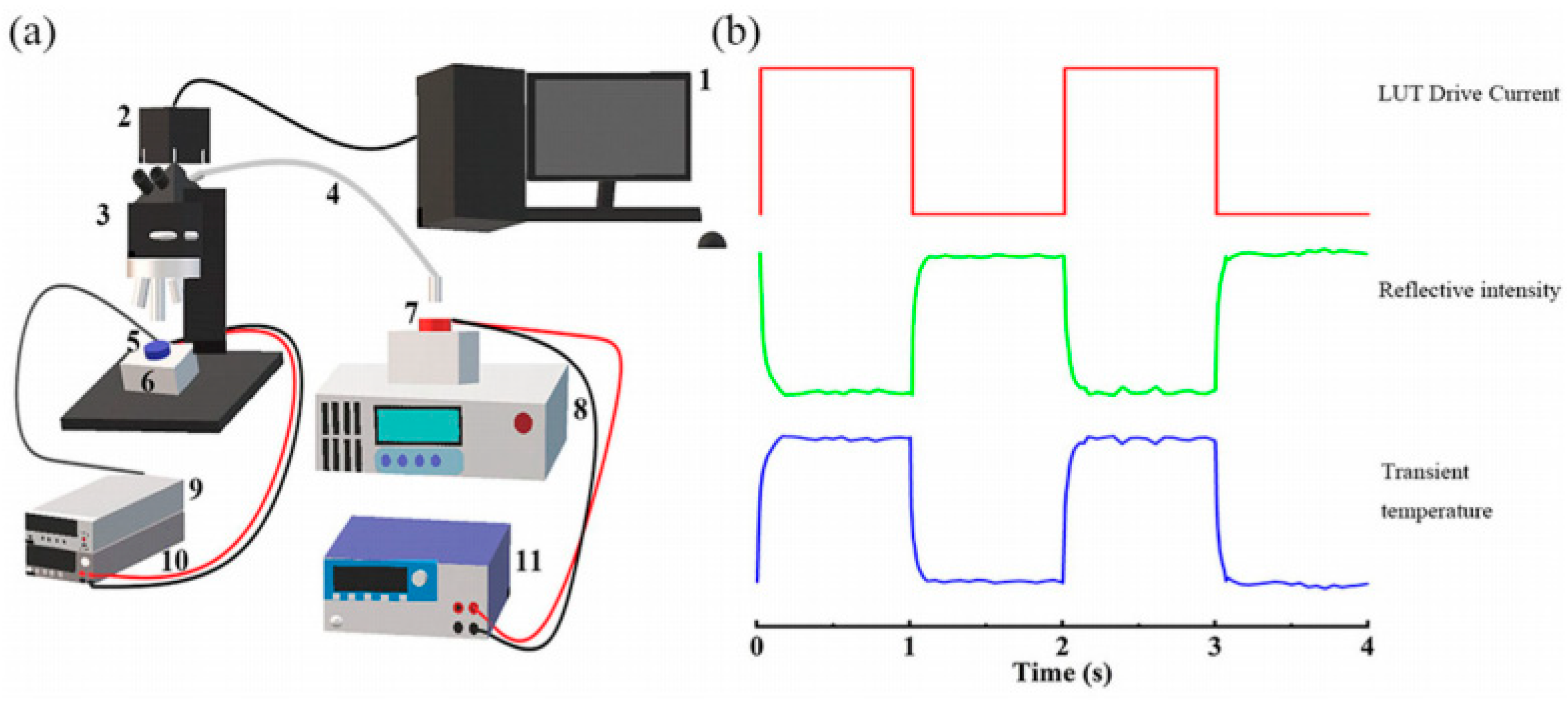
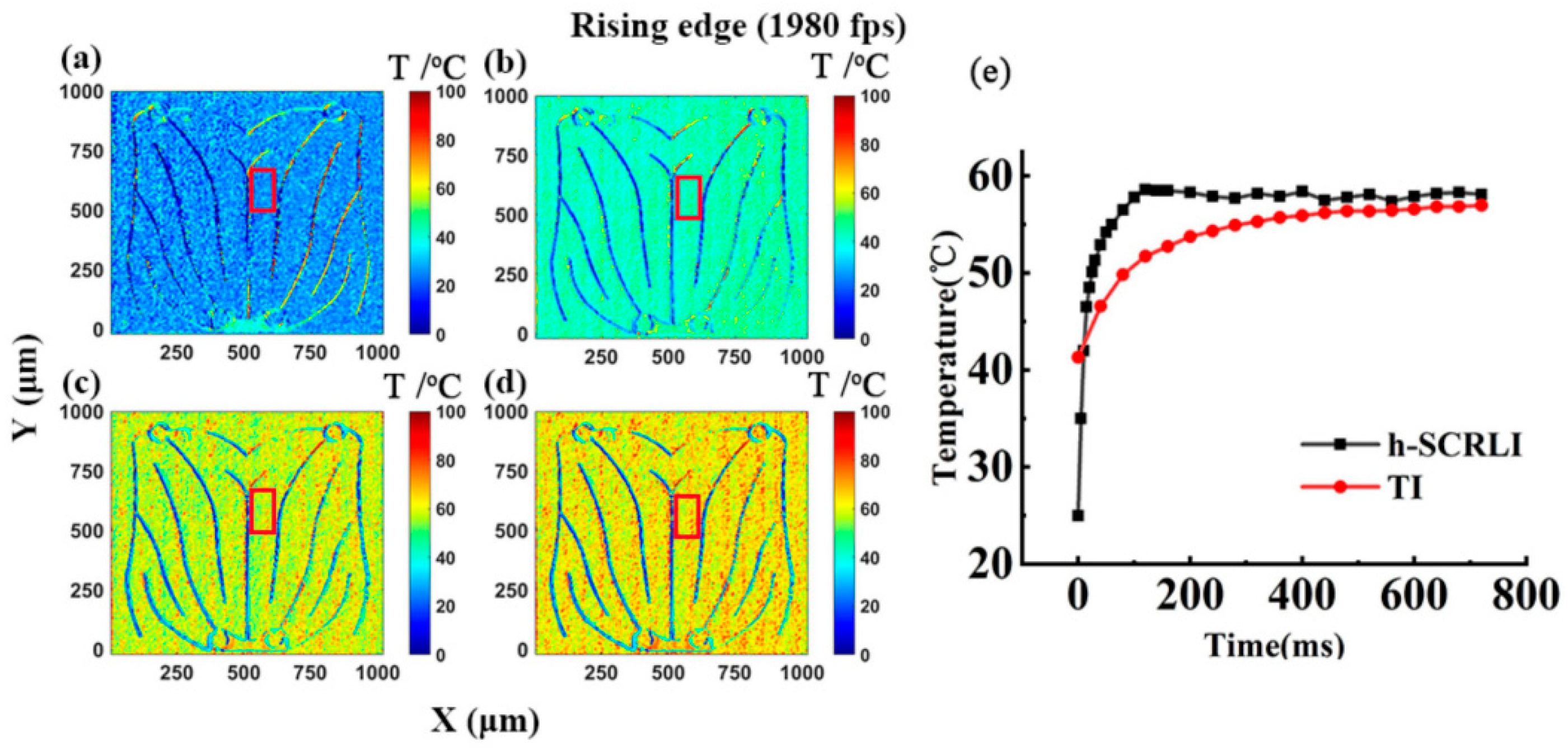
3.5. High-Speed Camera and Reflected Light Intensity Method (h-SCRLI)
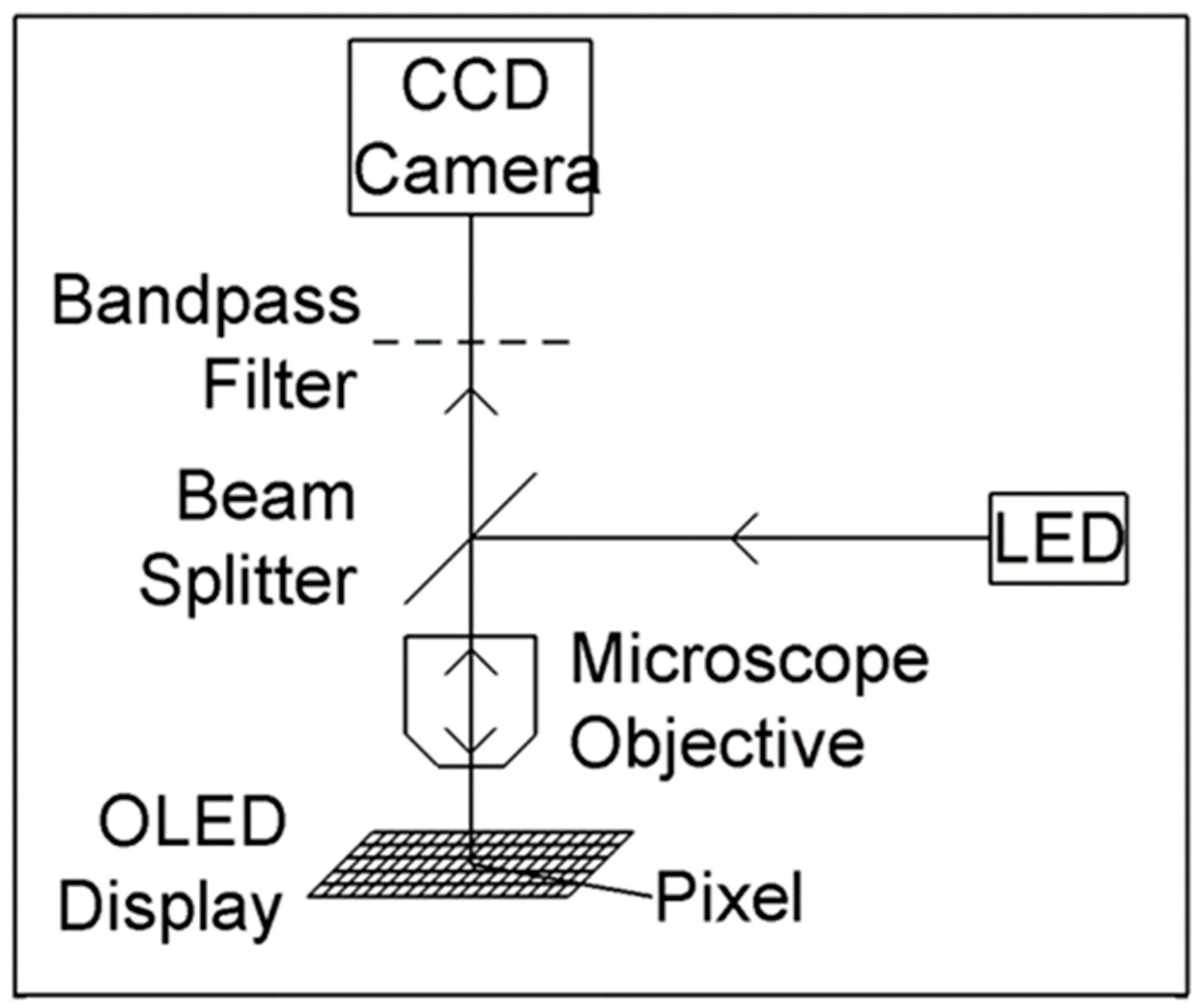
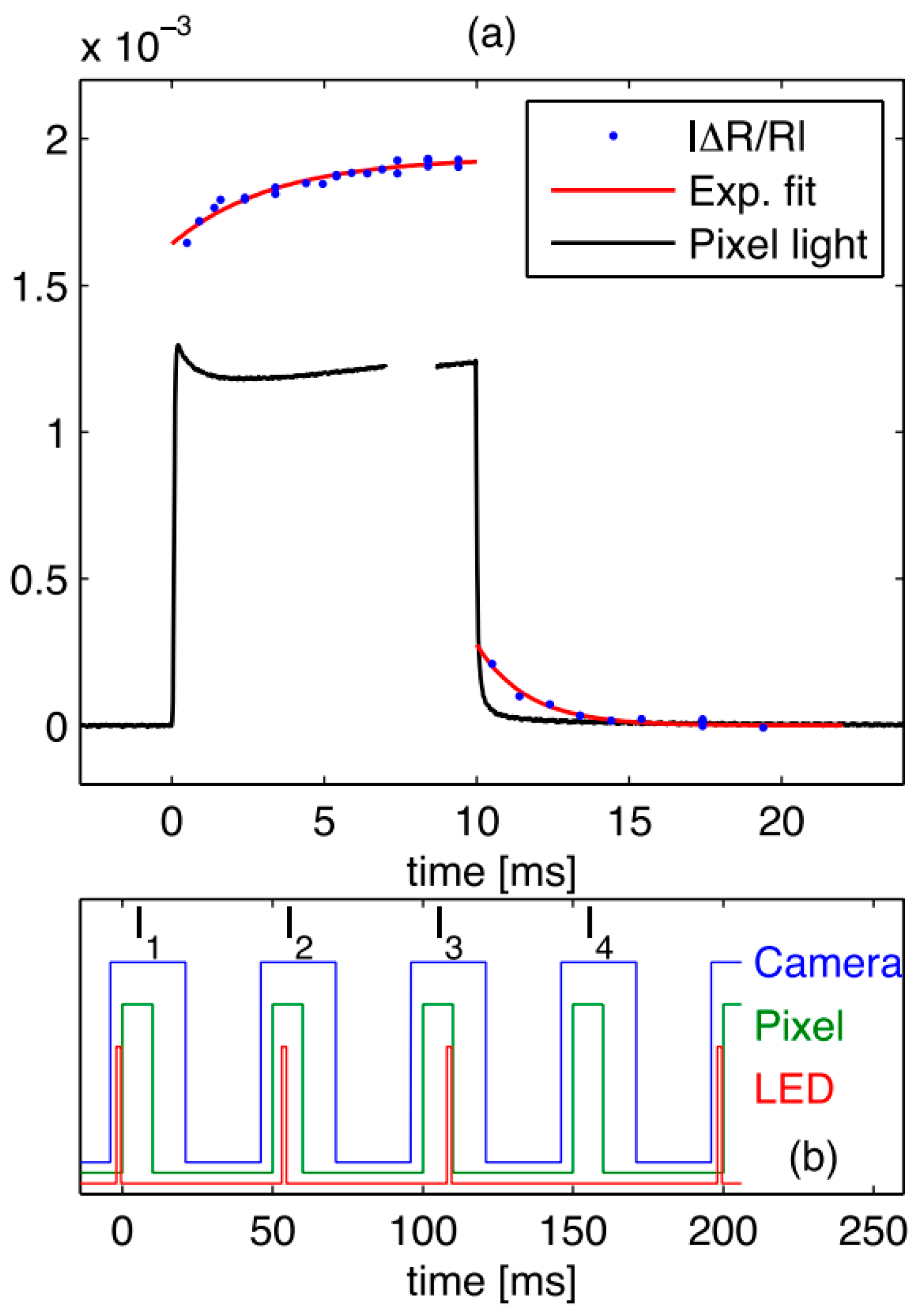
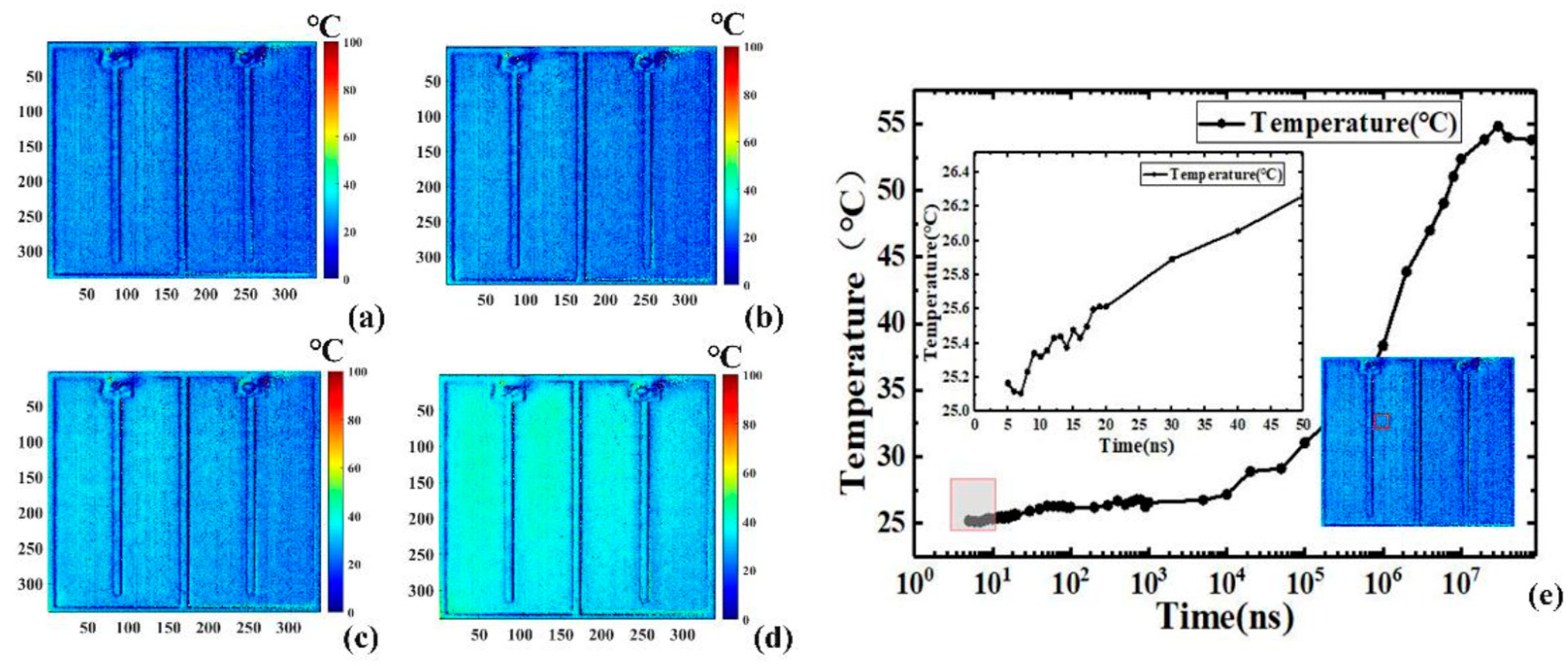
3.6. Micro High-Speed Transient Imaging Based on Reflected Light (μ_HSTI)
4. Summary
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Brown, K.S.; Marean, C.W.; Herries, A.I.R.; Jacobs, Z.; Tribolo, C.; Braun, D.; Roberts, D.L.; Meyer, M.C.; Bernatchez, J. Fire as an Engineering Tool of Early Modern Humans. Science 2009, 325, 859–862. [Google Scholar] [CrossRef]
- James, S.R.; Dennell, R.W.; Gilbert, A.S.; Lewis, H.T.; Gowlett, J.A.J.; Lynch, T.F.; McGrew, W.C.; Peters, C.R.; Pope, G.G.; Stahl, A.B. Hominid Use of Fire in the Lower and Middle Pleistocene: A Review of the Evidence and Comments and Replies. Curr. Anthropol. 1989, 30, 1–26. [Google Scholar] [CrossRef]
- Pyne, S.J. Fire: A Brief History; University of Washington Press: Seattle, WA, USA, 2019. [Google Scholar]
- Shivelbusch, W. The Industrialization of Light in the Nineteenth Century; University of California Press: Oakland, CA, USA, 1989. [Google Scholar]
- Bowers, B.; Anastas, P. Lengthening the Day: A History of Lighting Technology; Oxford University Press: Oxford, UK, 1998. [Google Scholar]
- Smith, N. The Whole Story of Light Bulbs. Eng. Technol. 2018, 13, 54–59. [Google Scholar] [CrossRef]
- Akasaki, I. Key Inventions in the History of Nitride-Based Blue LED and LD. J. Cryst. Growth 2007, 300, 2–10. [Google Scholar] [CrossRef]
- Narukawa, Y.; Narita, J.; Sakamoto, T.; Deguchi, K.; Yamada, T.; Mukai, T. Ultra-High Efficiency White Light Emitting Diodes. Jpn. J. Appl. Phys. 2006, 45, L1084–L1086. [Google Scholar] [CrossRef]
- Rossow, M. Introduction to Solid-State Lighting (LEDs); Wiley: Hoboken, NJ, USA, 2016. [Google Scholar]
- Kim, J.; Schubert, E.F. Transcending the Replacement Paradigm of Solid-State Lighting. Opt. Express 2008, 16, 21835–21842. [Google Scholar] [CrossRef]
- Wang, Z.; Gong, H.; Zhuang, P.; Fu, N.; Zhu, L.; Chen, Z.; Lu, Y. Transient 2D Junction Temperature Distribution Measurement by Short Pulse Driving and Gated Integration with Ordinary CCD Camera. Sensors 2022, 22, 5899. [Google Scholar] [CrossRef]
- Mohammad, S.N.; Morkoç, H. Progress and Prospects of Group-III Nitride Semiconductors. Prog. Quantum Electron. 1996, 20, 361–525. [Google Scholar] [CrossRef]
- Sun, H.; Mitra, S.; Subedi, R.C.; Zhang, Y.; Guo, W.; Ye, J.; Shakfa, M.K.; Ng, T.K.; Ooi, B.S.; Roqan, I.S. Unambiguously Enhanced Ultraviolet Luminescence of AlGaN Wavy Quantum Well Structures Grown on Large Misoriented Sapphire Substrate. Adv. Funct. Mater. 2019, 29, 1905445. [Google Scholar] [CrossRef]
- Zhu, L.H.; Zheng, Q.W.; Ruan, Y.J.; Guo, W.J.; Gao, Y.L.; Guo, Z.Q.; Lin, Y.; Wu, T.Z.; Chen, Z.; Lu, Y.J. Remote Online Two-Step Stress Lifetime Acceleration Test System for Ultraviolet Light-Emitting Diodes. IEEE Trans. Instrum. Meas. 2021, 70, 6009307. [Google Scholar] [CrossRef]
- Chhajed, S.; Xi, Y.; Li, Y.L.; Gessmann, T.; Schubert, E.F. Influence of junction temperature on chromaticity and color-rendering properties of trichromatic white-light sources based on light-emitting diodes. J. Appl. Phys. 2005, 97, 054506. [Google Scholar] [CrossRef]
- Kim, J.H.; Shin, M.W. Thermal behavior of remote phosphor in light-emitting diode packages. IEEE Electron. Device Lett. 2015, 36, 832–834. [Google Scholar] [CrossRef]
- Xiao, G.; Du, W.; Wang, Z.; Chen, G.; Zhu, L.; Gao, Y.; Chen, Z.; Guo, Z.; Lu, Y. Two-Dimensional Transient Temperature Distribution Measurement of GaN Light-Emitting Diode Using High Speed Camera. IEEE J. Electron. Devices Soc. 2021, 9, 663–666. [Google Scholar] [CrossRef]
- Chang, M.H.; Das, D.; Varde, P.V.; Pecht, M. Light Emitting Diodes Reliability Review. Microelectron. Reliab. 2012, 52, 762–782. [Google Scholar] [CrossRef]
- Rahman, F.; George, A.F.; Drinkard, R. Short-and Long-Term Reliability Studies of Broadband Phosphor-Converted Red, Green, and White Light-Emitting Diodes. IEEE Trans. Device Mater. Reliab. 2015, 16, 1–8. [Google Scholar] [CrossRef]
- Chen, Z.; Zhang, Q.; Wang, K.; Luo, X.; Liu, S. Reliability Test and Failure Analysis of High Power LED Packages. J. Semicond. 2011, 32, 14007. [Google Scholar] [CrossRef]
- Horng, R.H.; Lin, R.C.; Chiang, Y.C.; Chuang, B.H.; Hu, H.L.; Hsu, C.P. Failure Modes and Effects Analysis for High Power GaN-Based Light-Emitting Diodes Package Technology. Microelectron. Reliab. 2012, 52, 818–821. [Google Scholar] [CrossRef]
- Van Driel, W.D.; Fan, X.J.; Zhang, G.Q. Solid State Lighting Reliability Part 2; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Yang, S.C.; Lin, P.; Wang, C.P.; Huang, S.B.; Chen, C.L.; Chiang, P.F.; Lee, A.T.; Chu, M.T. Failure and DegradationMechanisms of High-Power White Light Emitting Diodes. Microelectron. Reliab. 2010, 50, 959–964. [Google Scholar] [CrossRef]
- Narendran, N.; Gu, Y.; Freyssinier, J.P.; Yu, H.; Deng, L. Solid-State Lighting: Failure Analysis of White LEDs. J. Cryst. Growth 2004, 268, 449–456. [Google Scholar] [CrossRef]
- Xie, B.; Liu, H.; Hu, R.; Wang, C.; Hao, J.; Wang, K.; Luo, X. Targeting Cooling for Quantum Dots in White QDs-LEDs by Hexagonal Boron Nitride Platelets with Electrostatic Bonding. Adv. Funct. Mater. 2018, 28, 1801407. [Google Scholar] [CrossRef]
- Weng, C.J. Advanced Thermal Enhancement and Management of LED Packages. Int. Commun. Heat Mass Transf. 2009, 36, 245–248. [Google Scholar] [CrossRef]
- Cengiz, C.; Muslu, A.M.; Azarifar, M.; Arik, M.; Dogruoz, B. Discrete Phase Analysis of Self Heating Particles Over an Immersion Liquid Cooled High Power Blue Light-Emitting Diode With Suspended Phosphor Particles. J. Heat Transfer 2022, 144, 102101. [Google Scholar] [CrossRef]
- Childs, P.R.N.; Greenwood, J.R.; Long, C.A. Review of Temperature Measurement. Rev. Sci. Instrum. 2000, 71, 2959–2978. [Google Scholar] [CrossRef]
- Blackburn, D.L. Temperature Measurements of Semiconductor Devices—A Review. In Proceedings of the Twentieth Annual IEEE Semiconductor Thermal Measurement and Management Symposium (IEEE Cat. No. 04CH37545), San Jose, CA, USA, 11 March 2004. [Google Scholar]
- Avenas, Y.; Dupont, L.; Khatir, Z. Temperature Measurement of Power Semiconductor Devices by Thermo-Sensitive Electrical Parameters—A Review. IEEE Trans. Power Electron. 2012, 27, 3081–3092. [Google Scholar] [CrossRef]
- Baker, N.; Liserre, M.; Dupont, L.; Avenas, Y. Improved Reliability of Power Modules: A Review of Online Junction Temperature Measurement Methods. IEEE Ind. Electron. Mag. 2014, 8, 17–27. [Google Scholar] [CrossRef]
- Kalker, S.; Ruppert, L.A.; Van der Broeck, C.H.; Kuprat, J.; Andresen, M.; Polom, T.A.; Liserre, M.; De Doncker, R.W. Reviewing Thermal Monitoring Techniques for Smart Power Modules. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 10, 1326–1341. [Google Scholar] [CrossRef]
- Qiu, X.Z.; Zhang, G.R.; Chen, W.J.; Yu, T.; Hou, X.M.; Zhang, Q.Z.; Xu, G.Q. Review of Igbt Junction Temperature Extraction and Estimation Methods. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2020; Volume 774, p. 12091. [Google Scholar]
- Sathik, M.H.M.; Pou, J.; Prasanth, S.; Muthu, V.; Simanjorang, R.; Gupta, A.K. Comparison of IGBT Junction Temperature Measurement and Estimation Methods—A Review. In Proceedings of the 2017 Asian Conference on Energy, Power and Transportation Electrification (ACEPT), Singapore, 24–26 October 2017. [Google Scholar]
- Ke, H.L.; Jing, L.; Hao, J.; Gao, Q.; Wang, Y.; Wang, X.X.; Sun, Q.; Xu, Z.J. Analysis of junction temperature and modification of luminous flux degradation for white LEDs in a thermal accelerated reliability test. Appl. Opt. 2016, 55, 5909–5916. [Google Scholar] [CrossRef] [PubMed]
- Trevisanello, L.; Meneghini, M.; Mura, G.; Vanzi, M.; Pavesi, M.; Meneghesso, G.; Zanoni, E. Accelerated life test of high brightness light emitting diodes. IEEE Trans. Device Mater. Reliab. 2008, 8, 304–311. [Google Scholar] [CrossRef]
- Szabó, P.; Steffens, O.; Lenz, M.; Farkas, G. Transient junction-to-case thermal resistance measurement methodology of high accuracy and high repeatability. IEEE Trans. Compon. Packag. Technol. 2005, 28, 630–636. [Google Scholar] [CrossRef]
- Shi, B.; Feng, S.; Shi, L.; Shi, D.; Zhang, Y.; Zhu, H. Junction temperature measurement method for power MOSFETs using turn-on delay of impulse signal. IEEE Trans. Power Electron. 2018, 33, 5274–5282. [Google Scholar] [CrossRef]
- Liu, Z.H.; Huang, J.E.; Gao, Y.L.; Guo, Z.Q.; Lin, Y.; Zhu, L.H.; Chen, Z.; Lu, Y.J. A continuous rectangular-wave method for junction temperature measurement of light-emitting diodes. IEEE Trans. Power Electron. 2019, 34, 10414–10424. [Google Scholar] [CrossRef]
- Xi, Y.; Schubert, E.F. Junction-temperature measurements in GaN UV light-emitting diodes using the diode forward voltage. Int. J. High Speed Electron. Syst. 2004, 14, 708–713. [Google Scholar] [CrossRef]
- Kim, D.S.; Holloway, C.; Han, B.; Bar-Cohen, A. Method for predicting junction temperature distribution in a high-power laser diode bar. Appl. Opt. 2016, 55, 7487–7496. [Google Scholar] [CrossRef] [PubMed]
- Lin, Y.; Gao, Y.L.; Lu, Y.J.; Zhu, L.H.; Zhang, Y.; Chen, Z. Study of temperature sensitive optical parameters and junction temperature determination of light-emitting diodes. Appl. Phys. Lett. 2012, 100, 202108. [Google Scholar] [CrossRef]
- Pollock, D.D. Thermocouples: Theory and Properties; CRC Press: Boca Raton, FL, USA, 1991. [Google Scholar]
- Christofferson, J.; Maize, K.; Ezzahri, Y.; Shabani, J.; Wang, X.; Shakouri, A. Microscale and nanoscale thermal characterization techniques. J. Electron. Packag. 2008, 130, 041101. [Google Scholar] [CrossRef]
- Cree. Solder-Point Temperature Measurement of Cree XLamp LEDs; Cree: Durham, NC, USA, 2021. [Google Scholar]
- OSRAM. Temperature Measurement with Thermocouples; OSRAM: Munich, Germany, 2018. [Google Scholar]
- Song, B.M.; Han, B.; Lee, J.H. Optimum Design Domain of LED-Based Solid State Lighting Considering Cost, Energy Consumption and Reliability. Microelectron. Reliab. 2013, 53, 435–442. [Google Scholar] [CrossRef]
- Faranda, R.; Guzzetti, S.; Lazaroiu, G.C.; Leva, S. Refrigerating Liquid Prototype for LED’s Thermal Management. Appl. Therm. Eng. 2012, 48, 155–163. [Google Scholar] [CrossRef]
- Jung, E.D.; Lee, Y.L. Development of a Heat Dissipating LED Headlamp with Silicone Lens to Replace Halogen Bulbs in Used Cars. Appl. Therm. Eng. 2015, 86, 143–150. [Google Scholar] [CrossRef]
- Rammohan, A.; Chandramohan, V.P. Experimental Analysis on Estimating Junction Temperature and Service Life of High Power LED Array. Microelectron. Reliab. 2021, 120, 114121. [Google Scholar]
- Xiao, Y.; Wu, T.; Dang, S.; Gao, Y.; Lin, Y.; Zhu, L.; Guo, Z.; Lu, Y.; Chen, Z. Determining Junction Temperature of LEDs by the Relative Reflected Intensity of the Incident Exciting Light. IEEE Trans. Electron. Devices 2017, 64, 2257–2260. [Google Scholar] [CrossRef]
- Shih, F.; Tsou, C.; Fang, W. A Monolithic Micromachined Thermocouple Probe With Electroplating Nickel for Micro-LED Inspection. J. Microelectromech. Syst. 2021, 30, 864–875. [Google Scholar] [CrossRef]
- Choi, H.; Choi, W.; Lim, J.; Choi, J. Integrated Microsensor for Precise, Real-Time Measurement of Junction Temperature of Surface-Mounted Light-Emitting Diode. Sens. Actuators A Phys. 2019, 298, 111578. [Google Scholar] [CrossRef]
- Cooper, T.E.; Field, R.J.; Meyer, J.F. Liquid Crystal Thermography and Its Application to the Study of Convective Heat Transfer. J. Heat Transfer 1975, 97, 442–450. [Google Scholar] [CrossRef]
- Csendes, A.; Szekely, V.; Rencz, M. Thermal mapping with liquid crystal method. Microelectron. Eng. 1996, 31, 281–290. [Google Scholar] [CrossRef]
- Cengiz, C.; Azarifar, M.; Arik, M. A critical review on the junction temperature measurement of light emitting diodes. Micromachines 2022, 13, 1615. [Google Scholar] [CrossRef]
- Lee, C.C.; Park, J. Temperature Measurement of Visible Light-Emitting Diodes Using Nematic Liquid Crystal Thermography With Laser Illumination. IEEE Photonics Technol. Lett. 2004, 16, 1706–1708. [Google Scholar] [CrossRef]
- Vollmer, M.; Möllmann, K.P. Infrared Thermal Imaging: Fundamentals, Research and Applications; John Wiley & Sons: Hoboken, NJ, USA, 2017. [Google Scholar]
- Corfa, A.; Gasse, A.; Bernabé, S.; Ribot, H. Analytical and FEM Simulations of the Thermal Spreading Effect in LED Modules and IR Thermography Validation. In Proceedings of the 2010 11th International Thermal, Mechanical & Multi-Physics Simulation, and Experiments in Microelectronics and Microsystems (EuroSimE), Bordeaux, France, 26–28 April 2010. [Google Scholar]
- Jang, H.; Lee, J.H.; Byon, C.; Lee, B.J. Innovative Analytic and Experimental Methods for Thermal Management of SMD-Type LED Chips. Int. J. Heat Mass Transf. 2018, 124, 36–45. [Google Scholar] [CrossRef]
- Chen, Z.Z.; Liu, P.; Qi, S.L.; Lin, L.; Pan, H.P.; Qin, Z.X.; Yu, T.J.; He, Z.K.; Zhang, G.Y. Junction Temperature and Reliability of High-Power Flip-Chip Light Emitting Diodes. Mater. Sci. Semicond. Process 2007, 10, 206–210. [Google Scholar] [CrossRef]
- Kim, M.M.; Giry, A.; Mastiani, M.; Rodrigues, G.O.; Reis, A.; Mandin, P. Microscale Thermometry: A Review. Microelectron. Eng. 2015, 148, 129–142. [Google Scholar] [CrossRef]
- Vertikov, A.; Kuball, M.; Nurmikko, A.V.; Maris, H.J. Time Resolved Pumpprobe Experiments With Subwavelength Lateral Resolution. Appl. Phys. Lett. 1996, 69, 2465–2467. [Google Scholar] [CrossRef]
- Cengiz, C.; Azarifar, M.; Arık, M. Thermal and Optical Characterization of White and Blue Multi-Chip LED Light Engines. In Proceedings of the InterSociety Conference on Thermal and Thermomechanical Phenomena in Electronic Systems, ITHERM, San Diego, CA, USA, 1–4 June 2021; pp. 285–293. [Google Scholar]
- Cheng, H.C.; Lin, J.Y.; Chen, W.H. On the Thermal Characterization of an RGB LED-Based White Light Module. Appl. Therm. Eng. 2012, 38, 105–116. [Google Scholar] [CrossRef]
- Chernyakov, A.E.; Bulashevich, K.A.; Karpov, S.Y.; Zakgeim, A.L. Experimental and Theoretical Study of Electrical, Thermal, and Optical Characteristics of InGaN/GaN High-power Flip-chip LEDs. Phys. Status Solidi 2013, 210, 466–469. [Google Scholar] [CrossRef]
- Katra, I.; Blumberg, D.G.; Lavee, H.; Sarah, P. Topsoil Moisture Patterns on Arid Hillsides—Micro-Scale Mapping by Thermal Infrared Images. J. Hydrol. 2007, 334, 359–367. [Google Scholar] [CrossRef]
- Varshni, Y.P. Temperature Dependence of the Energy Gap in Semiconductors. Physica 1967, 34, 149–154. [Google Scholar] [CrossRef]
- Ashdown, I.; Salsbury, M. Peak Wavelength Shifts and Opponent Color Theory. In Seventh International Conference on Solid State Lighting; SPIE: Bellingham, WA, USA, 2007; Volume 6669. [Google Scholar]
- Xi, Y.; Xi, J.Q.; Gessmann, T.; Shah, J.M.; Kim, J.K.; Schubert, E.F.; Fischer, A.J.; Crawford, M.H.; Bogart, K.H.A.; Allerman, A.A. Junction and Carrier Temperature Measurements in Deep-Ultraviolet Light-Emitting Diodes Using Three Different Methods. Appl. Phys. Lett. 2005, 86, 031907. [Google Scholar] [CrossRef]
- Arik, M.; Royce, C.; Kulkarni, K.S. Developing a Standard Measurement and Calculation Procedure for High Brightness LED Junction Temperature. In Proceedings of the Fourteenth Intersociety Conference on Thermal and Thermomechanical Phenomena in Electronic Systems (ITherm), Orlando, FL, USA, 27–30 May 2014; pp. 27–30. [Google Scholar]
- Xi, Y.; Gessmann, T.; Xi, J.; Kim, J.K.; Shah, J.M.; Fred Schubert, E.; Fischer, A.J.; Crawford, M.H.; Bogart, K.H.A.; Allerman, A.A. Junction Temperature in Ultraviolet Light-Emitting Diodes. Jpn. J. Appl. Phys. 2005, 44, 7260–7266. [Google Scholar] [CrossRef]
- Hong, E.; Narendran, N. A Method for Projecting Useful Life of LED Lighting Systems. In Third International Conference on Solid State Lighting; SPIE: Bellingham, WA, USA, 2004; Volume 5187, pp. 93–99. [Google Scholar]
- Wu, H.H.; Lin, K.H.; Lin, S.T. A Study on the Heat Dissipation of High Power Multi-Chip COB LEDs. Microelectron. J. 2012, 43, 280–287. [Google Scholar] [CrossRef]
- Shin, M.W.; Jang, S.H. Thermal Analysis of High Power LED Packages under the Alternating Current Operation. Solid State Electron. 2012, 68, 48–50. [Google Scholar] [CrossRef]
- Jayawardena, A.; Liu, Y.W.; Narendran, N. Analysis of Three Different Junction Temperature Estimation Methods for AC LEDs. Solid State Electron. 2013, 86, 11–16. [Google Scholar] [CrossRef]
- Wang, T.; Nakagawa, D.; Wang, J.; Sugahara, T.; Sakai, S. Photoluminescence Investigation of InGaN/GaN Single Quantum Well and Multiple Quantum Wells. Appl. Phys. Lett. 1998, 73, 3571–3573. [Google Scholar] [CrossRef]
- Wang, Y.; Pan, M.; Li, T. Comprehensive Study of Internal Quantum Efficiency of High-Brightness GaN-Based Light-Emitting Diodes by Temperature-Dependent Electroluminescence Method. In Proceedings of the Light-Emitting Diodes: Materials, Devices, and Applications for Solid State Lighting XVIII, San Francisco, CA, USA, 1–6 February 2014; Volume 9003. [Google Scholar]
- Hao, R.; Chen, L.; Wu, J.; Fan, D.; Wu, Y.; Liang, S. Effects of Growth Temperature Change in Quantum Well on Luminescence Performance and Optical Spectrum. Optik 2021, 235, 166606. [Google Scholar] [CrossRef]
- Kim, J.; Kim, S.; Kim, H.; Choi, W.J.; Jung, H. Effects of Carrier Leakage on Photoluminescence Properties of GaN-Based Light Emitting Diodes at Room Temperature. Curr. Opt. Photonics 2019, 3, 164–171. [Google Scholar]
- Li, J.; Chen, D.; Li, K.; Wang, Q.; Shi, M.; Cheng, C.; Leng, J. Carrier Dynamics in InGaN/GaN-Based Green LED under Different Excitation Sources. Crystals 2021, 11, 1061. [Google Scholar] [CrossRef]
- Takeuchi, T.; Sota, S.; Katsuragawa, M.; Komori, M.; Takeuchi, H.; Amano, H.; Akasaki, I. Quantum-Confined Stark Effect Due to Piezoelectric Fields in GaInN Strained Quantum Wells. Jpn. J. Appl. Phys. 1997, 36, L382–L385. [Google Scholar] [CrossRef]
- Zhu, L.H.; Du, W.J.; Huang, J.E.; Chen, H.T.; Gao, Y.L.; Gong, H.L.; Tong, C.D.; Wu, T.Z.; Guo, W.J.; Guo, Z.Q.; et al. 2-D Photo-Thermal Distribution and Structures of Flip-Chip Mini Light-Emitting Diodes by Microscopic Hyperspectral Imaging. IEEE Trans. Instrum. Meas. 2023, 72, 5009408. [Google Scholar] [CrossRef]
- Jin, J.; Gao, Y.; Ruan, Y.-J.; Gao, Y.-L.; Zhu, L.-H.; Guo, Z.-Q.; Lin, Y.; Chen, G.-L.; Chen, Z.; Lu, Y.-J. A Microscopic Hyperspectral-Based Centroid Wavelength Method for Measuring Two-Dimensional Junction Temperature Distribution of LEDs. IEEE Electron. Device Lett. 2019, 40, 506–509. [Google Scholar] [CrossRef]
- Lin, Y.; Xu, L.; Chen, L.; Liu, B.; Chen, G.; Lu, Y.; Liao, X.; Chen, Z.; Guo, Z. Influence of Phosphor Saturation on 2-D Temperature Measurement in Phosphor-Converted LEDs by Microscopic Hyperspectral Imaging. IEEE Trans. Electron. Devices 2023, 70, 2616–2621. [Google Scholar] [CrossRef]
- Ruppert, L.A.; Kalker, S.; De Doncker, R.W. Junction-temperature sensing of paralleled sic mosfets utilizing temperature sensitive optical parameters. In Proceedings of the 2021 IEEE Energy Conversion Congress and Exposition (ECCE), Vancouver, BC, Canada, 10–14 October 2021; pp. 5597–5604. [Google Scholar]
- Ruppert, L.A.; Wirsen, B.; Kalker, S.; De Doncker, R.W. Utilizing Electroluminescence of Silicon IGBTs for Junction Temperature Sensing. In Proceedings of the 2023 11th International Conference on Power Electronics and ECCE Asia (ICPE 2023-ECCE Asia), Jeju Island, Republic of Korea, 22–25 May 2023; pp. 3200–3205. [Google Scholar]
- Vermeersch, B.; De Mey, G. A shortcut to inverse Fourier transforms: Approximate reconstruction of transient heating curves from sparse frequency domain data. Int. J. Therm. Sci. 2010, 49, 1319–1332. [Google Scholar] [CrossRef]
- Vermeersch, B.; Christofferson, J.; Maize, K.; Shakouri, A.; De Mey, G. Time and frequency domain CCD-based thermoreflectance techniques for high-resolution transient thermal imaging. In Proceedings of the 2010 26th Annual IEEE Semiconductor Thermal Measurement and Management Symposium (SEMI-THERM), Santa Clara, CA, USA, 21–25 February 2010. [Google Scholar]
- Shieh, B.; Zeng, F.; Lee, S.R.; Yang, G. Measurement of Dynamic Junction Temperature for LED Flash Units of Camera. In Proceedings of the 2018 19th International Conference on Electronic Packaging Technology (ICEPT), Shanghai, China, 8–11 August 2018; pp. 1692–1695. [Google Scholar]
- Shieh, B.; Zeng, F.; Yang, G.; Zhao, F.; Sher, C.W.; Lee, S.R. Empirical Modeling and measurement of the Pulsed Junction Temperature of VCSEL. In Proceedings of the 2019 20th International Conference on Electronic Packaging Technology (ICEPT), Hong Kong, China, 12–15 August 2019; pp. 1–4. [Google Scholar]
- Hegedüs, J.; Hantos, G.; Lukács, M.; Bodnár, B.; Lipák, G.; Poppe, A. Lifetime test of pulse width modulated LEDs supplemented with thermal investigations. In IEEE Transactions on Components, Packaging and Manufacturing Technology; IEEE: Piscataway, NJ, USA, 2023. [Google Scholar]
- Mandrusiak, G.; She, X.; Waddell, A.M.; Acharya, S. On the transient thermal characteristics of silicon carbide power electronics modules. IEEE Trans. Power Electron. 2018, 33, 9783–9789. [Google Scholar] [CrossRef]
- Maize, K.; Shakouri, A. Transient thermal imaging using thermoreflectance. In Proceedings of the 2008 Twenty-Fourth Annual IEEE Semiconductor Thermal Measurement and Management Symposium, San Jose, CA, USA, 16–20 March 2008; pp. 55–58. [Google Scholar]
- Christofferson, J.; Ezzahri, Y.; Maize, K.; Shakouri, A. Transient thermal imaging of pulsed-operation superlattice micro-refrigerators. In Proceedings of the 25th IEEE Semitherm Symposium, San Jose, CA, USA, 15–19 March 2009; pp. 45–49. [Google Scholar]
- Kuball, M.; Hayes, J.M.; Uren, M.J.; Martin, I.; Birbeck, J.C.H.; Balmer, R.S.; Hughes, B.T. Measurement of temperature in active high-power AlGaN–GaN HFETs using Raman spectroscopy. IEEE Electron. Device Lett. 2002, 23, 7–9. [Google Scholar] [CrossRef]
- Rajasingam, S.; Pomeroy, J.W.; Kuball, M.; Uren, M.J.; Martin, T.; Herbert, D.C.; Hilton, K.P.; Balmer, R.S. Micro-Raman temperature measurements for electric field assessment in active AlGaN/GaNHFETs. IEEE Electron. Device Lett. 2004, 25, 456–458. [Google Scholar] [CrossRef]
- Kuball, M.; Rajasingam, S.; Sarua, A.; Uren, M.J.; Martin, T.; Hughes, B.T.; Hilton, K.P.; Balmer, R.S. Measurement of Temperature Distribution in Multifinger AlGaN/GaN Heterostructure Field-Effect Transistors Using Micro-Raman Spectroscopy. Appl. Phys. Lett. 2003, 82, 124–126. [Google Scholar] [CrossRef]
- Kuball, M.; Riedel, G.J.; Pomeroy, J.W.; Sarua, A.; Uren, M.J.; Martin, T.; Hilton, K.P.; Maclean, J.O.; Wallis, D.J. Time-Resolved Temperature Measurement of AlGaN/GaN Electronic Devices Using Micro-Raman Spectroscopy. IEEE Electron. Device Lett. 2007, 28, 86–89. [Google Scholar] [CrossRef]
- Das, R.S.; Agrawal, Y.K. Raman Spectroscopy: Recent Advancements, Techniques and Applications. Vib. Spectrosc. 2011, 57, 163–176. [Google Scholar] [CrossRef]
- Farzaneh, M.; Maize, K.; Lüeren, D.; Summers, J.A.; Mayer, P.M.; Raad, P.E.; Pipe, K.P.; Shakouri, A.; Ram, R.J.; Hudgings, J.A. CCD-Based Thermoreflectance Microscopy: Principles and Applications. J. Phys. D Appl. Phys. 2009, 42, 143001. [Google Scholar] [CrossRef]
- Ju, Y.S.; Goodson, K.E. Thermal Mapping of Interconnects Subjected to Brief Electrical Stresses. IEEE Electron. Device Lett. 1997, 18, 512–514. [Google Scholar] [CrossRef]
- Bahk, J.; Shakouri, A. Ultra-Fast Thermoreflectance Imaging for Electronic, Optoelectronic, and Thermal Devices. In Proceedings of the 2019 IEEE BiCMOS and Compound Semiconductor Integrated Circuits and Technology Symposium (BCICTS), Nashville, TN, USA, 3–6 November 2019; pp. 1–7. [Google Scholar]
- Katz, N.; Arango, A.; Hudgings, J. Mapping Temperature in OLED Displays Using CCD Thermoreflectance. IEEE Photonics Technol. Lett. 2013, 26, 194–197. [Google Scholar] [CrossRef]
- Kendig, D.; Yazawa, K.; Shakouri, A. Thermal Imaging of Encapsulated LEDs. In Proceedings of the 2011 27th Annual IEEE Semiconductor Thermal Measurement and Management Symposium, San Jose, CA, USA, 20–24 March 2011; pp. 310–313. [Google Scholar]
- Sarua, A.; Ji, H.; Kuball, M.; Uren, M.J.; Martin, T.; Hilton, K.P.; Balmer, R.S. Integrated micro-Raman/Infrared thermography probe for monitoring of self-heating in AlGaN/GaN transistor structures. IEEE Trans. Electron. Devices 2006, 3, 238–244. [Google Scholar] [CrossRef]
- Lin, Y.; Huang, J.E.; Gao, Y.; Gao, Y.L.; Chen, G.L.; Yang, C.; Li, Z.H.; Lei, S.D.; Ruan, Y.J.; Kang, P.C.; et al. Determining phosphor temperature in light-emitting diode based on divisional normalized emission power. IEEE Electron. Device Lett. 2019, 40, 1650–1653. [Google Scholar] [CrossRef]
- Tessier, G.; Pavageau, S.; Charlot, B.; Filloy, C.; Fournier, D.; Cretin, B.; Dilhaire, S.; Gomes, S.; Trannoy, N.; Vairac, P.; et al. Quantitative thermoreflectance imaging: Calibration method and validation on a dedicated integrated circuit. IEEE Trans. Compon. Packag. Technol. 2007, 30, 604–608. [Google Scholar] [CrossRef][Green Version]
- Meier, F.; Geyer, P.E.; Winter, S.V.; Cox, J.; Mann, M. BoxCar acquisition method enables single-shot proteomics at a depth of 10000 proteins in 100 minutes. Nat. Methods 2018, 15, 440–448. [Google Scholar] [CrossRef] [PubMed]
- Takuya, K.; Takashi, K.; Yuji, M. Multi-element hollow-core anti-resonant fiber for infrared thermal imaging. Opt. Exp. 2016, 24, 26565–26574. [Google Scholar]







| Measurement Method | Measurement Principle | Spatial Resolution | Advantages | Limitations |
|---|---|---|---|---|
| Micro-thermocouple | Seebeck effect | 50 μm | Low cost | Limited resolution |
| Readily available | Requires direct contact | |||
| Liquid Crystal Thermography (LCT) | Crystal phase transitions | 2–5 μm | Low cost | Not a direct indicator of the Tj |
| good spatial resolution | Temperature resolution is limited to the liquid crystal | |||
| Infrared (IR) Thermography | Planck blackbody emission | 3 μm | No contact | Typically limited spatial resolution |
| Provides temperature maps | Environmental influences are prone to errors | |||
| TSOP | Spectral Power Distribution | 2–3 μm | good spatial resolution | Expensive cost |
| No contact | The relationship between temperature and optical parameters needs to be measured | |||
| Heterodyne Method | cycled phase lag | 20 μm | No contact | Systematic errors cannot be corrected |
| Simple to implement | Limited resolution |
| Measurement Method | Measurement Principle | Temporal Resolution | Advantages | Limitations |
|---|---|---|---|---|
| T3ster | Electrical | >100 ns | High current | Slow acquisition time |
| PWM | Expensive cost | |||
| CRWM | Electrical | <200 ns | Error is less than FVM | One-dimensional drive measurements |
| Fast acquisition time | Measures the average Tj | |||
| Micro-Raman Spectroscopy | Phonon Frequency | >200 ns | Good spatial resolution | Slow acquisition time |
| No contact | May require an unobstructed view of the device | |||
| Thermal reflection | Reflectivity | 800 ps | No contact | Not direct indicator of the Tj |
| Good spatial resolution | May require an unobstructed view of the device | |||
| h-SCRLI | Reflectivity | Reach 68 μs at 14,600 fps | No contact | Not direct indicator of the Tj |
| Error is less than Thermal reflection | Acquisition time is determined by the camera | |||
| μ_HSTI | Reflectivity | 5 ns | Fast acquisition time | Not direct indicator of the Tj |
| No contact | Difficult to detect changes in the temperature distribution. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, X.; Gong, H.; Zhu, L.; Zheng, Z.; Lu, Y. LED Junction Temperature Measurement: From Steady State to Transient State. Sensors 2024, 24, 2974. https://doi.org/10.3390/s24102974
Zhao X, Gong H, Zhu L, Zheng Z, Lu Y. LED Junction Temperature Measurement: From Steady State to Transient State. Sensors. 2024; 24(10):2974. https://doi.org/10.3390/s24102974
Chicago/Turabian StyleZhao, Xinyu, Honglin Gong, Lihong Zhu, Zhenyao Zheng, and Yijun Lu. 2024. "LED Junction Temperature Measurement: From Steady State to Transient State" Sensors 24, no. 10: 2974. https://doi.org/10.3390/s24102974
APA StyleZhao, X., Gong, H., Zhu, L., Zheng, Z., & Lu, Y. (2024). LED Junction Temperature Measurement: From Steady State to Transient State. Sensors, 24(10), 2974. https://doi.org/10.3390/s24102974






