Multi-Channel Signals in Dynamic Force-Clamp Mode of Microcantilever Sensors for Detecting Cellular Peripheral Brush
Abstract
1. Introduction
2. Problem Formulation
2.1. Two-Domain Structure Model
2.2. Interpretation Models of Microcantilever Signal
3. Solution Methods
4. Results and Discussion
4.1. Model Verification
4.2. Peripheral Brush Effect on Cell Viscoelasticity
4.3. Peripheral Brush Effect on Quasi-Static Response of Microcantilever
4.4. Peripheral Brush Effect on Dynamic Response of Microcantilever
4.5. Limitations of Present Model and Future Developments
5. Conclusions
- (1)
- The complex modulus of the cell in the frequency domain, the quasi-static deflection/interaction force, and the higher frequency peak amplitude of the microcantilever enhance with the increase in brush density, segmental stiffness, and brush length. These results indicate the necessity of considering the influence of the peripheral brush in the establishment of the interpretation model of the microcantilever signal.
- (2)
- In AFM dynamic force-clamp mode, the quasi-static deflection/interaction force and higher frequency peak amplitude in double-peak resonance mode of the microcantilever can be used to obtain more indexes including brush density, segmental stiffness, and brush length for the diagnosis of cancerous cells.
- (3)
- However, the relevant AFM dynamic force-clamp experiments need to be further explored to verify the utilization of multi-channel signals with more indexes to identify cancerous cells.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Iyer, S.; Gaikwad, R.M.; Subba-Rao, V.; Woodworth, C.D.; Sokolov, I. Atomic force microscopy detects differences in the surface brush of normal and cancerous cells. Nat. Nanotechnol. 2009, 4, 389–393. [Google Scholar] [CrossRef] [PubMed]
- Wegmann, S.; Medalsy, I.D.; Mandelkow, E.; Müller, D.J. The fuzzy coat of pathological human Tau fibrils is a two-layered polyelectrolyte brush. Proc. Natl. Acad. Sci. USA 2012, 110, E313–E321. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Li, W.; Song, L.; Zhu, W. Cilia, adenomatous polyposis coli and associated diseases. Oncogene 2011, 31, 1475–1483. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Makarova, N.; Sokolov, I. Cell mechanics can be robustly derived from AFM indentation data using the brush model: Error analysis. Nanoscale 2022, 14, 4334–4347. [Google Scholar] [CrossRef] [PubMed]
- Dufrêne, Y.F.; Ando, T.; Garcia, R.; Alsteens, D.; Martinez-Martin, D.; Engel, A.; Gerber, C.; Müller, D.J. Imaging modes of atomic force microscopy for application in molecular and cell biology. Nat. Nanotechnol. 2017, 12, 295–307. [Google Scholar] [CrossRef]
- Benaglia, S.; Gisbert, V.G.; Perrino, A.P.; Amo, C.A.; Garcia, R. Fast and high-resolution mapping of elastic properties of biomolecules and polymers with bimodal AFM. Nat. Protoc. 2018, 13, 2890–2907. [Google Scholar] [CrossRef]
- Stewart, S.; Ou, W.; Aranda-Espinoza, H.; Rahaman, S.O.; He, X. Micromechanical characterizations and viscoelastic modeling reveal elastic and viscoelastic heterogeneities in ovarian tissue and the significant viscoelastic contribution to the apparent elastic modulus determined by AFM indentation. Acta Biomater. 2023, 168, 286–297. [Google Scholar] [CrossRef]
- Hadzipasic, M.; Zhang, S.; Huang, Z.; Passaro, R.; Sten, M.S.; Shankar, G.M.; Nia, H.T. Emergence of nanoscale viscoelasticity from single cancer cells to established tumors. Biomaterials 2024, 305, 122431. [Google Scholar] [CrossRef]
- Carvalho, E.M.; Kumar, S. Lose the stress: Viscoelastic materials for cell engineering. Acta Biomater. 2023, 163, 146–157. [Google Scholar] [CrossRef]
- Hang, J.T.; Kang, Y.; Xu, G.K.; Gao, H. A hierarchical cellular structural model to unravel the universal power-law rheological behavior of living cells. Nat. Commun. 2021, 12, 6067. [Google Scholar] [CrossRef]
- Efremov, Y.M.; Shpichka, A.I.; Kotova, S.L.; Timashev, P.S. Viscoelastic mapping of cells based on fast force volume and PeakForce Tapping. Soft Matter 2019, 15, 5455–5463. [Google Scholar] [CrossRef] [PubMed]
- Efremov, Y.M.; Wang, W.-H.; Hardy, S.D.; Geahlen, R.L.; Raman, A. Measuring nanoscale viscoelastic parameters of cells directly from AFM force-displacement curves. Sci. Rep. 2017, 7, 1541. [Google Scholar] [CrossRef] [PubMed]
- Ding, Y.; Xu, G.-K.; Wang, G.-F. On the determination of elastic moduli of cells by AFM based indentation. Sci. Rep. 2017, 7, 45575. [Google Scholar] [CrossRef] [PubMed]
- Starodubtseva, M.N.; Shkliarava, N.M.; Chelnokova, I.A.; Villalba, M.I.; Krylov, A.Y.; Nadyrov, E.A.; Kasas, S. Mechanical properties and nanomotion of BT-20 and ZR-75 breast cancer cells studied by atomic force microscopy and optical nanomotion detection method. Cells 2023, 12, 2362. [Google Scholar] [CrossRef] [PubMed]
- Efremov, Y.M.; Okajima, T.; Raman, A. Measuring viscoelasticity of soft biological samples using atomic force microscopy. Soft Matter 2020, 16, 64–81. [Google Scholar] [CrossRef] [PubMed]
- Efremov, Y.M.; Cartagena-Rivera, A.X.; Athamneh, A.I.M.; Suter, D.M.; Raman, A. Mapping heterogeneity of cellular mechanics by multi-harmonic atomic force microscopy. Nat. Protoc. 2018, 13, 2200–2216. [Google Scholar] [CrossRef]
- Smolyakov, G.; Cauquil, M.; Severac, C.; Lachaize, V.; Guilbeau-Frugier, C.; Sénard, J.-M.; Galés, C.; Dague, E. Biophysical properties of cardiomyocyte surface explored by multiparametric AFM. J. Struct. Biol. 2017, 198, 28–37. [Google Scholar] [CrossRef] [PubMed]
- Fernandez, J.M.; Li, H. Force-clamp spectroscopy monitors the folding trajectory of a single protein. Science 2004, 303, 1674–1678. [Google Scholar] [CrossRef]
- Denisov, N.N.; Shcheglova, L.A.; Sekatskii, S.K.; Dietler, G.; Nadtochenko, V.A. Single-molecule force spectroscopy of a protein globule covalently bound to a calcite surface. Russ. J. Phys. Chem. B+. 2008, 2, 350–353. [Google Scholar] [CrossRef]
- Benedetti, F.; Gazizova, Y.; Kulik, A.J.; Marszalek, P.E.; Klinov, D.V.; Dietler, G.; Sekatskii, S.K. Can dissipative properties of single molecules be extracted from a force spectroscopy experiment? Biophys. J. 2016, 111, 1163–1172. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Lyu, Q.; Zhang, N.-H.; Wu, J.-Z.; Ding, H. Double-peak resonant mapping of cellular viscoelasticity in force-clamp detection of atomic force microscope. J. Sound Vib. 2022, 527, 116869. [Google Scholar] [CrossRef]
- Bu, Y.; Li, L.; Wang, J. Power law creep and relaxation with the atomic force microscope: Determining viscoelastic property of living cells. Sci. China. Technol. Sci. 2019, 62, 781–786. [Google Scholar] [CrossRef]
- Chang, Z.; Zhang, L.; Hang, J.T.; Liu, W.; Xu, G.K. Viscoelastic multiscale mechanical indexes for assessing liver fibrosis and treatment outcomes. Nano Lett. 2023, 23, 9618–9625. [Google Scholar] [CrossRef] [PubMed]
- Li, R.; Bu, Y.; Yang, C.; Wang, J. Effects of lipid deposition on viscoelastic response in human hepatic cell line HepG2. Front. Physiol. 2021, 12, 684121. [Google Scholar] [CrossRef] [PubMed]
- Rodríguez-Nieto, M.; Mendoza-Flores, P.; García-Ortiz, D.; Montes-de-Oca, L.M.; Mendoza-Villa, M.; Barrón-González, P.; Espinosa, G.; Menchaca, J.L. Viscoelastic properties of doxorubicin-treated HT-29 cancer cells by atomic force microscopy: The fractional Zener model as an optimal viscoelastic model for cells. Biomech. Model. Mechan. 2019, 19, 801–813. [Google Scholar] [CrossRef]
- Schillers, H.; Medalsy, I.; Hu, S.; Slade, A.L.; Shaw, J.E. PeakForce tapping resolves individual microvilli on living cells. J. Mol. Recognit. 2015, 29, 95–101. [Google Scholar] [CrossRef]
- Sokolov, I.; Dokukin, M.E.; Guz, N.V. Method for quantitative measurements of the elastic modulus of biological cells in AFM indentation experiments. Methods 2013, 60, 202–213. [Google Scholar] [CrossRef] [PubMed]
- Chtcheglova, L.A.; Shubeita, G.T.; Sekatskii, S.K.; Dietler, G. Force spectroscopy with a small dithering of AFM tip: A method of direct and continuous measurement of the spring constant of single molecules and molecular complexes. Biophys. J. 2004, 86, 1177–1184. [Google Scholar] [CrossRef][Green Version]
- Khurana, S.; George, S.P. The role of actin bundling proteins in the assembly of filopodia in epithelial cells. Cell Adhesi. Migr. 2014, 5, 409–420. [Google Scholar] [CrossRef]
- Darling, E.M.; Zauscher, S.; Block, J.A.; Guilak, F. A thin-layer model for viscoelastic, stress-relaxation testing of cells using atomic force microscopy: Do cell properties reflect metastatic potential? Biophys. J. 2007, 92, 1784–1791. [Google Scholar] [CrossRef]
- Dokukin, M.; Ablaeva, Y.; Kalaparthi, V.; Seluanov, A.; Gorbunova, V.; Sokolov, I. Pericellular brush and mechanics of guinea pig fibroblast cells studied with AFM. Biophys. J. 2016, 111, 236–246. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Zhang, N.H.; Xing, J.J. Vibration analysis of linear coupled thermoviscoelastic thin plates by a variational approach. Int. J. Solids Struct. 2008, 45, 2583–2597. [Google Scholar] [CrossRef][Green Version]
- Xiao, H. DNA elastic nonlinearities as multiple smooth combinations of soft and hard linear springs. ZAMM Z. Angew. Math. Mech. 2012, 93, 50–56. [Google Scholar] [CrossRef]
- Hardij, J.; Cecchet, F.; Berquand, A.; Gheldof, D.; Chatelain, C.; Mullier, F.; Chatelain, B.; Dogné, J.M. Characterization of tissue factor-bearing extracellular vesicles with AFM: Comparison of air-tapping-mode AFM and liquid Peak Force AFM. J. Extracell. Vesicles 2013, 2, 21045. [Google Scholar] [CrossRef] [PubMed]
- Moreno-Flores, S.; Benitez, R.; Vivanco, M.d.; Toca-Herrera, J.L. Stress relaxation and creep on living cells with the atomic force microscope: A means to calculate elastic moduli and viscosities of cell components. Nanotechnology 2010, 21, 445101. [Google Scholar] [CrossRef]
- Lyu, Q.; Li, J.J.; Zhang, N.H. Quasi-static and dynamical analyses of a thermoviscoelastic Timoshenko beam using the differential quadrature method. Appl. Math. Mech.-Engl. Ed. 2019, 40, 549–562. [Google Scholar] [CrossRef]
- Zhang, N.H.; Cheng, C.J. A time domain method for quasi-static analysis of viscoelastic thin plates. Appl. Math. Mech. 2001, 22, 1001–1008. [Google Scholar]
- Lyu, Q.; Zhang, N.H.; Zhang, C.Y.; Wu, J.Z.; Zhang, Y.C. Effect of adsorbate viscoelasticity on dynamical responses of laminated microcantilever resonators. Compos. Struct. 2020, 250, 112553. [Google Scholar] [CrossRef]
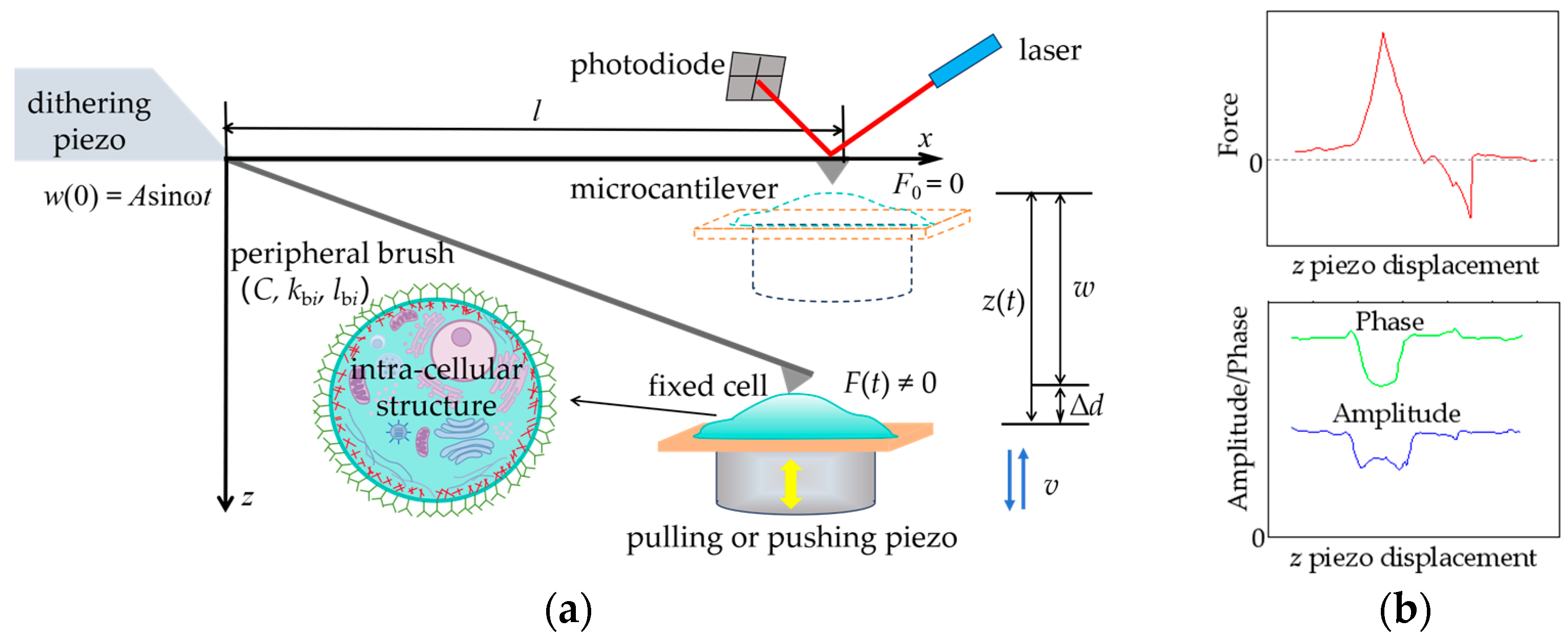
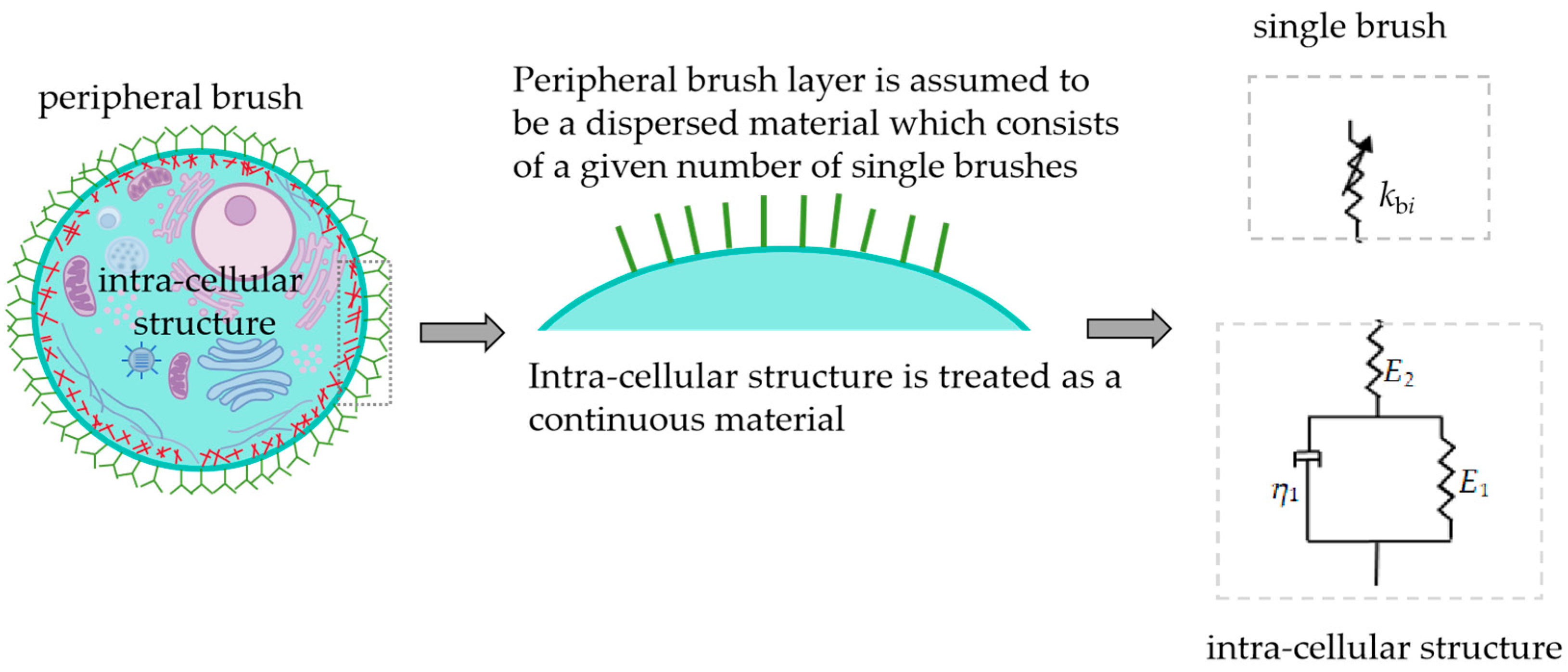

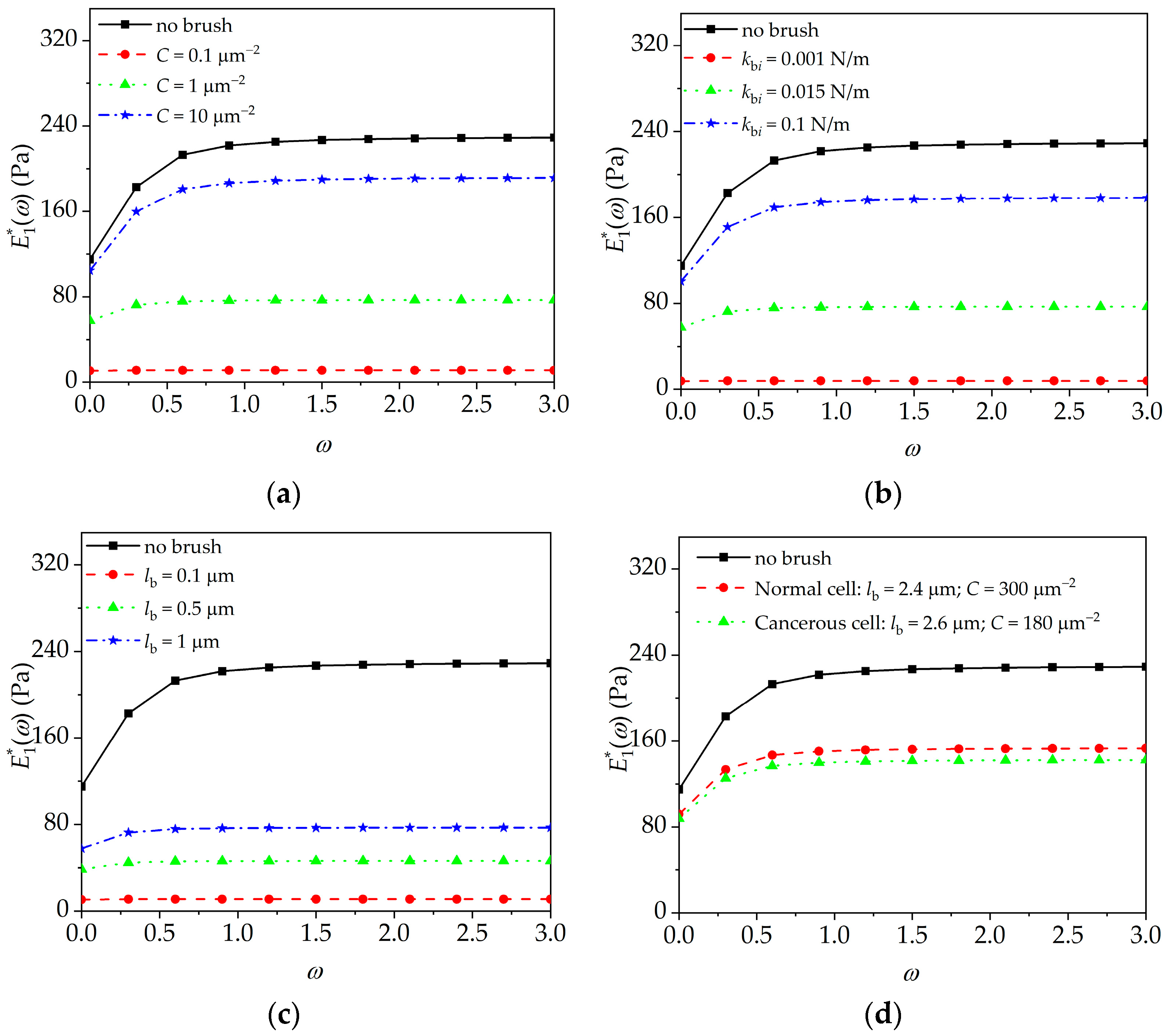
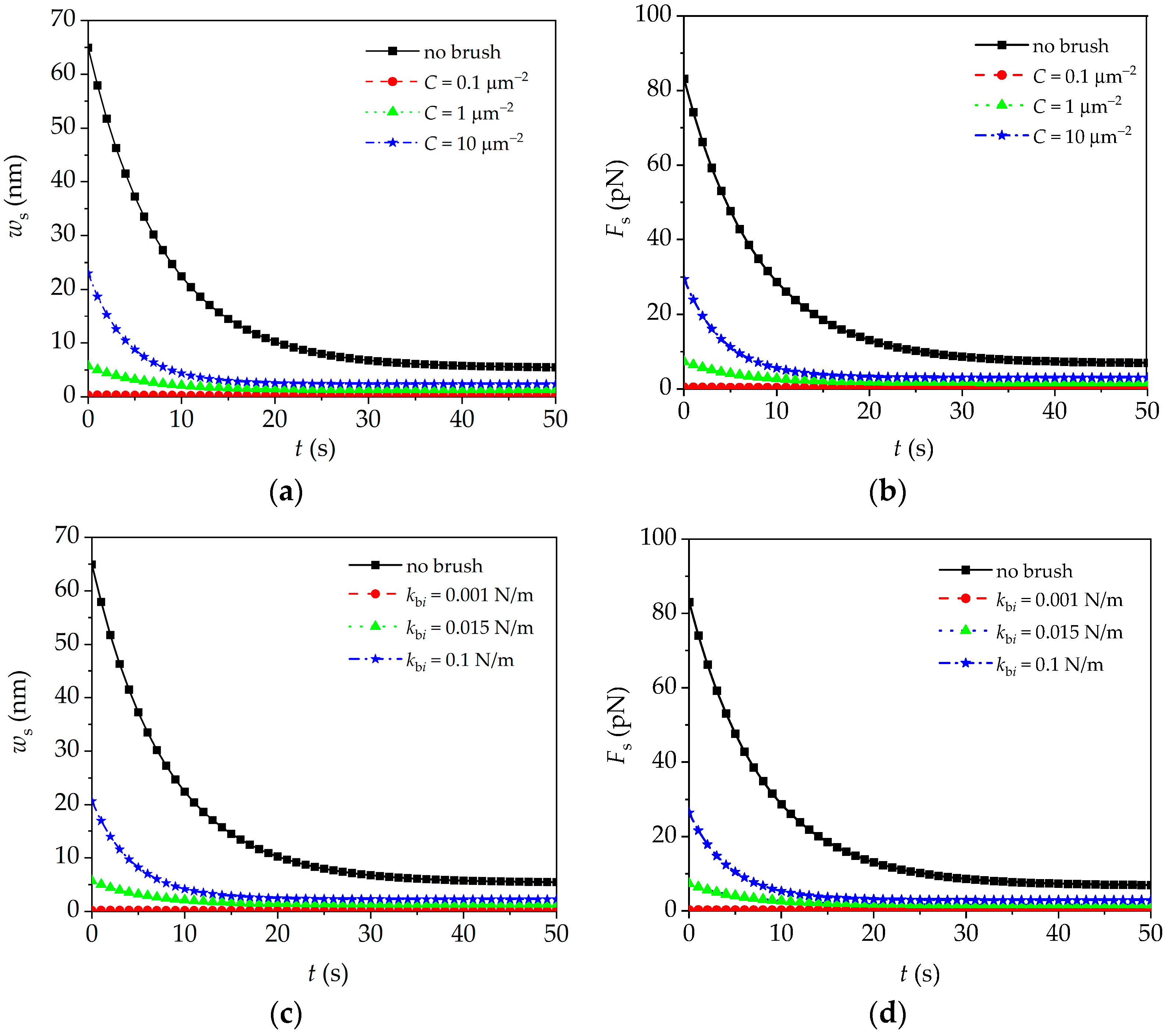



| Cellular Parameters | Darling [30] | SLS (No Brush) [21] | Present Model |
|---|---|---|---|
| e0i (Pa) | 230 ± 150 | 26.33 ± 1.67 × 103 | 185.65 ± 61.2 |
| e1i (Pa) | 210 ± 130 | 41.59 ± 3.3 × 103 | 21.05 ± 10.9 |
| e0i + e1i (Pa) | 440 ± 270 | 206.7 ± 72.1 | |
| αi (s−1) | 0.125 | 1.491 ± 0.467 | 0.474 |
| Ebi (Pa) | 56.7 ± 3.8 | ||
| R2 | 0.875 | 0.927 | 0.958 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lyu, Q.; Pei, F.; Zhao, Y.-L.; Ling, J.-W.; Zhang, N.-H. Multi-Channel Signals in Dynamic Force-Clamp Mode of Microcantilever Sensors for Detecting Cellular Peripheral Brush. Sensors 2024, 24, 6312. https://doi.org/10.3390/s24196312
Lyu Q, Pei F, Zhao Y-L, Ling J-W, Zhang N-H. Multi-Channel Signals in Dynamic Force-Clamp Mode of Microcantilever Sensors for Detecting Cellular Peripheral Brush. Sensors. 2024; 24(19):6312. https://doi.org/10.3390/s24196312
Chicago/Turabian StyleLyu, Qiang, Fan Pei, Ying-Long Zhao, Jia-Wei Ling, and Neng-Hui Zhang. 2024. "Multi-Channel Signals in Dynamic Force-Clamp Mode of Microcantilever Sensors for Detecting Cellular Peripheral Brush" Sensors 24, no. 19: 6312. https://doi.org/10.3390/s24196312
APA StyleLyu, Q., Pei, F., Zhao, Y.-L., Ling, J.-W., & Zhang, N.-H. (2024). Multi-Channel Signals in Dynamic Force-Clamp Mode of Microcantilever Sensors for Detecting Cellular Peripheral Brush. Sensors, 24(19), 6312. https://doi.org/10.3390/s24196312







