Principle and Applications of Thermoelectric Generators: A Review
Abstract
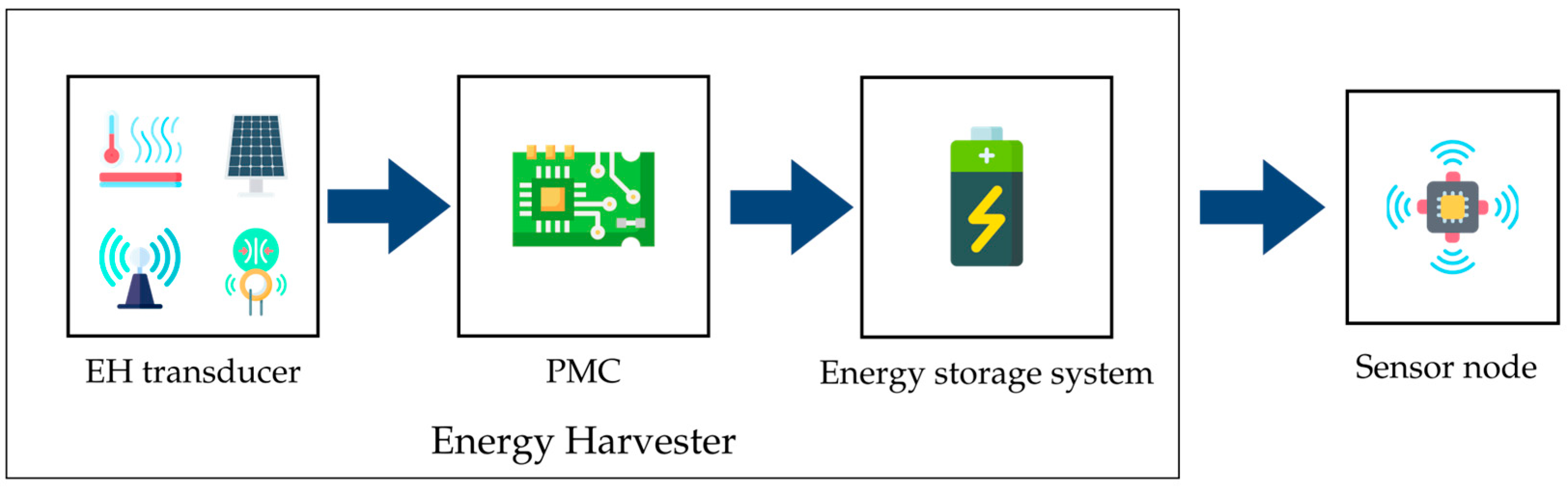
:1. Introduction
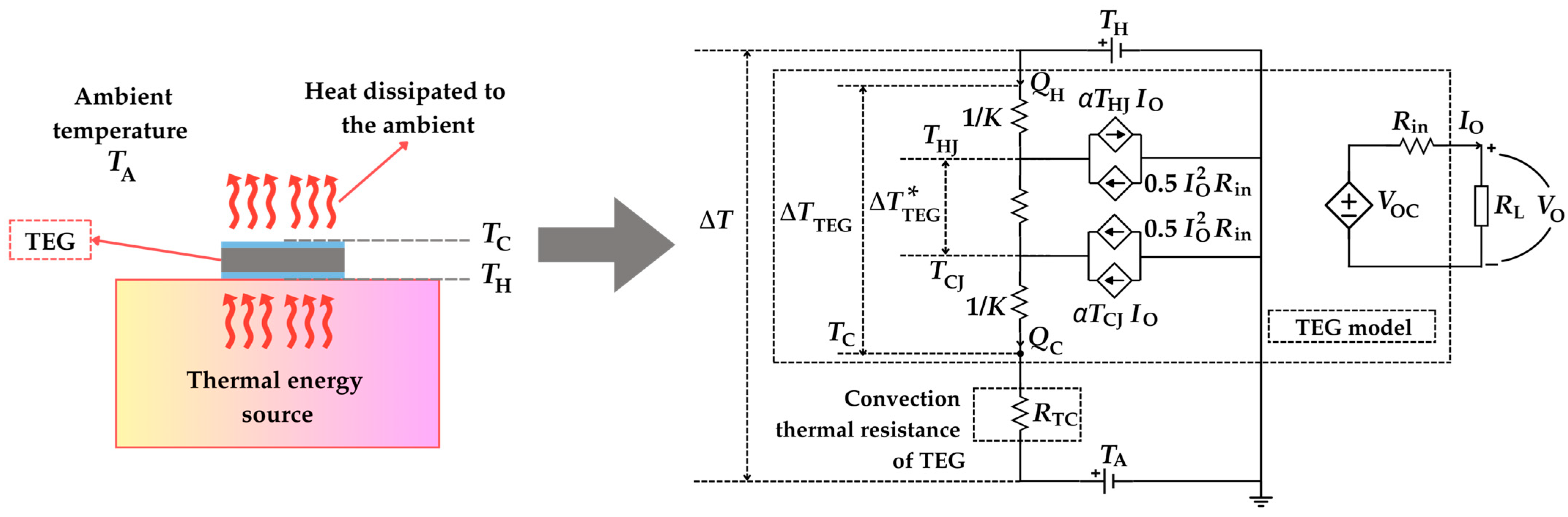
2. Operating Principle of a Thermoelectric Generator
2.1. Single-Thermocouple TEG
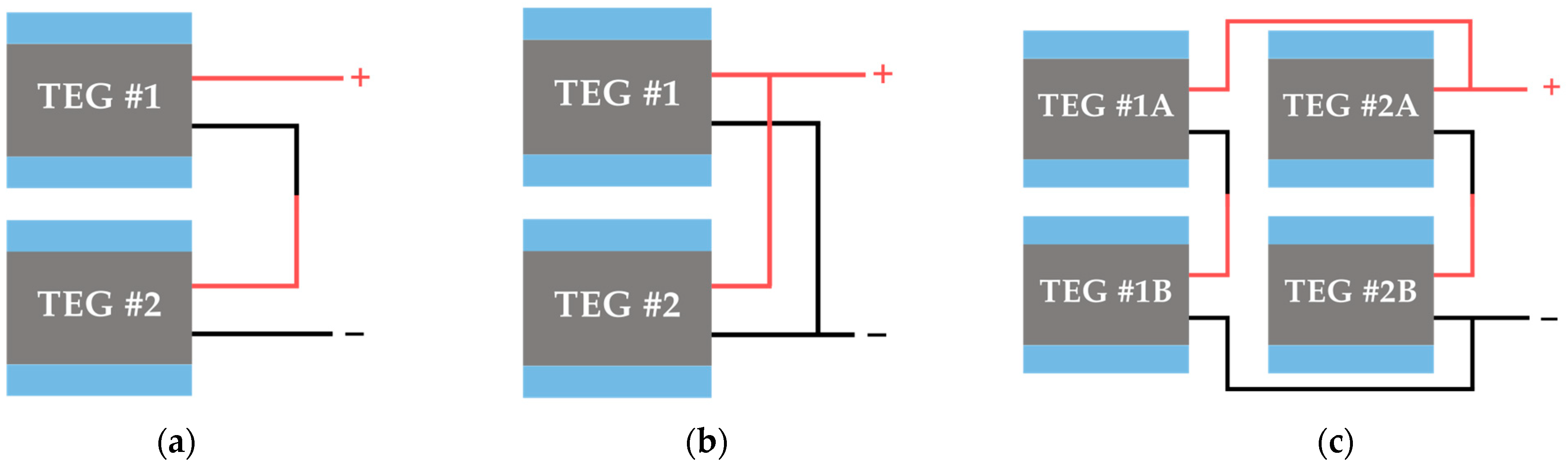
2.2. N-Thermocouple TEG
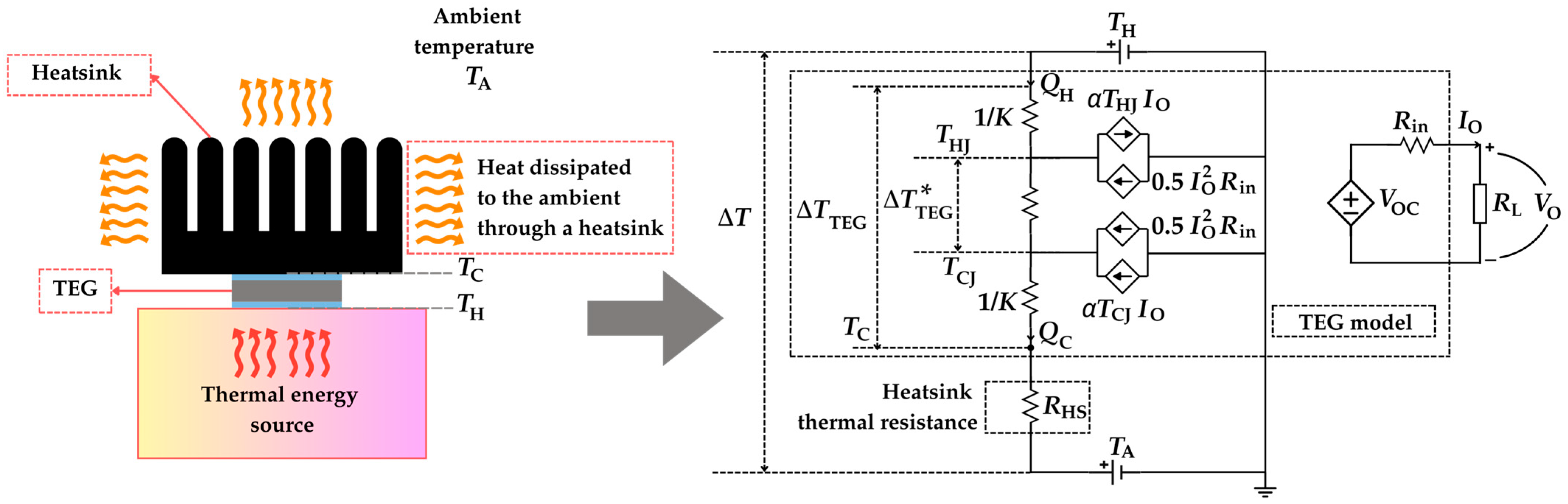
2.3. Enhancing TEG Performance
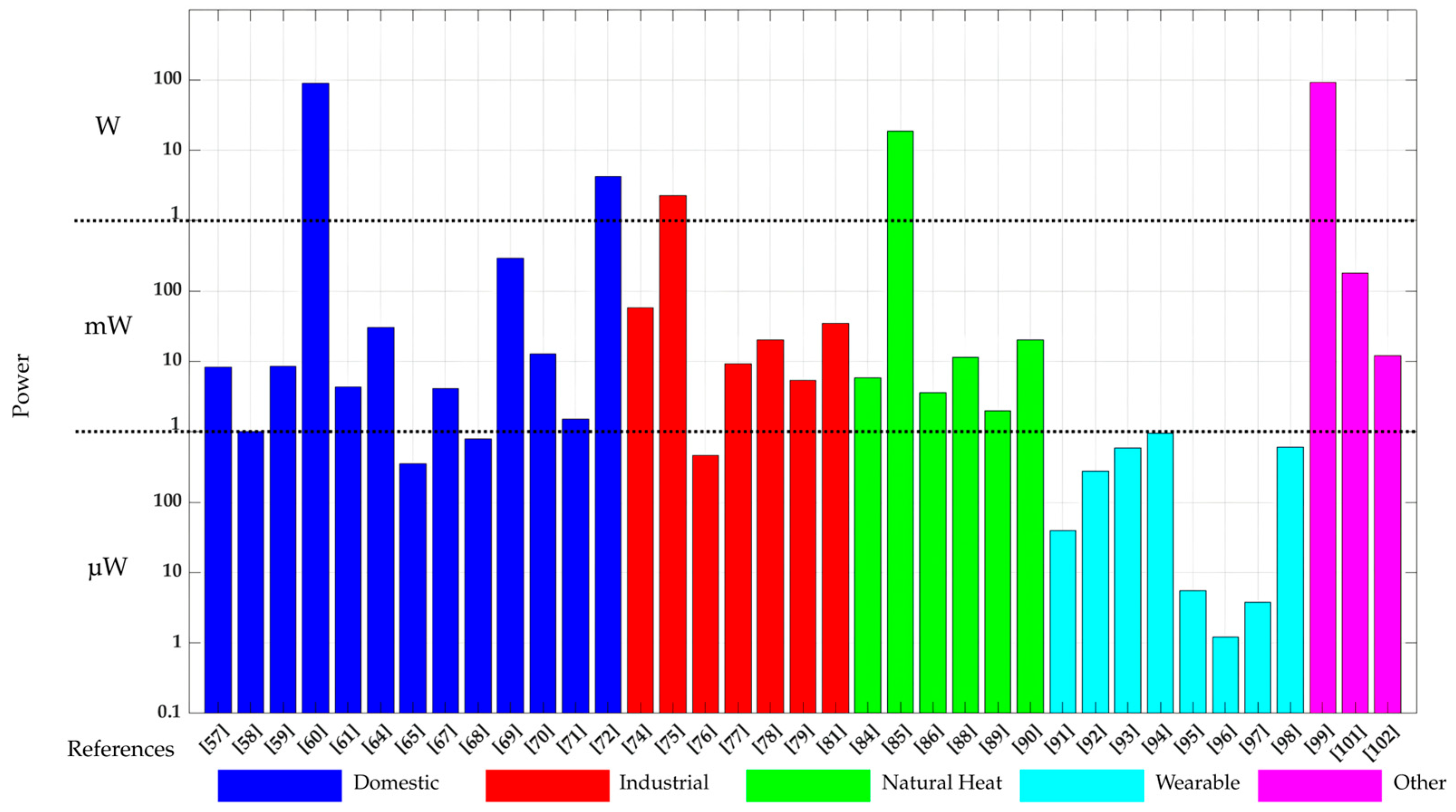
3. TEG Applications
3.1. Domestic Applications
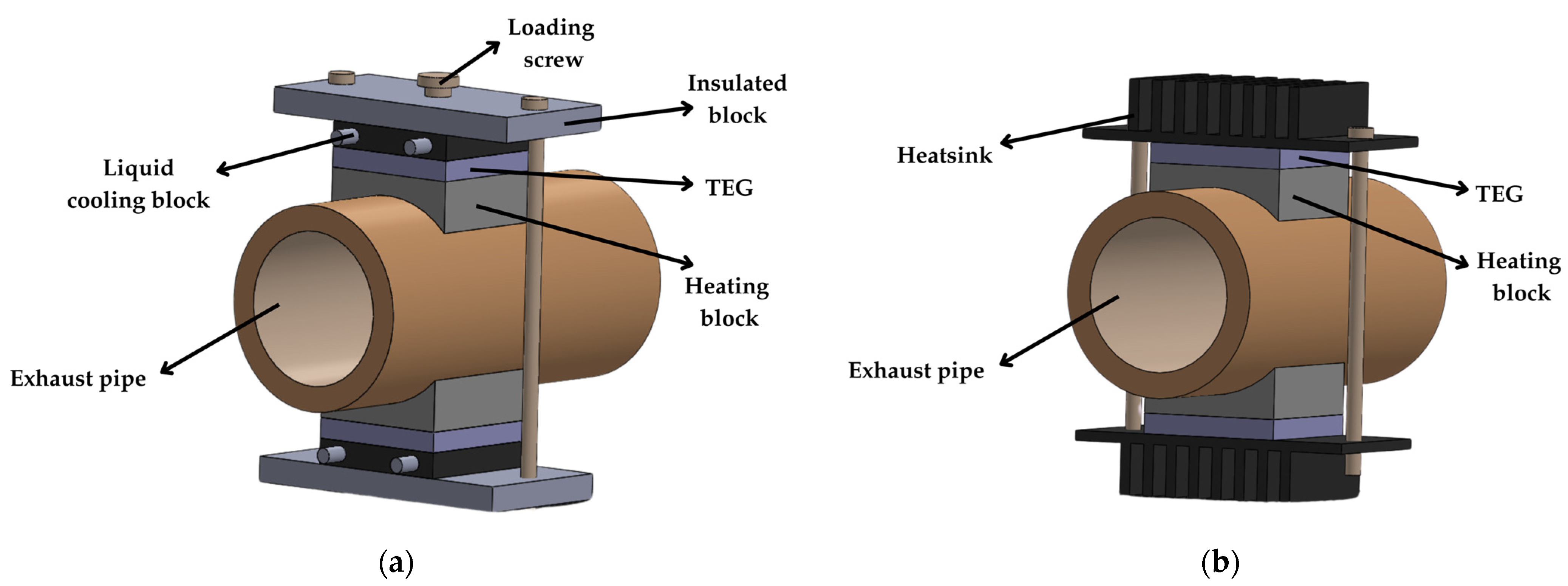
3.2. Industrial Applications
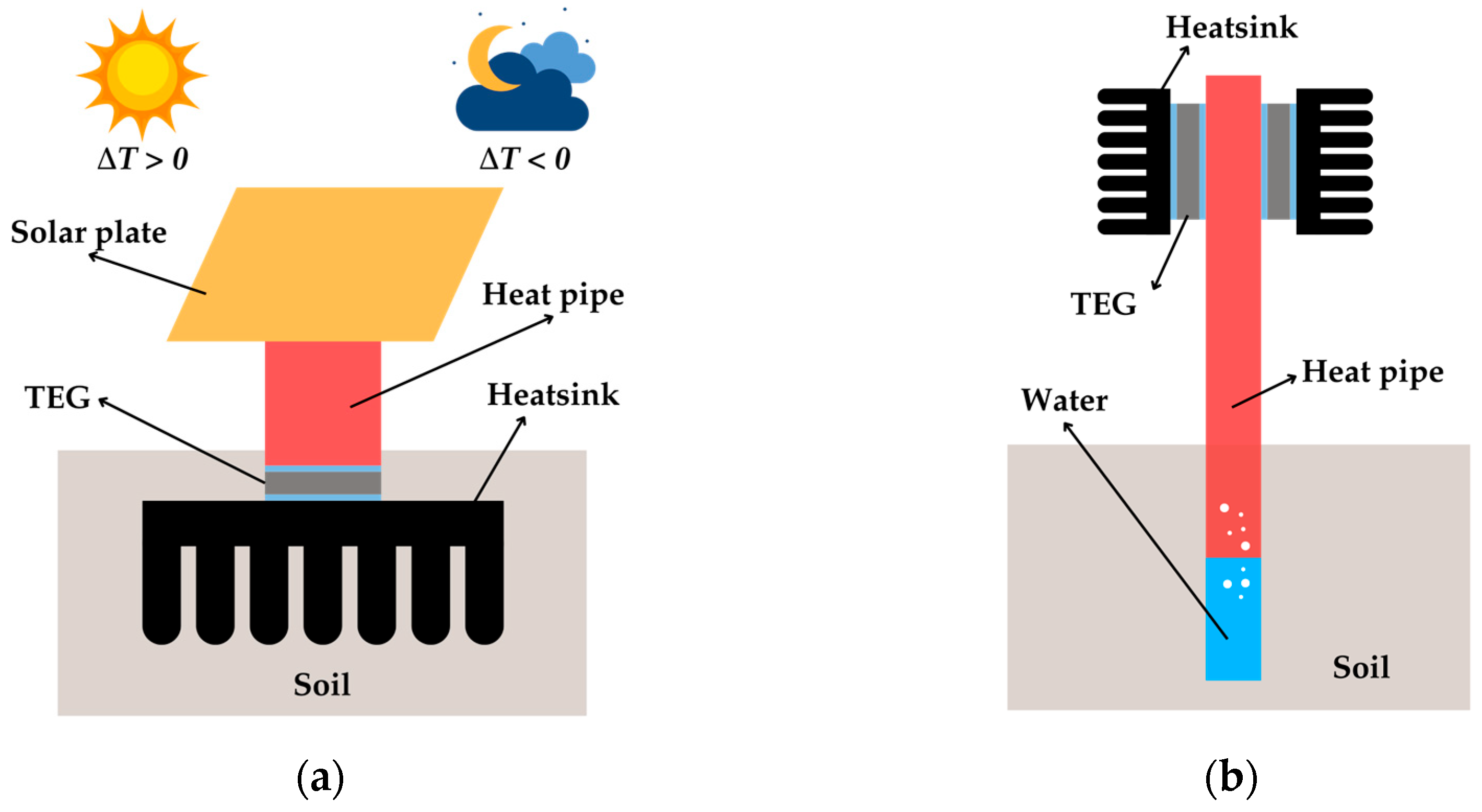
3.3. Natural Heat Applications
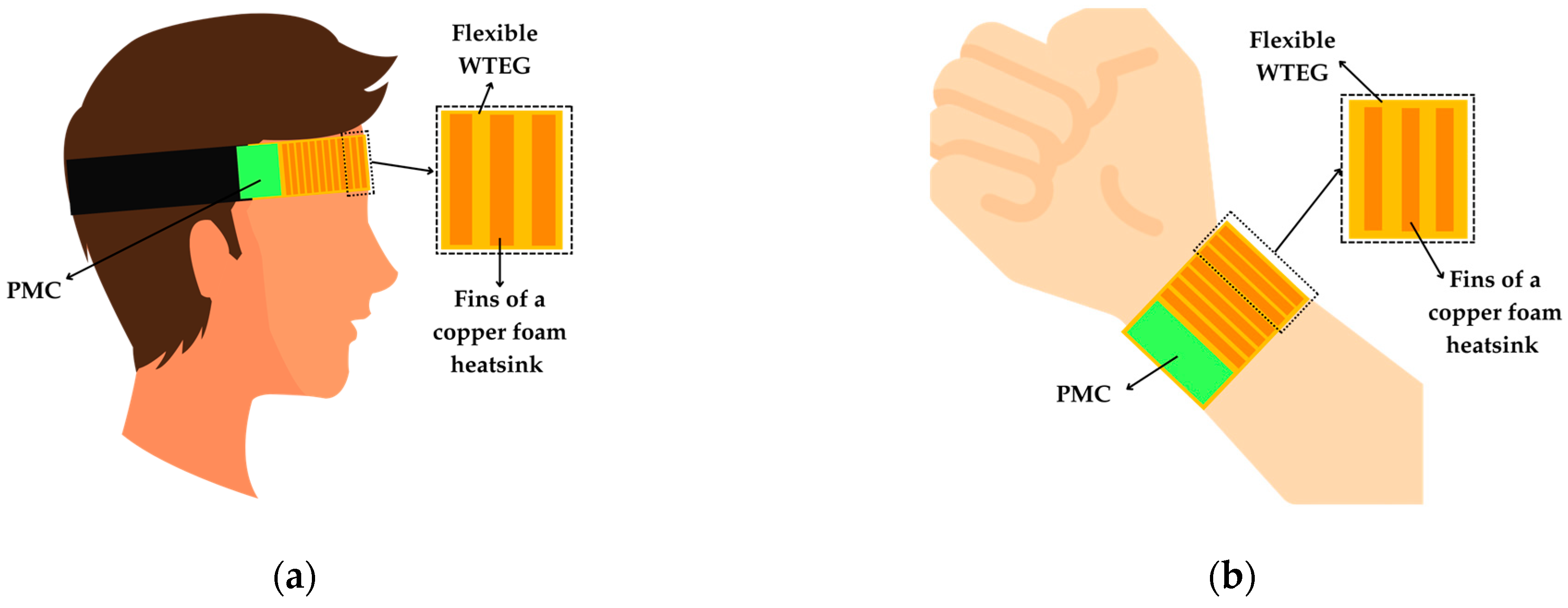
3.4. Wearable Applications
3.5. Other Applications
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Khorov, E.; Lyakhov, A.; Krotov, A.; Guschin, A. A Survey on IEEE 802.11ah: An Enabling Networking Technology for Smart Cities. Comput. Commun. 2015, 58, 53–69. [Google Scholar] [CrossRef]
- Majid, M.; Habib, S.; Javed, A.R.; Rizwan, M.; Srivastava, G.; Gadekallu, T.R.; Lin, J.C.-W. Applications of Wireless Sensor Networks and Internet of Things Frameworks in the Industry Revolution 4.0: A Systematic Literature Review. Sensors 2022, 22, 2087. [Google Scholar] [CrossRef] [PubMed]
- Lu, Y.; Papagiannidis, S.; Alamanos, E. Internet of Things: A Systematic Review of the Business Literature from the User and Organisational Perspectives. Technol. Forecast. Soc. Change 2018, 136, 285–297. [Google Scholar] [CrossRef]
- Schütze, A.; Helwig, N.; Schneider, T. Sensors 4.0—Smart Sensors and Measurement Technology Enable Industry 4.0. J. Sens. Sens. Syst. 2018, 7, 359–371. [Google Scholar] [CrossRef]
- Hidalgo-Leon, R.; Urquizo, J.; Silva, C.E.; Silva-Leon, J.; Wu, J.; Singh, P.; Soriano, G. Powering Nodes of Wireless Sensor Networks with Energy Harvesters for Intelligent Buildings: A Review. Energy Rep. 2022, 8, 3809–3826. [Google Scholar] [CrossRef]
- Famitafreshi, G.; Afaqui, M.S.; Melià-Seguí, J. A Comprehensive Review on Energy Harvesting Integration in IoT Systems from MAC Layer Perspective: Challenges and Opportunities. Sensors 2021, 21, 3097. [Google Scholar] [CrossRef]
- Wu, Z.; Cheng, T.; Wang, Z.L. Self-Powered Sensors and Systems Based on Nanogenerators. Sensors 2020, 20, 2925. [Google Scholar] [CrossRef]
- Xiao, H.; Qi, N.; Yin, Y.; Yu, S.; Sun, X.; Xuan, G.; Liu, J.; Xiao, S.; Li, Y.; Li, Y. Investigation of Self-Powered IoT Sensor Nodes for Harvesting Hybrid Indoor Ambient Light and Heat Energy. Sensors 2023, 23, 3796. [Google Scholar] [CrossRef]
- Liu, S.-Z.; Guo, W.-T.; Zhao, X.-H.; Tang, X.-G.; Sun, Q.-J. Self-Powered Sensing for Health Monitoring and Robotics. Soft Sci. 2025, 5, 14. [Google Scholar] [CrossRef]
- Meng, X.; Suh, I.-Y.; Xiao, X.; Pang, F.; Jeon, J.; Cho, D.S.; Kwon, Y.H.; Kim, S.-W. Triboelectric and Piezoelectric Technologies for Self-Powered Microbial Disinfection. Nano Energy 2024, 127, 109716. [Google Scholar] [CrossRef]
- Niu, Y.; Zeng, J.; Liu, X.; Li, J.; Wang, Q.; Li, H.; Rooij, N.F.D.; Wang, Y.; Zhou, G. A Photovoltaic Self-Powered Gas Sensor Based on All-Dry Transferred MoS2 /GaSe Heterojunction for ppb-Level NO2 Sensing at Room Temperature. Adv. Sci. 2021, 8, 2100472. [Google Scholar] [CrossRef]
- Liu, X.; Li, M.; Zhang, Y.; Zhu, X.; Guan, Y. Self-Powered Wireless Sensor Node Based on RF Energy Harvesting and Management Combined Design. Energy Convers. Manag. 2023, 292, 117393. [Google Scholar] [CrossRef]
- Azlor, M.; Martinez, B.; Gasulla, M.; Reverter, F. Study of the Applicability and Limitations of the FOCV Technique for Indoor Low-Area PV Cells. IEEE Trans. Instrum. Meas. 2025, 74, 2001409. [Google Scholar] [CrossRef]
- Chatterjee, A.; Lobato, C.N.; Zhang, H.; Bergne, A.; Esposito, V.; Yun, S.; Insinga, A.R.; Christensen, D.V.; Imbaquingo, C.; Bjørk, R.; et al. Powering Internet-of-Things from Ambient Energy: A Review. J. Phys. Energy 2023, 5, 022001. [Google Scholar] [CrossRef]
- Reverter, F. Toward Non-CPU Activity in Low-Power MCU-Based Measurement Systems. IEEE Trans. Instrum. Meas. 2020, 69, 15–17. [Google Scholar] [CrossRef]
- Ripoll-Vercellone, E.; Gasulla, M.; Reverter, F. Electronic Reading of a Mechanical Gas Meter Based on Dual Magnetic Sensing. Meas. Sci. Technol. 2021, 32, 097001. [Google Scholar] [CrossRef]
- Mathews, I.; Kantareddy, S.N.; Buonassisi, T.; Peters, I.M. Technology and Market Perspective for Indoor Photovoltaic Cells. Joule 2019, 3, 1415–1426. [Google Scholar] [CrossRef]
- Knight, C.; Davidson, J.; Behrens, S. Energy Options for Wireless Sensor Nodes. Sensors 2008, 8, 8037–8066. [Google Scholar] [CrossRef]
- Reverter, F. A Tutorial on Mechanical Sensors in the 70th Anniversary of the Piezoresistive Effect. Sensors 2024, 24, 3690. [Google Scholar] [CrossRef]
- Ferre, E.; Gasulla, M.; Reverter, F. Comparative Analysis of Low-Power PV Cells of Different Technologies under Different Types of Indoor Artificial Lighting. In Proceedings of the 2024 IEEE International Instrumentation and Measurement Technology Conference (I2MTC), Glasgow, UK, 20 May 2024; pp. 1–5. [Google Scholar]
- Reverter, F.; Gasulla, M. Optimal Inductor Current in Boost DC/DC Converters Regulating the Input Voltage Applied to Low-Power Photovoltaic Modules. IEEE Trans. Power Electron. 2017, 32, 6188–6196. [Google Scholar] [CrossRef]
- Owczarczak, R. Indoor Thermal Energy Harvesting for Battery-Free IoT Building Applications. Electrotech. Rev. 2023, 1, 134–138. [Google Scholar] [CrossRef]
- Covaci, C.; Gontean, A. Piezoelectric Energy Harvesting Solutions: A Review. Sensors 2020, 20, 3512. [Google Scholar] [CrossRef] [PubMed]
- Gasulla, M.; Ripoll-Vercellone, E.; Reverter, F. A Compact Thévenin Model for a Rectenna and Its Application to an RF Harvester with MPPT. Sensors 2019, 19, 1641. [Google Scholar] [CrossRef] [PubMed]
- Cuadras, A.; Gasulla, M.; Ferrari, V. Thermal Energy Harvesting through Pyroelectricity. Sens. Actuators A Phys. 2010, 158, 132–139. [Google Scholar] [CrossRef]
- Halim, M.A.; Rantz, R.; Zhang, Q.; Gu, L.; Yang, K.; Roundy, S. An Electromagnetic Rotational Energy Harvester Using Sprung Eccentric Rotor, Driven by Pseudo-Walking Motion. Appl. Energy 2018, 217, 66–74. [Google Scholar] [CrossRef]
- Zhang, Y.; Cao, J.; Liao, W.-H.; Zhao, L.; Lin, J. Theoretical Modeling and Experimental Verification of Circular Halbach Electromagnetic Energy Harvesters for Performance Enhancement. Smart Mater. Struct. 2018, 27, 095019. [Google Scholar] [CrossRef]
- Sabzpoushan, S.; Woias, P. Electret-Based Energy Harvesters: A Review. Nano Energy 2024, 131, 110167. [Google Scholar] [CrossRef]
- Ambrożkiewicz, B.; Czyż, Z.; Stączek, P.; Tiseira, A.; García-Tíscar, J. Performance Analysis of a Piezoelectric Energy Harvesting System. Adv. Sci. Technol. Res. J. 2022, 16, 179–185. [Google Scholar] [CrossRef]
- Peng, W.; Du, S. The Advances in Conversion Techniques in Triboelectric Energy Harvesting: A Review. IEEE Trans. Circuits Syst. I 2023, 70, 3049–3062. [Google Scholar] [CrossRef]
- Ferré, E.; Martinez, B.; Villaverde, M.; Gasulla, M.; Reverter, F. Low-Area PV Cells Under Indoor Artificial Lighting: A Comparative Study. IEEE Trans. Instrum. Meas. 2025, 74, 2004109. [Google Scholar] [CrossRef]
- Li, G.; Fan, Y.; Li, Q.; Zheng, Y.; Zhao, D.; Wang, S.; Dong, S.; Guo, W.; Tang, Y. A Review on Micro Combustion Powered Thermoelectric Generator: History, State-of-the-Art and Challenges to Commercialization. Renew. Sustain. Energy Rev. 2025, 207, 114897. [Google Scholar] [CrossRef]
- Wang, L.; Chu, T.; Yuan, S.; Zou, P.; Zhai, W.; Zheng, X.; Xia, M. Advances and Future Perspectives in Thermoelectric Cooling Technology. Energy Convers. Manag. 2025, 332, 119621. [Google Scholar] [CrossRef]
- Win, S.L.Y.; Chiang, Y.-C.; Huang, T.-L.; Lai, C.-M. Thermoelectric Generator Applications in Buildings: A Review. Sustainability 2024, 16, 7585. [Google Scholar] [CrossRef]
- Zoui, M.A.; Bentouba, S.; Stocholm, J.G.; Bourouis, M. A Review on Thermoelectric Generators: Progress and Applications. Energies 2020, 13, 3606. [Google Scholar] [CrossRef]
- Jaziri, N.; Boughamoura, A.; Müller, J.; Mezghani, B.; Tounsi, F.; Ismail, M. A Comprehensive Review of Thermoelectric Generators: Technologies and Common Applications. Energy Rep. 2020, 6, 264–287. [Google Scholar] [CrossRef]
- He, J.; Li, K.; Jia, L.; Zhu, Y.; Zhang, H.; Linghu, J. Advances in the Applications of Thermoelectric Generators. Appl. Therm. Eng. 2024, 236, 121813. [Google Scholar] [CrossRef]
- Tohidi, F.; Ghazanfari Holagh, S.; Chitsaz, A. Thermoelectric Generators: A Comprehensive Review of Characteristics and Applications. Appl. Therm. Eng. 2022, 201, 117793. [Google Scholar] [CrossRef]
- Aridi, R.; Faraj, J.; Ali, S.; Lemenand, T.; Khaled, M. Thermoelectric Power Generators: State-of-the-Art, Heat Recovery Method, and Challenges. Electricity 2021, 2, 359–386. [Google Scholar] [CrossRef]
- Reverter, F. A Tutorial on Thermal Sensors in the 200th Anniversary of the Seebeck Effect. IEEE Sens. J. 2021, 21, 22122–22132. [Google Scholar] [CrossRef]
- Dalola, S.; Ferrari, M.; Ferrari, V.; Guizzetti, M.; Marioli, D.; Taroni, A. Characterization of Thermoelectric Modules for Powering Autonomous Sensors. IEEE Trans. Instrum. Meas. 2009, 58, 99–107. [Google Scholar] [CrossRef]
- Ochieng, A.O.; Megahed, T.F.; Ookawara, S.; Hassan, H. Comprehensive Review in Waste Heat Recovery in Different Thermal Energy-Consuming Processes Using Thermoelectric Generators for Electrical Power Generation. Process Saf. Environ. Prot. 2022, 162, 134–154. [Google Scholar] [CrossRef]
- Wang, J.; Cao, P.; Li, X.; Song, X.; Zhao, C.; Zhu, L. Experimental Study on the Influence of Peltier Effect on the Output Performance of Thermoelectric Generator and Deviation of Maximum Power Point. Energy Convers. Manag. 2019, 200, 112074. [Google Scholar] [CrossRef]
- Kanimba, E.; Tian, Z. Modeling of a Thermoelectric Generator Device. In Thermoelectrics for Power Generation—A Look at Trends in the Technology; Skipidarov, S., Nikitin, M., Eds.; InTech: Houston, TX, USA, 2016; ISBN 978-953-51-2845-8. [Google Scholar]
- Qing, S.; Rezaniakolaei, A.; Rosendahl, L.A.; Gou, X. An Analytical Model for Performance Optimization of Thermoelectric Generator With Temperature Dependent Materials. IEEE Access 2018, 6, 60852–60861. [Google Scholar] [CrossRef]
- Mahan, G.D. Introduction to Thermoelectrics. APL Mater. 2016, 4, 104806. [Google Scholar] [CrossRef]
- Li, J.; Yang, L. Recent Development of Heat Sink and Related Design Methods. Energies 2023, 16, 7133. [Google Scholar] [CrossRef]
- Incropera, F.P.; Dewitt, D.P.; Bergman, T.L.; Lavine, A.S. Fundamentals of Heat and Mass Transfer, 6th ed.; John Wiley: Hoboken, NJ, USA, 2007; ISBN 978-0-471-45728-2. [Google Scholar]
- Elghool, A.; Basrawi, F.; Ibrahim, T.K.; Habib, K.; Ibrahim, H.; Idris, D.M.N.D. A Review on Heat Sink for Thermo-Electric Power Generation: Classifications and Parameters Affecting Performance. Energy Convers. Manag. 2017, 134, 260–277. [Google Scholar] [CrossRef]
- Yang, L.; Huang, J.; Zhou, F. Thermophysical Properties and Applications of Nano-Enhanced PCMs: An Update Review. Energy Convers. Manag. 2020, 214, 112876. [Google Scholar] [CrossRef]
- Kalbasi, R. Introducing a Novel Heat Sink Comprising PCM and Air—Adapted to Electronic Device Thermal Management. Int. J. Heat Mass Transf. 2021, 169, 120914. [Google Scholar] [CrossRef]
- Marjanović, M.; Prijić, A.; Randjelović, B.; Prijić, Z. A Transient Modeling of the Thermoelectric Generators for Application in Wireless Sensor Network Nodes. Electronics 2020, 9, 1015. [Google Scholar] [CrossRef]
- Vargas-Almeida, A.; Olivares-Robles, M.; Camacho-Medina, P. Thermoelectric System in Different Thermal and Electrical Configurations: Its Impact in the Figure of Merit. Entropy 2013, 15, 2162–2180. [Google Scholar] [CrossRef]
- Tang, M.; Wang, J.; Ou, Y.; Tang, Z. A Novel Thermoelectric Generation Array Reconfiguration to Reduce Mismatch Power Loss Under Nonuniform Temperature Distribution. Int. J. Energy Res. 2024, 2024, 7820395. [Google Scholar] [CrossRef]
- He, W.; Zhang, G.; Li, G.; Ji, J. Analysis and Discussion on the Impact of Non-Uniform Input Heat Flux on Thermoelectric Generator Array. Energy Convers. Manag. 2015, 98, 268–274. [Google Scholar] [CrossRef]
- Montecucco, A.; Siviter, J.; Knox, A.R. The Effect of Temperature Mismatch on Thermoelectric Generators Electrically Connected in Series and Parallel. Appl. Energy 2014, 123, 47–54. [Google Scholar] [CrossRef]
- Vangal, P.; Svenson, P. Examining Heat Recovery from Electric Light Bulbs Using Thermoelectric Generators. J. Emerg. Investig. 2018. [Google Scholar] [CrossRef] [PubMed]
- Weng, C.-C.; Huang, M.-J. A Study of Using a Thermoelectric Generator to Harvest Energy from a Table Lamp. Energy 2014, 76, 788–798. [Google Scholar] [CrossRef]
- Peng, Y.; Zhang, X.; Liu, J.; Li, S.; Mou, Y.; Xu, J.; Chen, M. Waste Heat Recycling of High-Power Lighting through Chips on Thermoelectric Generator. Energy Convers. Manag. 2021, 243, 114329. [Google Scholar] [CrossRef]
- Ramadan, M.; Ali, S.; Bazzi, H.; Khaled, M. New Hybrid System Combining TEG, Condenser Hot Air and Exhaust Airflow of All-Air HVAC Systems. Case Stud. Therm. Eng. 2017, 10, 154–160. [Google Scholar] [CrossRef]
- Mona, Y.; Chaichana, C.; Rattanamongkhonkun, K.; Thiangchanta, S. Energy Harvesting from Air Conditioners by Using a Thermoelectric Application. Energy Rep. 2022, 8, 456–462. [Google Scholar] [CrossRef]
- Yildiz, F.; Coogler, K.L. Low Power Energy Harvesting with a Thermoelectric Generator through an Air Conditioning Condenser. In Proceedings of the 360 of Engineering Education, Indianapolis, IN, USA, 15–18 June 2014; American Society for Engineering Education: Washington, DC, USA, 2014; p. 24.877.1. [Google Scholar] [CrossRef]
- Jaber Abdulhamed, A.; Al-Akam, A.; Abduljabbar, A.A.; Alkhafaji, M.H. Waste Heat Recovery from a Drier Receiver of an A/C Unit Using Thermoelectric Generators. Energy Eng. 2023, 120, 1729–1746. [Google Scholar] [CrossRef]
- Lineykin, S.; Sitbon, M.; Kuperman, A. Design and Optimization of Low-Temperature Gradient Thermoelectric Harvester for Wireless Sensor Network Node on Water Pipelines. Appl. Energy 2021, 283, 116240. [Google Scholar] [CrossRef]
- Machacek, Z.; Walendziuk, W.; Sotola, V.; Slanina, Z.; Petras, R.; Schneider, M.; Masny, Z.; Idzkowski, A.; Koziorek, J. An Investigation of Thermoelectric Generators Used as Energy Harvesters in a Water Consumption Meter Application. Energies 2021, 14, 3768. [Google Scholar] [CrossRef]
- Mileiko, S.; Cetinkaya, O.; Mackie, D.; Shafik, R.; Balsamo, D. A TEG-Based Non-Intrusive Ultrasonic System for Autonomous Water Flow Rate Measurement. IEEE Trans. Sustain. Comput. 2023, 8, 363–374. [Google Scholar] [CrossRef]
- Wang, W.; Cionca, V.; Wang, N.; Hayes, M.; O’Flynn, B.; O’Mathuna, C. Thermoelectric Energy Harvesting for Building Energy Management Wireless Sensor Networks. Int. J. Distrib. Sens. Netw. 2013, 9, 232438. [Google Scholar] [CrossRef]
- Van Toan, N.; Kim Tuoi, T.T.; Ono, T. High-Performance Flexible Thermoelectric Generator for Self-Powered Wireless BLE Sensing Systems. J. Power Sources 2022, 536, 231504. [Google Scholar] [CrossRef]
- Škalic, I.; Marinović, I.; Modrić, T. Energy Harvesting on AB-Class Power Amplifier Applying Thermoelectric Generators in Push–Pull Mode. Machines 2023, 11, 622. [Google Scholar] [CrossRef]
- Álvarez-Carulla, A.; Saiz-Vela, A.; Puig-Vidal, M.; López-Sánchez, J.; Colomer-Farrarons, J.; Miribel-Català, P.L. High-Efficient Energy Harvesting Architecture for Self-Powered Thermal-Monitoring Wireless Sensor Node Based on a Single Thermoelectric Generator. Sci. Rep. 2023, 13, 1637. [Google Scholar] [CrossRef]
- Lin, Q.; Chen, Y.-C.; Chen, F.; DeGanyar, T.; Yin, H. Design and Experiments of a Thermoelectric-Powered Wireless Sensor Network Platform for Smart Building Envelope. Appl. Energy 2022, 305, 117791. [Google Scholar] [CrossRef]
- Nuwayhid, R.Y.; Shihadeh, A.; Ghaddar, N. Development and Testing of a Domestic Woodstove Thermoelectric Generator with Natural Convection Cooling. Energy Convers. Manag. 2005, 46, 1631–1643. [Google Scholar] [CrossRef]
- Marinović, I.; Škalic, I. Improving Efficiency of AB-Class Audio Power Amplifier Using Thermoelectric Generators. Electronics 2024, 14, 34. [Google Scholar] [CrossRef]
- Boitier, V.; Estibals, B.; Seguier, L. Powering a Low Power Wireless Sensor in a Harsh Industrial Environment: Energy Recovery with a Thermoelectric Generator and Storage on Supercapacitors. EPE 2023, 15, 372–398. [Google Scholar] [CrossRef]
- Chen, J.; Zuo, L.; Wu, Y.; Klein, J. Modeling, Experiments and Optimization of an on-Pipe Thermoelectric Generator. Energy Convers. Manag. 2016, 122, 298–309. [Google Scholar] [CrossRef]
- Lopera, J.M.; Del Arco Rodriguez, H.; Perez Pereira, J.M.; De Castro, A.R.; Rendueles Vigil, J.L. Practical Issues in the Design of Wireless Sensors Supplied by Energy Harvesting Thermoelectric Generators. IEEE Trans. Ind. Applicat. 2019, 55, 996–1005. [Google Scholar] [CrossRef]
- Ding, X.; Ying, Z.; Zu, W.; Han, L. Experimental Study on Thermoelectric Energy Harvesting from Indoor High-Voltage Disconnector. IEEE Sens. J. 2024, 24, 2985–2995. [Google Scholar] [CrossRef]
- Liu, Y.; Riba, J.-R.; Moreno-Eguilaz, M.; Sanllehí, J. Application of Thermoelectric Generators for Low-Temperature-Gradient Energy Harvesting. Appl. Sci. 2023, 13, 2603. [Google Scholar] [CrossRef]
- Kadechkar, A.; Riba, J.-R.; Moreno-Eguilaz, M.; Perez, J. SmartConnector: A Self-Powered IoT Solution to Ease Predictive Maintenance in Substations. IEEE Sens. J. 2020, 20, 11632–11641. [Google Scholar] [CrossRef]
- Yousefi, E.; Abbas Nejad, A. Waste Heat Management in Electric Motors Using Thermoelectric Generator Integrated with Phase Change Material and Metal Foam-Embedded Heat Sink. Energy Rep. 2024, 11, 6199–6207. [Google Scholar] [CrossRef]
- Hou, L.; Tan, S.; Zhang, Z.; Bergmann, N.W. Thermal Energy Harvesting WSNs Node for Temperature Monitoring in IIoT. IEEE Access 2018, 6, 35243–35249. [Google Scholar] [CrossRef]
- Dias, P.C.; Morais, F.J.O.; De Morais Franca, M.B.; Ferreira, E.C.; Cabot, A.; Siqueira Dias, J.A. Autonomous Multisensor System Powered by a Solar Thermoelectric Energy Harvester with Ultralow-Power Management Circuit. IEEE Trans. Instrum. Meas. 2015, 64, 2918–2925. [Google Scholar] [CrossRef]
- Carvalhaes-Dias, P.; Cabot, A.; Siqueira Dias, J.A. Evaluation of the Thermoelectric Energy Harvesting Potential at Different Latitudes Using Solar Flat Panels Systems with Buried Heat Sink. Appl. Sci. 2018, 8, 2641. [Google Scholar] [CrossRef]
- Gao, M.; Su, C.; Cong, J.; Yang, F.; Wang, Y.; Wang, P. Harvesting Thermoelectric Energy from Railway Track. Energy 2019, 180, 315–329. [Google Scholar] [CrossRef]
- Catalan, L.; Alegria, P.; Araiz, M.; Astrain, D. Field Test of a Geothermal Thermoelectric Generator without Moving Parts on the Hot Dry Rock Field of Timanfaya National Park. Appl. Therm. Eng. 2023, 222, 119843. [Google Scholar] [CrossRef]
- Ga, L.; Zhang, Z.; Xu, D.; Li, W. Performance of Thermoelectric Conversion Device with Power Management Module Based on Shallow Soil-Air Temperature Difference. Case Stud. Therm. Eng. 2021, 28, 101582. [Google Scholar] [CrossRef]
- Paterova, T.; Prauzek, M.; Konecny, J.; Ozana, S.; Zmij, P.; Stankus, M.; Weise, D.; Pierer, A. Environment-Monitoring IoT Devices Powered by a TEG Which Converts Thermal Flux between Air and Near-Surface Soil into Electrical Energy. Sensors 2021, 21, 8098. [Google Scholar] [CrossRef] [PubMed]
- Amagai, Y.; Ichinose, A.; Ikawa, R.; Sakamoto, M.; Ogiya, T.; Konishi, M.; Okawa, K.; Sakamoto, N.; Kaneko, N.-H. Harvesting Thermal Energy from Spring Water Using a Flexible Thermoelectric Generator. Energy Convers. Manag. 2024, 313, 118605. [Google Scholar] [CrossRef]
- Quintans, C.; Marcos-Acevedo, J.; Martinez-Penalver, C. Thermoelectric Energy Harvesting System Based on Water-Stored Energy and Daily Ambient Temperature Variations. IEEE Sens. J. 2020, 20, 13919–13929. [Google Scholar] [CrossRef]
- Verma, G.; Sharma, V. A Novel Thermoelectric Energy Harvester for Wireless Sensor Network Application. IEEE Trans. Ind. Electron. 2018, 66, 3530–3538. [Google Scholar] [CrossRef]
- Lv, J.-R.; Ma, J.-L.; Dai, L.; Yin, T.; He, Z.-Z. A High-Performance Wearable Thermoelectric Generator with Comprehensive Optimization of Thermal Resistance and Voltage Boosting Conversion. Appl. Energy 2022, 312, 118696. [Google Scholar] [CrossRef]
- Shi, Y.; Wang, Y.; Mei, D.; Chen, Z. Wearable Thermoelectric Generator with Copper Foam as the Heat Sink for Body Heat Harvesting. IEEE Access 2018, 6, 43602–43611. [Google Scholar] [CrossRef]
- Xia, C.; Zhang, D.; Pedrycz, W.; Fan, K.; Guo, Y. Human Body Heat Based Thermoelectric Harvester with Ultra-Low Input Power Management System for Wireless Sensors Powering. Energies 2019, 12, 3942. [Google Scholar] [CrossRef]
- Thielen, M.; Sigrist, L.; Magno, M.; Hierold, C.; Benini, L. Human Body Heat for Powering Wearable Devices: From Thermal Energy to Application. Energy Convers. Manag. 2017, 131, 44–54. [Google Scholar] [CrossRef]
- Hyland, M.; Hunter, H.; Liu, J.; Veety, E.; Vashaee, D. Wearable Thermoelectric Generators for Human Body Heat Harvesting. Appl. Energy 2016, 182, 518–524. [Google Scholar] [CrossRef]
- Newby, S.; Mirihanage, W.; Fernando, A. Body Heat Energy Driven Knitted Thermoelectric Garments with Personal Cooling. Appl. Therm. Eng. 2025, 258, 124546. [Google Scholar] [CrossRef]
- Elmoughni, H.M.; Atalay, O.; Ozlem, K.; Menon, A.K. Thermoelectric Clothing for Body Heat Harvesting and Personal Cooling: Design and Fabrication of a Textile-Integrated Flexible and Vertical Device. Energy Technol. 2022, 10, 2200528. [Google Scholar] [CrossRef]
- Ahmed, R.; Zeeshan; Mehmood, M.U.; Mannan, A.; Lee, J.Y.; Lim, S.H.; Chun, W. Design and Performance Analysis of Powering a Wireless Earphone by a Thermoelectric Generator. IEEE Access 2021, 9, 54457–54465. [Google Scholar] [CrossRef]
- Kim, D.; Seo, S.; Kim, S.; Shin, S.; Son, K.; Jeon, S.; Han, S. Design and Performance Analyses of Thermoelectric Coolers and Power Generators for Automobiles. Sustain. Energy Technol. Assess. 2022, 51, 101955. [Google Scholar] [CrossRef]
- Merkel, J.; Yee, S.; Nelson, C.; Jenkins, B.; ElBidweihy, H.; Joyce, P.; Brownell, C.; Mechtel, D. High-Energy Laser Detection through Thermoelectric Generators. Opt. Eng. 2020, 59, 117105. [Google Scholar] [CrossRef]
- Shi, Y.; Wang, Y.; Deng, Y.; Gao, H.; Lin, Z.; Zhu, W.; Ye, H. A Novel Self-Powered Wireless Temperature Sensor Based on Thermoelectric Generators. Energy Convers. Manag. 2014, 80, 110–116. [Google Scholar] [CrossRef]
- Bonin, R.; Boero, D.; Chiaberge, M.; Tonoli, A. Design and Characterization of Small Thermoelectric Generators for Environmental Monitoring Devices. Energy Convers. Manag. 2013, 73, 340–349. [Google Scholar] [CrossRef]






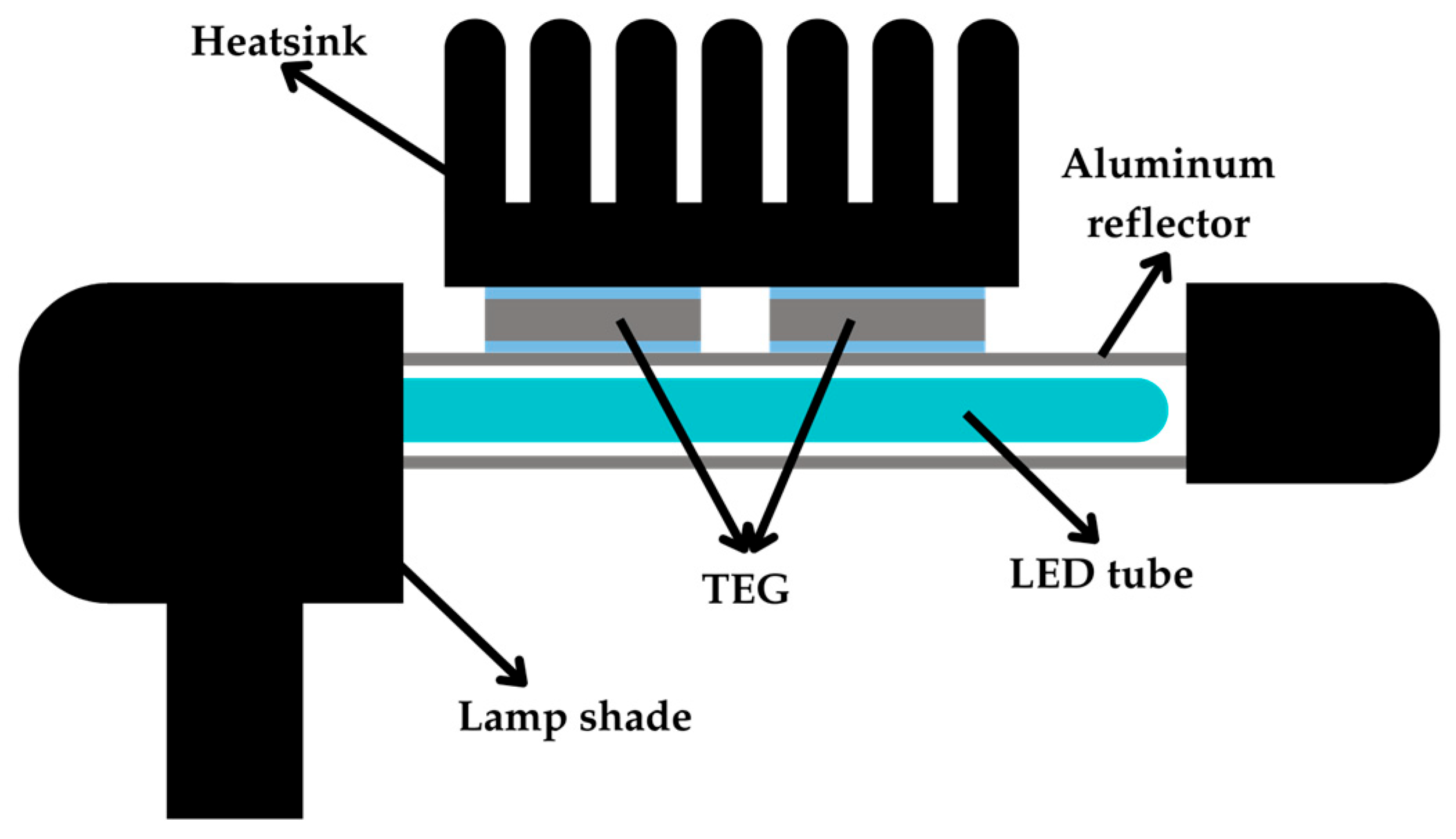
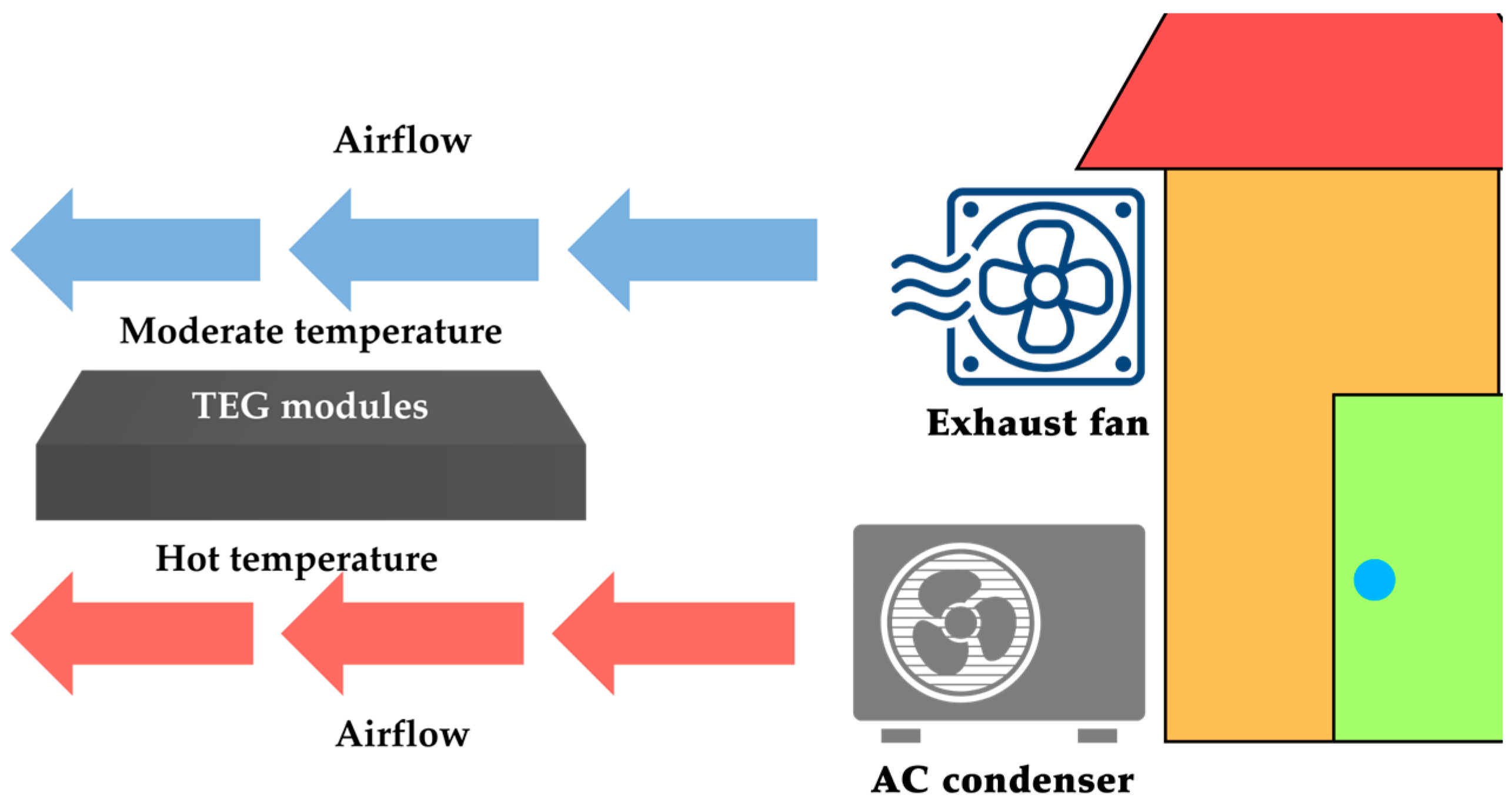

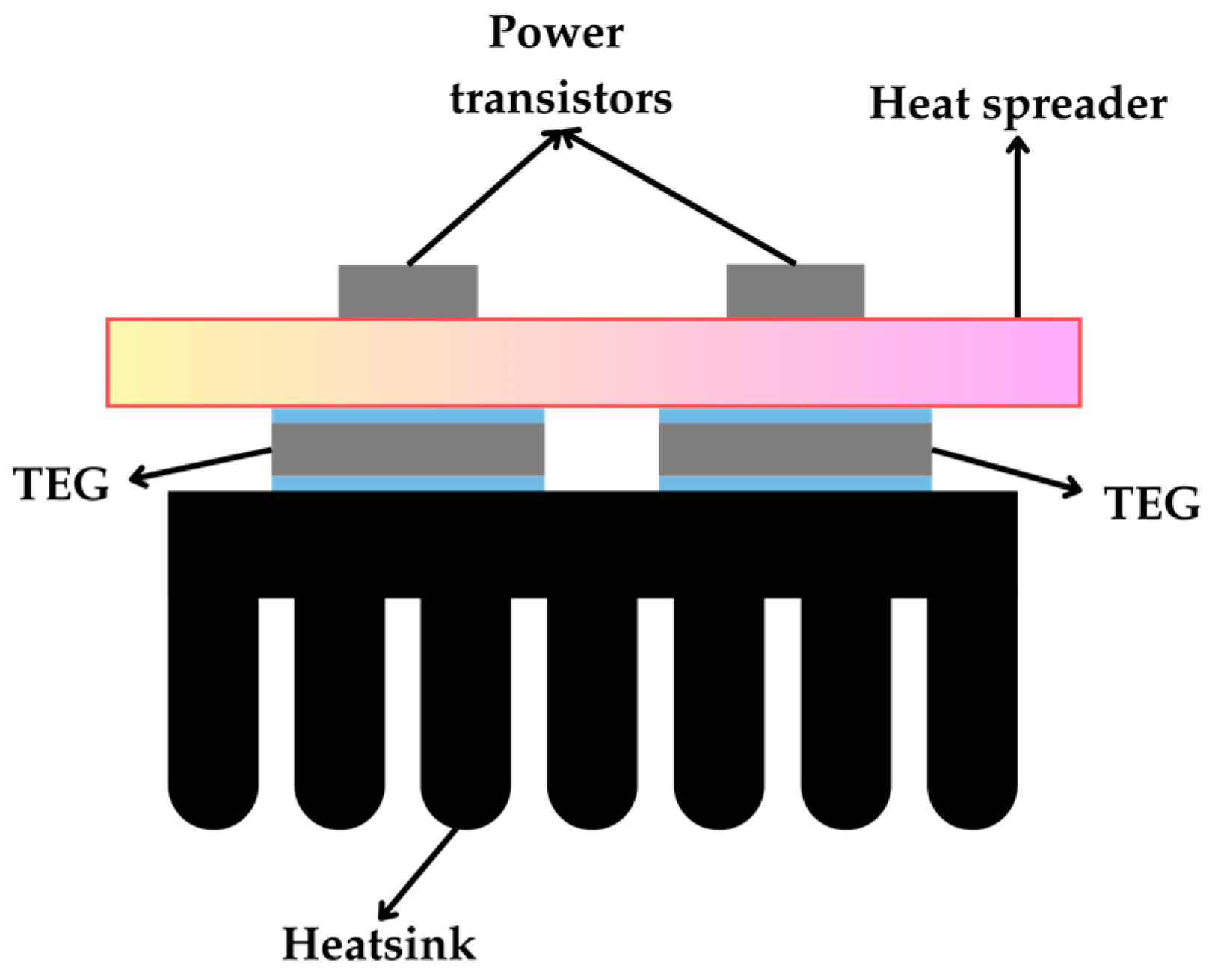
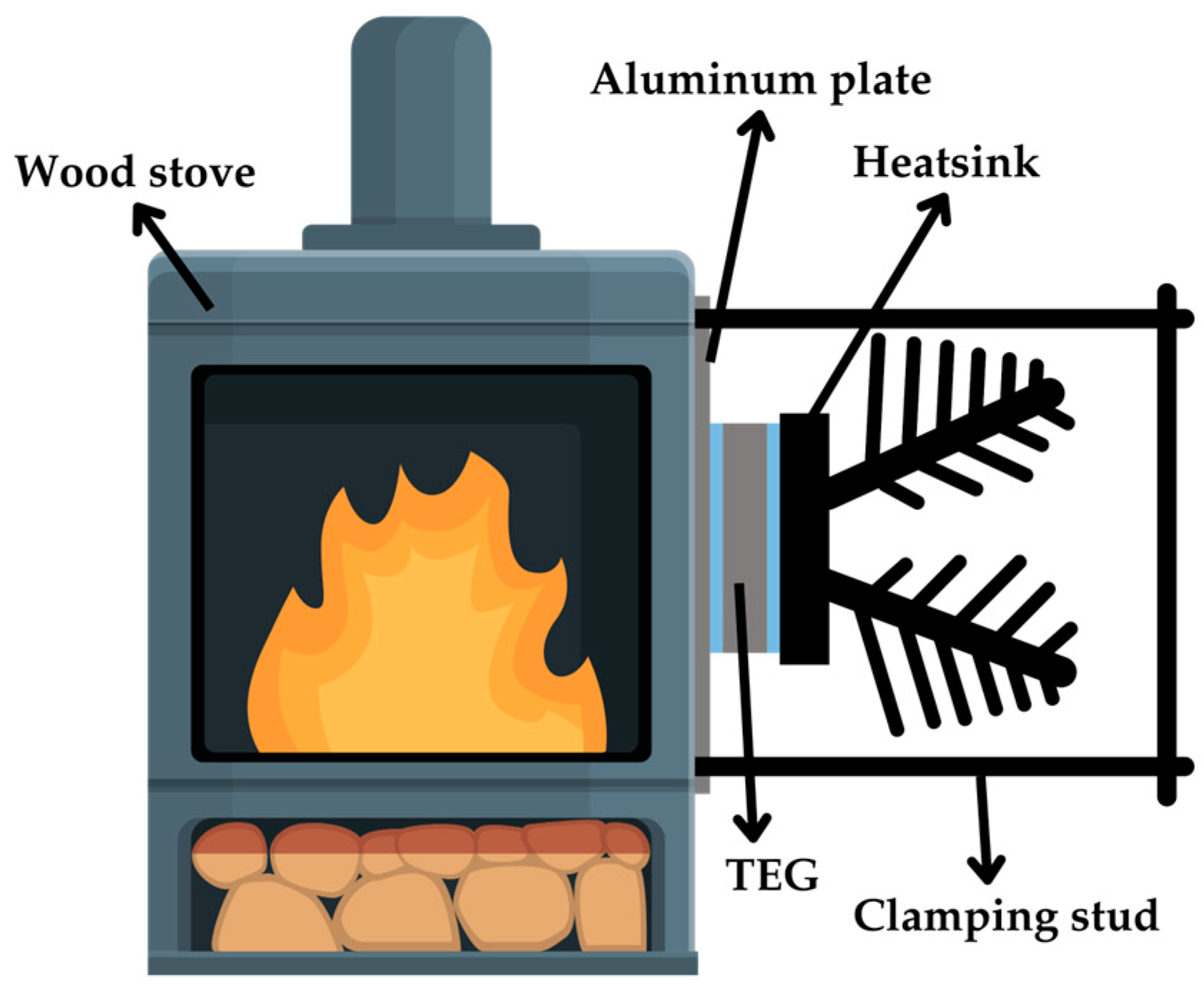



| Heatsink Type | Thermal Resistance | Complexity | Cost | Additional Requirements | Example |
|---|---|---|---|---|---|
| Passive | High | Very low | Very low | NA | Metal plate |
| Semi-active | Medium | Low | Low | NA | Fin heat sink |
| Active | Low | Medium | Medium | Additional power for operation | Fan-fin heat sink |
| Liquid | Very low | Very high | Very high | Additional power for continuous circulation | Liquid cold plate |
| Phase change | Very low (a) High (b) | High | High | Additional space | PCM based-heatsink |
| Ref. | Thermal Source | ΔT (°C) | ΔTTEG (°C) | TEG Module | TEG Size (mm) | Heatsink | Heatsink Size (mm) | TEG Config. | VOC (a) (V) | PMPP (a) (mW) |
|---|---|---|---|---|---|---|---|---|---|---|
| [57] | LED lamp | NR | 34 | TEC1-12706 | 40 × 40 × 3.9 | Semi active | NR | 4 TEGs (b) | 0.38 | 8.3 |
| [58] | NR | 2 | TGM-199-1.4-1.5 | 40 × 40 × 4.1 | Semi active | 102 × 51 × 22 | 2 TEGs (b) | 0.17–0.18 | 1 | |
| [59] | NR | 219 | Custom | 12 × 12 × 3 | Active | NR | Single | 1.23 V | 8.5 | |
| [60] | AC | NR | 30–134 | Custom | NR | NA | NA | NR | NR | 19,900–90,300 (d) |
| [61] | NR | NR | Model 9500 | 55 × 55 × 3.9 | Liquid | NR | 8 TEGs (b) | 0.47 | 4.3 | |
| [62] | NR | 16 | 1261G-7L31-24CX1 | 56 × 56 × 5 | Liquid | 63.5 × 63.5 | Single | 0.44 | NR | |
| [63] | 29 | NR | SP1848 27154 SA | 40 × 40 × 4 | Semi active | 76 × 105 × 44 | 18 TEGs (c) | 5.2 V | NR | |
| [64] | Pipeline | 7 | NR | TES1-24102 | 40 × 40 × 4 | Semi active | NR | 48 TEGs (b) | 10 V | 30 |
| [65] | 10 | NR | TES1-127060 | 40 × 40 × 3.6 | Semi active | NR | Single | NR | 0.35 | |
| [66] | 3–12 | NR | GM250-71-14-16 | 30 × 30 × 3.4 | Semi active | 40 × 40 | 2 TEGs | NR | NR | |
| [67] | Wall mount heater | 40 | NR | Custom | 50 × 50 × 3.4 | Semi active | NR | Single | 0.47 | 4.1 |
| [68] | Electric thermos pot | NR | >5 | Custom, flexible | 210 × 75 × 2.5 | Passive | NR | Single | 0.11 | 0.8 |
| [69] | Power amplifier | NR | 30 | GM200-127-14-10 | 40 × 40 × 3.4 | Semi active | 65 × 49 × 5 | Single | 1.7 V | 293 |
| [70] | MCU | NR | 13 | TGM-127-1.0-2.5 | 30 × 30 × 4.8 | NA | NA | Single | 0.47 | 12.7 |
| [71] | Window frame | NR | 5 | 1261G-7L3104CL | 30 × 30 × 3.7 | Passive | NR | 4 TEGs (b) | 0.10 | 1.5 |
| [72] | Wood stove | NR | 88 | Custom | 56 × 56 | Semi active | 180 × 125 × 136 | Single | 4.1 V | 4200 |
| Ref. | Thermal Source | ΔT (°C) | ΔTTEG (°C) | TEG Module | TEG Size (mm) | Heatsink | Heatsink Size (mm) | TEG Config. | VOC (a) (V) | PMPP (a) (mW) |
|---|---|---|---|---|---|---|---|---|---|---|
| [74] | Pipeline | 150 | NR | TEG1-30-30-8.5/200 | 40 × 40 × 3.4 | Semi active | 51 × 51 × 33 | Single | 2.1 | 58 |
| [75] | NR | 130–137 | Hz-2 | 29 × 29 × 5 | Semi active | 125 × 150 × 50 | 2 TEGs (b) | 8.1 | 2250 | |
| [76] | NR | 5 | CP20151 | 15 × 15 × 5 | Passive | NR | Single | 0.055 | 0.46 | |
| [77] | Busbar | NR | 28 | TEG1-381-1.4-1.2 | 90 × 30 × 3.41 | Semi active | 90 × 30 × 25 | Single | 0.42 | 9.2 |
| [78] | NR | 10 | GM250-157-14-16 | 40 × 40 × 4.1 | Semi active | 25 × 38 (base), 65 × 40 (top fin field) | 2 TEGs (b) | 0.79 | 20.3 | |
| [79] | 44 | NR | TG12-8 | 40 × 40 × 3.6 | Passive | NR | 2 TEGs (b) | NR | 5.4 | |
| [80] | Motor | NR | 19 | SP1848–271455A | 40 × 40 × 4 | Phase change and semi active | Phase change box: 40 × 40 × 40, fin heatsink: 40 × 40 × 14 | 4 TEGs (b) | 0.94 | NR |
| [81] | Hot wall | NR | 14 | TGM287-1.0-1.3 | 40 × 40 × 3.6 | Semi active | 88 × 88 × 23 | 2 TEGs (c) | 0.61 (TEG #1) 0.59 (TEG #2) | 35 (TEG #1) 33 (TEG #2) |
| Ref. | Thermal Source | ΔT (°C) | ΔTTEG (°C) | TEG Module | TEG Size (mm) | Heatsink | Heatsink Size (mm) | TEG Config. | VOC (a) (V) | PMPP (a) (mW) |
|---|---|---|---|---|---|---|---|---|---|---|
| [82] | Solar platform | <15 | 7 | TEG-241.1.0-1.2 | 40 × 40 | Semi active | NR | Single | 0.8 | NR |
| [83] | 20 | NR | TEG-241.1.0-1.2 | 40 × 40 | Semi active | NR | Single | NR | NR | |
| [84] | NR | 8 | NR | 54 × 54 × 3.4 | Semi active | 225 × 200 × 50 | Single | 0.5 | 5.8 | |
| [85] | Geo thermal | 138–155 | 86 (A) 98 (B) | TG128LS | 40 × 40 × 3.5 | Semi active | 104 × 28 × 0.3 | 10 TEGs (b) (A) 6 TEGs (b) (B) | NR | 18,800 (A) 15,300 (B) |
| [86] | NR | 25 | TG122.5 | 40 × 44 × 3.9 | Semi active | NR | 8 TEGs (b) | 0.7 | 3.6 | |
| [87] | NR | 14 | TEC1-12706 | 40 × 40 × 3.2 | Active | NR | Single | 0.7 | NR | |
| [88] | Water | 15 | NR | Custom, flexible | NR | Semi active | NR | 2 TEGs (b) | NR | 11.4 |
| [89] | 6 | NR | CP60440 | 40 × 40 × 4 | NR | NR | 2 TEGs (b) | NR | 2 | |
| [90] | NR | 3 | TEC1-12706 | 40 × 40 × 3.2 | Liquid | NR | 4 TEGs (b) | NR | 20 |
| Ref. | Thermal Source | ΔT (°C) | ΔTTEG (°C) | TEG Module | TEG Size (mm) | Heatsink | Heatsink Size (mm) | TEG Config. | VOC (a) (mV) | PMPP (a) (μW) |
|---|---|---|---|---|---|---|---|---|---|---|
| [91] | Head | 12 | NR | Custom | 35 × 30 × 3 | Semi active | NR | Single | 16 | 39 |
| [92] | Wrist | 45 | NR | Custom | 44 × 26 × 2.6 | Semi active | NR | Single | 50 | 276 |
| [93] | 7 | NR | GM200-71-14-16 | 30 × 30 × 3.4 | Semi active | 50 × 50 × 20 | 3 TEGs (c) | 110 | 581 | |
| [94] | NR | 6 | TPG-651 (μTEG) QC32-0.6-1.2 (mTEG) | NR (μTEG) 8 × 8 × 2.6 (mTEG) | Semi active | 14 × 14 × 6 | 7 TEGs (b) | NR | 852 (μTEG)959 (mTEG) | |
| [95] | Chest/wrist/arm/t-shirt | 19 | NR | Custom | 13 × 6.6 | Passive | 20 × 25 × 0.13 | Single | 15 | 5.5 |
| [96] | Clothes | 16 | NR | Custom, knitted | NR | NA | NA | Single | 9.3 | 1.2 |
| [97] | 14 | NR | Custom | 37 × 20 | Semi active | NR | Single | 3 | 3.8 | |
| [98] | Ear (d) | NR | 10 | Custom | D = 12 H = 4 | NA | NA | Single | 52 | 600 |
| Ref. | Thermal Source | ΔT (°C) | ΔTTEG (°C) | TEG Module | TEG Size (mm) | Heatsink | Heatsink Size (mm) | TEG Config. | VOC (a) (V) | PMPP (a) (mW) |
|---|---|---|---|---|---|---|---|---|---|---|
| [99] | Vehicle | NR | 90 | TGM-199-1.4-0.8 | 40 × 40 × 3.2 | Liquid | NR | 24 TEGs (b) | NR | 90,700 |
| [100] | Laser | NR | 14 | CP60240 | 20 × 20 × 4 | NA | NA | Single | 0.3 | NR |
| [101] | Wildfire | NR | 40 | TEC12706 | 40 × 40 × 3.9 | Phase change and semi active | Phase change box, fin heatsink: 40 × 40 × 11 | Single | 1.2 V | 180 |
| [102] | Catalytic burner | NR | 11 | GM250-127-1416 | 40 × 40 × 4.1 | Semi-active | NR | Single | 0.3 | 12 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ridwan, M.; Gasulla, M.; Reverter, F. Principle and Applications of Thermoelectric Generators: A Review. Sensors 2025, 25, 2484. https://doi.org/10.3390/s25082484
Ridwan M, Gasulla M, Reverter F. Principle and Applications of Thermoelectric Generators: A Review. Sensors. 2025; 25(8):2484. https://doi.org/10.3390/s25082484
Chicago/Turabian StyleRidwan, Mohamad, Manel Gasulla, and Ferran Reverter. 2025. "Principle and Applications of Thermoelectric Generators: A Review" Sensors 25, no. 8: 2484. https://doi.org/10.3390/s25082484
APA StyleRidwan, M., Gasulla, M., & Reverter, F. (2025). Principle and Applications of Thermoelectric Generators: A Review. Sensors, 25(8), 2484. https://doi.org/10.3390/s25082484








