Enhancing the Poor Flow and Tableting Problems of High Drug-Loading Formulation of Canagliflozin Using Continuous Green Granulation Process and Design-of-Experiment Approach
Abstract
:1. Introduction
2. Results and Discussion
2.1. Statistical and Diagnostic Analysis of the Design
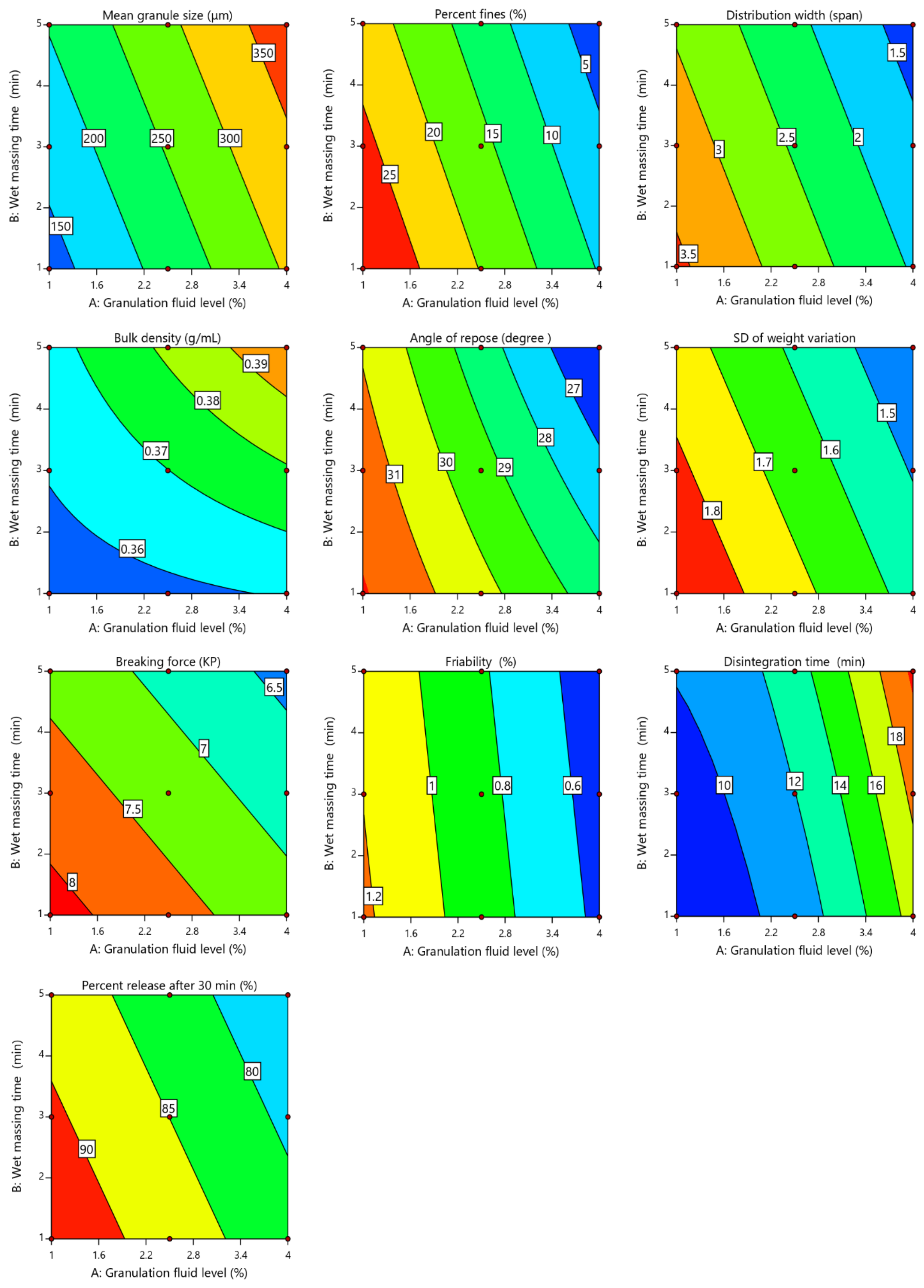
2.2. Influence of Process Variables on Granules Properties
2.2.1. Mean Granules Size (d50)
2.2.2. Granules’ Bulk Density
2.2.3. Granules’ Flow
2.3. Influence of Process Variables on Tablets’ Properties
2.3.1. Tablet Weight Variation
2.3.2. Tablet Breaking Force and Friability
2.3.3. Tablet Disintegration
2.3.4. Tablet Dissolution
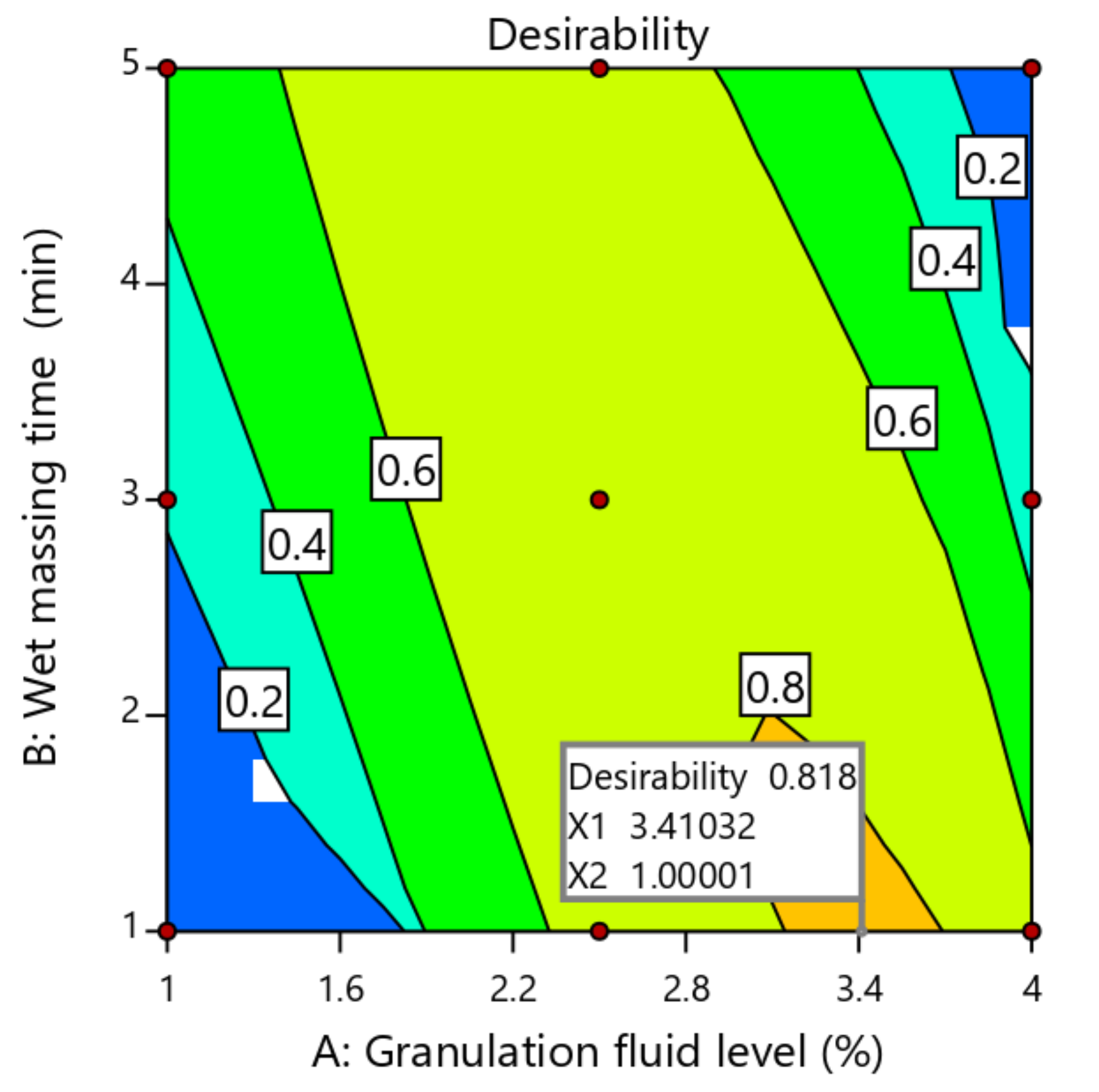
2.4. Optimization of Process Variables Using Desirability Function
3. Materials and Methods
3.1. Materials
3.2. Experimental Design
3.3. Manufacture of Granules and Tablets
3.4. Evaluation of Prepared Granules
3.4.1. Mean Granule Size (d50)
3.4.2. Granules’ Bulk Density
3.4.3. Granules Flow
3.5. Evaluation of Prepared Tablets
3.5.1. Weight Variation
3.5.2. Breaking Force
3.5.3. Friability
3.5.4. Tablet Disintegration
3.5.5. Tablet Dissolution
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Mosley, J.; Smith, L.; Everton, E.; Fellner, C. Sodium-glucose linked transporter 2 (SGLT2) inhibitors in the management of type-2 diabetes: A drug class overview. Pharm. Ther. 2015, 40, 451–462. [Google Scholar]
- Nauck, M. Update on developments with SGLT2 inhibitors in the management of type 2 diabetes. Drug Des. Devel. Ther. 2014, 8, 1335–1380. [Google Scholar] [CrossRef] [Green Version]
- Lajara, R. The potential role of sodium glucose co transporter 2 inhibitors in combination therapy for type 2 diabetes mellitus. Expert Opin. Pharm. 2014, 15, 2565–2585. [Google Scholar] [CrossRef] [Green Version]
- Shah, P.A.; Shrivastav, P.S. Ion-pair solid phase extraction for the simultaneous separation and quantitation of metformin and canagliflozin in human plasma by LC-MS/MS. Microchem. J. 2018, 143, 181–189. [Google Scholar] [CrossRef]
- Schaller, B.; Moroney, K.; Castro-Dominguez, B.; Cronin, B.; Belen-Girona, J.; Ruane, P.; Croker, D.; Walker, G. Systematic development of a high dosage formulation to enable direct compression of a poorly flowing API: A case study. Int. J. Pharm. 2019, 566, 615–630. [Google Scholar] [CrossRef] [PubMed]
- Shah, P.; Shrivastav, P.; Sharma, V.; Yadav, M. Challenges in simultaneous extraction and chromatographic separation of metformin and three SGLT-2 inhibitors in human plasma using LC–MS/MS. J. Pharm. Biomed. 2019, 175, 112790. [Google Scholar] [CrossRef]
- Cai, L.; Farber, L.; Zhang, D.; Li, F.; Farabaugh, J. A new methodology for high drug loading wet granulation formulation development. Int. J. Pharm. 2013, 441, 790–800. [Google Scholar] [CrossRef]
- Suresh, P.; Sreedhar, I.; Vaidhiswaran, R.; Venugopal, A. A comprehensive review on process and engineering aspects of pharmaceutical wet granulation. Chem. Eng. J. 2017, 328, 790–800. [Google Scholar] [CrossRef]
- Pohla, S.; Kleinebudde, P. A review of regime maps for granulation. Int. J. Pharm. 2020, 587, 119660. [Google Scholar] [CrossRef]
- Moravkar, K.K.; Ali, T.M.; Pawar, J.N.; Amin, P.D. Application of moisture activated dry granulation (MADG) process to develop high dose immediate release (IR) formulations. Adv. Powder Technol. 2017, 28, 1270–1280. [Google Scholar] [CrossRef]
- Takasaki, H.; Yonemochi, E.; Ito, M.; Wada, K.; Terada, K. The importance of binder moisture content in Metformin HCL high-dose formulations prepared by moist aqueous granulation (MAG). Results Pharm. Sci. 2015, 5, 1–7. [Google Scholar] [CrossRef] [Green Version]
- Takasaki, H.; Yonemochi, E.; Ito, M.; Wada, K.; Terada, K. The effect of water activity on granule characteristics and tablet properties produced by moisture activated dry granulation (MADG). Powder Technol. 2016, 294, 113–118. [Google Scholar] [CrossRef]
- Christensen, L.H.; Johansen, H.E.; Schaefer, T. Moisture-Activated dry Granulation in a high Shear Mixer. Drug Dev. Ind. Pharm. 1994, 20, 2195–2213. [Google Scholar] [CrossRef]
- Gajera, B.Y.; Shah, D.A.; Dave, R.H. Development of an amorphous nanosuspension by sonoprecipitation-formulation and process optimization using design of experiment methodology. Int. J. Pharm. 2019, 559, 348–359. [Google Scholar] [CrossRef] [PubMed]
- Mousavi, L.; Tamiji, Z.; Khoshayand, M.R. Applications and opportunities of experimental design for the dispersive liquid–liquid microextraction method—A review. Talanta 2018, 190, 335–356. [Google Scholar] [CrossRef] [PubMed]
- Grangeia, H.B.; Silva, C.; Simões, S.P.; Reis, M.S. Quality by design in pharmaceutical manufacturing: A systematic review of current status, challenges and future perspectives. Eur. J. Pharm. Biopharm. 2020, 147, 19–37. [Google Scholar] [CrossRef] [PubMed]
- Dejaegher, B.; Vander Heyden, Y. Experimental designs and their recent advances in set-up, data interpretation, and analytical applications. J. Pharm. Biomed. 2011, 56, 141–158. [Google Scholar] [CrossRef]
- Alalaiwe, A.; Fayed, M.H.; Alshahrani, S.M.; Alsulays, B.B.; Alshetaili, A.S.; Tawfeek, H.M.; Khafagy, E.S. Application of design of experiment approach for investigating the effect of partially pre-gelatinized starch on critical quality attributes of rapid orally disintegrating tablets. J. Drug Deliv. Sci. Tech. 2019, 49, 227–234. [Google Scholar] [CrossRef]
- Oka, S.; Kašpar, O.; Tokárová, V.; Sowrirajan, K.; Wu, H.; Khan, M.; Muzzio, F.; Štěpánek, F.; Ramachandran, R. A quantitative study of the effect of process parameters on key granule characteristics in a high shear wet granulation process involving a two component pharmaceutical blend. Adv. Powder Technol. 2015, 26, 315–322. [Google Scholar] [CrossRef]
- Takasaki, H.; Sakurai, A.; Katayama, T.; Matsuura, Y.; Ohyagi, N.; Mizoguchi, M.; Takano, J.; Wada, K.; Matsui, K.; Nagato, T.; et al. Importance of free water in controlling granule and tablet properties in a novel granulation method, green fluidized bed granulation (GFBG). Int. J. Pharm. 2019, 570, 118647. [Google Scholar] [CrossRef] [PubMed]
- Fayed, M.H.; Abdel-Rahman, S.I.; Alanazi, F.K.; Ahmed, M.O.; Tawfeek, H.M.; Al-Shdefat, R.I. New gentle-wing high-shear granulator: Impact of processing variables on granules and tablets characteristics of high-drug loading formulation using design of experiment approach. Drug Dev. Ind. Pharm. 2017, 43, 1584–1600. [Google Scholar] [CrossRef]
- Šantl, M.; Ilić, I.; Vrečer, F.; Baumgartner, S. A compressibility and compactibility study of real tableting mixtures: The impact of wet and dry granulation versus a direct tableting mixture. Int. J. Pharm. 2011, 414, 131–139. [Google Scholar] [CrossRef] [PubMed]
- Fayed, M.H.; Abdel-Rahman, S.I.; Alanazi, F.K.; Ahmed, M.O.; Tawfeek, H.M.; Ali, B.E. High shear granulation process: Assesing impact of formulation variables on granules and tablet characterestics of high drug loading formulation using design of experiment methodology. Act. Polon. Pharm. 2017, 74, 551–564. [Google Scholar]
- Arndt, O.-R.; Baggio, R.; Adam, A.K.; Harting, J.; Franceschinis, E.; Kleinebudde, P. Impact of Different Dry and Wet Granulation Techniques on Granule and Tablet Properties: A Comparative Study. J. Pharm. Sci. 2018, 107, 3143–3152. [Google Scholar] [CrossRef] [PubMed]
- Lee, A.R.; Kwon, S.Y.; Choi, D.H.; Park, E.S. Quality by Design (QbD) approach to optimize the formulation of a bilayer combination tablet (Telmiduo®) manufactured via high shear wet granulation. Int. J. Pharm. 2017, 534, 144–158. [Google Scholar] [CrossRef]
- Badawy, S.I.F.; Menning, M.M.; Gorko, M.A.; Gilbert, D.L. Effect of process parameters on compressibility of granulation manufactured in a high-shear mixer. Int. J. Pharm. 2000, 198, 51–61. [Google Scholar] [CrossRef]
- Badawy, S.I.F.; Narang, A.S.; LaMarche, K.R.; Subramanian, G.A.; Varia, S.A. Chapter 3—Mechanistic Basis for the Effects of Process Parameters on Quality Attributes in High Shear Wet Granulation. In Handbook of Pharmaceutical Wet Granulation; Narang, A.S., Badawy, S.I.F., Eds.; Academic Press: Cambridge, MA, USA, 2019; pp. 89–118. [Google Scholar]
- Basim, P.; Haware, R.V.; Dave, R.H. Tablet capping predictions of model materials using multivariate approach. Int. J. Pharm. 2019, 569, 118548. [Google Scholar] [CrossRef]
- Vercruysse, J.; Córdoba Díaz, D.; Peeters, E.; Fonteyne, M.; Delaet, U.; Van Assche, I.; De Beer, T.; Remon, J.P.; Vervaet, C. Continuous twin screw granulation: Influence of process variables on granule and tablet quality. Eur. J. Pharm. Biopharm. 2012, 82, 205–211. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ansari, M.A.; Stepanek, F. The effect of granule microstructure on dissolution rate. Powder Technol. 2008, 181, 104–114. [Google Scholar] [CrossRef]
- Khafagy, E.-S.; Fayed, M.H.; Alrabahi, S.H.; Gad, S.; Alshahrani, S.M.; Aldawsari, M. Defining design space for optimization of escitalopram ultra-fast melting tablet using suspension spray-coating technique: In-vitro and in-vivo evaluation. J. Drug Deliv. Sci. Tech. 2020, 57, 101631. [Google Scholar] [CrossRef]
- United States Pharmacopeia (USP 38-NF-33); The United States Pharmacopieial Convention: Rockville, MD, USA, 2015.
- Lotfy, H.; Mohamed, D.; Elshahed, M. Different mathematical processing of absorption, ratio and derivative spectra for quantification of mixtures containing minor component: An application to the analysis of the recently co-formulated antidiabetic drugs; canagliflozin and metformin. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2018, 189, 100–109. [Google Scholar] [CrossRef] [PubMed]


| Response | Model | p-Value | R2 | Adjusted R2 | Predicted R2 |
|---|---|---|---|---|---|
| Mean granule size (d50) | Linear | <0.0001 | 0.9872 | 0.9830 | 0.9700 |
| Percent fines | Linear | <0.0001 | 0.9816 | 0.9755 | 0.9621 |
| Distribution width | Linear | <0.0001 | 0.9787 | 0.9716 | 0.9480 |
| Bulk density | * 2FI | 0.0011 | 0.9510 | 0.9217 | 0.8011 |
| Angle of repose | 2FI | <0.0001 | 0.9917 | 0.9868 | 0.9558 |
| ** SD of weight variation | Linear | 0.0001 | 0.9531 | 0.9375 | 0.8913 |
| Breaking force | Linear | 0.0002 | 0.9381 | 0.9175 | 0.8353 |
| Friability | Linear | 0.0001 | 0.9487 | 0.9316 | 0.8565 |
| Disintegration time | Quadratic | 0.0003 | 0.9982 | 0.9953 | 0.9809 |
| Drug release at 30 min | Linear | 0.0003 | 0.9350 | 0.9134 | 0.8447 |
| Runs | Mean Granule Size (d50) (µm ± SD) | Percent Fines (% ± SD) | Distribution Width (span) | Bulk Density (gcm−3 ± SD) | Angle of Repose (Degree ± SD) |
|---|---|---|---|---|---|
| 1 | 117.12 ± 0.25 | 28.08 ± 0.013 | 3.64 ± 0.013 | 0.353 ± 0.013 | 32.11 ± 0.322 |
| 2 | 184.33 ± 0.21 | 26.51 ± 0.034 | 3.42 ± 0.023 | 0.365 ± 0.002 | 31.35 ± 0.535 |
| 3 | 206.11 ± 0.31 | 22.46 ± 0.012 | 2.74 ± 0.021 | 0.367 ± 0.025 | 31.13 ± 0.026 |
| 4 | 222.17 ± 0.26 | 20.61 ± 0.027 | 2.65 ± 0.022 | 0.357 ± 0.023 | 30.51 ± 0.411 |
| 5 | 246.32 ± 0.29 | 18.33 ± 0.037 | 2.41 ± 0.041 | 0.371 ± 0.006 | 29.23 ± 0.243 |
| 6 | 283.05 ± 0.34 | 12.72 ± 0.015 | 2.25 ± 0.036 | 0.376 ± 0.038 | 28.21 ± 0.334 |
| 7 | 312.45 ± 0.66 | 10.31 ± 0.024 | 1.94 ± 0.015 | 0.362 ± 0.009 | 28.54 ± 0.212 |
| 8 | 338.82 ± 0.34 | 4.16 ± 0.028 | 1.67 ± 0.034 | 0.377 ± 0.072 | 27.11 ± 0.722 |
| 9 | 379.14 ± 0.33 | 2.01 ± 0.024 | 1.26 ± 0.048 | 0.401 ± 0.006 | 26.23 ± 0.415 |
| Variables | Coefficient Estimate | Sum of Squares | Standard Error | F-Value | p-Value | 95 % CI * Low | 95 % CI High |
|---|---|---|---|---|---|---|---|
| Mean granule size (d50) (linear model) | |||||||
| Intercept | 254.39 | - | 3.58 | - | - | 245.64 | 263.14 |
| X1 | 87.14 | 455,562.02 | 4.38 | 395.83 | <0.0001 | 76.42 | 97.86 |
| X2 | 36.09 | 7816.36 | 4.38 | 67.91 | 0.0002 | 25.38 | 46.81 |
| Percent fines (linear model) | |||||||
| Intercept | 16.13 | - | 0.4897 | - | - | 14.93 | 17.33 |
| X1 | −10.10 | 611.45 | 0.5997 | 283.33 | <0.0001 | −11.56 | −8.63 |
| X2 | −3.63 | 79.28 | 0.5997 | 36.74 | 0.0009 | −5.10 | −2.17 |
| Distribution width (linear model) | |||||||
| Intercept | 2.44 | - | 0.0436 | - | - | 2.34 | 2.55 |
| X1 | −0.8217 | 4.05 | 0.0534 | 237.03 | <0.0001 | −0.9523 | −0.6911 |
| X2 | −0.3300 | 0.6534 | 0.0534 | 38.23 | 0.0008 | −0.4606 | −0.1994 |
| Bulk density (2FI model) | |||||||
| Intercept | 0.3699 | - | 0.0013 | - | - | 0.3665 | 0.3733 |
| X1 | 0.0092 | 0.0005 | 0.0016 | 32.12 | 0.0024 | 0.0050 | 0.0133 |
| X2 | 0.0120 | 0.0009 | 0.0016 | 55.05 | 0.0007 | 0.0078 | 0.0162 |
| X1 X2 | 0.0062 | 0.0002 | 0.0020 | 9.96 | 0.0252 | 0.0012 | 0.0113 |
| Angle of repose (2FI model) | |||||||
| Intercept | 29.38 | - | 0.0777 | - | - | 29.18 | 29.58 |
| X1 | −2.12 | 26.92 | 0.0951 | 496.13 | <0.0001 | −2.36 | −1.87 |
| X2 | −0.9317 | 5.21 | 0.0951 | 95.97 | 0.0002 | −1.18 | −0.6872 |
| X1 X2 | −0.3325 | 0.4422 | 0.1165 | 8.15 | 0.0356 | −0.6319 | −0.0331 |
| Runs | Weight (mg ± SD) | Thickness (mm ± SD) | Breaking Force (KP ± SD) | Friability (% ± SD) | Disintegration Time (min ± SD) | % Release after 30 min (% ± SD) |
|---|---|---|---|---|---|---|
| 1 | 399.55 ± 1.86 | 3.31 ± 0.014 | 7.92 ± 0.65 | 1.30 ± 0.03 | 9.34 ± 1.75 | 92.32 ± 3.15 |
| 2 | 397.83 ± 1.80 | 3.33 ± 0.006 | 7.89 ± 0.15 | 1.26 ± 0.11 | 9.27 ± 1.21 | 90.53 ± 2.15 |
| 3 | 400.91 ± 1.79 | 3.36 ± 0.005 | 7.31 ± 0.66 | 1.03 ± 0.06 | 10.09 ± 1.22 | 87.21 ± 3.34 |
| 4 | 399.71 ± 1.78 | 3.35 ± 0.03 | 7.89 ± 0.78 | 0.82 ± 0.02 | 10.65 ± 2.67 | 88.66 ± 2.55 |
| 5 | 399.82 ± 1.61 | 3.34 ± 0.004 | 7.33 ± 0.98 | 0.88 ± 0.04 | 12.14 ± 1.43 | 86.01 ± 2.97 |
| 6 | 398.15 ± 1.60 | 3.36 ± 0.007 | 6.89 ± 0.93 | 0.86 ± 0.05 | 13.38 ± 1.54 | 85.02 ± 3.88 |
| 7 | 398.35 ± 1.59 | 3.33 ± 0.006 | 7.12 ± 0.39 | 0.53 ± 0.01 | 16.86 ± 2.59 | 82.32 ± 3.97 |
| 8 | 397.61 ± 1.48 | 3.32 ± 0.005 | 6.86 ± 0.59 | 0.51 ± 0.02 | 18.47 ± 1.54 | 78.35 ± 4.61 |
| 9 | 401.22 ± 1.40 | 3.33 ± 0.03 | 6.22 ± 0.23 | 0.54 ± 0.01 | 20.29 ± 0.89 | 74.21 ± 4.15 |
| Variables | Coefficient Estimate | Sum of Squares | Standard Error | F-Value | p-Value | 95 % CI Low | 95 % CI High |
|---|---|---|---|---|---|---|---|
| SD of weight variation (linear model) | |||||||
| Intercept | 1.66 | - | 0.0132 | - | - | 1.62 | 1.69 |
| X1 | −0.1633 | 0.1601 | 0.0162 | 101.45 | <0.0001 | −0.2030 | −0.1237 |
| X2 | −0.0733 | 0.0323 | 0.0162 | 20.45 | 0.0040 | −0.1130 | −0.0337 |
| Breaking force (linear model) | |||||||
| Intercept | 7.27 | - | 0.0549 | - | - | 7.14 | 7.40 |
| X1 | −0.4867 | 1.42 | 0.0673 | 52.34 | 0.0004 | −0.6513 | −0.3221 |
| X2 | −0.4183 | 1.05 | 0.0673 | 38.67 | 0.0008 | −0.5829 | −0.2537 |
| Friability (linear model) | |||||||
| Intercept | 0.8589 | - | 0.0261 | - | - | 0.7949 | 0.9228 |
| X1 | −0.3350 | 0.06734 | 0.0320 | 109.57 | <0.0001 | −0.4133 | −0.2567 |
| X2 | −0.0367 | 0.0081 | 0.0320 | 1.31 | 0.2995 | −0.1150 | 0.0416 |
| Disintegration time (Quadratic model) | |||||||
| Intercept | 11.96 | - | 0.2135 | - | - | 11.28 | 12.64 |
| X1 | 4.49 | 120.87 | 0.1169 | 1471.88 | <0.0001 | 4.11 | 4.86 |
| X2 | 1.15 | 7.96 | 0.1169 | 96.98 | 0.0022 | 0.7795 | 1.52 |
| X1X2 | 0.6700 | 1.80 | 0.1432 | 21.88 | 0.0185 | 0.2142 | 1.12 |
| X12 | 2.00 | 7.97 | 0.2026 | 97.17 | 0.0022 | 1.35 | 2.64 |
| X22 | 0.1417 | 0.0401 | 0.2026 | 0.4891 | 0.5347 | −0.5030 | 0.7863 |
| Percent release at 30 min (linear model) | |||||||
| Intercept | 84.96 | - | 0.5714 | - | - | 83.56 | 86.36 |
| X1 | −5.86 | 206.27 | 0.6998 | 70.21 | 0.0002 | −7.58 | −4.15 |
| X2 | −2.81 | 47.38 | 0.6998 | 16.12 | 0.0070 | −4.52 | −1.10 |
| Variables | Target | Range | Weight | Importance Coefficient |
|---|---|---|---|---|
| Input | ||||
| * Granulation fluid | In range | 1–4% | 1 | − |
| Wet massing time | In range | 1–5 min | 1 | − |
| Output | ||||
| SD of weight variation | In range | 1.4–1.86 | 1 | − |
| Breaking force | In range | 6.22–7.92% | 1 | − |
| Friability | 0.6% | 0.51–1.3% | 1 | ++++ # |
| Disintegration time | 10 min | 9.27–20.29 min | 1 | +++++ # |
| Percent release at 30 min | 85% | 74.21–92.32% | 1 | +++++ # |
| Responses | Predicted Values | Observed Values (Mean ± SD) | Relative Error (%) |
|---|---|---|---|
| SD of weight variation | 1.63 | 1.58 | 3.06 |
| Breaking force (KP) | 7.39 | 7.15 ± 1.83 | 3.24 |
| Friability (%) | 0.69 | 0.71 ± 0.95 | −2.89 |
| Disintegration time (min) | 14.00 | 13.56 ± 0.76 | 3.14 |
| Percent release at 30 min | 84.21 | 87.25 ± 2.13 | −3.61 |
| Coded Levels | Granulation Fluid * (%) | Wet Massing Time (min) |
|---|---|---|
| −1 | 1 | 1 |
| 0 | 2.5 | 2 |
| 1 | 4 | 3 |
| Run | Granulation Fluid * (% w/w) | Wet Massing Time (min) |
|---|---|---|
| 1 | 1 | 1 |
| 2 | 1 | 2 |
| 3 | 1 | 3 |
| 4 | 2.5 | 1 |
| 5 | 2.5 | 2 |
| 6 | 2.5 | 3 |
| 7 | 4 | 1 |
| 8 | 4 | 2 |
| 9 | 4 | 3 |
| Ingredients | % w/w |
|---|---|
| Canagliflozin | 75 |
| Polyvinylpyrrolidone (K25) | 10 |
| Sodium starch glycolate | 4 |
| Colloidal silicon dioxide | 1.5 |
| Microcrystalline cellulose | 8.5 |
| Magnesium stearate | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Almutairy, B.K.; Khafagy, E.-S.; Alalaiwe, A.; Aldawsari, M.F.; Alshahrani, S.M.; Alsulays, B.B.; Alshetaili, A.S.; Alshehri, S.M.; Fayed, M.H. Enhancing the Poor Flow and Tableting Problems of High Drug-Loading Formulation of Canagliflozin Using Continuous Green Granulation Process and Design-of-Experiment Approach. Pharmaceuticals 2020, 13, 473. https://doi.org/10.3390/ph13120473
Almutairy BK, Khafagy E-S, Alalaiwe A, Aldawsari MF, Alshahrani SM, Alsulays BB, Alshetaili AS, Alshehri SM, Fayed MH. Enhancing the Poor Flow and Tableting Problems of High Drug-Loading Formulation of Canagliflozin Using Continuous Green Granulation Process and Design-of-Experiment Approach. Pharmaceuticals. 2020; 13(12):473. https://doi.org/10.3390/ph13120473
Chicago/Turabian StyleAlmutairy, Bjad K., El-Sayed Khafagy, Ahmed Alalaiwe, Mohammed F. Aldawsari, Saad M. Alshahrani, Bader B. Alsulays, Abdullah S. Alshetaili, Sultan M. Alshehri, and Mohamed H. Fayed. 2020. "Enhancing the Poor Flow and Tableting Problems of High Drug-Loading Formulation of Canagliflozin Using Continuous Green Granulation Process and Design-of-Experiment Approach" Pharmaceuticals 13, no. 12: 473. https://doi.org/10.3390/ph13120473
APA StyleAlmutairy, B. K., Khafagy, E.-S., Alalaiwe, A., Aldawsari, M. F., Alshahrani, S. M., Alsulays, B. B., Alshetaili, A. S., Alshehri, S. M., & Fayed, M. H. (2020). Enhancing the Poor Flow and Tableting Problems of High Drug-Loading Formulation of Canagliflozin Using Continuous Green Granulation Process and Design-of-Experiment Approach. Pharmaceuticals, 13(12), 473. https://doi.org/10.3390/ph13120473







