Appendix A.1
The model is subject to . The Hessian matrix of is negative definite and the profit function of is concave of and . Solving KKT conditions , , and , we can get three feasible decisions according to Complementary Slackness Theorem.
Decision MA: and .
Solving and the first order conditions give , and . and give and . . Therefore, Decision MA exists when .
Decision MB: and .
Solving the first order conditions gives and . gives , , and , respectively. . . Therefore, Decision MB exists when .
Decision MC: and .
Solving and the first order conditions give , and . and give and , respectively. Therefore, Decision MC exists when .
Appendix A.2
The supplier’s profit function is .
Let , and . .
Decision SA:
When the supplier anticipates that the manufacturer will adopt decision MA, then the corresponding profit function of the supplier is , which subjects to . The Hessian matrix is negative definite and the profit function is concave. Solving KKT conditions , , and , we get one feasible decision according to Complementary Slackness Theorem.
and . Solving the first order conditions gives and . and give and , respectively. . Therefore, Decision S-A exists when .
Decision SB:
When the supplier anticipates that the manufacturer will adopt decision MB, then the corresponding profit function of the supplier is , which subjects to . The Hessian matrix is negative definite and the profit function is concave. Solving the KKT conditions , , and , we get three feasible decisions according to Complementary Slackness Theorem.
Decision SB-1: and .
Solving the first order conditions gives , and . and give and , respectively. . Therefore, Decision SB-1 exists when .
Decision SB-2: and .
Solving the first order conditions gives and . and give and , respectively. . Therefore, Decision SB-2 exists when .
Decision SB-3: and
Solving the first order conditions gives , and . and give and , respectively. . Therefore, Decision SB-3 exists when .
Decision SC:
When the supplier anticipates that the manufacturer will adopt decision MC, then the corresponding profit function of the supplier is , which subjects to . The Hessian matrix is negative definite, and the profit function is concave. Solving the KKT conditions , , and , we get one feasible decision according to Complementary Slackness Theorem.
and . Solving the first order conditions gives and . and give and , respectively. . Therefore, Decision S-C exists when .
Substitute the supplier’s optimal interchangeable level of key component and wholesale price into the profits, then we can obtain the supplier’s and manufacturer’s profits (
Table A1).
Table A1.
The optimal profits.
Table A1.
The optimal profits.
| Decision | | |
|---|
| DA | | |
| DB-1 | | |
| DB-2 | | |
| DB-3 | | |
| DC | | |
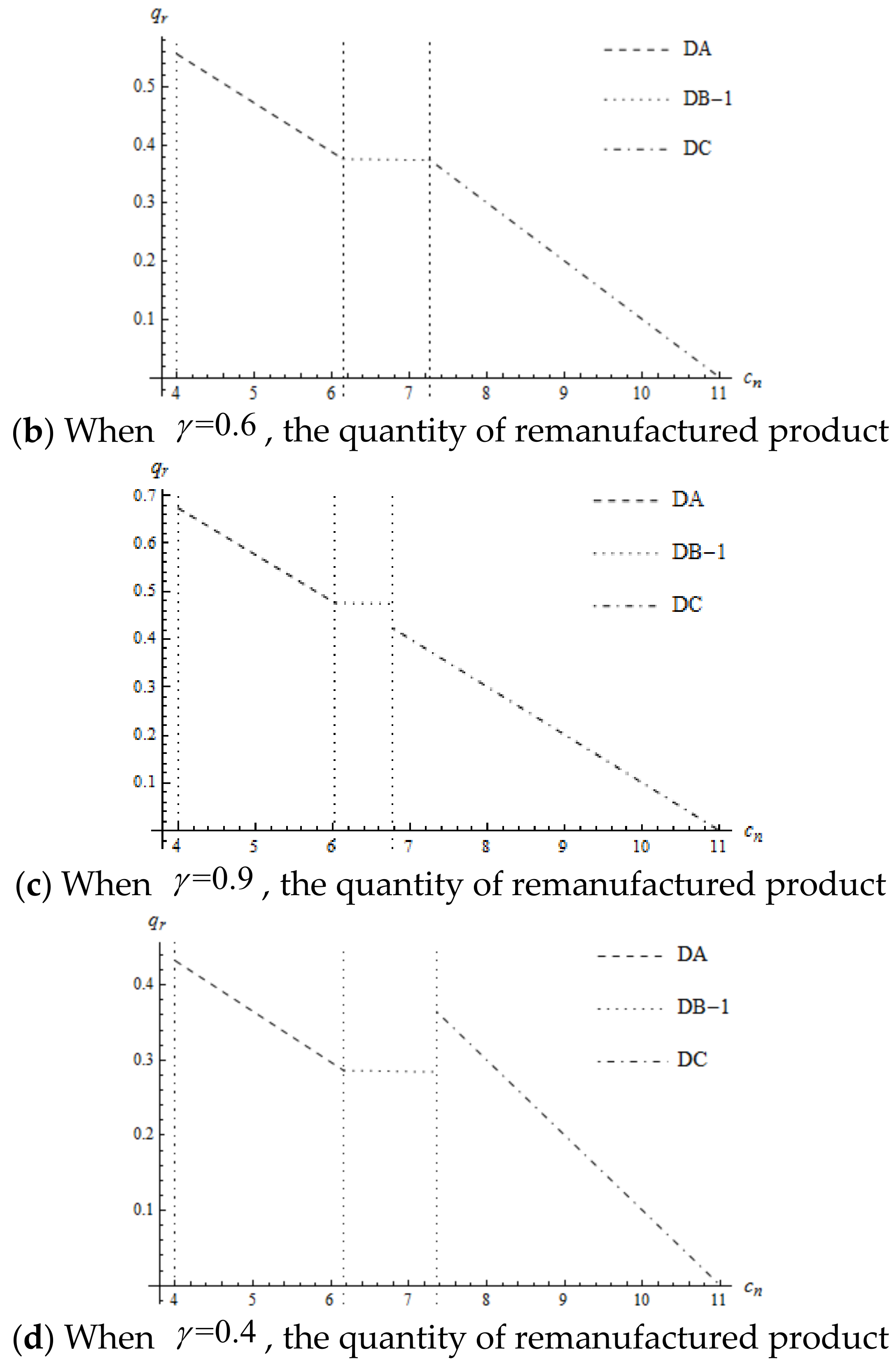
Compare boundary points of each decision, the results are as follows:
When , then .
When , then .
When , then .
(i)
When , .
When , let , . When , ; , .
When , .
When , .
(ii)
When , .
When , .
When , if , . When , ; ,.
When , .
When , .
(iii)
When , .
When , .
When , if , . If , if . When , ; , .
When , if , . if , if , . When , ; , .
When , .
When , .