Erosion Failure of a Soil Slope by Heavy Rain: Laboratory Investigation and Modified GA Model of Soil Slope Failure
Abstract
:1. Introduction
2. Experimental Test
2.1. Experimental Materials and Facilities
2.2. Experiment Results and Discussion
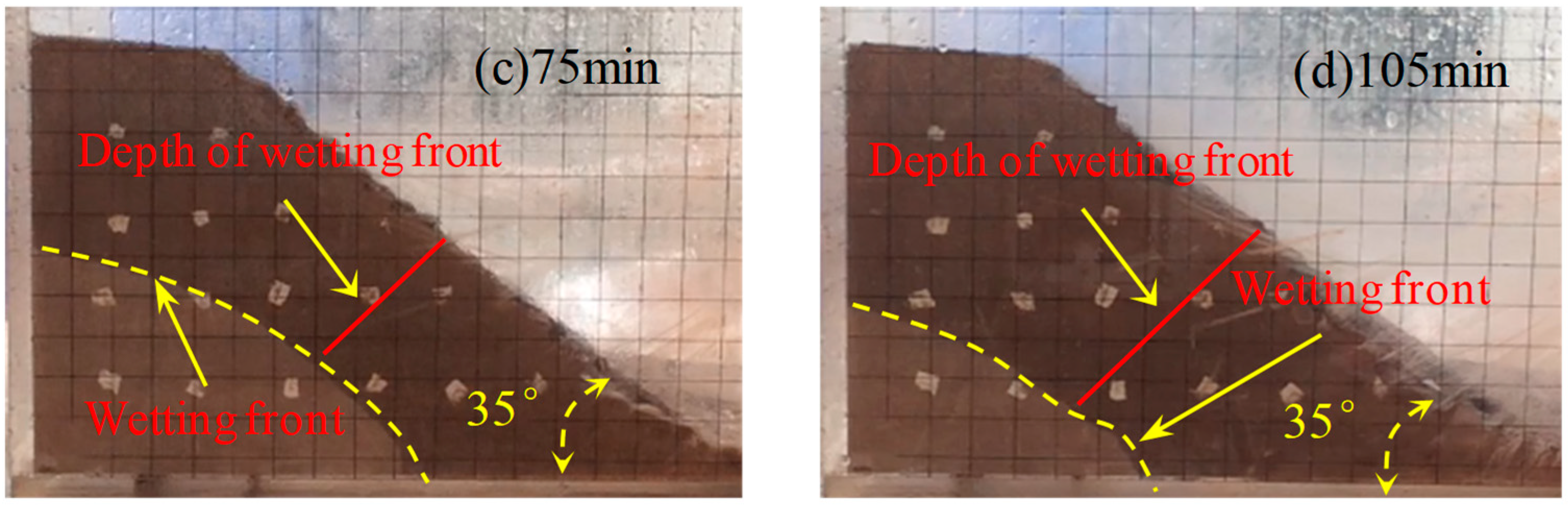
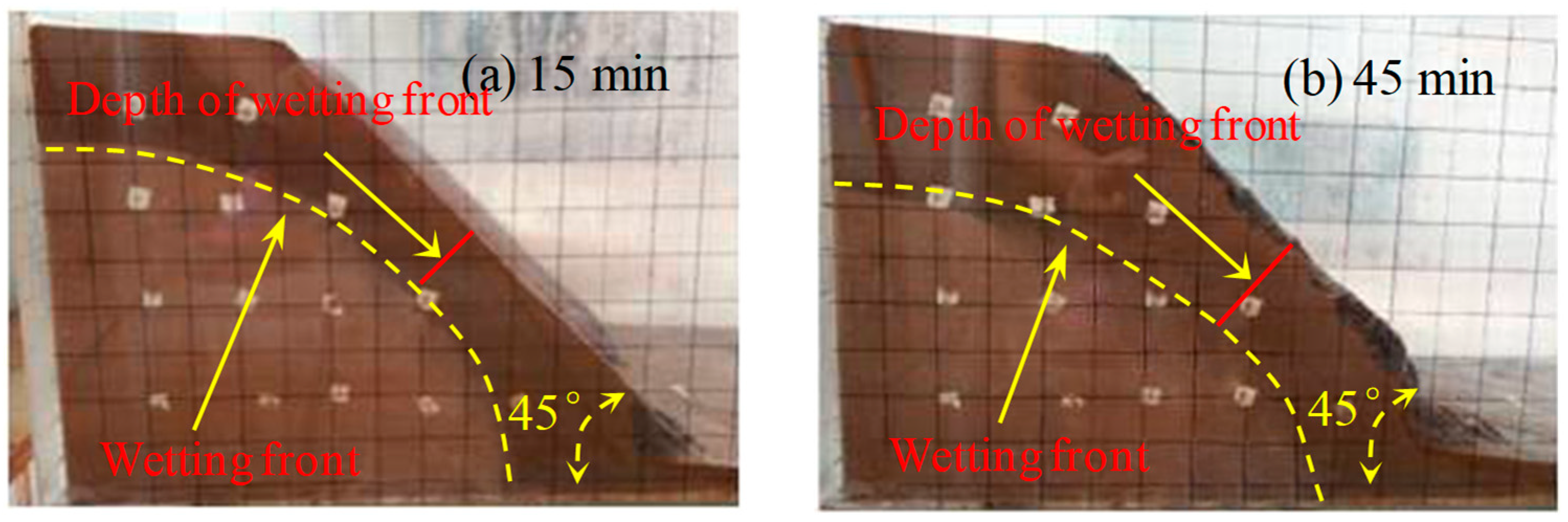
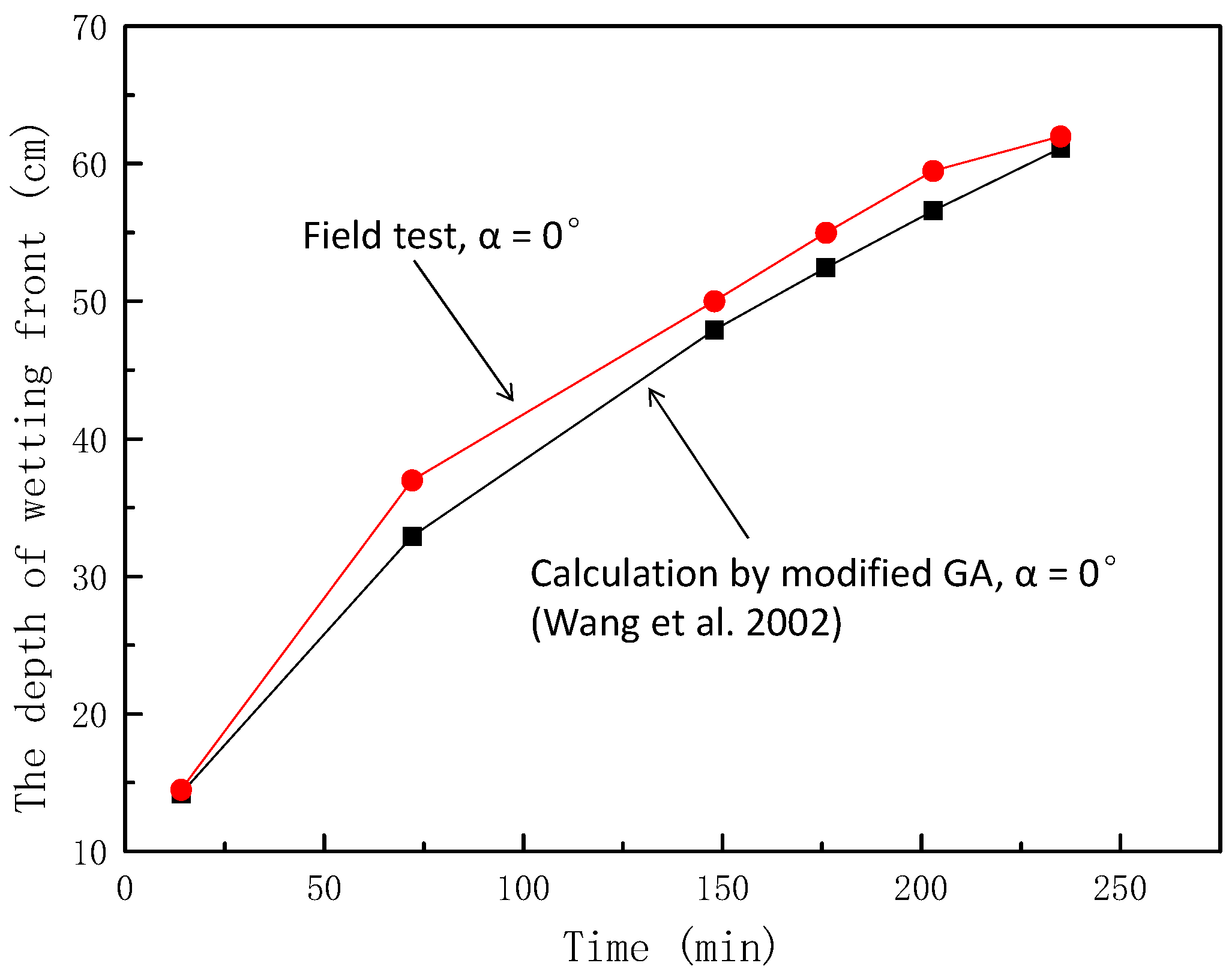
2.2.1. Rainfall Infiltration
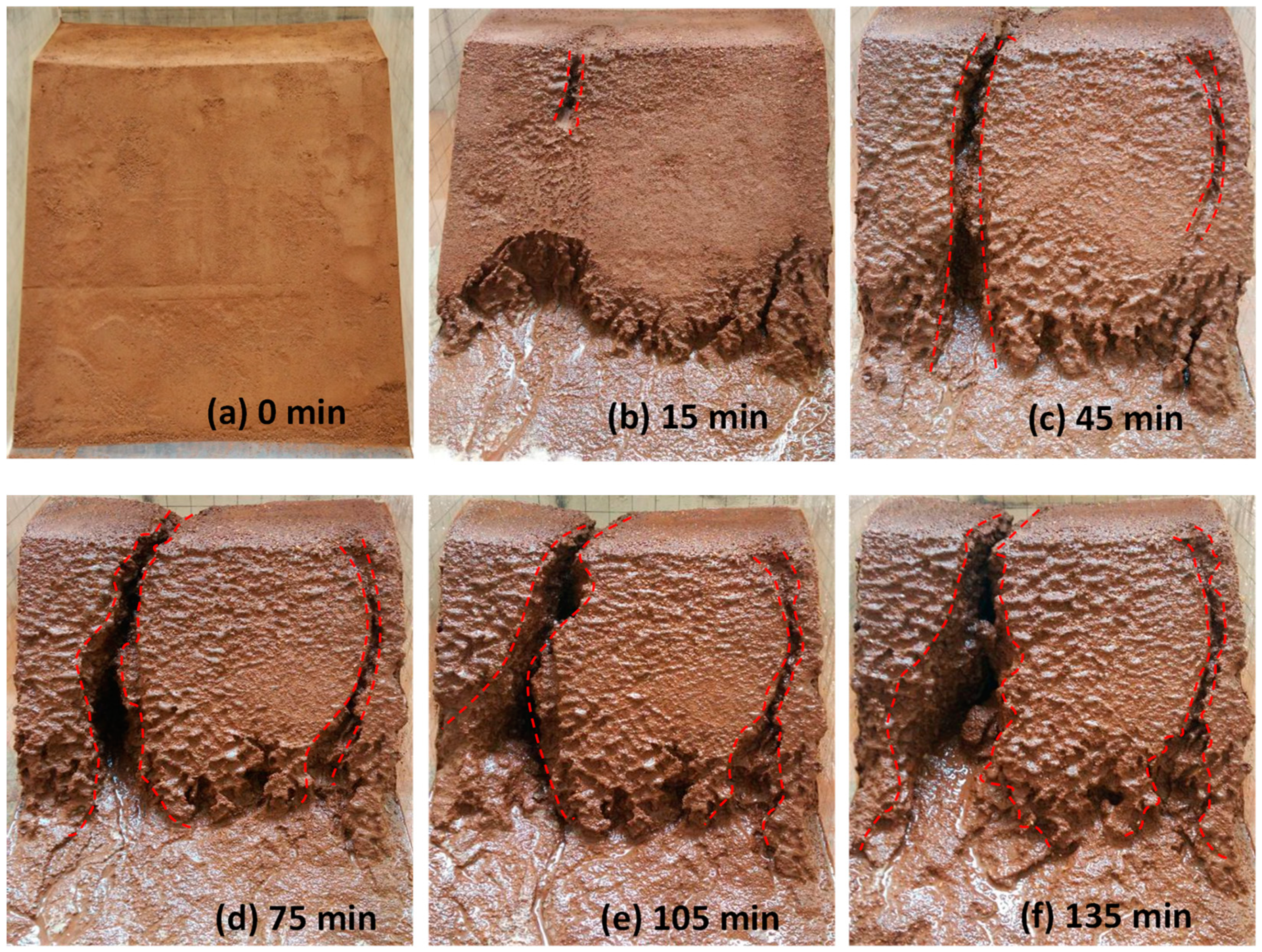
2.2.2. Runoff and Soil Erosion
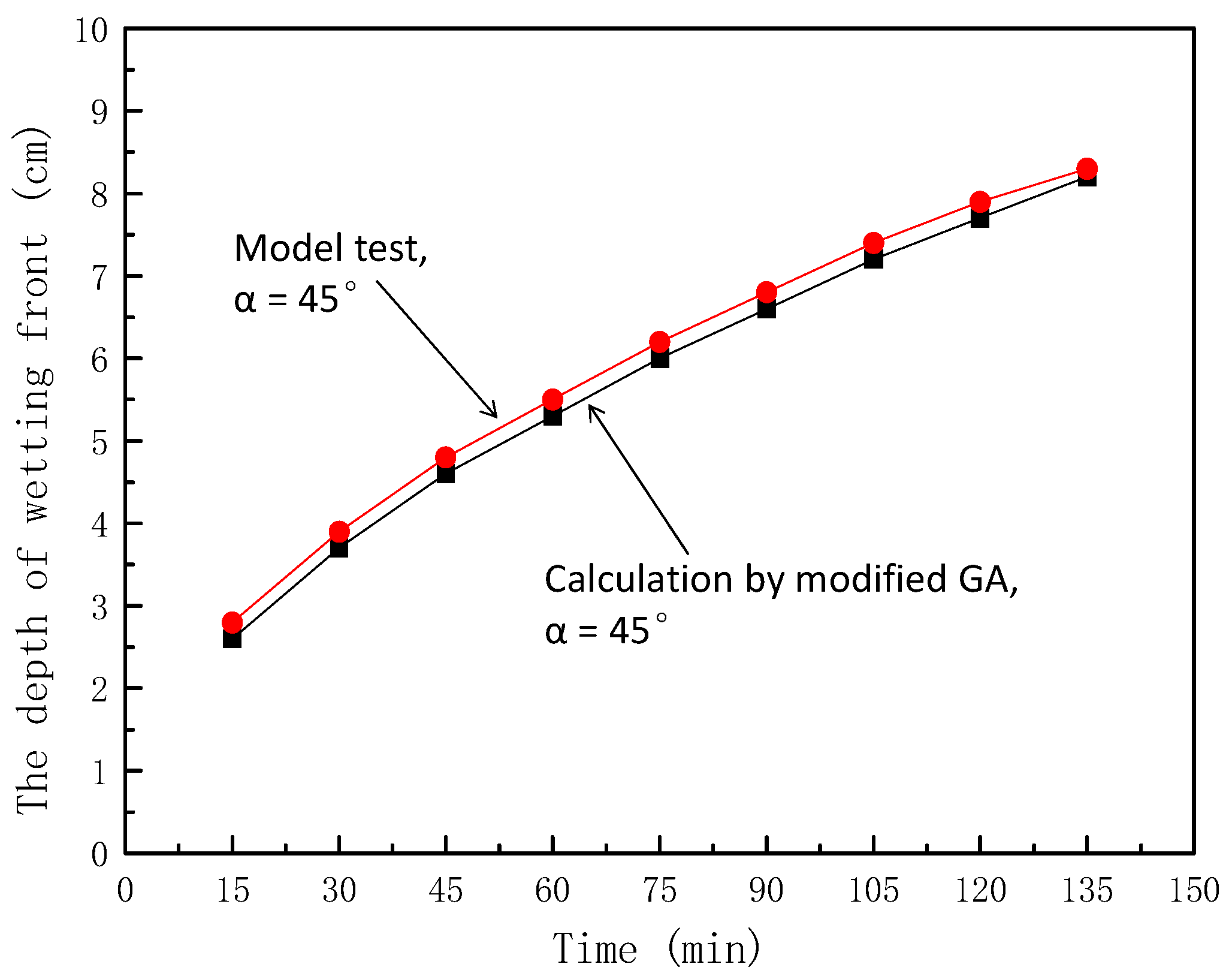
3. Rainfall Infiltration Model of the Soil Slope
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Liu, X.; Yan, E. Nonlinear method for evaluating the landslide stability. China Saf. Sci. J. 2003, 13, 34–36. [Google Scholar]
- Crosta, G.B.; Frattini, P. Rainfall-induced landslides and debris flows. Hydrol. Process. 2008, 22, 473–477. [Google Scholar] [CrossRef]
- Santoso, A.M.; Phoon, K.K.; Quek, S.T. Effects of soil spatial variability on rainfall-induced landslides. Comput. Struct. 2011, 89, 893–900. [Google Scholar] [CrossRef]
- Bui, D.T.; Ho, T.C.; Pradhan, B.; Pham, B.T.; Nhu, V.H.; Revhaug, I. GIS-based modeling of rainfall-induced landslides using data mining-based functional trees classifier with AdaBoost, Bagging, and MultiBoost ensemble frameworks. Environ. Earth Sci. 2016, 75, 1101. [Google Scholar]
- Himada, K.; Fujii, H.; Mcrii, T. Stability of unsaturated slopes considering changes of matric suction. Unsaturated Soils 1995, 1, 293–299. [Google Scholar]
- Gasmo, J.M.; Rahardjo, H.; Leong, E.C. Infiltration effects on stability of a residual soil slope. Comput. Geotech. 2000, 26, 145–165. [Google Scholar] [CrossRef]
- Chen, H.; Lee, C.F. A dynamic model for rainfall-induced landslides on natural slopes. Geomorphology 2003, 51, 269–288. [Google Scholar] [CrossRef]
- Chen, R.H.; Chen, H.P.; Chen, K.S.; Zhung, H.B. Simulation of a slope failure induced by rainfall infiltration. Environ. Geol. 2009, 58, 943–952. [Google Scholar] [CrossRef]
- Camera, C.; Masetti, M.; Apuani, T. Rainfall, infiltration, and groundwater flow in a terraced slope of Valtellina (Northern Italy): Field data and modelling. Environ. Earth Sci. 2012, 65, 1191–1202. [Google Scholar] [CrossRef]
- Chen, Y.L.; Irfan, M.; Uchimura, T.; Wu, Y.; Yu, F.W. Development of elastic wave velocity threshold for rainfall-induced landslide prediction and early warning. Landslides 2019. [Google Scholar] [CrossRef]
- Chen, Y.L.; Uchimura, T.; Irfan, M.; Huang, D.; Xie, J.R. Detection of water infiltration and deformation of unsaturated soils by elastic wave velocity. Landslides 2017, 14, 1715–1730. [Google Scholar] [CrossRef]
- Chen, Y.L.; Irfan, M.; Uchimura, T.; Nie, W.; Cheng, G.W. Elastic wave velocity monitoring as an emerging technique for rainfall-induced landslide prediction. Landslides 2018, 15, 1155–1172. [Google Scholar] [CrossRef]
- Chen, Y.L.; Irfan, M.; Uchimura, T.; Zhang, K. Feasibility of using elastic wave velocity monitoring for early warning of rainfall-induced slope failure. Sensors 2018, 18, 997. [Google Scholar] [CrossRef]
- Irfan, M.; Uchimura, T.; Chen, Y.L. Effects of soil deformation and saturation on elastic wave velocities in relation to prediction of rain-induced landslides. Eng. Geol. 2017, 230, 84–94. [Google Scholar] [CrossRef]
- Rahardjo, H.; Ong, T.H.; Rezaur, R.B.; Leong, E.C. Factors controlling instability of homogeneous soil slopes under rainfall. J. Geotech. Geoenviron. Eng. 2007, 133, 1532–1543. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, Y.; Han, D.; Mo, H. Case Study on Influence of Rainfall Permeation on Slope Stability. Chin. J. Rock Mech. Eng. 2004, 23, 920–924. [Google Scholar]
- Niu, W.; Ye, W.; Liu, S.; Yu, H. Limit analysis of a soil slope considering saturated-unsaturated seepage. Rock Soil Mech. 2009, 30, 2477–2481. [Google Scholar]
- Li, H.; Sun, H.; Sun, X.; Shang, Y. Influence of rainfall infiltration on slopes by physical model test. Chin. J. Geotech. Eng. 2009, 31, 589594. [Google Scholar]
- Zhan, L.; Wu, H.; Bao, C.; Gong, B. Artificial rainfall infiltration tests on a well-instrumented unsaturated expansive soil slope. Rock Soil Mech. 2003, 24, 151–158. [Google Scholar]
- Wang, R.; Yan, S.; Deng, W. Analysis of seepage stability of high filled embankment slope due to rainfall infiltration. China J. Highw. Transp. 2004, 17, 25–30. [Google Scholar]
- Rong, G.; Wang, S.; Wang, E.; Wang, J. Stability analysis of Yuanmo highway slope under intensive rainfall. Chin. J. Rock Mech. Eng. 2008, 27, 704–711. [Google Scholar]
- Wang, D.; Tang, H.; Li, C.; Ge, Y.; Yi, X. Stability analysis of colluvial landslide due to heavy rainfall. Rock Soil Mech. 2016, 37, 439–445. [Google Scholar]
- Pan, C. Study on Stability and Evaluation of Safety Influencing Factors of Reinforced Embankment under Heavy Rainfall; Chongqing University of Science and Technology: Chongqing, China, 2018. [Google Scholar]
- Zhang, J.; Han, T.; Dou, H. Stability of loess slope considering infiltration zonation. J. Cent. South Univ. (Sci. Technol.) 2014, 45, 4355–4361. [Google Scholar]
- Wang, W.; Wang, Z.; Wang, Q.; Zhang, J. Improvement and evaluation of the Green-Ampt model in loess soil. J. Hydraul. Eng. 2003, 35, 30–35. [Google Scholar]
- Wang, W.; Wang, Q.; Zhang, J.; Wang, Z.R. Soil hydraulic properties and correlation in qingwangchuan area of Gansu province. Sci. Soil Water Conserv. 2002, 41, 110–113. [Google Scholar]








| Moisture ω (%) | Bulk Density ρs (g·cm−3) | Void Ratio e | Saturation Sr | Liquid Limit wL | Plastic Limit Wp | Cohesive Force c (kPa) | Internal Friction Angle φ(°) |
|---|---|---|---|---|---|---|---|
| 12.5 | 2.73 | 0.8 | 0.414 | 32.8 | 23.56 | 23.9 | 12.51 |
| Test Number | Slope Dimension (cm) | Slope Angles α (°) | Rainfall (mm/min) |
|---|---|---|---|
| 1 | 45 × 35 × 20 | 35 | 1.7 |
| 2 | 45 × 35 × 20 | 40 | 1.7 |
| 3 | 45 × 35 × 20 | 45 | 1.7 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jing, X.; Chen, Y.; Pan, C.; Yin, T.; Wang, W.; Fan, X. Erosion Failure of a Soil Slope by Heavy Rain: Laboratory Investigation and Modified GA Model of Soil Slope Failure. Int. J. Environ. Res. Public Health 2019, 16, 1075. https://doi.org/10.3390/ijerph16061075
Jing X, Chen Y, Pan C, Yin T, Wang W, Fan X. Erosion Failure of a Soil Slope by Heavy Rain: Laboratory Investigation and Modified GA Model of Soil Slope Failure. International Journal of Environmental Research and Public Health. 2019; 16(6):1075. https://doi.org/10.3390/ijerph16061075
Chicago/Turabian StyleJing, Xiaofei, Yulong Chen, Changshu Pan, Tianwei Yin, Wensong Wang, and Xiaohua Fan. 2019. "Erosion Failure of a Soil Slope by Heavy Rain: Laboratory Investigation and Modified GA Model of Soil Slope Failure" International Journal of Environmental Research and Public Health 16, no. 6: 1075. https://doi.org/10.3390/ijerph16061075
APA StyleJing, X., Chen, Y., Pan, C., Yin, T., Wang, W., & Fan, X. (2019). Erosion Failure of a Soil Slope by Heavy Rain: Laboratory Investigation and Modified GA Model of Soil Slope Failure. International Journal of Environmental Research and Public Health, 16(6), 1075. https://doi.org/10.3390/ijerph16061075






