Field Study of the Interior Noise and Vibration of a Metro Vehicle Running on a Viaduct: A Case Study in Guangzhou
Abstract
:1. Introduction
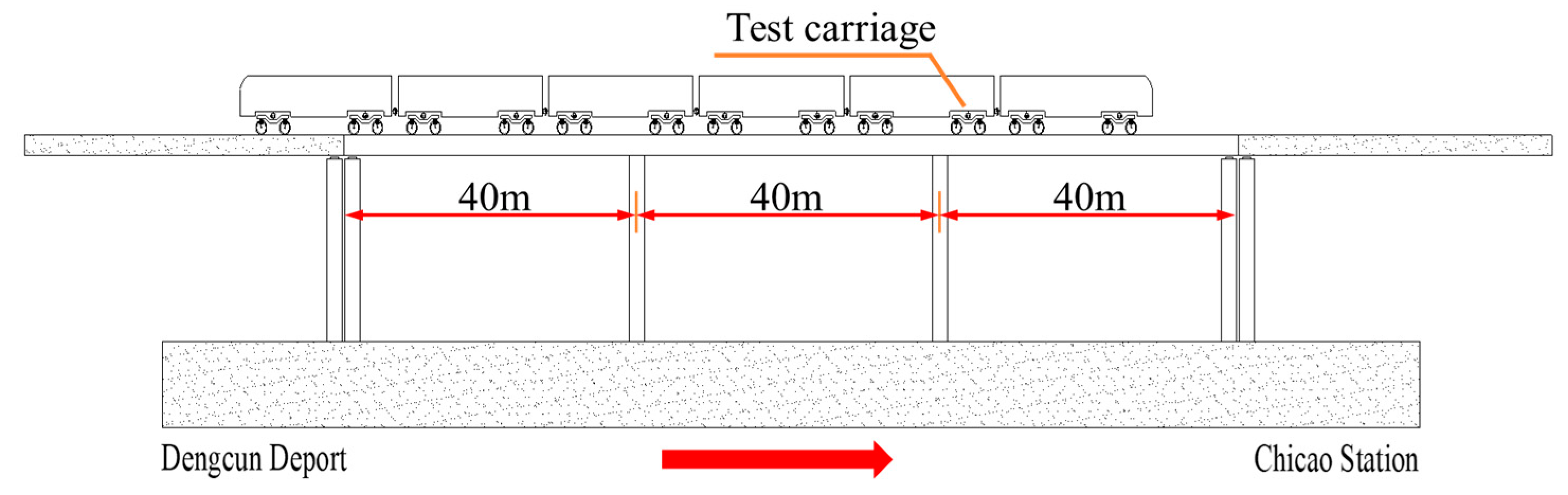
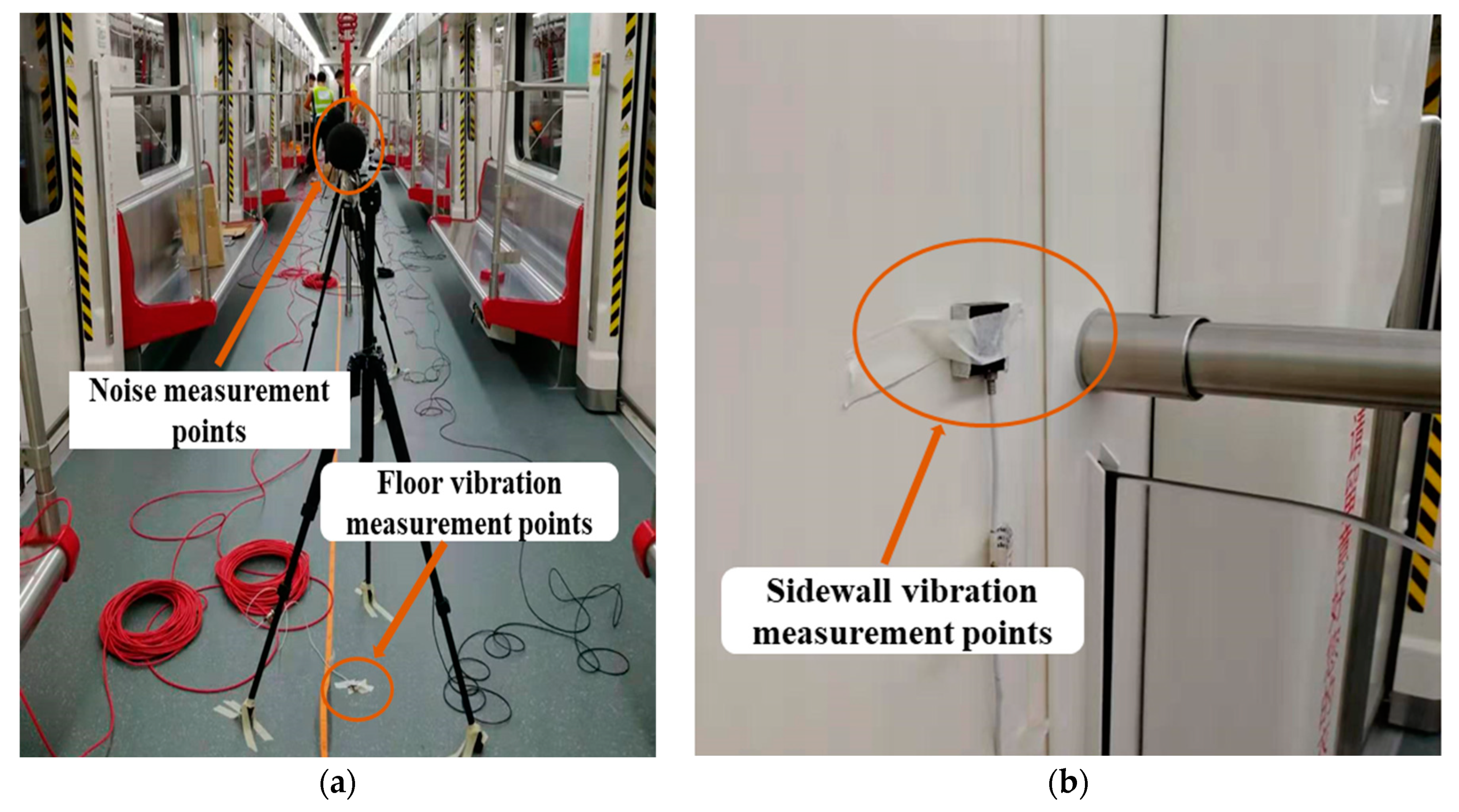
2. Description of the Measurements
2.1. Data Analysis Methods
2.2. Rail Condition
2.3. Continuous Rigid-Frame Box-Girder Bridge
2.4. Metro Vehicle
3. Results and Discussion
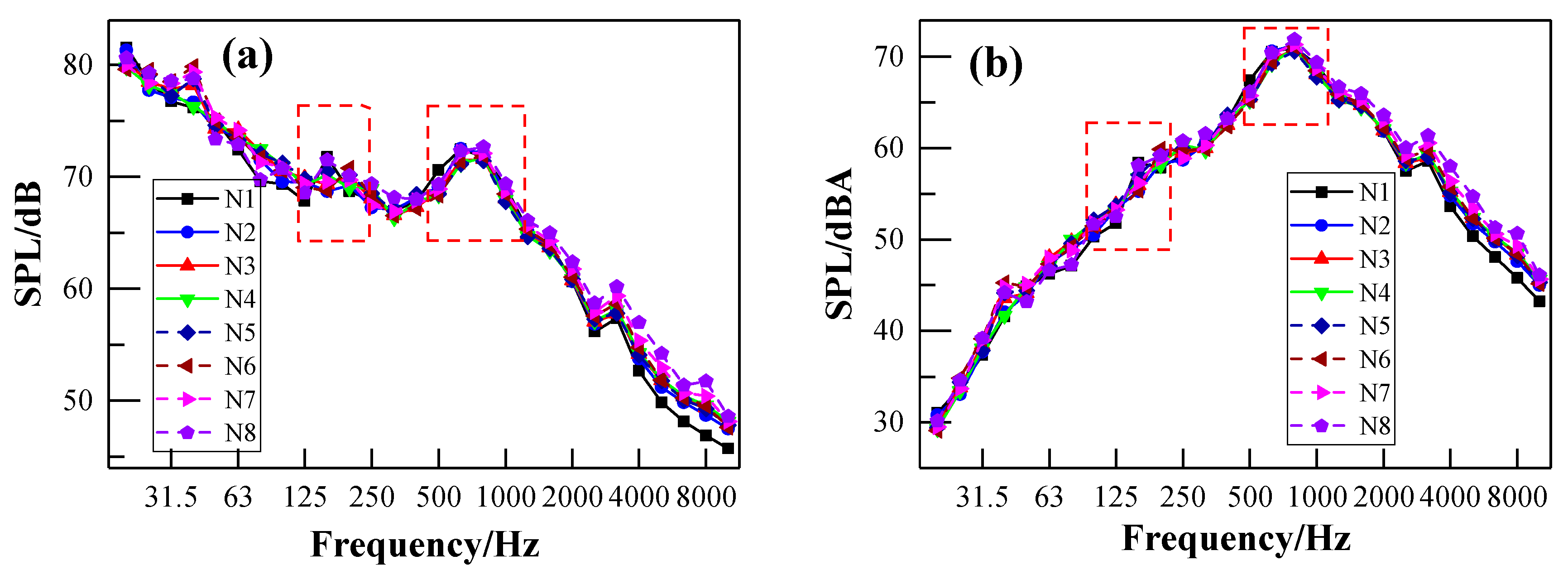
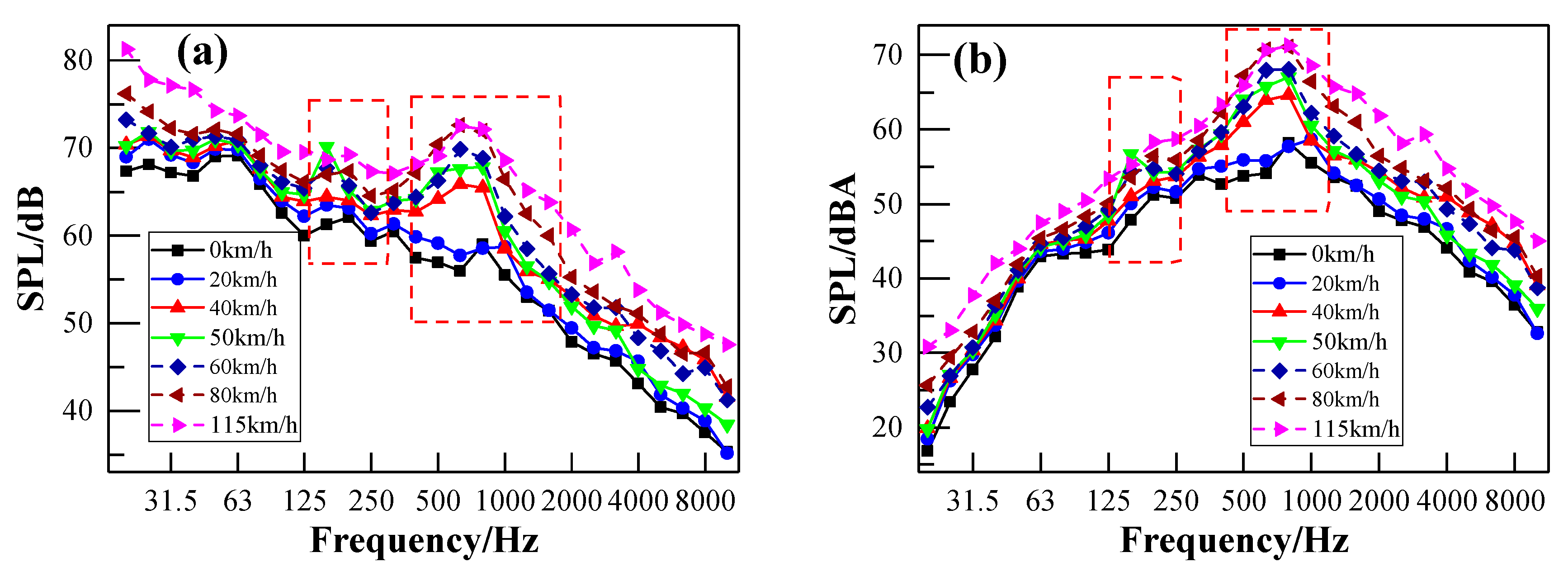
3.1. Interior Noise Spectra of the Metro Vehicle
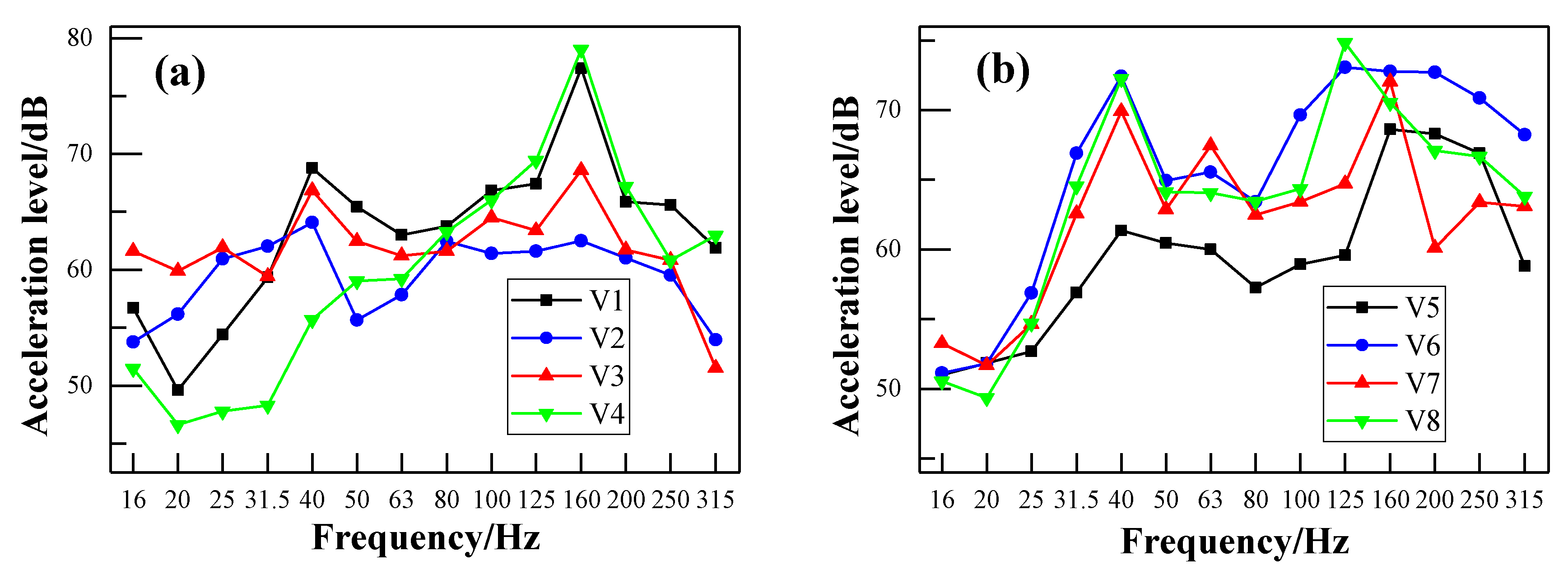
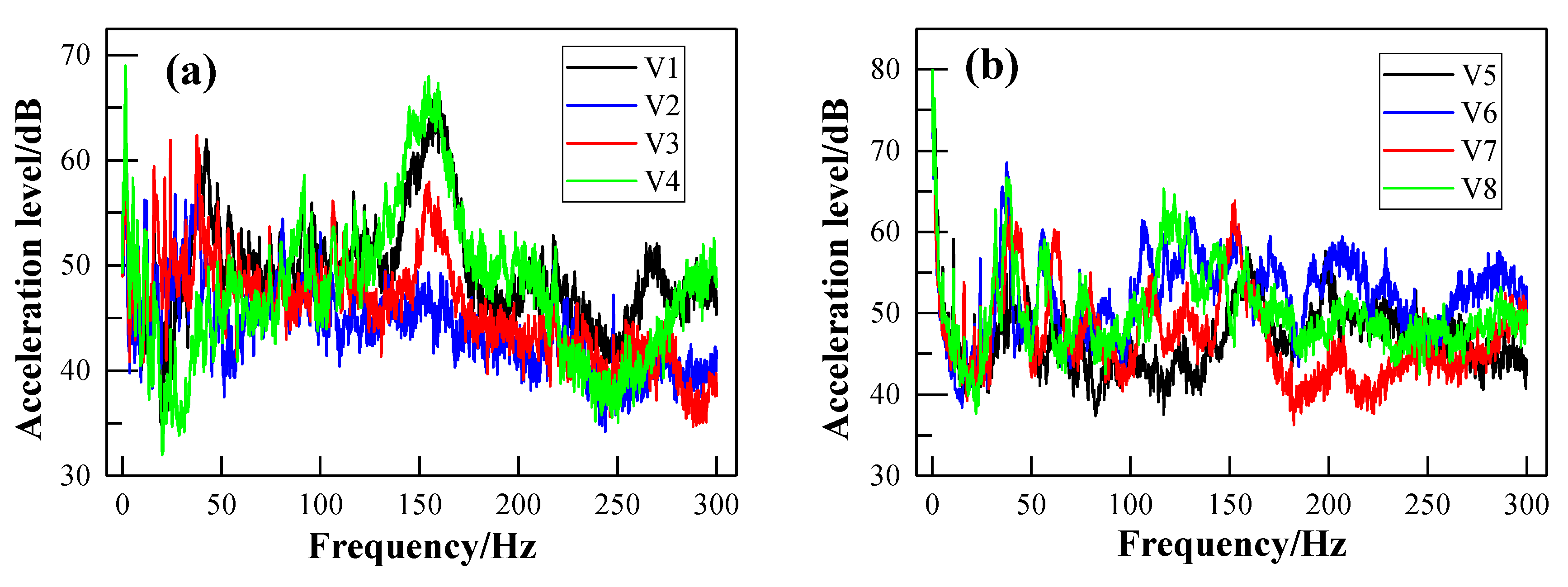
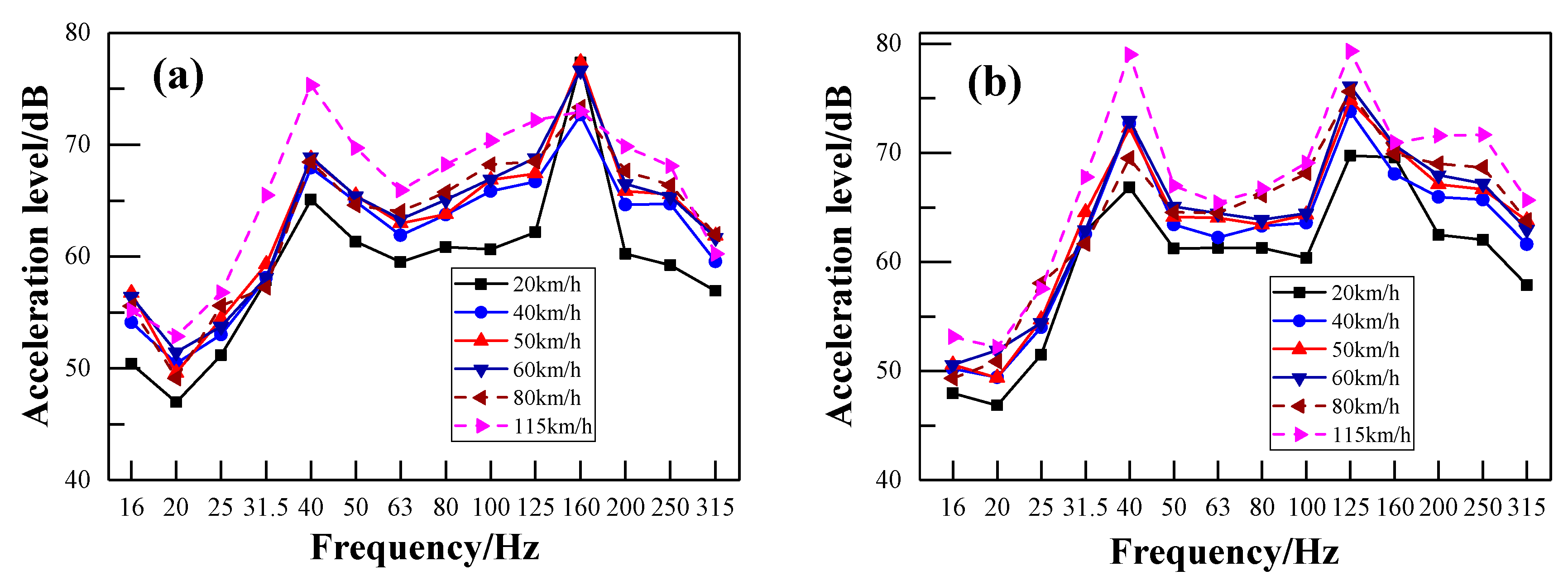
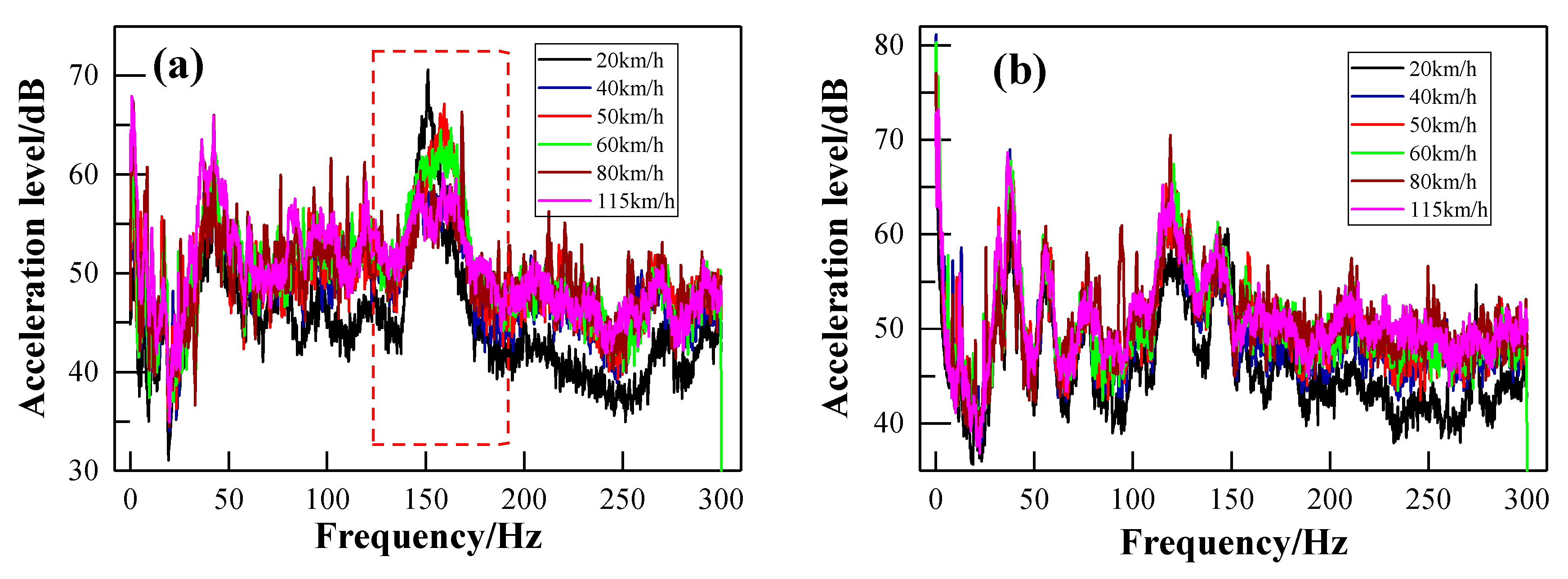
3.2. Vibration Spectra of the Metro Vehicle
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- China Urban Rail Transit Association. Report on statistics and analysis of urban rail transit in 2018. Urban Rail Transit 2019, 38, 18–36. [Google Scholar]
- Licitra, G.; Fredianelli, L.; Petri, D.; Vigotti, M.A. Annoyance evaluation due to overall railway noise and vibration in Pisa urban areas. Sci. Total Environ. 2016, 568, 1315–1325. [Google Scholar] [CrossRef]
- Bunn, F.; Zannin, P.H.T. Assessment of railway noise in an urban setting. Appl. Acoust. 2016, 104, 16–23. [Google Scholar] [CrossRef]
- Licitra, G.; Gallo, P.; Rossi, E.; Brambilla, G. A novel method to determine multiexposure priority indices tested for pisa action plan. Appl. Acoust. 2011, 72, 505–510. [Google Scholar] [CrossRef]
- Cueto, J.L.; Petrovici, A.M.; Hernández, R.; Fernández, F. Analysis of the impact of bus signal priority on urban noise. Acta Acust. United Acust. 2017, 103, 561–573. [Google Scholar] [CrossRef]
- Licitra, G.; Cerchiai, M.; Teti, L.; Ascari, E.; Bianco, F.; Chetoni, M. Performance Assessment of Low-Noise Road Surfaces in the Leopoldo Project: Comparison and Validation of Different Measurement Methods. Coatings 2015, 5, 3–25. [Google Scholar] [CrossRef]
- Ruiz-Padillo, A.; Ruiz, D.P.; Torija, A.J.; Ramos-Ridao, Á. Selection of suitable alternatives to reduce the environmental impact of road traffic noise using a fuzzy multi-criteria decision model. Environ. Impact Assess. Rev. 2016, 61, 8–18. [Google Scholar] [CrossRef] [Green Version]
- Gagliardi, P.; Teti, L.; Licitra, G. A statistical evaluation on flight operational characteristics affecting aircraft noise during take-off. Appl. Acoust. 2018, 134, 8–15. [Google Scholar] [CrossRef]
- Iglesias-Merchan, C.; Diaz-Balteiro, L.; Solino, M. Transportation planning and quiet natural areas preservation: Aircraft overflights noise assessment in a national park. Transp. Res. Part D Transp. Environ. 2015, 41, 1–12. [Google Scholar] [CrossRef]
- Michaud, D.S.; Feder, K.; Keith, S.E.; Voicescu, S.A.; Marro, L.; Than, J.; van den Berg, F. Exposure to wind turbine noise: Perceptual responses and reported health effects. J. Acoust. Soc. Am. 2016, 139, 1443–1454. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fredianelli, L.; Gallo, P.; Licitra, G.; Carpita, S. Analytical assessment of wind turbine noise impact at receiver by means of residual noise determination without the wind farm shutdown. Noise Control Eng. J. 2017, 65, 417–433. [Google Scholar] [CrossRef]
- Bolognese, M.; Fidecaro, F.; Palazzuoli, D.; Licitra, G. Port noise and complaints in the north tyrrhenian sea and framework for remediation. Environments 2020, 7, 17. [Google Scholar] [CrossRef] [Green Version]
- Fredianelli, L.; Nastasi, M.; Bernardini, M.; Fidecaro, F.; Licitra, G. Pass-by characterization of noise emitted by different categories of seagoing ships in ports. Sustainability 2020, 12, 1740. [Google Scholar] [CrossRef] [Green Version]
- Gershon, R.R.M.; Qureshi, K.A.; Barrera, M.A.; Erwin, M.J.; Goldsmith, F. Health and safety hazards associated with subways: A review. J. Urban Health 2005, 82, 10–20. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Münzel, T.; Sørensen, M.; Gori, T.; Schmidt, F.P.; Rao, X.; Brook, J.; Chen, L.C.; Brook, R.D.; Rajagopalan, S. Environmental stressors and cardio-metabolic disease: Part I-epidemiologic evidence supporting a role for noise and air pollution and effects of mitigation strategies. Eur. Heart J. 2017, 38, 550–556. [Google Scholar] [CrossRef] [Green Version]
- Guski, R.; Schreckenberg, D.; Schuemer, R. WHO Environmental Noise Guidelines for the European Region: A Systematic Review on Environmental Noise and Annoyance. Int. J. Environ. Res. Public Health 2017, 14, 1539. [Google Scholar] [CrossRef] [Green Version]
- Johnson, T.A.; Cooper, S.; Stamper, G.C.; Chertoff, M. Noise exposure questionnaire: A tool for quantifying annual noise exposure. J. Am. Acad. Audiol. 2017, 28, 14–35. [Google Scholar] [CrossRef] [Green Version]
- Muzet, A. Environmental noise, sleep and health. Sleep Med. Rev. 2007, 11, 135–142. [Google Scholar] [CrossRef]
- Dratva, J.; Phuleria, H.C.; Foraster, M.; Gaspoz, J.M.; Keidel, D.; Künzli, N.; Schindler, C. Transportation noise and blood pressure in a population-based sample of adults. Environ. Health Perspect. 2012, 120, 50–55. [Google Scholar] [CrossRef]
- Babisch, W.; Beule, B.; Schust, M.; Kersten, N.; Ising, H. Traffic noise and risk of myocardial infarction. Epidemiology 2005, 16, 33–40. [Google Scholar] [CrossRef]
- Bernardini, M.; Fredianelli, L.; Fidecaro, F.; Gagliardi, P.; Nastasi, M.; Licitra, G. Noise Assessment of Small Vessels for Action Planning in Canal Cities. Environments 2019, 6, 31. [Google Scholar] [CrossRef] [Green Version]
- Thompson, D. Railway Noise and Vibration: Mechanisms, Modelling and Means of Control; Elsevier: Amsterdam, The Netherlands, 2008. [Google Scholar]
- Eade, P.W.; Hardy, A.E.J. Railway vehicle internal noise. J. Sound Vib. 1977, 51, 403–415. [Google Scholar] [CrossRef]
- Forssén, J.; Tober, S.; Corakci, A.C.; Frid, A.; Kropp, W. Modelling the interior sound field of a railway vehicle using statistical energy analysis. Appl. Acoust. 2012, 73, 307–311. [Google Scholar] [CrossRef] [Green Version]
- Zheng, X.; Hao, Z.; Wang, X.; Mao, J. A full-spectrum analysis of high-speed train interior noise under multi-physical-field coupling excitations. Mech. Syst. Signal Process. 2016, 75, 525–543. [Google Scholar] [CrossRef]
- Dai, W.Q.; Zheng, X.; Luo, L.; Hao, Z.Y.; Qiu, Y. Prediction of high-speed train full-spectrum interior noise using statistical vibration and acoustic energy flow. Appl. Acoust. 2019, 145, 205–219. [Google Scholar] [CrossRef]
- Shi, Y.; Xiao, Y.G.; Kang, Z.C. Interior noise investigation for a passenger room of a high speed train under wheel-rail excitation. J. Vib. Shock 2009, 28, 95–98. [Google Scholar]
- Han, J.; Xiao, X.B.; Wu, Y.; Wen, Z.F.; Zhao, G.T. Effect of rail corrugation on metro interior noise and its control. Appl. Acoust. 2018, 130, 63–70. [Google Scholar] [CrossRef]
- Li, L.; Thompson, D.; Xie, Y.S.; Zhu, Q.; Luo, Y.Y.; Lei, Z.Y. Influence of rail fastener stiffness on railway vehicle interior noise. Appl. Acoust. 2019, 145, 69–81. [Google Scholar] [CrossRef] [Green Version]
- Fan, R.P.; Su, Z.Q.; Meng, G.; He, C.C. Application of sound intensity and partial coherence to identify interior noise sources on the high speed train. Mech. Syst. Signal Process. 2014, 46, 481–493. [Google Scholar] [CrossRef]
- Noise Limit and Measurement for Train of Urban Rail Transit; GB 14892; Standardization Administration: Beijing, China, 2012. (In Chinese)
- Railway Applications. Acoustics. Measurement of Noise Inside Railbound Vehicles; ISO 3381; ISO: Geneva, Switzerland, 2011.
- Persson, K.; Björkman, M. Annoyance due to low frequency noise and the use of the dB (A) scale. J. Sound Vib. 1988, 127, 491–497. [Google Scholar] [CrossRef]
- Torija, A.J.; Flindell, I.H.; Self, R.H. Subjective dominance as a basis for selecting frequency weightings. J. Acoust. Soc. Am. 2016, 140, 843–854. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Seidman, M.D.; Standring, R.T. Noise and quality of life. Int. J. Environ. Res. Public Health 2010, 7, 3730–3738. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ma, K.W.; Wong, H.M.; Mak, C.M. A systematic review of human perceptual dimensions of sound: Meta-analysis of semantic differential method applications to indoor and outdoor sounds. Build. Environ. 2018, 133, 123–150. [Google Scholar] [CrossRef]
- Ning, J.; Lin, J.H.; Zhang, B. Time-frequency processing of track irregularities in high-speed train. Mech. Syst. Signal Process. 2016, 66, 339–348. [Google Scholar] [CrossRef]







| Points | Speeds | ||||||
|---|---|---|---|---|---|---|---|
| 0 km/h | 20 km/h | 40 km/h | 50 km/h | 60 km/h | 80 km/h | 115 km/h | |
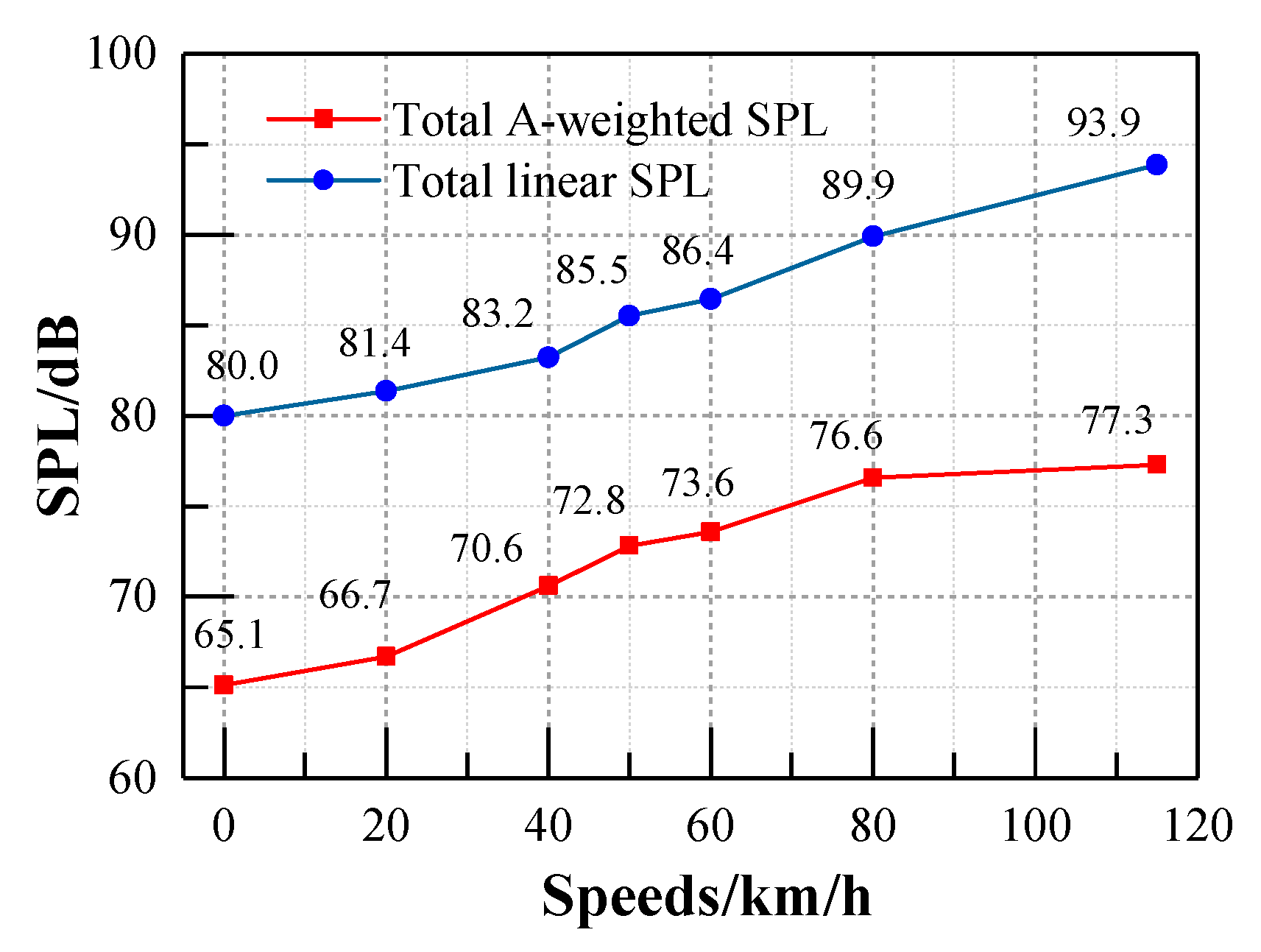
| N1 | 80.0 | 81.4 | 83.2 | 85.5 | 86.4 | 89.9 | 93.9 |
| N2 | 79.5 | 80.6 | 82.9 | 85.1 | 86.2 | 89.8 | 94.2 |
| N3 | 79.5 | 80.5 | 82.7 | 84.9 | 86.1 | 89.6 | 94.4 |
| N4 | 79.4 | 80.5 | 82.7 | 84.9 | 86.1 | 89.5 | 94.4 |
| N5 | 79.3 | 81.0 | 83.7 | 85.5 | 86.7 | 89.9 | 94.8 |
| N6 | 80.0 | 81.3 | 83.7 | 85.8 | 86.9 | 90.2 | 95.1 |
| N7 | 79.9 | 81.3 | 83.7 | 85.9 | 87.0 | 90.5 | 95.4 |
| N8 | 79.1 | 81.0 | 83.9 | 85.7 | 86.7 | 90.1 | 94.9 |
| Points | Speeds | ||||||
|---|---|---|---|---|---|---|---|
| 0 km/h | 20 km/h | 40 km/h | 50 km/h | 60 km/h | 80 km/h | 115 km/h | |
| N1 | 65.1 | 66.7 | 70.6 | 72.8 | 73.6 | 76.6 | 77.3 |
| N2 | 65.1 | 66.2 | 70.6 | 72.6 | 73.4 | 76.4 | 77.4 |
| N3 | 64.8 | 66.2 | 70.0 | 72.1 | 72.8 | 75.7 | 76.7 |
| N4 | 64.8 | 66.4 | 70.3 | 72.2 | 72.9 | 76.0 | 76.8 |
| N5 | 65.0 | 66.8 | 70.3 | 72.4 | 73.0 | 76.0 | 76.7 |
| N6 | 64.9 | 66.9 | 70.3 | 72.5 | 72.9 | 76.1 | 77.0 |
| N7 | 64.4 | 67.1 | 70.6 | 72.8 | 73.4 | 76.3 | 77.4 |
| N8 | 64.0 | 67.8 | 70.8 | 73.3 | 73.9 | 76.9 | 77.9 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, L.; Chen, Z.; Zou, Y.; He, X.; Cai, C.; Yu, K.; Zhu, X. Field Study of the Interior Noise and Vibration of a Metro Vehicle Running on a Viaduct: A Case Study in Guangzhou. Int. J. Environ. Res. Public Health 2020, 17, 2807. https://doi.org/10.3390/ijerph17082807
Yan L, Chen Z, Zou Y, He X, Cai C, Yu K, Zhu X. Field Study of the Interior Noise and Vibration of a Metro Vehicle Running on a Viaduct: A Case Study in Guangzhou. International Journal of Environmental Research and Public Health. 2020; 17(8):2807. https://doi.org/10.3390/ijerph17082807
Chicago/Turabian StyleYan, Lei, Zhou Chen, Yunfeng Zou, Xuhui He, Chenzhi Cai, Kehui Yu, and Xiaojie Zhu. 2020. "Field Study of the Interior Noise and Vibration of a Metro Vehicle Running on a Viaduct: A Case Study in Guangzhou" International Journal of Environmental Research and Public Health 17, no. 8: 2807. https://doi.org/10.3390/ijerph17082807
APA StyleYan, L., Chen, Z., Zou, Y., He, X., Cai, C., Yu, K., & Zhu, X. (2020). Field Study of the Interior Noise and Vibration of a Metro Vehicle Running on a Viaduct: A Case Study in Guangzhou. International Journal of Environmental Research and Public Health, 17(8), 2807. https://doi.org/10.3390/ijerph17082807






