Study of Anti-Sliding Stability of a Dam Foundation Based on the Fracture Flow Method with 3D Discrete Element Code
Abstract
:1. Introduction
2. Methodology
2.1. Seepage in a Single Fracture
2.2. Numerical Simulation Method and Verification
2.3. Hydraulic-Mechanical Coupling with Strength Reduction Method in DEM
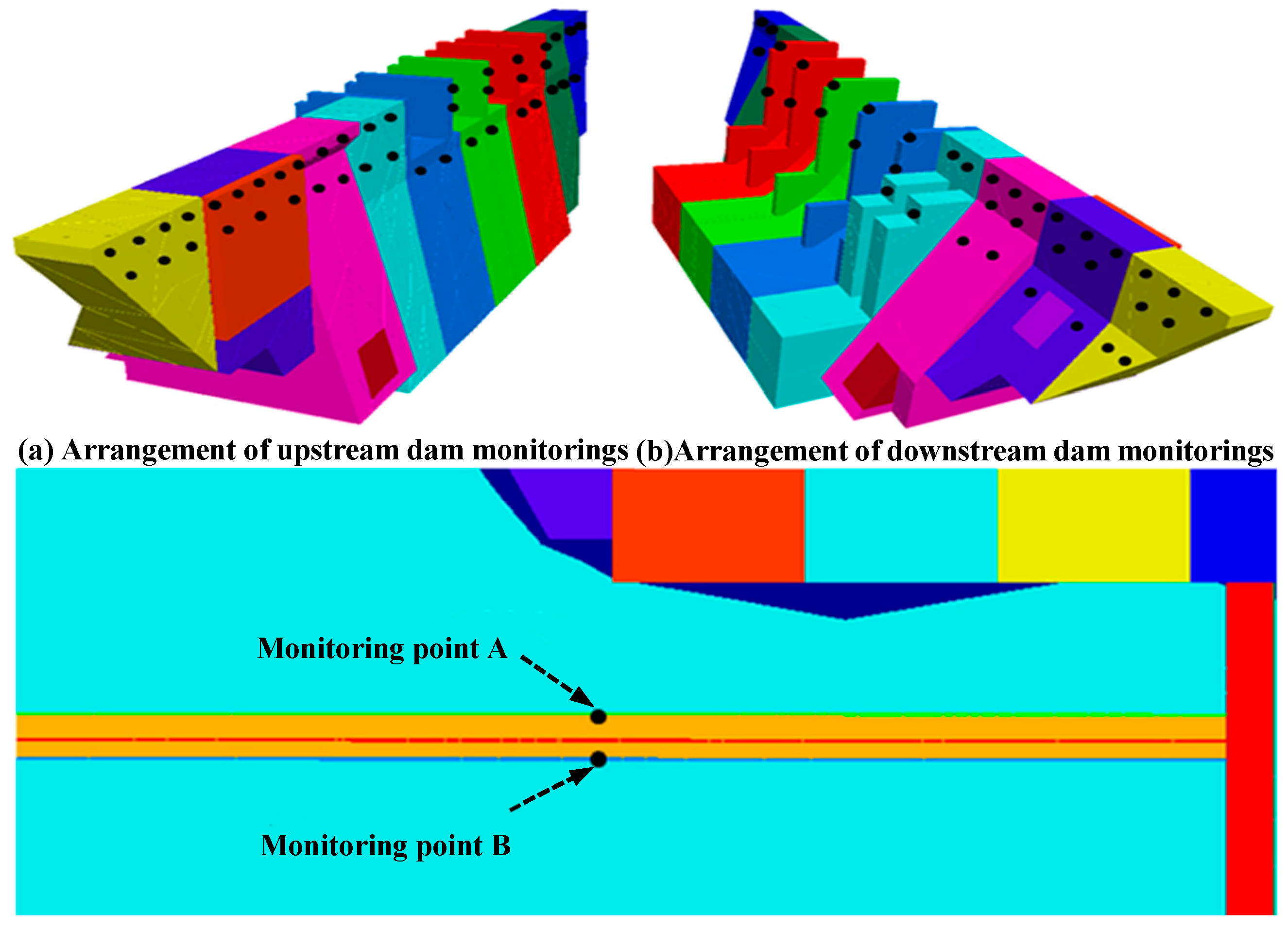
3. Analytical Method of Seepage in Dam Foundation
3.1. Dam Foundation Model
3.2. Hydraulic-Mechanical Coupling Calculation
3.3. Numerical Results Analysis about Dam Deformation
4. Discussion
- (a)
- The discrete element method can fully reflect the deformation character caused by the difference between dam foundation rock parameters and structural plane parameters, which may affect the safety factor of local dam sections.
- (b)
- The strength reduction method in combination with the hydraulic-mechanical analysis produces an efficient solution that can express the continuous motion in the model, even in an unstable system.
- (c)
- The strength reduction method, based on the discrete element method, can automatically search for the minimum safety factor and the corresponding failure modes under the actual combination of structural planes, which is closer to a real-world situation.
5. Conclusions
- (a)
- The modified cubic law considered the tortuosity and roughness of the fracture that improves fracture network models, which provided an approach for accurately calculating hydraulic properties and made the analysis method of fractured rock mass more applicable.
- (b)
- The discrete element method was used to analyze the hydraulic-mechanical coupling problem in a fractured rock mass, and it was deemed suitable for analyzing such a problem. The results of analysis were more reasonable and were found to be closer to the actual problems in a fractured rock mass.
- (c)
- The strength reduction method based on the discrete element method has the advantage that it can be used to analyze the stability of the complex dam-foundation system. It can automatically search for the minimum safety factor and the failure mode under the condition of a combination of structural planes. This method can produce results more in line with the true state of dam foundation damage and is also suitable for the analysis of hydraulic-mechanical intersection failure under the action of a complex fractured system.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Jing, L. A review of techniques, advances and outstanding issues in numerical modelling for rock mechanics and rock engineering. Int. J. Rock Mech. Min Sci. 2003, 40, 283–353. [Google Scholar] [CrossRef]
- He, J.; Chen, S.H.; Shahrour, I. Back Analysis of Equivalent Permeability Tensor for Fractured Rock Masses from Packer Tests. Rock Mech. Rock Eng. 2011, 44, 491. [Google Scholar] [CrossRef]
- Cundall, P.A. A computer model for simulating progressive large scale movements in blocky rock systems. Int. Soc. Rock Mech. 1971, 2, 129–136. [Google Scholar]
- Shi, G.H. Discontinuous Deformation Analysis—A New Model for the Statics and Dynamics of Block Systems. Ph.D. Thesis, University of California, Berkeley, CA, USA, 1988. [Google Scholar]
- Shi, G.H. Block System Modeling by Discontinuous Deformation Analysis; Computation Mechanics Publications: Southampton, UK, 1993. [Google Scholar]
- Munjiza, A.; Owen, D.R.J.; Bicanic, N. A. combined finite-discrete element method in transient dynamics of fracturing solids. Eng. Comput. 1995, 12, 145–174. [Google Scholar] [CrossRef]
- Rougier, E.; Bradley, C.R.; Broom, S.T. The Combined Finite-Discrete Element Method applied to the Study of Rock Fracturing Behavior in 3D. Proceeding of the Conference 45th US Rock Mechanics/Geomechanics Symposium, San Francisco, CA, USA, 26–29 June 2011. [Google Scholar]
- Lorig, L.J.; Hart, R.D.; Cundall, P.A. Slope Stability Analysis of Jointed Rock Using Distinct Element Method. Transp. Res. Rec. 1991, 1330, 1–9. [Google Scholar]
- Lorig, L.J.; Cundall, P.A.; Damjanac, B. A Three-Dimensional Model for Rock Slopes Based on Micromechanics. Proceeding of the 44th U.S. Rock Mechanics Symposium and 5th U.S.-Canada Rock Mechanics Symposium, Salt Lake City, UT, USA, 27–30 June 2010. [Google Scholar]
- Javadi, M.; Sharifzadeh, M.; Shahriar, K. A new geometrical model for non-linear fluid flow through rough fractures. J. Hydrol. 2010, 389, 18–30. [Google Scholar] [CrossRef]
- Parashar, R.; Reeves, D.M. On iterative techniques for computing flow in large two-dimensional discrete fracture networks. J. Comput. Appl. Math. 2012, 236, 4712–4724. [Google Scholar] [CrossRef]
- Zheng, J.; Deng, J.; Zhang, G. Validation of Monte Carlo simulation for discontinuity locations in space. Comput. Geotech. 2015, 67, 103–109. [Google Scholar] [CrossRef]
- Leung, C.T.; Zimmerman, R.W. Estimating the hydraulic conductivity of two-dimensional fracture networks. Transp. Porous Media 2012, 93, 777–797. [Google Scholar] [CrossRef]
- Liu, R.; Jiang, Y.; Li, B.; Wang, X. A fractal model for characterizing fluid flow in fractured rock masses based on randomly distributed rock fracture networks. Comput. Geotech. 2015, 65, 45–55. [Google Scholar] [CrossRef]
- Zafarani, A.; Detwiler, R.L. Solute Transport in Three-Dimensional Variable-Aperture Discrete Fracture Networks. AGU Fall Meet. Abstr. 2012, 1, 4. [Google Scholar]
- Guo, L.; Li, X.; Zhou, Y.; Zhang, Y. Generation and verification of three-dimensional network of fractured rock masses stochastic discontinuities based on digitalization. Environ. Earth Sci. 2015, 73, 7075–7088. [Google Scholar] [CrossRef]
- He, G.; Wang, E.; Liu, X. Modified governing equation and numerical simulation of seepage flow in a single fracture with three-dimensional roughness. Arab. J. Geosci. 2016, 9, 1–20. [Google Scholar] [CrossRef]
- Tsang, Y.W.; Tsang, C.F. Channel model of flow through fractured media. Water Resour. Res. 1987, 23, 467–479. [Google Scholar] [CrossRef]
- Gudmundsson, A. Fracture dimensions, displacements and fluid transport. J. Struct. Geol. 2000, 22, 1221–1231. [Google Scholar] [CrossRef]
- Tsang, Y.W.; Witherspoon, P.A. The dependence of fracture mechanical and fluid flow properties on fracture roughness and sample size. J. Geophys. Res. 1983, 88, 2359–2366. [Google Scholar] [CrossRef]
- Barton, N.; Bandis, S.; Bakhtar, K. Strength deformationed conductivity coupling of rock joints. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1985, 22, 121–140. [Google Scholar] [CrossRef]
- Rutqvist, J. Determination of hydraulic normal stiffness of fractures in hard rock from well testing. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1995, 32, 513–523. [Google Scholar] [CrossRef]
- Detournay, E. Hydraulic Conductivity of Closed Rock Fracture: An Experimental and Analytical Study. In Proceedings of the 13th Canadian Rock Mechanics Symposium, Toronto, Canada, 28–29 May 1980; pp. 168–173. [Google Scholar]
- Alvarez, T.A.; Cording, E.J.; Mikhail, A. Hydromechanical Behavior of Rock Joints: A Re-interpretation of Published Experiments. In Proceedings of the 35th U.S. Symposium, University of Nevada, Reno, NV, USA, 5–7 June 1995; pp. 665–671. [Google Scholar]
- Cappaa, F.; Guglielmi, Y. Hydromechanical interactions in a fractured carbonate reservoir inferred from hydraulic and mechanical measurements. Int. J. Rock Mech. Min. Sci. 2005, 42, 287–306. [Google Scholar] [CrossRef]
- Dawson, E.M.; Roth, W.H. Slope stability analysis by strength reduction. Geotechnique 1999, 49, 835–840. [Google Scholar] [CrossRef]
- NB/T 35026-2014. Design Code for Concrete Gravity Dam; Beijing China Electric Power Press: Beijing, China, 2014. [Google Scholar]
- Brandon, J.R. U S Bureau of Reclamation Report REC-ERC-74-10; Engineering and Research Center, U S Bureau of Reclamation: Denver, CO, USA, 1974.
- Davis, R.O.; Selvadurai, A.P.S. Plasticity and Geomechanics; Cambridge University Press: Cambridge, UK, 2002; Volume 48, pp. 149–151. [Google Scholar]
- Wei, L.; Hudson, J.A. A hybrid discrete-continuum approach to model hydro-mechanical behaviour of jointed rocks. Eng. Geol. 1998, 49, 317–325. [Google Scholar] [CrossRef]









| Measuring Point | Discontinuous Types | Hydraulic Parameters | Mechanical Parameters | |||
|---|---|---|---|---|---|---|
| Hydraulic Conductivity/(m·s−1) | Storativity | Measured Hydraulic Aperture/(m) | Initial Normal Stiffness/(GPa·m−1) | Initial Normal Stress/(Pa) | ||
| 1 | Fault F2 | 5.7 × 10−5 | 0.7 × 10−8 | 2.6 × 10−4 | 132 | 1.99 × 105 |
| 2 | Fault F12 | - | - | - | 66 | 5.79 × 104 |
| 3 | Bedding plane S2 | - | - | - | 20 | - |
| 4 | Fault F11 | 2.3 × 10−4 | 2..6 × 10−8 | 5.3 × 10−4 | 1.4 | 5.1 × 104 |
| 5 | Fault F11 | 1.9 × 10−4 | 1.9 × 10−8 | 4.8 × 10−4 | 7.2 | 1.58 × 105 |
| 6 | Bedding plane S2 | 7.6 × 10−6 | 3.4 × 10−11 | 9.6 × 10−5 | 20 | - |
| 8 | Bedding plane S2 | 3..8 × 10−6 | 2.1 × 10−11 | 6.8 × 10−5 | 20 | - |
| 9a | Bedding plane S2 | 3.2 × 10−6 | 1.8 × 10−11 | 6.2 × 10−5 | 20 | - |
| 9b | Bedding plane S3 | 9.0 × 10−7 | 0.5 × 10−11 | 3.3 × 10−5 | 20 | - |
| 10 | Fault F2 | 2.0 × 10−4 | 3.0 × 10−11 | 4.9 × 10−4 | - | - |
| Rock Mass Quality Classification | Bulk Density | Shear Strength of Rock Mass | Shear Strength of Concrete/Rock | Deformation Modulus | Allowable Bearing Capacity | Poisson Ratio | ||
|---|---|---|---|---|---|---|---|---|
| γ/(KN/m3) | f′ | c′/(MPa) | f′ | c′/(MPa) | /(GPa) | /(MPa) | ||
| III1B | 24.5 | 1.0 | 0.90 | 1.00 | 0.90 | 6.50 | 4.50 | 0.23 |
| III2B | 23.5 | 0.85 | 0.70 | 0.95 | 0.75 | 5.50 | 3.50 | 0.26 |
| IV1B | 22.5 | 0.75 | 0.45 | 0.80 | 0.45 | 3.50 | 2.50 | 0.29 |
| IV2B | 22.0 | 0.55 | 0.35 | 0.70 | 0.34 | 2.50 | 2.00 | 0.33 |
| IIIc | 21.5 | 0.60 | 0.55 | 0.70 | 0.60 | 2.0 | 1.50 | 0.33 |
| IV1c | 20.5 | 0.55 | 0.35 | 0.55 | 0.35 | 1.40 | 1.30 | 0.35 |
| IV2c | 20.0 | 0.50 | 0.26 | 0.45 | 0.25 | 1.10 | 0.90 | 0.37 |
| V | 18.0 | 0.40 | 0.13 | 0.40 | 0.10 | 0.40 | 0.60 * 0.40 * | 0.39 |
| Number of Structural Plane | Shear Strength | |
|---|---|---|
| f′ | c′/(MPa) | |
| F1, F12, F13, C15,C30 | 0.40 | 0.06 |
| C13 | 0.30 | 0.05 |
| C16 | 0.35 | 0.07 |
| C12 | 0.30 | 0.04 |
| C07, C09, C10, C17, C18, C19 | 0.24 | 0.02 |
| C01, C03, C04, C11, C14 | 0.17 | 0.001 |
| C05, C06, C25 | 0.23 | 0.02 |
| C20 | 0.36 | 0.08 |
| Segment between the dam sections | 0.33 | - |
| Interface between concrete dam and bedrock | 0.95 | 0.74 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, C.; Yang, W.; Chu, W.; Shen, J.; Kong, Y. Study of Anti-Sliding Stability of a Dam Foundation Based on the Fracture Flow Method with 3D Discrete Element Code. Energies 2017, 10, 1544. https://doi.org/10.3390/en10101544
Shi C, Yang W, Chu W, Shen J, Kong Y. Study of Anti-Sliding Stability of a Dam Foundation Based on the Fracture Flow Method with 3D Discrete Element Code. Energies. 2017; 10(10):1544. https://doi.org/10.3390/en10101544
Chicago/Turabian StyleShi, Chong, Wenkun Yang, Weijiang Chu, Junliang Shen, and Yang Kong. 2017. "Study of Anti-Sliding Stability of a Dam Foundation Based on the Fracture Flow Method with 3D Discrete Element Code" Energies 10, no. 10: 1544. https://doi.org/10.3390/en10101544
APA StyleShi, C., Yang, W., Chu, W., Shen, J., & Kong, Y. (2017). Study of Anti-Sliding Stability of a Dam Foundation Based on the Fracture Flow Method with 3D Discrete Element Code. Energies, 10(10), 1544. https://doi.org/10.3390/en10101544






