Envelope Design Optimization by Thermal Modelling of a Building in a Warm Climate
Abstract
:HIGHLIGHTS:
- The envelope design of a residential building has been optimized for a warm climate.
- Several envelope design options have been evaluated and compared.
- Walls with a high internal areal heat capacity are preferable in a warm climate.
- The absence of insulating layers on slab-on-ground floor provides lower temperature in summer.
- Low emissivity glass and low solar absorbance roof reduce heat loads.
1. Introduction
1.1. Building Optimization
1.2. Envelope Measures for Energy Savings
- low values of the decrement factor;
- high values of the internal thermal areal capacity;
- high values of the time shift.
1.3. Comfort Assessment and Thermal Behaviour
- hc = convective heat transfer coefficient
- hr = linear radiative heat transfer coefficient
- Tdb = air (dry bulb) temperature
- Tmr = mean radiant temperature
2. Methodology
2.1. Building Prototype
2.2. Building Dynamic Simulations
- Building (Type 56) contains information on the building envelope; i.e., orientation of walls, material properties, stratigraphy, floor and roof, windows properties and thermal loads;
- Soil characteristics (Type 501);
- Overhangs (Type 34) reports information on shading elements for each orientation (North overhang, West overhang, East overhang, South overhang);
- Weather data (Type 15) includes the weather data location. To set up the model, Types must be connected in a logical way as shown in Figure 2.
- -
- Beam radiation distribution—standard mode. Userdefined distribution factors for surfaces so called GEOSURF values are applied. The value of GEOSURF represents the fraction of the total entering direct solar radiation that strikes the surface.
- -
- Diffuse radiation distribution—standard mode. The standard mode is based on aborption-transmission weighted area ratios for all surfaces of a zone.
- -
- Longwave radiation exchange of surfaces within a zone—standard mode. The standard mode is based on the starnode approach. No user defined emissivities of inside surfaces nor radiation exchange over more than one airnode can be taken into account.
2.3. Envelope Optimization Steps
2.3.1. External Walls Selection
2.3.2. Slab on Ground Floor Evaluation
2.3.3. Analysis of the Roof Solar Absorbance
2.3.4. Evaluation of the Windows Position
2.3.5. Sizing of Windows
2.3.6. Study of the Impact of Window Types
2.3.7. Assessment of Shading
2.3.8. Estimation of the Internal Heat Loads
- a family of four people within the building;
- a load of 5 W/m2 due to the use of appliances from 8:00 to 20:00;
- a load of 3 W/m2 due to the use of appliances from 20:00 to 8:00.
2.3.9. Ground boundary conditions
2.3.10. Comfort Evaluation
- Clothing factor = 1 (CLO);
- Metabolic rate = 1.2 (MET);
- Relative air velocity = 0.15 m/s;
- Relative humidity value = 50%.
3. Results and Discussion
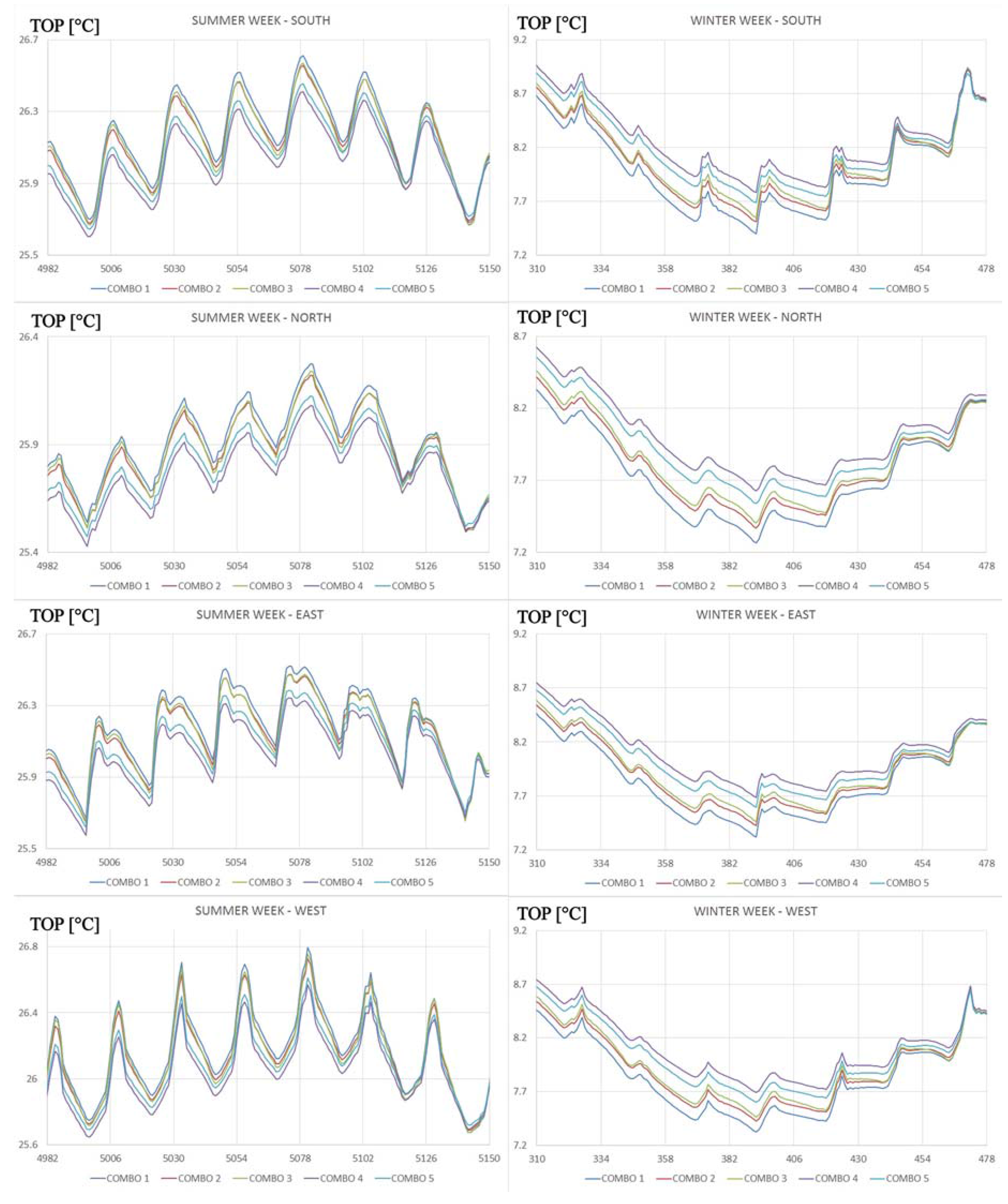
3.1. External Walls
3.2. Slab on Ground Floor
3.3. Roof Absorbance
3.4. Windows Position
3.5. Windows Sizing
3.6. Window Types
3.7. Shading
3.8. Internal Heat Loads
3.9. PMV and PPD
4. Conclusions
Author Contributions
Conflicts of Interest
Nomenclature
| TOP | operative air temperature (°C) |
| U | Steady thermal transmittance (W/m2K) |
| Ymm | thermal admittance (W/m2K) |
| Ymn | periodic thermal transmittance (W/m2K) |
| c | specific heat capacity (J/kgK) |
| d | thickness of a layer (m) |
| fd | decrement factor |
| g | solar factor |
| ∆t | time shift: time lead (if positive), or time lag (if negative) (s or h) |
| Ms | total surface mass (excluding coats) (Kg/m2) |
| Greek letters | |
| κ | areal heat capacity (kJ/m2K) |
| λ | design thermal conductivity(W/m K) |
| ρ | density (kg/m3) |
| Subscripts | |
| m,n | for the thermal zones |
| g | glass |
| f | frame |
| 1 | internal |
| 2 | external |
References
- Ruamsuke, K.; Dhakal, S.; Marpaung, C.O.P. Energy and economic impacts of the global climate change policy on Southeast Asian countries: A general equilibrium analysis. Energy 2015, 81, 446–461. [Google Scholar] [CrossRef]
- European Commission. Taking Stock of the Europe 2020 Strategy for Smart, Sustainable and Inclusive Growth; European Commission: Brussels, Belgium, 2014. [Google Scholar]
- Eurostat. Final Energy Consumption by Sector. 2014. Available online: http://ec.europa.eu/eurostat/data/database (accessed on 8 October 2017).
- Li, D.H.W.; Yang, L.; Lam, J.C. Impact of climate change on energy use in the built environment in different climate zones—A review. Energy 2012, 42, 103–112. [Google Scholar] [CrossRef]
- D’Agostino, D. Assessment of the progress towards the establishment of definitions of Nearly Zero Energy Buildings (nZEBs) in European Member States. J. Build. Eng. 2015, 1, 20–32. [Google Scholar] [CrossRef]
- EU, Directive 2012/27/EU. European Parliament and of the Council of 25 October 2012 on energy efficiency, amending Directives 2009/125/EC and 2010/30/EU and repealing Directives 2004/8/EC and 2006/32/EC. Off. J. Eur. Union 2012, L315/1. [Google Scholar]
- EU, Directive 2010/31/EU. European Parliament and of the Council of 19 May 2010 on the Energy Performance of Buildings (recast). Off. J. Eur. Union 2010, 13–35. [Google Scholar]
- Yu, J.; Tian, L.; Yang, C.; Xu, X.; Wang, J. Sensitivity analysis of energy performance for high-rise residential envelope in hot summer and cold winter zone of China. Energy Build. 2013, 64, 264–274. [Google Scholar] [CrossRef]
- Baglivo, C.; Congedo, P.M. High performance precast external walls for cold climate by a multi-criteria methodology. Energy 2016, 115, 561–576. [Google Scholar] [CrossRef]
- Raji, B.; Tenpierik, M.J.; van den Dobbelsteen, A. An assessment of energy-saving solutions for the envelope design of high-rise buildings in temperate climates: A case study in the Netherlands. Energy Build. 2016, 124, 210–221. [Google Scholar] [CrossRef]
- Al-Sanea, S.A.; Zedan, M.F.; Al-Hussain, S.N. Effect of thermal mass on performance of insulated building walls and the concept of energy savings potential. Appl. Energy 2012, 89, 430–442. [Google Scholar] [CrossRef]
- Tang, C.; Chen, S.; Tang, Z.; Wu, P.; Trofimova, P. Building Information Modeling and Building Performance Optimization. Encycl. Sustain. Technol. 2017, 311–320. [Google Scholar]
- Bouchlaghem, N.M.; Letherman, K.M. Numerical optimization applied to the thermal design of buildings. Build. Environ. 1990, 25, 117–124. [Google Scholar] [CrossRef]
- Wilson, A.J.; Templeman, A.B. An approach to the optimum thermal design of office buildings. Build. Environ. 1976, 11, 39–50. [Google Scholar] [CrossRef]
- Wang, W.; Rivard, H.; Zmeureanu, R. An object-oriented framework for simulation-based green building design optimization with genetic algorithms. Adv. Eng. Inform. 2005, 19, 5–23. [Google Scholar] [CrossRef]
- Wright, J.A.; Loosemore, H.A.; Farmani, R. Optimization of building thermal design and control by multi-criterion genetic algorithm. Energy Build. 2002, 34, 959–972. [Google Scholar] [CrossRef]
- Sahu, M.; Bhattacharjee, B.; Kaushik, S.C. Thermal design of air-conditioned building for tropical climate using admittance method and genetic algorithm. Energy Build. 2012, 53, 1–6. [Google Scholar] [CrossRef]
- Eisenhower, B.; O’Neill, Z.; Narayanan, S.; Fonoberov, V.A.; Mezi, I. A methodology for Meta model based optimization in building energy models. Energy Build. 2012, 47, 292–301. [Google Scholar] [CrossRef]
- Zhou, L.; Haghighat, F. Optimization of ventilation system design and operation in office environment. Part I: Methodology. Energy Build. 2009, 44, 651–656. [Google Scholar] [CrossRef]
- Vera, J.T.; Laukkanen, T.; Sirén, K. Multi-objective optimization of hybrid photovoltaic–thermal collectors integrated in a DHW heating system. Energy Build. 2014, 74, 78–90. [Google Scholar] [CrossRef]
- Stavrakakis, G.M.; Zervas, P.L.; Sarimveis, H.; Markatos, N.C. Optimization of window-openings design for thermal comfort in naturally ventilated buildings. Appl. Math. Model. 2012, 36, 93–211. [Google Scholar] [CrossRef]
- Rapone, G.; Saro, O. Optimisation of curtain wall facades for office buildings by means of PSO algorithm. Energy Build. 2012, 45, 189–196. [Google Scholar] [CrossRef]
- Chantrelle, F.P.; Lahmidi, H.; Keilholz, W.; el Mankibi, M.; Michel, P. Development of a multicriteria tool for optimizing the renovation of buildings. Appl. Energy 2011, 88, 1386–1394. [Google Scholar] [CrossRef]
- Huang, H.; Kato, S.; Hu, R. Optimum design for indoor humidity by coupling Genetic Algorithm with transient simulation based on Contribution Ratio of Indoor Humidity and Climate analysis. Energy Build. 2012, 47, 208–216. [Google Scholar] [CrossRef]
- Chen, H.; Ooka, R.; Kato, S. Study on optimum design method for pleasant outdoor thermal environment using genetical gorithms (GA) and coupled simulation of convection, radiation and conduction. Energy Build. 2008, 43, 18–30. [Google Scholar] [CrossRef]
- Hamdy, M.; Hasan, A.; Siren, K. A multi-stage optimization method for cost-optimal and nearly-zero-energy building solutions in line with the EPBD-recast 2010. Energy Build. 2013, 56, 189–203. [Google Scholar] [CrossRef]
- García-Segura, T.; Yepes, V. Multiobjective optimization of post-tensioned concrete box-girder road bridges considering cost, CO2 emissions, and safety. Eng. Struct. 2016, 125, 325–336. [Google Scholar] [CrossRef]
- Martí, J.V.; García-Segura, T.; Yepes, V. Structural design of precast-prestressed concrete U-beam road bridges based on embodied energy. J. Clean. Prod. 2016, 120, 231–240. [Google Scholar] [CrossRef]
- Ferrara, M.; Fabrizio, E.; Virgone, J.; Filippi, M. A simulation-based optimization method for cost-optimal analysis of nearly Zero Energy Buildings. Energy Build. 2014, 84, 442–457. [Google Scholar] [CrossRef]
- Attia, S.; Hamdy, M.; O’Brien, W.; Carlucci, S. Assessing gaps and needs for integrating building performance optimization tools in net zero energy buildings design. Energy Build. 2013, 60, 110–124. [Google Scholar] [CrossRef]
- Dombaycı, Ö.A.; Gölcü, M.; Pancar, Y. Optimization of insulation thickness for external walls using different energy-sources. Appl. Energy 2006, 83, 921–928. [Google Scholar] [CrossRef]
- Aste, N.; Manfren, M.; Marenzi, G. Building Automation and Control Systems and performance optimization: A framework for analysis. Renew. Sustain. Energy Rev. 2017, 75, 313–330. [Google Scholar] [CrossRef]
- Valdiserri, P.; Biserni, C.; Garai, M. Energy performance of a ventilation system for an apartment according to the Italian regulation. Int. J. Energy Environ. Eng. 2016, 7, 353–359. [Google Scholar] [CrossRef]
- Baglivo, C.; Congedo, P.M.; Fazio, A.; Laforgia, D. Multi-objective optimization analysis for high efficiency external walls of zero energy buildings (ZEB) in the Mediterranean climate. Energy Build. 2014, 84, 483–492. [Google Scholar] [CrossRef]
- Baglivo, C.; Congedo, P.M.; Fazio, A. Multi-criteria optimization analysis of external walls according to ITACA protocol for zero energy buildings in the Mediterranean climate. Build. Environ. 2014, 82, 467–480. [Google Scholar] [CrossRef]
- Baglivo, C.; Congedo, P.M. Design method of high performance precast external walls for warm climate by multi-objective optimization analysis. Energy 2015, 90, 1645–1661. [Google Scholar] [CrossRef]
- Sarkar, A.; Bose, S. Exploring impact of opaque building envelope components on thermal and energy performance of houses in lower western Himalayans for optimal selection. J. Build. Eng. 2016, 7, 170–182. [Google Scholar] [CrossRef]
- Cardinale, N.; Rospi, G.; Stefanizzi, P. Energy and microclimatic performance of Mediterranean vernacular buildings: The Sassi district of Matera and the Trulli district of Alberobello. Build. Environ. 2013, 59, 590–598. [Google Scholar] [CrossRef]
- Minne, E.; Wingrove, K.; Crittenden, J.C. Influence of climate on the environmental and economic life cycle assessments of window options in the United States. Energy Build. 2015, 102, 293–306. [Google Scholar] [CrossRef]
- Xamán, J.; Pérez-Nucamendi, C.; Arce, J.; Hinojosa, J.; Álvarez, G.; Zavala-Guillén, I. Thermal analysis for a double pane window with a solar control film for using in cold and warm climates. Energy Build. 2014, 76, 429–439. [Google Scholar] [CrossRef]
- Cappelletti, F.; Prada, A.; Romagnoni, P.; Gasparella, A. Passive performance of glazed components in heating and cooling of an open-space office under controlled indoor thermal comfort. Build. Environ. 2014, 72, 131–144. [Google Scholar] [CrossRef]
- Fanger, P.O.; Melikov, A.K.; Hanzawa, H.; Ring, J. Turbulence and draft. ASHR J. 1989, 31, 18–23. [Google Scholar]
- ASHRAE Standard 55. Thermal Environment Conditions for Human Occupancy; American Society of Heating, Refrigerating and Air-Conditioning Engineers, Inc.: Atlanta, GA, USA, 2013. [Google Scholar]
- Fanger, P.O. Thermal Comfort, Analysis and Application in Environmental Engineering; Mcgraw-Hill: New York, NY, USA, 1972. [Google Scholar]
- Pourshaghaghy, A.; Omidvari, M. Examination of thermal comfort in a hospital using PMV–PPD model. Appl. Ergon. 2012, 43, 1089–1095. [Google Scholar] [CrossRef] [PubMed]
- ISO 7730. Ergonomics of the Thermal Environment—Analytical Determination and Interpretation of Thermal Comfort Using Calculation of the PMV and PPD Indices and Local Thermal Comfort; International Standardization Organization: Geneva, Switzerland, 2005. [Google Scholar]
- Andersson, J.; Boerstra, A.; Clements-Croome, D.; Fitzner, K.; Hanssen, S.O. Indoor Climates and Productivity in Offices; REHVA (Federation of European Heating, Ventilation and Air Conditioning Associations): Brussels, Belgium, 2006. [Google Scholar]
- D’Agostino, D.; Congedo, P.M. CFD modeling and moisture dynamics implications of ventilation scenarios in historical buildings. Build. Environ. 2014, 79, 181–193. [Google Scholar] [CrossRef]
- Kephalopoulos, S.; Geiss, O.; Barrero-Moreno, J.; D’Agostino, D.; Paci, D. Promoting Healthy and Energy Efficient Buildings in the European Union—National Implementation of Related Requirements of the Energy Performance Buildings Directive (2010/31/EU); European Commission’s Science and Knowledge Service: Brussels, Belgium, 2016. [Google Scholar] [CrossRef]
- Seppänen, O.; Fisk, W.J.; Faulkner, D. Cost benefit analysis of the night-time ventilative cooling. In Proceedings of the 7th International Conference on Healthy Buildings 2003, Singapore, 7–11 December 2003; Volume 3, pp. 394–397. [Google Scholar]
- Hasan, A.; Vuolle, M.; Sirén, K. Minimisation of life cycle cost of a detached house using combined simulation and optimization. Build. Environ. 2008, 43, 2022–2034. [Google Scholar] [CrossRef]
- UNI 10375:2011. Calculation Method of the Indoor Temperature of a Room in the Warm Period. 2011. Available online: https://infostore.saiglobal.com/store/details.aspx?ProductID=1497581 (accessed on 8 October 2017).
- Castro-Lacouture, D.; Sefair, J.A.; Flórez, L.; Medaglia, A.L. Optimization model for the selection of materials using a LEED-based greenbuilding rating system in Colombia. Build. Environ. 2009, 44, 1162–1170. [Google Scholar] [CrossRef]
- Stazi, F.; Naspi, F.; Ulpiani, G.; Di Perna, C. Indoor air quality and thermal comfort optimization in classrooms developing an automatic system for windows opening and closing. Energy Build. 2017, 732–746. [Google Scholar] [CrossRef]
- Arif, M.; Katafygiotou, M.; Mazroei, A.; Kaushik, A.; Elsarrag, E. Impact of indoor environmental quality on occupant well-being and comfort: A review of the literature. Int. J. Sustain. Built Environ. 2016, 5, 1–11. [Google Scholar]
- Baglivo, C.; Congedo, P.M.; D’Agostino, D.; Zacà, I. Cost-optimal analysis and technical comparison between standard and high efficient mono-residential buildings in a warm climate. Energy 2015, 83, 560–575. [Google Scholar] [CrossRef]
- Zacà, I.; D’Agostino, D.; Congedo, P.M.; Baglivo, C. Data of cost-optimality and technical solutions for high energy performance buildings in warm climate. Data Brief 2015, 4, 222–225. [Google Scholar] [CrossRef] [PubMed]
- Zacà, I.; D’Agostino, D.; Congedo, P.M.; Baglivo, C. Assessment of cost-optimality and technical solutions in high performance multi-residential buildings in the Mediterranean area. Energy Build. 2015, 102, 250–265. [Google Scholar] [CrossRef]
- Congedo, P.M.; Baglivo, C.; D’Agostino, D.; Zacà, I. Cost-optimal design for nearly zero energy office buildings located in warm climates. Energy 2015, 91, 967–982. [Google Scholar] [CrossRef]
- Congedo, P.M.; Baglivo, C.; Zacà, I. High performance solutions and data for nzebs offices located in warm climates. Data Brief 2015, 5, 502–505. [Google Scholar] [CrossRef] [PubMed]
- Valdiserri, P.; Biserni, C. Energy performance of an existing office building in the northern part of Italy: Retrofitting actions and economic assessment. Sustain. Cities Soc. 2016, 27, 65–72. [Google Scholar] [CrossRef]
- Italian Standard UNI EN 10339. Impianti Aeraulici a Fini di Benessere. Generalità, Classificazione e Requisiti. Regole per la Richiesta d’Offerta, l’Offerta, l’Ordine e la Fornitura. 1995. Available online: http://store.uni.com/catalogo/index.php/uni-10339-1995.html (accessed on 8 October 2017).
- International Organization for Standardization, ISO 13786:2007. Thermal Performance of Building Components—Dynamic Thermal Characteristics—Calculation Methods. 2007. Available online: https://www.iso.org/standard/40892.html (accessed on 8 October 2017).
- Malvoni, M.; Baglivo, C.; Congedo, P.M.; Laforgia, D. CFD modelling to evaluate the thermal performances of window frames in accordance with the ISO 10077. Energy 2016, 111, 430–438. [Google Scholar] [CrossRef]
- Baglivo, C.; Malvoni, M.; Congedo, P.M. Data resulting from the CFD analysis of ten window frames according to the UNI EN ISO 10077-2. Data Brief 2016, 8, 963–965. [Google Scholar] [CrossRef] [PubMed]
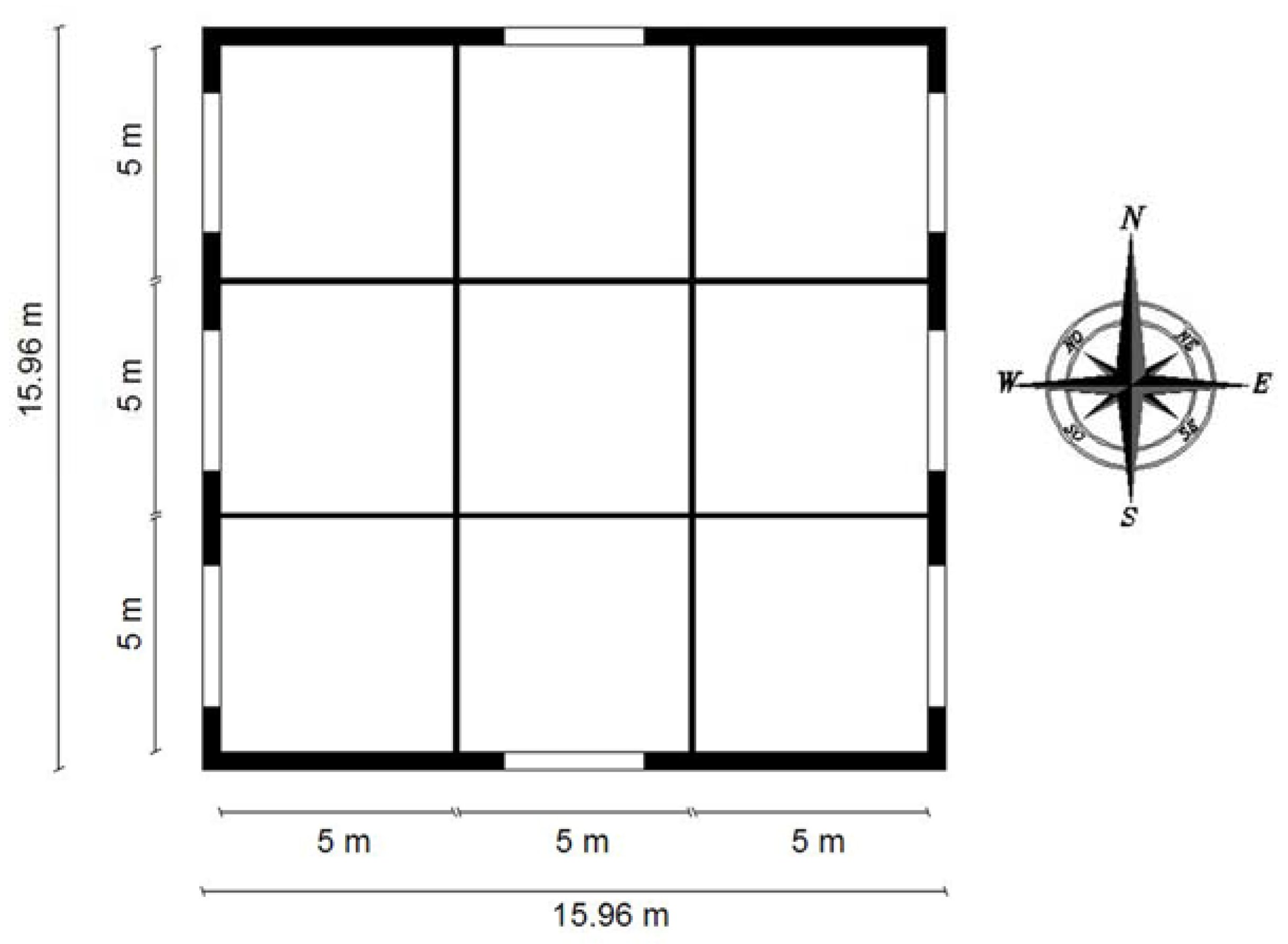










| FIRST STEP | External wall selection | Combo 1,2,3,4,5 |
| SECOND STEP | Slab on ground floor evaluation | Combo 4,6 |
| THIRD STEP | Analysis of the roof solar absorbance | Combo 6,7,8 |
| FOURTH STEP | Evaluation of the windows position | Combo 9,10 |
| FIFTH STEP | Sizing of windows | Combo 10,11,12 |
| SIXTH STEP | Study of the impact of window types | Combo 10,13,14 |
| SEVENTH STEP | Assessment of shading | Combo 10,15 |
| EIGHTH STEP | Estimation of the internal heat loads | Combo 10,15,16,17 |
| Combo | Window Position (Section 2.3.4, Figure 3) | Internal Gains (Section 2.3.8, Table 3) | Wall (Section 2.3.1, Table 4 and Table 5) | Slab on Ground Floor (Section 2.3.2, Table 4, Table 5 and Table 6) | Windows (Section 2.3.5, Section 2.3.6, Table 7) | Ceiling Absorptance (Section 2.3.3) | Shading (Section 2.3.7) |
|---|---|---|---|---|---|---|---|
| Combo 1 | NO WINDOWS | NO | W1 | IGF | NG | C0.5 (solar absorbance of 0.5) | NO |
| Combo 2 | W2 | IGF | NG | C0.5 (solar absorbance of 0.5) | |||
| Combo 3 | W3 | IGF | NG | C0.5 (solar absorbance of 0.5) | |||
| Combo 4 | W4 | IGF | NG | C0.5 (solar absorbance of 0.5) | |||
| Combo 5 | W5 | IGF | NG | C0.5 (solar absorbance of 0.5) | |||
| Combo 4 | NO WINDOWS | NO | W4 | IGF | NG | C0.5 (solar absorbance of 0.5) | NO |
| Combo 6 | W4 | NIGF | NG | C0.5 (solar absorbance of 0.5) | |||
| Combo 6 | NO WINDOWS | NO | W4 | NIGF | NG | C0.5 (solar absorbance of 0.5) | NO |
| Combo 7 | W4 | NIGF | NG | C0.3 (solar absorbance of 0.3) | |||
| Combo 8 | W4 | NIGF | NG | C0.7 (solar absorbance of 0.7) | |||
| Combo 9 | POSITION 1 | NO | W4 | NIGF | G1 (1/8 of the floor surface) | C0.3 (solar absorbance of 0.3) | NO |
| Combo 10 | POSITION 2 | W4 | NIGF | G1 (1/8 of the floor surface) | C0.3 (solar absorbance of 0.3) | ||
| Combo 10 | POSITION 2 | NO | W4 | NIGF | G1 (1/8 of the floor surface) | C0.3 (solar absorbance of 0.3) | NO |
| Combo 11 | POSITION 2 | W4 | NIGF | G1 (1/7 of the floor surface) | C0.3 (solar absorbance of 0.3) | ||
| Combo 12 | POSITION 2 | W4 | NIGF | G1 (1/9 of the floor surface) | C0.3 (solar absorbance of 0.3) | ||
| Combo 10 | POSITION 2 | NO | W4 | NIGF | G1 (1/8 of the floor surface) | C0.3 (solar absorbance of 0.3) | NO |
| Combo 13 | POSITION 2 | W4 | NIGF | G2 (1/8 of the floor surface) | C0.3 (solar absorbance of 0.3) | ||
| Combo 14 | POSITION 2 | W4 | NIGF | G3 (1/8 of the floor surface) | C0.3 (solar absorbance of 0.3) | ||
| Combo 10 | POSITION 2 | NO | W4 | NIGF | G1 (1/8 of the floor surface) | C0.3 (solar absorbance of 0.3) | NO |
| Combo 15 | POSITION 2 | NO | W4 | NIGF | G1 (1/8 of the floor surface) | C0.3 (solar absorbance of 0.3) | YES |
| Combo 16 | POSITION 2 | YES | W4 | NIGF | G1 (1/8 of the floor surface) | C0.3 (solar absorbance of 0.3) | YES |
| Combo 17 | POSITION 2 | YES | W4 | NIGF | G1 (1/8 of the floor surface) | C0.3 (solar absorbance of 0.3) | NO |
| Type Gain | Total Heat Gain (W) | Hour | |
|---|---|---|---|
| From | To | ||
| Occupants | 44 | 0:00 | 24:00 |
| Artificial lighting | 125 | 8:00 | 20:00 |
| Electronic equipment | 75 | 20:00 | 8:00 |
| Properties | Material | λ (W/m K) Thermal Conductivity | c (J/Kg K) Specific Heat Capacity | ρ (kg/m3) Density | Thicknesses (cm) |
|---|---|---|---|---|---|
| Wall | Bricks | 0.13 | 1000 | 600 | 20 |
| Tuff stone blocks | 1.7 | 850 | 2300 | 10 | |
| Polystyrene | 0.034 | 1700 | 35 | 8 | |
| Ground Floor | Stoneware flooring | 1.47 | 850 | 1700 | 1.5 |
| Concrete (1200 kg/m3) | 0.47 | 850 | 1200 | 8 | |
| Concrete (1600 kg/m3) | 0.73 | 850 | 1600 | 5 | |
| EPS polystyrene panel | 0.035 | 850 | 35 | 5 | |
| Crawl space with igloo | 0.072 | 850 | 1000 | 16 | |
| Screed ordinary concrete | 1.06 | 850 | 1700 | 5 | |
| Gravel | 1.2 | 850 | 1700 | 1 | |
| Roof | Hollow-core concrete | 0.743 | 850 | 1800 | 25 |
| Concrete (400 kg/m3) | 0.19 | 850 | 400 | 10 | |
| XPS polystyrene panel | 0.04 | 850 | 35 | 8 | |
| Tuff stone blocks | 1.7 | 850 | 2300 | 10 | |
| Tuff stone tiles | 0.55 | 850 | 1600 | 5 |
| External Walls | U | Y12 | Y22 | Y11 | fd | Ms | ∆t | k1 | k2 | d | |
|---|---|---|---|---|---|---|---|---|---|---|---|
 | W/m2K | W/m2K | W/m2K | W/m2K | Kg/m2 | h | kJ/m2K | kJ/m2K | m | ||
| W1 |  | 0.243 | 0.033 | 10.559 | 0.409 | 0.136 | 352.8 | 13.3 | 5.95 | 145.38 | 0.38 |
| W2 |  | 0.243 | 0.032 | 10.631 | 1.9 | 0.132 | 352.8 | 13.57 | 26.56 | 146.28 | 0.38 |
| W3 |  | 0.243 | 0.008 | 2.233 | 0.424 | 0.033 | 352.8 | 13.51 | 5.91 | 30.74 | 0.38 |
| W4 |  | 0.243 | 0.019 | 0.423 | 5.841 | 0.078 | 352.8 | 14.53 | 80.43 | 5.93 | 0.38 |
| W5 |  | 0.243 | 0.021 | 2.221 | 5.973 | 0.086 | 352.8 | 15.15 | 82.22 | 30.56 | 0.38 |
| Ground Floor | U W/m2K | D m | |
|---|---|---|---|
| IGF |  | 0.233 | 0.415 |
| NIGF |  | 0.349 | 0.365 |
| Window Measures | Ug (W/m2K) | g | % Frame | Uf (W/m2K) | |
|---|---|---|---|---|---|
| G1 | 6/16/6 Argon | 1.3 | 0.333 | 15 | 2.27 |
| G2 | 4/16/4 Argon | 1.4 | 0.589 | 15 | 2.27 |
| G3 | 4/8/4/8/4 Krypton | 0.7 | 0.407 | 15 | 2.27 |
| Mean surface temperature (°C) | 10 |
| Amplitude of surface temperature (°C) | 5 |
| Soil thermal conductivity (kJ/h m K) | 8.72 |
| Soil density (kg/m3) | 3200 |
| Soil specific heat (kJ/kg K) | 0.84 |
| Summer Peaks (°C) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Step | Combo | TOP_S | TOP_SE | TOP_W | TOP_E | TOP_N | TOP_SW | TOP_CENTRAL | TOP_NW | TOP_NE |
| First Step | Combo 1 | 26.61 | 27.03 | 26.79 | 26.51 | 26.27 | 27.24 | 26.16 | 26.94 | 26.62 |
| Combo 2 | 26.56 | 26.95 | 26.73 | 26.47 | 26.22 | 27.15 | 26.12 | 26.84 | 26.55 | |
| Combo 3 | 26.57 | 26.99 | 26.76 | 26.47 | 26.24 | 27.21 | 26.12 | 26.90 | 26.59 | |
| Combo 4 | 26.41 | 26.75 | 26.57 | 26.34 | 26.08 | 26.91 | 25.99 | 26.61 | 26.38 | |
| Combo 5 | 26.45 | 26.80 | 26.61 | 26.38 | 26.12 | 26.95 | 26.04 | 26.66 | 26.43 | |
| Second Step | Combo 4 | 26.41 | 26.75 | 26.57 | 26.34 | 26.08 | 26.91 | 25.99 | 26.61 | 26.38 |
| Combo 6 | 25.55 | 25.96 | 25.71 | 25.48 | 25.22 | 26.12 | 25.07 | 25.81 | 25.58 | |
| Third Step | Combo 6 | 25.55 | 25.96 | 25.71 | 25.48 | 25.22 | 26.12 | 25.07 | 25.81 | 25.58 |
| Combo 7 | 24.99 | 25.44 | 25.15 | 24.92 | 24.66 | 25.60 | 24.46 | 25.28 | 25.05 | |
| Combo 8 | 26.10 | 26.47 | 26.26 | 26.04 | 25.78 | 26.62 | 25.66 | 26.33 | 26.09 | |
| Fourth Step | Combo 9 | 28.31 | 28.90 | 29.07 | 28.67 | 27.65 | 29.43 | 27.48 | 29.09 | 28.55 |
| Combo 10 | 27.70 | 28.11 | 28.36 | 27.93 | 26.84 | 28.21 | 26.82 | 27.46 | 27.22 | |
| Fifth Step | Combo 10 | 27.70 | 28.11 | 28.36 | 27.93 | 26.84 | 28.21 | 26.82 | 27.46 | 27.22 |
| Combo 11 | 28.05 | 28.45 | 28.80 | 28.33 | 27.12 | 28.56 | 27.13 | 27.76 | 27.49 | |
| Combo 12 | 27.42 | 27.83 | 28.02 | 27.61 | 26.62 | 27.94 | 26.58 | 27.23 | 27.00 | |
| Sixth Step | Combo 10 | 27.70 | 28.11 | 28.36 | 27.93 | 26.84 | 28.21 | 26.82 | 27.46 | 27.22 |
| Combo 13 | 29.81 | 30.16 | 30.87 | 30.29 | 28.46 | 30.22 | 28.61 | 29.13 | 28.83 | |
| Combo 14 | 28.21 | 28.61 | 29.06 | 28.63 | 27.26 | 28.69 | 27.27 | 27.86 | 27.64 | |
| Seventh Step | Combo 10 | 27.70 | 28.11 | 28.36 | 27.93 | 26.84 | 28.21 | 26.82 | 27.46 | 27.22 |
| Combo 15 | 25.83 | 26.21 | 26.09 | 25.79 | 25.56 | 26.36 | 25.24 | 26.13 | 25.90 | |
| Eighth Step | Combo 10 | 27.70 | 28.11 | 28.36 | 27.93 | 26.84 | 28.22 | 26.82 | 27.46 | 27.22 |
| Combo 15 | 25.83 | 26.21 | 26.09 | 25.79 | 25.56 | 26.36 | 25.24 | 26.13 | 25.90 | |
| Combo 16 | 28.91 | 29.05 | 29.21 | 28.21 | 28.68 | 29.22 | 28.66 | 29.04 | 28.81 | |
| Combo 17 | 30.78 | 30.94 | 31.47 | 30.98 | 29.97 | 31.05 | 30.24 | 30.37 | 30.12 | |
| Winter Peaks (°C) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Step | Combo | TOP_S | TOP_SE | TOP_W | TOP_E | TOP_N | TOP_SW | TOP_CENTRAL | TOP_NW | TOP_NE |
| First Step | Combo 1 | 7.40 | 7.23 | 7.32 | 7.32 | 7.27 | 7.23 | 7.46 | 7.13 | 7.13 |
| Combo 2 | 7.51 | 7.35 | 7.43 | 7.42 | 7.37 | 7.35 | 7.55 | 7.24 | 7.24 | |
| Combo 3 | 7.55 | 7.38 | 7.46 | 7.46 | 7.41 | 7.39 | 7.58 | 7.27 | 7.27 | |
| Combo 4 | 7.78 | 7.65 | 7.69 | 7.69 | 7.63 | 7.65 | 7.76 | 7.53 | 7.52 | |
| Combo 5 | 7.68 | 7.54 | 7.59 | 7.59 | 7.54 | 7.55 | 7.68 | 7.43 | 7.42 | |
| Second Step | Combo 4 | 7.78 | 7.65 | 7.69 | 7.69 | 7.63 | 7.65 | 7.76 | 7.53 | 7.52 |
| Combo 6 | 7.55 | 7.44 | 7.46 | 7.46 | 7.40 | 7.44 | 7.53 | 7.32 | 7.31 | |
| Third Step | Combo 6 | 7.55 | 7.44 | 7.46 | 7.46 | 7.40 | 7.44 | 7.53 | 7.32 | 7.31 |
| Combo 7 | 7.43 | 7.335 | 7.34 | 7.34 | 7.29 | 7.33 | 7.39 | 7.20 | 7.20 | |
| Combo 8 | 7.67 | 7.55 | 7.58 | 7.58 | 7.52 | 7.55 | 7.65 | 7.43 | 7.426 | |
| Fourth Step | Combo 9 | 7.98 | 7.79 | 7.81 | 7.79 | 7.70 | 7.81 | 7.96 | 7.62 | 7.59 |
| Combo 10 | 8.22 | 8.06 | 7.95 | 7.94 | 7.76 | 8.07 | 8.10 | 7.66 | 7.65 | |
| Fifth Step | Combo 10 | 8.22 | 8.06 | 7.95 | 7.94 | 7.76 | 8.07 | 8.10 | 7.66 | 7.65 |
| Combo 11 | 8.31 | 8.15 | 8.02 | 8.01 | 7.82 | 8.16 | 8.19 | 7.71 | 7.70 | |
| Combo 12 | 8.14 | 7.99 | 7.89 | 7.88 | 7.72 | 8.00 | 8.03 | 7.62 | 7.61 | |
| Sixth Step | Combo 10 | 8.22 | 8.06 | 7.95 | 7.94 | 7.76 | 8.07 | 8.10 | 7.66 | 7.65 |
| Combo 13 | 9.01 | 8.82 | 8.61 | 8.59 | 8.32 | 8.83 | 8.79 | 8.19 | 8.17 | |
| Combo 14 | 8.52 | 8.35 | 8.21 | 8.19 | 7.99 | 8.36 | 8.35 | 7.88 | 7.87 | |
| Seventh Step | Combo 10 | 8.22 | 8.06 | 7.95 | 7.94 | 7.76 | 8.07 | 8.10 | 7.66 | 7.65 |
| Combo 15 | 7.38 | 7.27 | 7.29 | 7.29 | 7.23 | 7.27 | 7.42 | 7.15 | 7.14 | |
| Eighth Step | Combo 10 | 8.22 | 8.06 | 7.95 | 7.94 | 7.76 | 8.07 | 8.10 | 7.66 | 7.65 |
| Combo 15 | 7.38 | 7.27 | 7.29 | 7.29 | 7.23 | 7.27 | 7.42 | 7.151 | 7.14 | |
| Combo 16 | 10.41 | 10.07 | 10.35 | 10.35 | 10.30 | 10.07 | 10.78 | 10.00 | 10.00 | |
| Combo 17 | 11.25 | 10.86 | 11.00 | 10.99 | 10.83 | 10.87 | 11.46 | 10.51 | 10.50 | |
| Y12 | Y22 | Y11 | fd | ∆t | k1 | k2 | Summer TOP | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| W/m2K | W/m2K | W/m2K | h | kJ/m2K | kJ/m2K | S | SE | W | E | N | SW | CENTRAL | NW | NE | ||
| W1 | 0.033 | 10.559 | 0.409 | 0.136 | 13.3 | 5.95 | 145.38 | 26.61 | 27.03 | 26.79 | 26.51 | 26.27 | 27.24 | 26.16 | 26.94 | 26.62 |
| W2 | 0.032 | 10.631 | 1.9 | 0.132 | 13.57 | 26.56 | 146.28 | 26.56 | 26.95 | 26.73 | 26.47 | 26.22 | 27.15 | 26.12 | 26.84 | 26.55 |
| W3 | 0.008 | 2.233 | 0.424 | 0.033 | 13.51 | 5.91 | 30.74 | 26.57 | 26.99 | 26.76 | 26.47 | 26.24 | 27.21 | 26.12 | 26.9 | 26.59 |
| W4 | 0.019 | 0.423 | 5.841 | 0.078 | 14.53 | 80.43 | 5.93 | 26.41 | 26.75 | 26.57 | 26.34 | 26.08 | 26.91 | 25.99 | 26.61 | 26.38 |
| W5 | 0.021 | 2.221 | 5.973 | 0.086 | 15.15 | 82.22 | 30.56 | 26.45 | 26.8 | 26.61 | 26.38 | 26.12 | 26.95 | 26.04 | 26.66 | 26.43 |
| Y12 | Y22 | Y11 | fd | ∆t | k1 | k2 | Winter TOP | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| W/m2K | W/m2K | W/m2K | h | kJ/m2K | kJ/m2K | S | SE | W | E | N | SW | CENTRAL | NW | NE | ||
| W1 | 0.033 | 10.559 | 0.409 | 0.136 | 13.3 | 5.95 | 145.38 | 7.4 | 7.23 | 7.32 | 7.32 | 7.27 | 7.23 | 7.46 | 7.13 | 7.13 |
| W2 | 0.032 | 10.631 | 1.9 | 0.132 | 13.57 | 26.56 | 146.28 | 7.51 | 7.35 | 7.43 | 7.42 | 7.37 | 7.35 | 7.55 | 7.24 | 7.24 |
| W3 | 0.008 | 2.233 | 0.424 | 0.033 | 13.51 | 5.91 | 30.74 | 7.55 | 7.38 | 7.46 | 7.46 | 7.41 | 7.39 | 7.58 | 7.27 | 7.27 |
| W4 | 0.019 | 0.423 | 5.841 | 0.078 | 14.53 | 80.43 | 5.93 | 7.78 | 7.65 | 7.69 | 7.69 | 7.63 | 7.65 | 7.76 | 7.53 | 7.52 |
| W5 | 0.021 | 2.221 | 5.973 | 0.086 | 15.15 | 82.22 | 30.56 | 7.68 | 7.54 | 7.59 | 7.59 | 7.54 | 7.55 | 7.68 | 7.43 | 7.42 |
| Combo 17—Operative Air Temperature (°C) | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| Annual Temperature | S | SE | W | E | N | SW | Central | NW | NE |
| Minimum Value | 11.25 | 10.86 | 11.00 | 10.99 | 10.83 | 10.87 | 11.46 | 10.52 | 10.51 |
| Middle Value | 21.09 | 20.94 | 20.74 | 20.72 | 20.17 | 20.96 | 20.73 | 20.11 | 20.09 |
| Maximum Value | 30.78 | 30.94 | 31.47 | 30.98 | 29.97 | 31.05 | 30.24 | 30.37 | 30.12 |
| Average Monthly Temperature | S | SE | W | E | N | SW | Central | NW | NE |
| January | 13.62 | 13.37 | 13.00 | 12.98 | 12.62 | 13.39 | 13.19 | 12.46 | 12.45 |
| February | 15.25 | 15.10 | 14.74 | 14.79 | 14.25 | 15.08 | 14.80 | 14.14 | 14.18 |
| March | 15.58 | 15.43 | 15.07 | 15.10 | 14.54 | 15.42 | 15.09 | 14.45 | 14.47 |
| April | 18.61 | 18.44 | 18.41 | 18.31 | 17.79 | 18.51 | 18.33 | 17.75 | 17.68 |
| May | 21.19 | 21.16 | 21.29 | 21.25 | 20.69 | 21.19 | 21.04 | 20.74 | 20.71 |
| June | 25.28 | 25.27 | 25.58 | 25.53 | 25.05 | 25.31 | 25.30 | 25.10 | 25.07 |
| July | 28.45 | 28.47 | 28.73 | 28.66 | 28.12 | 28.52 | 28.39 | 28.22 | 28.18 |
| August | 29.50 | 29.43 | 29.46 | 29.39 | 28.75 | 29.48 | 29.24 | 28.80 | 28.75 |
| September | 27.64 | 27.45 | 27.11 | 27.09 | 26.35 | 27.47 | 27.10 | 26.29 | 26.27 |
| October | 24.49 | 24.23 | 23.69 | 23.64 | 22.97 | 24.26 | 23.80 | 22.86 | 22.83 |
| November | 19.03 | 18.73 | 18.26 | 18.30 | 17.79 | 18.71 | 18.54 | 17.57 | 17.60 |
| December | 15.30 | 15.00 | 14.55 | 14.53 | 14.10 | 15.02 | 14.79 | 13.90 | 13.89 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baglivo, C.; Congedo, P.M.; Di Cataldo, M.; Coluccia, L.D.; D’Agostino, D. Envelope Design Optimization by Thermal Modelling of a Building in a Warm Climate. Energies 2017, 10, 1808. https://doi.org/10.3390/en10111808
Baglivo C, Congedo PM, Di Cataldo M, Coluccia LD, D’Agostino D. Envelope Design Optimization by Thermal Modelling of a Building in a Warm Climate. Energies. 2017; 10(11):1808. https://doi.org/10.3390/en10111808
Chicago/Turabian StyleBaglivo, Cristina, Paolo Maria Congedo, Matteo Di Cataldo, Luigi Damiano Coluccia, and Delia D’Agostino. 2017. "Envelope Design Optimization by Thermal Modelling of a Building in a Warm Climate" Energies 10, no. 11: 1808. https://doi.org/10.3390/en10111808
APA StyleBaglivo, C., Congedo, P. M., Di Cataldo, M., Coluccia, L. D., & D’Agostino, D. (2017). Envelope Design Optimization by Thermal Modelling of a Building in a Warm Climate. Energies, 10(11), 1808. https://doi.org/10.3390/en10111808








