Design and Analysis of a New Torque Vectoring System with a Ravigneaux Gearset for Vehicle Applications
Abstract
:1. Introduction
2. Current Torque Vectoring Differential
3. Design of New Torque Vectoring Differential
4. Modeling
5. Simulation Results
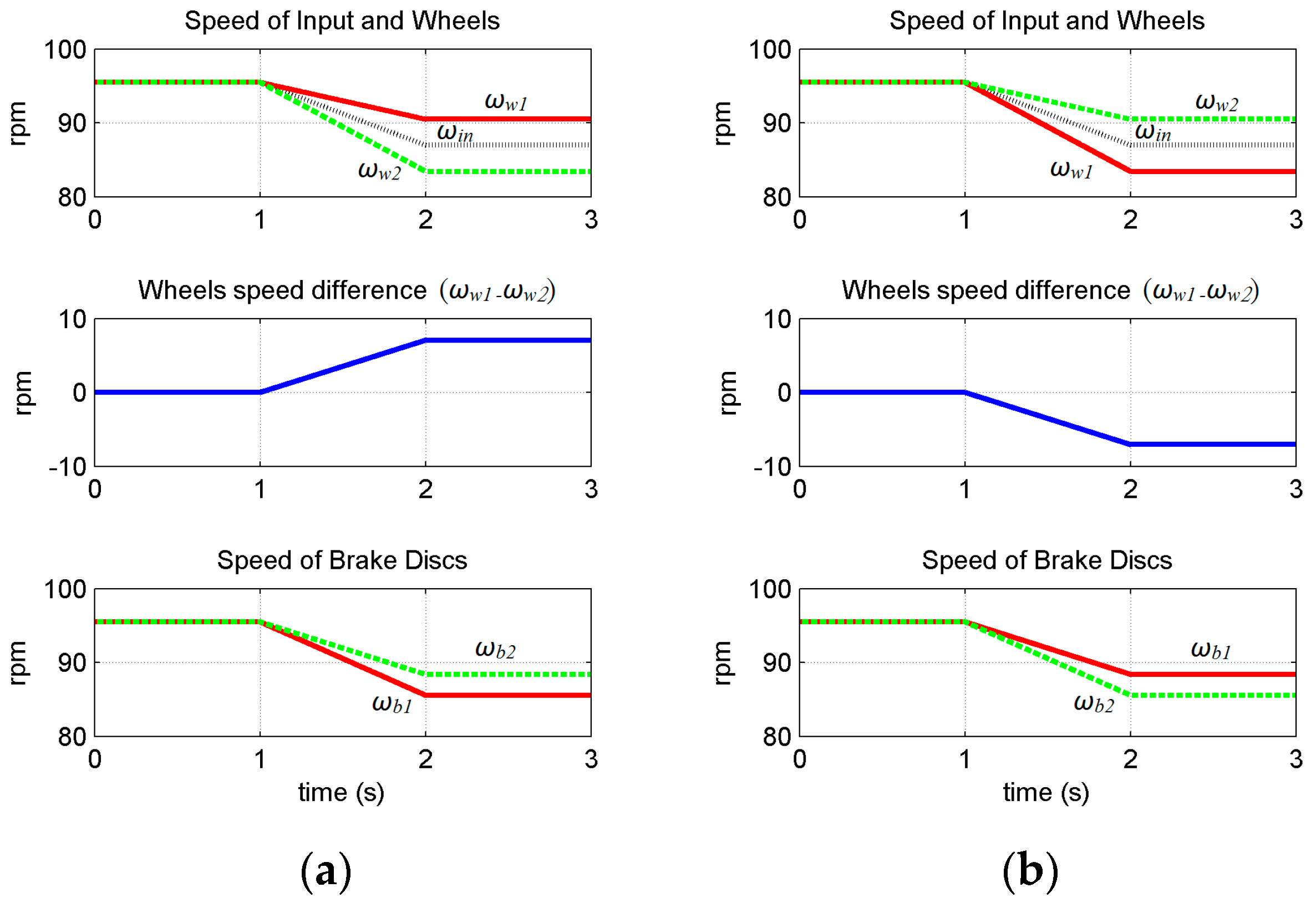
6. Analysis of the Numerical Simulation
7. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Nomenclature
| DYC | direct yaw-moment control |
| ESP | electronic stability program |
| 4WS | four-wheel steering |
| TVD | torque vectoring differentials |
| DG | differential gearset |
| SPC-TVD | superposition clutch TVD |
| STC-TVD | stationary clutch TVD |
| Rav-TVD | Ravigneaux TVD |
| FPG | function power graph |
| DoF | degree-of-freedom |
| SA | solid axle |
| OD | open differential |
| P controller | Proportional controller |
| NA | no actuation |
| CAE | computer-aided engineering |
| CAD | computer aided design |
| 3D | three-dimensional |
| RL | rear left |
| RR | rear right |
| NS | neutral-steering |
| OS | over-steering |
| US | under-steering |
| Subscripts | |
| W1, W2 | left and right wheels |
| C1, C2 | clutches |
| B1, B2 | brakes |
| G, G0, G1, G2 | gear pairs |
| PG1, PG2 | planetary gearsets |
| IN | input of the engine power |
| r | ring gear |
| c | carrier |
| ss | small sun gear |
| sl | large sun gear |
Appendix A
| Parameters | Value |
|---|---|
| 1. Dimensions of the original Rav-TVD | |
| Radii of ring gear Rr | 60 mm |
| Radii of large sun gear Rsl | 40 mm |
| Radii of small sun gear Rss | 30 mm |
| 2. Dimensions of the original vehicle | |
| Mass | 1200 kg |
| Wheel radius (unloaded) | 310 mm |
| Tire width | 210 mm |
| Wheel base | 2.6 m |
| Truck width | 1.8 m |
| Inertia of the wheel | 0.3381 kg·m2 |
| Inertia of the powertrain | 1 kg·m2 |
| Rolling resistance coefficient of the tire | 0.01 |
| Aerodynamic drag coefficient Cd | 0.3 |
| Air density ρ | 1.204 kg/m³ |
| Frontal area of the vehicle A | 1.5 m² |
| 3. Setting of the original system parameters | |
| Gear ratio of ring gear and large sun gear isl (Rr/Rsl) | 1.5 |
| Gear ratio of ring gear and small sun gear iss (Rr/Rss) | 2.0 |
| Max. press-on force of the brakes B1 & B2 (Corresponding braking torque) | 7200 N (230 N·m) |
| Vehicle speed | 60 km/h |
| Steering angle (starts at t = 0.2 s) | 5 degree |
References
- Shibahata, Y.; Shimada, K.; Tomari, T. Improvement of vehicle maneuverability by direct yaw moment control. Veh. Syst. Dyn. 1993, 22, 465–481. [Google Scholar] [CrossRef]
- Geng, C.; Mostefai, L.; Denaï, M.; Hori, Y. Direct yaw-moment control of an in-wheel-motored electric vehicle based on body slip angle fuzzy observer. IEEE Trans. Ind. Electron. 2009, 56, 1411–1419. [Google Scholar] [CrossRef] [Green Version]
- Yim, S.; Park, Y.; Yi, K. Design of active suspension and electronic stability program for rollover prevention. Int. J. Automot. Technol. 2010, 11, 147–153. [Google Scholar] [CrossRef]
- Furukawa, Y.; Yuhara, N.; Sano, S.; Takeda, H.; Matsushita, Y. A review of four-wheel steering studies from the viewpoint of vehicle dynamics and control. Veh. Syst. Dyn. 1989, 18, 151–186. [Google Scholar] [CrossRef]
- Shibahata, Y. Torque Distributing Mechanism in Differential. U.S. Patent No. 5387161, 7 February 1995. [Google Scholar]
- Richardson, J.A. Speed Reduction Gearset and Torque Split Differential Mechanism. U.S. Patent No. 5,643,129, 1 January 1997. [Google Scholar]
- Gumpoltsberger, G.; Baasch, D. Transmission for Distributing a Drive Torque. U.S. Patent No. 7,056,252, 6 June 2006. [Google Scholar]
- Gradu, M. Differential with Torque Vectoring Capabilities. U.S. Patent No. 7,238,140, 3 July 2007. [Google Scholar]
- Platt, W. Continuously Variable Torque Vectoring Axle Assembly. U.S. Patent No. 7,951,035, 31 May 2011. [Google Scholar]
- Wheals, J.C.; Baker, H.; Ramsey, K.; Turner, W. Torque vectoring AWD driveline: Design, simulation, capabilities and control (No. 2004-01-0863). SAE Tech. Pap. 2004. [Google Scholar] [CrossRef]
- Kakalis, L.; Cheli, F.; Sabbioni, E. The Development of a Brake based Torque Vectoring System for a Sport Vehicle Performance Improvement. In Proceedings of the 6th International Conference on Informatics in Control, Automation and Robotics, Intelligent Control Systems and Optimization, Milan, Italy, 2–5 July 2009; pp. 298–304. [Google Scholar]
- Lin, C.; Xu, Z. Wheel torque distribution of four-wheel-drive electric vehicles based on multi-objective optimization. Energies 2015, 8, 3815–3831. [Google Scholar] [CrossRef]
- De Pinto, S.; Camocardi, P.; Sorniotti, A.; Gruber, P.; Perlo, P.; Viotto, F. Torque-Fill Control and Energy Management for a Four-Wheel-Drive Electric Vehicle Layout With Two-Speed Transmissions. IEEE Trans. Ind. Appl. 2017, 53, 447–458. [Google Scholar] [CrossRef]
- De Novellis, L.; Sorniotti, A.; Gruber, P.; Shead, L.; Ivanov, V.; Hoepping, K. Torque vectoring for electric vehicles with individually controlled motors: State-of-the-art and future developments. In Proceedings of the 26th Electric Vehicle Symposium, Los Angeles, CA, USA, 6–9 May 2012. [Google Scholar]
- Deur, J.; Ivanović, V.; Hancock, M.; Assadian, F. Modeling and analysis of active differential dynamics. J. Dyn. Syst. Meas. Control 2010, 132, 061501. [Google Scholar] [CrossRef]
- Chen, I.M.; Yang, T.H.; Liu, T. Function Power Graph A Novel Methodology for Powertrain and Hybrid System Conceptual Design and Analysis. In Proceedings of the 14th IFToMM World Congress, Taipei, Taiwan, 25–30 October 2015; pp. 544–552. [Google Scholar]
- Wang, C.; Zhao, Z.; Zhang, T.; Dai, X.; Yuan, X. Development of a compact compound power-split hybrid transmission based on altered Ravigneaux gear set. SAE Tech. Pap. 2014. [Google Scholar] [CrossRef]
- Zhang, Y.; Ma, X.; Yin, C.; Yuan, S. Development and Simulation of a Type of Four-Shaft ECVT for a Hybrid Electric Vehicle. Energies 2016, 9, 141. [Google Scholar] [CrossRef]
- Zhao, L.; Zhou, Y.; Zheng, L. Modeling and simulation of AMT clutch actuator based on simulationX. In Proceedings of the CiSE 2009. International Conference on Computational Intelligence and Software Engineering, Wuhan, China, 11–13 December 2009; pp. 1–5. [Google Scholar]
- Chen, L.; Xi, G.; Yin, C.L. Model referenced adaptive control to compensate slip-stick transition during clutch engagement. Int. J. Automot. Technol. 2011, 12, 913–920. [Google Scholar] [CrossRef]
- Guo, W.; Wang, S.H.; Su, C.G.; Li, W.Y.; Xu, X.Y.; Cui, L.Y. Method for precise controlling of the at shift control system. Int. J. Automot. Technol. 2014, 15, 683–698. [Google Scholar] [CrossRef]
- Farkas, Z.; Jóri, I.J.; Kerényi, G. The Application and Modelling Possibilities of CVT in Tractor. In Proceedings of the 5th International Conference Multidisciplinary, Baia Mare, Romania, 23–24 May 2003; pp. 145–150. [Google Scholar]
- Ji, J.; Jang, M.J.; Kwon, O.E.; Chai, M.J.; Kim, H.S. Power transmission dynamics in micro and macro slip regions for a metal v-belt continuously variable transmission under external vibrations. Int. J. Automot. Technol. 2014, 15, 1119–1128. [Google Scholar] [CrossRef]
- Kim, D.M.; Kim, S.C.; Noh, D.K.; Jang, J.S. Jerk phenomenon of the hydrostatic transmission through the experiment and analysis. Int. J. Automot. Technol. 2015, 16, 783–790. [Google Scholar] [CrossRef]
- Tang, P.; Wang, S.; Liu, Y.; Xu, X. Analysis of the oil pressure rule during the shift process of automatic transmission. In Proceedings of the 2010 Seventh International Conference on Fuzzy Systems and Knowledge Discovery (FSKD), Yantai, China, 10–12 August 2010; Volume 1, pp. 109–113. [Google Scholar]
- Pengxiang, T.; Shuhan, W.; Xiangyang, X.; Wenyong, L.; Lin, S.; Guoru, Z. Notice of Retraction Design of system pressure valve of 8-speed automatic transmission. In Proceedings of the 2010 International Conference on Computer Application and System Modeling (ICCASM), Taiyuan, China, 22–24 October 2010; Volume 4. [Google Scholar]
- Wang, S.H.; Xu, X.Y.; Liu, Y.F.; Dai, Z.K.; Tenberge, P.; Qu, W. Design and dynamic simulation of hydraulic system of a new automatic transmission. J. Cent. South Univ. Technol. 2009, 16, 697–701. [Google Scholar] [CrossRef]
- Wei, G.; Xiangyang, X.; Yongxin, C.; Yang, Y. Simulation of powertrain and dynamics of automobile based on SimulationX. In Proceedings of the 2011 6th IEEE Conference on Industrial Electronics and Applications (ICIEA), Beijing, China, 21–23 June 2011; pp. 2326–2330. [Google Scholar]
- Dai, Z.; Liu, Y.; Xu, X.; Wang, S. The Application of Multi-domain Physical System Simulation Method in the Study of Automatic Transmissions. In Proceedings of the WCSE’09. WRI World Congress on Software Engineering, Xiamen, China, 19–21 May 2009; Volume 2, pp. 504–508. [Google Scholar]
- Belmon, L.; Yan, J.; Abel, A. Modelling and Simulation of DCT Gearshifting for Real-Time and High-Fidelity Analysis. In Proceedings of the FISITA 2012 World Automotive Congress, Beijing, China, 27–30 November 2013; pp. 399–411. [Google Scholar]
- Li, W.; Abel, A.; Todtermuschke, K.; Zhang, T. Hybrid vehicle power transmission modeling and simulation with simulationX. In Proceedings of the ICMA 2007, International Conference on Mechatronics and Automation, Harbin, China, 5–8 August 2007; pp. 1710–1717. [Google Scholar]
- Ma, X.; Zhang, Y.; Yin, C. Kinematic Study and Mode Analysis of a New 2-Mode Hybrid Transmission. In Proceedings of the FISITA 2012 World Automotive Congress, Beijing, China, 27–30 November 2013; pp. 309–318. [Google Scholar]
- Abel, A.; Adir, A.; Blochwitz, T.; Greenberg, L.; Salman, T. Development and verification of complex hybrid systems using synthesizable monitors. In Proceedings of the Haifa Verification Conference, Haifa, Israel, 5–7 November 2013; pp. 182–198. [Google Scholar]
- Bös, M. Subsystem- and full-vehicle-simulation of mobile machines using Simulation X. In Proceedings of the 15th ITI Symposium, Dresden, Germany, 14–15 November 2012. [Google Scholar]
- Tüschen, T. SIMULATORS–‘auto. mobile-driving simulator’–suspensions design of a wheel-based driving simulator. In Proceedings of the 7th International Munich Chassis Symposium 2016, Munich, Germany, 14–15 June 2016; Springer Fachmedien Wiesbaden: Wiesbaden, Germany, 2017; pp. 411–434. [Google Scholar]
















| No. | Connected Units | Effect of the Two Brakes to the Speed Ratio between IN-W1 Shafts | |||
|---|---|---|---|---|---|
| IN | W1 | B1 | B2 | ||
| 1 | r | c | sl | ss | ∆ |
| 2 * | r | sl | ss | c | ∆ |
| 3 | r | ss | c | sl | ● |
| 4 | c | r | sl | ss | ∆ |
| 5 | c | sl | r | ss | ● |
| 6 | c | ss | r | sl | ∆ |
| 7 | sl | r | c | ss | ● |
| 8 | sl | c | r | ss | ● |
| 9 | sl | ss | r | c | ● |
| 10 | ss | r | c | sl | ● |
| 11 | ss | c | r | sl | ● |
| 12 | ss | sl | r | c | ● |
| Parameter | Value |
|---|---|
| Inertia of the input shaft Iin | 0.18 (kg·m2) |
| Inertia of the wheels Iw1 and Iw2 | 2.70 (kg·m2) |
| Inertia of the brakes Ib1 and Ib2 | 0.01 (kg·m2) |
| Radii of the ring gear Rr | 60 (mm) |
| Radii of the large sun gear Rsl | 40 (mm) |
| Radii of the small sun gear Rss | 30 (mm) |
| Torque on the input shaft Tin | 20 (N·m) |
| Torque on the wheels Tw1 and Tw2 | −10 (N·m) |
| Torque applied to the brakes Tb1 and Tb2 | −5 (N·m) |
| Parameter | Variation | Value | Result (y-Axis at t = 5 s) | Difference |
|---|---|---|---|---|
| Gear ratio of ring gear and large sun gear isl | +10% | 1.65 | 1.910 m | −5.45% |
| 0 | 1.5 | 2.020 m | 0% | |
| −10% | 1.35 | 2.144 m | +6.14% | |
| Gear ratio of ring gear and small sun gear iss | +10% | 2.2 | −1.642 m | +19.35% |
| 0 | 2 | −2.036 m | 0% | |
| −10% | 1.8 | −2.432 m | −19.45% | |
| Max. press-on force of the brake B1 | +10% | 7920 N | 2.213 m | +9.55% |
| 0 | 7200 N | 2.020 m | 0% | |
| −10% | 6480 N | 1.826 m | −9.60% | |
| Max. press-on force of the brake B2 | +10% | 7920 N | −2.233 m | +9.68% |
| 0 | 7200 N | −2.036 m | 0% | |
| −10% | 6480 N | −1.840 m | −9.63% |
| Parameter | Value | The Radius of Curvature of the Vehicle Trajectory | ||
|---|---|---|---|---|
| No Brakes Engaged | Brake B1 Is Engaged | Brake B2 Is Engaged | ||
| Vehicle speed | 30 km/h | - | 1273 m | 1268 m |
| 60 km/h | - | 1450 m | 1436 m | |
| 75 km/h | - | 1589 m | 1570 m | |
| 90 km/h | - | × | × | |
| Steering angle | 1 degree | 167.1 m | 149.7 m | 188.9 m |
| 3 degree | 56.4 m | 53.7 m | 59.0 m | |
| 5 degree | 37.8 m | 36.7 m | 38.6 m | |
| 7 degree | × | × | × | |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.-F.; Chen, I.-M.; Chang, J.; Liu, T. Design and Analysis of a New Torque Vectoring System with a Ravigneaux Gearset for Vehicle Applications. Energies 2017, 10, 2157. https://doi.org/10.3390/en10122157
Chen Y-F, Chen I-M, Chang J, Liu T. Design and Analysis of a New Torque Vectoring System with a Ravigneaux Gearset for Vehicle Applications. Energies. 2017; 10(12):2157. https://doi.org/10.3390/en10122157
Chicago/Turabian StyleChen, Yu-Fan, I-Ming Chen, Joshua Chang, and Tyng Liu. 2017. "Design and Analysis of a New Torque Vectoring System with a Ravigneaux Gearset for Vehicle Applications" Energies 10, no. 12: 2157. https://doi.org/10.3390/en10122157
APA StyleChen, Y.-F., Chen, I.-M., Chang, J., & Liu, T. (2017). Design and Analysis of a New Torque Vectoring System with a Ravigneaux Gearset for Vehicle Applications. Energies, 10(12), 2157. https://doi.org/10.3390/en10122157




