An Improved Method for Energy and Resource Assessment of Waves in Finite Water Depths
Abstract
:1. Introduction
2. Waves in Finite Water Depths
2.1. Wavelength in Finite Water Depth
2.2. Approximations for Wave Energy Calculation
2.2.1. Deep Water Conditions
2.2.2. Spectral Moments
2.2.3. Wave Statistics
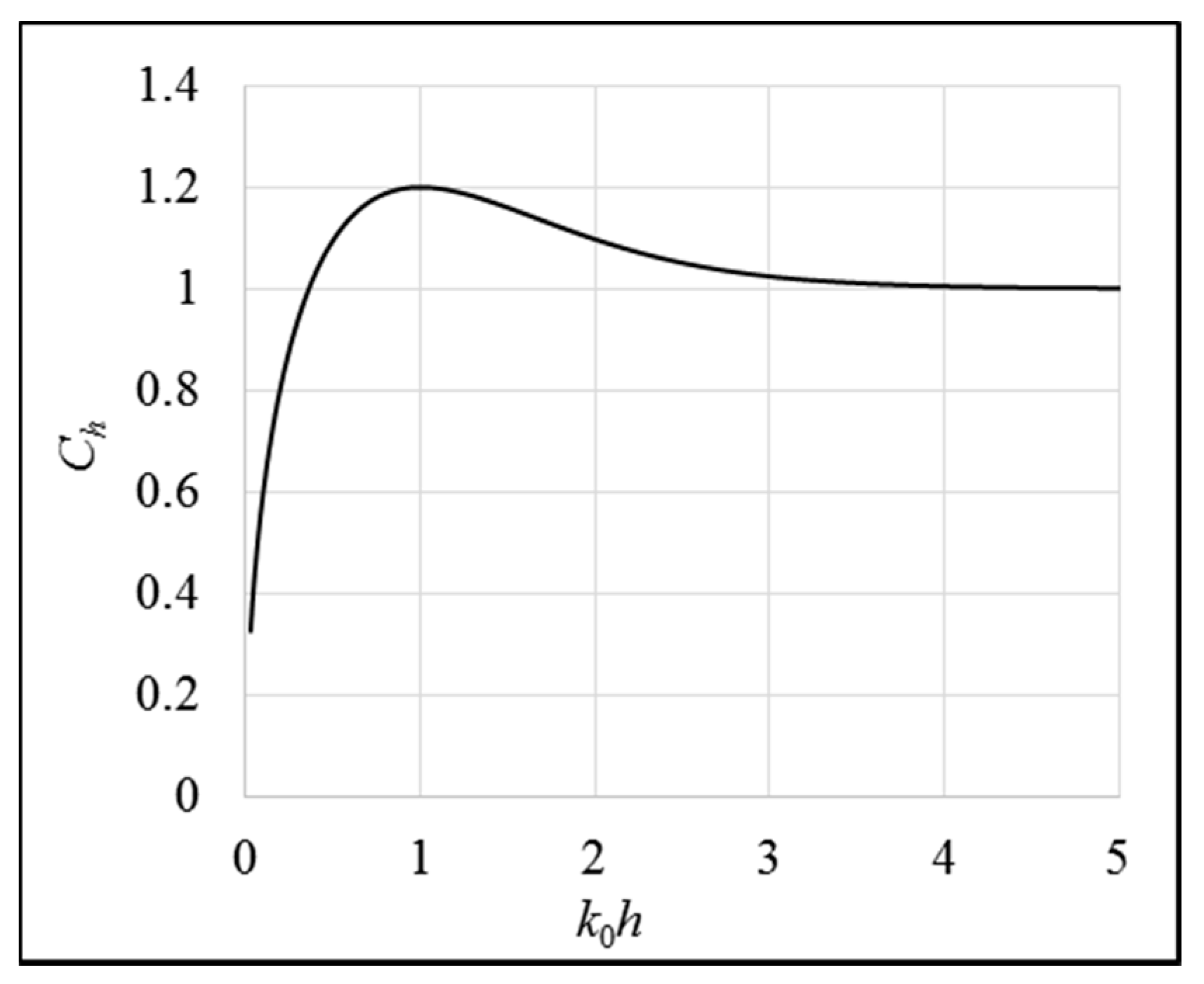
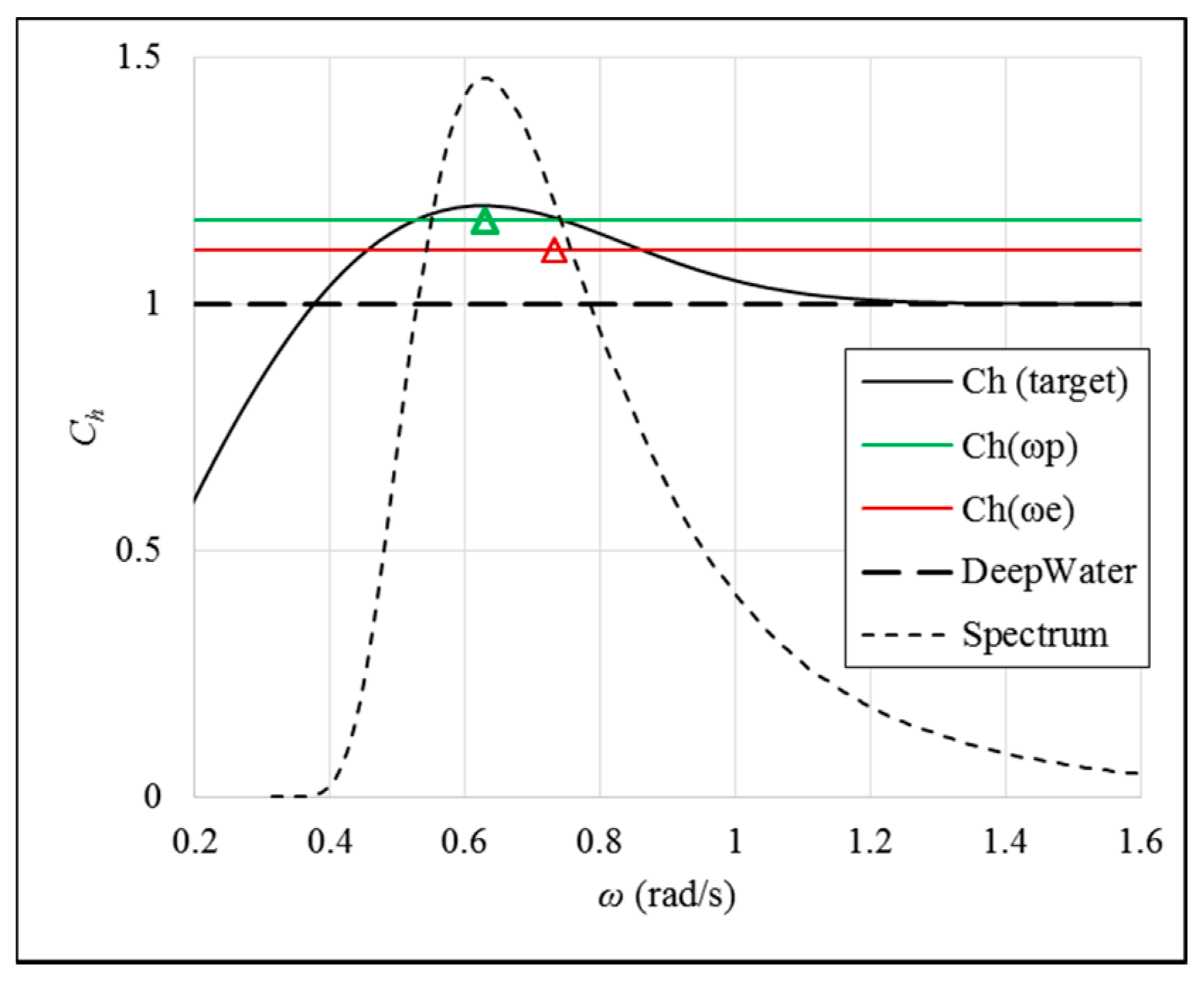
2.3. Zero-Order Modification Method
3. New Methods of Approximations
3.1. Approximations to the Modification Coefficient Ch
3.2. Wave Energy Approximations Methods
3.2.1. 3rd-Order Method
3.2.2. 4th-Order Method
3.2.3. 5th-Order Method
4. Wave Energy Assessment in Finite Water Depths
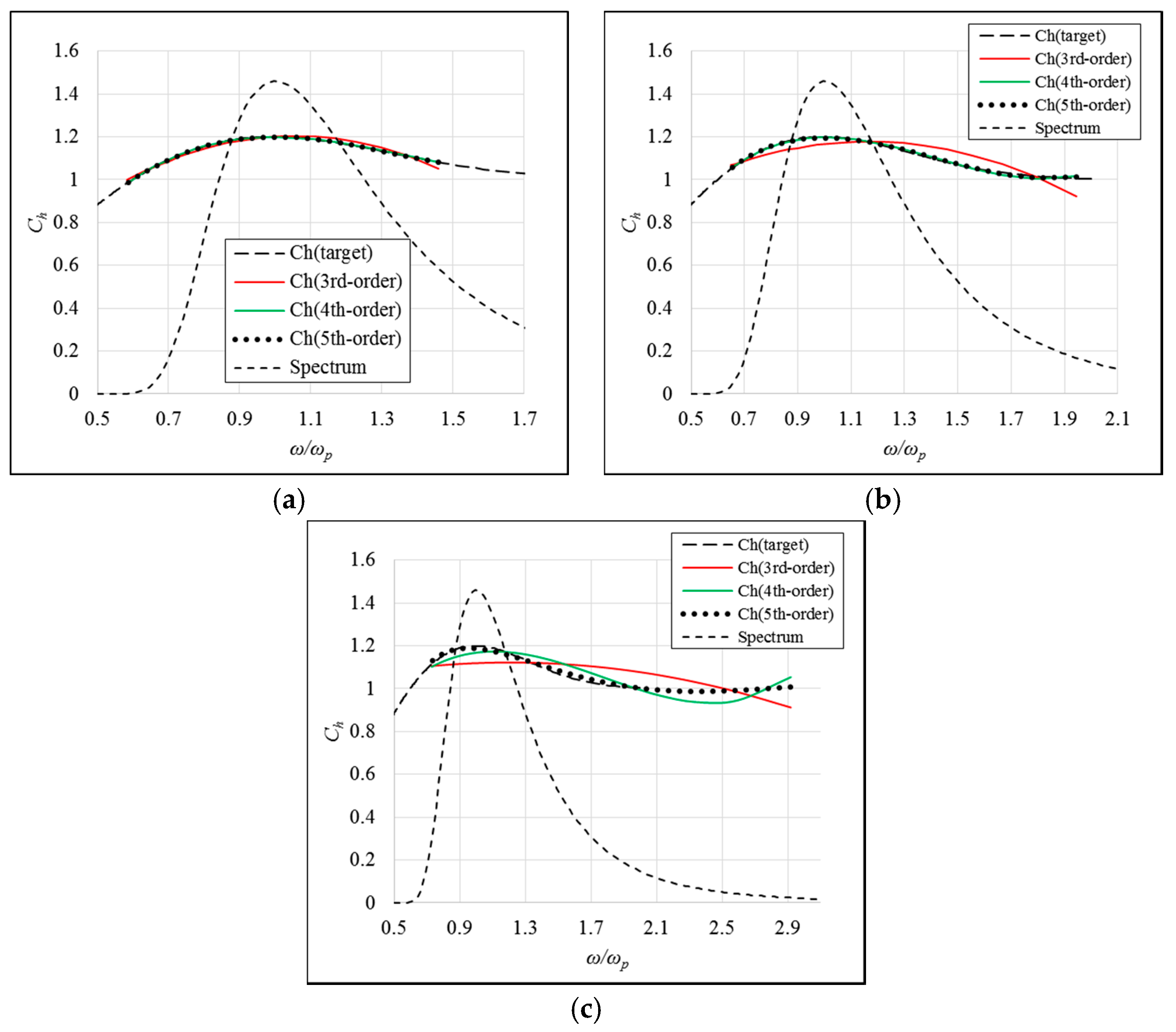
4.1. Bretschneider Spectra (Hm0 = 2 m for All Cases)
4.2. Standard JONSWAP Spectra (Hm0 = 2 m for All Cases)
- (1)
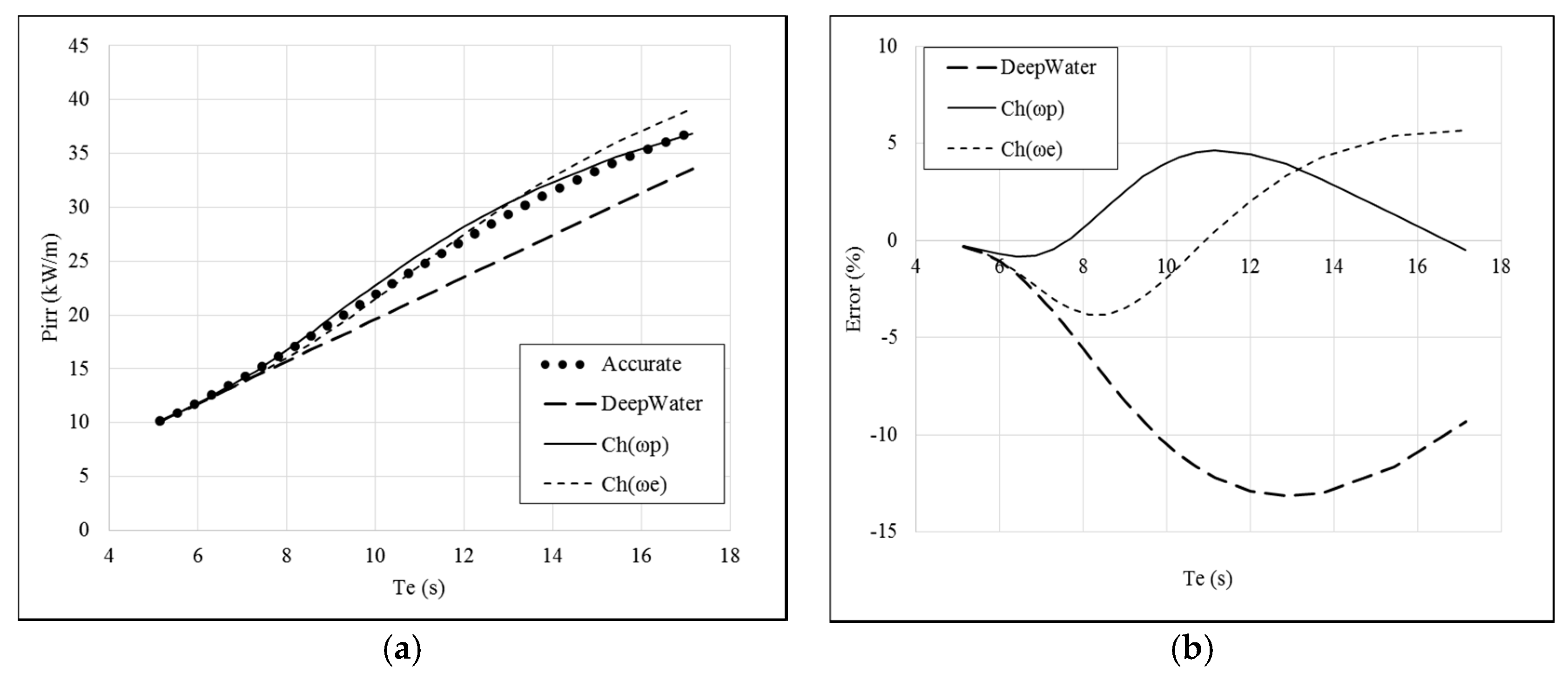
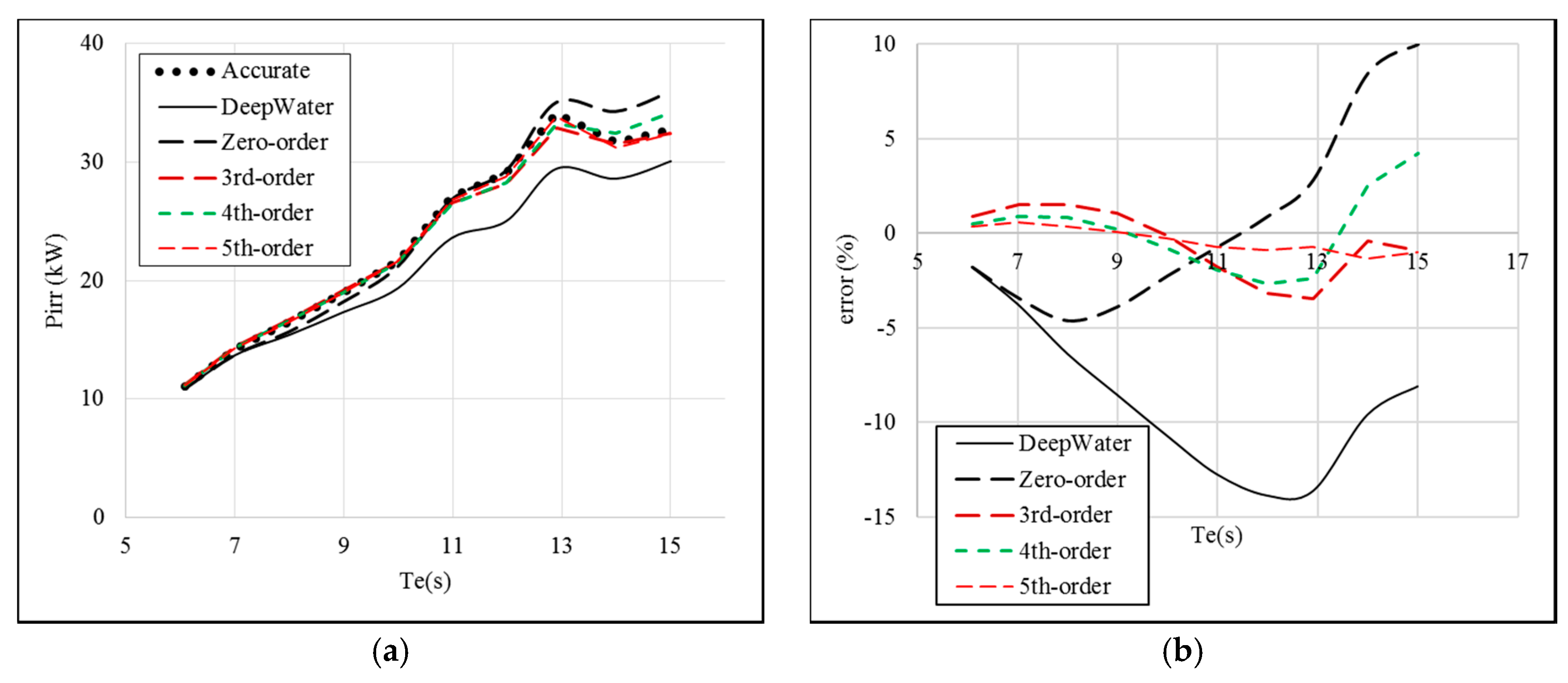
- The deep water condition gives underestimations of the wave power with a maximal error being about 14.5%;
- (2)
- The correction using the zero-order method is better than the 3rd-order method, with a slightly smaller maximal error (3.5% vs. 6%).
- (3)
- The 4th-order and 5th-order methods are very good approximations, with maximal errors being 2.5% and 1.5% respectively for the 25 m and 50 m water depths.
4.3. Measured Waves at AMETS
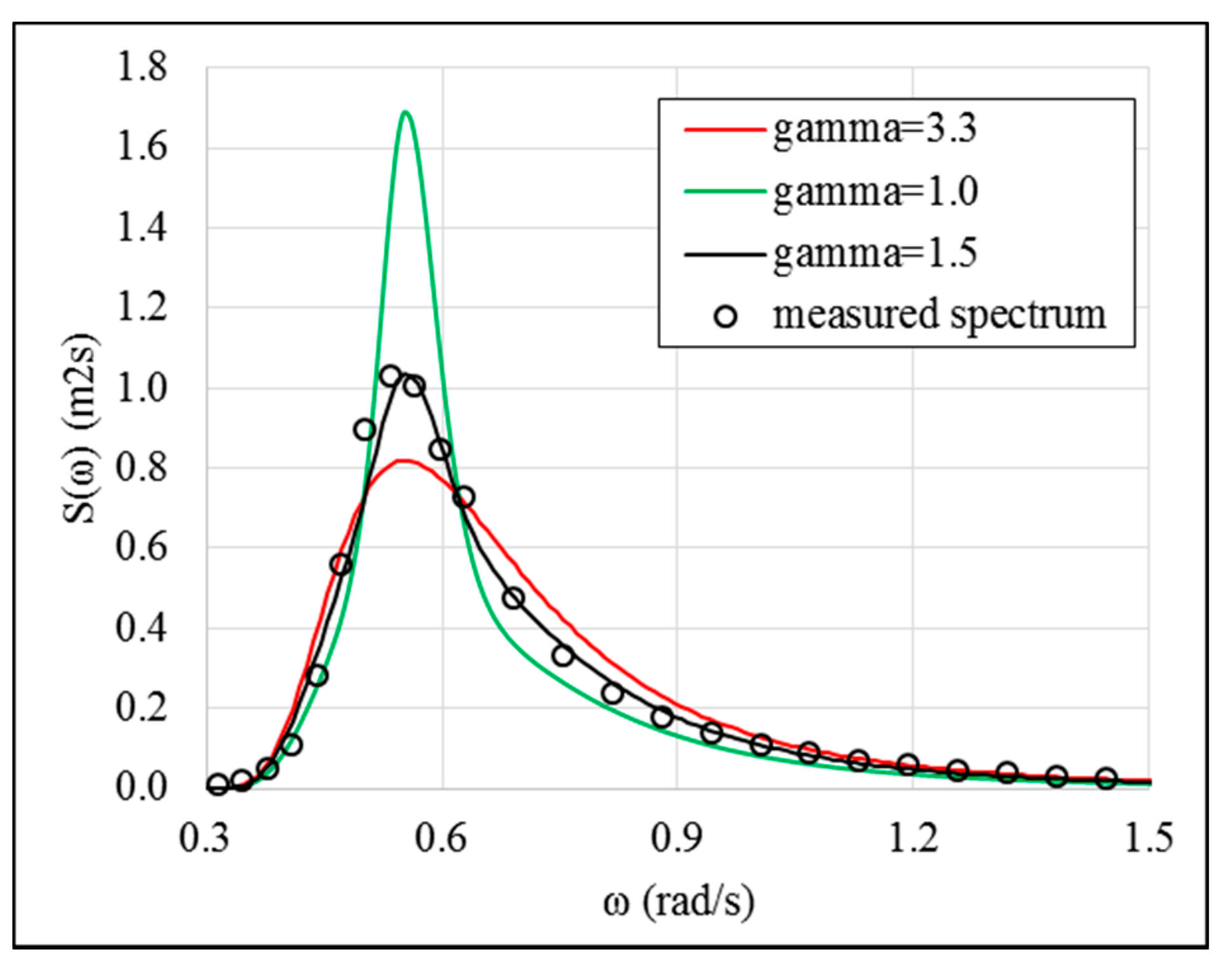
4.3.1. Measured Spectra
4.3.2. Wave Energy Assessments
5. Results and Analysis
5.1. Bin Size and Wave Energy Assessment
5.2. Scatter Diagram and Wave Energy Assessment
6. Conclusions
- (1)
- The examples using the theoretical spectra (Bretschneider and JONSWAP) have shown that the proposed method can significantly improve the assessment accuracy of wave energy calculation at finite water depths, reducing the maximal error from about 14% to less than 1.0% for the individual wave state for the 5th-order method, with the 3rd-order and 4th-order methods presenting slightly better and more reliable results than the zero-order method.
- (2)
- The proposed 5th-order method can considerably improve the assessment of the annual mean wave power for the measured sea waves, with a maximal error less than 1.0% for the AMETS data. In comparison, the deep water formulas give an error between 9.5% and 11% under-estimation, and the zero-order method gives a maximal error about 4.5%. The 3rd-order and 4th-order methods give more reliable results than the zero-order method.
- (3)
- Bin size in the scatter diagram may be important for assessing the wave resources. From the example in the study, the maximum bin size allowed by the IEC TS 62600-101 seems appropriate for guaranteeing very accurate assessment of wave energy and resources.
- (4)
- Based on the proposed method, the 5th-order method gives very consistently accurate wave energy calculation. For employing the 5th-order approximation method, the relevant parameters must be provided. Hence to make the wave data more useful, it is suggested that in addition to the scatter diagram (from which the significant wave height, Hm0 and energy period, Te can be decided), the calculated spectral peak period, Tp, spectral mean period T01 and zero upcrossing period T02 are ideally provided.
- (5)
- Other statistical parameters (if available) can also be used for further improving the assessment of the wave energy in finite water depths. For instance, in shipbuilding industry and research, the wave peak to peak period, T24 (=) is often suggested ([27]). Such additional parameter (parameters) could allow higher-order approximation (more than 5th-order) and thus better and more accurate assessments can be expected.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Cornett, A. A global wave energy resource assessment. In Proceedings of the International Offshore and Polar Engineering Conference, Vancouver, BC, Canada, 6–11 July 2008. [Google Scholar]
- Mork, G.; Barstow, S.F.; Kabuth, A.; Teresa Pontes, M. Assessing the global wave energy potential. In Proceedings of the OMAE2010 29th International Conference on Ocean, Offshore Mechanics and Arctic Engineering, Shanghai, China, 6–11 June 2010. [Google Scholar]
- Gunn, K.; Stock-Williams, C. Quantifying the global wave power resource. Renew. Energy 2012, 44, 296–304. [Google Scholar] [CrossRef]
- Arinaga, R.A.; Cheung, K.F. Atlas of global wave energy from 10 years of reanalysis and hindcast data. Renew. Energy 2012, 39, 49–64. [Google Scholar] [CrossRef]
- Iglesias, G.; Lopez, M.; Carballo, R.; Castro, A.; Fraguela, J.; Frigaard, P. Wave energy potential in galicia (nw spain). Renew. Energy 2009, 34, 2323–2333. [Google Scholar] [CrossRef]
- Iglesias, G.; Carballo, R. Wave energy and nearshore hot spots: The case of the se bay of biscay. Renew. Energy 2010, 35, 490–500. [Google Scholar] [CrossRef]
- Rusu, L.; Guedes Soares, C. Wave energy assessments in the azores islands. Renew. Energy 2012, 45, 183–196. [Google Scholar] [CrossRef]
- Rusu, L. Assessment of the wave energy in the black sea based on a 15-year hindcast with data assimilation. Energies 2015, 8, 10370–10388. [Google Scholar] [CrossRef]
- Reikard, G.; Robertson, B.; Buckham, B.; Bidlot, J.-R.; Hiles, C. Simulating and forecasting ocean wave energy in western canada. Ocean Eng. 2015, 103, 223–236. [Google Scholar] [CrossRef]
- Lenee-Bluhm, P.; Paasch, R.; Ozkan-Haller, H.T. Characterizing the wave energy resource of the us pacific northwest. Renew. Energy 2011, 36, 2106–2119. [Google Scholar] [CrossRef]
- Hughes, M.G.; Heap, A.D. National-scale wave energy resource assessment for australia. Renew. Energy 2010, 35, 1783–1791. [Google Scholar] [CrossRef]
- Dufour, G.; Michard, B.; Cosquer, E.; Feragu, E. Emacop project: Assessment of wave energy resource along france’s coastlines. In Proceedings of the Coasts, Marine Structures and Breakwaters 2013, Edinburgh, UK, 18–20 September 2013. [Google Scholar]
- Cahill, B.G.; Lewis, A. Wave energy resource characteristion of the altantic marine energy test site. Int. J. Mar. Energy 2013, 1, 3–15. [Google Scholar] [CrossRef]
- Atan, R.; Goggins, J.; Nash, S. A detailed assessment of the wave energy resource at the atlantic marine energy test site. Energies 2016, 9, 967. [Google Scholar] [CrossRef]
- Magagna, D.; Uihleih, A. Ocean energy development in europe: Current status and future perspectives. Int. J. Mar. Energy 2015, 11, 84–104. [Google Scholar] [CrossRef]
- Sheng, W.; Li, H. A Method for Energy and Resource Assessment of Waves in Finite Water Depths. Energies 2017, 10, 460. [Google Scholar] [CrossRef]
- Forrest, S. A methodology for nearshore wave resource assessment. In Proceedings of the International Conference on Ocean Energy, Bilbao, Spain, 6–8 October 2010. [Google Scholar]
- Folley, M.; Whittaker, T. Analysis of the nearshore wave energy resource. Renew. Energy 2009, 34, 1709–1715. [Google Scholar] [CrossRef]
- Folley, M. The wave energy resource. In Handbook of Ocean Wave Energy; Pecher, A., Kofoed, J.P., Eds.; SpringerOpen: London, UK, 2017; Volume 7. [Google Scholar]
- Booij, N.; Ris, R.C.; Holthuijsen, L.H. Third-generation wave model for coastal regions 1. Model description and validation. J. Geophys. Res. 1999, 104, 7649–7666. [Google Scholar] [CrossRef]
- Ris, R.C.; Holthuijsen, L.H.; Booij, N. A third-generation wave model for coastal regions, 2. Verification. J. Geophys. Res. 1999, 104, 7667–7681. [Google Scholar] [CrossRef]
- Reguero, B.G.; Vidal, C.; Menendez, M.; Mendez, F.J.; Minguez, R.; Losada, I. Evaluation of global wave energy resource. In Proceedings of the IEEE Oceans 2011, Santander, Spain, 6–9 June 2011. [Google Scholar]
- Holthuijsen, L.H. Waves in Oceanic and Coastal Waters; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Newman, J.N. Marine Hydrodynamics; The MIT Press: Cambridge, MA, USA, 1977. [Google Scholar]
- Tucker, M.J.; Pitt, E.G. Waves in Ocean Engineering; Elsevier Science Ltd.: Oxford, UK, 2001. [Google Scholar]
- International Electrotechnical Commission. Marine Energy-Wave, Tidal and Other Water Current Converters- Part 101: Wave Energy Resource Assessment and Characterization; IEC TS 62600–101; International Electrotechnical Commission: London, UK, 2015. [Google Scholar]
- Stansberg, C.T.; Contento, G.; Hong, S.W.; Irani, M.; Ishida, S.; Mercier, R.M.; Wang, Y.; Wolfram, J. The specialist committee on waves: Final report and recommendations to the 23rd ITTC (International Towing Tank Conference). In Proceedings of the 23rd International Towing Tank Conference, Venice, Italy, 8–14 September 2002. [Google Scholar]
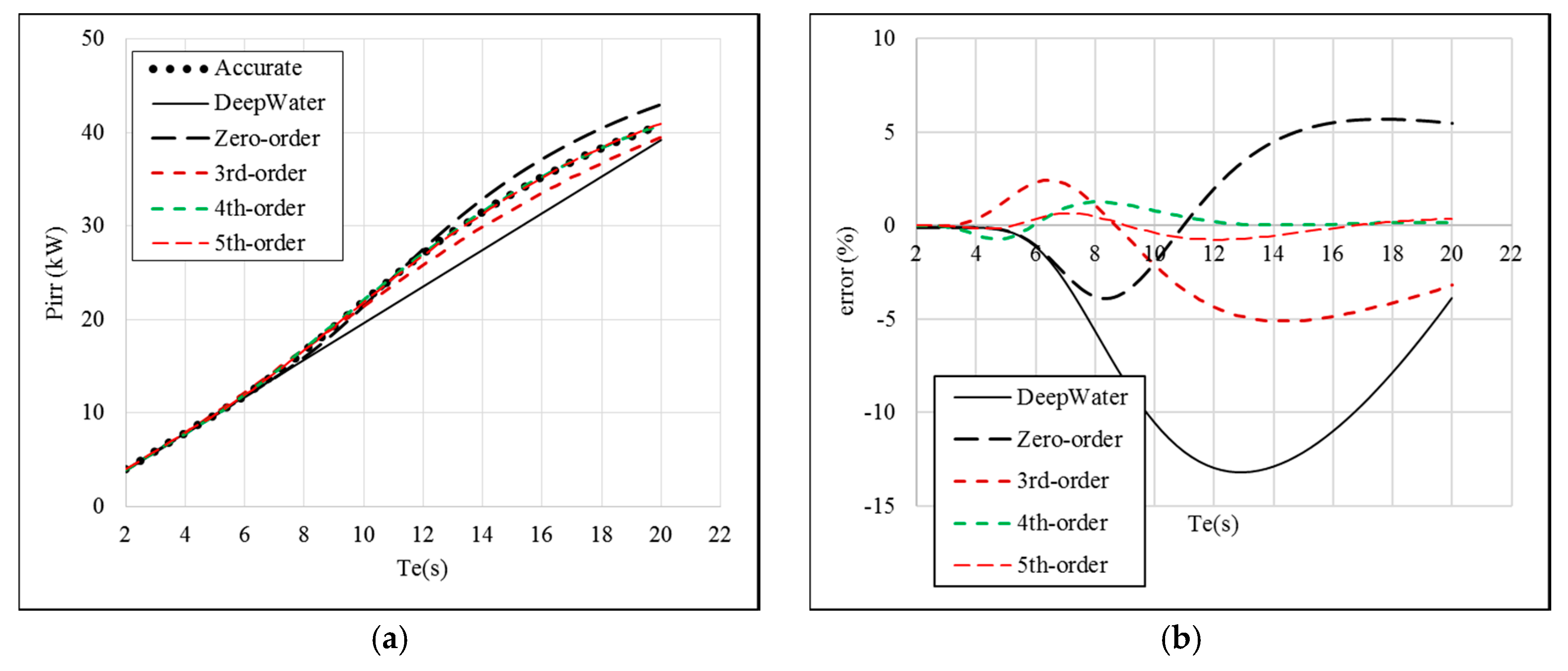
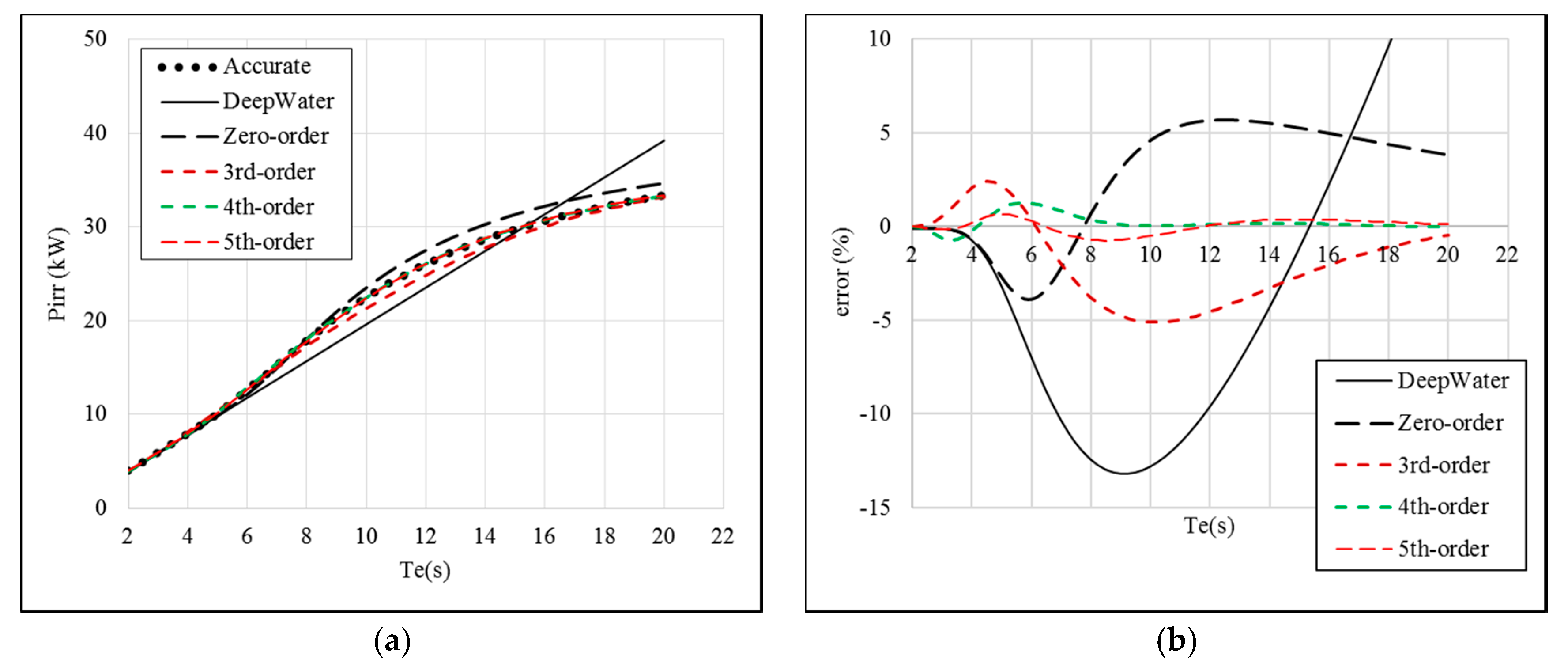

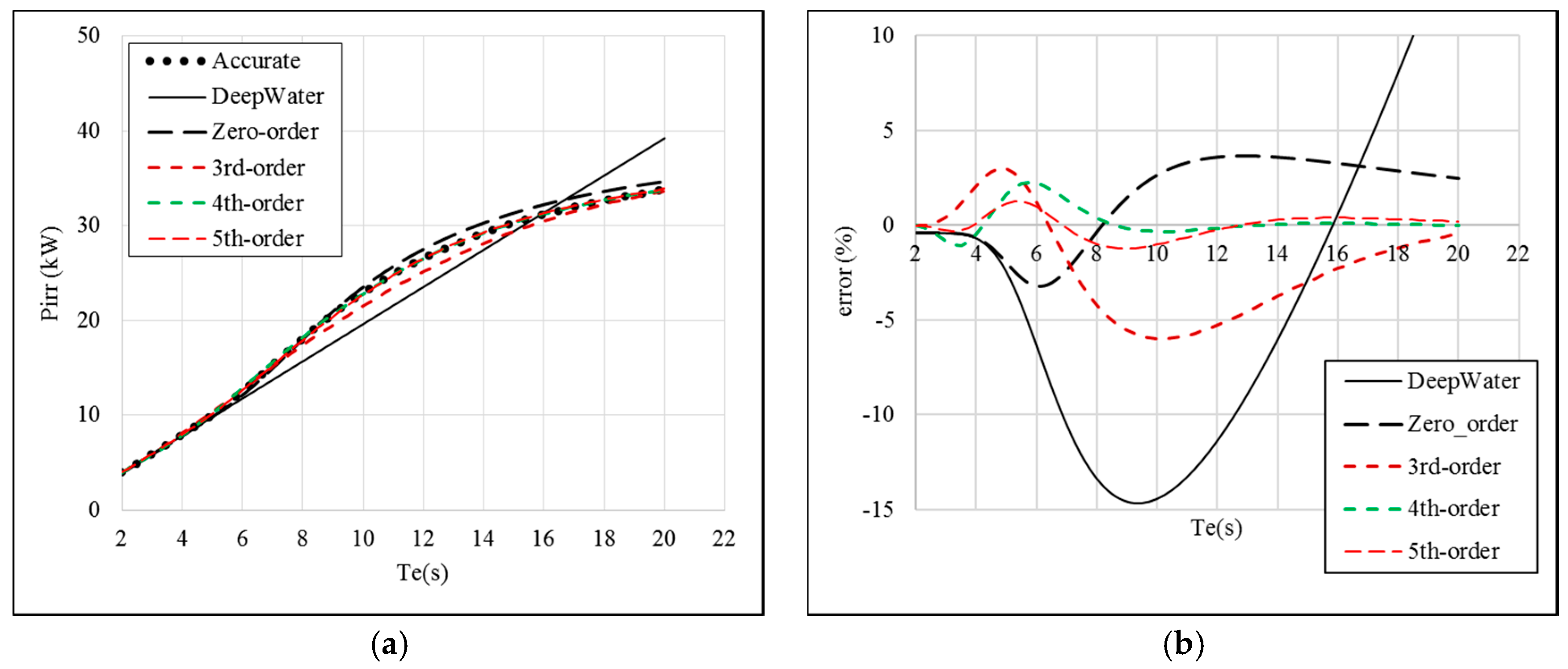


| Water Depth (m) | Method | Individual Spectra (I) | Average Spectra (II) | Error1 (%) between (I) and (II) | ||
|---|---|---|---|---|---|---|
| Pirr (kW/m) | Error0 (%) | Pirr (kW/m) | Error0 (%) | |||
| 50 | Accurate | 37.59 | - | 37.59 | - | 0.00 |
| DeepWater | 33.80 | −10.08 | 33.80 | −10.08 | 0.00 | |
| zero-order with ωe | 37.09 | −1.33 | 37.09 | −1.33 | 0.00 | |
| zero-order with ωp | 38.99 | 3.72 | 39.04 | 3.86 | 0.13 | |
| 3rd-order | 36.68 | −2.42 | 36.68 | −2.42 | 0.00 | |
| 4th-order | 38.48 | 2.37 | 38.48 | 2.37 | 0.00 | |
| 5th-order | 37.34 | −0.67 | 37.33 | −0.69 | −0.03 | |
| 25 | Accurate | 37.97 | - | 37.97 | - | 0.00 |
| DeepWater | 33.80 | −10.98 | 33.80 | −10.98 | 0.00 | |
| zero-order with ωe | 39.53 | 4.11 | 39.54 | 4.13 | 0.03 | |
| zero-order with ωp | 38.45 | 1.26 | 38.51 | 1.42 | 0.16 | |
| 3rd-order | 36.17 | −4.74 | 36.17 | −4.74 | 0.00 | |
| 4th-order | 38.68 | 1.87 | 38.68 | 1.87 | 0.00 | |
| 5th-order | 38.33 | 0.95 | 38.33 | 0.95 | 0.00 | |
| Method | Bin Size: 1.0 m & 1.0 s | Bin Size: 2.0 m & 2.0 s | ||
|---|---|---|---|---|
| Pirr (kW/m) | Error1 (%) | Pirr (kW/m) | Error1 (%) | |
| Accurate | 37.59 | 0.00 | 37.59 | 0.00 |
| DeepWater | 33.80 | 0.00 | 33.57 | −0.68 |
| zero-order with ωe | 37.08 | −0.03 | 36.78 | −0.84 |
| zero-order with ωp | 39.06 | 0.18 | 38.87 | −0.31 |
| 3rd-order | 36.69 | 0.03 | 36.45 | −0.63 |
| 4th-order | 38.48 | 0.00 | 38.2 | −0.73 |
| 5th-order | 37.33 | −0.03 | 37.05 | −0.78 |
| Water Depth (m) | Method | Individual Spectra (I) | Scatter Diagram (II) | Error1 (%) between (I) and (II) | ||
|---|---|---|---|---|---|---|
| Pirr (kW/m) | Error0 (%) | Pirr (kW/m) | Error0 (%) | |||
| 50 | Accurate | 37.59 | - | 37.59 | - | - |
| DeepWater | 33.8 | −10.08 | 34.08 | −9.34 | 0.21 | |
| zero-order with ωe | 37.09 | −1.33 | 37.4 | −0.51 | 0.49 | |
| zero-order with ωp | 38.99 | 3.72 | 38.96 | 3.64 | 0.03 | |
| 3rd-order | 36.68 | −2.42 | 36.96 | −1.68 | 0.16 | |
| 4th-order | 38.48 | 2.37 | 38.87 | 3.41 | 0.16 | |
| 5th-order | 37.34 | −0.67 | 37.41 | −0.48 | −0.03 | |
| 25 | Accurate | 37.97 | - | 37.97 | - | - |
| DeepWater | 33.8 | −10.98 | 34.08 | −10.24 | 0.21 | |
| zero-order with ωe | 39.53 | 4.11 | 39.84 | 4.92 | 0.18 | |
| zero-order with ωp | 38.45 | 1.26 | 38.62 | 1.71 | 0.16 | |
| 3rd-order | 36.17 | −4.74 | 36.5 | −3.87 | 0.19 | |
| 4th-order | 38.68 | 1.87 | 39.34 | 3.61 | 0.36 | |
| 5th-order | 38.33 | 0.95 | 38.57 | 1.58 | −0.03 | |
| Method | Bin Size: 0.5 m and 1.0 s | Bin Size: 1.0 m and 1.0 s | Bin size: 2.0 m and 2.0 s | |||
|---|---|---|---|---|---|---|
| Pirr (kW/m) | Error1 (%) | Pirr (kW/m) | Error1 (%) | Pirr (kW/m) | Error1 (%) | |
| Accurate | 37.59 | 0.00 | 37.59 | 0.00 | 37.59 | 0.00 |
| DeepWater | 33.87 | 0.21 | 34.08 | 0.83 | 34.29 | 1.45 |
| zero-order with ωe | 37.17 | 0.22 | 37.4 | 0.84 | 37.65 | 1.51 |
| zero-order with ωp | 39.03 | 0.10 | 38.96 | −0.08 | 36.87 | −5.44 |
| 3rd-order | 36.72 | 0.11 | 36.96 | 0.76 | 37.2 | 1.42 |
| 4th-order | 38.5 | 0.05 | 38.87 | 1.01 | 39.56 | 2.81 |
| 5th-order | 37.32 | −0.05 | 37.41 | 0.19 | 37.57 | 0.62 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sheng, W.; Li, H.; Murphy, J. An Improved Method for Energy and Resource Assessment of Waves in Finite Water Depths. Energies 2017, 10, 1188. https://doi.org/10.3390/en10081188
Sheng W, Li H, Murphy J. An Improved Method for Energy and Resource Assessment of Waves in Finite Water Depths. Energies. 2017; 10(8):1188. https://doi.org/10.3390/en10081188
Chicago/Turabian StyleSheng, Wanan, Hui Li, and Jimmy Murphy. 2017. "An Improved Method for Energy and Resource Assessment of Waves in Finite Water Depths" Energies 10, no. 8: 1188. https://doi.org/10.3390/en10081188
APA StyleSheng, W., Li, H., & Murphy, J. (2017). An Improved Method for Energy and Resource Assessment of Waves in Finite Water Depths. Energies, 10(8), 1188. https://doi.org/10.3390/en10081188





