Integrated Photovoltaic Inverters Based on Unified Power Quality Conditioner with Voltage Compensation for Submarine Distribution System
Abstract
:1. Introduction
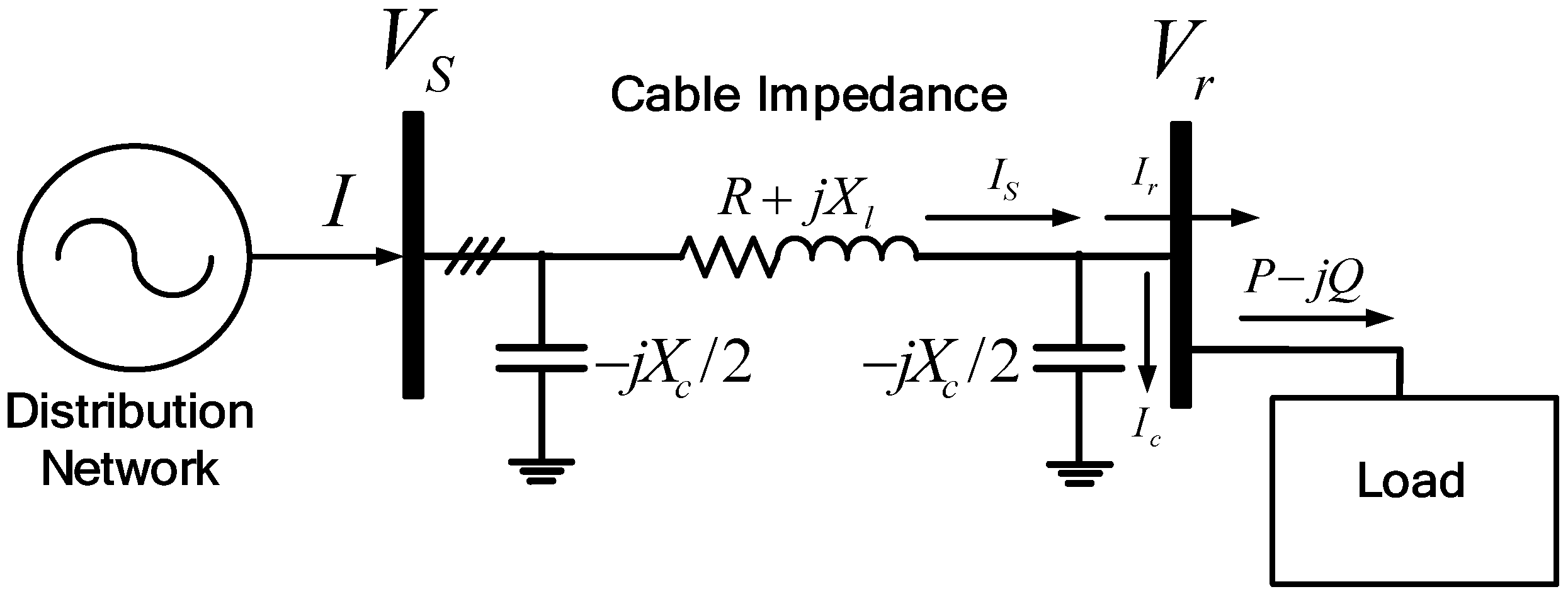
2. Modeling and Analysis of Submarine Cable
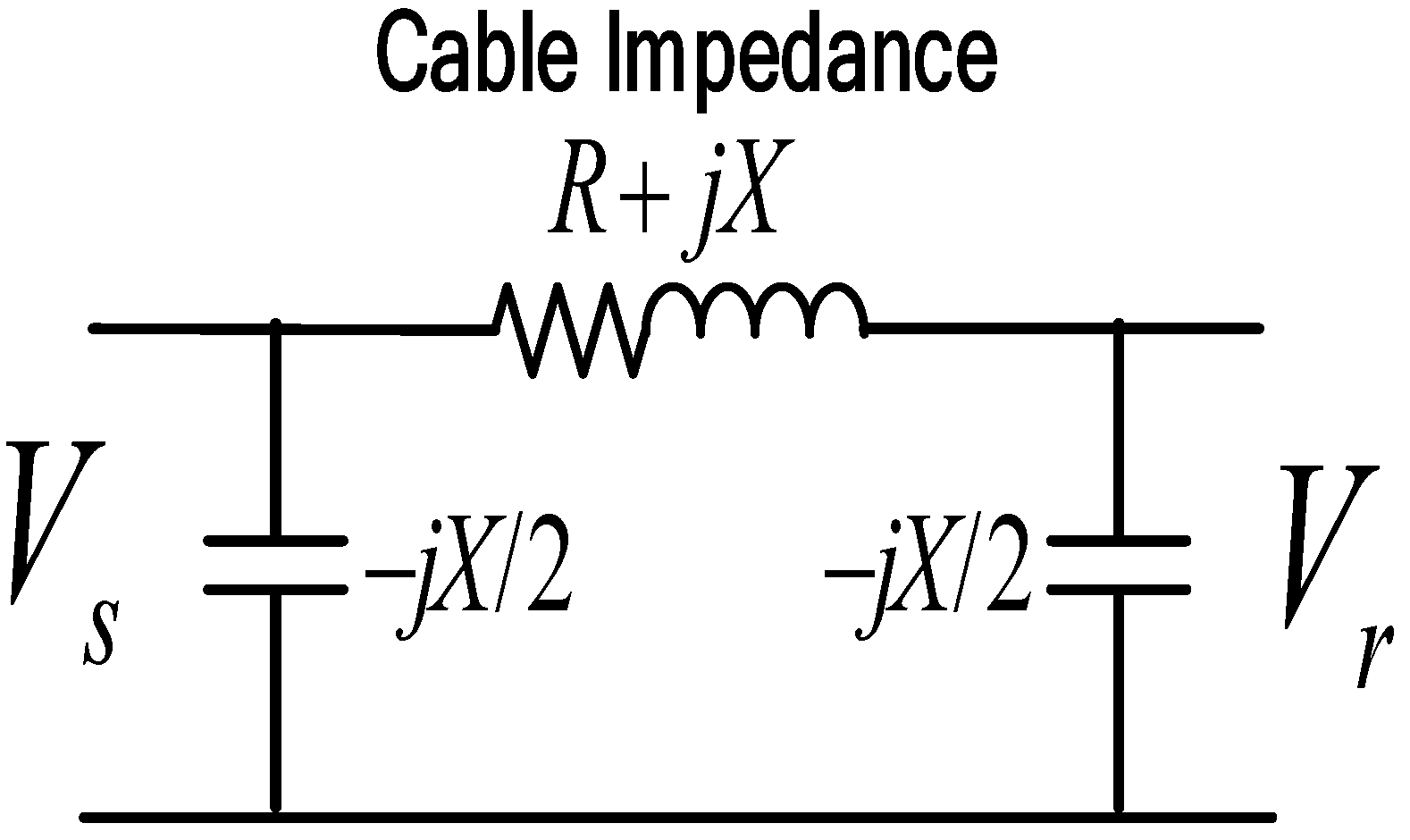
2.1. Modeling of Submarine Cable
2.2. Voltage Profile of Submarine Cable
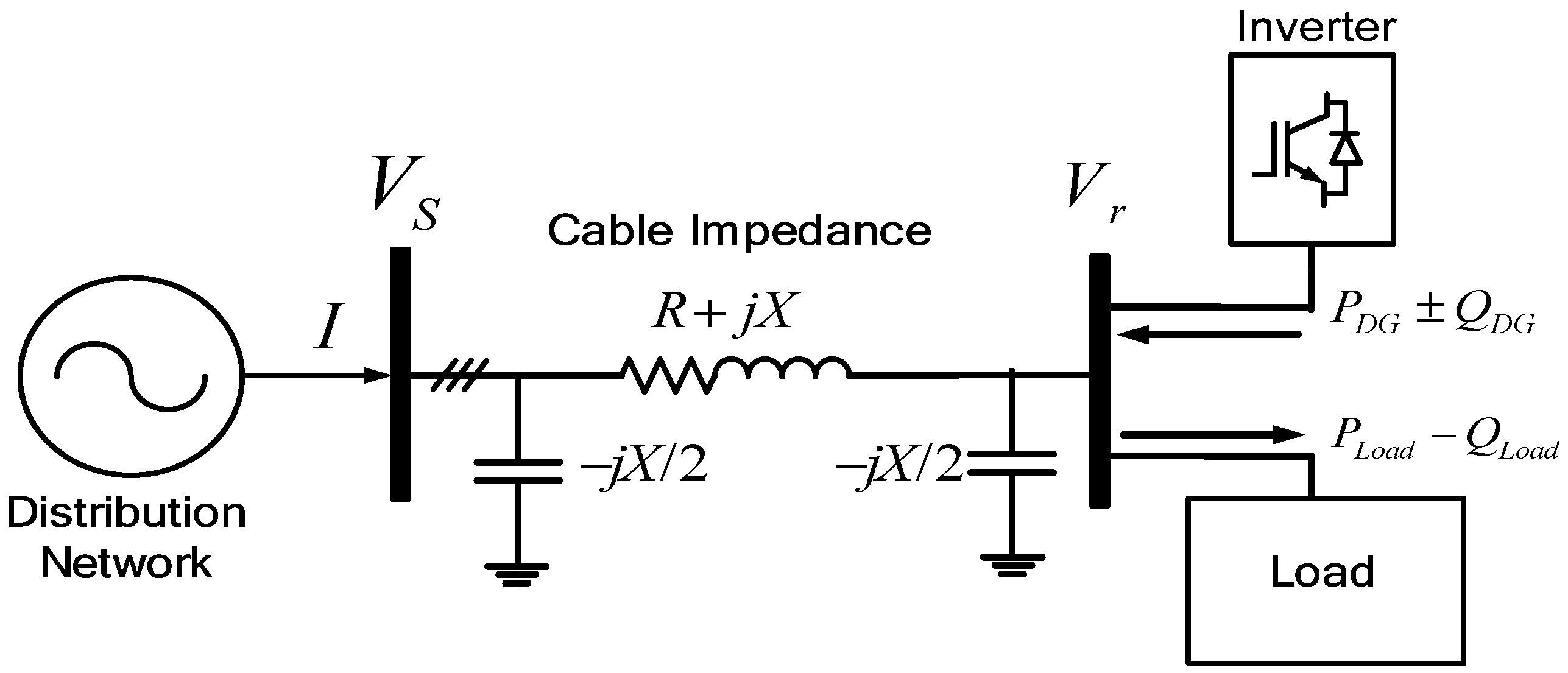
3. Configuration and Operation of Proposed PV Inverter with Voltage Compensation
3.1. Proposed PV Inverter
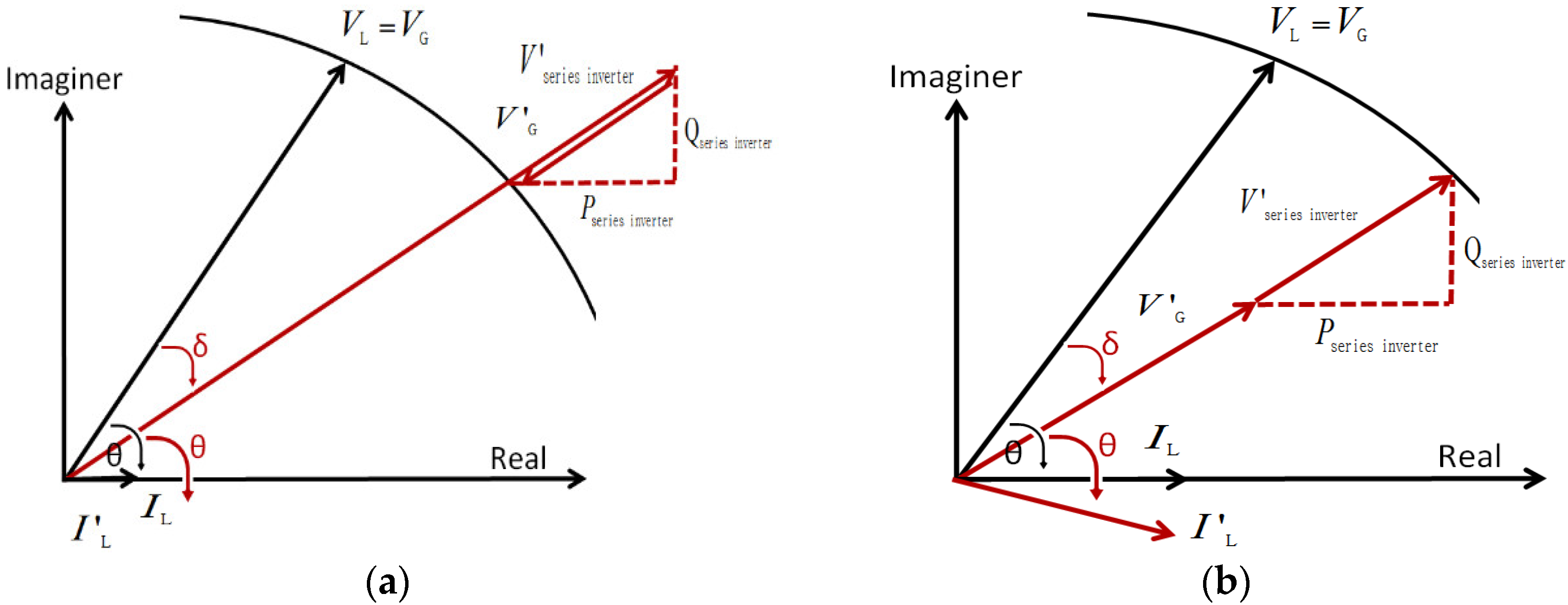
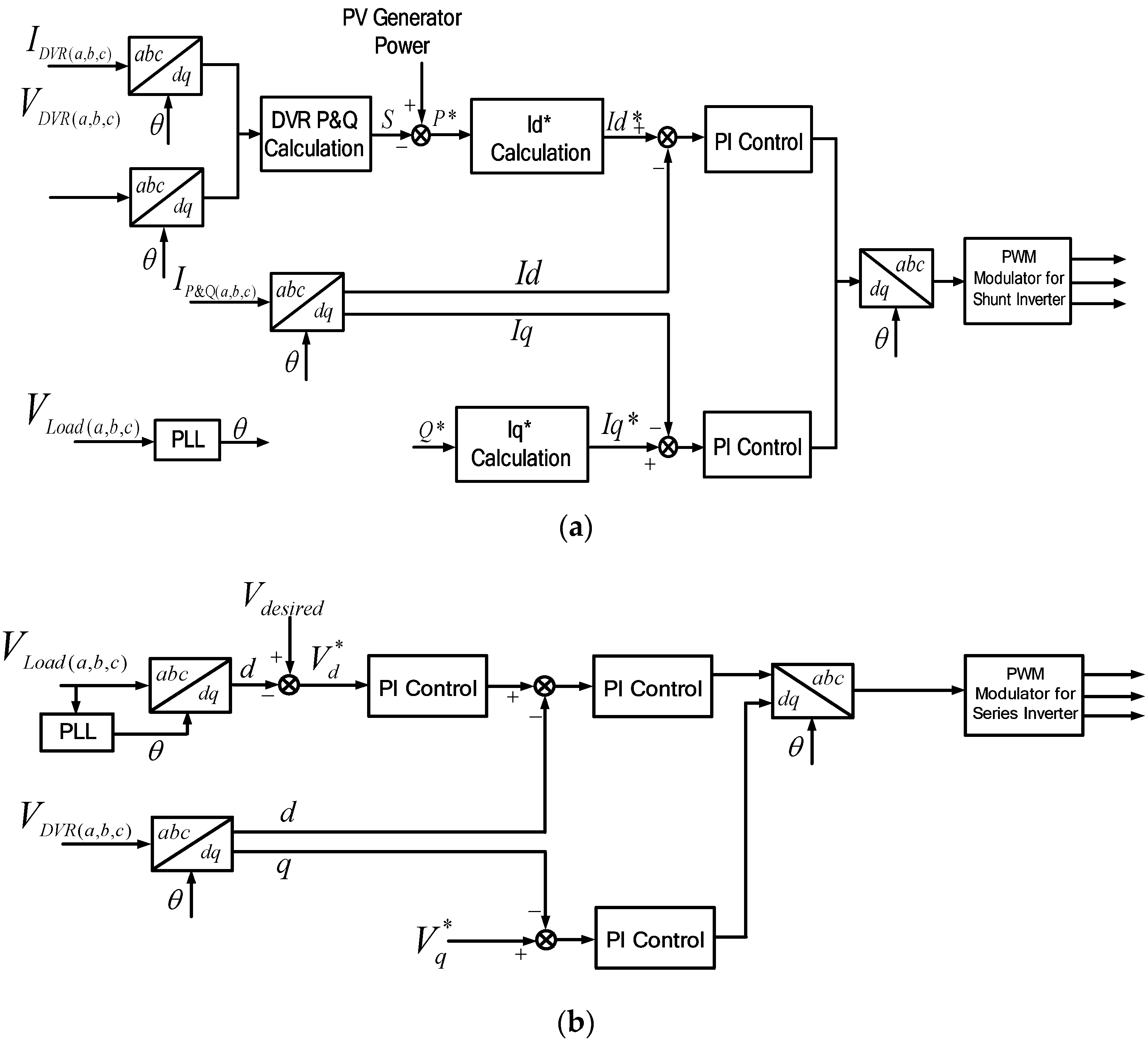
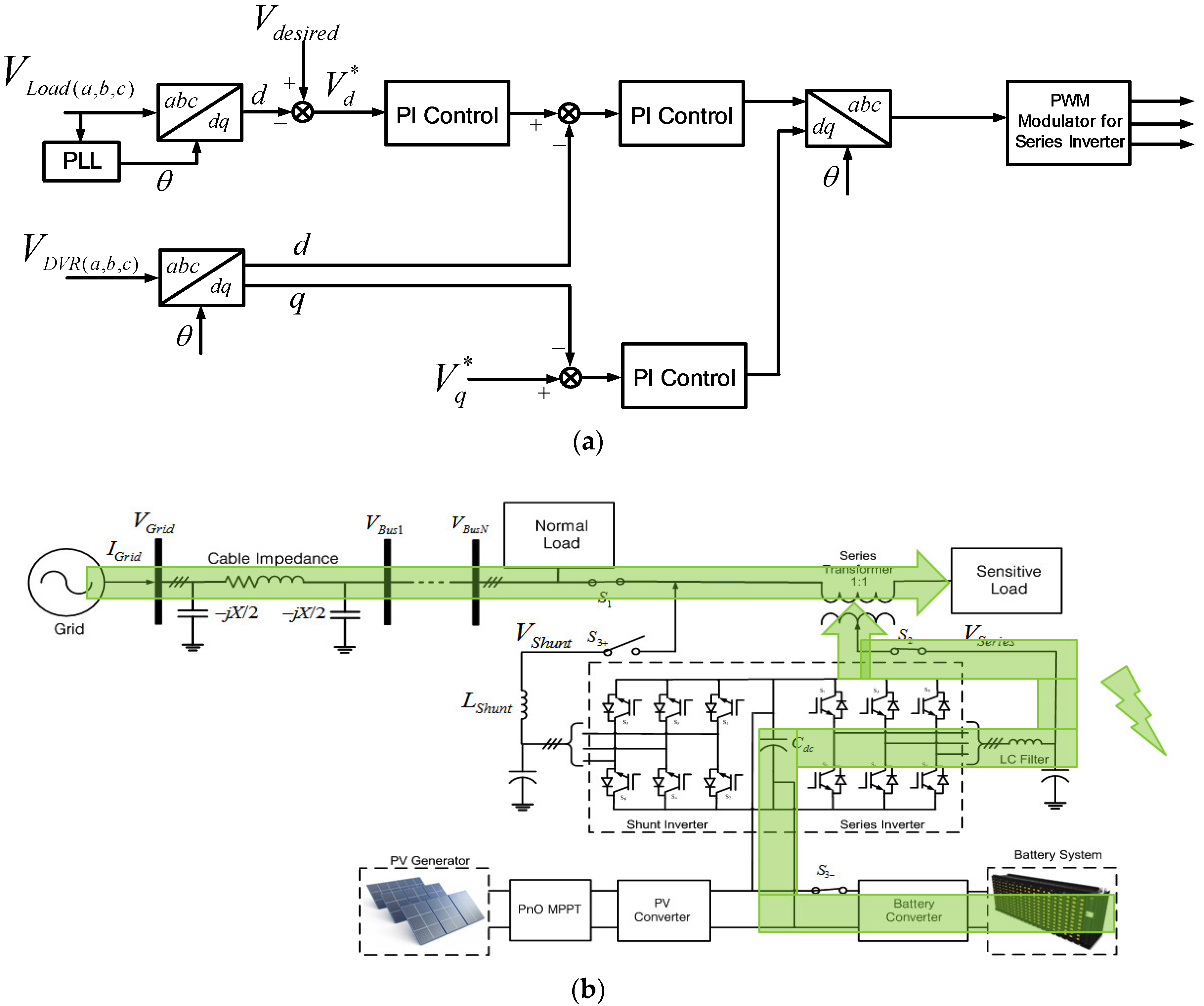
3.2. Operation of Series Inverter
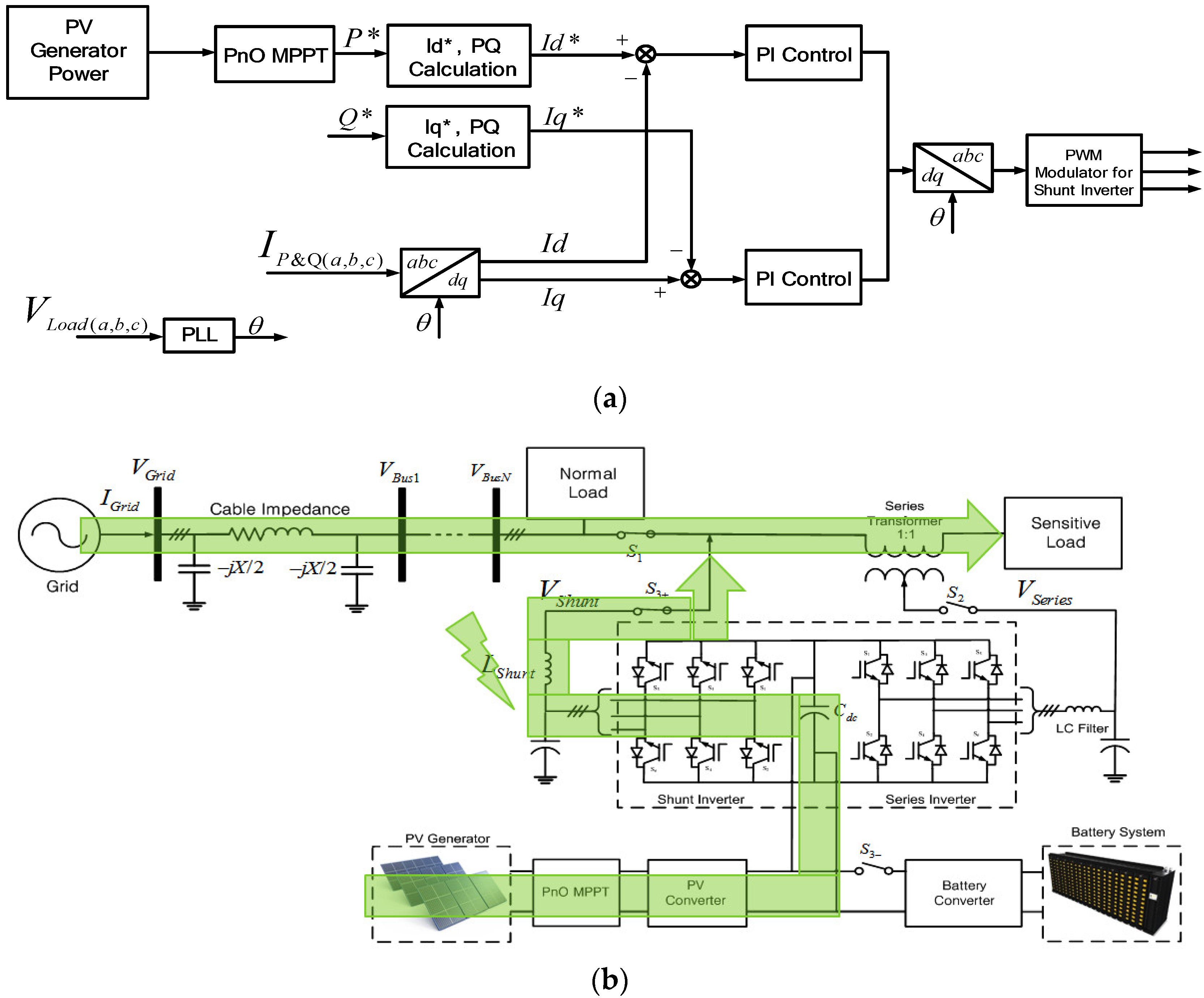
3.3. Operation of Shunt Inverter
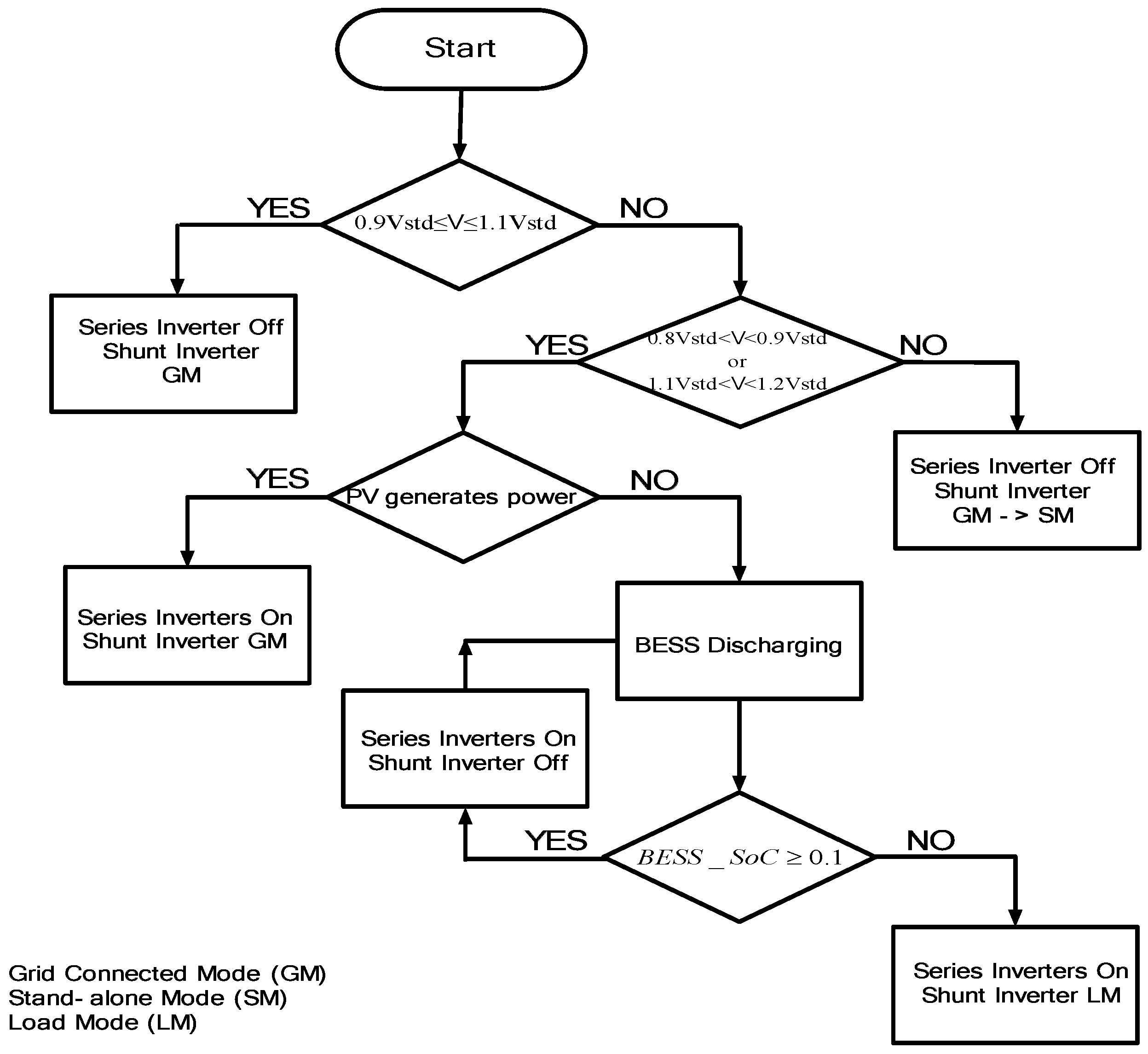
4. System Operation Mode and Control of Proposed PV Inverter
4.1. Normal Mode
4.2. Voltage Compensation Mode with PV Power
4.3. Stand-Alone Mode
4.4. Voltage Compensation Mode from BESS
4.5. Voltage Compensation Mode from Grid
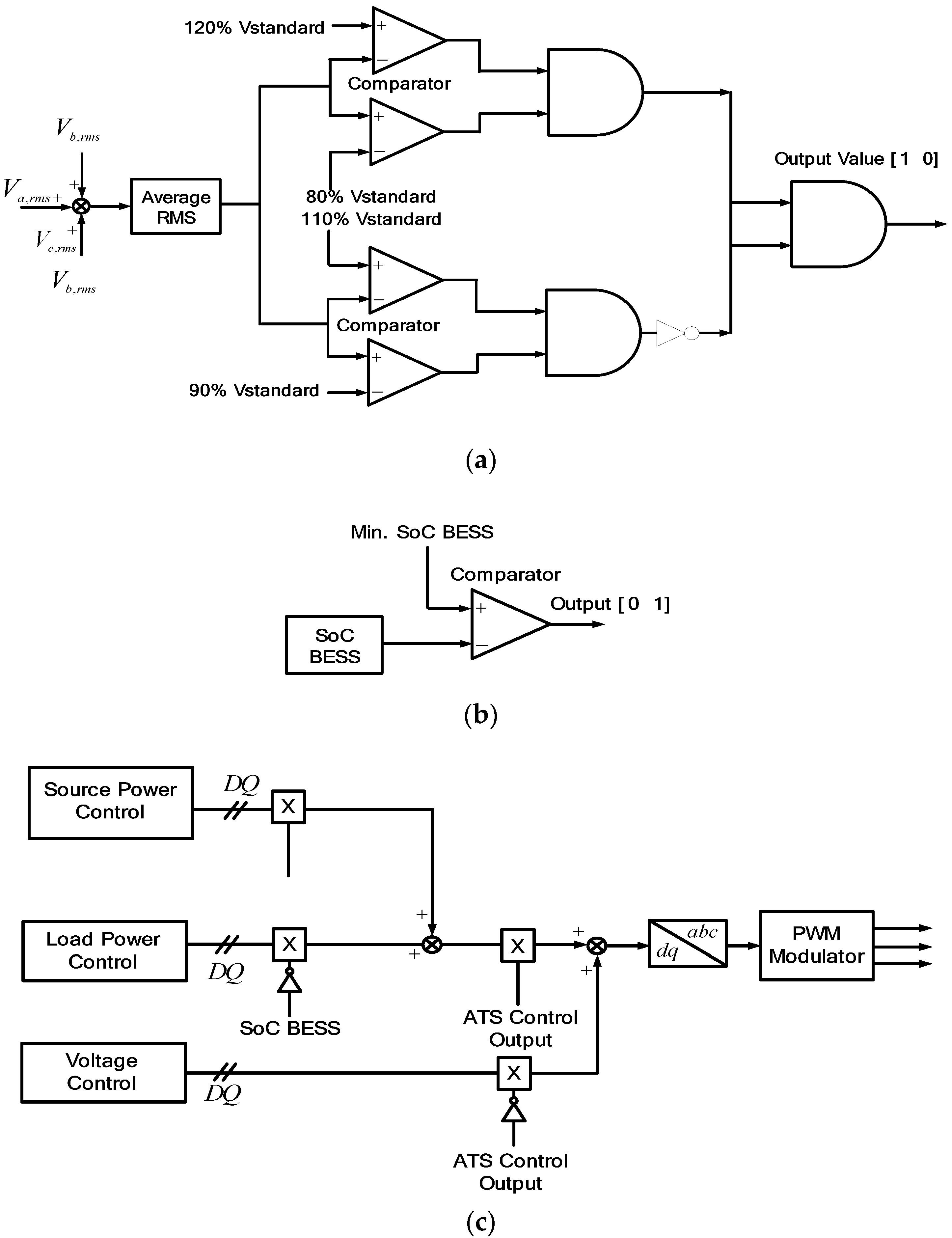
4.6. Automatic Transfer Switches
5. Simulation Results and Discussion
5.1. Simulation Conditions and Parameters
5.2. Undervoltage Case
5.3. Overvoltage Case
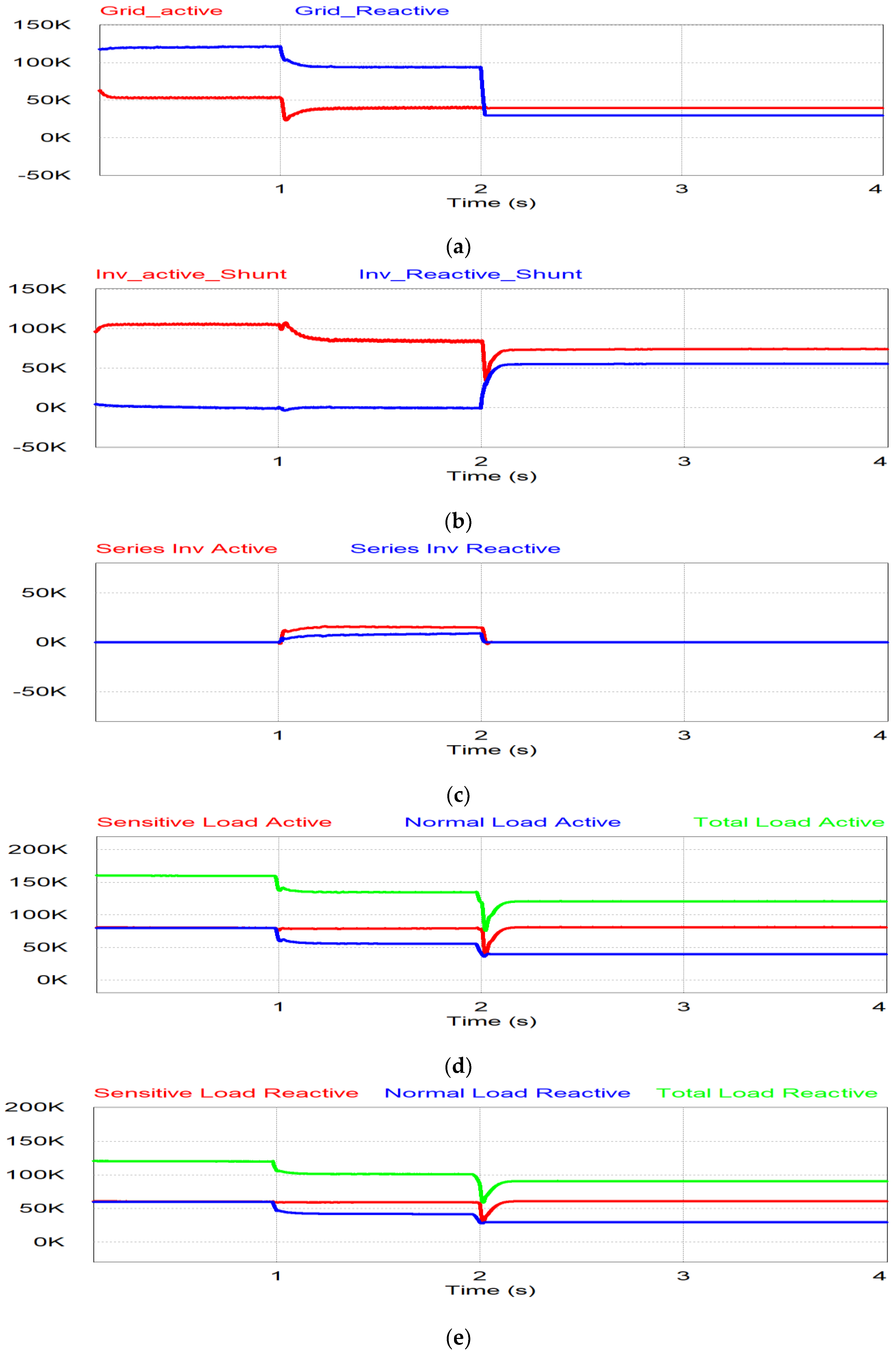
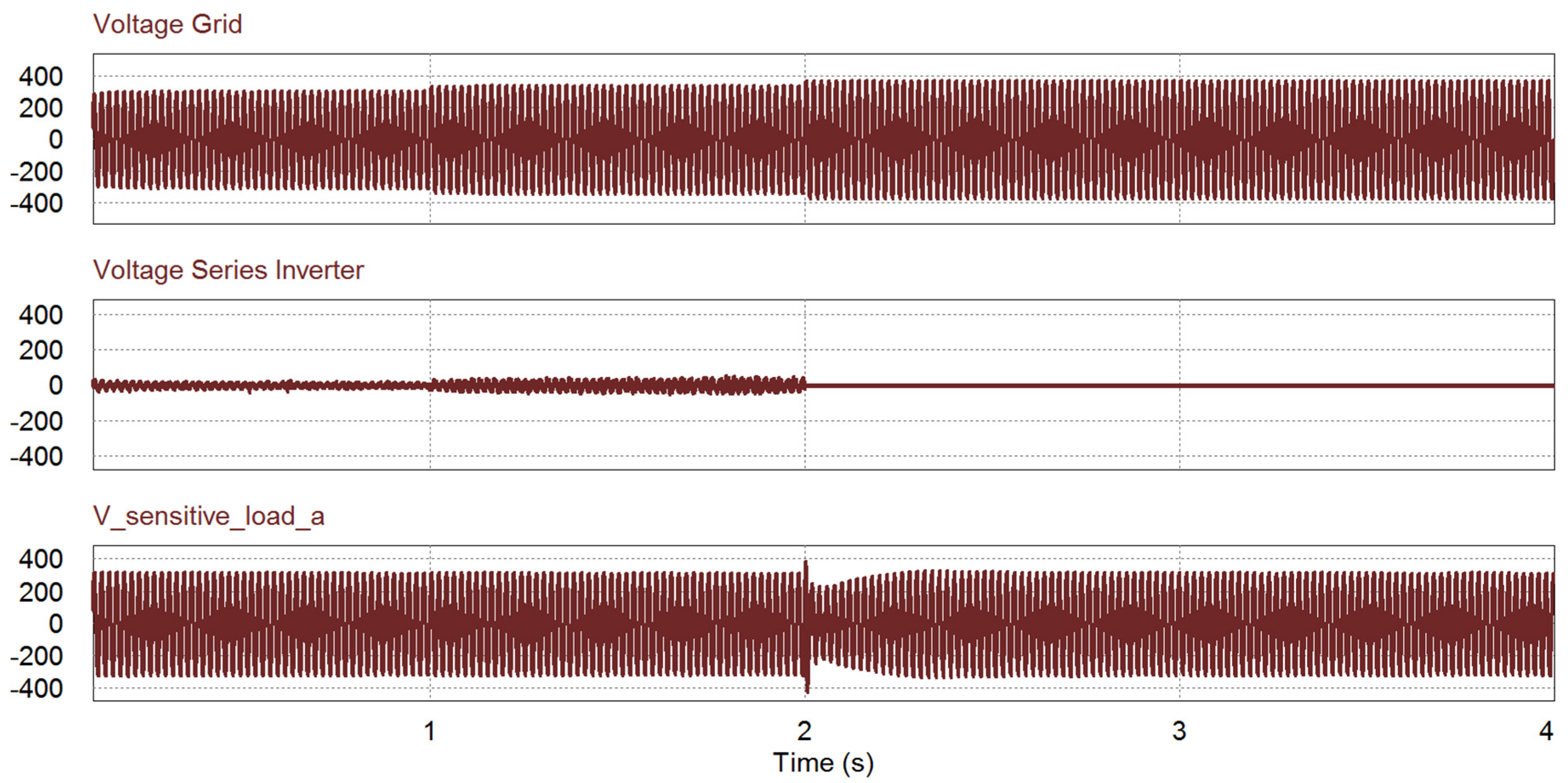
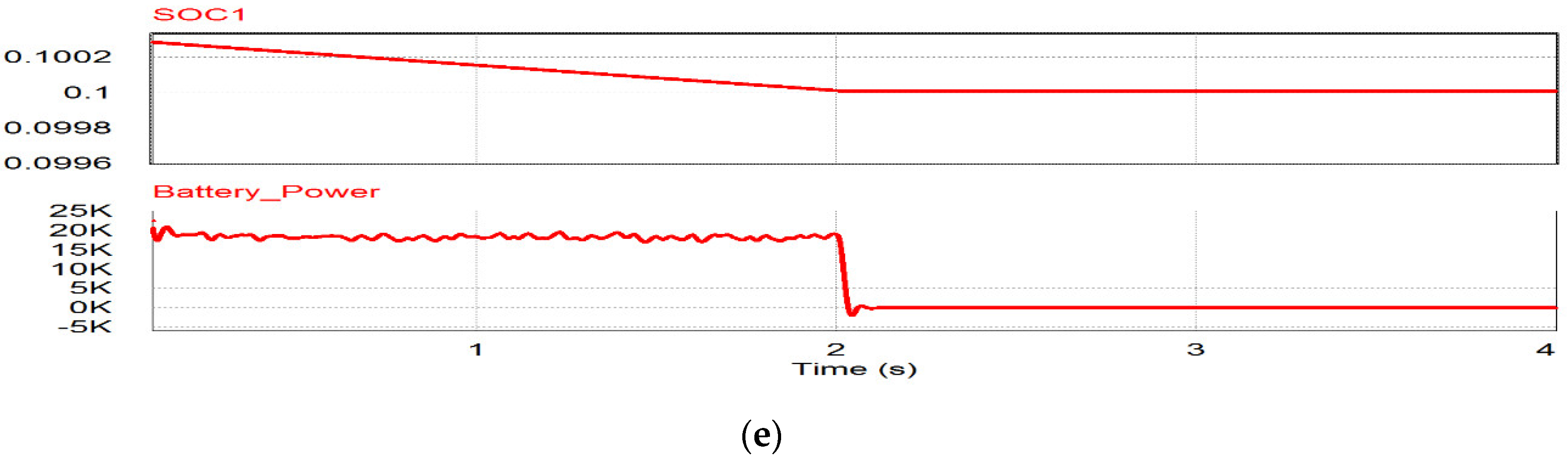
5.4. Voltage Compensation without PV Power Case
5.5. Voltage Compensation with Power from Grid Case
6. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- United Nations. Kyoto Protocol to the United Nations Framework Convention on Climate Change; United Nations: New York, NY, USA, 1998. [Google Scholar]
- IEA International Energy Agency. Snapshot of Global Photovoltaic Markets: Report IEA PVPS T1-33. 2018. Available online: http://www.iea-pvps.org/fileadmin/dam/public/report/statistics/IEA-PVPS_-_A_Snapshot_of_Global_PV_-_1992-2017.pdf (accessed on 8 July 2018).
- Guide for Planning DC Links Terminating at AC Systems Locations Having Low Short-Circuit Capacities, Part I: AC/DC Interaction Phenomena; IEEE Std. 1204-1997; IEEE: Piscataway, NJ, USA, 1997.
- Keyhani, A. Design of Smart Power Grid Renewable Energy Systems, 2nd ed.; Wiley-IEEE Press: Piscataway, NJ, USA, 2015. [Google Scholar]
- IEEE Recommended Practice for Monitoring Electric Power Quality; IEEE Std. 1159-2009; IEEE: Piscataway, NJ, USA, 1999.
- Nagpal, M.; Martinich, T.G.; Bimbhra, A.; Sydor, D. Damaging open-phase overvoltage disturbance on a shunt-compensated 500-kV line initiated by unintended trip. IEEE Trans. Power Deliv. 2015, 30, 412–419. [Google Scholar] [CrossRef]
- Chavan, G.; Acharya, S.; Bhattacharya, S.; Das, D.; Imam, H. Application of static synchronous series compensators in mitigating Ferranti effect. In Proceedings of the 2016 IEEE Power and Energy Society General Meeting (PESGM), Boston, MA, USA, 1–5 July 2016. [Google Scholar]
- Natesan, C.; Ajithan, K.; Palani, P.; Kandhasamy, P. Survey on microgrid: Power quality improvement techniques. Renew. Energy 2014, 342019. [Google Scholar] [CrossRef]
- AppalaNaidu, T. The Role of Dynamic Voltage Restorer (DVR) in improving power quality. In Proceedings of the 2016 International Conference on Advances in Electrical, Electronics, Information, Communication and Bio-Informatics (AEEICB), Chennai, India, 27–28 February 2016. [Google Scholar]
- Fernandes, D.; Costa, F.; Martins, J.; Lock, A.; Silva, E.; Vitorino, M. Sensitive load voltage compensation Performed by a Suitable Control Method. IEEE Trans. Ind. Appl. 2017, 53, 4877–4885. [Google Scholar] [CrossRef]
- Sin, C.; Choi, J. Compensation of current harmonics caused by local nonlinear load for grid-connected converter. In Proceedings of the IFEEC 2017-ECCE Asia, Kaohshiung, Taiwan, 3–7 June 2017. [Google Scholar]
- Jayaprakash, P.; Singh, B.; Kothari, P.; Chandra, A.; Al-Haddad, K. Control of reduced-rating dynamic voltage restorer with a battery energy storage system. IEEE Trans. Ind. Appl. 2014, 50, 1295–1303. [Google Scholar] [CrossRef]
- Gee, A.; Robinson, F.; Yuan, W. A Superconducting magnetic energy storage-emulator/battery supported dynamic voltage restorer. IEEE Trans. Energy Convers. 2017, 32, 55–64. [Google Scholar] [CrossRef]
- Choi, S.; Li, J.; Vilathgamuwa, D. A generalized voltage compensation strategy for mitigating the impacts of voltage sags/swells. IEEE Trans. Power Deliv. 2005, 20, 2289–2297. [Google Scholar] [CrossRef]
- Khadkikar, V. Enhancing electric power quality using UPQC: A comprehensive overview. IEEE Trans. Power Electron. 2012, 27, 2284–2297. [Google Scholar] [CrossRef]
- Graovac, D.; Katic, V.; Rufer, A. Power quality compensation using universal power quality conditioning system. IEEE Power Eng. Rev. 2000, 20, 58–60. [Google Scholar]
- Somayajula, D.; Crow, M. An integrated dynamic voltage restorer-ultracapacitor design for improving power quality of the distribution grid. IEEE Trans. Sustain. Energy 2015, 6, 616–624. [Google Scholar] [CrossRef]
- Karimian, M.; Jalilian, A. Proportional-repetitive control of a dynamic voltage restorer (DVR) for power quality improvement. In Proceedings of the 17th Conference Electrical Power Distribution, Tehran, Iran, 1–6 May 2012. [Google Scholar]
- Fernandes, D.; Costa, F.; Martins, J.; Lock, A.; Silva, E.; Vitorino, M. Sensitive load voltage compensation with a suitable control method. In Proceedings of the IEEE Energy Conversion Congress Exposition, Montreal, QC, Canada, 20–24 September 2015. [Google Scholar]
- Balaguer, I.; Lei, Q.; Yang, S.; Supatti, U.; Peng, F. Control for grid-connected and intentional islanding operations of distributed power generation. IEEE Trans. Ind. Electron. 2011, 58, 147–157. [Google Scholar] [CrossRef]
- Ramadesigan, V.; Northrop, P.; De, S.; Santhanagopalan, S.; Braarz, R.; Subramanian, V. Modeling and simulation of lithium-ion batteries from a system engineering perspective. J. Electrochem. Soc. 2012, 159, R31–R45. [Google Scholar] [CrossRef]
- Domenico, D.; Creef, Y.; Prada, E.; Duchene, P.; Bernard, J.; Sauvan-Moynot, V. A review of approaches for the design of Li-ion BMS estimation functions. J. Oil Gas Sci. Technol. 2013, 68, 127–135. [Google Scholar] [CrossRef]
- Grainger, J.; William, D.S. Power System Analysis; McGraw-Hill Inc.: New York, NY, USA, 1994. [Google Scholar]
- Song-Manguelle, J.; Harfman, T.M.; Chi, S.; Gunturi, S.K.; Datta, R. Power transfer capability of HVAC cable for subsea transmission and distribution system. IEEE Trans. Ind. Appl. 2014, 50, 2382–2391. [Google Scholar] [CrossRef]
- Jothibasu, S.; Mishra, M.A. Control scheme for storageless DVR based on characterization of voltage sags. IEEE Trans. Power Deliv. 2014, 29, 2261–2269. [Google Scholar] [CrossRef]
- Nielsen, J.G.; Blaabjerg, F. A detailed comparison of system topologies for dynamic voltage restorers. IEEE Trans. Ind. Appl. 2015, 41, 1272–1280. [Google Scholar] [CrossRef]
- Woodley, N.H.; Morgan, L.; Sundaram, A. Experience with an inverter-based dynamic voltage restorer. IEEE Trans. Power Delivery 1999, 14, 1181–1186. [Google Scholar] [CrossRef]
- Sanchez, P.; Acha, E.; Calderon, J.; Feliu, V.; Cerrada, A. A versatile control scheme for a dynamic voltage restorer for power quality improvement. IEEE Trans. Power Delivery 2009, 24, 277–284. [Google Scholar] [CrossRef]
- Lam, C.S.; Wong, M.C.; Han, Y.D. Voltage swell and overvoltage compensation with unidirectional power flow controlled dynamic voltage restorer. IEEE Trans. Power Delivery 2008, 23, 2513–2521. [Google Scholar]
- Sadigh, A.; Smedley, K. Review of voltage compensation methods in dynamic voltage restorer DVR. In Proceedings of the IEEE Power and Energy Society General Meeting, San Diego, CA, USA, 22–26 July 2012. [Google Scholar]
- Rauf, A.M.; Khadkikar, V. An enhanced voltage sag compensation scheme for dynamic voltage restorer. IEEE Trans. Ind. Electron. 2015, 62, 2683–2692. [Google Scholar] [CrossRef]








| Part of Circuit | Descriptions | Value |
|---|---|---|
| Grid | Main Voltage | 220; 190; 150 V |
| 220; 230; 264 V | ||
| Frequency | 50 Hz | |
| Battery | Capacity | 50 Ah |
| Undervoltage Load | Sensitive Load Active | 80 kW |
| Sensitive Load Reactive | 60 kVar | |
| Overvoltage Load | Sensitive Load Active | 72 kW |
| Sensitive Load Reactive | 50 kVar | |
| DC Capacitor | Capacity | Cdc = 10 mF |
| PV System | Max Power | 113 kW |
| Open Circuit Voltage | 684 V | |
| Short Circuit Current | 180 A | |
| Vmpp | 675 V | |
| Immp | 168 A | |
| Series Connection | Transformer | 1:1 |
| Filter | Cse = 50 µF, Lse = 1 mH | |
| PI-1, PI-2 & PI-3 | 20 | |
| Shunt Connection | Filter | Csh = 20 µF, Lsh = 2 mH |
| PI-1 & PI-2 (Power Control) | 200 | |
| PI-1 & PI-2 (Voltage and Load Mode) | 10 | |
| PWM | Sampling Frequency | 5 kHz |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Simatupang, D.P.; Choi, J. Integrated Photovoltaic Inverters Based on Unified Power Quality Conditioner with Voltage Compensation for Submarine Distribution System. Energies 2018, 11, 2927. https://doi.org/10.3390/en11112927
Simatupang DP, Choi J. Integrated Photovoltaic Inverters Based on Unified Power Quality Conditioner with Voltage Compensation for Submarine Distribution System. Energies. 2018; 11(11):2927. https://doi.org/10.3390/en11112927
Chicago/Turabian StyleSimatupang, Desmon Petrus, and Jaeho Choi. 2018. "Integrated Photovoltaic Inverters Based on Unified Power Quality Conditioner with Voltage Compensation for Submarine Distribution System" Energies 11, no. 11: 2927. https://doi.org/10.3390/en11112927
APA StyleSimatupang, D. P., & Choi, J. (2018). Integrated Photovoltaic Inverters Based on Unified Power Quality Conditioner with Voltage Compensation for Submarine Distribution System. Energies, 11(11), 2927. https://doi.org/10.3390/en11112927





