Transient Modelling of Rotating and Stationary Cylindrical Heat Pipes: An Engineering Model
Abstract
:1. Introduction
2. Model Description
2.1. Model Geometry
2.2. Model Assumptions
- An axisymmetric geometry is chosen, requiring the omission of gravity, which is unidirectional in reality.
- A one-dimensional approach is taken for the flow of liquid and vapor.
- Thermal expansion of the heat pipe container and the wick is neglected.
- The dynamics of the vapor is much faster than the dynamics of the other components [3]. Therefore, the vapor is assumed to be always saturated, taking into account the mass flows of the phase change and its associated latent heat.
2.3. Governing Physics
2.3.1. Wall Component
2.3.2. Liquid Component
2.3.3. Vapor Component
2.4. Boundary Conditions
2.5. Numerical Solution
3. Results and Discussion
3.1. Transient Behaviour Validations
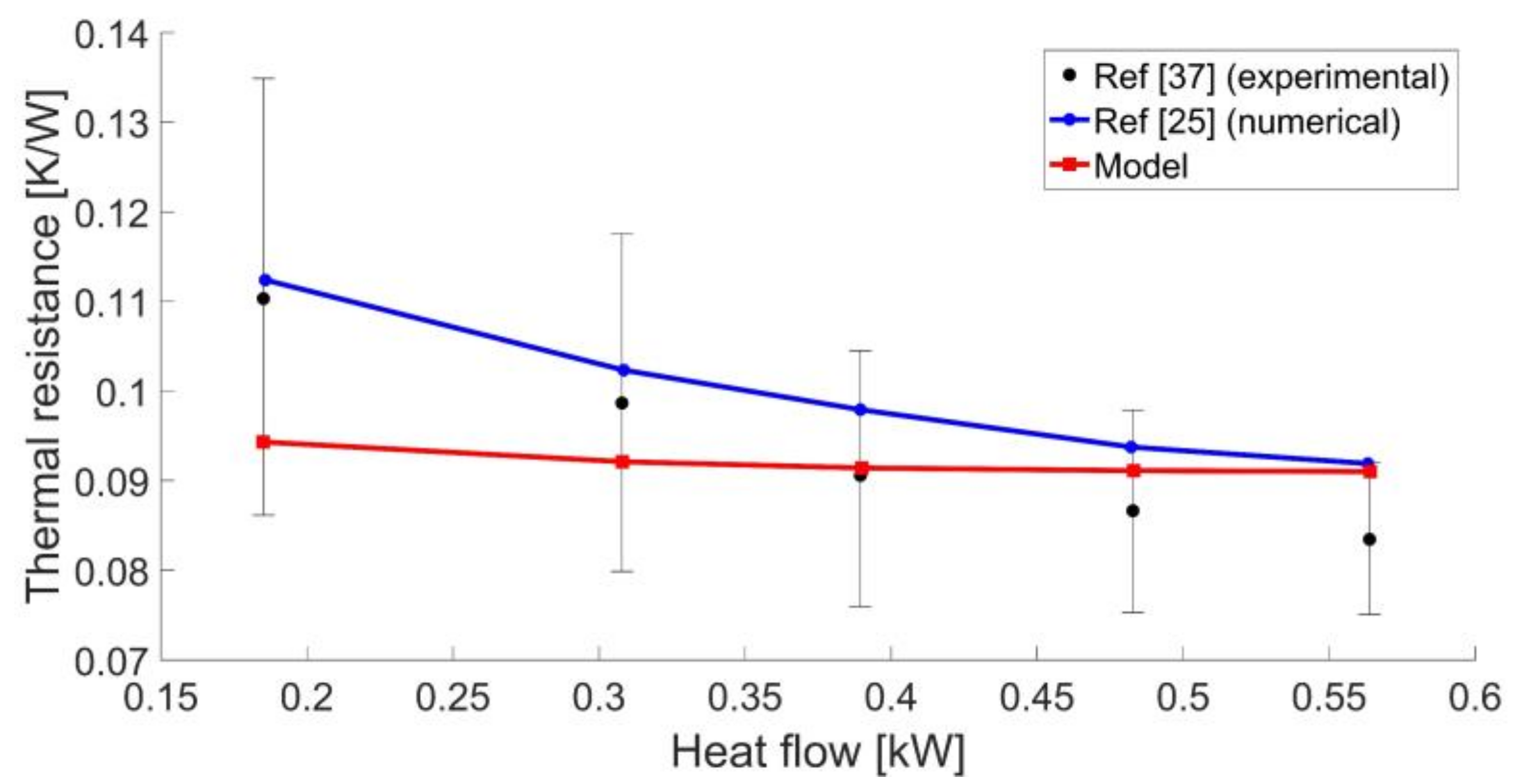
3.2. Steady-State Behaviour Validations
3.3. Computational Efficiency
3.4. Parametric Study
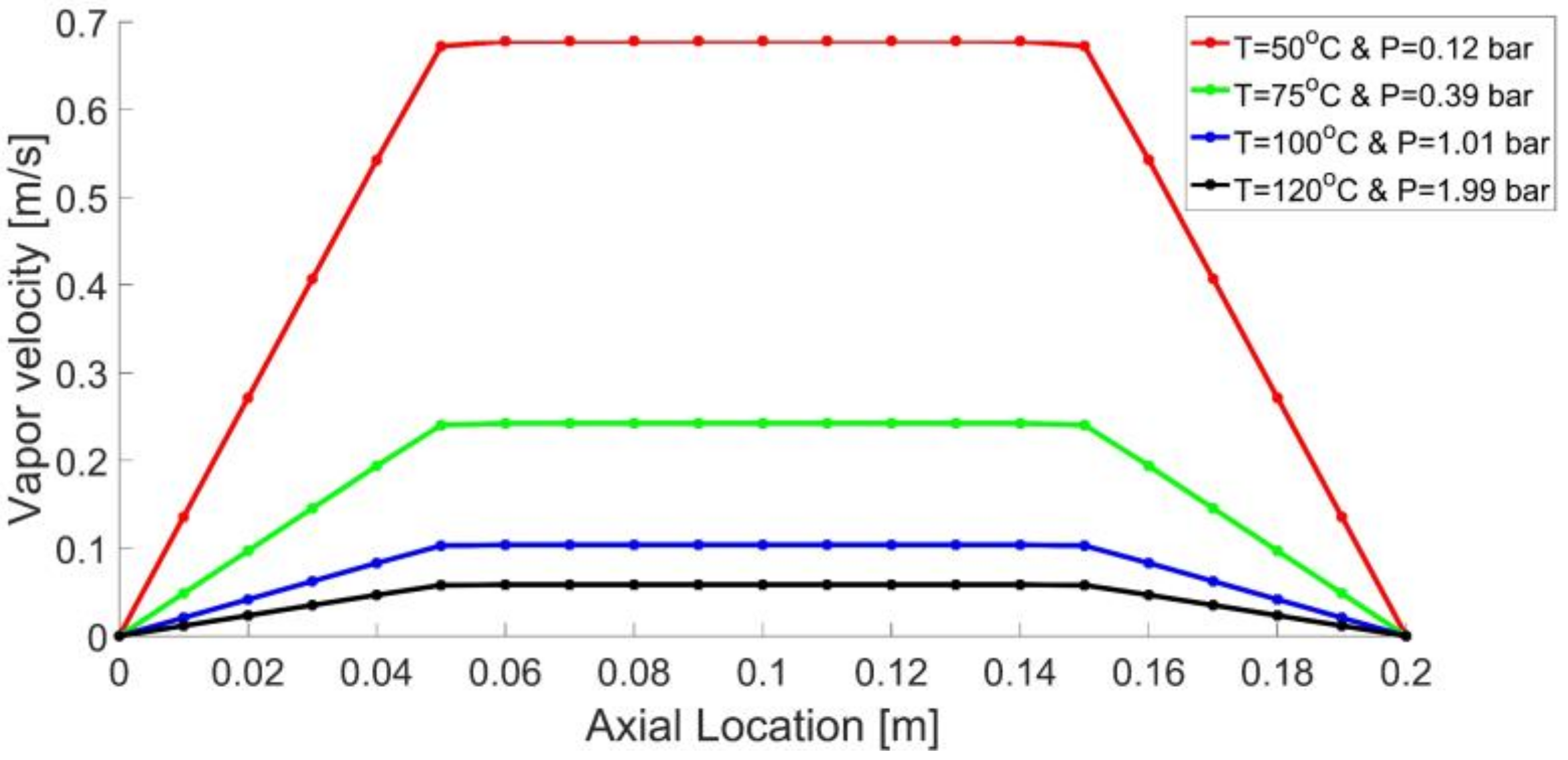
3.4.1. Effect of Operating Temperature on Vapor Dynamics
3.4.2. Effect of Rotational Speed on Liquid Height Distribution
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Cross-sectional area of the node in the axial direction, m | |
| Coefficients of polynomial velocity profile | |
| Specific heat, J/(kg·K) | |
| Thickness, m | |
| Correction coefficient for capillary pressure | |
| Heat transfer coefficient, W/(m2·K) | |
| Latent heat, J/kg | |
| Wick permeability, m2 | |
| Thermal conductivity, W/(m·K) | |
| Heat pipe total length, m | |
| Molecular weight, kg/mol | |
| Mass, kg | |
| Mass flow rate, kg/s | |
| Heat flow, W | |
| Heat flux, W/m2 | |
| Pressure, Pa | |
| Thermal resistance, K/W | |
| Universal gas constant, J/(mol·K) | |
| Radial coordinate, radius, m | |
| Radii of the resistance, m | |
| Temperature, K | |
| Time, s | |
| Velocity, m/s | |
| Volume, m3 | |
| Axial coordinate | |
| Greek symbols | |
| Viscous term in momentum equation, kg/(m2·s2) | |
| Difference | |
| Length of the node in the axial direction, m | |
| Distance between the adjacent node centers, m | |
| Porosity | |
| Viscosity, Pa·s | |
| Density, kg/m3 | |
| Surface tension, N/m | |
| Angular coordinate | |
| Rotational speed, rad/s | |
| Subscripts | |
| Capillary | |
| Convection | |
| Effective | |
| Inner | |
| Liquid | |
| Outer | |
| Phase change | |
| Radiation | |
| Solid | |
| Vapor | |
| Wick | |
| Ambient | |
References
- Faghri, A. Review and advances in heat pipe science and technology. J. Heat Trans. 2012, 134, 123001. [Google Scholar] [CrossRef]
- Reay, D.; McGlen, R.; Kew, P. Heat pipes: Theory, design and applications, 6th ed.; Butterworth-Heinemann: Oxford, UK, 2014; pp. 15–43. ISBN 978-0-08-098266-3. [Google Scholar]
- Zuo, Z.J.; Faghri, A. A network thermodynamic analysis of the heat pipe. Int. J. Heat Mass Transf. 1998, 41, 1473–1484. [Google Scholar] [CrossRef]
- Grover, G.M.; Cotter, T.P.; Erickson, G.F. Structures of very high thermal conductance. J. Appl. Phys. 1964, 35, 1990–1991. [Google Scholar] [CrossRef]
- Ballback, L.J. The operation of a rotating wickless heat pipe. Ph.D. Thesis, US Naval Postgraduate School, Monterey, CA, USA, 1969. [Google Scholar]
- Kim, K.S.; Won, M.H.; Kim, J.W.; Back, B.J. Heat pipe cooling technology for desktop PC CPU. Appl. Therm. Eng. 2003, 23, 1137–1144. [Google Scholar] [CrossRef]
- Lin, L.; Ponnappan, R.; Leland, J. High performance miniature heat pipe. Int. J. Heat Mass Transf. 2002, 45, 3131–3142. [Google Scholar] [CrossRef]
- Ivanova, M.; Avenas, Y.; Schaeffer, C.; Dezord, J.B.; Schulz-Harder, J. Heat pipe integrated in direct bonded copper (DBC) technology for cooling of power electronics packaging. IEEE Trans. Power Electron. 2006, 21, 1541–1547. [Google Scholar] [CrossRef]
- Vasiliev, L.L. Micro and miniature heat pipes—Electronic component coolers. Appl. Therm. Eng. 2008, 28, 266–273. [Google Scholar] [CrossRef]
- Turner, R. The “constant temperature” heat pipe—A unique device for the thermal control of spacecraft components. In Proceedings of the AIAA 4th Thermophysics Conference, San Francisco, CA, USA, 16–18 June 1969; pp. 69–632. [Google Scholar] [CrossRef]
- Liang, J.; Gan, Y.; Li, Y. Investigation on the thermal performance of a battery thermal management system using heat pipe under different ambient temperatures. Energy Convers. Manag. 2018, 155, 1–9. [Google Scholar] [CrossRef]
- Kuboth, S.; König-Haagen, A.; Brüggemann, D. Numerical Analysis of Shell-and-Tube Type Latent Thermal Energy Storage Performance with Different Arrangements of Circular Fins. Energies 2017, 10, 274. [Google Scholar] [CrossRef]
- Jen, T.C.; Gutierrez, G.; Eapen, S.; Barber, G.; Zhao, H.; Szuba, P.S.; Labataille, J.; Manjunathaiah, J. Investigation of heat pipe cooling in drilling applications.: Part I: Preliminary numerical analysis and verification. Int. J. Mach. Tool Manu. 2002, 42, 643–652. [Google Scholar] [CrossRef]
- Ponnappan, R.; He, Q.; Leland, J. Test results of a high-speed rotating heat pipe. In Proceedings of the AIAA 32nd Thermophysics Conference, Atlanta, GA, USA, 23–25 June 1997; p. 2543. [Google Scholar] [CrossRef]
- Kukharskii, M.P. Closed evaporative cooling of rotating electrical machines. J. Eng. Phys. 1974, 27, 1090–1096. [Google Scholar] [CrossRef]
- Groll, M.; Krahling, H.; Munzel, W.D. Heat pipes for cooling of an electric motor. J. Energy 1978, 2, 363–367. [Google Scholar] [CrossRef]
- Celik, M.; Devendran, K.; Paulussen, G.; Pronk, P.; Frinking, F.; de Jong, W.; Boersma, B.J. Experimental and numerical investigation of contact heat transfer between a rotating heat pipe and a steel strip. Int. J. Heat Mass Transf. 2018, 122, 529–538. [Google Scholar] [CrossRef]
- Xuan, Y.; Hong, Y.; Li, Q. Investigation on transient behaviors of flat plate heat pipes. Exp. Therm. Fluid Sci. 2004, 28, 249–255. [Google Scholar] [CrossRef]
- Ferrandi, C.; Iorizzo, F.; Mameli, M.; Zinna, S.; Mameli, M. Lumped parameter model of sintered heat pipe: Transient numerical analysis and validation. Appl. Therm. Eng. 2013, 50, 1280–1290. [Google Scholar] [CrossRef]
- Wits, W.W.; Kok, J.B. Modeling and validating the transient behavior of flat miniature heat pipes manufactured in multilayer printed circuit board technology. J. Heat Trans. 2011, 133, 081401. [Google Scholar] [CrossRef]
- Tournier, J.M.; El-Genk, M.S. A heat pipe transient analysis model. Int. J. Heat Mass Transf. 1994, 37, 753–762. [Google Scholar] [CrossRef]
- Solomon, A.B.; Ramachandran, K.; Asirvatham, L.G.; Pillai, B.C. Numerical analysis of a screen mesh wick heat pipe with Cu/water nanofluid. Int. J. Heat Mass Transf. 2014, 75, 523–533. [Google Scholar] [CrossRef]
- Daniels, T.C.; Al-Jumaily, F.K. Investigations of the factors affecting the performance of a rotating heat pipe. Int. J. Heat Mass Transf. 1975, 18, 961–973. [Google Scholar] [CrossRef]
- Bertossi, R.; Guilhem, N.; Ayel, V.; Romestant, C.; Bertin, Y. Modeling of heat and mass transfer in the liquid film of rotating heat pipes. Int. J. Therm. Sci. 2012, 52, 40–49. [Google Scholar] [CrossRef]
- Song, F.; Ewing, D.; Ching, C.Y. Fluid flow and heat transfer model for high-speed rotating heat pipes. Int. J. Heat Mass Transf. 2003, 46, 4393–4401. [Google Scholar] [CrossRef]
- Uddin, Z.; Harmand, S.; Ahmed, S. Computational modeling of heat transfer in rotating heat pipes using nanofluids: A numerical study using PSO. Int. J. Therm. Sci. 2017, 112, 44–54. [Google Scholar] [CrossRef]
- Hassan, H.; Harmand, S. Effect of using nanofluids on the performance of rotating heat pipe. Appl. Math. Model. 2015, 39, 4445–4462. [Google Scholar] [CrossRef]
- Harley, C.; Faghri, A. Two-dimensional rotating heat pipe analysis. J. Heat Trans. 1995, 117, 202–208. [Google Scholar] [CrossRef]
- Baker, J.; Ponnappan, R.; Leland, J. A computational model of transport within a rotating heat pipe—Part 1—Formulation. In Proceedings of the AIAA 37th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 11–14 January 1999. [Google Scholar] [CrossRef]
- Lian, W.; Chang, W.; Xuan, Y. Numerical investigation on flow and thermal features of a rotating heat pipe. Appl. Therm. Eng. 2016, 101, 92–100. [Google Scholar] [CrossRef]
- Zhu, N.; Vafai, K. Analysis of cylindrical heat pipes incorporating the effects of liquid–vapor coupling and non-Darcian transport—A closed form solution. Int. J. Heat Mass Transf. 1999, 42, 3405–3418. [Google Scholar] [CrossRef]
- Aghvami, M.; Faghri, A. Analysis of flat heat pipes with various heating and cooling configurations. Appl. Therm. Eng. 2011, 31, 2645–2655. [Google Scholar] [CrossRef]
- Hansen, G.; Næss, E.; Kristjansson, K. Analysis of a vertical flat heat pipe using potassium working fluid and a wick of compressed nickel foam. Energies 2016, 9, 170. [Google Scholar] [CrossRef]
- Nemec, P.; Čaja, A.; Malcho, M. Mathematical model for heat transfer limitations of heat pipe. Math. Comput. Model. 2013, 57, 126–136. [Google Scholar] [CrossRef]
- Chan, S.H.; Kanai, Z.; Yang, W.T. Theory of a rotating heat pipe. J. Nucl. Energy 1971, 25, 479–487. [Google Scholar] [CrossRef]
- Lin, L.; Faghri, A. Heat transfer in micro region of a rotating miniature heat pipe. Int. J. Heat Mass Transf. 1999, 42, 1363–1369. [Google Scholar] [CrossRef]
- Song, F.; Ewing, D.; Ching, C.Y. Heat transfer in the evaporator section of moderate-speed rotating heat pipes. Int. J. Heat Mass Transf. 2008, 51, 1542–1550. [Google Scholar] [CrossRef]
- Song, F.; Ewing, D.; Ching, C.Y. Experimental investigation on the heat transfer characteristics of axial rotating heat pipes. Int. J. Heat Mass Transf. 2004, 47, 4721–4731. [Google Scholar] [CrossRef]
- Huang, L.; El-Genk, M.S.; Tournier, J.M. Transient performance of an inclined water heat pipe with a screen wick. In Proceedings of the ASME 29th National Heat Transfer Conference, Atlanta, GA, USA, 8–11 August 1993. [Google Scholar]




| Case | Mesh Size | Computational Time | Relative Error |
|---|---|---|---|
| m = 1.2 g | 80 | 100% (Ref) | 0% (Ref) |
| 40 | 28.7% | <0.01% | |
| 20 | 9.0% | 0.01% | |
| 10 | 3.2% | 0.06% | |
| m = 0.9 g | 80 | 100% (Ref) | 0% (Ref) |
| 40 | 29.4% | 0.04% | |
| 20 | 9.6% | 0.16% | |
| 10 | 3.4% | 0.55% |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Celik, M.; Paulussen, G.; Van Erp, D.; De Jong, W.; Boersma, B.J. Transient Modelling of Rotating and Stationary Cylindrical Heat Pipes: An Engineering Model. Energies 2018, 11, 3458. https://doi.org/10.3390/en11123458
Celik M, Paulussen G, Van Erp D, De Jong W, Boersma BJ. Transient Modelling of Rotating and Stationary Cylindrical Heat Pipes: An Engineering Model. Energies. 2018; 11(12):3458. https://doi.org/10.3390/en11123458
Chicago/Turabian StyleCelik, Metin, Geert Paulussen, Dennis Van Erp, Wiebren De Jong, and Bendiks Jan Boersma. 2018. "Transient Modelling of Rotating and Stationary Cylindrical Heat Pipes: An Engineering Model" Energies 11, no. 12: 3458. https://doi.org/10.3390/en11123458
APA StyleCelik, M., Paulussen, G., Van Erp, D., De Jong, W., & Boersma, B. J. (2018). Transient Modelling of Rotating and Stationary Cylindrical Heat Pipes: An Engineering Model. Energies, 11(12), 3458. https://doi.org/10.3390/en11123458





