LCL Filter Design with EMI Noise Consideration for Grid-Connected Inverter
Abstract
:1. Introduction
2. LCL Filter Design Procedure
2.1. Components of the LCL Filter
2.2. Simulation Results
3. Magnetic Material in the LCL Filter Design
3.1. Core Material Selection
3.2. 3D Printing Technology for Bobbin Design
4. Inductor Winding Design
4.1. Parasitic Components in the Two Types of Wound Inductors
4.2. EMI Noise Model with LCL Filter Consideration
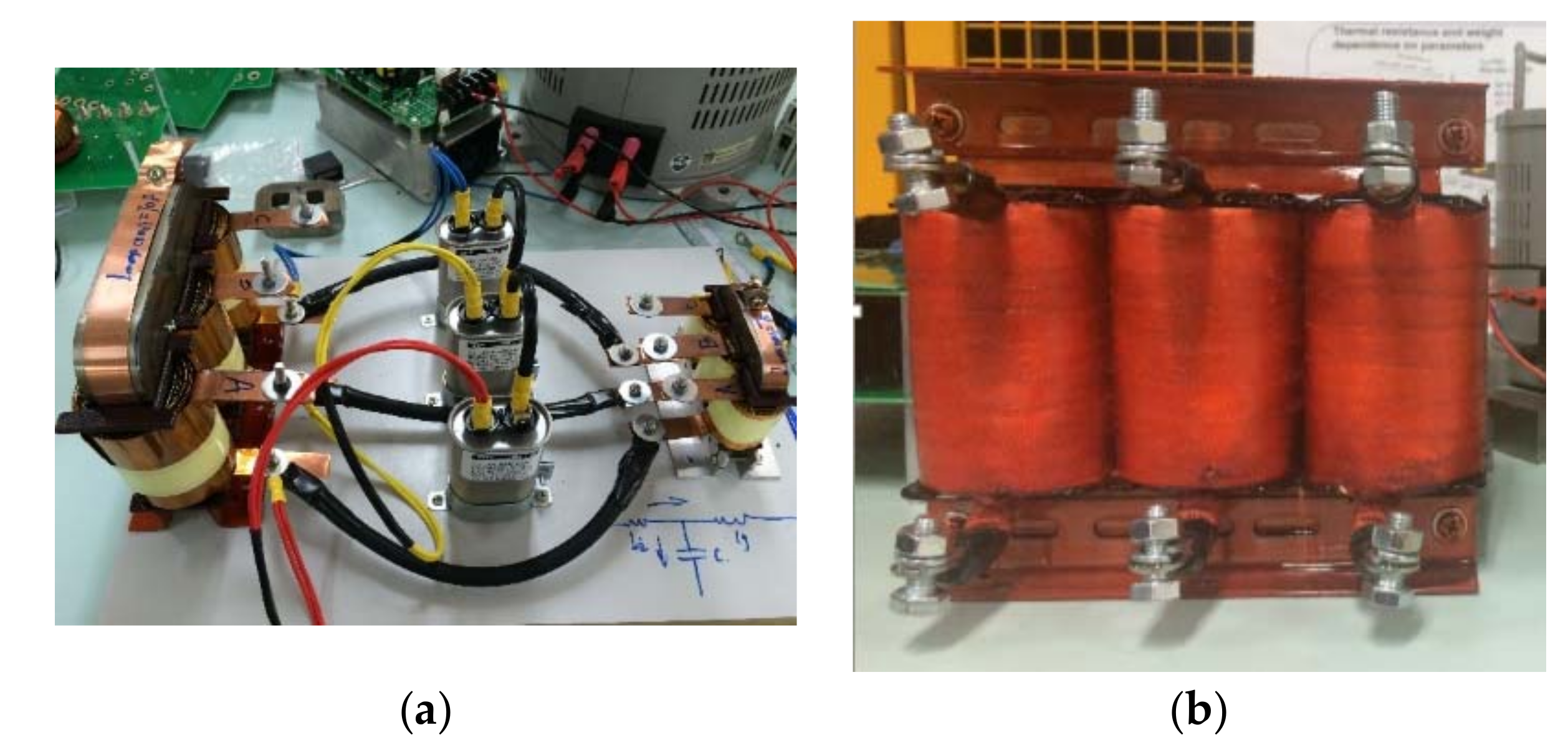
5. Experiment Results
5.1. Copper Wire Inductor Analysis Results
5.2. Copper Foil Inductor Analysis Results
6. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Rosero, J.; Ortega, J.; Aldabas, E.; Romeral, L. Moving towards a more electric aircraft. IEEE Aerosp. Electron. Syst. Mag. 2007, 22, 3–9. [Google Scholar] [CrossRef] [Green Version]
- Sinnett, M. 787 No-bleed systems: Saving fuel and enhancing operational efficiencies. Aero Quart. 2007, 18, 6–11. [Google Scholar]
- Sarlioglu, B.; Morris, C.T. More electric aircraft: Review, challenges, and opportunities for commercial transport aircraft. IEEE Trans. Transp. Electr. 2015, 1, 54–64. [Google Scholar] [CrossRef]
- Cao, W.; Mecrow, B.C.; Atkinson, G.J.; Bennett, J.W.; Atkinson, D.J. Overview of electric motor technologies used for more electric aircraft (MEA). IEEE Trans. Ind. Electron. 2012, 59, 3523–3531. [Google Scholar]
- Bhangu, B.S.; Rajashekara, K. Electric starter generators: Their integration into gas turbine engines. IEEE Ind. Appl. Mag. 2014, 20, 14–22. [Google Scholar] [CrossRef]
- Donghua, P.; Xinbo, R.; Chenlei, B.; Weiwei, L.; Xuehua, W. Magnetic Integration of the LCL Filter in Grid-Connected Inverters. IEEE Trans. Power Electron. 2014, 29, 1573–1578. [Google Scholar] [CrossRef]
- Zhang, X. Passive Component Weight Reduction for Three Phase Power Converters; Virginia Polytechnic Institute and State University: Blacksburg, VA, USA, 2014. [Google Scholar]
- Wang, S.; Lee, F.C.; Van Wyk, J.D. Design of inductor winding capacitance cancellation for EMI suppression. IEEE Trans. Power Electron. 2006, 21, 1825–1832. [Google Scholar] [CrossRef]
- Stadler, A.; Huber, R.; Stolzke, T.; Gulden, C. Analytical calculation of copper losses in litz-wire windings of gapped inductors. IEEE Trans. Magn. 2014, 50, 81–84. [Google Scholar] [CrossRef]
- Hamalainen, H.; Pyrhonen, J.; Nerg, J.; Talvitie, J. AC resistance factor of litz-wire windings used in low-voltage high-power generators. IEEE Trans. Ind. Electron. 2014, 61, 693–700. [Google Scholar] [CrossRef]
- Burkart, R.M.; Uemura, H.; Kolar, J.W. Optimal Inductor Design for 3-Phase Voltage-Source PWM Converters Considering Different Magnetic Materials and a Wide Switching Frequency Range. In Proceedings of the International Power Electronics Conference (IPEC-Hiroshima 2014-ECCE ASIA), Hiroshima, Japan, 18–21 May 2014; pp. 891–898. [Google Scholar]
- Fan, T.; Li, Q.; Wen, X. Development of a high power density motor made of amorphous alloy cores. IEEE Trans. Ind. Electron. 2014, 61, 4510–4518. [Google Scholar] [CrossRef]
- Ji, J.; Wu, W.; He, Y.; Lin, Z.; Blaabjerg, F.; Chung, H. A simple differential mode EMI suppressor for the LLCL-filter based single phase grid-tied transformerless inverter. IEEE Trans. Ind. Electron. 2014. [Google Scholar] [CrossRef]
- Cheng, W.; He, X.; Xu, S.; Sun, W. Analysis of common-mode electromagnetic interference noise in a flyback converter using a self-supply power control integrated circuit. IET Power Electron. 2015, 8, 1749–1757. [Google Scholar] [CrossRef]
- Yang, X.; Long, Z.; Wen, Y.; Huang, H.; Palmer, P.R. Investigation of the trade-off between switching losses and EMI generation in Gaussian S-shaping for high-power IGBT switching transients by active voltage control. IET Power Electron. 2016, 9, 1979–1984. [Google Scholar] [CrossRef]
- Bishnoi, H.; Mattavelli, P.; Burgos, R.P.; Boroyevich, D. EMI filter design of DC-fed motor-drives using behavioral EMI models. In Proceedings of the 17th European Conference on Power Electronics and Applications (EPE’15 ECCE-Europe), Geneva, Switzerland, 8–10 September 2015; pp. 1–10. [Google Scholar]
- Weimin, W.; Yunjie, S.; Zhe, L.; Yuanbin, H.; Min, H.; Blaabjerg, F.; Chung, H.S.h. A modified LLCL filter with the reduced conducted EMI noise. IEEE Trans. Power Electron. 2014, 29, 3393–3402. [Google Scholar] [CrossRef]
- Liserre, M.; Blaabjerg, F.; Hansen, S. Design and control of an LCL-filter-based three-phase active rectifier. IEEE Trans. Ind. Appl. 2005, 41, 1281–1291. [Google Scholar] [CrossRef]
- Yang, S.F.; Huang, T.H. Design of single-turn spiral inductors with embedding a strong-coupling LC resonator for interference suppression. IEEE Trans. Electromagn. Compat. 2017, 59, 919–926. [Google Scholar] [CrossRef]
- Zheng, X.; Xiao, L.; Lei, Y.; Wang, Z. Optimisation of LCL filter based on closed-loop total harmonic distortion calculation model of the grid-connected inverter. IET Power Electron. 2015, 8, 860–868. [Google Scholar] [CrossRef]
- Muhlethaler, J.; Schweizer, M.; Blattmann, R.; Kolar, J.W.; Ecklebe, A. Optimal design of LCL harmonic filters for three-phase PFC rectifiers. IEEE Trans. Power Electron. 2013, 28, 3114–3125. [Google Scholar] [CrossRef]
- Weimin, W.; Yunjie, S.; Zhe, L.; Tianhao, T.; Blaabjerg, F.; Chung, H.S.h. A new type LCL filter with in-series parallel resonant circuit for single-phase grid-tied inverter. IEEE Trans. Ind. Electron. 2014, 61, 4640–4644. [Google Scholar] [CrossRef]
- Patel, Y.; Pixler, D.; Nasiri, A. Analysis and design of TRAP and LCL filters for active switching converters. In Proceedings of the 2010 IEEE International Symposium on Industrial Electronics (ISIE), Bari, Italy, 4–7 July 2010; pp. 638–643. [Google Scholar]
- Langella, R.; Testa, A.; Alii, E. IEEE Recommended Practice and Requirements for Harmonic Control in Electric Power Systems; IEEE: Piscataway, NJ, USA, 2014. [Google Scholar]
- Jiao, Y.; Lee, F.C. LCL filter design and inductor current ripple analysis for a three-level NPC grid interface converter. IEEE Trans. Power Electron. 2015, 30, 4659–4668. [Google Scholar] [CrossRef]













| Maximum Harmonic Current Distortion in Percent of I1 | |
|---|---|
| 4.0% | |
| 2.0% | |
| 1.5% | |
| 0.6% | |
| 0.3% | |
| THD | 5% |
| Material | Ferrite | Power Iron | Nanocrystalline | Amorphous |
|---|---|---|---|---|
| (T) | 0.49 | 0.6–1.3 | 1.56 | 1.2 |
| 16.9 | 1798 | 2.3 | 0.053 | |
| 1.25 | 1.02 | 1.32 | 1.81 | |
| 2.35 | 1.89 | 2.1 | 1.74 |
| Parameters | Li | Lg |
|---|---|---|
| Core type | AFEC-90a | AFEC-48a |
| Foil size | ||
| n | 14 | 5 |
| 1 mm | 0.5 mm |
| Parameters | Copper Foil | Copper Wire | ||
|---|---|---|---|---|
| Estimated | Improved | Estimated | Improved | |
| 90 | 84 | 90 | 82 | |
| 103 | 110 | 237 | 260 | |
| 4922 | 4922 | 3525 | 3525 | |
| 10.8 | 3.8 | 10.6 | 2 | |
| 67.3 | 190 | 180 | 1000 | |
| 1916 | 1916 | 626 | 626 | |
| 0.65 | 0.46 | 1.13 | 0.9 | |
| 11.9 | 22 | 0.25 | 30 | |
| 237 | 237 | 578 | 578 | |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Lai, C.-M. LCL Filter Design with EMI Noise Consideration for Grid-Connected Inverter. Energies 2018, 11, 1646. https://doi.org/10.3390/en11071646
Liu Y, Lai C-M. LCL Filter Design with EMI Noise Consideration for Grid-Connected Inverter. Energies. 2018; 11(7):1646. https://doi.org/10.3390/en11071646
Chicago/Turabian StyleLiu, Yitao, and Ching-Ming Lai. 2018. "LCL Filter Design with EMI Noise Consideration for Grid-Connected Inverter" Energies 11, no. 7: 1646. https://doi.org/10.3390/en11071646
APA StyleLiu, Y., & Lai, C.-M. (2018). LCL Filter Design with EMI Noise Consideration for Grid-Connected Inverter. Energies, 11(7), 1646. https://doi.org/10.3390/en11071646






