Feature Extraction and Comprehension of Partial Discharge Characteristics in Transformer Oil from Rated AC Frequency to Very Low Frequency
Abstract
1. Introduction
2. Experimental Setup and Analysis Method
2.1. Test System, Procedure, and Sample Preparation
2.2. Analysis Method
3. Experimental Results
3.1. Statistical Parameters of Discharge
3.1.1. PDIV
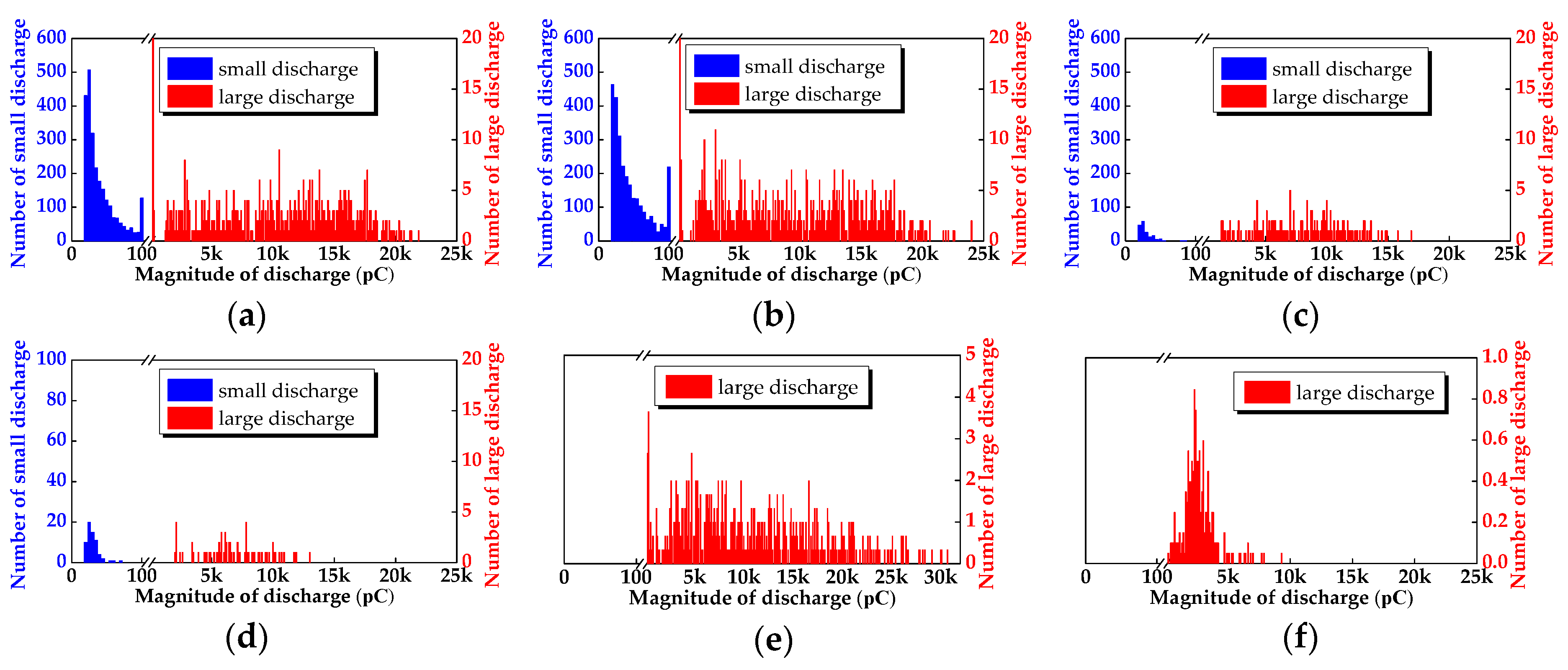
3.1.2. Maximum Magnitude of Discharge
3.1.3. Mean Magnitude of Discharge
3.1.4. Pulse Repetition Rate
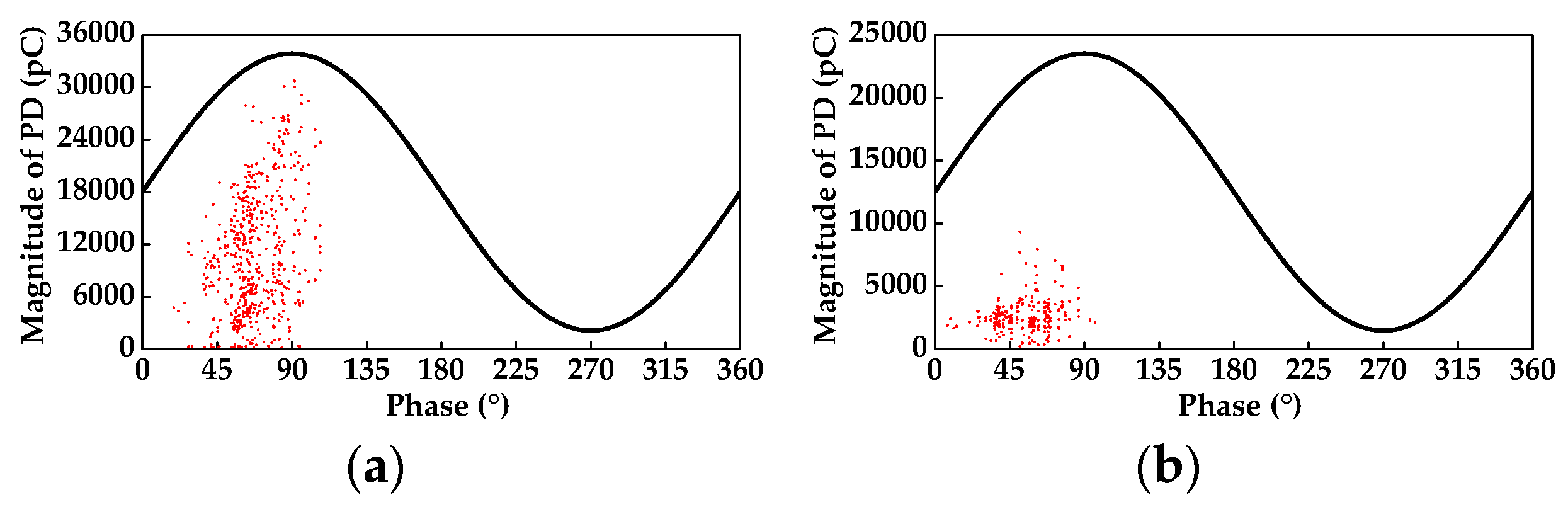
3.2. PRPD
3.3. Statistical Patterns and Characteristic Parameters
3.3.1. Statistical Patterns
3.3.2. Characteristic Parameters
4. Discussion and Analysis
4.1. Influence on Electric Field Distribution of Frequency
4.2. Influence on Discharge Process of Frequency
5. Conclusions
- (1)
- Some fundamental characteristics remained, even the frequency decreases. Discharge in positive half cycle initiates earlier than that in negative half cycle. The magnitude of discharge in positive half cycle is obviously larger than that in negative half cycle. Discharge in both cycle distributes in the vicinity of voltage peak.
- (2)
- With the decrease of frequency, the inception voltage increases, which, under 0.1 Hz, is 2.4 times of that under rated AC frequency. The maximum and average magnitude of discharge and pulse repetition rate first increase slightly and then decrease obviously. For the voltage amplitude of 20 kV, Qmax+ under 5 Hz decreases by about 40% compared with 50 Hz. Qmean+ decreases by about 45%. R+ decreases by about 85%.
- (3)
- The range of the qmean–φ, n–φ, and n–q patterns shortens. The right side of the mountain shape of qmean–φ pattern gradually disappears. The range of the vertical axis in positive cycle under 5 Hz decreases by about 25%. The n–φ pattern appears several peaks in positive cycle under 0.1 Hz. The range of the vertical axis in positive cycle under 5 Hz decreases by about 75%. The part of the large discharge in n–q pattern changes from a turtle back shape to a right-angled triangle. The range of horizontal axis under 5 Hz decreases by about 50%.
- (4)
- The skewness of all of the statistical patterns decreases and then increases under 0.1 Hz. Sk+(qmean–φ) decreases from −0.0232 under 50 Hz to −0.4283 under 1 Hz, and then increases to −0.1525 under 0.1 Hz. Ku(qmax–φ) and Ku(qmean–φ) increase. The value of Asy(qmax–φ) and Asy(qmean–φ) under each frequency is near zero. Cc(qmax–φ), Cc(qmean–φ), and Cc(n–φ) decrease, indicating the similarity of the outlines of statistical patterns decrease.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Glowacz, A.; Glowacz, W.; Glowacz, Z. Recognition of armature current of DC generator depending on rotor speed using FFT, MSAF-1 and LDA. Eksploat. Niezawodn. Maint. Reliab. 2015, 17, 64–69. [Google Scholar] [CrossRef]
- Glowacz, A.; Glowacz, W.; Glowacz, Z.; Kozik, J.; Gutten, M.; Korenciak, D.; Khan, Z.F.; Irfan, M.; Carletti, E. Fault diagnosis of three phase induction motor using current signal, MSAF-Ratio15 and selected classifiers. Arch. Metall. Mater. 2017, 62, 2413–2419. [Google Scholar] [CrossRef]
- Gutten, M.; Korenciak, D.; Kucera, M.; Sebok, M.; Opielak, M.; Zukowski, P.; Koltunowicz, T.N. Maintenance diagnostics of transformers considering the influence of short-circuit currents during operation. Eksploat. Niezawodn. Maint. Reliab. 2017, 19, 459–466. [Google Scholar] [CrossRef]
- Lei, T.; Faifer, M.; Ottoboni, R.; Toscani, S. On-line fault detection technique for voltage transformers. Measurement 2017, 108, 193–200. [Google Scholar] [CrossRef]
- Zhu, L.; Feng, H.; Ji, S.; Rehman, H.; Wu, X. Primary Differential pulse method for partial discharge detection of oil-immersed inverted current transformers. IEEE Trans. Power Deliv. 2018, 33, 1492–1494. [Google Scholar] [CrossRef]
- Saravanakumar, N.; Sathiyasekar, K. Efficient method for location and detection of partial discharge in transformer oil by DOA estimation of circular array of ultrasonic sensors. J. Instrum. 2018, 13, P01026. [Google Scholar] [CrossRef]
- Ma, G.; Zhou, H.; Shi, C.; Li, Y.; Zhang, Q. Distributed partial discharge detection in a power transformer based on phase-shifted FBG. IEEE Sens. J. 2018, 18, 2788–2795. [Google Scholar] [CrossRef]
- Bhimani, B.V. Resistance and capacitance measurement on high-voltage insulation at very-low frequencies. Trans. Am. Inst. Electr. Eng. 1961, 80, 155–163. [Google Scholar]
- Fard, M.A.; Reid, A.J.; Hepburn, D.M. Analysis of HVDC superimposed harmonic voltage effects on partial discharge behavior in solid dielectric media. IEEE Trans. Dielectr. Electr. Insul. 2017, 24, 7–16. [Google Scholar] [CrossRef]
- Eager, G.S.; Katz, C.; Fryszczyn, B.; Densley, J.; Bernstein, B.S. High voltage VLF testing of power cables. IEEE Trans. Power Deliv. 1997, 12, 565–570. [Google Scholar] [CrossRef]
- Bhimani, B.V. Very-low-frequency high-potential testing. IEEE Trans. Power App. Syst. 1961, 80, 148–155. [Google Scholar] [CrossRef]
- Miller, R.; Black, I.A. Partial discharge measurements over the frequency range 0.1 Hz to 50 Hz. IEEE Trans. Electr. Insul. 1977, 12, 224–233. [Google Scholar] [CrossRef]
- Haga, K.; Yoneyama, M. Breakdown strength and dielectric characteristics of oil-impregnated paper cable insulation at very-low-frequencies. Electr. Eng. Jpn. 1968, 88, 39–48. [Google Scholar]
- Miller, R.; Black, I.A.; Gray, V.N. A variable low frequency high voltage generator using valves with fibre optic light guide control. J. Phys. E Sci. Instrum. 1975, 8, 748–750. [Google Scholar] [CrossRef]
- Miller, R.; Black, I.A. Partial discharge energy measurements on electrical machine insulation when energized at frequencies between 0.1 Hz and power frequency. IEEE Trans. Electr. Insul. 1979, 14, 127–135. [Google Scholar] [CrossRef]
- Bodega, R.; Morshuis, P.H.F.; Lazzaroni, M.; Wester, F.J. PD recurrence in cavities at different energizing methods. IEEE Trans. Instrum. Meas. 2004, 53, 251–258. [Google Scholar] [CrossRef]
- Wester, F.J.; Guilski, E.; Smit, J.J. Detection of partial discharges at different AC voltage stresses in power cables. IEEE Electr. Insul. Mag. 2007, 23, 28–43. [Google Scholar] [CrossRef]
- Illias, H.; Chen, G.; Lewin, P.L. Partial discharge behavior within a spherical cavity in a solid dielectric material as a function of frequency and amplitude of the applied voltage. IEEE Trans. Dielectr. Electr. Insul. 2011, 18, 432–443. [Google Scholar] [CrossRef]
- Forssen, C.; Edin, H. Partial discharges in a cavity at variable applied frequency part 1: Measurements. IEEE Trans. Dielectr. Electr. Insul. 2008, 15, 1601–1609. [Google Scholar] [CrossRef]
- Forssen, C.; Edin, H. Partial discharges in a cavity at variable applied frequency Part 2: Measurements and Modeling. IEEE Trans. Dielectr. Electr. Insul. 2008, 15, 1610–1616. [Google Scholar] [CrossRef]
- Fynes-Clinton, D.; Nyamupangedengu, C. Partial discharge characterization of cross-linked polyethylene medium voltage power cable termination defects at very low frequency (0.1 Hz) and power frequency test voltages. IEEE Electr. Insul. Mag. 2016, 32, 15–23. [Google Scholar] [CrossRef]
- Yang, Z.; Zhu, D. High Voltage and Insulation Technology, 2nd ed.; China Electric Power Press: Beijing, China, 2007. [Google Scholar]
- Zhang, L.; Zhao, L. Modeling of the partial discharge process in a liquid dielectric: Effect of applied voltage, gap distance, and electrode type. Energies 2013, 6, 934–952. [Google Scholar]
- Pompili, M.; Mazzetti, C.; Bartnikas, R. Phase relationship of PD pulses in dielectric liquids under ac conditions. IEEE Trans. Dielectr. Electr. Insul. 2000, 7, 113–117. [Google Scholar] [CrossRef]
- Swati, K.; Yadav, K.S.; Sarathi, R.; Vinu, R.; Danikas, M.G. Understanding corona discharge activity in titania nanoparticles dispersed in transformer oil under AC and DC voltages. IEEE Trans. Dielectr. Electr. Insul. 2017, 24, 2325–2336. [Google Scholar] [CrossRef]
- Beroual, A.; Khaled, U.; Noah, P.S.M.; Sitorus, H. Comparative study of breakdown voltage of mineral, synthetic and natural oils and based mineral oil mixtures under AC and DC voltages. Energies 2017, 10, 511. [Google Scholar] [CrossRef]
- Korobeynikov, S.M.; Bychkov, A.L.; Chimitova, E.V.; Demin, V.A. Statistical analysis of partial discharge characteristics in transformer oil at the “point-plane” electrode at alternating voltage. Probl. Reg. Energ. 2017, 2, 36–44. [Google Scholar]
- Florkowski, M.; Florkowska, B.; Furgal, J.; Zydron, P. Impact of high voltage harmonics on interpretation of partial discharge patterns. IEEE Trans. Dielectr. Electr. Insul. 2013, 20, 2009–2016. [Google Scholar] [CrossRef]
- Deng, J.; Wang, M.; Zhou, Y.; Zhou, Z.; Zhang, Y.; Zhang, L.; Liu, X. Partial discharge characteristics of uniform gap in oil-impregnated paper insulation under switching impulse voltage. IEEE Trans. Dielectr. Electr. Insul. 2017, 23, 3584–3592. [Google Scholar] [CrossRef]
- Tang, J.; Wang, J.; Li, J.; Tan, Z. Statistical parameter method for PD pattern recognition. High Volt. Eng. 2002, 28, 4–6. [Google Scholar]
- Gulski, E.; Kreuger, F.H. Computer aided recognition of discharge sources. IEEE Trans. Dielectr. Electr. Insul. 1992, 27, 82–92. [Google Scholar] [CrossRef]
- Candela, R.; Mirelli, G.; Schifani, R. PD recognition by means of statistical and fractal parameters and a neural network. IEEE Trans. Dielectr. Electr. Insul. 2000, 7, 87–94. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhou, Z.; Zhang, L.; Zhang, Y.; Mo, Y.; Sun, J. Characterization and comprehension of corona partial discharge in air under power frequency to very low frequency voltage. Plasma Sci. Technol. 2017, 20, 054016. [Google Scholar] [CrossRef]
- Liao, R.; Duan, L.; Wang, K.; Yang, L.; Yuan, L.; Guo, C. Partial discharge characteristics of point-plane model in oil-paper insulation under AC voltage. High Volt. Eng. 2014, 40, 648–654. [Google Scholar]
- Beroual, A.; Zahn, M.; Badent, A.; Kist, K.; Schwabhe, A.J.; Yamashitica, H.; Yamashitica, K.; Danikas, M.; Chadband, W.G.; Torshin, Y. Propagation and structure of streamers in liquid dielectrics. IEEE Electr. Insul. Mag. 1998, 14, 6–17. [Google Scholar] [CrossRef]
- Liang, X.; Zhou, Y.; Zeng, R. High Voltage Engineering, 3rd ed.; Tsinghua University Press: Beijing, China, 2015. [Google Scholar]
- Feng, C.; Ma, X. Introduction of Engineering Electromagnetic Field; Higher Education Press: Beijing, China, 2000. [Google Scholar]
- Schmidt, W.F. Electronic conduction processes in dielectric liquid. IEEE Trans. Electr. Insul. 1984, EI-19, 389–418. [Google Scholar] [CrossRef]
- Borsi, H.; Gockenbach, E.; Schroder, U.; Schilier, G. Contribution to the clarification of partial discharge behaviour in insulating liquids using the schlieren technique. Int. Trans. Electr. Energy Syst. 1991, 1, 271–279. [Google Scholar] [CrossRef]
- Velasco, J.; Frascella, R.; Albarracín, R.; Burgos, J.C.; Dong, M.; Ren, M.; Yang, L. Comparison of positive streamers in liquid dielectrics with and without nanoparticles simulated with finite-element software. Energies 2018, 11, 361. [Google Scholar] [CrossRef]
















| Discharge Pattern | Symbol | Characteristic Parameters |
|---|---|---|
| Phase distribution of maximum magnitude of discharge | qmax–φ | Sk+, Sk−, Ku+, Ku−, Asy, Cc |
| Phase distribution of mean magnitude of discharge | qmean–φ | Sk+, Sk−, Ku+, Ku−, Asy, Cc |
| Phase distribution of numbers of discharge | n–φ | Sk+, Sk−, Ku+, Ku−, Asy, Cc |
| Number distribution of discharge magnitude | n–q | Sk, Ku |
| Frequency | 50 Hz | 30 Hz | 10 Hz | 5 Hz | 1 Hz | 0.1 Hz |
|---|---|---|---|---|---|---|
| Insulating oil | 2.182 | 2.183 | 2.197 | 2.230 | 2.365 | 3.782 |
| Oil-paper | 4.566 | 4.574 | 4.596 | 4.616 | 4.715 | 5.137 |
| Ratio of dielectric constant | 2.0926 | 2.0953 | 2.0919 | 2.0700 | 1.9937 | 1.3581 |
| Frequency | 50 Hz | 30 Hz | 10 Hz | 5 Hz | 1 Hz | 0.1 Hz |
|---|---|---|---|---|---|---|
| Electric field intensity (kV/mm) | 25.4078 | 25.4140 | 25.4068 | 25.3592 | 25.1886 | 23.2400 |
| Deviation ratio to 50 Hz | - | +0.0247% | −0.0038% | −0.1911% | −0.8622% | −8.5297% |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Z.; Zhou, Y.; Huang, X.; Zhang, Y.; Wang, M.; Guo, S. Feature Extraction and Comprehension of Partial Discharge Characteristics in Transformer Oil from Rated AC Frequency to Very Low Frequency. Energies 2018, 11, 1702. https://doi.org/10.3390/en11071702
Zhou Z, Zhou Y, Huang X, Zhang Y, Wang M, Guo S. Feature Extraction and Comprehension of Partial Discharge Characteristics in Transformer Oil from Rated AC Frequency to Very Low Frequency. Energies. 2018; 11(7):1702. https://doi.org/10.3390/en11071702
Chicago/Turabian StyleZhou, Zhongliu, Yuanxiang Zhou, Xin Huang, Yunxiao Zhang, Mingyuan Wang, and Shaowei Guo. 2018. "Feature Extraction and Comprehension of Partial Discharge Characteristics in Transformer Oil from Rated AC Frequency to Very Low Frequency" Energies 11, no. 7: 1702. https://doi.org/10.3390/en11071702
APA StyleZhou, Z., Zhou, Y., Huang, X., Zhang, Y., Wang, M., & Guo, S. (2018). Feature Extraction and Comprehension of Partial Discharge Characteristics in Transformer Oil from Rated AC Frequency to Very Low Frequency. Energies, 11(7), 1702. https://doi.org/10.3390/en11071702





