Development of an Electrostatic Precipitator with Porous Carbon Electrodes to Collect Carbon Particles
Abstract
1. Introduction
2. Calculation of the Corona Discharge Model
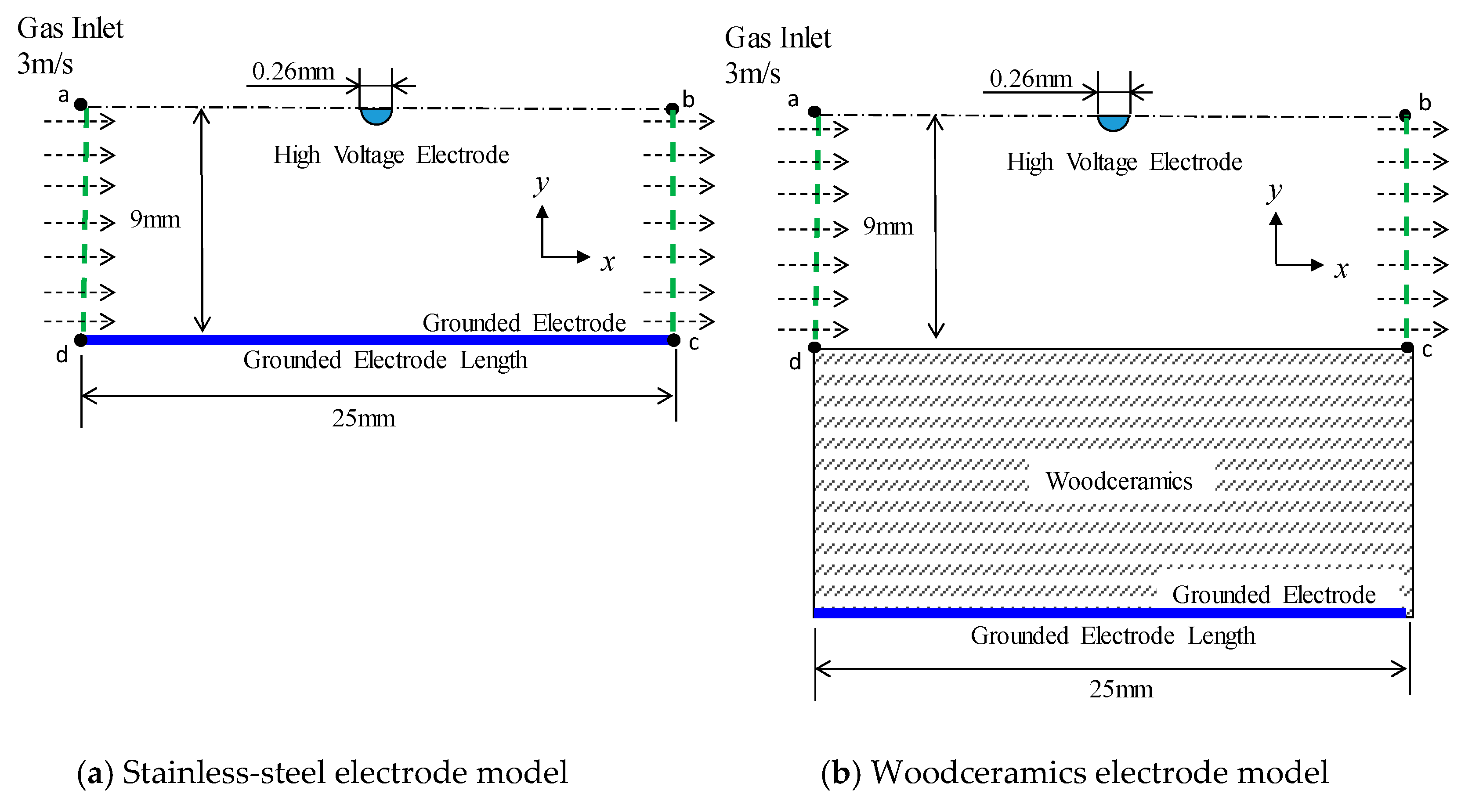
2.1. Calculation Model
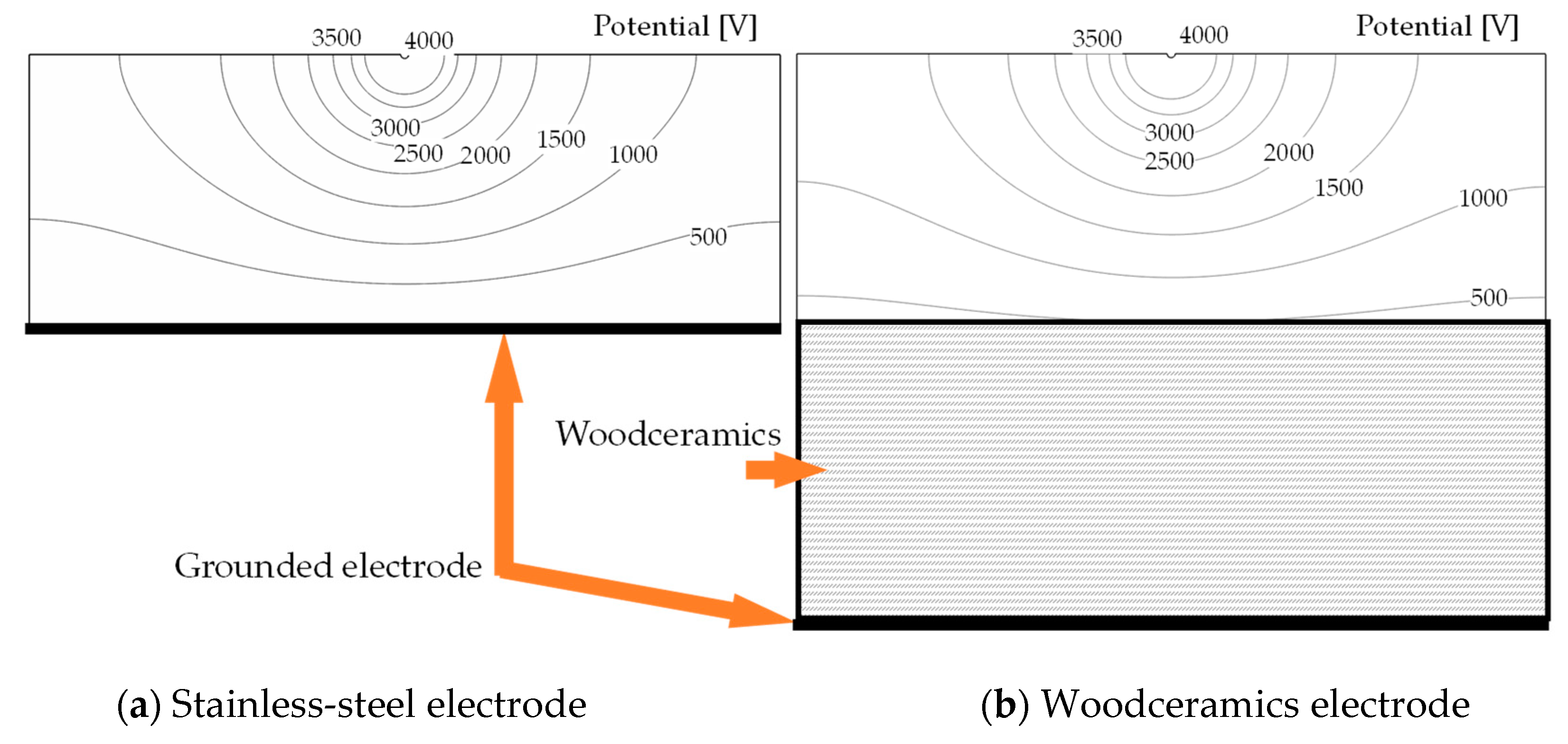
2.2. Numerical Results
3. Experimental Methods
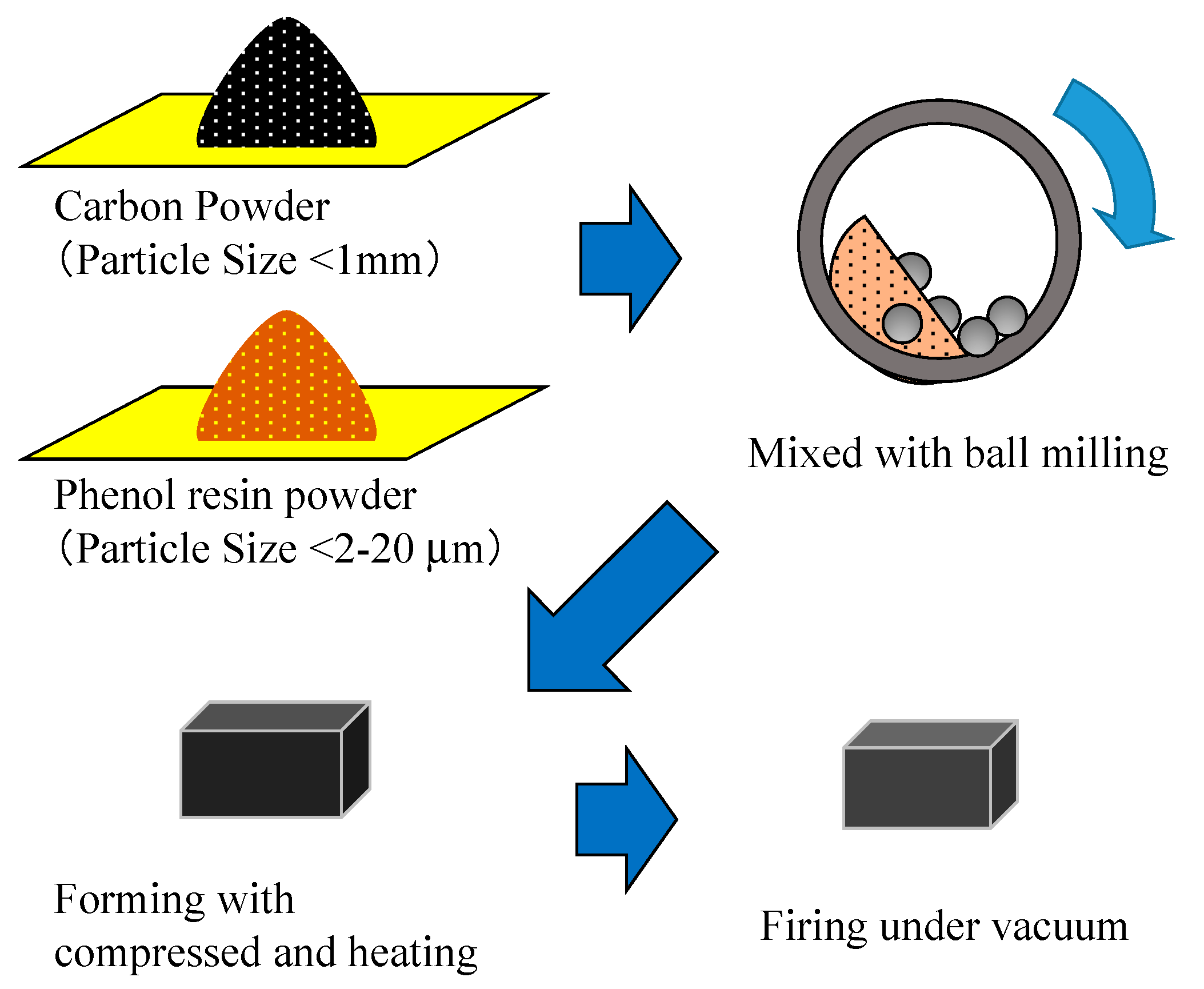
3.1. Woodceramics
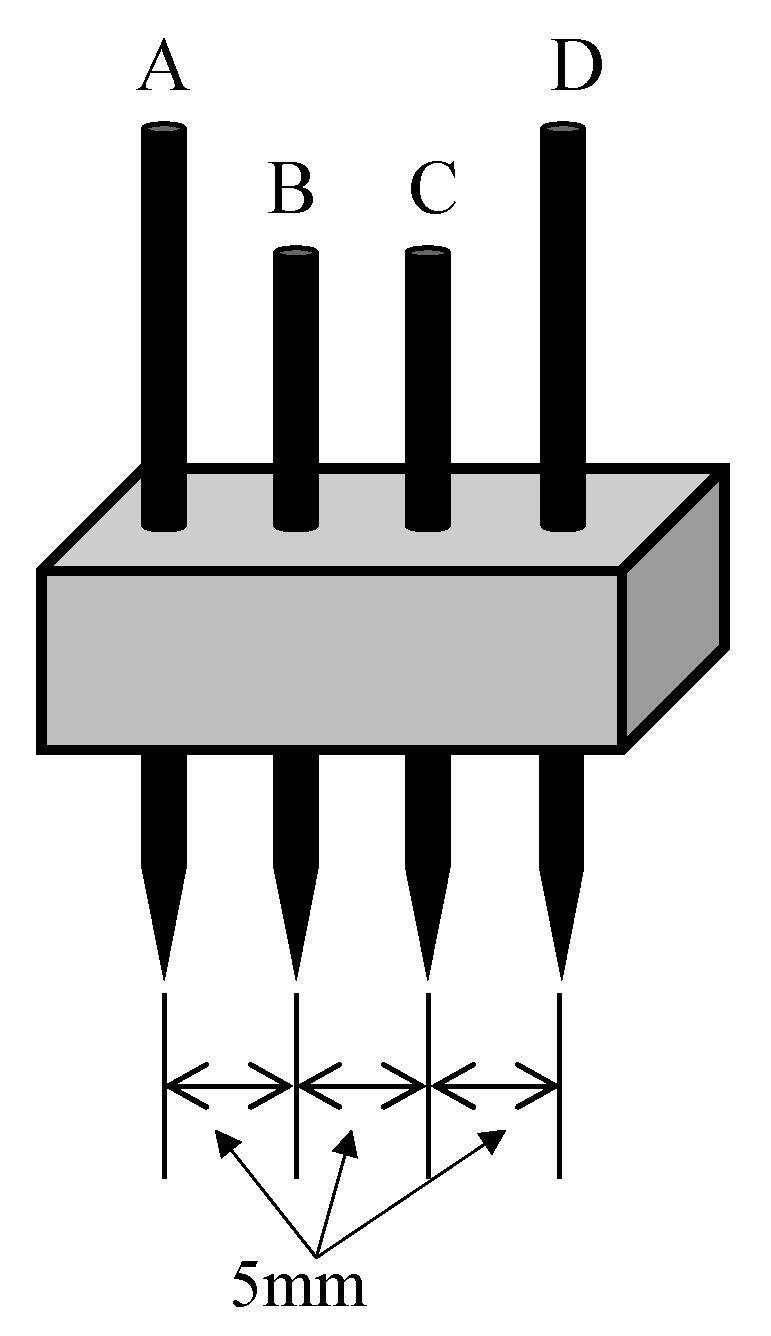
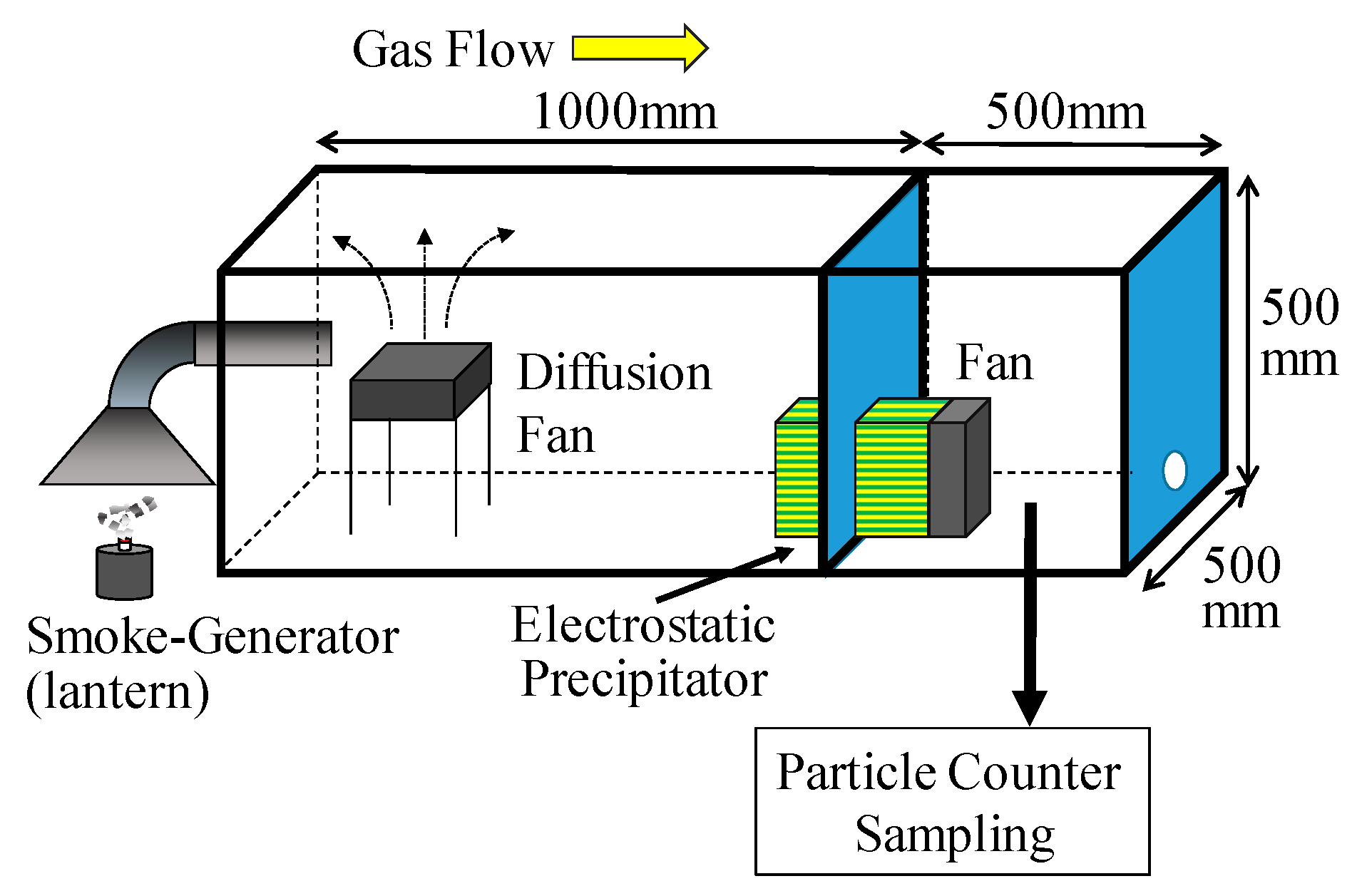
3.2. Experimental Setup and Conditions
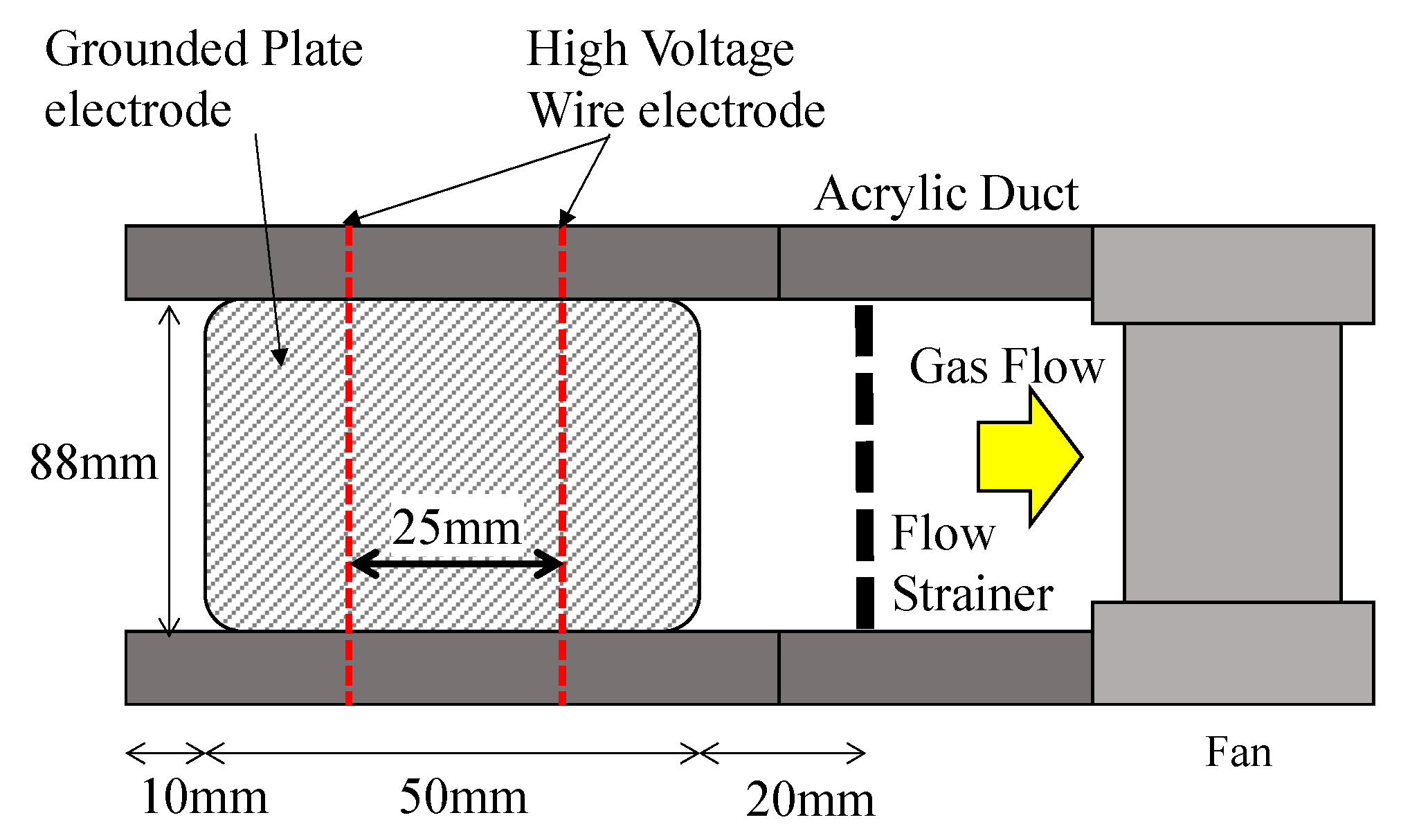
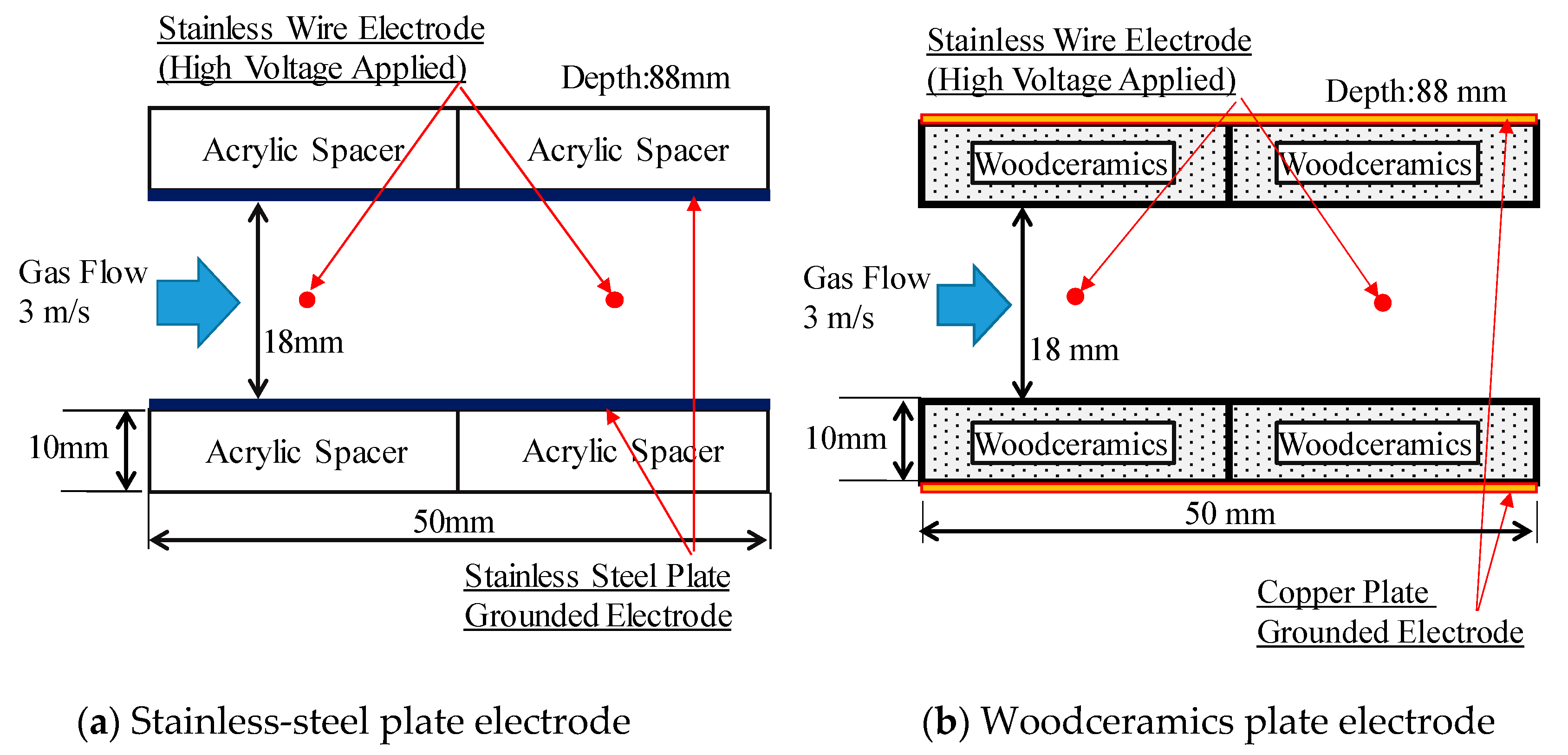
3.3. Electrostatic Precipitator
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Pajdowski, P.; Puchałka, B. The Process of Diesel Particulate Filter Regeneration under Real Driving Conditions. IOP Conf. Ser. Earth Environ. Sci. 2019, 214, 012114. [Google Scholar] [CrossRef]
- Godish, T. Indoor Air Pollution Control, 3rd ed.; Lewis Publishers: Chelsea, MI, USA, 1991; pp. 247–263. [Google Scholar]
- White, H.J. Industrial Electrostatic Precipitation; Addison-Wesley Publishing: Reading, MA, USA, 1963; pp. 319–329. [Google Scholar]
- Takahashi, T.; Zukeran, A.; Ehara, Y.; Ito, T.; Takamatsu, T.; Kawakami, H. Influence of Re-entrainment Phenomena on Particle Deposit in Electrostatic Precipitator. IEEJ Trans. Fundam. Mater. 1999, 119, 254–260. [Google Scholar] [CrossRef][Green Version]
- Yamamoto, T.; Abe, T.; Mimura, T.; Otsuka, N.; Ito, Y.; Ehara, Y.; Zukeran, A. Electrohydrodynamically Assisted Electrostatic Precipitator for the Collection of Low-Resistivity Dust. IEEE Trans. Ind. Appl. 2009, 45, 2178–2184. [Google Scholar] [CrossRef]
- Masuda, S.; Moon, J.-D. Electrostatic Precipitation of Carbon Soot from Diesel Engine Exhaust. IEEE Trans. Ind. Appl. 1983, 19, 1104–1111. [Google Scholar] [CrossRef]
- Kawada, Y.; Jindai, W.; Zukeran, A.; Ehara, Y.; Ito, T.; Kawakami, H.; Takahashi, T. Influence of Lyphophile on Preventing Re-entrainment in Spraying Surfactant Type Electrostatic Precipitator. In Proceedings of the 7th International Conference of Electrostatic Precipitation, Kyonju, Korea, 20–25 September 1998. [Google Scholar]
- Kubo, T.; Kawada, Y.; Takahashi, T.; Ehara, Y.; Ito, T.; Zukeran, A.; Takamatsu, T. The relation between shape of particles and collection efficiency by electrostatic precipitators. J. Aerosol Sci. 2000, 31, 452–453. [Google Scholar] [CrossRef]
- Takahashi, T.; Kawada, Y.; Zukeran, A.; Ehara, Y.; Ito, T. Inhibitory effect of coating electrode with dielectric sheets on re-entrainment in electrostatic precipitator. J. Aerosol Sci. 1998, 29, S485–S486. [Google Scholar] [CrossRef]
- Shibata, K.; Kasai, K.; Okabe, T.; Saito, K. Development of Porous Carbon Material “Woodceramics”—Electrical Properties with in Low-Temperature Region. J. Soc. Sci. Jpn. 1995, 44, 284–287. (In Japanese) [Google Scholar] [CrossRef]
- Kawada, Y.; Shimizu, H. C-H Bonds and Phenol Resin Contents in Woodceramics under Fabrication. In Proceedings of the 25th Annual Meeting of MRS-J, Yokohama, Japan, 8–10 December 2015. [Google Scholar]
- Kawada, Y.; Shimizu, H.; Ohkawa, M.; Mori, S.; Kakishita, K. Elect of Woodceramics Grounded Electrode on Electrostatic Precipitation with Positive Corona Discharge. Trans. Mater. Res. Soc. Jpn. 2018, 43, 187–190. [Google Scholar] [CrossRef]
- Kawada, Y.; Shimizu, H.; Ohkawa, M.; Mori, S.; Kakishita, K. A Study on Woodceramics Collector in Electrostatic Precipitator. In Proceedings of the Annual Meeting of IESJ, Osaka, Japan, 11–12 September 2017; pp. 27–30. (In Japanese). [Google Scholar]
- Takeuchi, N. Simulation of Ionic Wind Induced by Corona Discharge Using COMSOL Multiphysics. J. Inst. Electrost. Jpn. 2016, 40, 168–171. (In Japanese) [Google Scholar]
- Kawada, Y.; Shimizu, H.; Zukeran, A. Numerical Study of the Suitable Precharger Grounded Electrode Length in Two-Stage-Type Electrostatic Precipitators. IEEE Trans. Ind. Appl. 2019, 55, 833–839. [Google Scholar] [CrossRef]
- The Institute of Electrostatics Japan (Ed.) Handbook of Electrostatics; Ohmsha, Ltd.: Tokyo, Japan, 1980. (In Japanese) [Google Scholar]
- Chang, J.S.; Kelly, A.J.; Crowley, J.M. (Eds.) Handbook of Electrostatic Processes; Marcel Dekker, Inc.: New York, NY, USA, 1995. [Google Scholar]
- Waker, P.L., Jr.; Thrower, P.A. (Eds.) Chemistry and Physics of Carbon, Volume 16; Marcel Dekker, Inc.: New York, NY, USA, 1981; p. 141. [Google Scholar]
- Okabe, T. (Ed.) Woodceramics; Uchida Rokakuho Publishing: Tokyo, Japan, 1996; p. 65. (In Japanese) [Google Scholar]





| In Air | Woodceramics | |
|---|---|---|
| Mobility (m2/V·s) | 2.34 × 10−4 | 1.00 |
| Diffusion constant (m2/s) | 2.89 × 10−6 | 0.256 |
| Relative permittivity | 1.00 | 5.68 |
| Gas flow (m/s) | - | 0 |
| Resistivity (Ω·cm) | ||
|---|---|---|
| Stainless-Steel | Woodceramics | |
| Average | 0.0218 | 96.2 |
| Max. | 0.032 | 137 |
| Min. | 0.0143 | 67.3 |
| Particle Size Range (μm) | Room Air (Num/L) | Target Gas (Num/L) |
|---|---|---|
| 0.3–0.5 | 1.6 × 104 | 1.2 × 105 |
| 0.5–1.0 | 9.6 × 102 | 5.8 × 103 |
| 1.0–2.0 | 3.0 × 10 | 5.8 × 102 |
| 2.0–5.0 | 0 | 1.7 × 102 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kawada, Y.; Shimizu, H. Development of an Electrostatic Precipitator with Porous Carbon Electrodes to Collect Carbon Particles. Energies 2019, 12, 2805. https://doi.org/10.3390/en12142805
Kawada Y, Shimizu H. Development of an Electrostatic Precipitator with Porous Carbon Electrodes to Collect Carbon Particles. Energies. 2019; 12(14):2805. https://doi.org/10.3390/en12142805
Chicago/Turabian StyleKawada, Yoshihiro, and Hirotaka Shimizu. 2019. "Development of an Electrostatic Precipitator with Porous Carbon Electrodes to Collect Carbon Particles" Energies 12, no. 14: 2805. https://doi.org/10.3390/en12142805
APA StyleKawada, Y., & Shimizu, H. (2019). Development of an Electrostatic Precipitator with Porous Carbon Electrodes to Collect Carbon Particles. Energies, 12(14), 2805. https://doi.org/10.3390/en12142805




