1. Introduction
As one of the most widely utilized devices for heat transfer in industry, heat exchangers play a significant role in many fields, including chemical engineering, petroleum processing, power systems, and waste heat recovery. The shell and tube heat exchangers (STHXs) occupy more than 35% to 40% among various types owing to its simple manufacturing, high pressure-resistant ability, and low cost [
1,
2]. Baffles within the shell side are employed to support tubes, prevent vibration, guide flow direction, and increase fluid velocity. Thus, the structures of baffles exert an important influence on the thermal-hydraulic performance of the STHXs. The conventional shell and tube heat exchanger with segmental baffles (STHX-SG) provides a zigzag flow in the shell side, which have many advantages, such as high heat transfer performance, versatile adaptability, and simple maintenance. However, there still exist some drawbacks with the STHX-SG, for instance, high pressure loss, large flow dead zones, easy fouling, and flow-induced vibration. Therefore, numerous studies are focused on improving the comprehensive performance of the STHXs by overcoming the weaknesses above.
Various measures have been successfully applied to augment the heat transfer on the tube side, including shaped tubes, tube inserts, and turbulators [
3,
4,
5,
6,
7,
8,
9,
10]. While for the shell side, shaped tubes are also adopted to produce secondary flow and vortices with the fluid around tubes. Shirvan et al. [
11] proposed the cosine wave tube to enhance the shell side performance in the STHX-SG, and optimized the tube geometry to maximize the thermal performance factor. Liu et al. [
12] investigated numerically the shell side performance of the rod baffle heat exchangers with spirally corrugated tubes (RBHXsSCT). It was found that the tubes can augment the comprehensive performance of the RBHXsSCT when compared to the rod baffle heat exchangers with plain tubes (RBHXs). He et al. [
13] carried out numerical simulations on the RBHXs with elliptic tubes and circular tubes, respectively. Results showed that the former one is an effective structure to improve the heat transfer rate of the non-Newtonian fluid. Yang et al. [
14] reported a novel RBHX with eccentric spiral tubes, and analyzed the thermal-hydraulic characteristics both in shell side and tube side. It was concluded that the new structure has better performance on the tube side and slightly worse one on the shell side than the RBHX with plain tubes.
On the other hand, extensive attention is focused on modifying the baffle configurations to change the flow manner of shell-side fluid. The STHXs with helical baffles, first introduced by Lutcha and Nemcansky [
15] and industrialized by ABB Lummus [
16], are one of the popular research objects. Wang et al. [
17,
18,
19] designed the STHX with continuous helical baffles, and it can generate ideal helical flow to enhance the shell side performance. However, due to the manufacture difficulties and high cost brought by the complex structures, their industrial applications are still restricted. Therefore, discontinuous helical baffles are developed gradually [
20,
21,
22]. The longitudinal flow pattern on the shell side is also investigated by many researchers. Wang et al. [
23,
24] designed a new structure named double shell-pass RBHX (DS-RBHX), and the shell side is divided into two parts with a sleeve. Both the numerical and experimental studies revealed that the DS-RBHX performs better in flow and heat transfer than the single shell-pass RBHX. The STHXs with two different clamping type anti-vibration baffles were proposed by Yu et al. [
25], and it is concluded that the new types of baffles are effective against the flow-induced vibration at the expense of lower comprehensive performance. Lei et al. [
26] designed the novel STHXs with two types of louver baffles, which can produce oblique flow to reduce dead regions and increase local heat transfer. The round orifice plate baffle is a special type of supporting structure to generate longitudinal flow, among which the trefoil-hole baffles and quatrefoil-hole baffles are mostly used because of the high reliability in anti-vibration and anti-fouling. You et al. [
27] reported a research on the shell side performance of the STHX with trefoil-hole baffles. They found that this kind of baffles can produce high-speed flush against tube walls, which intensively strengthens the flow resistance and the heat transfer coefficient. Maakoul et al. [
28] numerically compared shell side performance among STHXs with trefoil-hole, helical, and segmental baffles, respectively. The results indicated that the trefoil-hole baffles augment the heat transfer with large pressure loss, and using helical baffles can achieve the highest comprehensive performance. Ma et al. [
29] found that the shell side performance of the STHXs with trefoil-hole baffles is superior to that with quatrefoil-hole baffles. Meanwhile, the larger opening height can reduce the flow resistance, and thus display higher comprehensive performance for both STHXs.
The inclined baffles are commonly used to enhance heat transfer in tubes or channels, and their utilization in heat exchangers is proposed and studied recently [
30,
31,
32]. Raj et al. [
33] preliminarily investigated the impact of inclination angle on the thermal-hydraulic characteristics of the STHXs with inclined baffles. They concluded that the heat transfer performance changes slightly with the increasing inclination angle while the pressure drop decreases obviously, and 20° baffle inclination angle is the optimal parameter within their research ranges. Kumaresan et al. [
34] found that the combination structure of 30% baffle cut and 35° baffle inclination angle has the higher heat transfer rate with minimum pressure drop.
The CFD method, which can provide detailed flow and temperature distributions along with accurate results, has become a prevailing strategy to predict the behaviors of STHXs. There are four main models, i.e., the unit model, the periodic model, the porous model, and the whole model, to investigate the shell side performance of STHXs. Yang et al. [
35] compared these models on different aspects, and summarized a method to select the most proper model in practical applications. At the meantime, the optimization algorithms with the surrogate modeling technique have shown the effectiveness to determine the optimum structures in many applications. Wang et al. [
36] achieved the best solutions for the STHX with staggered baffles by adopting the artificial neural network (ANN) and multi-objective genetic algorithm (MOGA). Wen et al. [
37] optimized the structure of the STHXs with helical baffles by adopting the response surface methodology and MOGA. Zheng et al. [
38] achieved the optimal Pareto front for the tube fitted vortex rod inserts by implementing ANN with MOGA.
However, few studies on shell side performance of the STHXs with inclined baffles have been reported. Further, the overall investigation and structure optimization on the STHXs combined with inclined baffles and trefoil-hole baffles has not been carried out. Therefore, considering the effects on decreasing pressure drop of inclined baffles and diminishing dead regions of trefoil-hole baffles, the novel structure, namely the STHX with inclined trefoil-hole baffles (STHX-IT), is proposed in this work. Numerical simulations are performed to investigate the flow and heat transfer characteristics of the STHX-IT, and the STHX-SG is selected as a reference. Meanwhile, the parametric analysis is carried out to discuss the effects of inclination angle θ, trefoil-hole number n, and baffle cut δ on the shell side performance. Finally, the combination of CFD, ANN, and MOGA are used to explore the optimal configurations for the STHX-IT.
2. Physical Models
In this study, the numerical simulations on the shell side of heat exchangers are conducted. According to our previous work by Yang et al. [
35], the whole model can provide the most accurate results for the shell-side simulations, so it is adopted in this work.
Figure 1 presents the geometric structures of the STHX-SG and STHX-IT. These heat exchangers have the same dimension for the shell, inlet, outlet, and tubes. Thirty-seven heat transfer tubes with the triangle arrangement are deployed in these models. Meanwhile, both STHX-SG and STHX-IT have seven baffles with the same baffle cut and baffle spacing. The detailed parameters of the STHX-IT model are listed in
Table 1. The main difference between these two models lies in the baffles. The baffles of the STHX-SG are placed perpendicular to the central axis of the cylindrical shell and have no trefoil-holes, while it is quite distinct for the STHX-IT. There are three key configuration parameters for the STHX-IT, which are inclination angle
θ, trefoil-hole number
n, and baffle cut
δ, respectively. As illustrated in
Figure 2,
θ is defined as the angle between the direction of baffle plane and the vertical plane of the shell central axis, and
δ is defined as the ratio of the baffle cut height to the inner diameter of the shell, while
ht denotes the trefoil opening height.
Figure 3 depicts the diverse trefoil-hole locations of baffles with different values of
n. The STHX-SG model with the baffle cut δ of 25% and the STHX-IT model with inclination angle
θ of 10°, trefoil-hole number
n of 5, baffle cut
δ of 25% are investigated and compared initially.
5. Conclusions
In this work, a novel shell and tube heat exchanger with inclined trefoil-hole baffles (STHX-IT) is proposed and numerically investigated to examine the heat transfer performance and pressure drop. The comparison studies between the STHX-IT and STHX-SG are also conducted. The parametric analysis of the inclination angle θ, the trefoil-hole number n, and the baffle cut δ, is carried out for the STHX-IT. The multi-objective optimization of the design variables is implemented by adopting the combination of CFD, ANN, and MOGA, whose aim is to obtain the maximum heat transfer rate with the minimum pressure drop. The major conclusions are outlined as follows:
(1) The inclined trefoil-hole baffles of the STHX-IT can generate oblique flow in the shell side, and uniform flow patterns and temperature distributions are observed through the numerical results.
(2) Compared with the conventional STHX-SG, the STHX-IT has lower heat transfer rate with more significant reduction in the pressure drop, thus possessing superior comprehensive performance characterized by the heat transfer coefficient per pressure drop.
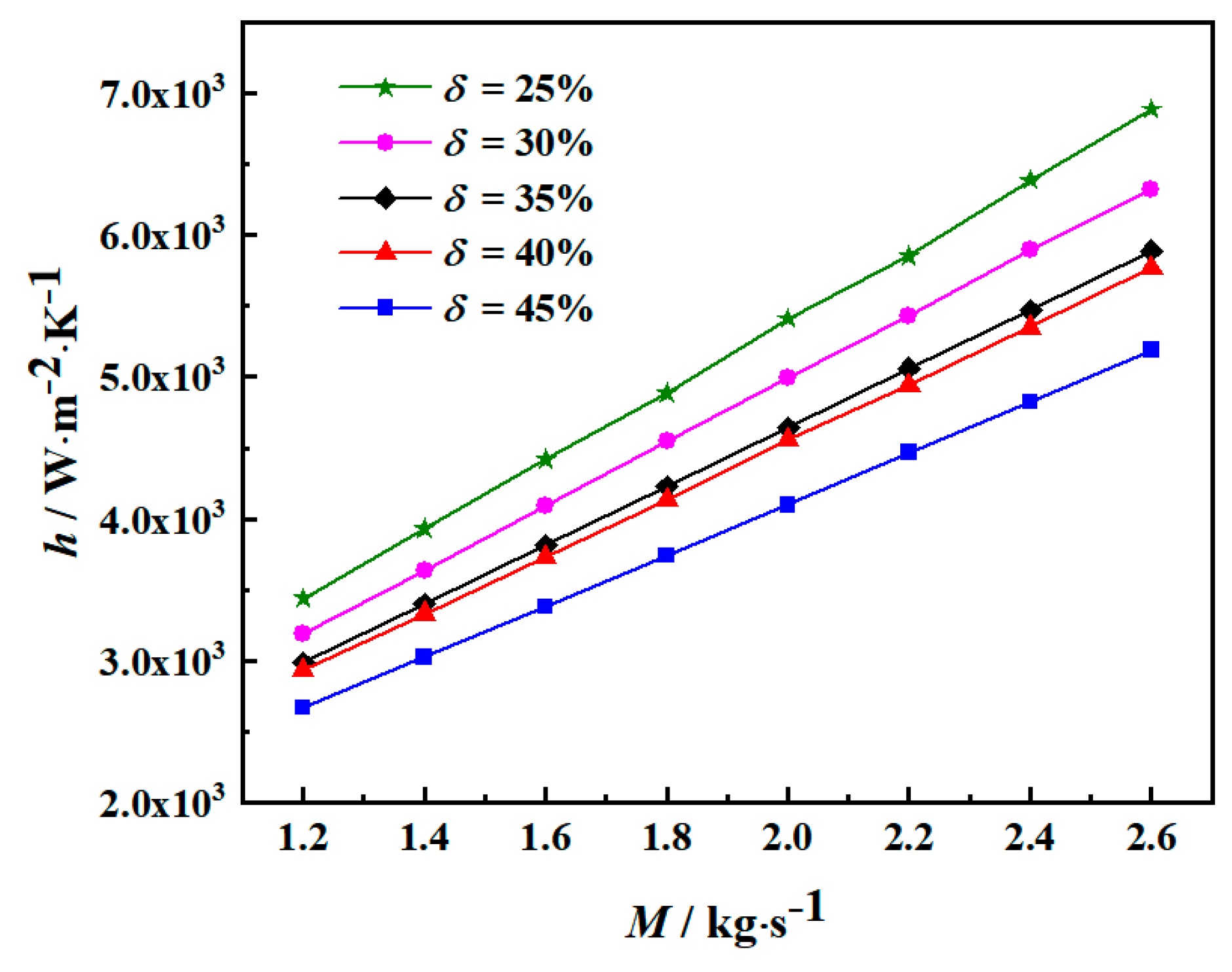
(3) According to the parametric studies, the heat transfer coefficient and pressure drop simultaneously decrease slightly with the inclination angle θ growing, and the comprehensive performance is approximately equal. The trefoil-hole number n and the baffle cut δ have more notable influence on the shell side performance. In detail, the larger trefoil-hole number and larger baffle cut both reduce the heat transfer rate and pressure drop, and bring in better comprehensive performance.
(4) The Pareto front corresponding to the best thermal-hydraulic performance is obtained by multi-objective optimization for the STHX-IT. The design parameters of θ = 5.38°, n = 6, and δ = 43% is chosen as the optimal structure by using the TOPSIS, whose Q/Δp can achieve 2.34 times as much as that of the STHX-SG.
As a final, the new STHX-IT proposed in this work is effective to enhance the comprehensive performance of shell side, which provides a meaningful solution to replace the STHX-SG in some industrial applications. In addition, the suitable combination of structural parameters can achieve optimum performance. According to the above results, small inclination angle, a certain number of trefoil-holes, and reasonable baffle cut should be considered.