A Thermo-Electric Apparatus for Thermal Diffusivity and Thermal Conductivity Measurements
Abstract
:1. Introduction
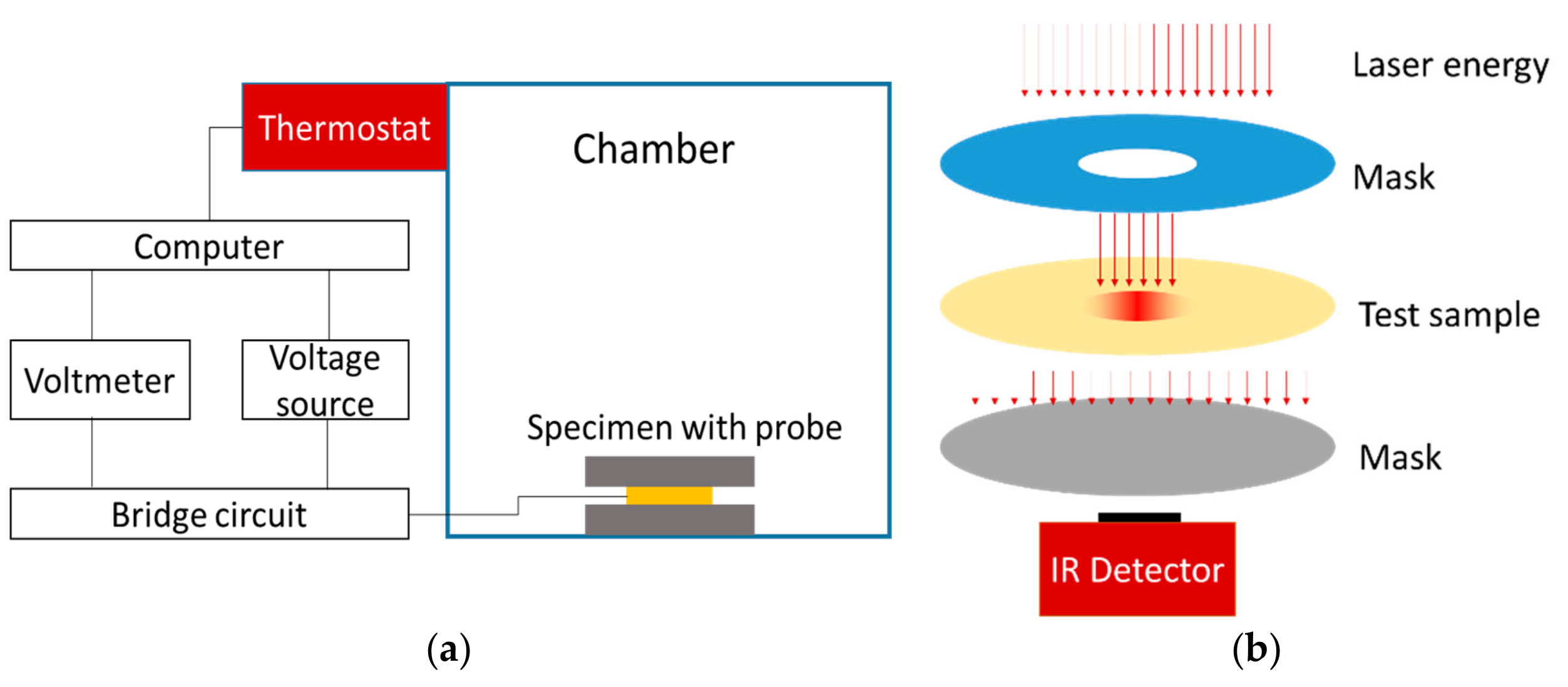
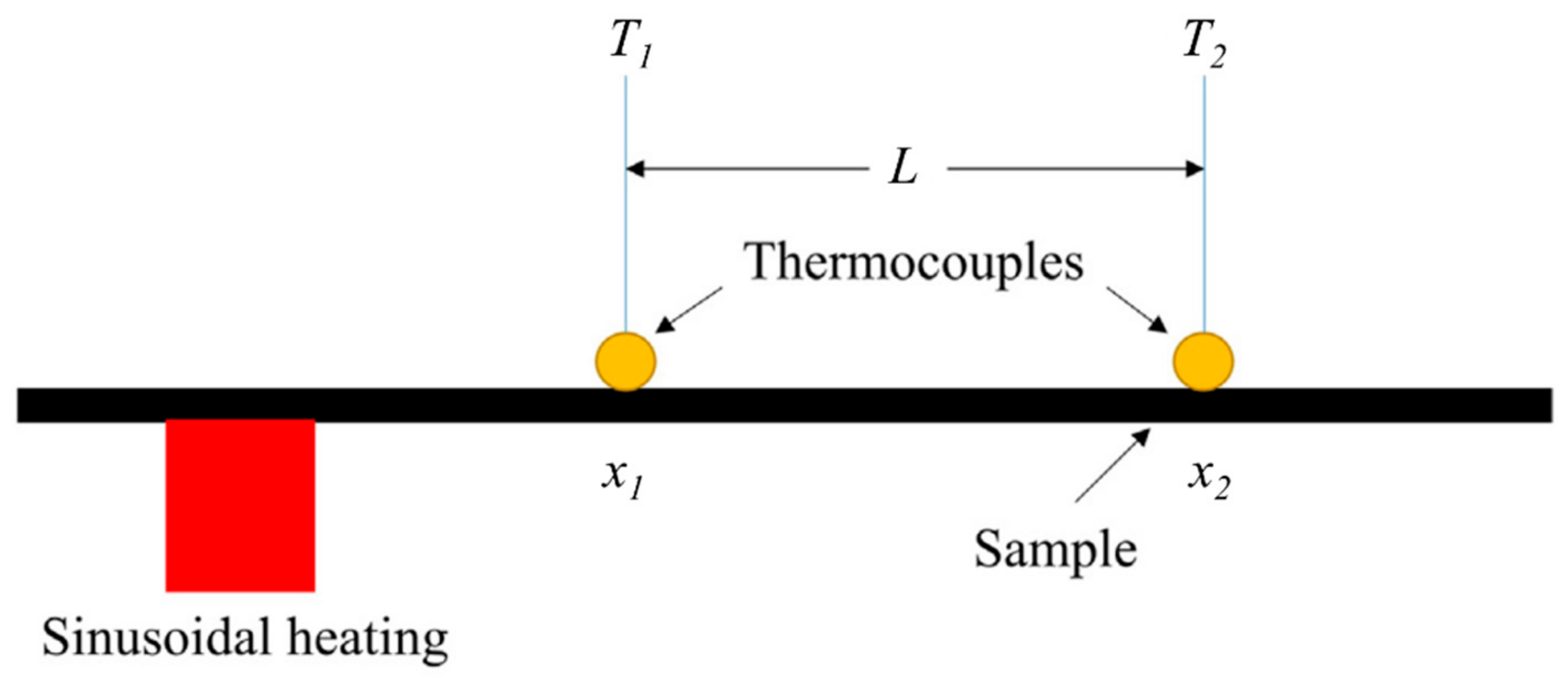
2. Principle and Experimental
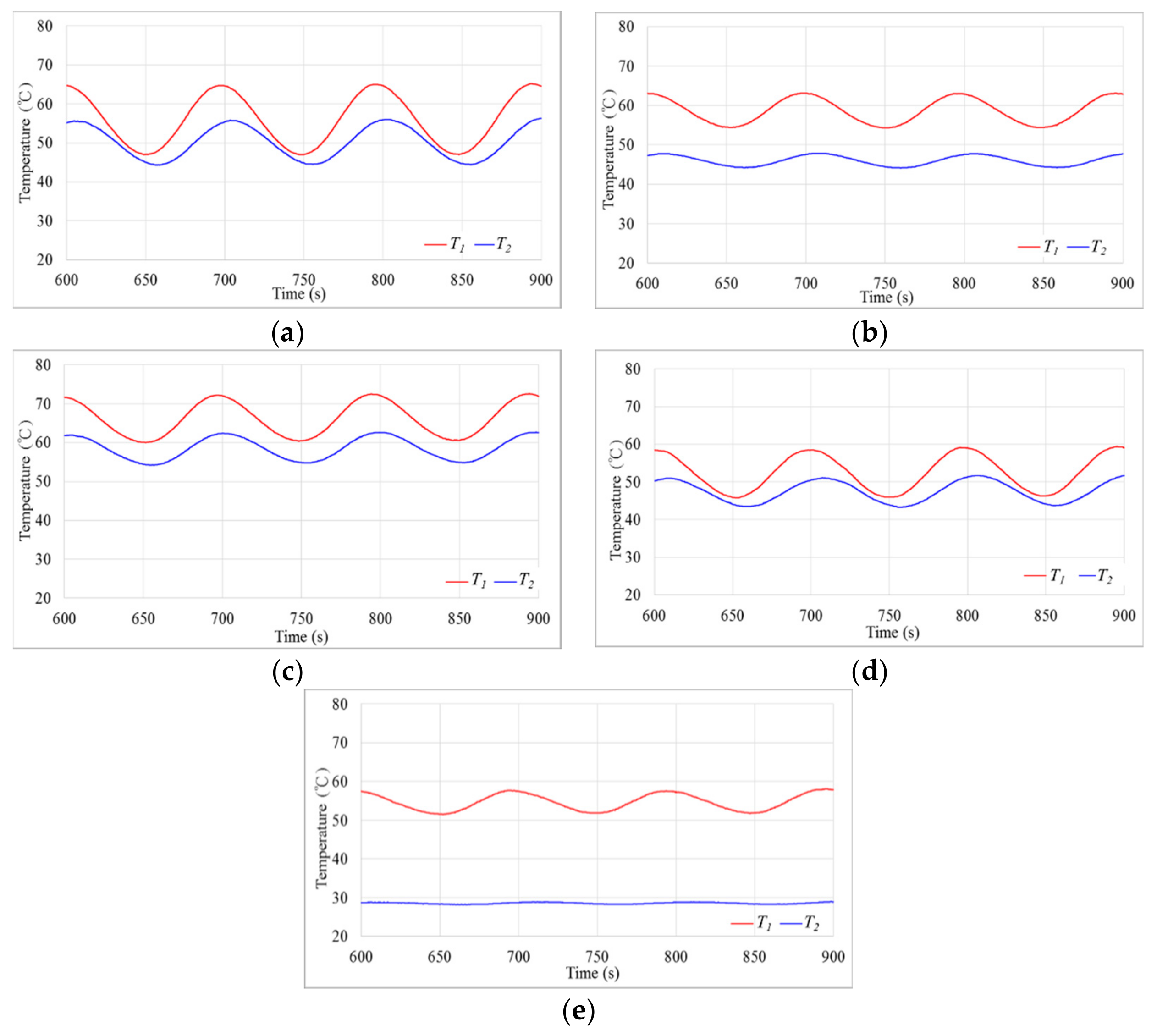
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Angstrom, A.J. A new method of determining the thermal conductivity of solids. Ann. Phys. 1861, 2, 513–530. [Google Scholar]
- Kosky, P.G.; Maylotte, D.H.; Gallo, J.P. Ångström methods applied to simultaneous measurements of thermal diffusivity and heat transfer coefficients: Part 1, theory. Int. Commun. Heat Mass Transf. 1999, 26, 1051–1059. [Google Scholar] [CrossRef]
- Maylotte, D.H.; Kosky, P.G.; Gallo, J.P. Ångström methods applied to simultaneous measurements of thermal diffusivity and heat transfer coefficients: Part 2, experimental. Int. Commun. Heat Mass Transf. 1999, 26, 1061–1068. [Google Scholar] [CrossRef]
- Gustavsson, M.; Karawacki, E.; Gustafsson, S.E. Thermal conductivity, thermal diffusivity, and specific heat of thin samples from transient measurements with hot disk sensors. Rev. Sci. Instrum. 1994, 65, 3856–3859. [Google Scholar] [CrossRef]
- Parker, W.J.; Jenkins, R.J.; Butler, C.P.; Abbott, G.L. Flash method of determining thermal diffusivity, heat capacity, and thermal conductivity. J. Appl. Phys. 1961, 32, 1679–1684. [Google Scholar] [CrossRef]
- Cerceo, M.; Childers, H.M. Thermal diffusivity by electron bombardment heating. J. Appl. Phys. 1963, 34, 1445–1449. [Google Scholar] [CrossRef]
- Topolnicki, J. The application of heat wave in measurement of thermal diffusivity coefficient. Bulletin of the Polish academy of sciences. Tech. Sci. 1984, 32, 415–422. [Google Scholar]
- Lopez-Baeza, E.; De La Rubia, J.; Goldsmid, H.J. Angstrom’s thermal diffusivity method for short samples. J. Phys. D Appl. Phys. 1987, 20, 1156. [Google Scholar] [CrossRef]
- Shinzato, K.; Baba, T. A laser flash apparatus for thermal diffusivity and specific heat capacity measurements. J. Therm. Anal. Calorim. 2001, 64, 413–422. [Google Scholar] [CrossRef]
- Wagoner, G.; Skokova, K.A.; Levan, C.D. Angstrom’s method for thermal property measurements of carbon fibers and composites; The American Carbon Society, Carbon Conference; Wagoner Enterprises, Inc.: Lake Road, OH, USA, 1999. [Google Scholar]
- Zhu, Y. Heat-loss modified Angstrom method for simultaneous measurements of thermal diffusivity and conductivity of graphite sheets: The origins of heat loss in Angstrom method. Int. Commun. Heat Mass Transf. 2016, 92, 784–791. [Google Scholar] [CrossRef]
- Morikawa, J.; Hashimoto, T. New technique for Fourier transform thermal analysis. J. Therm. Anal. Calorim. 2001, 64, 403–412. [Google Scholar] [CrossRef]
- Lytle, A.L. Ångström’s Method of Measuring Thermal Conductivity; Physics Department, The College of Wooster: Wooster, OH, USA, 2000. [Google Scholar]
- Huang, C.; Lin, W.K.; Chen, J.K. Important factors affecting the thermal resistance and thermal diffusivity of vapor chambers. Appl. Therm. Eng. 2017, 126, 1148–1155. [Google Scholar] [CrossRef]
- Visser, E.P.; Versteegen, E.H.; Van Enckevort, W.J. Measurement of thermal diffusion in thin films using a modulated laser technique: Application to chemical-vapor-deposited diamond films. J. Appl. Phys. 1992, 71, 3238–3248. [Google Scholar] [CrossRef]
- Howling, D.H.; Mendoza, E.; Zimmerman, J.E. Preliminary experiments on the temperature-wave method of measuring specific heats of metals at low temperatures. Proceedings of the Royal Society of London. Series A Math. Phys. Sci. 1955, 229, 86–109. [Google Scholar]
- Gallego, N.C.; Edie, D.D.; Nysten, B.; Issi, J.P.; Treleaven, J.W.; Deshpande, G.V. The thermal conductivity of ribbon-shaped carbon fibers. Carbon 2000, 38, 1003–1010. [Google Scholar] [CrossRef]
- Zhang, G.; Liu, C.; Fan, S. Directly measuring of thermal pulse transfer in one-dimensional highly aligned carbon nanotubes. Sci. Rep. 2013, 3, 2549. [Google Scholar] [CrossRef] [PubMed]




| Test Material | |||
|---|---|---|---|
| Red copper (Cu) | 8.96 | 0.39 | 398 |
| Brass (Bs) | 8.21 | 0.38 | 105 |
| Silver (Ag) | 10.49 | 0.23 | 429 |
| Aluminium (AL1050) | 2.68 | 0.91 | 237 |
| Aluminium Nitride (AlN) | 3.26 | 0.74 | 140 |
| Test Material | M [℃] | N [℃] | Δt [s] | α [mm2/s] | K2 |
|---|---|---|---|---|---|
| Red copper (Cu) | 4.8 | 2.5 | 6.0 | 114.97 | 401.76 |
| Brass (Bs) | 3.7 | 1.1 | 12.2 | 30.40 | 94.87 |
| Silver (Ag) | 7.9 | 3.9 | 3.6 | 177.08 | 427.24 |
| Aluminium (AL1050) | 7.4 | 2.7 | 5.0 | 89.27 | 217.70 |
| Aluminium Nitride (AlN) | 3.0 | 1.3 | 9.0 | 59.79 | 144.24 |
| Test Material | (±) Error | Standard Error (%) | ||
|---|---|---|---|---|
| Red copper (Cu) | 398 | 401.76 | 3.76 | 0.94 |
| Brass (Bs) | 105 | 94.87 | 10.13 | 9.65 |
| Silver (Ag) | 429 | 427.24 | 1.76 | 0.41 |
| Aluminium (AL1050) | 237 | 217.70 | 19.30 | 8.14 |
| Aluminium Nitride (AlN) | 140 | 144.24 | 4.24 | 3.03 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, W.-H.; Lin, W.-K.; Tsai, C.-H.; Wu, P.-H.; Wu, S.-K. A Thermo-Electric Apparatus for Thermal Diffusivity and Thermal Conductivity Measurements. Energies 2019, 12, 4238. https://doi.org/10.3390/en12224238
Zhang W-H, Lin W-K, Tsai C-H, Wu P-H, Wu S-K. A Thermo-Electric Apparatus for Thermal Diffusivity and Thermal Conductivity Measurements. Energies. 2019; 12(22):4238. https://doi.org/10.3390/en12224238
Chicago/Turabian StyleZhang, Wen-Hua, Wei-Keng Lin, Ching-Huang Tsai, Pei-Hsun Wu, and Shih-Kuo Wu. 2019. "A Thermo-Electric Apparatus for Thermal Diffusivity and Thermal Conductivity Measurements" Energies 12, no. 22: 4238. https://doi.org/10.3390/en12224238
APA StyleZhang, W.-H., Lin, W.-K., Tsai, C.-H., Wu, P.-H., & Wu, S.-K. (2019). A Thermo-Electric Apparatus for Thermal Diffusivity and Thermal Conductivity Measurements. Energies, 12(22), 4238. https://doi.org/10.3390/en12224238





