Optimal Operation of Battery Storage for a Subscribed Capacity-Based Power Tariff Prosumer—A Norwegian Case Study
Abstract
:1. Introduction
1.1. Optimal Operation of Battery Storage
1.2. The Importance of Considering Battery Degradation
1.3. Objective
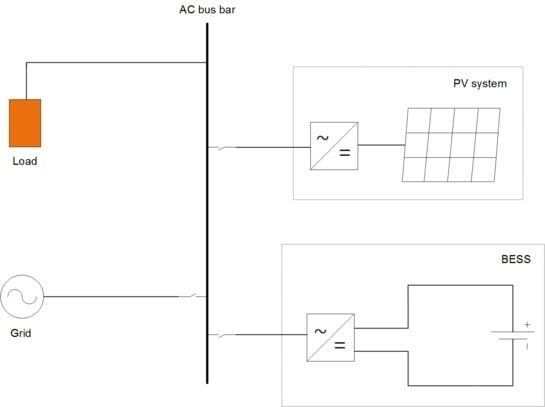
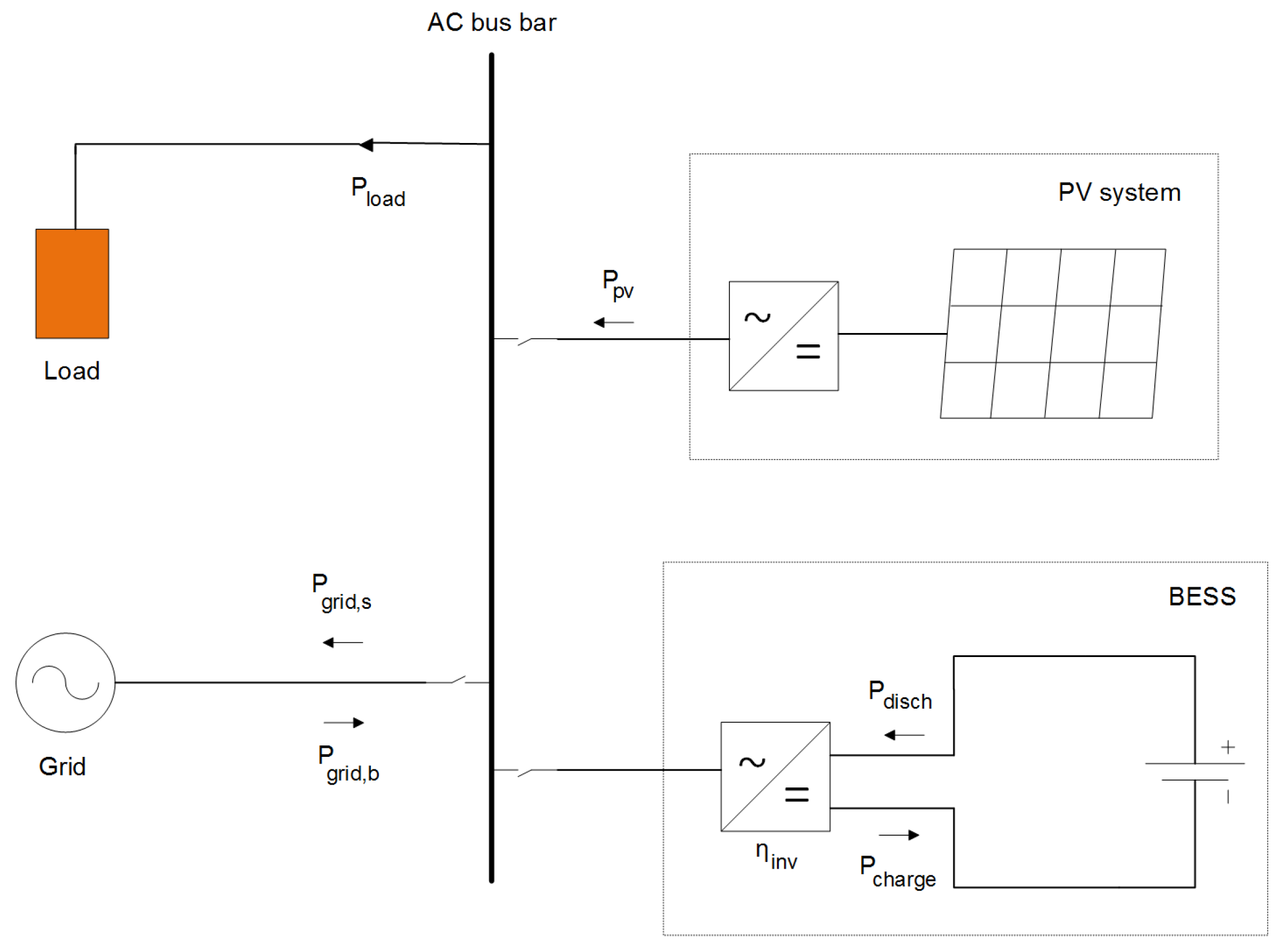
2. Mathematical Model and Problem Formulations
2.1. Power Balance
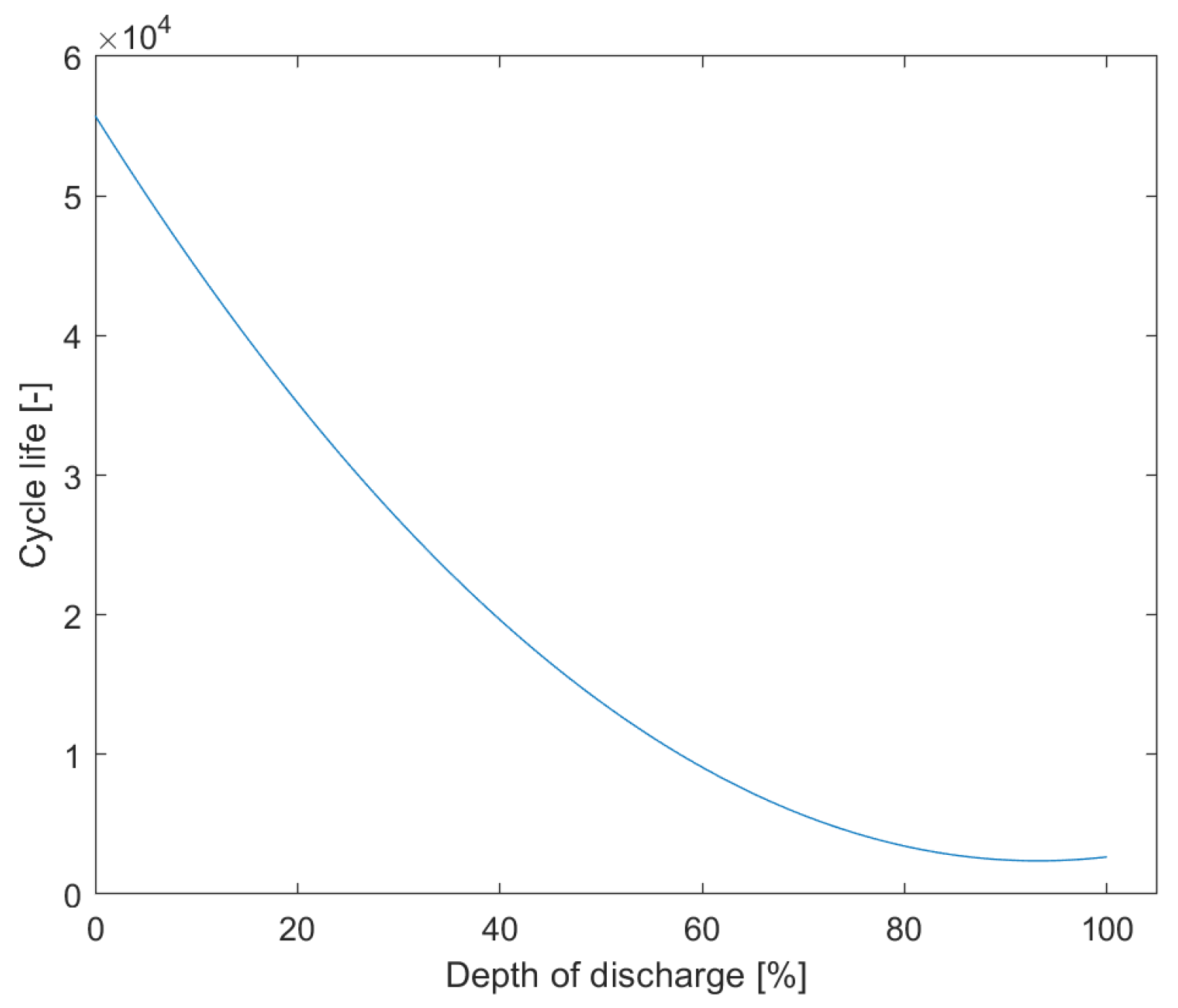
2.2. Battery Degradation
2.3. The Cost of Battery Storage
2.4. The Cost of Energy
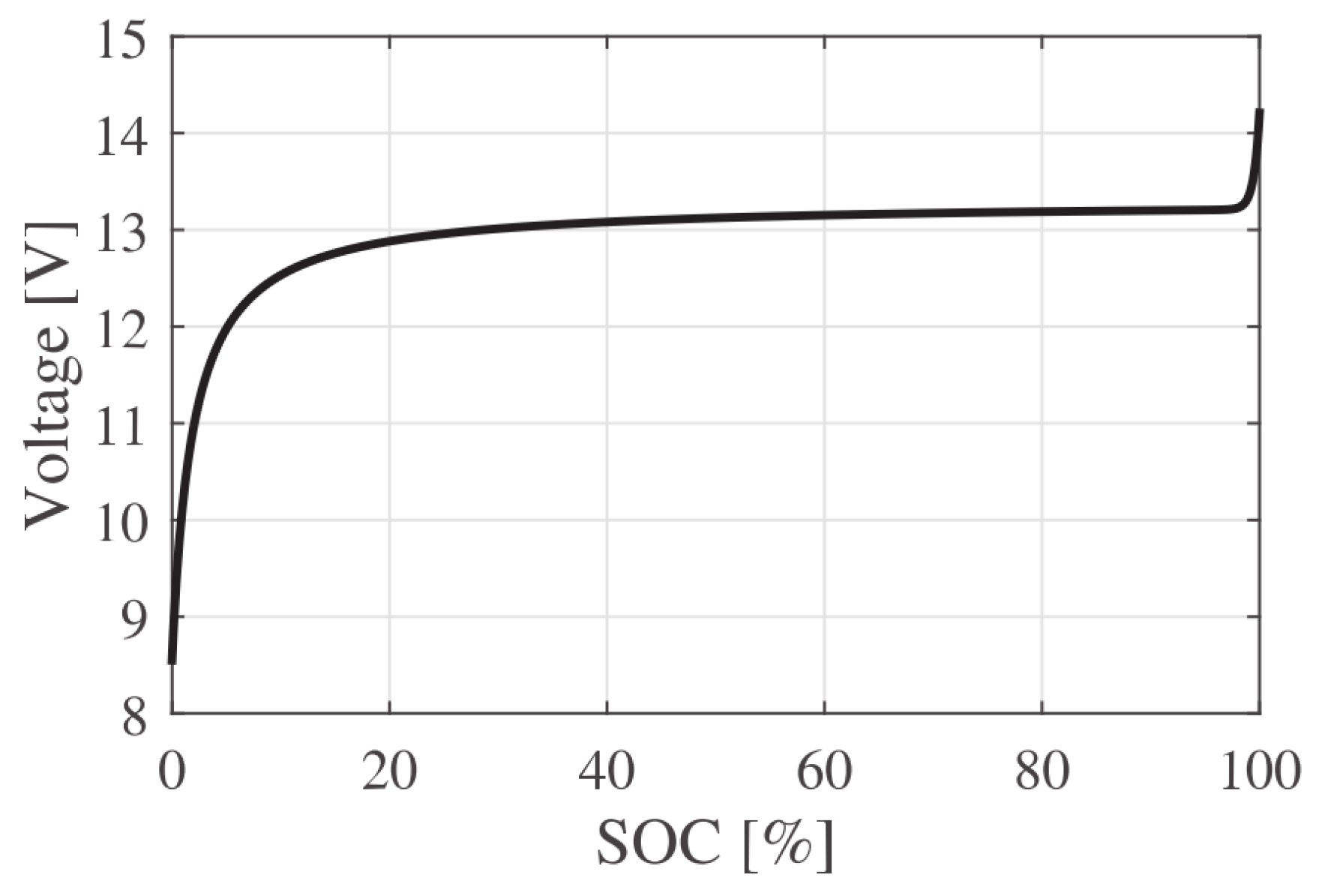
2.5. Battery Model
2.6. The Objective Function
2.7. Linear Programming
2.8. Overall Optimisation Framework
3. Case Study
3.1. The Cost of Electricity
3.2. Estimating Yearly Load and Production
3.3. Estimating and
- The system was simulated for a given, constant value of for different values of . The resulting system costs were found, along with the value of that resulted in the lowest total system cost for the given .
- This step was repeated for different values of , from 0 kWh to 350 kWh.
- The lowest total system cost for each value of was plotted, as shown in Figure 6.
4. Results
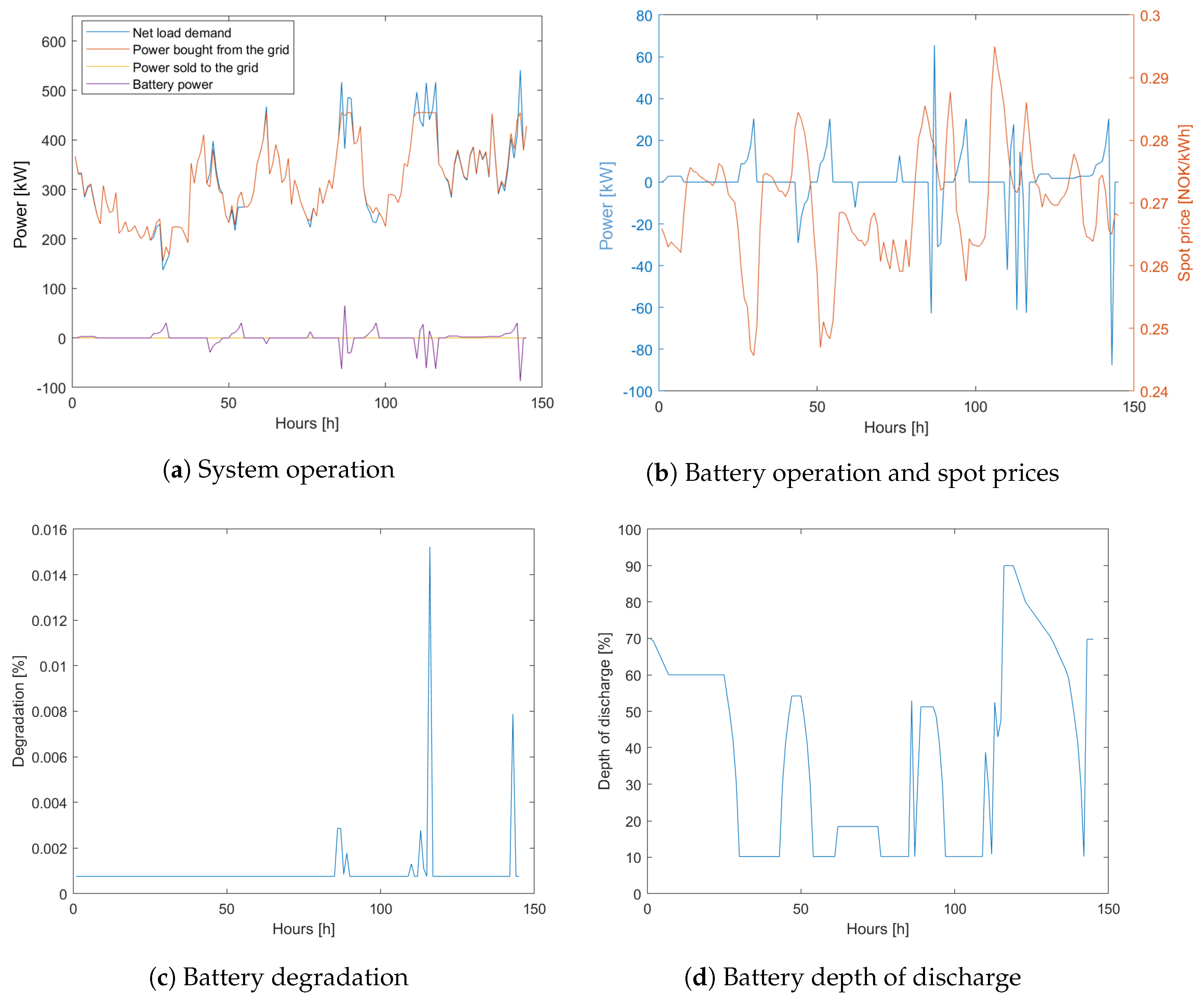
4.1. The Base Case
4.2. The Proposed System
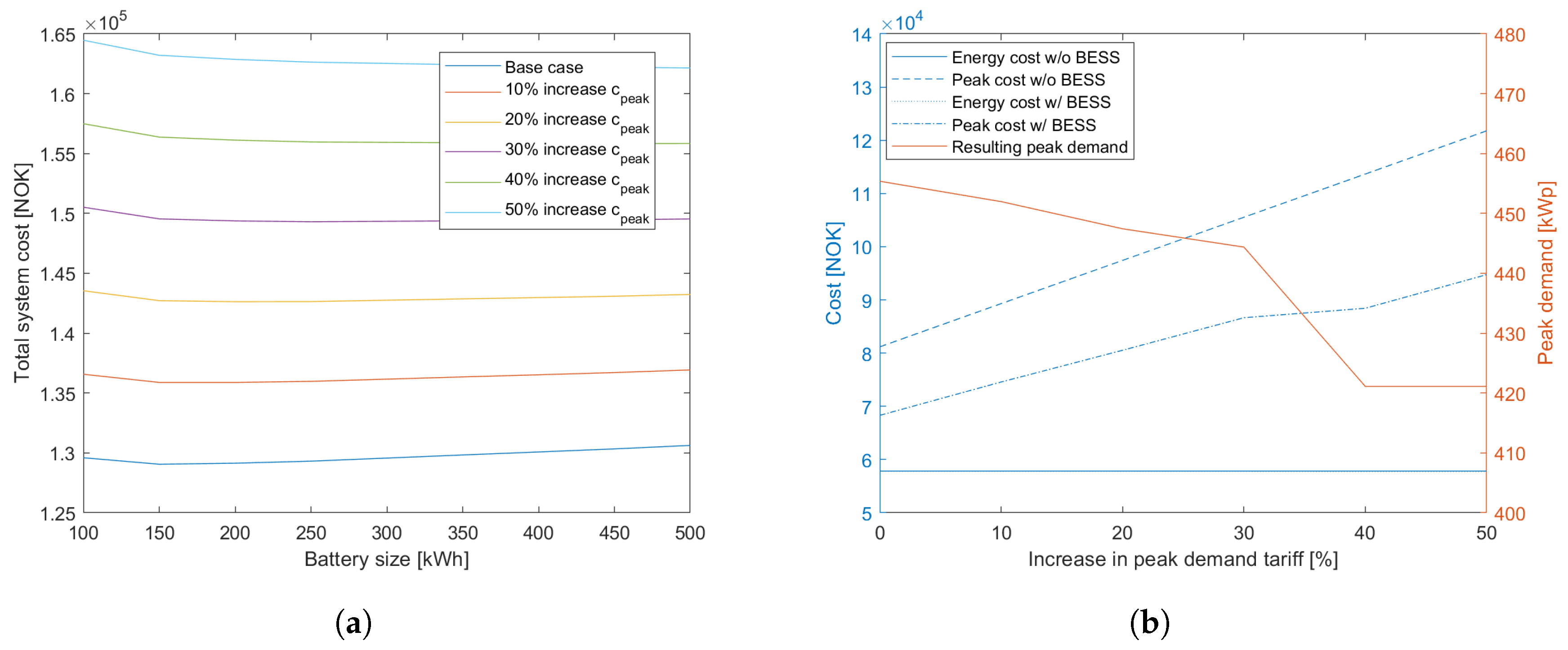
4.3. Sensitivity Analyses
4.3.1. Sensitivity on the Cost of the Battery (SA1)
4.3.2. Sensitivity on the Peak Demand Tariff (SA2)
4.3.3. Sensitivity on the Energy Tariff (SA3)
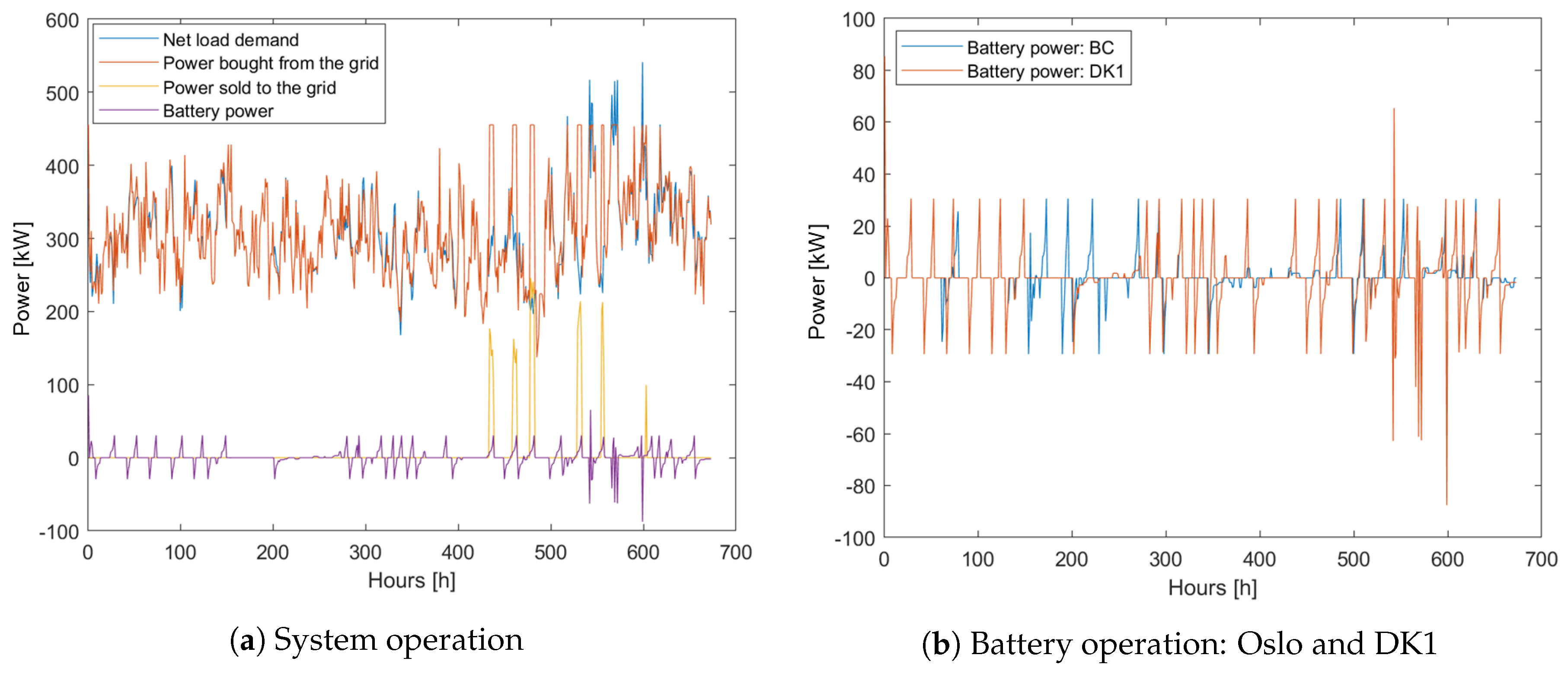
4.4. System Operation in the Future: A 2030 Scenario
4.4.1. The Base Case in a 2030 Scenario
4.4.2. The Proposed System in a 2030 Scenario
5. Discussion
5.1. The Proposed System
5.2. Sensitivity Analyses
5.2.1. SA1 and SA2
5.2.2. SA3
5.3. A 2030 Scenario
6. Conclusions
- Installing a battery storage system is economically attractive, with a net savings on the total system cost of 0.64% yearly. The cost of peak power is reduced by 13.9%, and the savings from peak shaving operation alone is enough to compensate for the yearly cost of the battery. Moreover, the battery is able to account for all energy bought from the grid while still providing 0.1% cost savings through price arbitrage operations.
- The battery seeks to keep the cyclic aging equal to the calendric aging whenever possible, thus charging or discharging only small amounts of power in each time step. In this way, the battery can perform price arbitrage operation while keeping the cost of degradation to a minimum. Even during hours of negative spot prices the battery only charges up to this limit, as the cost of degradation exceeds the possible revenue from charging with negative prices.
- The battery degradation is found to be 7.15% yearly. This gives the battery an expected lifetime of 14 years, which is a reduction from the expected idle shelf life of only one year.
- The simulations are also carried out using an assumed 2030 scenario, where the cost of the battery is reduced, the peak demand charge is increased, and the spot prices are more volatile. The results reveal that implementing a battery storage system is even more profitable in the expected future: the total system costs decrease by 4.15%.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Dagdougui, H.; Mary, N.; Beraud-Sudreau, A.; Dessaint, L. Power management strategy for sizing battery system for peak load limiting in a university campus. In Proceedings of the 2016 IEEE Smart Energy Grid Engineering (SEGE), Oshawa, ON, Canada, 21–24 August 2016; pp. 308–312. [Google Scholar] [CrossRef]
- Dufo-López, R. Optimisation of size and control of grid-connected storage under real time electricity pricing conditions. Appl. Energy 2015, 140, 395–408. [Google Scholar] [CrossRef]
- Sakti, A.; Gallagher, K.G.; Sepulveda, N.; Uckun, C.; Vergara, C.; de Sisternes, F.J.; Dees, D.W.; Botterud, A. Enhanced representations of lithium-ion batteries in power systems models and their effect on the valuation of energy arbitrage applications. J. Power Sources 2017, 342, 279–291. [Google Scholar] [CrossRef]
- Nottrott, A.; Kleissl, J.; Washom, B. Energy dispatch schedule optimization and cost benefit analysis for grid-connected, photovoltaic-battery storage systems. Renew. Energy 2013, 55, 230–240. [Google Scholar] [CrossRef]
- Hesse, H.C.; Martins, R.; Musilek, P.; Naumann, M.; Truong, C.N.; Jossen, A. Economic optimization of component sizing for residential battery storage systems. Energies 2017, 10, 835. [Google Scholar] [CrossRef]
- Abdulla, K.; de Hoog, J.; Muenzel, V.; Suits, F.; Steer, K.; Wirth, A.; Halgamuge, S. Optimal Operation of Energy Storage Systems Considering Forecasts and Battery Degradation. IEEE Trans. Smart Grid 2016, 9, 2086–2096. [Google Scholar] [CrossRef]
- Ranaweera, I.; Midtgård, O.M. Optimization of operational cost for a grid-supporting PV system with battery storage. Renew. Energy 2016, 88, 262–272. [Google Scholar] [CrossRef]
- Wankmüler, F.; Thimmapuram, P.R.; Gallagher, K.G.; Botterud, A. Impact of battery degradation on energy arbitrage revenue of grid-level energy storage. J. Energy Storage 2017, 10, 56–66. [Google Scholar] [CrossRef]
- Eller, A.; Gauntlett, D. Energy Storage Trends and Opportunities in Emerging Markets; Navigant Consulting Inc.: Boulder, CO, USA, 2017. [Google Scholar]
- IRENA. Electricity Storage and Renewables: Costs and Markets to 2030; IRENA: Abu Dhabi, UAE, 2017; p. 132. ISBN 978-92-9260-038-9. [Google Scholar]
- Vetter, J.; Novák, P.; Wagner, M.R.; Veit, C.; Möller, K.C.; Besenhard, J.O.; Winter, M.; Wohlfahrt-Mehrens, M.; Vogler, C.; Hammouche, A. Ageing mechanisms in lithium-ion batteries. J. Power Sources 2005, 147, 269–281. [Google Scholar] [CrossRef]
- Smith, K.; Markel, T.; Pesaran, A. PHEV Battery Trade-Off Study and Standby Thermal Control. 2009. Available online: http://www.nrel.gov/docs/fy09osti/45048.pdf (accessed on 21 November 2019).
- Wang, Y.; Zhou, Z.; Botterud, A.; Zhang, K.; Ding, Q. Stochastic coordinated operation of wind and battery energy storage system considering battery degradation. J. Mod. Power Syst. Clean Energy 2016, 4. [Google Scholar] [CrossRef]
- Omar, N.; Monem, M.A.; Firouz, Y.; Salminen, J.; Smekens, J.; Hegazy, O.; Gaulous, H.; Mulder, G.; Van den Bossche, P.; Coosemans, T.; et al. Lithium iron phosphate based battery–Assessment of the aging parameters and development of cycle life model. Appl. Energy 2014, 113, 1575–1585. [Google Scholar] [CrossRef]
- Yang, Y.; Li, H.; Aichhorn, A.; Zheng, J.; Greenleaf, M. Sizing Strategy of Distributed Battery Storage System With High Penetration of Photovoltaic for Voltage Regulation and Peak Load Shaving. IEEE Trans. Smart Grid 2014, 5, 982–991. [Google Scholar] [CrossRef]
- Andre, D.; Appel, C.; Soczka-Guth, T.; Sauer, D.U. Advanced mathematical methods of SOC and SOH estimation for lithium-ion batteries. J. Power Sources 2013, 224, 20–27. [Google Scholar] [CrossRef]
- Ortega-Vazquez, M.A. Optimal scheduling of electric vehicle charging and vehicle-to-grid services at household level including battery degradation and price uncertainty. IET Gener. Transm. Distrib. 2014, 8, 1007–1016. [Google Scholar] [CrossRef]
- Keil, P.; Schuster, S.F.; Wilhelm, J.; Travi, J.; Hauser, A.; Karl, R.C.; Jossen, A. Calendar Aging of Lithium-Ion Batteries. J. Electrochem. Soc. 2016, 163, A1872–A1880. [Google Scholar] [CrossRef]
- He, G.; Chen, Q.; Kang, C.; Pinson, P.; Xia, Q. Optimal Bidding Strategy of Battery Storage in Power Markets Considering Performance-Based Regulation and Battery Cycle Life. IEEE Trans. Smart Grid 2016, 7, 2359–2367. [Google Scholar] [CrossRef]
- Schmalstieg, J.; Käbitz, S.; Ecker, M.; Sauer, D.U. A holistic aging model for Li(NiMnCo)O2 based 18650 lithium-ion batteries. J. Power Sources 2014, 257, 325–334. [Google Scholar] [CrossRef]
- Curry, C. Lithium-Ion Battery Costs and Market; Bloomberg New Energy Finance: London, UK, 2017. [Google Scholar]
- Nord Pool. Nord Pool Group. 2018. Available online: https://www.nordpoolgroup.com/ (accessed on 21 November 2019).
- Statnett. Fleksibilitet i det Nordiske Kraftmarkedet. 2018. Available online: https://www.statnett.no/globalassets/for-aktorer-i-kraftsystemet/planer-og-analyser/2018-Fleksibilitet-i-det-nordiske-kraftmarkedet-2018-2040 (accessed on 21 November 2019).
- Heipcke, S. Applications of optimization with Xpress-MP. Dash Optim. Blisworth UK 2002, 17, 30–32. [Google Scholar]
- Asker Municipality. FutureBuilt-prosjekt: Holmen svømmehall. 2018. Available online: https://www.asker.kommune.no/samfunnsutvikling/futurebuilt/holmen-svommehall/ (accessed on 21 November 2019).
- Nord Pool. Historical Market Data; Nord Pool: Lysaker, Norway, 2018. [Google Scholar]
- Agder Energi. Plusskunde. 2018. Available online: https://www.aenett.no/bygge-og-grave/tilknytning-til-nett/produksjon-av-strom/plusskunde/ (accessed on 21 November 2019).
- Hafslund Net. Priser På Nettleie-Bedrift; Hafslundnett: Oslo, Norway, 2018. [Google Scholar]
- Aas, B.; SIAT; Berglund, F.; Norwegian University of Science and Technology. Personal communication, May 2018.
- EPEX SPOT. Negative Prices; EPEX SPOT: Paris, France, 2018. [Google Scholar]








| Demand Tariffs | |
|---|---|
| Winter 1 (Jan., Feb., Dec.) | 150 NOK/kWp/month |
| Winter 2 (Mar., Nov.) | 77 NOK/kWp/month |
| Summer (Apr.–Oct.) | 11 NOK/kWp/month |
| (a) | |
| Parameter | Value |
| Pload,t | Input data |
| Ppv,t | Input data |
| cel,t | Day-ahead prices, Oslo (2017) |
| cfeed-in,t | 0.04 NOK/kWh |
| cpeak,m | See Table 1 |
| 150 kW | |
| 150 kWh | |
| 98% | |
| 96% | |
| SOCmin | 10% |
| SOCmax | 90% |
| Lcal | 15 years |
| cbat | 3600 NOK/kWh (Based on cost estimates of NMC BESS for 2016 [10]) |
| (b) | |
| Parameter | Value |
| T | 8760 |
| M | 12 |
| 1 h | |
| 455.38 kW | |
| 0 kWh | |
| SOHinit | 100% |
| Energy drawn from the grid (net load) | 2,243,653 kWh |
| Total cost of energy | 612,767 NOK |
| Total cost of peak power | 315,952 NOK |
| Total cost of electricity | 928,719 NOK |
| Energy drawn from the grid | 2,245,284 kWh |
| Compared to the BC | +1631 kWh |
| Total cost of energy | 612,112 NOK |
| Compared to the BC | −655 NOK |
| Total cost of peak power | 271,998 NOK |
| Compared to the BC | −43,954 NOK |
| Total system cost (objective function) | 922,747 NOK |
| Compared to the BC | −5972 NOK |
| Total degradation | 7.15% |
| Total cost of degradation | 38,636 NOK |
| Compared to the BC | +38,636 NOK |
| Energy drawn from the grid (net load) | 2,243,653 kWh |
| Total cost of energy | 626,480 NOK |
| Total cost of peak power | 410,737 NOK |
| Total cost of electricity | 1,037,217 NOK |
| Energy drawn from the grid | 2,272,023 kWh |
| Compared to the BC (2030) | +28,370 kWh |
| Compared to the proposed system (2018) | +26,739 kWh |
| Feed-back to the grid | 25,574 kWh |
| Revenue generated from feed-back | 1023 NOK |
| Total degradation | 1.47% |
| Total cost of degradation | 19,836 NOK |
| Compared to the BC (2030) | +19,836 NOK |
| Compared to the proposed system (2018) | −18,800 NOK |
| Total cost of energy | 622,383 kWh |
| Compared to the BC (2030) | −4097 NOK |
| Compared to the proposed system (2018) | +10,271 kWh |
| Total cost of peak power | 353,041 NOK |
| Compared to the BC (2030) | −57,696 NOK |
| Compared to the proposed system (2018) | +81,043 kWh |
| Total system cost (objective function) | 994,238 NOK |
| Compared to the BC (2030) | −42,979 NOK |
| Compared to the proposed system (2018) | +71,491 kWh |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Berglund, F.; Zaferanlouei, S.; Korpås, M.; Uhlen, K. Optimal Operation of Battery Storage for a Subscribed Capacity-Based Power Tariff Prosumer—A Norwegian Case Study. Energies 2019, 12, 4450. https://doi.org/10.3390/en12234450
Berglund F, Zaferanlouei S, Korpås M, Uhlen K. Optimal Operation of Battery Storage for a Subscribed Capacity-Based Power Tariff Prosumer—A Norwegian Case Study. Energies. 2019; 12(23):4450. https://doi.org/10.3390/en12234450
Chicago/Turabian StyleBerglund, Frida, Salman Zaferanlouei, Magnus Korpås, and Kjetil Uhlen. 2019. "Optimal Operation of Battery Storage for a Subscribed Capacity-Based Power Tariff Prosumer—A Norwegian Case Study" Energies 12, no. 23: 4450. https://doi.org/10.3390/en12234450
APA StyleBerglund, F., Zaferanlouei, S., Korpås, M., & Uhlen, K. (2019). Optimal Operation of Battery Storage for a Subscribed Capacity-Based Power Tariff Prosumer—A Norwegian Case Study. Energies, 12(23), 4450. https://doi.org/10.3390/en12234450