Kinetics and Reactor Design Aspects of Selective Methanation of CO over a Ru/γ-Al₂O₃ Catalyst in CO₂/H₂ Rich Gases ^†

Abstract

Polymer electrolyte membrane fuel cells (PEMFCs) for household applications utilize H₂ produced from natural gas via steam reforming followed by a water gas shift (WGS) unit. The H₂-rich gas contains CO₂ and small amounts of CO, which is a poison for PEMFCs. Today, CO is mostly converted by addition of O₂ and preferential oxidation, but H₂ is then also partly oxidized. An alternative is selective CO methanation, studied in this work. CO₂ methanation is then a highly unwanted reaction, consuming additional H₂. The kinetics of CO methanation in CO₂/H₂ rich gases were studied with a home-made Ru catalyst in a fixed bed reactor at 1 bar and 160–240 °C. Both CO and CO₂ methanation can be well described by a Langmuir Hinshelwood approach. The rate of CO₂ methanation is slow compared to CO. CO₂ is directly converted to methane, i.e., the indirect route via reverse water gas shift (WGS) and subsequent CO methanation could be excluded by the experimental data and in combination with kinetic considerations. Pore diffusion may affect the CO conversion (>200 °C). The kinetic equations were applied to model an adiabatic fixed bed methanation reactor of a fuel cell appliance.

1. Introduction

In recent years, the interest in proton-exchange membrane fuel cells (PEMFCs), also known as polymer electrolyte membrane fuel cells, for stationary applications such as households or office buildings has increased [1]. PEMFCs cogenerate electrical power and heat (heated water) from hydrogen (and O₂/air) and reach about 80% overall efficiency. Home fuel cells cannot generate at all times exactly the needed amount of both heat and electricity, and are typically combined with a traditional furnace (e.g., with natural gas as fuel) and households are also connected to the electrical grid to cover the need of heat and electricity not produced by the fuel cell.

Today, pure H₂ is rarely directly available. Hence, home fuel cells currently use H₂ produced from natural gas from the gas grid. Natural gas is firstly converted by steam reforming (CH₄ + H₂O ↔ CO + 3H₂) followed by a water gas shift (WGS) reactor (CO + H₂O ↔ CO₂ + H₂) to decrease the concentration of CO (and to increase the output of H₂). However, the WGS is limited by thermodynamic constraints, and the H₂ rich gas still contains small amounts of CO at the outlet of the WGS, typically 0.5–1 vol% CO [2,3]. Unfortunately, even traces of CO deactivate the anode electro-catalyst of the PEMFC. Therefore, the CO content must not exceed 10 ppm for Pt-anodes and 100 ppm for PtRu-anodes [4,5]. Hence, the CO content in the operating gas has to be further reduced below these threshold values, preventing a degradation of the fuel cell catalyst.

CO preferential oxidation (CO-PROX) has so far been a reliable technique as a fine purification step of the feed gas downstream the WGS unit [2,6,7,8,9,10]. In order to achieve the required low CO concentration permanently, a two-step system is usually needed [4]. In addition, this process requires an additional, closely controlled, low rate O₂ supply to keep the unwanted oxidation of hydrogen as low as possible [2,10].

Selective CO methanation is considered an attractive alternative to preferential CO oxidation for the removal of CO from H₂ rich gases for PEMFC applications [11,12]. Here, no additional reactant is needed such as air for CO-PROX, but the risk of undesired CO₂ methanation, which also consumes additional H₂, is likely to occur.

In the course of selective CO methanation of a reformate gas, three main reactions can take place, CO methanation, Equation (1), CO₂ methanation, Equation (2), and the reverse-water-gas-shift (RWGS) reaction, Equation (3).

$$\mathrm{CO}+3{\mathrm{H}}_2\leftrightarrow {\mathrm{CH}}_4+{\mathrm{H}}_2\mathrm{O}{\Delta \mathrm{H}}_{298}^0=-206\mathrm{kJ}·{\mathrm{mol}}^{-1}$$

(1)

$${\mathrm{CO}}_2+4{\mathrm{H}}_2\leftrightarrow {\mathrm{CH}}_4+2{\mathrm{H}}_2\mathrm{O}{\Delta \mathrm{H}}_{298}^0=-165\mathrm{kJ}·{\mathrm{mol}}^{-1}$$

(2)

$${\mathrm{CO}}_2+{\mathrm{H}}_2\leftrightarrow \mathrm{CO}+{\mathrm{H}}_2\mathrm{O}{\Delta \mathrm{H}}_{298}^0=41\mathrm{kJ}·{\mathrm{mol}}^{-1}$$

(3)

Both CO₂ methanation and reverse water gas shift (RWGS) are unwanted and have to be suppressed, as valuable H₂ is consumed and CO is even produced, respectively. The development of a catalyst with high activity and selectivity for CO methanation at low temperatures, optimally at temperatures of the off-gas of the WGS unit of around 200 °C, is therefore important. Ruthenium catalysts show these characteristics for selective CO methanation [13,14,15,16,17,18].

Based on own screening experiments with a variety of Co and Ru catalysts with regard to activity and selectivity for selective CO methanation [19], a 2 wt% Ru/γ-Al₂O₃ catalyst was selected as most suitable for this application. This Ru catalyst was prepared and applied for the investigation of the CO methanation reaction under varying reaction conditions, starting from gas mixtures containing only CO and H₂ as well as CO₂ and H₂, respectively, to gas mixtures including H₂O and both CO and CO₂, representing a more realistic reformate gas comparable to practical applications [20]. In addition to 0.5–1 vol% CO, gas streams leaving the RWGS unit typically contain 10–25 vol% CO₂ and 5–20% H₂O (rest H₂) [12,21,22]. Kinetic studies were conducted to investigate the influence of temperature and concentration of each gas component on the reaction rate. Based on the respective results, a kinetic model was developed to predict the conversions of CO and CO₂. Finally, an adiabatic fixed reactor was simulated to determine the amount of a methanation catalyst needed for a typical household fuel cell system.

2. Experimental

2.1. Catalyst Preparation and Characterization

The 2 wt% Ru/γ-Al₂O₃ catalyst was prepared by incipient wetness impregnation (

Table 1. Characteristic data of the Ru catalyst.

Parameter	Value
Particle radius, rp	1.25 mm
Shell thickness, dsh	0.35 mm
Density of particle, ρp	980 kg·m−3
Porosity of particle, εp	0.49
Tortuosity, τp	2
Average pore diameter, dpore	9.3 nm
BET surface area	215 m2·g−1
Ru particle size	6 nm

). Al₂O₃ spheres with a diameter of 2.5 mm (Sasol Germany GmbH) were impregnated with the respective amount of Ru(NO)(NO₃)₃ solution in dilute nitric acid. The catalyst was dried under vacuum in a rotary evaporator (60 °C, 8 h). After reduction with H₂ (10% in N₂, 350 °C, 4 h), the catalyst was utilized in the methanation of CO or CO₂. The catalyst was characterized by energy dispersive X-ray spectroscopy (EDX), indicating that a core shell catalyst with a thickness of the Ru containing shell of 0.35 mm was obtained. The shell represents 62% of the particle volume, and the Ru content in this shell is therefore about 3 wt% compared to the average content of 2%. H₂ pulse chemisorption experiments were performed after reduction in H₂ (350 °C, 30 min). The Ru particle size of 6 nm was calculated on the basis of a 1:1 stoichiometry for the number of Ru atoms exposed on the catalyst surface to the number of chemisorbed H atoms at 110 °C.

2.2. Experimental Set-up

The experimental set-up consists of a fixed-bed reactor made of steel with an internal diameter of 1.4 cm and a length of 80 cm. The catalyst was positioned in the middle of the tube and diluted with (inactive) quartz sand in a ratio of 1:2 by mass in order to keep the catalyst temperature constant and to prevent hot spots. The reactor was thermostated by an oil heating system, guaranteeing isothermal conditions. Two thermocouples in a guide tube were positioned in the catalyst bed to monitor the temperature. Feed gases relevant to those of a reformate gas containing CO, CO₂, H₂, and H₂O were used. The concentrations of all these reactants were varied by substituting the reactant of interest by N₂, typically 40%, to study the kinetics of each gas; thereby, the inlet concentrations of the other reactants and the total pressure were kept. Experiments took place at atmospheric pressure at temperatures of 160–250 °C. The gas composition leaving the reactor (CO, CO₂, H_2, and CH₄) was analyzed with a gas analyzer (X-STREAM Enhanced Process Gas Analyzer, Emerson) and by gas chromatography, although higher hydrocarbons were only formed to a very small extent. The flow rates of the feed gases were adjusted by mass flow controllers and for steam addition by a water saturator. The feed gas could be analyzed by using a bypass. During the kinetic experiments, the gas was directed first to the saturator (in case of steam addition) and then to the reactor and gas analyzer.

2.3. Kinetic Measurements

The reactor was charged with 2 g of the catalyst diluted with 4 g of quartz sand. The catalyst bed length was 5 cm. Hence, the pressure drop was considered to be negligible. The measurements were carried out at atmospheric pressure using mixtures of CO, CO₂, H₂, H₂O, and N₂. First, the influence of each gas on the reaction rates was examined. Both for CO and for CO₂ methanation, a kinetic expression based on a Langmuir–Hinshelwood approach turned out to be suitable. The temperature was then varied from 160–190 °C for CO and 190–235 °C for CO₂. Initially, the residence time was small to keep conversion of CO and CO₂ low (<10%) and ensure differential conditions for a direct determination of the rates (r_i = − dc_i/dτ ≈ c_i,in X_i/τ). The CO content was varied from 0.4–1.4 vol%, CO₂ from 0–20%, H₂ from 50–90%, and H₂O from 0–20%. Integral kinetic measurements were also conducted to prove the kinetic expressions for a wide range of CO conversion up to almost 100%.

3. Results

3.1. CO Methanation Kinetics

As already mentioned, the kinetics of CO methanation follow a Langmuir–Hinshelwood approach:

$$-\frac{{\mathrm{d}C}_{\mathrm{CO}}}{\mathrm{d}\mathsf{\tau }}=r_{\mathrm{CO}}=\frac{k_{\mathrm{CO}}{\left(T\right)c}_{\mathrm{CO}}{c}_{{\mathrm{H}}_2}}{{\left({1+K}_1{c}_{\mathrm{CO}}{+K}_2{c}_{{\mathrm{H}}_2\mathrm{O}}\right)}^2}$$

(4)

where C_i (i = CO, H₂, H₂O) is the gas concentration, τ is the (modified) residence time defined as the ratio of catalyst mass to volumetric gas flow at reaction conditions (p, T), K₁ and K₂ are adsorption constants for CO and H₂O, respectively, and k_CO(T) represents the reaction rate constant according to the Arrhenius law:

$$k_{\mathrm{CO}}\left(T\right){=k}_{0,\mathrm{CO}}{e}^{\frac{-E_{\mathrm{A},\mathrm{CO}}}{RT}}$$

(5)

k₀ is the pre-exponential factor, and E_A,i the activation energy. The influence of temperature on the adsorption constants (K₁, K₂) turned out to be negligible. The parameters of the reaction rate of CO methanation finally obtained based on experiments at varied temperatures and gas concentrations are listed in

Table 2. Parameters of the kinetics of CO methanation (see Equations (4) and (5)).

Parameter	Value
EA,CO	90 kJ·mol−1
k0,CO	3.61 × 107 m6·s−1·kg−1·mol−1
K1	23 m3·mol−1
K2	0.3 m3·mol−1

.

Figure 1 exemplarily shows the influence of the CO concentration on the rate of CO methanation at different temperatures, whereby the CO conversion was kept low (<10%) by adjusting short residence times and high (standard) volume rates of 12.5 l h⁻¹ to 43.5 l h⁻¹ (STP), respectively, to ensure differential conditions. Higher hydrocarbons (HCs) from ethane to pentane, with a maximum for C₃ and declining values for higher C-numbers, were also detected at low temperatures; so, the Ru catalyst also has a certain Fischer–Tropsch activity. The selectivity to higher HCs was 30 C% at 162 °C, but as the temperature rises this selectivity decreases strongly, until at about 220 °C no higher HCs and only methane are formed. Formation of CO₂ was never observed, indicating that the WGS reaction (reverse of Equation (3)) does not take place. Figure 1 shows that the reaction rate of CO passes through a maximum and decreases with higher CO concentrations as expected for a Langmuir–Hinshelwood rate expression. The agreement of the experimental data at higher temperatures of up to about 220 °C, where almost complete CO conversion is reached, and of the calculations based on numerical integration of Equation (4) utilizing the kinetic parameters (Table 2) is quite satisfactory (Figure 2).

The addition of CO₂ to a feed gas consisting of 1.13 vol% CO, 10% H₂O, and 55% H₂ (rest N₂) showed no influence on the CO conversion, even if 16% CO₂ were added (Figure 2). The conversion of CO₂ was less than 2% even for the highest temperature of 220 °C, where 90% conversion of CO (to methane) was already reached.

3.2. Kinetics of CO₂ Methanation

CO₂ is also subject to methanation, although to a lower extent compared to CO. So, the reaction of CO₂ with H₂ was also studied, at first without CO in the feed. Thereby, CO was not detected for T < 200 °C, and even for T > 200 °C the content was less than 25 ppm. The possible role of the RWGS and the question whether CO₂ is directly converted to methane or via RWGS and subsequent CO methanation is discussed in detail in Section 3.3. For CO₂ methanation, a Langmuir–Hinshelwood approach was also used to fit the experimental data:

$$-\frac{{\mathrm{d}C}_{{\mathrm{CO}}_2}}{\mathrm{d}\mathsf{\tau }}{=r}_{{\mathrm{CO}}_2}=\frac{k_{{\mathrm{CO}}_2}{\left(T\right)c}_{{\mathrm{CO}}_2}{c}_{{\mathrm{H}}_2}}{{\left({1+K}_3{\left(T\right)c}_{{\mathrm{CO}}_2}{+K}_4{\left(T\right)c}_{\mathrm{CO}}{+K}_5{c}_{{\mathrm{H}}_2\mathrm{O}}\right)}^2},$$

(6)

c_i (i = CO, CO₂, H₂, H₂O) is the gas concentration, τ is the residence time defined as the ratio of catalyst mass to the volume flow at reactor temperature and atmospheric pressure, K₃(T), K₄(T) and K₅ are the adsorption constants for CO₂, CO and H₂O, respectively, and k_i(T) is the reaction rate constant according to the Arrhenius law (Equation (5)). The influence of temperature on the adsorptions K_i is given by:

$$K_{\mathrm{i}}\left(T\right){=K}_{0,\mathrm{i}}{e}^{\frac{-\Delta H_i}{RT}}$$

(7)

CO, CO₂, and H₂O have an influence on the reaction rate of CO₂, but the CO concentration has the highest impact. For instance, at 190 °C, the adsorption constant for CO (K₄) is 14.6 m³·mol⁻¹, and thus much higher than the value for CO₂ (K₃) with 0.17 m³·mol⁻¹ or for H₂O (K₅) with 1.1 m³·mol⁻¹. The adsorption constant for H₂O turned out to be independent of temperature. Most probably, the active catalyst surface (Ru) is blocked by adsorbed CO due to strong adsorption of CO compared to CO₂ and H₂O [23]. This leads to a low activity for (dissociative) adsorption of CO₂. The kinetic parameters of Equation (6) are presented in

Table 3. Parameters of the kinetics of CO₂ methanation (see Equations (6) and (7)).

Parameter	Value
EA,CO2	81 kJ·mol−1
k0,CO2	2.8 ×104 m6·s−1·kg−1·mol−1
K0,3	8.4 m3·mol−1
ΔH3	15 kJ·mol−1
K0,4	1.15 ×10−13 m3·mol−1
ΔH4	−125 kJ·mol−1
K5	1.1 m3·mol−1

.

Note that formally the adsorption of CO₂ turned out to be surprisingly endothermic (Table 3). It should be also emphasized that, although unsurprisingly the constant K₄ for CO “adsorption” in case of CO₂ methanation is at 190 °C similar to the respective parameter K₁ for CO methanation, 15 m³ mol⁻¹ compared to 23 m³·mol⁻¹, (see Equation (4) and Table 2 and Table 3), but K₁ turned out to be independent of temperature, whereas the rate of CO₂ methanation could only be described accurately in the whole range of the investigated temperature by assuming a strong influence of temperature on K₄. Hence, the physical meaning of the constants K_i, although denoted here as adsorption constants, is highly questionable, but at least they formally describe the reaction rate(s) quite well.

Figure 3 shows the influence of the CO₂ concentration on the reaction rate. The degree of CO₂ conversion was kept below 10 % to realize differential conditions (r_CO2 = − dc_CO2/dτ ≈ c_CO2,in X_CO2/τ). The volume rate was 16 to 22 l h⁻¹ (STP). The agreement of the experimental data and the rates calculated by Equation (6) is satisfactory. The rate of CO₂ methanation increases with increasing CO₂ concentration to a maximum and then decreases for high CO₂ concentrations (>5 mol·m⁻³ = 18 vol-%), as shown in Figure 3 by the calculation for 234 °C and high values of c_CO2. The only product found for CO₂ conversion is methane, which is a first indication of a negligible activity of the catalyst for the RWGS, Equation (3), an aspect discussed below in more detail.

If CO is added to the feed gas containing CO₂, the CO₂ reaction is strongly suppressed. Figure 4a compares the calculated initial reaction rates of CO and CO₂ at different CO concentrations for 190 °C and 230 °C. For clarity, the ratio of both rates is also shown (Figure 4b).

At a relative high temperature (230 °C), the reaction rate of CO₂ is in the same order of magnitude than the rate for CO (Figure 4b). So, about the same amount of H₂ is consumed by both reactions. To the contrary, the r_CO2-to-r_CO ratio is lower than one (for c_CO > 0.03 mol m⁻³ = 0.1 vol%) at 190 °C and strongly declines with increasing c_CO. The reason is (formally) the much lower adsorption constant for CO (K₄), 15 m³·mol⁻¹ at 190 °C and only 1.1 m³·mol⁻¹ at 230 °C.

It must be emphasized that the rates and the rate ratios given in Figure 4 were calculated for a content of CO and of CO₂ relevant for selective methanation, i.e., the CO₂ content is about an order of magnitude higher compared to CO. For example, at 230 °C and a CO content of 1% (0.3 mol m⁻³), the ratio of the rates (r_CO2/r_CO) is about one (Figure 4b), but the ratio of the concentrations (c_CO,in/c_CO2,in) is only 0.05. The change of conversion X with residence time, dX_i/dτ, equals the term r_i/c_i,in, and the ratio of the (differential change) of the conversion of CO and CO₂, dX_CO2/dX_CO, is given by the term r_CO2/c_CO2,in × c_CO,in/r_CO = 0.05 r_CO2/r_CO. Hence, the (initial) change of X_CO2 with residence time τ is 20 times smaller compared to CO, even if both rates are equal.

3.3. Reaction Mechanism of CO and CO₂ Methanation

The mechanism of CO and CO₂ methanation have been the subject of several studies. It is generally agreed that hydrogenation of CO proceeds via dissociation of CO into C and O atoms followed by hydrogenation to CH₄ and H₂O [16,24,25]. For CO₂ methanation, there are two different opinions on the nature of the mechanism:

• CO₂ is converted into CO prior to methanation, and direct methanation of CO₂ does not take place [26,27,28,29].
• CO₂ is directly converted into methane without CO as intermediate [1,30].

For the Ru catalyst used here, the rate of CO conversion is high. So, even if only CO₂ (and H₂) are used as reactants, it is hard to distinguish which of the two proposed mechanisms is true. If, for example, the first mechanism with CO as intermediate is true, the concentration of CO may be nevertheless small and hard to detect. Here, two approaches were used to estimate the role of direct and indirect CO₂ methanation, respectively:

• The rate of RWGS can be estimated and compared with the measured CO₂ rate based on the (easily) measurable rate of the reverse WGS reaction and some thermodynamic considerations.
• The rate of CO₂ conversion must equal the rate of RWGS, if (hypothetically) only indirect CO₂ methanation via CO takes place. The RWGS is then the rate determining step followed by fast CO methanation. A calculation based on the CO and CO₂ rate equations (as determined in this work) lead to the concentration of the intermediate CO, which should correspond to the observed values. If the calculated CO yield is much higher than the one observed, this is an indication of the dominance of direct CO₂ methanation and vice versa.

Approach 1: Unfortunately, the rate of the RWGS could not be directly measured here, because methanation of CO is fast hindering the decision whether direct CO₂ methanation or the RWGS followed by CO methanation takes place. However, the rate of RWGS can at least be estimated based on the measurable rate of the WGS, Equation (8), and the equilibrium constant K_c. The rate of the WGS was investigated with 20% CO and 20% H₂O in N₂ (m_cat = 2 g, V_STP = 8 l h⁻¹, 200–260 °C). The conversion of CO was less than 4%. (Remark: The equilibrium CO conversion of the WGS for these conditions is higher than 90%; hence, only the WGS without any influence of the RWGS was measured.) The simplifying assumption used here is that the WGS is first order both with respect to CO and H₂O. It has to be noted that this assumption was not experimentally proved and verified. Nevertheless, the rate of the WGS obtained by this assumption is still a rough but good estimation to show (see below) whether CO₂ is most probably directly converted (hydrogenated) to methane or indirectly via CO. Using this assumption, the rate constant k_WGS was determined, yielding a pre-exponential factor, k_WGS,0, of 5.18×10⁴ m³ mol⁻¹·kg⁻¹·s⁻¹ and an activation energy, E_A,WGS, of 97 kJ/mol.

The equilibrium constant K_C of the WGS is approximately the ratio of the rate constants of WGS and RWGS, and thus the rate constant of the RWGS was calculated (k_RWGS = k_WGS/K_C).

$$\mathrm{CO}+{\mathrm{H}}_2\mathrm{O}\leftrightarrow {\mathrm{CO}}_2+{\mathrm{H}}_2{\Delta \mathrm{H}}_{298}^0=-41\mathrm{kJ}{\mathrm{mol}}^{-1}$$

(8)

According to [31], the equilibrium constant K_C of the WGS can be determined by:

$$K_{\mathrm{c}}\left(T\right)=\frac{c_{{\mathrm{CO}}_2}{c}_{{\mathrm{H}}_2}}{c_{\mathrm{CO}}{c}_{{\mathrm{H}}_2\mathrm{O}}}={0.0147e}^{\frac{4577.8}{T}}$$

(9)

So finally, the rate of the RWGS is given by r_RWGS = k_WGS/K_C·c_CO2·c_H2. For 190 °C and a feed gas containing 20% CO₂, 55% H₂, and 25 vol% N₂, and the conditions used here (m_cat = 2 g, V_STP = 8 l h⁻¹), r_RWGS is only 0.0005 mmol CO₂ kg⁻¹·s⁻¹ compared to the much higher measured reaction rate of CO₂, r_CO2, of 0.29 mmol CO₂ kg⁻¹·s⁻¹. Hence, CO₂ is most probably not consumed by the RWGS followed by CO methanation, but only by direct methanation.

Approach 2: If the hypothesis would be correct that CO₂ methanation is just an indirect reaction and only occurs via RWGS and subsequent CO methanation, then the measured reaction rate of CO₂ should only reflect the rate of RWGS. The concentration of the intermediate CO during the conversion of CO₂ with H₂ can then be calculated based on the CO and CO₂ reaction rates as determined in this work, Equation (4) and Equation (6). Figure 5a,b show the results both of the calculation and of two experiments for a feed gas with 6 vol% CO₂ and 55% H₂ (rest N₂), if either the temperature (140–252 °C) or the residence time (for 223 °C and 234 °C) is increased. The calculated volumetric content of the “intermediate” CO can now be compared with experimental data: The calculation leads to relative high values of the CO content of up to 400 ppm, although no CO was experimentally detected for T < 200 °C. Even for 223 °C and 9% CO₂ conversion (12% measured), the simulation leads to 240 ppm CO, whereas the experimental value is only 20 ppm. For 234 °C, X_{CO2,calculated} is 12% (still in acceptable agreement with 16% measured) and 190 ppm CO are calculated compared to the experimental value of 25 ppm CO. These results again lead to the conclusion that the indirect pathway of CO₂ methanation is not or only to a very low extent taking place, and that CO₂ is directly hydrogenated to CH₄ on the used Ru catalyst.

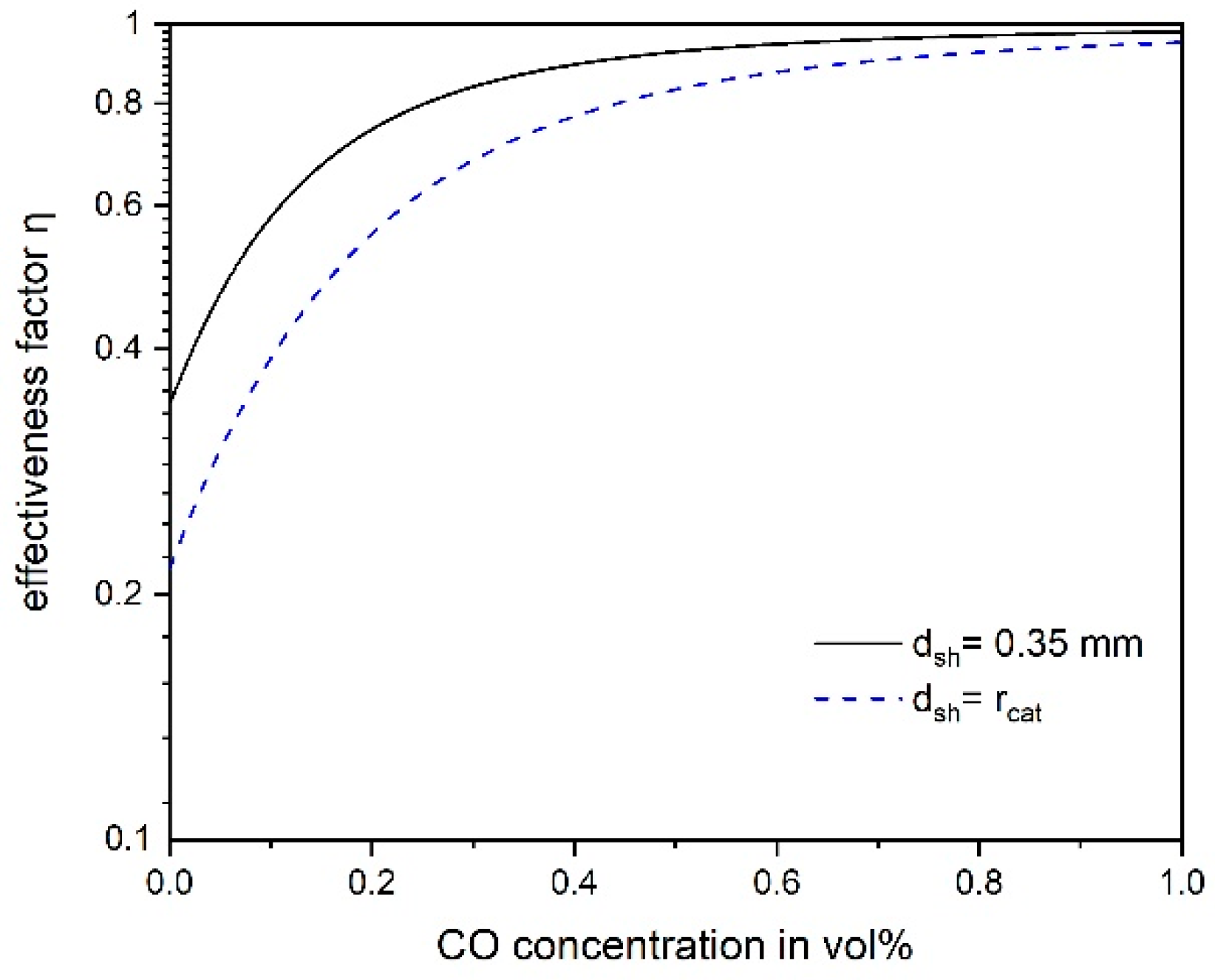
3.4. Influence of Pore Diffusion on the Rate of CO Methanation

A pore diffusion resistance lead to internal concentration gradients and a lower effective rate compared to the intrinsic chemical rate; this has to be considered in the rate equation [32]. For this purpose, the generalized Thiele modulus Φ_gen for an irreversible reaction with arbitrary kinetics (here Langmuir–Hinshelwood approach, Equation (4)), was used [33]:

$${\Phi }_{\mathit{gen}}=\frac{V_{\mathrm{p}}}{A_{\mathrm{p}}}\frac{r_{\mathrm{CO}}{\rho }_p}{\sqrt{{2D}_{\mathrm{CO},\mathrm{eff}}{\left(T\right)\rho }_{\mathrm{p}}{{\displaystyle \int }}_0^{c_{\mathrm{CO}}}{r}_{\mathrm{CO}}{\mathrm{d}c}_{\mathrm{CO}}}}$$

(10)

V_p is the particle volume, A_p the outer surface, r_co the reaction rate of CO, ρ_p the particle density, and D_CO,eff the effective diffusion coefficient, D_CO,eff = ε_p/τ_p [1/D_mol + 1/D_Kn]⁻¹, which also considers the particle porosity (ε_p) and tortuosity (τ_p). The binary diffusion coefficient for CO in H₂ is calculated based on D₀ for T₀ = 273 K and 1 bar (0.65 cm²·s⁻¹), D_mol = D₀ (T/T₀)^1.75, and the Knudsen diffusivity is given by D_Kn = (d_pore/3) [8RT/(π M_CO)]^0.5.

It had to be considered here that the active component (Ru) is concentrated in an outer shell with a thickness d_shell of 0.35 mm. In this case, V_p has to be replaced by the volume of the shell, V_shell = 4/3 π [r_p³ − (r_p − d_sh)³]. Hence, the rate constant k_shell is higher than the measured average value k, and the ratio can be calculated by:

$$\frac{k_{\mathit{shell}}}{k}=\frac{m_p}{m_{\mathit{shell}}}=\frac{V_p}{V_{\mathit{shell}}}={\left(1-{\left(1-\frac{d_{\mathit{sh}}}{r_p}\right)}^3\right)}^{-1}$$

(11)

m_p and m_shell is the mass of the catalyst and catalyst shell, respectively. The solution of Equation (10) finally leads to:

$${\Phi }_{\mathit{gen}}=\left(1-{\left(1-\frac{d_{\mathit{sh}}}{r_p}\right)}^3\right)\frac{r_p}{3}\frac{k_{\mathit{shell}}{\rho }_p}{\sqrt{{2D}_{\mathit{CO},\mathit{eff}}{\rho }_p\frac{k_{\mathit{shell}}{C}_{{\mathrm{H}}_2}}{K_1^2}\left(\frac{b}{K_1{C}_{\mathrm{CO}}+b}+\ln \left(K_1{C}_{\mathrm{CO}}+b\right)-1-\ln b\right)}}$$

(12)

The factor b is defined as b = 1 + K₂ C_H2O. The effectiveness factor with regard to pore diffusion is [32]:

$$\mathsf{\eta }=\frac{r_{\mathrm{CO},\mathrm{eff}}}{r_{\mathrm{CO}}}=\frac{{\tanh \Phi }_{\mathit{gen}}}{{\Phi }_{\mathit{gen}}}$$

(13)

The parameters used for the calculation of the effectiveness factor are listed in Table 1.

Figure 6 shows that at high CO concentrations the effectiveness factor is close to 1, i.e., pore diffusion has almost no influence on the effective rate. For lower CO concentrations (<0.5%), important for selective CO methanation, the factor is less than one and the influence of pore diffusion has to be considered, e.g., for the design of a technical reactor.

Figure 6 also shows the advantage of the shell catalyst compared to a catalyst with uniform Ru distribution (denoted as “full” catalyst); for the slow CO₂ methanation, pore diffusion does not play a role for the conditions relevant here. Figure 7 depicts the influence of temperature on the effectiveness factor. At low temperatures, the factor approaches a value of one, but at temperatures above about 200 °C, the internal mass transport is not negligible and was therefore considered in the modelling of a technical methanation reactor (see below).

Hence in the following, the effect of pore diffusion was considered. Figure 8 shows measured and modelled values of the conversion of CO (numerical integration of Equation (4)) including influence of pore diffusion) for two different feed gases with 1.41 and 0.5 vol% CO and 55 vol% H₂ in N₂. For the given temperatures of below 200 °C, the conversion and reaction rates are almost unaffected by pore diffusion (see also Figure 7), and therefore not really reflected by the experimental data.

3.5. Simulation of CO Methanation in an Adiabatic Fixed Bed Reactor Suitable for a Household PEMFC

The kinetic equations for CO and CO₂ methanation were now used to model the selective methanation of CO in a CO₂/H₂ rich gas stream also containing steam, where both methanation reactions take place simultaneously. The simulation was performed using Matlab and then compared with the respective experimental values.

The mass balance of a fixed bed reactor and steady state conditions is as follows:

$$-u_s\frac{{\mathrm{d}C}_i}{\mathrm{d}z}=\sum r_{i,\mathit{eff}}{\rho }_b$$

(14)

u_s is the superficial gas velocity, and p_b the bulk density (500 kg·m⁻³) of the catalyst (bed porosity is 49%). The effective rate r_i,eff (= η r_i) considers the influence of pore diffusion for CO methanation; for CO₂ methanation, η is almost one even for temperatures up to 250 °C.

In Figure 9, the measured degrees of conversion of CO and CO₂ in the isothermal lab-scale reactor are compared with the simulation for a gas containing CO, CO₂, H₂O, and H₂, both with and without considering the influence of pore diffusion, which lead to similar results as the influence of pore diffusion is small. The agreement is quite well, and the simultaneous methanation of CO and CO₂ are satisfactory described by the kinetic equations.

In case of a household application, the exothermic methanation could be conducted in a simple adiabatic fixed bed reactor without the need of a cooling system. For the simulation of the reactor, not only the mass balance, but also the temperature change in axial direction has to be considered by a respective heat balance (i = CO, CO₂; n = all components of the gas):

$$\frac{\mathrm{d}T}{\mathrm{d}z}=\frac{\sum r_{i,\mathit{eff}}{(-\Delta }_R{H}_i{)\rho }_b}{\sum c_n{c}_{p,n}{u}_s}$$

(15)

The dimensioning of fuel-cell appliances is usually orientated to the average load demand of a household. In Germany, a four-person household consumes, on average, 5000 kWh electrical energy per year [34]. This is equivalent to 0.6 kW average power. Unfortunately, the load profiles of demand and production of electricity by the fuel-cell system are different. So, in times when the fuel-cell does not cover the demand, the additional need is covered by the power grid. If the fuel-cell produces more electricity than needed, the excess power is fed into the grid. The same happens with the heat demand. In times where heat production of the fuel-cell is not sufficient a peak boiler will ensure the domestic comfort.

The Viessmann company (Germany) sales fuel cell appliances for one- or two-family households for production of electrical power and heat. For example, the model “Vitovalor PT2” has a fuel cell with an electric power of 0.75 kW_el and a heat power of 1.1 kW_th [35]. The corresponding H₂ flow rate for a typical overall efficiency of 80% can then be estimated based on the lower heating value of H₂ (10.78 MJ·m⁻³). The resulting flow rate is 772 L H₂ h⁻¹, which corresponds to a total flow rate of 971 l h⁻¹ for a reformate consisting of 0.5% CO, 10% CO₂, 10% H₂O, and 79.5% H₂. In Figure 10 and

Table 4. Basic data of an adiabatic reactor for selective CO-methanation for three different initial temperatures.

Tin in °C	Tout in °C	mcat in kg	XCO in %	XCO2 in %	XH2 in %	EH2	CO Content at Reactor Outlet
120	210	4.25	98.00	0.61	2.16	1.17	Limit value of 100 ppm just reached
	225	6.40	99.99	1.68	2.73	1.48	<1 ppm
	238	8.50	99.99	2.60	3.19	1.73	<1 ppm
130	221	2.60	98.00	0.74	2.22	1.20	Limit value of 100 ppm just reached
	234	3.90	99.95	1.59	2.69	1.45	3 ppm
	245	5.20	99.99	2.37	3.08	1.67	<1 ppm
140	233	1.70	98.00	0.90	2.30	1.24	Limit value of 100 ppm just reached
	244	2.55	99.86	1.60	2.69	1.45	7 ppm
	253	3.40	99.99	2.25	3.02	1.63	<1 ppm

, the results of the simulations are given for different gas inlet temperatures. The RWGS reaction, which may play a role at temperatures above 240 °C, was not taken into consideration.

Considering the target value of 10 ppm CO (X_CO = 99.8%) in the product gas of the methanation reactor for an inlet temperature of 120 °C, 5.1 kg catalyst are needed, 1.05% CO₂ are simultaneously converted and the gas outlet temperature is 217 °C.

Taking into consideration that the target value of 100 ppm CO in the product gas of the methanation reactor has to be achieved, this corresponds to 98% CO conversion and 1.85% H₂ conversion. The further rise in temperature and H₂ consumption is caused by the unwanted CO₂ methanation. The excess of H₂ conversion is here characterized by the factor E_H2 = X_H2/0.0185, which is the ratio of the actual H₂ conversion to the minimum conversion of 1.85%, if only CO methanation takes place and the residual CO content of 100 ppm needed for PtRu-anodes is just reached. Table 4 indicates that an inlet temperature of 130 °C is an appropriate value to avoid very high outlet temperatures (>240 °C). In this case, 2.6 kg of catalyst (52 g Ru) would be needed. The outlet temperature is then 221 °C, which is a reasonable value to limit the RWGS and CO₂ methanation. E_H2 would then be 1.2, i.e., 20% more hydrogen (H₂ conversion of 2.22% compared to 1.85%) is consumed compared to the ideal case without CO₂ conversion instead of here 0.7%. In order to be on the safe side, a certain surplus of the reactor size is advisable. Therefore, Table 4 also shows the reactor parameters, if the reactor length and mass of catalyst would be by a factor 1.5 or 2 larger compared to 100 ppm residual CO content. For a factor of 1.5 (m_cat = 3.9 kg) and T_in = 130 °C, the resulting values are X_CO = 99.95% (residual CO content of 3 ppm), X_CO2 = 1.6%, and E_H2 = 1.45. The feed gas of the fuel cell would then consist of 77.2% H₂, 9.8% CO₂, 10.8% H₂O, and 2.1% CH₄. The reactor size is then 7.8 L, e.g., a cylinder with a length of 44 cm and diameter of 15 cm. An option is also an increase of the Ru content (here 2 wt-%), which would decrease the size and mass of the reactor further.

4. Conclusions

The activity and selectivity of a 2 wt% Ru supported on γ-Al₂O₃ egg-shell catalyst for CO methanation in CO₂/H₂ rich gases was investigated. A kinetic model based on a Langmuir-Hinshelwood approach for both reactions was determined. The agreement with measurements in a fixed bed reactor is very satisfactory. CO₂ methanation is slow compared to CO methanation, at least at temperatures below 200 °C. CO₂ is directly converted to methane; the indirect route via RWGS and CO methanation could be excluded by respective measurements and kinetic considerations. Pore diffusion may affect the CO conversion at high temperatures (>200 °C). The kinetic equations were applied to model an adiabatic fixed bed methanation reactor suitable for a fuel cell for household appliances. The catalyst mass needed to reach a residual CO content of 100 ppm for feed gas with 0.5% CO, 10% CO₂, 10% H₂O, and 79.5% H₂ is about 3 kg, which seems to a reasonable value. The H₂ consumption is 20% higher compared to the ideal case without any conversion of CO₂.