Effects of Geometric Parameters on the Physical Mechanisms of Supersonic Fluidic Oscillators
Abstract
:1. Introduction
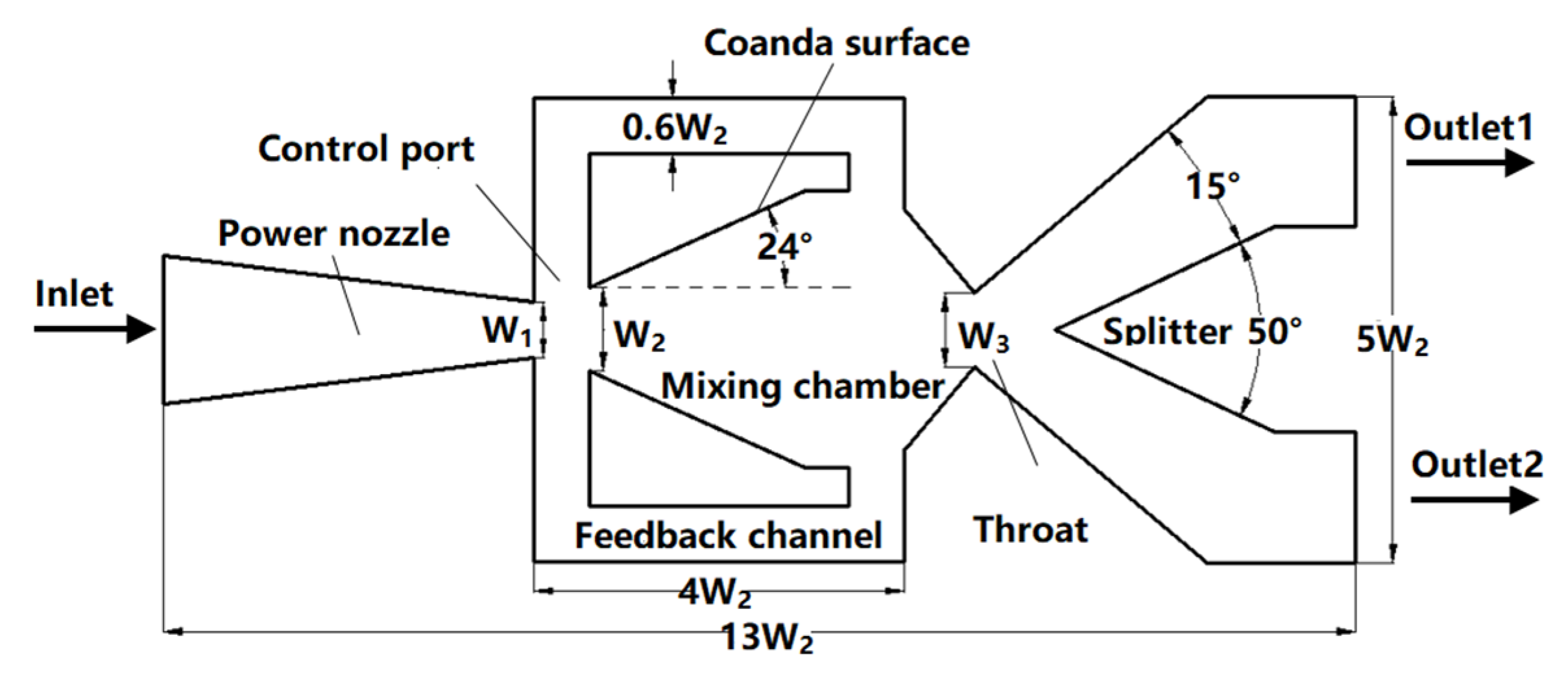
2. Oscillator Structure and Numerical Method
3. Results and Analysis
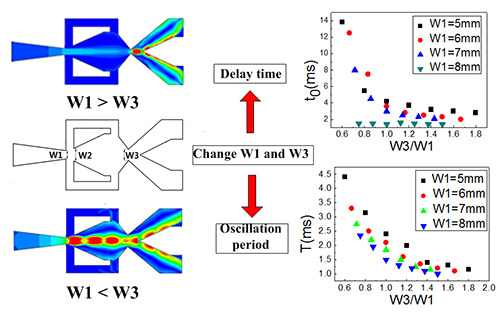
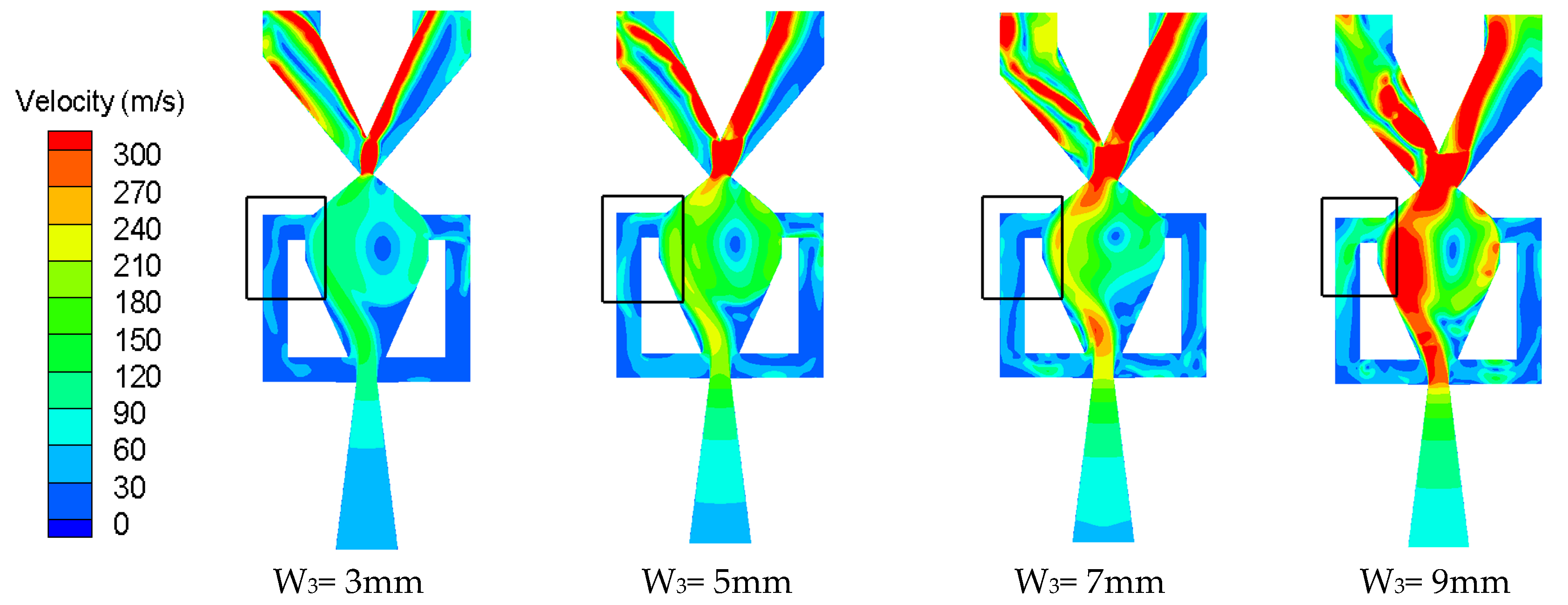
3.1. Effect of Power Nozzle Exit Width and Throat Width on the Delay Time
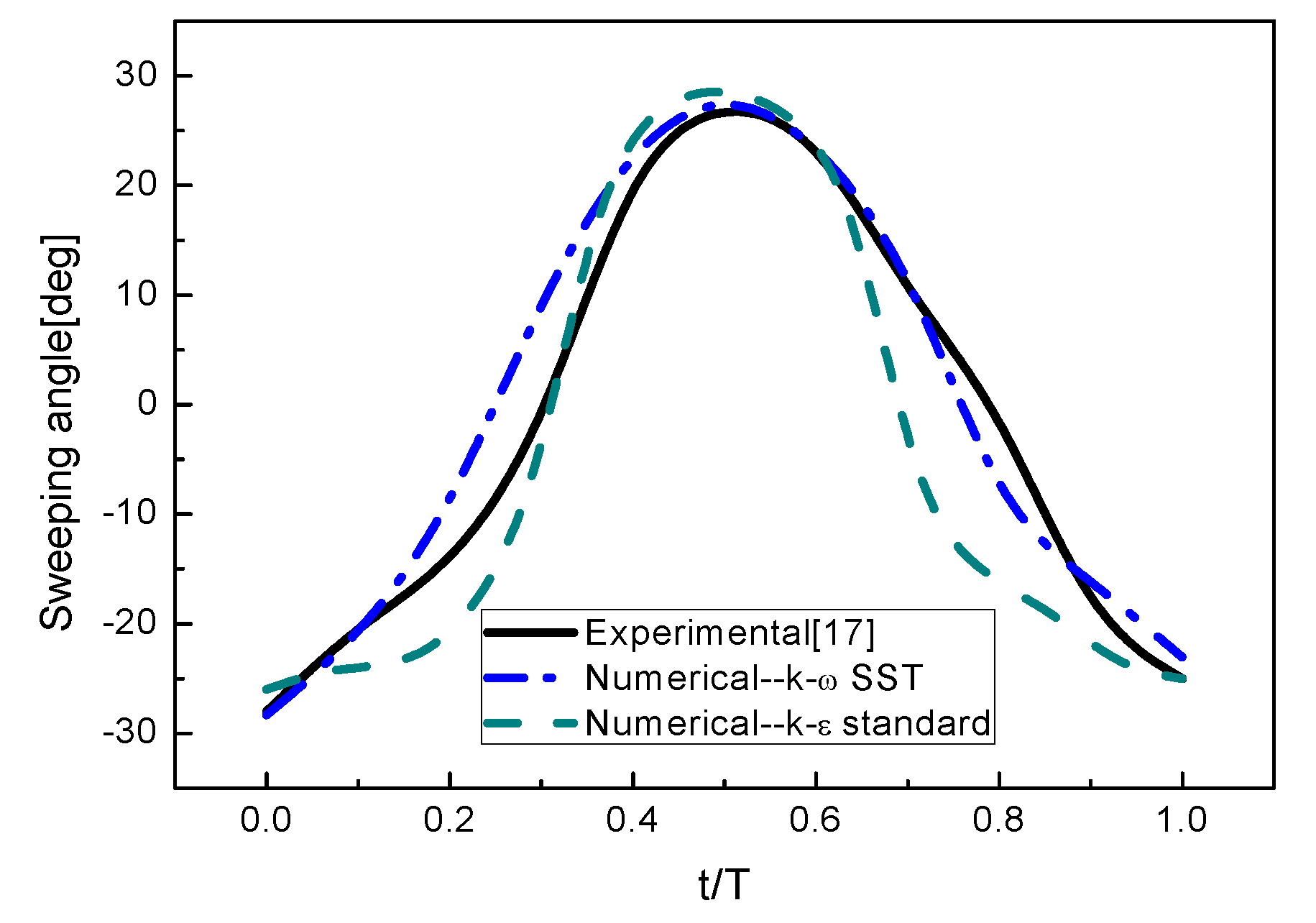
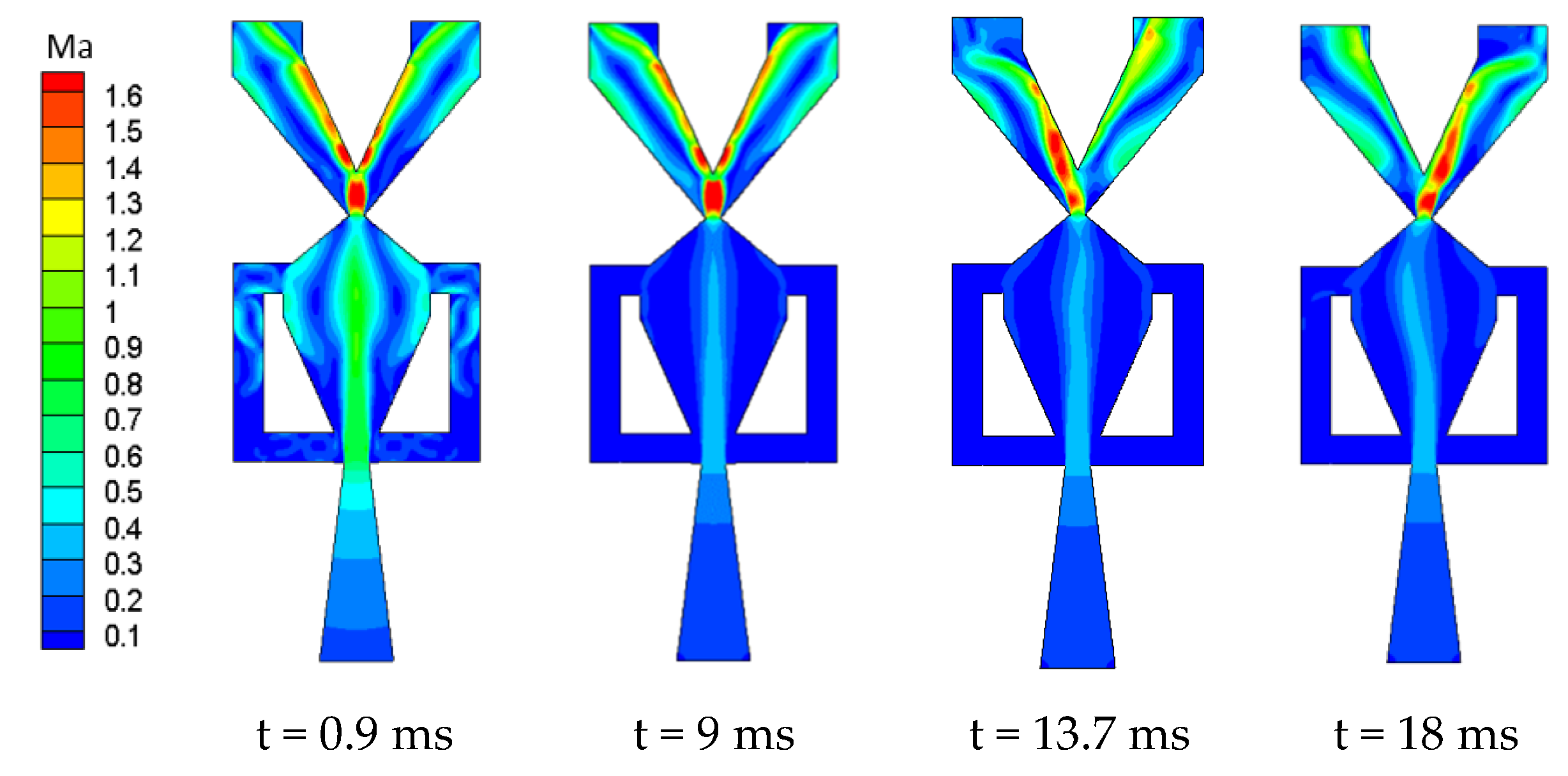
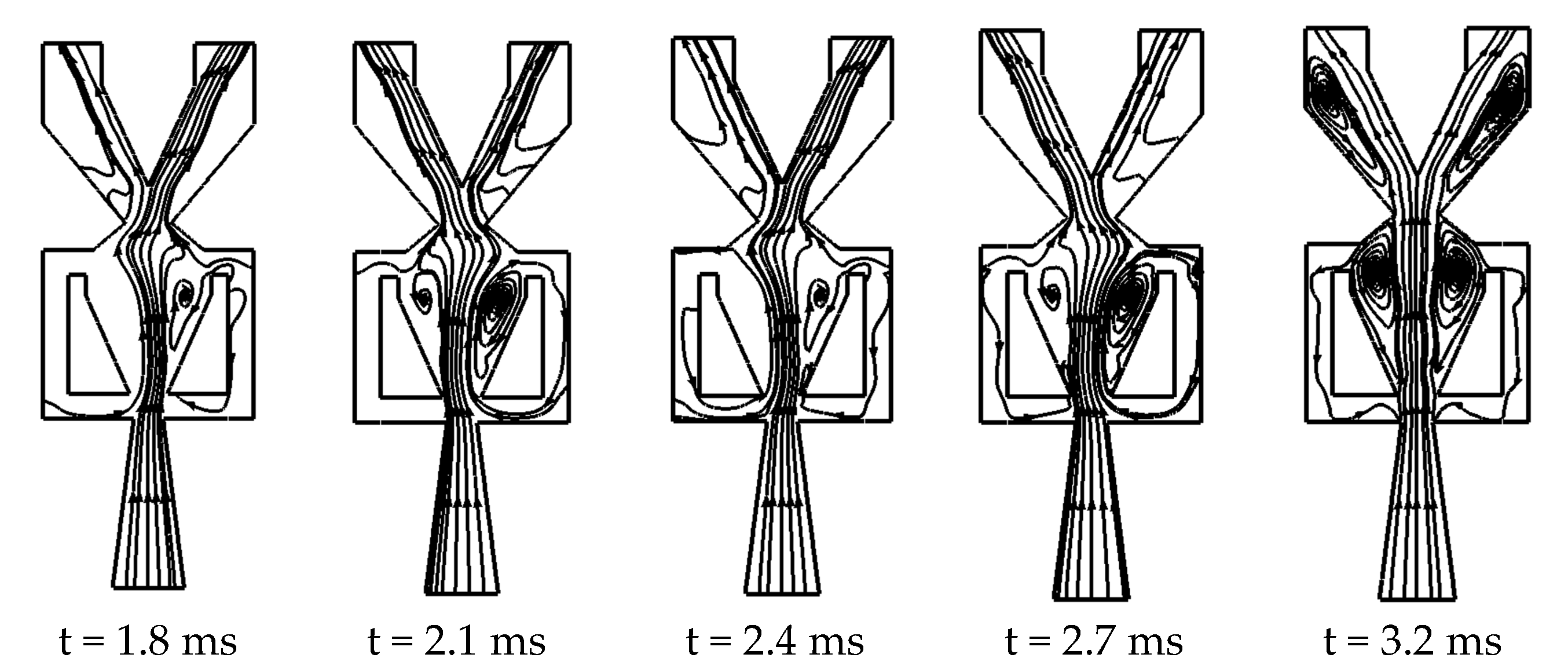
3.2. Activation Mechanism of Oscillator
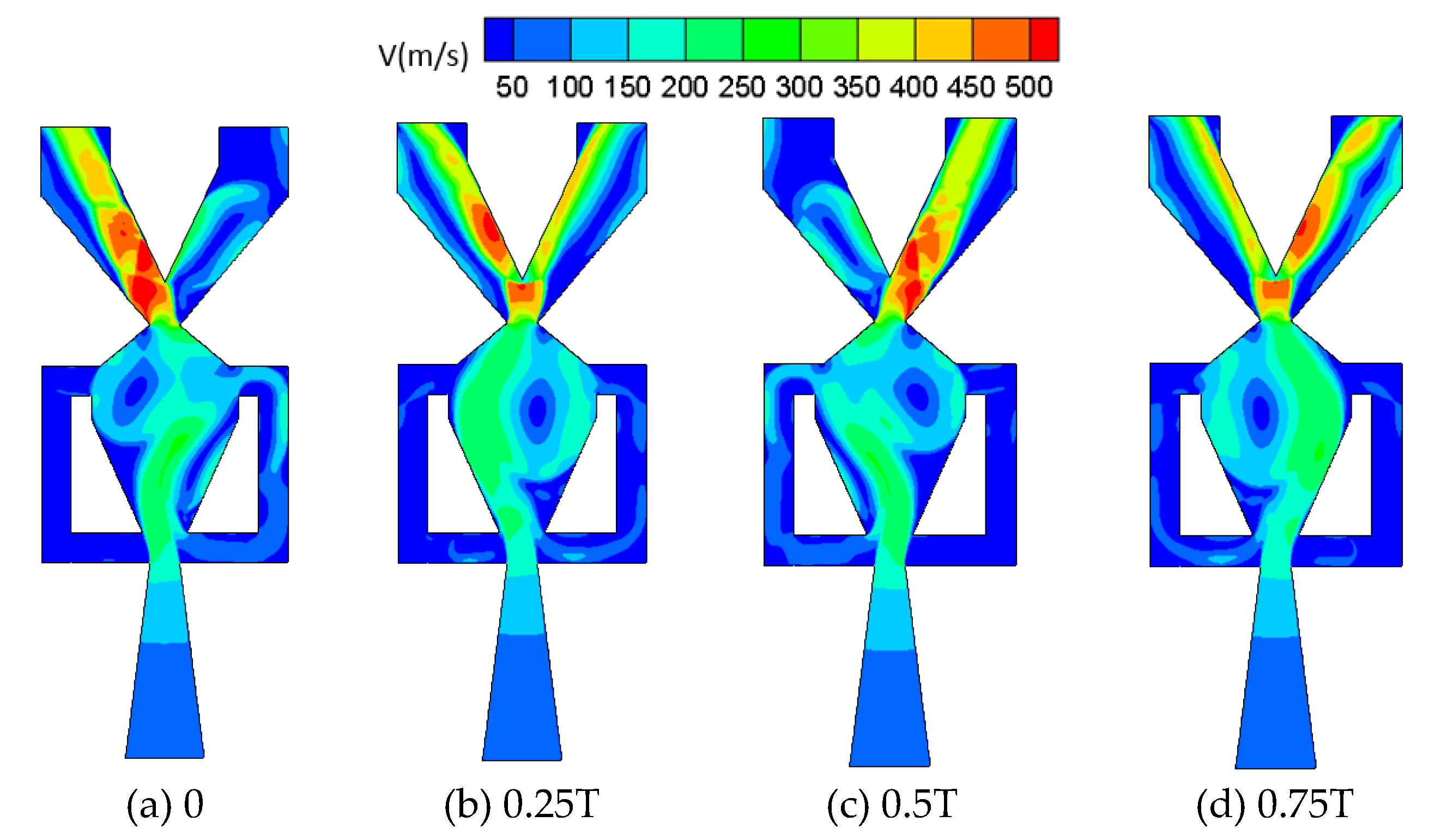
3.3. Effect of Power Nozzle Exit Width and Throat Width on the Oscillation Period
4. Conclusions
- (1)
- In the range of parameters studied in this paper, when the power nozzle exit width (W1) is much smaller than the inlet width of the mixing chamber (W2), the delay time t0 of the oscillator decreases as W3 increases. When W1 is close to W2, the delay time t0 of the oscillator does not change significantly with variations in the throat width.
- (2)
- When W1 and W2 are held constant, the oscillators studied in this paper have a critical throat width (Wcr). When the width of the throat exceeds this critical value, not enough fluid flows back to the control port through the feedback channel. The jet cannot be deflected to attach to the side wall and no oscillating jet can form.
- (3)
- When W1 is held constant, the oscillation period gradually decreases as W3 increases, but the rate of decrease becomes progressively smaller. When W3 is held constant, the oscillation period decreases with any reduction in W1.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| T | = Time of a stable oscillation period (s) |
| Ma | = Mach number of fluid in the jet oscillator |
| W1 | = Power nozzle exit width (mm) |
| W2 | = Inlet width of the mixing chamber (mm) |
| W3 | = Outlet width of the mixing chamber (mm) |
| Wcr | = Corresponding W3 value when the jet cannot oscillate(mm) |
| t | = Working time of the jet oscillator (s) |
| tc | = Time spent in the feedback channel (s) |
| t0 | = Delay time for the initiation of oscillation (s) |
| f | = Frequency of the fluidic oscillator (Hz) |
| ts | = Sweeping time of the oscillator in one period (s) |
References
- Raman, G.; Raghu, S. Cavity resonance suppression using miniature fluidic oscillators. AIAA J. 2012, 42, 2608–2612. [Google Scholar] [CrossRef]
- Guyot, D.; Paschereit, C.O.; Raghu, S. Active combustion control using a fluidic oscillator for asymmetric fuel flow modulation. Int. J. Flow Control 2009, 1, 155–166. [Google Scholar] [CrossRef]
- Woszidlo, R.; Wygnanski, I. Parameters governing separation control with sweeping jet actuators. In Proceedings of the 29th AIAA Applied Aerodynamics Conference, Honolulu, HI, USA, 27–30 June 2011. [Google Scholar]
- Seifert, A.; Stalnov, O.; Sperber, D.; Arwatz, G.; Palei, V.; David, S.; Dayan, I.; Fono, I. Large trucks drag reduction using active flow control. In Proceedings of the 46th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 7–10 January 2008. [Google Scholar]
- Annino, R.; Leone, J. The use of coanda wall attachment fluidic switches as gas chromatographic valves. J. Chromatogr. Sci. 1982, 20, 19–26. [Google Scholar] [CrossRef]
- Gregory, J.; Sullivan, J.; Raghu, S. Jet interaction studies in a fluidic oscillator. In Proceedings of the APS Division of Fluid Dynamics Meeting, Chicago, IL, USA, 20–22 November 2005. [Google Scholar]
- Bosenberg, W.R.; Drobshoff, A.; Alexander, J.I.; Myers, L.E.; Byer, R.L. Continuous-wave singly-resonant intracavity optical parametric oscillator based on periodically-poled LiNbO3. Optics. Lett. 1998, 23, 837–839. [Google Scholar]
- Li, Y.; Someya, S.; Koso, T.; Aramaki, S.; Okamoto, K. Characterization of periodic flow structure in a small-scale feedback fluidic oscillator under low-Reynolds-number water flow. Flow Meas. Instrum. 2013, 33, 179–187. [Google Scholar]
- Von Gosen, F.; Ostermann, F.; Woszidlo, R.; Nayeri, C.; Paschereit, C.O. Experimental investigation of compressibility effects in a fluidic oscillator. In Proceedings of the 53rd AIAA Aerospace Sciences Meeting, Kissimmee, FL, USA, 5–9 January 2015.
- Wu, X.; Dong, J. Study on the reflux and switching characteristics of a jet-controlled oscillator. Sci. Technol. Eng. 2016, 16, 314–318. [Google Scholar]
- Bobusch, B.C.; Woszidlo, R.; Kruger, O.; Paschereit, C.O. Numerical investigations on geometric parameters affecting the oscillation properties of a fluidic oscillator. In Proceedings of the 21st AIAA Computational Fluid Dynamics Conference, San Diego, CA, USA, 24–27 June 2013. [Google Scholar]
- Gokoglu, S.; Kuczmarski, M.; Culley, D.; Raghu, S. Numerical studies of a supersonic fluidic diverter actuator for flow control. In Proceedings of the 5th Flow Control Conference, Chicago, IN, USA, 28 June–1 July 2010. [Google Scholar]
- Gokoglu, S.; Kuczmarski, M.; Culley, D.; Raghu, S. Numerical studies of a fluidic diverter for flow control. In Proceedings of the 39th AIAA Fluid Dynamics Conference, San Antonio, TX, USA, 22–25 June 2009. [Google Scholar]
- Raman, G.; Hailye, M.; Rice, E.J. Flip-flop jet nozzle extended to supersonic flows. AIAA J. 1993, 31, 1028–1035. [Google Scholar] [CrossRef]
- Bobusch, B.C.; Woszidlo, R.; Bergada, J.M.; Nayeri, C.N.N.; Paschereit, C.O. Experimental study of the internal flow structures inside a fluidic oscillator. Exp. Fluids 2013, 54. [Google Scholar] [CrossRef]
- Gaertlein, S.; Woszidlo, R.; Ostermann, F.; Nayeri, C.N.; Paschereit, C.O. The time-resolved internal and external flow field properties of a fluidic oscillator. In Proceedings of the 52nd AIAA Aerospace Sciences Meeting, National Harbor, MD, USA, 13–17 January 2014. [Google Scholar]
- Park, S.; Ko, H.; Kang, M.; Lee, Y. An experimental study of the characteristics of a supersonic fluidic oscillator utilizing the design of experiment. In Proceedings of the AIAA Aviation 2019 Forum, Dallas, TX, USA, 17–21 June 2019. [Google Scholar]
- Krüger, O.; Bobusch, B.C.; Woszidlo, R.; Paschereit, C.O. Numerical modeling and validation of the flow in a fluidic oscillator. AIAA J. 2013, 2013–3087. [Google Scholar] [CrossRef]


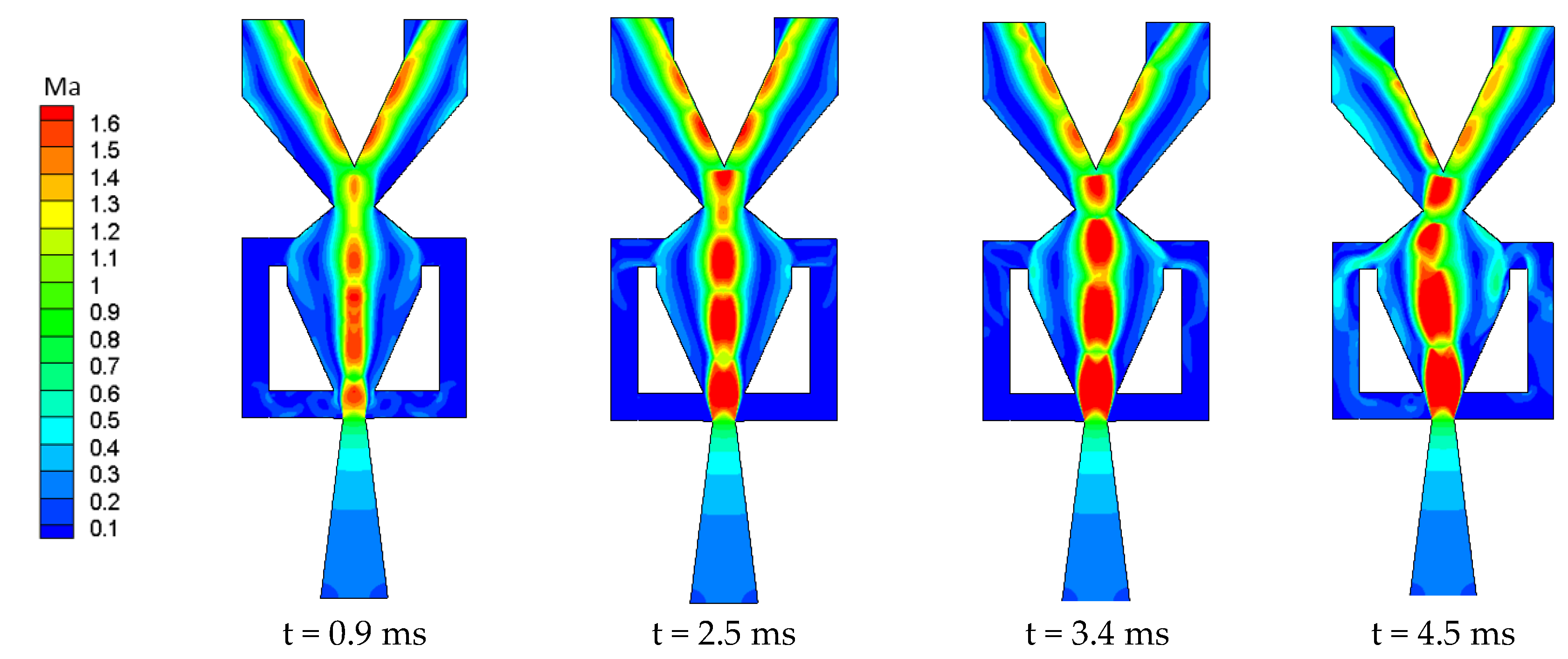
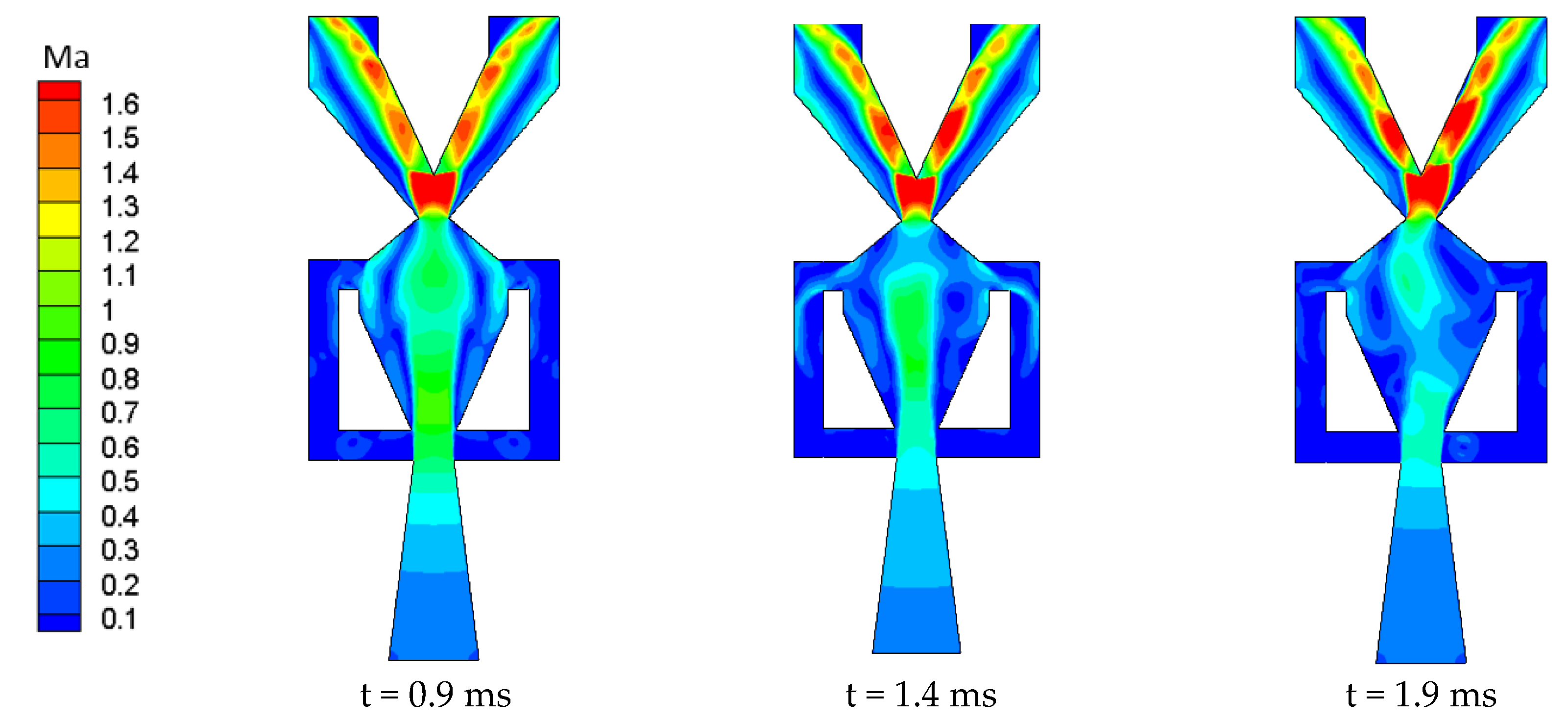
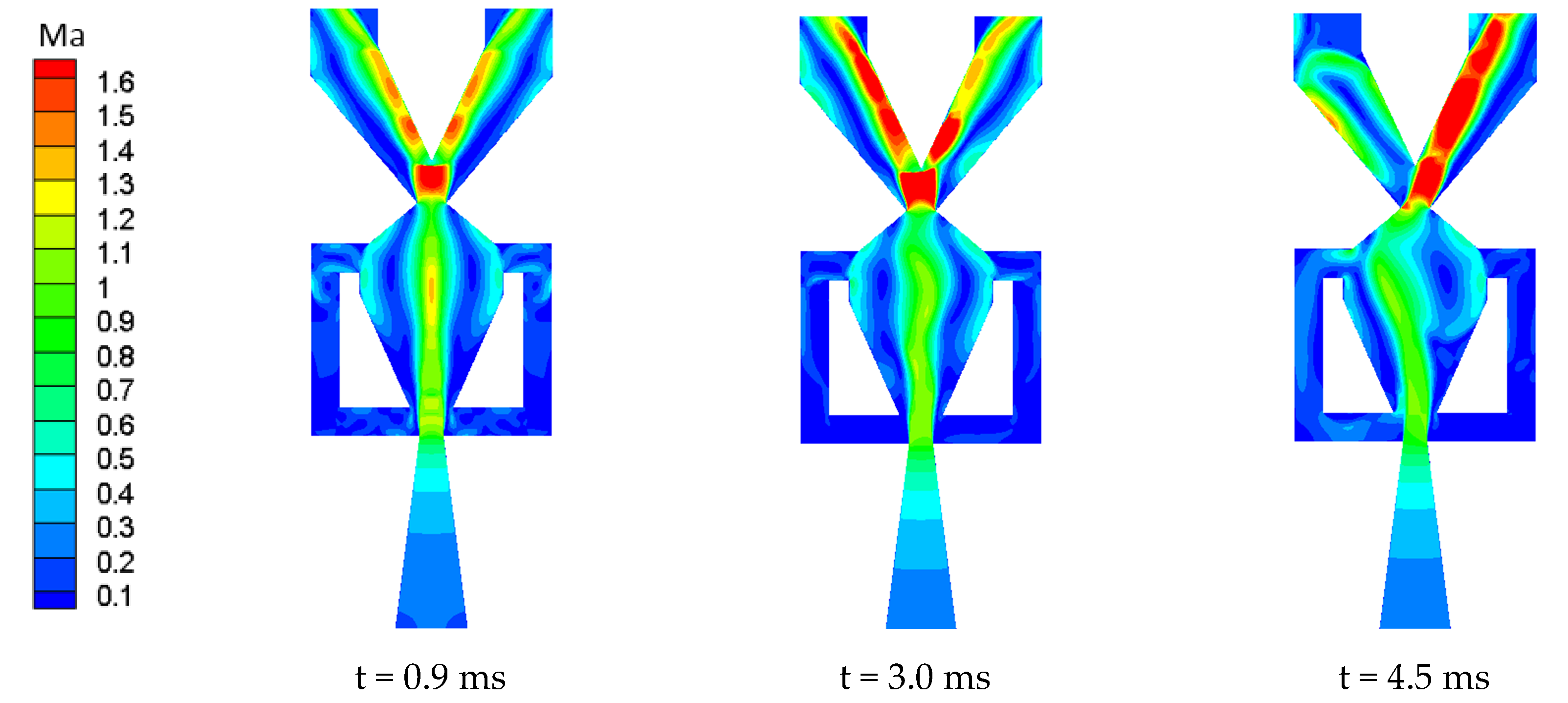

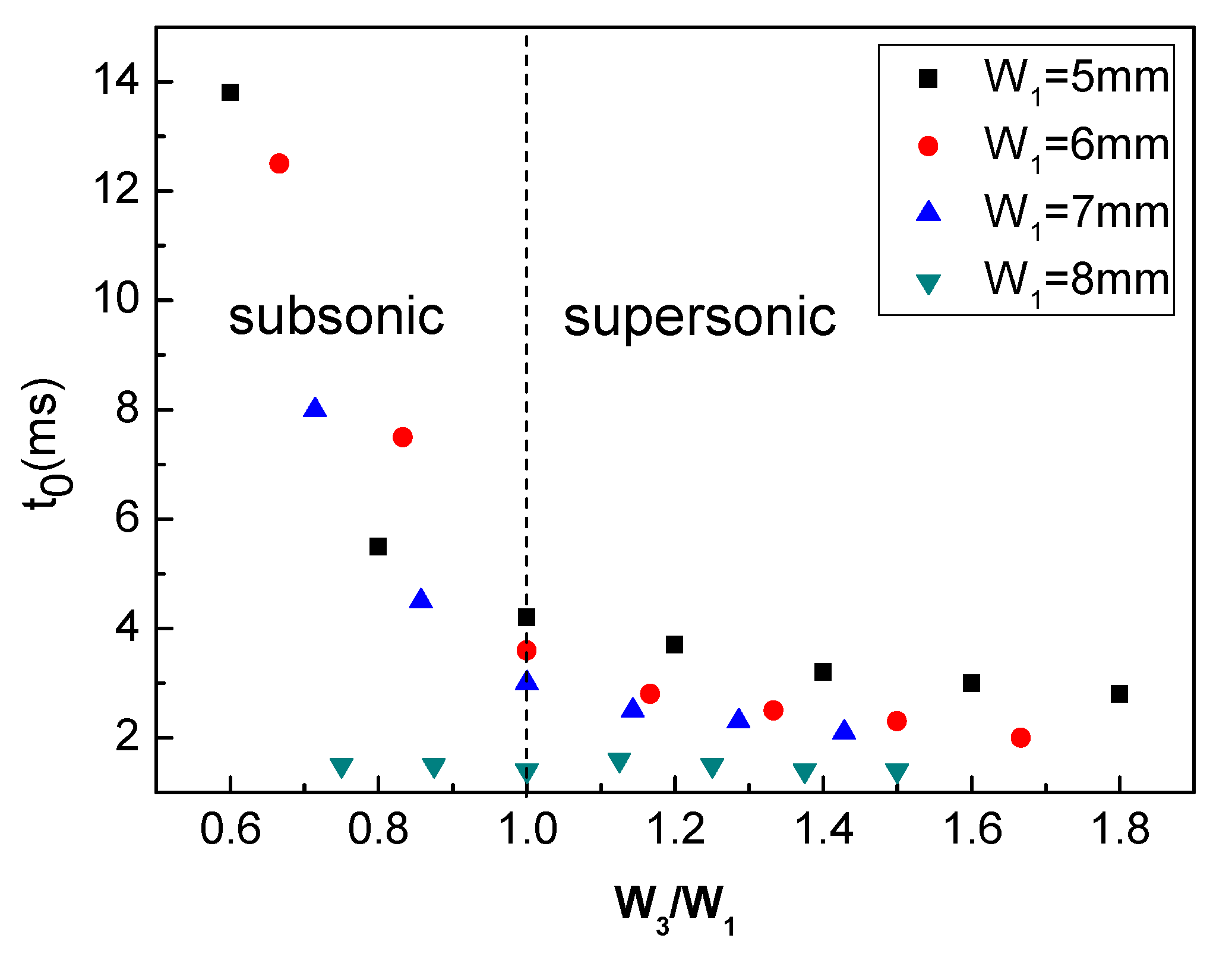



| W1 (mm) | 5 | 6 | 7 | 8 |
| Wcr (mm) | 10 | 10.5 | 11 | 13 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sang, Y.; Shan, Y.; Lei, H.; Tan, X.; Zhang, J. Effects of Geometric Parameters on the Physical Mechanisms of Supersonic Fluidic Oscillators. Energies 2020, 13, 3919. https://doi.org/10.3390/en13153919
Sang Y, Shan Y, Lei H, Tan X, Zhang J. Effects of Geometric Parameters on the Physical Mechanisms of Supersonic Fluidic Oscillators. Energies. 2020; 13(15):3919. https://doi.org/10.3390/en13153919
Chicago/Turabian StyleSang, Yongjun, Yong Shan, Han Lei, Xiaoming Tan, and Jingzhou Zhang. 2020. "Effects of Geometric Parameters on the Physical Mechanisms of Supersonic Fluidic Oscillators" Energies 13, no. 15: 3919. https://doi.org/10.3390/en13153919
APA StyleSang, Y., Shan, Y., Lei, H., Tan, X., & Zhang, J. (2020). Effects of Geometric Parameters on the Physical Mechanisms of Supersonic Fluidic Oscillators. Energies, 13(15), 3919. https://doi.org/10.3390/en13153919